See "Concordance + Center" in this journal, a search
suggested by the new URL "geometry.center."
Friday, November 17, 2023
The Center
Comments Off on The Center
Tuesday, July 2, 2019
Depth Psychology Meets Inscape Geometry
An illustration from the previous post may be interpreted
as an attempt to unbokeh an inscape —
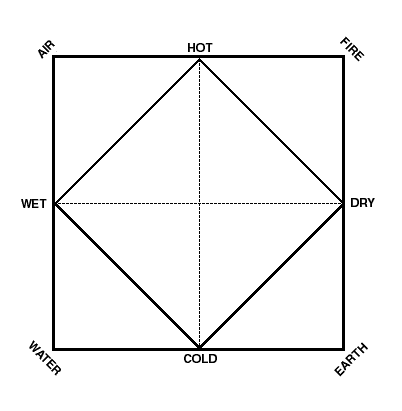
The 15 lines above are Euclidean lines based on pairs within a six-set.
For examples of Galois lines so based, see Six-Set Geometry:
Comments Off on Depth Psychology Meets Inscape Geometry
Thursday, October 26, 2017
A Center
This post was suggested by a New York Times obituary this evening —
"Tom Mathews, Promoter of Liberal Causes and Candidates, Dies at 96."
Mathews reportedly died on October 14, 2017.
"Mr. Mathews and his business partner Roger Craver 'dreamed for years
of finding the perfect citizen-candidate,' the authors wrote, 'a man or
woman of the center-left with a feel for issues, a history of independence,
a winning television manner and, most important of all, a center — a core
of beliefs more important to him or her than getting elected.'
Dream on.
From the date of Mathews's death:
Posts now tagged A Center for Krauss —
Comments Off on A Center
Saturday, November 14, 2015
Sunday, May 4, 2014
Geometry for Scarlett
Scarlett Johansson stars in a new film, "Lucy," due to be
released on August 8, directed by Luc Besson, auteur of
The Fifth Element (1997). In other pop culture…
"There have long been rumors of a mythical Ninth Element
that grants ultimate power to the Wizard who masters it.
The Order of Magick says there is no such thing. But…."
— Website of Magicka: The Ninth Element Novel
See also, in this journal, Holy Field as well as Power of the Center.
Comments Off on Geometry for Scarlett
Saturday, August 17, 2013
Up-to-Date Geometry
The following excerpt from a January 20, 2013, preprint shows that
a Galois-geometry version of the large Desargues 154203 configuration,
although based on the nineteenth-century work of Galois* and of Fano,**
may at times have twenty-first-century applications.
Atkinson's paper does not use the square model of PG(3,2), which later
in 2013 provided a natural view of the large Desargues 154203 configuration.
See my own Classical Geometry in Light of Galois Geometry. Atkinson's
"subset of 20 lines" corresponds to 20 of the 80 Rosenhain tetrads
mentioned in that later article and pictured within 4×4 squares in Hudson's
1905 classic Kummer's Quartic Surface.
* E. Galois, definition of finite fields in "Sur la Théorie des Nombres,"
Bulletin des Sciences Mathématiques de M. Férussac,
Vol. 13, 1830, pp. 428-435.
** G. Fano, definition of PG(3,2) in "Sui Postulati Fondamentali…,"
Giornale di Matematiche, Vol. 30, 1892, pp. 106-132.
Comments Off on Up-to-Date Geometry
Wednesday, August 1, 2012
Elementary Finite Geometry
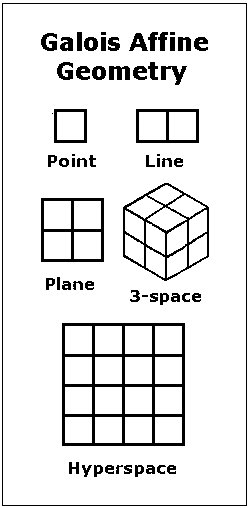
I. General finite geometry (without coordinates):
A finite affine plane of order n has n^2 points.
A finite projective plane of order n has n^2 + n + 1
points because it is formed from an order-n finite affine
plane by adding a line at infinity that contains n + 1 points.
Examples—
|
|
|
II. Galois finite geometry (with coordinates over a Galois field):
A finite projective Galois plane of order n has n^2 + n + 1
points because it is formed from a finite affine Galois 3-space
of order n with n^3 points by discarding the point (0,0,0) and
identifying the points whose coordinates are multiples of the
(n-1) nonzero scalars.
Note: The resulting Galois plane of order n has
(n^3-1)/(n-1)= (n^2 + n + 1) points because
(n^2 + n + 1)(n – 1) =
(n^3 + n^2 + n – n^2 – n – 1) = (n^3 – 1) .
III. Related art:
Another version of a 1994 picture that accompanied a New Yorker
article, "Atheists with Attitude," in the issue dated May 21, 2007:
The Four Gods of Borofsky correspond to the four axes of
symmetry of a square and to the four points on a line at infinity
in an order-3 projective plane as described in Part I above.
Those who prefer literature to mathematics may, if they like,
view the Borofsky work as depicting
"Blake's Four Zoas, which represent four aspects
of the Almighty God" —Wikipedia
Comments Off on Elementary Finite Geometry
Wednesday, June 16, 2010
Geometry of Language
(Continued from April 23, 2009, and February 13, 2010.)
Paul Valéry as quoted in yesterday’s post:
“The S[elf] is invariant, origin, locus or field, it’s a functional property of consciousness” (Cahiers, 15:170 [2: 315])
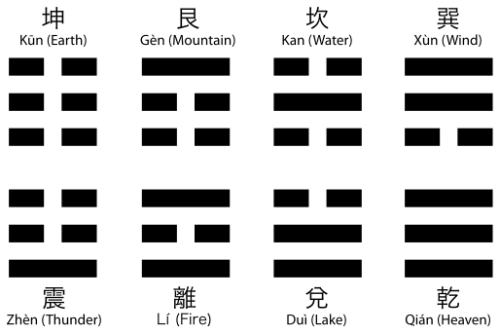
The geometric example discussed here yesterday as a Self symbol may seem too small to be really impressive. Here is a larger example from the Chinese, rather than European, tradition. It may be regarded as a way of representing the Galois field GF(64). (“Field” is a rather ambiguous term; here it does not, of course, mean what it did in the Valéry quotation.)
From Geometry of the I Ching—
The above 64 hexagrams may also be regarded as
the finite affine space AG(6,2)— a larger version
of the finite affine space AG(4,2) in yesterday’s post.
That smaller space has a group of 322,560 symmetries.
The larger hexagram space has a group of
1,290,157,424,640 affine symmetries.
From a paper on GL(6,2), the symmetry group
of the corresponding projective space PG(5,2),*
which has 1/64 as many symmetries—
For some narrative in the European tradition
related to this geometry, see Solomon’s Cube.
* Update of July 29, 2011: The “PG(5,2)” above is a correction from an earlier error.
Comments Off on Geometry of Language
Saturday, June 12, 2010
Holy Geometry
The late mathematician V.I. Arnold was born on this date in 1937.
"By groping toward the light we are made to realize
how deep the darkness is around us."
— Arthur Koestler, The Call Girls: A Tragi-Comedy
Light
Choosing light rather than darkness, we observe Arnold's birthday with a quotation from his 1997 Paris talk 'On Teaching Mathematics.'
"The Jacobi identity (which forces the heights of a triangle to cross at one point) is an experimental fact…."
The "experimental fact" part, perhaps offered with tongue in cheek, is of less interest than the assertion that the Jacobi identity forces the altitude-intersection theorem.
Albert Einstein on that theorem in the "holy geometry book" he read at the age of 12—
"Here were assertions, as for example the intersection of the three altitudes of a triangle in one point, which– though by no means evident– could nevertheless be proved with such certainty that any doubt appeared to be out of the question. This lucidity and certainty made an indescribable impression upon me.”
Arnold's much less evident assertion about altitudes and the Jacobi identity is discussed in "Arnol'd, Jacobi identity, and orthocenters" (pdf) by Nikolai V. Ivanov.
Ivanov says, without giving a source, that the altitudes theorem "was known to Euclid." Alexander Bogomolny, on the other hand, says it is "a matter of real wonderment that the fact of the concurrency of altitudes is not mentioned in either Euclid's Elements or subsequent writings of the Greek scholars. The timing of the first proof is still an open question."
For other remarks on geometry, search this journal for the year of Arnold's birth.
Comments Off on Holy Geometry
Tuesday, June 8, 2010
From Plato to Finite Geometry
Comments Off on From Plato to Finite Geometry
Wednesday, April 28, 2010
Eightfold Geometry
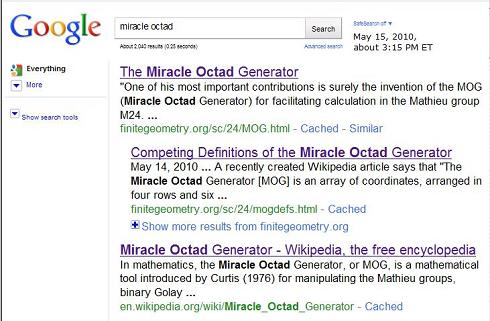
Related web pages:
Miracle Octad Generator,
Generating the Octad Generator,
Geometry of the 4×4 Square
Related folklore:
"It is commonly known that there is a bijection between the 35 unordered triples of a 7-set [i.e., the 35 partitions of an 8-set into two 4-sets] and the 35 lines of PG(3,2) such that lines intersect if and only if the corresponding triples have exactly one element in common." –"Generalized Polygons and Semipartial Geometries," by F. De Clerck, J. A. Thas, and H. Van Maldeghem, April 1996 minicourse, example 5 on page 6
The Miracle Octad Generator may be regarded as illustrating the folklore.
Update of August 20, 2010–
For facts rather than folklore about the above bijection, see The Moore Correspondence.
Comments Off on Eightfold Geometry
Thursday, April 22, 2010
Mere Geometry
Stanford Encyclopedia of Philosophy —
“Mereology (from the Greek μερος, ‘part’) is the theory of parthood relations: of the relations of part to whole and the relations of part to part within a whole. Its roots can be traced back to the early days of philosophy, beginning with the Presocratics….”
A non-Euclidean* approach to parts–
Corresponding non-Euclidean*
projective points —
Richard J. Trudeau in The Non-Euclidean Revolution, chapter on “Geometry and the Diamond Theory of Truth”–
“… Plato and Kant, and most of the philosophers and scientists in the 2200-year interval between them, did share the following general presumptions:
(1) Diamonds– informative, certain truths about the world– exist.
(2) The theorems of Euclidean geometry are diamonds.
Presumption (1) is what I referred to earlier as the ‘Diamond Theory’ of truth. It is far, far older than deductive geometry.”
Trudeau’s book was published in 1987. The non-Euclidean* figures above illustrate concepts from a 1976 monograph, also called “Diamond Theory.”
Although non-Euclidean,* the theorems of the 1976 “Diamond Theory” are also, in Trudeau’s terminology, diamonds.
* “Non-Euclidean” here means merely “other than Euclidean.” No violation of Euclid’s parallel postulate is implied.
Comments Off on Mere Geometry
Tuesday, December 22, 2009
Wednesday, November 27, 2024
Hoarding Space*
The domain bitcube.space has now been renewed for another year.
It leads to — among other things — the following remarks . . .
|
Towards a Philosophy of Real Mathematics, by David Corfield, Cambridge U. Press, 2003, p. 206: “Now, it is no easy business defining what one means by the term conceptual…. I think we can say that the conceptual is usually expressible in terms of broad principles. A nice example of this comes in the form of harmonic analysis, which is based on the idea, whose scope has been shown by George Mackey (1992) to be immense, that many kinds of entity become easier to handle by decomposing them into components belonging to spaces invariant under specified symmetries.” For a simpler example of this idea, see the entities in The Diamond Theorem, the decomposition in A Four-Color Theorem, and the space in Geometry of the 4×4 Square. The decomposition differs from that of harmonic analysis, although the subspaces involved in the diamond theorem are isomorphic to Walsh functions– well-known as discrete analogues of the trigonometric functions of traditional harmonic analysis. |
* See that phrase in this journal.
Saturday, September 28, 2024
Architectural Singularity
Embedded in the Sept. 26 New Yorker review of Coppola's
Megalopolis is a ghostly transparent pyramidal figure . . .
The pyramidal figure is not unrelated to Scandia.tech —
|
American Mathematical Monthly, Vol. 92, No. 6 LETTERS TO THE EDITOR Material for this department should be prepared exactly the same way as submitted manuscripts (see the inside front cover) and sent to Professor P. R. Halmos, Department of Mathematics, University of Santa Clara, Santa Clara, CA 95053 Editor: Miscellaneum 129 ("Triangles are square," June-July 1984 Monthly ) may have misled many readers. Here is some background on the item. That n2 points fall naturally into a triangular array is a not-quite-obvious fact which may have applications (e.g., to symmetries of Latin-square "k-nets") and seems worth stating more formally. To this end, call a convex polytope P an n-replica if P consists of n mutually congruent polytopes similar to P packed together. Thus, for n ∈ ℕ, (A) An equilateral triangle is an n-replica if and only if n is a square. Does this generalize to tetrahedra, or to other triangles? A regular tetrahedron is not a (23)-replica, but a tetrahedron ABCD with edges AB, BC, and CD equal and mutually orthogonal is an n-replica if and only if n is a cube. Every triangle satisfies the "if" in (A), so, letting T be the set of triangles, one might surmise that (B) ∀ t ∈ T (t is an n-replica if and only if n is a square). This, however, is false. A. J. Schwenk has pointed out that for any m ∈ ℕ, the 30°-60°-90° triangle is a (3m2)-replica, and that a right triangle with legs of integer lengths a and b is an ((a2 + b2)m2)-replica. As Schwenk notes, it does not seem obvious which other values of n can occur in counterexamples to (B). Shifting parentheses to fix (B), we get a "square-triangle" lemma:
(C) (∀ t ∈ T, t is an n-replica) if and only if n is a square.
Steven H. Cullinane
501 Follett Run Road Warren, PA 16365 |
Comments Off on Architectural Singularity
Thursday, September 12, 2024
Structures
The New York Times asks above,
"Are art and science forever divided?
Or are they one and the same?"
A poet's approach . . .
“The old man of ‘Sailing to Byzantium’ imagined the city’s power
as being able to ‘gather’ him into ‘the artifice of eternity’—
presumably into ‘monuments of unageing intellect,’ immortal and
changeless structures representative of or embodying all knowledge,
linked like a perfect machine at the center of time.”
— Karl Parker, Yeats’ Two Byzantiums
A mathematician's approach . . .
Compare and contrast the 12-dimensional extended binary Golay code
with the smaller 8-dimensional code below, which also has minimum
weight 8 . . .
From Sept. 20, 2022 —
Perhaps someone can prove there is no way that adding more generating
codewords can turn the cube-motif code into the Golay code, or perhaps
someone can supply such generating codewords.
Comments Off on Structures
Tuesday, July 2, 2024
Chinatown
|
Updated 8:18 PM EDT, Tue July 2, 2024 "Robert Towne, the Oscar-winning screenwriter of a number of acclaimed movies, including the classic 1974 noir thriller 'Chinatown' starring Jack Nicholson and Faye Dunaway, has died. He was 89 years old. The news was confirmed by Towne’s publicist Carri McClure, who said he died on Monday 'peacefully at home surrounded by his loving family.' No cause of death was provided. Towne won the Academy Award for best original screenplay for 'Chinatown,' which last month celebrated 50 years since being released." |
Related imagery . . .
Comments Off on Chinatown
Thursday, May 23, 2024
Logo Design: The Maltese Parrot
"The stuff that dreams are made of." — Bogart
But seriously . . .
|
From OSF . . . Among the positions that take this independence even further is Susanne Langer's approach towards meaning. Long before Derrida, she suggested in her chapter "The logic of signs and symbols" that we should understand meaning not as a relation to an author at all. Influenced by music and musical notation, she defines meaning instead as the function of a term from which a pattern emerges:
It is better, perhaps, to say: "Meaning is not a Reference: Langer, Susanne K., 1948 [1954]. Philosophy in a New Key: A Study in the Symbolism of Reason, Rite, and Art. Mentor Book. |
Comments Off on Logo Design: The Maltese Parrot
Sunday, February 4, 2024
Microsoft Copilot Doggerel
A poem written yesterday by Microsoft Copilot —
The prompt: "Write a poem … about the Cullinane diamond theorem."
|
In the mind of the mathematician,
Four-diamond figure, a 4×4 array,
Every G-image of the four-diamond figure,
The symmetry of the line diagrams,
And this symmetry must be shared by the entire pattern,
The 35 structures of the 840 = 35 x 24 G-images,
So here lies the beauty of the Cullinane diamond theorem, |
Actually, the complete prompt, suggested by a Log24 post of
12:12 PM ET yesterday, was . . .
"Write a poem in the style of Wallace Stevens
about the Cullinane diamond theorem."
Copilot still has a thing or two to learn about poetic style.
Comments Off on Microsoft Copilot Doggerel
Monday, January 29, 2024
Self as Imago Dei: Hofstadter vs. Valéry
|
Google search result: Saint Anselm College https://www.anselm.edu › Documents › Brown by M Brown · 2014 · Cited by 14 — Thomas insists that the image of God exists most perfectly in the acts of the soul, for the soul is that which is most perfect in us and so best images God, and … 11 pages |
For a Douglas Hofstadter version of the Imago Dei , see the
"Gödel, Escher, Bach" illustration in the Jan. 15 screenshot below —
Tuesday, June 15, 2010
|
Comments Off on Self as Imago Dei: Hofstadter vs. Valéry
Thursday, December 28, 2023
Basque Country Art Book
Book description at Amazon.com, translated by Google —
|
Las matemáticas como herramienta
Mathematics as a tool by Raúl Ibáñez Torres Kindle edition in Spanish, 2023 Although the relationship between mathematics and art can be traced back to ancient times, mainly in geometric and technical aspects, it is with the arrival of the avant-garde and abstract art at the beginning of the 20th century that mathematics takes on greater and different relevance: as a source of inspiration and as a tool for artistic creation. Let us think, for example, of the importance of the fourth dimension for avant-garde movements or, starting with Kandisnky and later Max Bill and concrete art, the vindication of mathematical thinking in artistic creation. An idea that would have a fundamental influence on currents such as constructivism, minimalism, the fluxus movement, conceptual art, systematic art or optical art, among others. Following this approach, this book analyzes, through a variety of examples and activities, how mathematics is present in contemporary art as a creative tool. And it does so through five branches and the study of some of its mathematical topics: geometry (the Pythagorean theorem), topology (the Moebius strip), algebra (algebraic groups and matrices), combinatorics (permutations and combinations) and recreational mathematics (magic and Latin squares). |
From the book ("Cullinane Diamond Theorem" heading and picture of
book's cover added) —
Publisher: Los Libros de La Catarata (October 24, 2023)
Author: Raúl Ibáñez Torres, customarily known as Raúl Ibáñez
(Ibáñez does not mention Cullinane as the author of the above theorem
in his book (except indirectly, quoting Josefine Lyche), but he did credit
him fully in an earlier article, "The Truchet Tiles and the Diamond Puzzle"
(translation by Google).)
About Ibáñez (translated from Amazon.com by Google):
Mathematician, professor of Geometry at the University of the Basque Country
and scientific disseminator. He is part of the Chair of Scientific Culture of the
UPV/EHU and its blog Cuaderno de Cultura Cientifica. He has been a scriptwriter
and presenter of the program “Una de Mates” on the television program Órbita Laika.
He has collaborated since 2005 on the programs Graffiti and La mechanica del caracol
on Radio Euskadi. He has also been a collaborator and co-writer of the documentary
Hilos de tiempo (2020) about the artist Esther Ferrer. For 20 years he directed the
DivulgaMAT portal, Virtual Center for the Dissemination of Mathematics, and was a
member of the dissemination commission of the Royal Spanish Mathematical Society.
Author of several books, including The Secrets of Multiplication (2019) and
The Great Family of Numbers (2021), in the collection Miradas Matemáticas (Catarata).
He has received the V José María Savirón Prize for Scientific Dissemination
(national modality, 2010) and the COSCE Prize for the Dissemination of Science (2011).
Comments Off on Basque Country Art Book
Tuesday, December 5, 2023
The Annenberg Knell
From a Log24 search for the above phrase . . .
|
"For many of us, the geometry course sounded the death knell — "Shape and Space in Geometry" © 1997-2003 Annenberg/CPB. All rights reserved. |
See also Annenberg Hall.
Comments Off on The Annenberg Knell
Sunday, November 26, 2023
Stella for Macbeth
"Play Stella by Starlight for Lady Macbeth" — Bob Dylan
|
For enthusiasts of arithmetic rather than geometry —
"4 + 12 = 16."
And for fans of Christoper Nolan — Window Panes :
Comments Off on Stella for Macbeth
Sunday, January 22, 2023
The Stillwell Dichotomies
| Number | Space |
| Arithmetic | Geometry |
| Discrete | Continuous |
Related literature —
From a "Finite Fields in 1956" post —
The Nutshell:
Related Narrative:
Comments Off on The Stillwell Dichotomies
Wednesday, December 28, 2022
The Santa Fe Institute as Magisterium Wannabe
"The novelist Cormac McCarthy has been a fixture around
the Santa Fe Institute since its embryonic stages in the
early 1980s. Cormac received a MacArthur Award in 1981
and met one of the members of the board of the MacArthur
Foundation, Murray Gell-Mann, who had won the Nobel Prize
in physics in 1969. Cormac and Murray discovered that they
shared a keen interest in just about everything under the sun
and became fast friends. When Murray helped to found the
Santa Fe Institute in 1984, he brought Cormac along, knowing
that everyone would benefit from this cross-disciplinary
collaboration." — https://www.santafe.edu/news-center/news/
cormac-and-sfi-abiding-friendship
Joy Williams, review of two recent Cormac McCarthy novels —
"McCarthy has pocketed his own liturgical, ecstatic style
as one would a coin, a ring, a key, in the service of a more
demanding and heartless inquiry through mathematics and
physics into the immateriality, the indeterminacy, of reality."
A Demanding and Heartless Coin, Ring, and Key:
COIN
RING
"We can define sums and products so that the G-images of D generate
an ideal (1024 patterns characterized by all horizontal or vertical "cuts"
being uninterrupted) of a ring of 4096 symmetric patterns. There is an
infinite family of such 'diamond' rings, isomorphic to rings of matrices
over GF(4)."
KEY
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in
Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference
(July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis,
James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97.
For those who prefer a "liturgical, ecstatic style" —
Comments Off on The Santa Fe Institute as Magisterium Wannabe
Wednesday, December 14, 2022
Plan 9 From Moriarty
Some related mathematical windmills —
|
For the eight-limbed star at the top of the quaternion array She drew from her handbag a pale grey gleaming implement that looked by quick turns to me like a knife, a gun, a slim sceptre, and a delicate branding iron—especially when its tip sprouted an eight-limbed star of silver wire. “The test?” I faltered, staring at the thing. “Yes, to determine whether you can live in the fourth dimension or only die in it.” — Fritz Leiber, short story, 1959 |
See as well . . .
Comments Off on Plan 9 From Moriarty
Sunday, December 11, 2022
Pawner versus Pawnee
A music producer pawns his current drum device
and acquires a demonic 1970s machine.
Related material —
This post was suggested by a remark made during the filming
of "Edge of Tomorrow," by a Log24 post on the new Nolan film
about Oppenheimer, and by the work of a different Edge:
"… a reality that only my notes can provide."
— Kinbote in Nabokov's novel Pale Fire
Comments Off on Pawner versus Pawnee
Friday, May 6, 2022
Interality and the Bead Game
WIkipedia on the URL suffix ".io" —
"In computer science, "IO" or "I/O" is commonly used
as an abbreviation for input/output, which makes the
.io domain desirable for services that want to be
associated with technology. .io domains are often used
for open source projects, application programming
interfaces ("APIs"), startup companies, browser games,
and other online services."
An association with the Bead Game from a post of April 7, 2018 —
|
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate A less poetic meditation on the above 4x4x4 design cube —
"I saw that in the alternation between front and back, See also a related remark by Lévi-Strauss in 1955:
"…three different readings become possible: |
The recent use by a startup company of the URL "interality.io" suggests
a fourth reading for the 1955 list of Lévi-Strauss — in and out —
i.e., inner and outer group automorphisms — from a 2011 post
on the birthday of T. S. Eliot :
A transformation:
Click on the picture for details.
Comments Off on Interality and the Bead Game
Sunday, April 24, 2022
Structuralism: Three Betweens
|
Tuesday, November 3, 2009 Filed under: General,Geometry — Tags: Glasperlenspiel, Solomon Marcus — m759 @ 10:10 PM Book review by Jadran Mimica in Oceania, Vol. 74, 2003: "In his classic essay of 1955 'The Structural Study of Myth' Levi-Strauss came up with a universal formula of mythopoeic dynamics [fx(a) : fy(b) :: fx(b) : fa-1(y)] that he called canonical 'for it can represent any mythic transformation'. This formulation received its consummation in the four massive Mythologiques volumes, the last of which crystallises the fundamental dialectics of mythopoeic thought: that there is 'one myth only' and the primal ground of this 'one' is 'nothing'. The elucidation of the generative matrix of the myth-work is thus completed as is the self-totalisation of both the thinker and his object." So there. At least one mathematician has claimed that the Levi-Strauss formula makes sense. (Jack Morava, arXiv pdf, 2003.) I prefer the earlier (1943) remarks of Hermann Hesse on transformations of myth: "…in the spirit of the Glass Bead Game, everything actually was all-meaningful, that every symbol and combination of symbols led not hither and yon, not to single examples, experiments, and proofs, but into the center, the mystery and innermost heart of the world, into primal knowledge. Every transition from major to minor in a sonata, every transformation of a myth or a religious cult, every classical or artistic formulation was, I realized in that flashing moment, if seen with a truly meditative mind, nothing but a direct route into the interior of the cosmic mystery, where in the alternation between inhaling and exhaling, between heaven and earth, between Yin and Yang, holiness is forever being created." |
Comments Off on Structuralism: Three Betweens
Tuesday, September 7, 2021
Raiders of the Lost Symbol … Continues*
A Log24 search for "Watercourse" leads to . . .
("Watercourse" is in the Customer review link.)
The "five years ago" link leads to . . .
|
"What modern painters are trying to do,
— James J. Gibson in Leonardo An example of invariant structure:
The three line diagrams above result from the three partitions, into pairs of 2-element sets, of the 4-element set from which the entries of the bottom colored figure are drawn. Taken as a set, these three line diagrams describe the structure of the bottom colored figure. After coordinatizing the figure in a suitable manner, we find that this set of three line diagrams is invariant under the group of 16 binary translations acting on the colored figure. A more remarkable invariance — that of symmetry itself — is observed if we arbitrarily and repeatedly permute rows and/or columns and/or 2×2 quadrants of the colored figure above. Each resulting figure has some ordinary or color-interchange symmetry. This sort of mathematics illustrates the invisible "form" or "idea" behind the visible two-color pattern. Hence it exemplifies, in a way, the conflict described by Plato between those who say that "real existence belongs only to that which can be handled" and those who say that "true reality consists in certain intelligible and bodiless forms." |
* See that title in this journal.
Comments Off on Raiders of the Lost Symbol … Continues*
Monday, July 5, 2021
Do Hillbillies Dream of Dinner Parties?
The title was suggested by a New Yorker photo caption
about Yale on June 19, 2021 —
"Amy Chua, a celebrity professor at the top-ranked
law school in the country, is at the center of a
campus-wide fracas known as 'Dinner Party-gate.' "
Other recent Yale material —
Remarks related to New Haven and geometry —
.jpg)
Comments Off on Do Hillbillies Dream of Dinner Parties?
Thursday, May 27, 2021
Saturday, March 6, 2021
An Echo from Ahab
From The New York Times today —
From Log24 yesterday, Echo Arc —
Related quote from Polonius — “To thine own self be true ….”
Comments Off on An Echo from Ahab
Monday, July 13, 2020
The Lexicographic Octad Generator (LOG)*
The lexicographic Golay code
contains, embedded within it,
the Miracle Octad Generator.
By Steven H. Cullinane, July 13, 2020
Background —
The Miracle Octad Generator (MOG)
of R. T. Curtis (Conway-Sloane version) —
A basis for the Golay code, excerpted from a version of
the code generated in lexicographic order, in
"Constructing the Extended Binary Golay Code"
Ben Adlam
Harvard University
August 9, 2011:
000000000000000011111111
000000000000111100001111
000000000011001100110011
000000000101010101010101
000000001001011001101001
000000110000001101010110
000001010000010101100011
000010010000011000111010
000100010001000101111000
001000010001001000011101
010000010001010001001110
100000010001011100100100
Below, each vector above has been reordered within
a 4×6 array, by Steven H. Cullinane, to form twelve
independent Miracle Octad Generator vectors
(as in the Conway-Sloane SPLAG version above, in
which Curtis's earlier heavy bricks are reflected in
their vertical axes) —
01 02 03 04 05 . . . 20 21 22 23 24 --> 01 05 09 13 17 21 02 06 10 14 18 22 03 07 11 15 19 23 04 08 12 16 20 24 0000 0000 0000 0000 1111 1111 --> 0000 11 0000 11 0000 11 0000 11 as in the MOG. 0000 0000 0000 1111 0000 1111 --> 0001 01 0001 01 0001 01 0001 01 as in the MOG. 0000 0000 0011 0011 0011 0011 --> 0000 00 0000 00 0011 11 0011 11 as in the MOG. 0000 0000 0101 0101 0101 0101 --> 0000 00 0011 11 0000 00 0011 11 as in the MOG. 0000 0000 1001 0110 0110 1001 --> 0010 01 0001 10 0001 10 0010 01 as in the MOG. 0000 0011 0000 0011 0101 0110 --> 0000 00 0000 11 0101 01 0101 10 as in the MOG. 0000 0101 0000 0101 0110 0011 --> 0000 00 0101 10 0000 11 0101 01 as in the MOG. 0000 1001 0000 0110 0011 1010 --> 0100 01 0001 00 0001 11 0100 10 as in the MOG. 0001 0001 0001 0001 0111 1000 --> 0000 01 0000 10 0000 10 1111 10 as in the MOG. 0010 0001 0001 0010 0001 1101 --> 0000 01 0000 01 1001 00 0110 11 as in the MOG. 0100 0001 0001 0100 0100 1110 --> 0000 01 1001 11 0000 01 0110 00 as in the MOG. 1000 0001 0001 0111 0010 0100 --> 10 00 00 00 01 01 00 01 10 01 11 00 as in the MOG (heavy brick at center).
Update at 7:41 PM ET the same day —
A check of SPLAG shows that the above result is not new:
And at 7:59 PM ET the same day —
Conway seems to be saying that at some unspecified point in the past,
M.J.T. Guy, examining the lexicographic Golay code, found (as I just did)
that weight-8 lexicographic Golay codewords, when arranged naturally
in 4×6 arrays, yield certain intriguing visual patterns. If the MOG existed
at the time of his discovery, he would have identified these patterns as
those of the MOG. (Lexicographic codes have apparently been
known since 1960, the MOG since the early 1970s.)
* Addendum at 4 AM ET the next day —
See also Logline (Walpurgisnacht 2013).
Comments Off on The Lexicographic Octad Generator (LOG)*
Thursday, February 27, 2020
Occult Writings
From the author who in 2001 described "God's fingerprint"
(see the previous post) —
From the same publisher —
From other posts tagged Triskele in this journal —
Other geometry for enthusiasts of the esoteric —
|
Monday, November 4, 2019
As Above, So Below*
|
|
|
Comments Off on Occult Writings
Tuesday, January 28, 2020
Very Stable Kool-Aid
Two of the thumbnail previews
from yesterday's 1 AM post …
Further down in the "6 Prescott St." post, the link 5 Divinity Avenue
leads to …
|
A Letter from Timothy Leary, Ph.D., July 17, 1961
Harvard University July 17, 1961
Dr. Thomas S. Szasz Dear Dr. Szasz: Your book arrived several days ago. I've spent eight hours on it and realize the task (and joy) of reading it has just begun. The Myth of Mental Illness is the most important book in the history of psychiatry. I know it is rash and premature to make this earlier judgment. I reserve the right later to revise and perhaps suggest it is the most important book published in the twentieth century. It is great in so many ways–scholarship, clinical insight, political savvy, common sense, historical sweep, human concern– and most of all for its compassionate, shattering honesty. . . . . |
The small Morton Prince House in the above letter might, according to
the above-quoted remarks by Corinna S. Rohse, be called a "jewel box."
Harvard moved it in 1978 from Divinity Avenue to its current location at
6 Prescott Street.
Related "jewel box" material for those who
prefer narrative to mathematics —
"In The Electric Kool-Aid Acid Test , Tom Wolfe writes about encountering
'a young psychologist,' 'Clifton Fadiman’s nephew, it turned out,' in the
waiting room of the San Mateo County jail. Fadiman and his wife were
'happily stuffing three I-Ching coins into some interminable dense volume*
of Oriental mysticism' that they planned to give Ken Kesey, the Prankster-
in-Chief whom the FBI had just nabbed after eight months on the lam.
Wolfe had been granted an interview with Kesey, and they wanted him to
tell their friend about the hidden coins. During this difficult time, they
explained, Kesey needed oracular advice."
— Tim Doody in The Morning News web 'zine on July 26, 2012**
Oracular advice related to yesterday evening's
"jewel box" post …
A 4-dimensional hypercube H (a tesseract ) has 24 square
2-dimensional faces. In its incarnation as a Galois tesseract
(a 4×4 square array of points for which the appropriate transformations
are those of the affine 4-space over the finite (i.e., Galois) two-element
field GF(2)), the 24 faces transform into 140 4-point "facets." The Galois
version of H has a group of 322,560 automorphisms. Therefore, by the
orbit-stabilizer theorem, each of the 140 facets of the Galois version has
a stabilizer group of 2,304 affine transformations.
Similar remarks apply to the I Ching In its incarnation as
a Galois hexaract , for which the symmetry group — the group of
affine transformations of the 6-dimensional affine space over GF(2) —
has not 322,560 elements, but rather 1,290,157,424,640.
* The volume Wolfe mentions was, according to Fadiman, the I Ching.
** See also this journal on that date — July 26, 2012.
Comments Off on Very Stable Kool-Aid
Sunday, January 26, 2020
Looking Glass Meets Rabbit Hole
The New York Times promoting paranoia on Jan. 24, 2020 —
"The fruit of that victory was a new economic logic that I call
'surveillance capitalism.' Its success depends upon one-way-mirror
operations engineered for our ignorance and wrapped in a fog of
misdirection, euphemism and mendacity. . . ."
"It’s not surprising that so many of us rushed to follow the bustling
White Rabbit down his tunnel into a promised digital Wonderland
where, like Alice, we fell prey to delusion."
|
Block those metaphors. |
Comments Off on Looking Glass Meets Rabbit Hole
Friday, November 8, 2019
Glitch
The terms glitch and cross-carrier in the previous post
suggest a review —
|
|
For some backstory, see Glitch, Gerard Manley Hopkins, Inscape —
particularly the post A Balliol Star.
Comments Off on Glitch
Sunday, July 7, 2019
Schoolgirl Problem
Anonymous remarks on the schoolgirl problem at Wikipedia —
"This solution has a geometric interpretation in connection with
Galois geometry and PG(3,2). Take a tetrahedron and label its
vertices as 0001, 0010, 0100 and 1000. Label its six edge centers
as the XOR of the vertices of that edge. Label the four face centers
as the XOR of the three vertices of that face, and the body center
gets the label 1111. Then the 35 triads of the XOR solution correspond
exactly to the 35 lines of PG(3,2). Each day corresponds to a spread
and each week to a packing."
See also Polster + Tetrahedron in this journal.
There is a different "geometric interpretation in connection with
Galois geometry and PG(3,2)" that uses a square model rather
than a tetrahedral model. The square model of PG(3,2) last
appeared in the schoolgirl-problem article on Feb. 11, 2017, just
before a revision that removed it.
Comments Off on Schoolgirl Problem
Tuesday, May 28, 2019
Quaternion at Candlebrow
From a Groundhog Day post in 2009 —
|
The Candlebrow Conference The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries. "Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–"
"Um, Professor–"…. … Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"…. … In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions…. "Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions." "Build a time machine." "Not the way I would have put it, but if you like, fine." Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up…. "We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly." "Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened. Above, the devastation had begun. |
"As if the professor had lectured it into being . . . ."
See other posts now tagged McLuhan Time.
Comments Off on Quaternion at Candlebrow
Wednesday, February 13, 2019
April 18, 2003 (Good Friday), Continued
"The purpose of mathematics cannot be derived from an activity
inferior to it but from a higher sphere of human activity, namely,
religion."
— Igor Shafarevitch, 1973 remark published as above in 1982.
"Perhaps."
— Steven H. Cullinane, February 13, 2019
|
From Log24 on Good Friday, April 18, 2003 — . . . What, indeed, is truth? I doubt that the best answer can be learned from either the Communist sympathizers of MIT or the “Red Mass” leftists of Georgetown. For a better starting point than either of these institutions, see my note of April 6, 2001, Wag the Dogma. See, too, In Principio Erat Verbum , which notes that “numbers go to heaven who know no more of God on earth than, as it were, of sun in forest gloom.” Since today is the anniversary of the death of MIT mathematics professor Gian-Carlo Rota, an example of “sun in forest gloom” seems the best answer to Pilate’s question on this holy day. See
“Examples are the stained glass windows Motto of Plato’s Academy † The Exorcist, 1973 |
Detail from an image linked to in the above footnote —
"And the darkness comprehended it not."
Id est :
A Good Friday, 2003, article by
a student of Shafarevitch —
"… there are 25 planes in W . . . . Of course,
replacing {a,b,c} by the complementary set
does not change the plane. . . ."
Of course.
See. however, Six-Set Geometry in this journal.
Comments Off on April 18, 2003 (Good Friday), Continued
Saturday, July 21, 2018
Comic-Con 2018
|
"For many of us, the geometry course sounded the death knell — "Shape and Space in Geometry"
© 1997-2003 Annenberg/CPB. All rights reserved. |
Comments Off on Comic-Con 2018
Friday, July 13, 2018
Segue for Harlan Ellison
From a Log24 post of March 13, 2003 —
|
"For many of us, the geometry course sounded the death knell — "Shape and Space in Geometry"
© 1997-2003 Annenberg/CPB. All rights reserved. |
Comments Off on Segue for Harlan Ellison
Saturday, April 7, 2018
Sides
The FBI holding cube in "The Blacklist" —
" 'The Front' is not the whole story . . . ."
— Vincent Canby, New York Times film review, 1976,
as quoted in Wikipedia.
See also Solomon's Cube in this journal.
Some may view the above web page as illustrating the
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate
in the spirit of the Glass Bead Game, everything actually
was all-meaningful, that every symbol and combination of
symbols led not hither and yon, not to single examples,
experiments, and proofs, but into the center, the mystery
and innermost heart of the world, into primal knowledge.
Every transition from major to minor in a sonata, every
transformation of a myth or a religious cult, every classical
or artistic formulation was, I realized in that flashing moment,
if seen with a truly meditative mind, nothing but a direct route
into the interior of the cosmic mystery, where in the alternation
between inhaling and exhaling, between heaven and earth,
between Yin and Yang, holiness is forever being created.”
A less poetic meditation on the above 4x4x4 design cube —
"I saw that in the alternation between front and back,
between top and bottom, between left and right,
symmetry is forever being created."
See also a related remark by Lévi-Strauss in 1955:
"…three different readings become possible:
left to right, top to bottom, front to back."
Comments Off on Sides
Wednesday, April 12, 2017
Contracting the Spielraum
The contraction of the title is from group actions on
the ninefold square (with the center subsquare fixed)
to group actions on the eightfold cube.
From a post of June 4, 2014 …
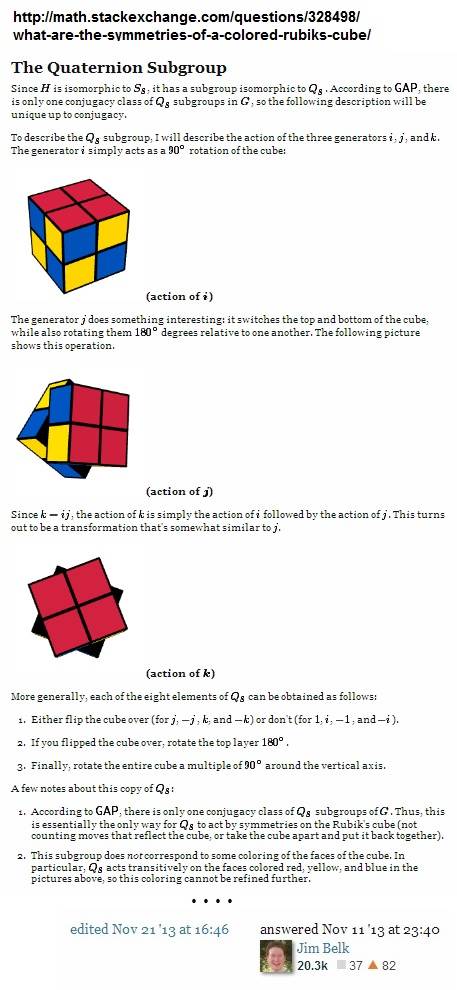
At math.stackexchange.com on March 1-12, 2013:
“Is there a geometric realization of the Quaternion group?” —
The above illustration, though neatly drawn, appeared under the
cloak of anonymity. No source was given for the illustrated group actions.
Possibly they stem from my Log24 posts or notes such as the Jan. 4, 2012,
note on quaternion actions at finitegeometry.org/sc (hence ultimately
from my note “GL(2,3) actions on a cube” of April 5, 1985).
Comments Off on Contracting the Spielraum
Saturday, February 18, 2017
Verbum
The Log24 version (Nov. 9, 2005, and later posts) —
|
VERBUM
|
See also related material in the previous post, Transformers.
Comments Off on Verbum
Sunday, October 2, 2016
Westworld
On a new HBO series that opens at 9 PM ET tonight —
|
Watching Westworld , you can sense a grand mythology unfolding before your eyes. The show’s biggest strength is its world-building, an aspect of screenwriting that many television series have botched before. Often shows will rush viewers into plot, forgetting to instill a sense of place and of history, that you’re watching something that doesn’t just exist in a vacuum but rather is part of some larger ecosystem. Not since Lost can I remember a TV show so committed to immersing its audience into the physical space it inhabits. (Indeed, Westworld can also be viewed as a meta commentary on the art of screenwriting itself: brainstorming narratives, building characters, all for the amusement of other people.) Westworld is especially impressive because it builds two worlds at once: the Western theme park and the futuristic workplace. The Western half of Westworld might be the more purely entertaining of the two, with its shootouts and heists and chases through sublime desert vistas. Behind the scenes, the theme park’s workers show how the robot sausage is made. And as a dystopian office drama, the show does something truly original. — Adam Epstein at QUARTZ, October 1, 2016 |
"… committed to immersing its audience
into the physical space it inhabits…."
See also, in this journal, the Mimsy Cube —
|
"Mimsy Were the Borogoves," "… he lifted a square, transparent crystal block, small enough to cup in his palm– much too small to contain the maze of apparatus within it. In a moment Scott had solved that problem. The crystal was a sort of magnifying glass, vastly enlarging the things inside the block. Strange things they were, too. Miniature people, for example– They moved. Like clockwork automatons, though much more smoothly. It was rather like watching a play."
|
Comments Off on Westworld
Saturday, May 14, 2016
The Hourglass Code
A version of the I Ching’s Hexagram 19:
From Katherine Neville's The Eight , a book on the significance
of the date April 4 — the author's birthday —
|
The Eight by Katherine Neville —
“What does this have to do with why we’re here?” |
Related material: Posts now tagged Hourglass Code.
See also the hourglass in a search for Pilgrim's Progress Illustration.
Comments Off on The Hourglass Code
Tuesday, May 3, 2016
Symmetry
A note related to the diamond theorem and to the site
Finite Geometry of the Square and Cube —
The last link in the previous post leads to a post of last October whose
final link leads, in turn, to a 2009 post titled Summa Mythologica .
Some may view the above web page as illustrating the
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate
in the spirit of the Glass Bead Game, everything actually
was all-meaningful, that every symbol and combination of
symbols led not hither and yon, not to single examples,
experiments, and proofs, but into the center, the mystery
and innermost heart of the world, into primal knowledge.
Every transition from major to minor in a sonata, every
transformation of a myth or a religious cult, every classical
or artistic formulation was, I realized in that flashing moment,
if seen with a truly meditative mind, nothing but a direct route
into the interior of the cosmic mystery, where in the alternation
between inhaling and exhaling, between heaven and earth,
between Yin and Yang, holiness is forever being created.”
A less poetic meditation on the above web page* —
"I saw that in the alternation between front and back,
between top and bottom, between left and right,
symmetry is forever being created."
Update of Sept. 5, 2016 — See also a related remark
by Lévi-Strauss in 1955: "…three different readings
become possible: left to right, top to bottom, front
to back."
* For the underlying mathematics, see a June 21, 1983, research note.
Comments Off on Symmetry
Thursday, April 21, 2016
The Alchemist’s Chessboard
Material related to the previous post and to Alfred Bester's
1981 followup to The Stars My Destination titled The Deceivers —
|
The Lapis Philosophorum :
"The lapis was thought of as a unity and therefore often stands for the prima materia in general."
"Its discoverer was of the opinion that he had produced the equivalent of the primordial protomatter which exploded into the Universe." And from Bester's The Deceivers : Meta Physics "'… Think of a match. You've got a chemical head of potash, antimony, and stuff, full of energy waiting to be released. Friction does it. But when Meta excites and releases energy, it's like a stick of dynamite compared to a match. It's the chess legend for real.' 'I don't know it.' 'Oh, the story goes that a philosopher invented chess for the amusement of an Indian rajah. The king was so delighted that he told the inventor to name his reward and he'd get it, no matter what. The philosopher asked that one grain of rice be placed on the first square of the chessboard, two on the second, four on the third, and so on to the sixty-fourth.' 'That doesn't sound like much.'" Related material : |
Comments Off on The Alchemist’s Chessboard
Tuesday, December 15, 2015
Square Triangles
Click image for some background.
Exercise: Note that, modulo color-interchange, the set of 15 two-color
patterns above is invariant under the group of six symmetries of the
equilateral triangle. Are there any other such sets of 15 two-color triangular
patterns that are closed as sets , modulo color-interchange, under the six
triangle symmetries and under the 322,560 permutations of the 16
subtriangles induced by actions of the affine group AGL(4,2)
on the 16 subtriangles' centers , given a suitable coordinatization?
Comments Off on Square Triangles
Thursday, July 2, 2015
Deepening the Spielraum
(A sequel to Expanding the Spielraum (Feb. 3, 2015))
"Knowledge, wisdom even, lies in depth, not extension."
— Tim Parks in The New York Review of Books ,
5 PM ET on June 26, 2015
See also Log24 posts on the following figure —
Comments Off on Deepening the Spielraum
Thursday, June 11, 2015
Omega
Omega is a Greek letter, Ω , used in mathematics to denote
a set on which a group acts.
For instance, the affine group AGL(3,2) is a group of 1,344
actions on the eight elements of the vector 3-space over the
two-element Galois field GF(2), or, if you prefer, on the Galois
field Ω = GF(8).
Related fiction: The Eight , by Katherine Neville.
Related non-fiction: A remark by Werner Heisenberg
in this journal on Saturday, June 6, 2015, the eightfold cube ,
and the illustrations below —
|
Mathematics
The Fano plane block design |
Magic
The Deathly Hallows symbol— |
Comments Off on Omega
Friday, May 8, 2015
Spielraum
Review:
Illustrating the Spiegel-Spiel des Gevierts
|
"At the point of convergence
by Octavio Paz, translated by |
Friday December 5, 2008
|
Comments Off on Spielraum
Saturday, April 25, 2015
Ghosts and Shadows
For Poetry Month
From the home page of Alexandre Borovik:
Book in progress: Shadows of the Truth
This book (to be published soon) can be viewed
as a sequel to Mathematics under the Microscope ,
but with focus shifted on mathematics as it was
experienced by children (well, by children who
became mathematicians). The cover is designed
by Edmund Harriss.
See also Harriss's weblog post of Dec. 27, 2008, on the death
of Harold Pinter: "The Search for the Truth Can Never Stop."
This suggests a review of my own post of Dec. 3, 2012,
"The Revisiting." A figure from that post:
Comments Off on Ghosts and Shadows
Thursday, February 26, 2015
A Simple Group
Comments Off on A Simple Group
Wednesday, December 3, 2014
Pyramid Dance
Oslo artist Josefine Lyche has a new Instagram post,
this time on pyramids (the monumental kind).
My response —
Wikipedia's definition of a tetrahedron as a
"triangle-based pyramid" …
… and remarks from a Log24 post of August 14, 2013 :
|
Norway dance (as interpreted by an American)
I prefer a different, Norwegian, interpretation of "the dance of four."
Related material: |
See also some of Burkard Polster's triangle-based pyramids
and a 1983 triangle-based pyramid in a paper that Polster cites —
(Click image below to enlarge.)
Some other illustrations that are particularly relevant
for Lyche, an enthusiast of magic :
From On Art and Magic (May 5, 2011) —
|
|
(Updated at about 7 PM ET on Dec. 3.)
Comments Off on Pyramid Dance
Tuesday, October 21, 2014
Art as a Tool
Two news items on art as a tool:
Two Log24 posts related to the 3×3 grid, the underlying structure for China’s
ancient Lo Shu “magic” square:
Finally, leftist art theorist Rosalind Krauss in this journal
on AntiChristmas, 2010:
Which is the tool here, the grid or Krauss?
Comments Off on Art as a Tool
Tools
(Night at the Museum continues.)
"Strategies for making or acquiring tools
While the creation of new tools marked the route to developing the social sciences,
the question remained: how best to acquire or produce those tools?"
— Jamie Cohen-Cole, “Instituting the Science of Mind: Intellectual Economies
and Disciplinary Exchange at Harvard’s Center for Cognitive Studies,”
British Journal for the History of Science vol. 40, no. 4 (2007): 567-597.
Obituary of a co-founder, in 1960, of the Center for Cognitive Studies at Harvard:
"Disciplinary Exchange" —
In exchange for the free Web tools of HTML and JavaScript,
some free tools for illustrating elementary Galois geometry —
The Kaleidoscope Puzzle, The Diamond 16 Puzzle,
The 2x2x2 Cube, and The 4x4x4 Cube
"Intellectual Economies" —
In exchange for a $10 per month subscription, an excellent
"Quilt Design Tool" —
This illustrates not geometry, but rather creative capitalism.
Related material from the date of the above Harvard death: Art Wars.
Comments Off on Tools
Tuesday, July 15, 2014
Photo Opportunity
"I need a photo opportunity, I want a shot at redemption.
Don't want to end up a cartoon in a cartoon graveyard."
– Paul Simon
|
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less.
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951) |
For background on the planes illustrated above,
see Diamond theory in 1937.
Comments Off on Photo Opportunity
Thursday, June 26, 2014
Study This Example, Part II
(Continued from 10:09 AM today)
The quotation below is from a webpage on media magnate
Walter Annenberg.
Annenberg Hall at Harvard, originally constructed to honor
the Civil War dead, was renamed in 1996 for his son Roger,
Harvard Class of ’62.
www.broadcastpioneers.com/
walterannenberg.html —
“It was said that Roger was ‘moody and sullen’
spending large parts of his time reading poetry
and playing classical music piano. It had been
reported that Roger attempted suicide at the
age of eleven by slitting his wrists. He recovered
and was graduated Magna Cum Laude from
Episcopal Academy in our area. For awhile,
Roger attended Harvard, but he was removed
from the school’s rolls after Roger stopped doing
his school work and spent almost all his time
reading poetry in his room. He then was sent to
an exclusive and expensive treatment center
in Bucks County, Pennsylvania. At that facility,
Roger became more remote. It was said that he
often didn’t recognize or acknowledge his father.
On August 7, 1962, Roger Annenberg died from
an overdose of sleeping pills.”
A more appropriate Annenberg memorial, an article
in The Atlantic magazine on June 25, notes that…
“Among those who ended up losing their battles
with mental illness through suicide are
Virginia Woolf, Ernest Hemingway, Vincent van Gogh,
John Berryman, Hart Crane, Mark Rothko, Diane Arbus,
Anne Sexton, and Arshile Gorky.”
Comments Off on Study This Example, Part II
Thursday, June 5, 2014
Twisty Quaternion Symmetry
The previous post told how user58512 at math.stackexchange.com
sought in 2013 a geometric representation of Q8 , the quaternion group.
He ended up displaying an illustration that very possibly was drawn,
without any acknowledgement of its source, from my own work.
On the date that user58512 published that illustration, he further
pursued his March 1, 2013, goal of a “twisty” quaternion model.
On March 12, 2013, he suggested that the quaternion group might be
the symmetry group of the following twisty-cube coloring:
Illustration by Jim Belk
Here is part of a reply by Jim Belk from Nov. 11, 2013, elaborating on
that suggestion:
Belk argues that the colored cube is preserved under the group
of actions he describes. It is, however, also preserved under a
larger group. (Consider, say, rotation of the entire cube by 180
degrees about the center of any one of its checkered faces.) The
group Belk describes seems therefore to be a symmetry group,
not the symmetry group, of the colored cube.
I do not know if any combination puzzle has a coloring with
precisely the quaternion group as its symmetry group.
(Updated at 12:15 AM June 6 to point out the larger symmetry group
and delete a comment about an arXiv paper on quaternion group models.)
Comments Off on Twisty Quaternion Symmetry
Wednesday, February 5, 2014
Mystery Box II
Continued from previous post and from Sept. 8, 2009.
Examination of the box's contents does not solve
the contents' real mystery. That requires knowledge
of the non-Euclidean geometry of Galois space.
In this case, without that knowledge, prattle (as in
today's online New York Times ) about creativity and
"thinking outside the box" is pointless.
Comments Off on Mystery Box II
Wednesday, January 15, 2014
Entertainment Theory
From "Entertainment," a 1981 story by M. A. Foster—
|
"For some time, Cormen had enjoyed a peculiar suspicion, which he had learned from his wanderings around the city, and cultivated with a little notebook, in which he had made a detailed series of notes and jottings, as well as crude, but effective, charts and maps of certain districts. 'Cormen's Problem,' as it was known, was familiar to the members of the circle in which he moved; in fact, if he had not been so effective with his productions and so engaging in his personality, they might have considered him a bore. It seemed, so the suspicion went, that the city was slowly shrinking, as evidenced by abandoned districts along the city edges. Beyond the empty houses were ruins, and beyond that, traces of foundations and street lines. Moreover, it had recently dawned on him that there were no roads out of the city, although there were no restraints. One hardly noticed this—it was the norm. But like many an easy assumption, once broken it became increasingly obvious. Cormen's acquaintances were tolerant of his aberration, but generally unsympathetic. What he needed was proof, something he could demonstrate in black and white—and color if required. But the city was reluctant, so it appeared, to give up its realities so easily. The Master Entertainment Center, MEC, would not answer direct queries about this, even though it would obediently show him presentations, pictorial or symbolic as he required, of the areas in question. But it was tiresome detail work, in which he had to proceed completely on his own." |
Lily Collins in City of Bones (2013)—
American Folk Art (see August 23, 2011) —
See as well Ballet Blanc .
Comments Off on Entertainment Theory
Tuesday, August 20, 2013
The 20
In memory of author Elmore Leonard—
A graphic symbol and a search for "Nowhere"*
in this journal yield…
|
Pictorial version |
"Cotton Mather died
— Wallace Stevens, |
* See previous post.
Comments Off on The 20
Saturday, May 11, 2013
Core
Promotional description of a new book:
"Like Gödel, Escher, Bach before it, Surfaces and Essences will profoundly enrich our understanding of our own minds. By plunging the reader into an extraordinary variety of colorful situations involving language, thought, and memory, by revealing bit by bit the constantly churning cognitive mechanisms normally completely hidden from view, and by discovering in them one central, invariant core— the incessant, unconscious quest for strong analogical links to past experiences— this book puts forth a radical and deeply surprising new vision of the act of thinking."
"Like Gödel, Escher, Bach before it…."
Or like Metamagical Themas .
Rubik core:
Non- Rubik cores:
|
Of the odd nxnxn cube:
|
Of the even nxnxn cube:
|
Related material: The Eightfold Cube and…
"A core component in the construction
is a 3-dimensional vector space V over F2 ."
— Page 29 of "A twist in the M24 moonshine story,"
by Anne Taormina and Katrin Wendland.
(Submitted to the arXiv on 13 Mar 2013.)
Comments Off on Core
Tuesday, March 19, 2013
Mathematics and Narrative (continued)
Angels & Demons meet Hudson Hawk
Dan Brown's four-elements diamond in Angels & Demons :
The Leonardo Crystal from Hudson Hawk :
Mathematics may be used to relate (very loosely)
Dan Brown's fanciful diamond figure to the fanciful
Leonardo Crystal from Hudson Hawk …
-
Compare Brown's fictional Illuminati Diamond to the
nonfictional figures in The Diamond Theorem and
Theme and Variations. -
Compare the fictional Leonardo Crystal to Hudson's
nonfictional desmic system of tetrahedra (above), and
see, in Rosenhain and Göpel Tetrads in PG(3,2), how
the diamond theorem is related to Hudson's work.
For the tetrads ' relationship to tetrahedra , see
Hudson's own book.
"Giving himself a head rub, Hawk bears down on
the three oddly malleable objects. He TANGLES
and BENDS and with a loud SNAP, puts them together,
forming the Crystal from the opening scene."
— A screenplay of Hudson Hawk
Happy birthday to Bruce Willis.
Comments Off on Mathematics and Narrative (continued)
Thursday, March 7, 2013
Ten Years After
Rock guitarist Alvin Lee, a founder of
the band Ten Years After , died
on March 6, 2013 (Michelangelo's
birthday). In his memory, a figure
from a post Ten Years Before —
Plato's reported motto for his Academy:
"Let no one ignorant of geometry enter."
For visual commentary by an artist ignorant
of geometry, see a work by Sol LeWitt.
For verbal commentary by an art critic ignorant
of geometry, see a review of LeWitt by
Robert Hughes—
"A Beauty Really Bare" (TIME, Feb. 6, 2001).
See also Ten Years Group and Four Gods.
Comments Off on Ten Years After
Tuesday, February 19, 2013
Configurations
Yesterday's post Permanence dealt with the cube
as a symmetric model of the finite projective plane
PG(2,3), which has 13 points and 13 lines. The points
and lines of the finite geometry occur in the cube as
the 13 axes of symmetry and the 13 planes through
the center perpendicular to those axes. If the three
axes lying in a plane that cuts the cube in a hexagon
are supplemented by the axis perpendicular to that
plane, each plane is associated with four axes and,
dually, each axis is associated with four planes.
My web page on this topic, Cubist Geometries, was
written on February 27, 2010, and first saved to the
Internet Archive on Oct. 4, 2010.
For a more recent treatment of this topic that makes
exactly the same points as the 2010 page, see p. 218
of Configurations from a Graphical Viewpoint , by
Tomaž Pisanski and Brigitte Servatius, published by
Springer on Sept. 23, 2012 (date from both Google
Books and Amazon.com):
For a similar 1998 treatment of the topic, see Burkard Polster's
A Geometrical Picture Book (Springer, 1998), pp. 103-104.
The Pisanski-Servatius book reinforces my argument of Jan. 13, 2013,
that the 13 planes through the cube's center that are perpendicular
to the 13 axes of symmetry of the cube should be called the cube's
symmetry planes , contradicting the usual use of of that term.
That argument concerns the interplay between Euclidean and
Galois geometry. Pisanski and Servatius (and, in 1998, Polster)
emphasize the Euclidean square and cube as guides* to
describing the structure of a Galois space. My Jan. 13 argument
uses Galois structures as a guide to re-describing those of Euclid .
(For a similar strategy at a much more sophisticated level,
see a recent Harvard Math Table.)
Related material: Remarks on configurations in this journal
during the month that saw publication of the Pisanski-Servatius book.
* Earlier guides: the diamond theorem (1978), similar theorems for
2x2x2 (1984) and 4x4x4 cubes (1983), and Visualizing GL(2,p)
(1985). See also Spaces as Hypercubes (2012).
Comments Off on Configurations
Friday, January 18, 2013
Solomon’s Rep-tiles
"Rep-tiles Revisited," by Viorel Nitica, in MASS Selecta: Teaching and Learning Advanced Undergraduate Mathematics , American Mathematical Society,
"The goal of this note is to take a new look at some of the most amazing objects discovered in recreational mathematics. These objects, having the curious property of making larger copies of themselves, were introduced in 1962 by Solomon W. Golomb [2], and soon afterwards were popularized by Martin Gardner [3] in Scientific American…."
2. S. W. Golomb: "Replicating Figures in the Plane," Mathematical Gazette 48, 1964, 403-412
3. M. Gardner: "On 'Rep-tiles,' Polygons That Can Make Larger and Smaller Copies of Themselves," Scientific American 208, 1963, 154-157
Two such "amazing objects"—
|
Triangle |
Square |
For a different approach to the replicating properties of these objects, see the square-triangle theorem.
For related earlier material citing Golomb, see Not Quite Obvious (July 8, 2012; scroll down to see the update of July 15.).
Golomb's 1964 Gazette article may now be purchased at JSTOR for $14.
Comments Off on Solomon’s Rep-tiles
Thursday, January 17, 2013
Brazil Revisited
Yesterday's post Treasure Hunt, on a Brazilian weblog,
suggests a review of Brazil in this journal. The post
most relevant to yesterday's remarks is from
August 15, 2003, with a link, now broken, to the work
of Brazilian artist Nicole Sigaud* that also uses the
four half-square tiles used in 1704 by Sebastien Truchet
and somewhat later by myself in Diamond Theory
(see a 1977 version).
A more recent link that works:
|
http://vismath9.tripod.com/sigaud/e-index.html ANACOM PROJECT
APPLICATIONS
© 1997 – 2002 Nicole Sigaud |
* Sigaud shares the interests of her fellow Brazilian
whose weblog was the subject of yesterday's
Treasure Hunt.—
"For many years I have dedicated myself to the study
of medieval magic, demonology, Kabbalah, Astrology,
Alchemy, Tarot and divination in general."
— Nicole Sigaud (translated by Google) in a self-profile:
http://www.recantodasletras.com.br/autor.php?id=78359.
I do not share the interest of these authors in such matters,
except as they are reflected in the works of authors like
Charles Williams and Umberto Eco.
Comments Off on Brazil Revisited
Saturday, December 29, 2012
Mapping Problem
A mapping problem posed (informally) in 1985
and solved 27 years later, in 2012:
See also Finite Relativity and Finite Relativity: The Triangular Version.
(A note for fans of the recent film Looper (see previous post)—
Hunter S. Thompson in this journal on February 22, 2005 …
Hunter S. Thompson, photos from The New York Times
… and on March 3, 2009.)
Comments Off on Mapping Problem
Monday, December 24, 2012
All Over Again
"… the movement of analogy
begins all over once again."
See A Reappearing Number in this journal.
Illustrations:
Figure 1 —
Background: MOG in this journal.
Figure 2 —
Background —
Comments Off on All Over Again
Sunday, December 9, 2012
Eve’s Menorah
"Now the serpent was more subtle
than any beast of the field…."
— Genesis 3:1
"“The serpent’s eyes shine
As he wraps around the vine….”
– Don Henley
"Nine is a vine."
— Folk rhyme
Click images for some background.
Comments Off on Eve’s Menorah
Thursday, November 29, 2012
Conceptual Art
Quotes from the Bremen site
http://dada.compart-bremen.de/ —
" 'compArt | center of excellence digital art' is a project
at the University of Bremen, Germany. It is dedicated
to research and development in computing, design,
and teaching. It is supported by Rudolf Augstein Stiftung,
the University of Bremen, and Karin und Uwe Hollweg Stiftung."
See also Stiftung in this journal.
Comments Off on Conceptual Art
Monday, November 19, 2012
Poetry and Truth
From today's noon post—
"In all his poems with all their enchantments
for the poet himself, there is the final enchantment
that they are true. The significance of the poetic act
then is that it is evidence. It is instance and illustration.
It is an illumination of a surface,
the movement of a self in the rock.
Above all it is a new engagement with life.
It is that miracle to which the true faith of the poet
attaches itself."
— Wallace Stevens at Bard College, March 30, 1951
Stevens also said at Bard that
"When Joan of Arc said:
Have no fear: what I do, I do by command.
My brothers of Paradise tell me what I have to do.
these words were the words of an hallucination.
No matter what her brothers of Paradise drove her to do,
what she did was never a poetic act of faith in reality
because it could not be."
There are those who would dispute this.
Some related material:
"Ageometretos me eisito."—
"Let no one ignorant of geometry enter."—
Said to be a saying of Plato, part of the
seal of the American Mathematical Society—
A poetic approach to geometry—
"A surface" and "the rock," from All Saints' Day, 2012—
— and from 1981—
Some mathematical background for poets in Purgatory—
"… the Klein correspondence underlies Conwell's discussion
of eight heptads. These play an important role in another
correspondence, illustrated in the Miracle Octad Generator
of R. T. Curtis, that may be used to picture actions
of the large Mathieu group M24."
Comments Off on Poetry and Truth
Sunday, November 18, 2012
Sermon
Happy birthday to…
Today's sermon, by Marie-Louise von Franz—
For more on the modern physicist analyzed by von Franz,
see The Innermost Kernel , by Suzanne Gieser.
Another modern physicist, Niels Bohr, died
on this date in 1962…
|
The circle above is marked with a version For the square, see the diamond theorem. "Two things of opposite natures seem to depend — Wallace Stevens, |
Comments Off on Sermon
Sunday, July 8, 2012
Not Quite Obvious
"That n 2 points fall naturally into a triangular array
is a not-quite-obvious fact which may have applications…
and seems worth stating more formally."
— Steven H. Cullinane, letter in the
American Mathematical Monthly 1985 June-July issue
If the ancient Greeks had not been distracted by
investigations of triangular (as opposed to square )
numbers, they might have done something with this fact.
A search for occurrences of the phrase
"n2 [i.e., n 2 ] congruent triangles"
indicates only fairly recent (i.e., later than 1984) results.*
Some related material, updated this morning—
|
This suggests a problem—
What mappings of a square array of n 2 points to
In the figure above, whether |
* Update of July 15, 2012 (11:07 PM ET)—
Theorem on " rep-n 2 " (Golomb's terminology)
triangles from a 1982 book—
Comments Off on Not Quite Obvious
Saturday, May 26, 2012
Harriot’s Cubes
See also Finite Geometry and Physical Space.
Related material from MacTutor—
The paper by J. W. Shirley, Binary numeration before Leibniz, Amer. J. Physics 19 (8) (1951), 452-454, contains an interesting look at some mathematics which appears in the hand written papers of Thomas Harriot [1560-1621]. Using the photographs of the two original Harriot manuscript pages reproduced in Shirley’s paper, we explain how Harriot was doing arithmetic with binary numbers. Leibniz [1646-1716] is credited with the invention [1679-1703] of binary arithmetic, that is arithmetic using base 2. Laplace wrote:-
However, Leibniz was certainly not the first person to think of doing arithmetic using numbers to base 2. Many years earlier Harriot had experimented with the idea of different number bases…. |
For a discussion of Harriot on the discrete-vs.-continuous question,
see Katherine Neal, From Discrete to Continuous: The Broadening
of Number Concepts in Early Modern England (Springer, 2002),
pages 69-71.
Comments Off on Harriot’s Cubes
Tuesday, May 22, 2012
Included Middle
|
"In logic, the law of excluded middle (or the principle of excluded middle) is the third of the so-called three classic laws of thought. It states that for any proposition, either that proposition is true, or its negation is. The law is also known as the law (or principle) of the excluded third (or of the excluded middle), or, in Latin, principium tertii exclusi. Yet another Latin designation for this law is tertium non datur: 'no third (possibility) is given.'" |
"Clowns to the left of me, jokers to the right"
— Songwriter who died on January 4, 2011.
Online NY Times on the date of the songwriter's death—
"A version of this review appeared in print
on January 4, 2011, on page C6 of the New York edition."
"The philosopher Hubert Dreyfus and his former student
Sean Dorrance Kelly have a story to tell, and it is not
a pretty tale for us moderns. Ours is an age of nihilism,
they say, meaning not so much that we have nothing
in which to believe, but that we don’t know how to choose
among the various things to which we might commit
ourselves. Looking down from their perches at Berkeley
and Harvard, they see the 'human indecision that
plagues us all.'"
For an application of the excluded-middle law, see
Non-Euclidean Blocks and Deep Play.
Violators of the law may have trouble* distinguishing
between "Euclidean" and "non-Euclidean" phenomena
because their definition of the latter is too narrow,
based only on examples that are historically well known.
See the Non-Euclidean Blocks footnote.
* Followers of the excluded-middle law will avoid such
trouble by noting that "non-Euclidean" should mean
simply "not Euclidean in some way "— not necessarily
in a way contradicting Euclid's parallel postulate.
But see Wikipedia's defense of the standard, illogical,
usage of the phrase "non-Euclidean."
Postscript—
Tertium Datur
"Here I am, stuck in the middle with you."
Comments Off on Included Middle
Saturday, May 19, 2012
G8
"The group of 8" is a phrase from politics, not mathematics.
Of the five groups of order 8 (see today's noon post),
the one pictured* in the center, Z2 × Z2 × Z2 , is of particular
interest. See The Eightfold Cube. For a connection of this
group of 8 to the last of the five pictured at noon, the
quaternion group, see Finite Geometry and Physical Space.
* The picture is of the group's cycle graph.
Comments Off on G8
Thursday, May 3, 2012
Everybody Comes to Rick’s
“The key is the cocktail that begins the proceedings.”
– Brian Harley, Mate in Two Moves
See also yesterday's Endgame , as well as Play and Interplay
from April 28… and, as a key, the following passage from
an earlier April 28 post—
| Euclidean geometry has long been applied to physics; Galois geometry has not. The cited webpage describes the interplay of both sorts of geometry— Euclidean and Galois, continuous and discrete— within physical space— if not within the space of physics . |
Comments Off on Everybody Comes to Rick’s
Friday, March 23, 2012
Embedding the Stone
"Imbedding the God character in a holy book's very detailed narrative
and building an entire culture around this narrative
seems by itself to confer a kind of existence on Him."
— John Allen Paulos in the philosophy column "The Stone,"
New York Times online, Oct. 24, 2010
A related post from Log24 later that year—
Sunday, November 28, 2010
|
"Next come the crown of thorns and Jesus' agonized crawl across the stage,
bearing the weight of his own crucifix. And at last, after making
yet another entrance, Mr. Nolan strikes the pose immortalized
in centuries of art, clad in a demure loincloth, arms held out to his sides,
one leg artfully bent in front of the other, head hanging down
in tortured exhaustion. Gently spotlighted, he rises from the stage
as if by magic, while a giant cross, pulsing with hot gold lights,
descends from above to meet him. Mr. Lloyd Webber's churning guitar rock
hits a climactic note, and the audience erupts in excited applause."
— Charles Isherwood, review of "Jesus Christ Superstar" in today's New York Times
Other remarks on embedding —
Part I
Review of a new book on linguistics, embedding, and a South American tribe—
"Imagine a linguist from Mars lands on Earth to survey the planet's languages…."
— Chronicle of Higher Education , March 20, 2012
Part II
The Embedding , by Ian Watson (Review of a 1973 novel from Shakespeare's birthday, 2006)
Comments Off on Embedding the Stone
Wednesday, March 21, 2012
Square-Triangle Theorem
(Continued from March 18, 2012)
Found in a search this evening—
How Does One Cut a Triangle? by Alexander Soifer
(Second edition, Springer, 2009. First edition published
by Soifer's Center for Excellence in Mathematical Education,
Colorado Springs, CO, in 1990.)
This book, of xxx + 174 pages, covers questions closely related
to the "square-triangle" result I published in a letter to the
editor of the June-July 1985 American Mathematical Monthly
(Vol. 92, No. 6, p. 443). See Square-Triangle Theorem.
Soifer's four pages of references include neither that letter
nor the Monthly item, "Miscellaneum 129: Triangles are square"
of a year earlier that prompted the letter.
Comments Off on Square-Triangle Theorem
Sunday, March 18, 2012
Square-Triangle Diamond
The diamond shape of yesterday's noon post
is not wholly without mathematical interest …
"Every triangle is an n -replica" is true
if and only if n is a square.
The 16 subdiamonds of the above figure clearly
may be mapped by an affine transformation
to 16 subsquares of a square array.
(See the diamond lattice in Weyl's Symmetry .)
Similarly for any square n , not just 16.
There is a group of 322,560 natural transformations
that permute the centers of the 16 subsquares
in a 16-part square array. The same group may be
viewed as permuting the centers of the 16 subtriangles
in a 16-part triangular array.
(Updated March 29, 2012, to correct wording and add Weyl link.)
Comments Off on Square-Triangle Diamond
Saturday, March 17, 2012
The Purloined Diamond
The diamond from the Chi-rho page
of the Book of Kells —
The diamond at the center of Euclid's
Proposition I, according to James Joyce
(i.e., the Diamond in the Mandorla) —
“He pointed at the football
on his desk. ‘There it is.’”
– Glory Road
Comments Off on The Purloined Diamond
Tuesday, January 31, 2012
Tesseract
|
"… a finite set with n elements Tesseract formed from a 4-set—
The same 16 subsets or points can
"There is such a thing as a 4-set." |
Update of August 12, 2012:
Figures like the above, with adjacent vertices differing in only one coordinate,
appear in a 1950 paper of H. S. M. Coxeter—
Comments Off on Tesseract
Saturday, January 14, 2012
Defining Form (continued)
Detail of Sylvie Donmoyer picture discussed
here on January 10—
The "13" tile may refer to the 13 symmetry axes
in the 3x3x3 Galois cube, or the corresponding
13 planes through the center in that cube. (See
this morning's post and Cubist Geometries.)
Comments Off on Defining Form (continued)
Sunday, January 1, 2012
Sunday Shul
"… myths are stories, and like all narratives
they unravel through time, whereas grids
are not only spatial to start with,
they are visual structures that explicitly reject
a narrative or sequential reading of any kind."
— Rosalind Krauss in "Grids,"
October (Summer 1979), 9: 50-64.
Counterexample—
The Ninefold Square
See Coxeter and the Aleph and Ayn Sof—
| Mathematics and Narrative, Illustrated |
|
 Mathematics |
 Narrative |
Comments Off on Sunday Shul
Saturday, November 12, 2011
Professor Dodge
From today's previous post, a fragmentary thought—
"Professor Dodge and the underground artists
whose work he helped save are the subjects of a book…"
|
Jim Dodge, Stone Junction (a novel first published in 1989) From pages 206-208, Kindle Edition— `Have you seen it?' Volta hesitated. `Well, I've dreamed it.' Daniel shook his head. `I'm getting lost. You want me to vanish into your dreams?' `Good Lord, no,' Volta blanched. `That's exactly what I don't want you to do.' `So, what is it exactly you do want me to do?' `Steal the diamond.' `So, it's a diamond?' `Yes, though it's a bit like saying the ocean is water. The diamond is perfectly spherical,* perfectly clear— though it seems to glow— and it's about two-thirds the size of a bowling ball. I think of it as the Diamond. Capital D.' `Who owns it?' `No one. The United States government has it at the moment. We want it. And to be honest with you, Daniel, I particularly want it, want it dearly. I want to look at it, into it, hold it in my hands. I had a vision involving a spherical diamond, a vision that changed my life, and I want to confirm that it was a vision of something real, the spirit embodied, the circuit complete.' Daniel was smiling. `You're going to love this. That dream I wanted to talk to you about, my first since the explosion? It just happened to feature a raven with a spherical diamond in its beak. Obviously, it wasn't as big as a bowling ball, and there was a thin spiral flame running edge to edge through its center, which made it seem more coldly brilliant than warmly glowing, but it sounds like the same basic diamond to me.' `And what do you think it is?' `I think it's beautiful.' Volta gave him a thin smile. `If I were more perverse than I already lamentably am, I would say it is the Eye of the Beholder. In fact, I don't know what it is.' `It might be a dream,' Daniel said. `Very possibly,' Volta agreed, `but I don't think so. I think— feel , to be exact— that the Diamond is an interior force given exterior density, the transfigured metaphor of the prima materia , the primordial mass, the Spiritus Mundi . I'm assuming you're familiar with the widely held supposition that the entire universe was created from a tiny ball of dense matter which exploded, sending pieces hurtling into space, expanding from the center. The spherical diamond is the memory, the echo, the ghost of that generative cataclysm; the emblematic point of origin. Or if, as some astrophysicists believe, the universe will reach some entropic point in its expansion and begin to collapse back into itself, in that case the Diamond may be a homing point, the seed crystal, to which it will all come hurtling back together— and perhaps through itself, into another dimension entirely. Or it might be the literal Philosopher's Stone we alchemists speak of so fondly. Or I might be completely wrong. That's why I want to see it. If I could actually stand in its presence, I'm convinced I'd know what it is. I would even venture to say, at the risk of rabid projection, that it wants to be seen and known.' `But you're not even sure it exists,' Daniel said. `Right? And hey, it's tough to steal something that doesn't exist, even if you can be invisible. The more I think about this the less sense it makes.' * Here Dodge's mystical vision seems akin to that of Anthony Judge in "Embodying the Sphere of Change" (St. Stephen's Day, 2001). Actually, the cube, not the sphere, is the best embodiment of Judge's vision. |
See also Tuesday's "Stoned" and the 47 references
to the term "bowling" in the Kindle Stone Junction .
Furthermore… Live from New York, it's Saturday Night!
Comments Off on Professor Dodge
Friday, October 28, 2011
The Soul’s Code
James Hillman reportedly died on Thursday, October 27, 2011.
For some commentary, see Wednesday's link to 779—
|
|
Comments Off on The Soul’s Code
Thursday, September 8, 2011
Starring the Diamond
"In any geometry satisfying Pappus's Theorem,
the four pairs of opposite points of 83
are joined by four concurrent lines."
— H. S. M. Coxeter (see below)
Continued from Tuesday, Sept. 6—
The Diamond Star
The above is a version of a figure from Configurations and Squares.
Yesterday's post related the the Pappus configuration to this figure.
Coxeter, in "Self-Dual Configurations and Regular Graphs," also relates Pappus to the figure.
Some excerpts from Coxeter—
The relabeling uses the 8 superscripts
from the first picture above (plus 0).
The order of the superscripts is from
an 8-cycle in the Galois field GF(9).
The relabeled configuration is used in a discussion of Pappus—
(Update of Sept. 10, 2011—
Coxeter here has a note referring to page 335 of
G. A. Miller, H. F. Blichfeldt, and L. E. Dickson,
Theory and Applications of Finite Groups , New York, 1916.)
Coxeter later uses the the 3×3 array (with center omitted) again to illustrate the Desargues configuration—
The Desargues configuration is discussed by Gian-Carlo Rota on pp. 145-146 of Indiscrete Thoughts—
"The value of Desargues' theorem and the reason why the statement of this theorem has survived through the centuries, while other equally striking geometrical theorems have been forgotten, is in the realization that Desargues' theorem opened a horizon of possibilities that relate geometry and algebra in unexpected ways."
Comments Off on Starring the Diamond
Monday, August 8, 2011
Diamond Theory vs. Story Theory (continued)
Richard J. Trudeau, a mathematics professor and Unitarian minister, published in 1987 a book, The Non-Euclidean Revolution , that opposes what he calls the Story Theory of truth [i.e., Quine, nominalism, postmodernism] to what he calls the traditional Diamond Theory of truth [i.e., Plato, realism, the Roman Catholic Church]. This opposition goes back to the medieval "problem of universals" debated by scholastic philosophers.
(Trudeau may never have heard of, and at any rate did not mention, an earlier 1976 monograph on geometry, "Diamond Theory," whose subject and title are relevant.)
From yesterday's Sunday morning New York Times—
"Stories were the primary way our ancestors transmitted knowledge and values. Today we seek movies, novels and 'news stories' that put the events of the day in a form that our brains evolved to find compelling and memorable. Children crave bedtime stories…."
— Drew Westen, professor at Emory University
From May 22, 2009—
|
The above ad is by
Diamond from last night’s
|
|
For further details, see Saturday's correspondences |
Comments Off on Diamond Theory vs. Story Theory (continued)
Saturday, August 6, 2011
Correspondences
Comme de longs échos qui de loin se confondent
Dans une ténébreuse et profonde unité….
— Baudelaire, “Correspondances ”
From “A Four-Color Theorem”—
Figure 1
Note that this illustrates a natural correspondence
between
(A) the seven highly symmetrical four-colorings
of the 4×2 array at the left of Fig. 1, and
(B) the seven points of the smallest
projective plane at the right of Fig. 1.
To see the correspondence, add, in binary
fashion, the pairs of projective points from the
“points” section that correspond to like-colored
squares in a four-coloring from the left of Fig. 1.
(The correspondence can, of course, be described
in terms of cosets rather than of colorings.)
A different correspondence between these 7 four-coloring
structures and these 7 projective-line structures appears in
a structural analysis of the Miracle Octad Generator
(MOG) of R.T. Curtis—
Figure 2
| Here the correspondence between the 7 four-coloring structures (left section) and the 7 projective-line structures (center section) is less obvious, but more fruitful. It yields, as shown, all of the 35 partitions of an 8-element set (an 8-set ) into two 4-sets. The 7 four-colorings in Fig. 2 also appear in the 35 4×4 parts of the MOG that correspond, in a way indicated by Fig. 2, to the 35 8-set paritions. This larger correspondence— of 35 4×2 arrays with 35 4×4 arrays— is the MOG, at least as it was originally defined. See The MOG, Generating the Octad Generator, and Eightfold Geometry.
For some applications of the Curtis MOG, see |
Comments Off on Correspondences
Sunday, June 26, 2011
Paradigms Lost
Continued from March 10, 2011 — A post that says
"If Galois geometry is thought of as a paradigm shift
from Euclidean geometry, both… the Kuhn cover
and the nine-point affine plane may be viewed…
as illustrating the shift."
Yesterday's posts The Fano Entity and Theology for Antichristmas,
together with this morning's New York Times obituaries (below)—
—suggest a Sunday School review from last year's
Devil's Night (October 30-31, 2010)—
|
Sunday, October 31, 2010 ART WARS – m759 @ 2:00 AM … There is a Cave – Paradise Lost , by John Milton
|
See also Ash Wednesday Surprise and Geometry for Jews.
Comments Off on Paradigms Lost
Wednesday, June 8, 2011
For Saint Peter
… and Arthur Koestler
|
The theme of the January 2010 issue of the
Notices of the American Mathematical Society was “Mathematics and the Arts.”
Related material:
|
See also two posts from the day Peter Jennings died—
Comments Off on For Saint Peter
Monday, May 23, 2011
The Stoner Series
A reader comments on yesterday afternoon's New York Times
"The Stone" column by Justin E.H. Smith—
"I did indeed appreciate Mr. Smith’s essay.
And I’m curious as to what future contributions of his,
to the Stoner series, that we can look forward to."
From August 24, 2010—
|
|
Happy day 23 of Mental Health Month.
Comments Off on The Stoner Series
Sunday, May 22, 2011
Asterisk*
A year ago today—
Art Space
|
Pictorial version |
|
| “Space: what you damn well have to see.” – James Joyce, Ulysses |
|
* See Vonnegut.
Comments Off on Asterisk*
Sunday, May 8, 2011
Matrix
From Thomas Mann, "Schopenhauer," 1938, in Essays of Three Decades , translated by H. T. Lowe-Porter, Alfred A. Knopf, 1947, pp. 372-410—
Page 372: THE PLEASURE we take in a metaphysical system, the gratification purveyed by the intellectual organization of the world into a closely reasoned, complete, and balanced structure of thought, is always of a pre-eminently aesthetic kind. It flows from the same source as the joy, the high and ever happy satisfaction we get from art, with its power to shape and order its material, to sort out life's manifold confusions so as to give us a clear and general view.
Truth and beauty must always be referred the one to the other. Each by itself, without the support given by the other, remains a very fluctuating value. Beauty that has not truth on its side and cannot have reference to it, does not live in it and through it, would be an empty chimera— and "What is truth?"
Comments Off on Matrix
Sunday, May 1, 2011
Delos
The late translator Helen Lane in Translation Review , Vol. 5, 1980—
"Among the awards, I submit, should be one for the entire oeuvre of a lifetime "senior" translator— and one for the best first translation…. Similar organization, cooperation, and fund-finding for a first-rate replacement for the sorely missed Delos ."
This leads to one of the founders of Delos , the late Donald Carne-Ross, who died on January 9, 2010.
For one meditation on the date January 9, see Bridal Birthday (last Thursday).
Another meditation, from the date of Carne-Ross's death—
Saturday, January 9, 2010
Positional Meaning"The positional meaning of a symbol derives from its relationship to other symbols in a totality, a Gestalt, whose elements acquire their significance from the system as a whole." – Victor Turner, The Forest of Symbols , Ithaca, NY, Cornell University Press, 1967, p. 51, quoted by Beth Barrie in "Victor Turner." To everything, turn, turn, turn …
|
See also Delos in this journal.
Comments Off on Delos
Wednesday, April 27, 2011
Block That Metaphor–
A Note on Galois Geometry
|
Simple groups as the (Click image to enlarge.)
|
Points, lines, etc., as the
|
Related material —
(Click images for some background.)
|
Building blocks and
|
Building blocks and |
Comments Off on Block That Metaphor–
Tuesday, April 26, 2011
Unity and Multiplicity
Today's earlier post mentions one approach to the concepts of unity and multiplicity. Here is another.
Unity:
The 3×3×3 Galois Cube
Multiplicity:
One of a group, GL(3,3), of 11,232
natural transformations of the 3×3×3 Cube
See also the earlier 1985 3×3 version by Cullinane.
Comments Off on Unity and Multiplicity
Friday, April 22, 2011
Romancing the Hyperspace
For the title, see Palm Sunday.
"There is a pleasantly discursive treatment of
Pontius Pilate's unanswered question 'What is truth?'" — H. S. M. Coxeter, 1987
From this date (April 22) last year—
|
Richard J. Trudeau in The Non-Euclidean Revolution , chapter on "Geometry and the Diamond Theory of Truth"– "… Plato and Kant, and most of the philosophers and scientists in the 2200-year interval between them, did share the following general presumptions: (1) Diamonds– informative, certain truths about the world– exist. Presumption (1) is what I referred to earlier as the 'Diamond Theory' of truth. It is far, far older than deductive geometry." Trudeau's book was published in 1987. The non-Euclidean* figures above illustrate concepts from a 1976 monograph, also called "Diamond Theory." Although non-Euclidean,* the theorems of the 1976 "Diamond Theory" are also, in Trudeau's terminology, diamonds. * "Non-Euclidean" here means merely "other than Euclidean." No violation of Euclid's parallel postulate is implied. |
Trudeau comes to reject what he calls the "Diamond Theory" of truth. The trouble with his argument is the phrase "about the world."
Geometry, a part of pure mathematics, is not about the world. See G. H. Hardy, A Mathematician's Apology .
Comments Off on Romancing the Hyperspace
Friday, April 8, 2011
Concepts of Space
Part I — Roberta Smith in today's New York Times —
"… the argument that painting may ultimately be about
little more than the communication of some quality of
light and space, however abstract or indirect."
– Review of "Rooms With a View" at the Met
|
Pictorial version |
|
“Space: what you damn well have to see.”
– James Joyce, Ulysses
Part II — Window from A Crooked House
"Teal lifted the blind a few inches. He saw nothing, and raised it a little more—still nothing. Slowly he raised it until the window was fully exposed. They gazed out at—nothing.
Nothing, nothing at all. What color is nothing? Don't be silly! What shape is it? Shape is an attribute of something . It had neither depth nor form. It had not even blackness. It was nothing ."
Part III — Not So Crooked: The Cabinet of Dr. Montessori
An April 5 Wall Street Journal article on Montessori schools, and…
A cabinet from Dr. Montessori's own
explanation of her method
Part IV — Pilate Goes to Kindergarten and The Seven
Comments Off on Concepts of Space
Tuesday, March 29, 2011
Diamond Star
From last night's note on finite geometry—
"The (83, 83) Möbius-Kantor configuration here described by Coxeter is of course part of the larger (94, 123) Hesse configuration. Simply add the center point of the 3×3 Galois affine plane and the four lines (1 horizontal, 1 vertical, 2 diagonal) through the center point." An illustration—
This suggests a search for "diamond+star."
Comments Off on Diamond Star
Wednesday, March 16, 2011
Time and Chance (continued)
Accidental Time and Space
New York Lottery today— midday 987, evening 522.
Time
The midday 987 may be interpreted as "…nine, eight, seven, …."—
"The countdown as we know it, 10-9-8-u.s.w.,
was invented by Fritz Lang in 1929 for
the Ufa film Die Frau im Mond . He put it into
the launch scene to heighten the suspense.
'It is another of my damned "touches,"' Fritz Lang said."
Space
The evening 522 suggests the date 5/22. From that date last year—
Art Space (2:02 AM EDT)
|
Pictorial version |
|
“Space: what you damn well have to see.”
– James Joyce, Ulysses
Comments Off on Time and Chance (continued)
Monday, March 7, 2011
Point Taken
Recommended— An essay (part 1 of 5 parts) in today's New York TImes—
|
I don’t want to die in |
"I agree with one of the earlier commenters that this is a piece of fine literary work. And in response to some of those who have wondered 'WHAT IS THE POINT?!' of this essay, I would like to say: Must literature always answer that question for us (and as quickly and efficiently as possible)?"
For an excellent survey of the essay's historical context, see The Stanford Encyclopedia of Philosophy article
"The Incommensurability of Scientific Theories,"
First published Wed., Feb. 25, 2009,
by Eric Oberheim and Paul Hoyningen-Huene.
Related material from this journal—
Paradigms, Paradigms Lost, and a search for "mere geometry." This last includes remarks contrasting Euclid's definition of a point ("that which has no parts") with a later notion useful in finite geometry.
See also (in the spirit of The Abacus Conundrum )…
(Note the Borges epigraph above.)
Comments Off on Point Taken
Monday, February 28, 2011
His Kind of Diamond
In memory of Jane Russell —
H.S.M. Coxeter's classic
Introduction to Geometry (2nd ed.):
Note the resemblance of the central part to
a magical counterpart— the Ojo de Dios
of Mexico's Sierra Madre.
Related material— page 55 of Polly and the Aunt ,
by Mary E. Blatchford.
Comments Off on His Kind of Diamond
Tuesday, January 11, 2011
Soul and Spirit
This morning's post, "Shining," gave James Hillman's 1976 remarks
on the distinction between soul and spirit .
The following images may help illustrate these concepts.
The distinction as illustrated by Jeff Bridges —
|
Soul
|
Spirit
|
The mirror has two faces (at least).
Postscript from a story, "The Zahir," in the Borges manner,
by Mark Jason Dominus (programmer of the quilt designs above)—
"I left that madhouse gratefully."
Dominus is also the author of…
Comments Off on Soul and Spirit
Saturday, January 8, 2011
True Grid (continued)
"Rosetta Stone" as a Metaphor
in Mathematical Narratives
For some backgound, see Mathematics and Narrative from 2005.
Yesterday's posts on mathematics and narrative discussed some properties
of the 3×3 grid (also known as the ninefold square ).
For some other properties, see (at the college-undergraduate, or MAA, level)–
Ezra Brown, 2001, "Magic Squares, Finite Planes, and Points of Inflection on Elliptic Curves."
His conclusion:
When you are done, you will be able to arrange the points into [a] 3×3 magic square,
which resembles the one in the book [5] I was reading on elliptic curves….
This result ties together threads from finite geometry, recreational mathematics,
combinatorics, calculus, algebra, and number theory. Quite a feat!
5. Viktor Prasolov and Yuri Solvyev, Elliptic Functions and Elliptic Integrals ,
American Mathematical Society, 1997.
Brown fails to give an important clue to the historical background of this topic —
the word Hessian . (See, however, this word in the book on elliptic functions that he cites.)
Investigation of this word yields a related essay at the graduate-student, or AMS, level–
Igor Dolgachev and Michela Artebani, 2009, "The Hesse Pencil of Plane Cubic Curves ."
From the Dolgachev-Artebani introduction–
In this paper we discuss some old and new results about the widely known Hesse
configuration of 9 points and 12 lines in the projective plane P2(k ): each point lies
on 4 lines and each line contains 3 points, giving an abstract configuration (123, 94).
PlanetMath.org on the Hesse configuration—
A picture of the Hesse configuration–
(See Visualizing GL(2,p), a note from 1985).
Related notes from this journal —
From last November —
|
From the December 2010 American Mathematical Society Notices—
Related material from this journal— Consolation Prize (August 19, 2010) |
From 2006 —
|
Sunday December 10, 2006
“Function defined form, expressed in a pure geometry
– J. G. Ballard on Modernism
“The greatest obstacle to discovery is not ignorance –
— Daniel J. Boorstin, |
Also from 2006 —
|
Sunday November 26, 2006
Rosalind Krauss "If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World , for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete. Or, to take a more up-to-date example…."
"He was looking at the nine engravings and at the circle,
"And it's whispered that soon if we all call the tune
The nine engravings of The Club Dumas
An example of the universal*– or, according to Krauss,
"This is the garden of Apollo, the field of Reason…."
For more on the field of reason, see
A reasonable set of "strange correspondences" Unreason is, of course, more popular. * The ninefold square is perhaps a "concrete universal" in the sense of Hegel: "Two determinations found in all philosophy are the concretion of the Idea and the presence of the spirit in the same; my content must at the same time be something concrete, present. This concrete was termed Reason, and for it the more noble of those men contended with the greatest enthusiasm and warmth. Thought was raised like a standard among the nations, liberty of conviction and of conscience in me. They said to mankind, 'In this sign thou shalt conquer,' for they had before their eyes what had been done in the name of the cross alone, what had been made a matter of faith and law and religion– they saw how the sign of the cross had been degraded."
– Hegel, Lectures on the History of Philosophy ,
"For every kind of vampire, |
And from last October —
|
Friday, October 8, 2010
Starting Out in the Evening This post was suggested by last evening's post on mathematics and narrative and by Michiko Kakutani on Vargas Llosa in this morning's New York Times .
"One must proceed cautiously, for this road— of truth and falsehood in the realm of fiction— is riddled with traps and any enticing oasis is usually a mirage."
– "Is Fiction the Art of Lying?"* by Mario Vargas Llosa,
* The Web version's title has a misprint— |
Comments Off on True Grid (continued)
Tuesday, December 28, 2010
Insane Symmetry
Continued from yesterday's Church Diamond and from Dec. 17's Fare Thee Well —
|
The San Francisco Examiner last year
on New Year's Eve — Entertainment
Discover the modern art of Amish quilts By: Leslie Katz 12/31/09 1:00 AM Arts editor
Quilts made by Amish women in Pennsylvania, Household handicrafts and heirlooms made by American women seen as precursors to modern art is one underlying thesis of “Amish Abstractions: Quilts from the Collection of Faith and Stephen Brown,” a provocative exhibit on view at the de Young Museum through June. Curated by Jill D’Alessandro of the Fine Arts Museums of San Francisco, the show features about 50 full-size and crib quilts made between 1880 and 1940 in Pennsylvania and the Midwest during what experts consider the apex of Amish quilt-making production. Faith and Stephen Brown, Bay Area residents who began collecting quilts in the 1970s after seeing one in a shop window in Chicago and being bowled over by its bold design, say their continued passion for the quilts as art is in part because they’re so reminiscent of paintings by modern masters like Mark Rothko, Josef Albers, Sol LeWitt and Ellsworth Kelly — but the fabric masterpieces came first. “A happy visual coincidence” is how the Browns and D’Alessandro define the connection, pointing to the brilliance in color theory, sophisticated palettes and complex geometry that characterize both the quilts and paintings. “There’s an insane symmetry to these quilts,” says D’Alessandro…. Read more at the San Francisco Examiner . |
The festive nature of the date of the above item, New Year's Eve, suggests Stephen King's
All work and no play makes Jack a dull boy.
and also a (mis)quotation from a photographer's weblog—
"Art, being bartender, is never drunk."
— Quotation from Peter Viereck misattributed to Randall Jarrell in
Art as Bartender and the Golden Gate.
By a different photographer —
See also…
We may imagine the bartender above played by Louis Sullivan.
Comments Off on Insane Symmetry
Wednesday, December 22, 2010
Reflections
Published on November 10, 2009 —
The above book may be regarded as an ironic answer to a question posed here on that date—
|
“Public commentators assumed the air of kindergarten teachers who had to protect their children from thinking certain impermissible and intolerant thoughts.” – David Brooks in the Nov. 10, 2009, New York Times What else is new? |
For related kindergarten thoughts, see Finite Geometry and Physical Space.
For the connection of the kindergarten thoughts to reflections, see A Simple Reflection Group of Order 168.
Comments Off on Reflections
Friday, December 17, 2010
Fare Thee Well
Excerpt from a post of 8 AM May 26, 2006 —
|
A Living Church "The man who lives in contact with what he believes to be a living Church is a man always expecting to meet Plato and Shakespeare to-morrow at breakfast." – G. K. Chesterton
|
A related scene from the opening of Blake Edwards's "S.O.B." —
Comments Off on Fare Thee Well
Friday, December 10, 2010
Cruel Star, Part II
Symmetry, Duality, and Cinema
— Title of a Paris conference held June 17, 2010
From that conference, Edward Frenkel on symmetry and duality—
"Symmetry plays an important role in geometry, number theory, and quantum physics. I will discuss the links between these areas from the vantage point of the Langlands Program. In this context 'duality' means that the same theory, or category, may be described in two radically different ways. This leads to many surprising consequences."
Related material —
See also "Black Swan" in this journal, Ingmar Bergman's production of Yukio Mishima's "Madame de Sade," and Duality and Symmetry, 2001.
This journal on the date of the Paris conference
had a post, "Nighttown," with some remarks about
the duality of darkness and light. Its conclusion—
"By groping toward the light we are made to realize
how deep the darkness is around us."
— Arthur Koestler, The Call Girls: A Tragi-Comedy,
Random House, 1973, page 118
Comments Off on Cruel Star, Part II
Sunday, December 5, 2010
Hanukkah Continues —
Dan Brown Meets
The Exorcist
in…
The 973 Code
Baphomet with Ouroboros Pendant
$140 Code: 973
____________________________________
Meanwhile, our hero…
In this production, Jeff Goldblum is played by
David Ben-Zvi of the University of Texas at Austin
Geometry Research Group —
Comments Off on Hanukkah Continues —
Monday, November 29, 2010
Philosopher’s Stone
Happy Ending
Part I —
Plato's
Tombstone
Part II —
Star and Diamond
United
(See previous post and
a note on design.)
Comments Off on Philosopher’s Stone
Sunday, November 28, 2010
The Embedding
The New York Times Magazine this morning on a seminar on film theory at Columbia University—
"When the seminar reconvened after the break, Schamus said, 'Let’s dive into the Meno,' a dialogue in which Plato and Socrates consider virtue. 'The heart of it is the mathematical proof.' He rose from his seat and went to the whiteboard, where he drew figures and scribbled numbers as he worked through the geometry. 'You can only get the proof visually,' he concluded, stepping back and gazing at it. Plato may be skeptical about the category of the visual, he said, but 'you are confronted with a visual proof that gets you back to the idea embedded in visuality.'"
The Meno Embedding
See also Plato's Code and
Plato Thanks the Academy.
Comments Off on The Embedding
Tuesday, November 23, 2010
Art Object
There is more than one way
to look at a cube.
From Cambridge U. Press on Feb. 20, 2006 —
and from this journal on June 30, 2010 —
In memory of Wu Guanzhong, Chinese artist
who died in Beijing on June 25, 2010 —
See also this journal on Feb. 20, 2006
(the day The Cube was published).
Comments Off on Art Object
Monday, November 8, 2010
Study of O
Today's previous entry discussed a musical offering by Coltrane, with a link to some spiritual background on a mathematician from India who died on October 16, 1983. Here is a pictorial offering, more in the spirit of Bach than of Coltrane, from the day of that death—
Click on the image for some context.
Comments Off on Study of O
