From a post on Trevanian in December 2005 —
"And we may see
the meadow in December,
icy white and crystalline."
"Midnight Sun"
From a post on Trevanian in December 2005 —
"And we may see
the meadow in December,
icy white and crystalline."
"The King turned pale, and shut his notebook hastily."
— Alice in Wonderland , quoted here on December 22.
Book description at Amazon.com, translated by Google —
|
Las matemáticas como herramienta
Mathematics as a tool by Raúl Ibáñez Torres Kindle edition in Spanish, 2023 Although the relationship between mathematics and art can be traced back to ancient times, mainly in geometric and technical aspects, it is with the arrival of the avant-garde and abstract art at the beginning of the 20th century that mathematics takes on greater and different relevance: as a source of inspiration and as a tool for artistic creation. Let us think, for example, of the importance of the fourth dimension for avant-garde movements or, starting with Kandisnky and later Max Bill and concrete art, the vindication of mathematical thinking in artistic creation. An idea that would have a fundamental influence on currents such as constructivism, minimalism, the fluxus movement, conceptual art, systematic art or optical art, among others. Following this approach, this book analyzes, through a variety of examples and activities, how mathematics is present in contemporary art as a creative tool. And it does so through five branches and the study of some of its mathematical topics: geometry (the Pythagorean theorem), topology (the Moebius strip), algebra (algebraic groups and matrices), combinatorics (permutations and combinations) and recreational mathematics (magic and Latin squares). |
From the book ("Cullinane Diamond Theorem" heading and picture of
book's cover added) —
Publisher: Los Libros de La Catarata (October 24, 2023)
Author: Raúl Ibáñez Torres, customarily known as Raúl Ibáñez
(Ibáñez does not mention Cullinane as the author of the above theorem
in his book (except indirectly, quoting Josefine Lyche), but he did credit
him fully in an earlier article, "The Truchet Tiles and the Diamond Puzzle"
(translation by Google).)
About Ibáñez (translated from Amazon.com by Google):
Mathematician, professor of Geometry at the University of the Basque Country
and scientific disseminator. He is part of the Chair of Scientific Culture of the
UPV/EHU and its blog Cuaderno de Cultura Cientifica. He has been a scriptwriter
and presenter of the program “Una de Mates” on the television program Órbita Laika.
He has collaborated since 2005 on the programs Graffiti and La mechanica del caracol
on Radio Euskadi. He has also been a collaborator and co-writer of the documentary
Hilos de tiempo (2020) about the artist Esther Ferrer. For 20 years he directed the
DivulgaMAT portal, Virtual Center for the Dissemination of Mathematics, and was a
member of the dissemination commission of the Royal Spanish Mathematical Society.
Author of several books, including The Secrets of Multiplication (2019) and
The Great Family of Numbers (2021), in the collection Miradas Matemáticas (Catarata).
He has received the V José María Savirón Prize for Scientific Dissemination
(national modality, 2010) and the COSCE Prize for the Dissemination of Science (2011).

"Los embaldosados" means the tilings .
The image referencing Robert M. Pirsig is
from "Classic Romantic," Dec. 19, 2020.

Translated by Google as . . .
The Truchet Tiles and the Diamond Puzzle and
The Art of the Simple Truchet Tile.
About the author:
Raúl Ibáñez is a professor in the Department of Mathematics
at the UPV/EHU and collaborator with the Chair of Scientific Culture.
About his school:
The University of the Basque Country
(Basque: Euskal Herriko Unibertsitatea, EHU ;
Spanish: Universidad del País Vasco, UPV ; UPV/EHU)
is a Spanish public university of the Basque Autonomous Community.
— Wikipedia
For an authority on Japanese art —
Text Tiles*

Compare to and contrast with …
Remarks on art, contemplation. and Puritanism
from a recent Princeton University Press book —
“Lucy Lippard distinguished Asian art
(ego-less and contemplative)
from New York Minimalism
(moralistic and puritanical).”
— Mathematics and Art ,
Princeton U. Press, Fall 2015
* Update of Aug. 24, 2016 — See also Nov. 2, 2014.
Yesterday's post Treasure Hunt, on a Brazilian weblog,
suggests a review of Brazil in this journal. The post
most relevant to yesterday's remarks is from
August 15, 2003, with a link, now broken, to the work
of Brazilian artist Nicole Sigaud* that also uses the
four half-square tiles used in 1704 by Sebastien Truchet
and somewhat later by myself in Diamond Theory
(see a 1977 version).
A more recent link that works:
|
http://vismath9.tripod.com/sigaud/e-index.html ANACOM PROJECT
APPLICATIONS
© 1997 – 2002 Nicole Sigaud |
* Sigaud shares the interests of her fellow Brazilian
whose weblog was the subject of yesterday's
Treasure Hunt.—
"For many years I have dedicated myself to the study
of medieval magic, demonology, Kabbalah, Astrology,
Alchemy, Tarot and divination in general."
— Nicole Sigaud (translated by Google) in a self-profile:
http://www.recantodasletras.com.br/autor.php?id=78359.
I do not share the interest of these authors in such matters,
except as they are reflected in the works of authors like
Charles Williams and Umberto Eco.
Review of an often-cited Leonardo article that is
now available for purchase online…
|
The Tiling Patterns of Sebastien Truchet Authors: Cyril Stanley Smith and Pauline Boucher
Source: Leonardo , Vol. 20, No. 4, Published by: The MIT Press Stable URL: http://www.jstor.org/stable/1578535 . |
Smith and Boucher give a well-illustrated account of
the early history of Truchet tiles, but their further remarks
on the mathematics underlying patterns made with
these tiles (see the diamond theorem* of 1976) are
worthless.
For instance…
Excerpt from pages 383-384—
"A detailed analysis of Truchet's
patterns touches upon the most fundamental
questions of the relation between
mathematical formalism and the structure
of the material world. Separations
between regions differing in density
require that nothing be as important as
something and that large and small cells of
both must coexist. The aggregation of
unitary choice of directional distinction
at interfaces lies at the root of all being
and becoming."
* This result is about Truchet-tile patterns, but the
underlying mathematics was first discovered by
investigating superimposed patterns of half-circles .
See Half-Circle Patterns at finitegeometry.org.
… Chomsky vs. Santa
From a New Yorker weblog yesterday—
"Happy Birthday, Noam Chomsky." by Gary Marcus—
"… two titans facing off, with Chomsky, as ever,
defining the contest"
"Chomsky sees himself, correctly, as continuing
a conversation that goes back to Plato, especially
the Meno dialogue, in which a slave boy is
revealed by Socrates to know truths about
geometry that he hadn’t realized he knew."
See Meno Diamond in this journal. For instance, from
the Feast of Saint Nicholas (Dec. 6th) this year—
The Meno Embedding

For related truths about geometry, see the diamond theorem.
For a related contest of language theory vs. geometry,
see pattern theory (Sept. 11, 16, and 17, 2012).
See esp. the Sept. 11 post, on a Royal Society paper from July 2012
claiming that
"With the results presented here, we have taken the first steps
in decoding the uniquely human fascination with visual patterns,
what Gombrich* termed our ‘sense of order.’ "
The sorts of patterns discussed in the 2012 paper —

"First steps"? The mathematics underlying such patterns
was presented 35 years earlier, in Diamond Theory.
* See Gombrich-Douat in this journal.
The new June/July issue of the AMS Notices
on a recent Paris exhibit of art and mathematics—
Mathématiques, un dépaysement soudain
Exhibit at the Fondation Cartier, Paris
October 21, 2011–March 18, 2012
… maybe walking
into the room was supposed to evoke the kind of
dépaysement for which the exhibition is named
(the word dépaysement refers to the sometimes
disturbing feeling one gets when stepping outside
of one’s usual reference points). I was with
my six-year-old daughter, who quickly gravitated
toward the colorful magnetic tiles on the wall that
visitors could try to fit together. She spent a good
half hour there, eventually joining forces with a
couple of young university students. I would come
and check on her every once in a while and heard
some interesting discussions about whether or not
it was worth looking for patterns to help guide the
placing of the tiles. The fifteen-year age difference
didn’t seem to bother anyone.
The tiles display was one of the two installations
here that offered the visitor a genuine chance to
engage in mathematical activity, to think about
pattern and structure while satisfying an aesthetic
urge to make things fit and grow….
The Notices included no pictures with this review.
A search to find out what sort of tiles were meant
led, quite indirectly, to the following—
The search indicated it is unlikely that these Truchet tiles
were the ones on exhibit.
Nevertheless, the date of the above French weblog post,
1 May 2011, is not without interest in the context of
today's previous post. (That post was written well before
I had seen the new AMS Notices issue online.)

Title of a treatise by Dominique Douat—
"Méthode pour faire une infinité de desseins différens avec des carreaux mi-partis de deux couleurs par une ligne diagonale : ou observations du Père Dominique Doüat Religieux Carmes de la Province de Toulouse sur un mémoire inséré dans l'Histoire de l'Académie Royale des Sciences de Paris l'année 1704, présenté par le Révérend Père Sébastien Truchet religieux du même ordre, Académicien honoraire " (Paris, 1722)
"The earliest (and perhaps the rarest) treatise on the theory of design"
— E. H. Gombrich, 1979, in The Sense of Order
A facsimile version (excerpts, 108 pp., Feb. 5, 2010) of this treatise is available from
http://jacques-andre.fr/ed/ in a 23.1 MB pdf.
Sample page—
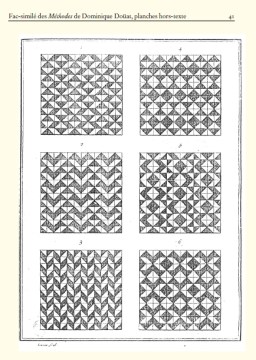
For a treatise on the finite geometry underlying such designs (based on a monograph I wrote in 1976, before I had heard of Douat or his predecessor Truchet), see Diamond Theory.
Misunderstanding
in the Theory of Design
"Whether or not we can follow the theorist in his demonstrations, there is one misunderstanding we must avoid at all cost. We must not confuse the analyses of geometrical symmetries with the mathematics of combination and permutation….
The earliest (and perhaps the rarest) treatise on the theory of design drives home this insight with marvellous precision."
— E. H. Gombrich, 1979, in
The Sense of Order
This is perhaps the stupidest remark I have ever read. The "treatise on the theory of design" that Gombrich refers to is
For some background, see
Truchet & Types:
Tiling Systems and Ornaments, and
Certain of the Truchet/Douat patterns have rather intriguing mathematical properties, sketched in my website Diamond Theory. These properties become clear if and only if we we do what Gombrich declares that we must not do: "confuse the analyses of geometrical symmetries with the mathematics of combination and permutation." (The verb "confuse" should, of course, be replaced by the verb "combine.")
My Personal Thorny Crown
Kirk Varnedoe, 57, art historian and former curator of the Museum of Modern Art, died Thursday, August 14, 2003.
From his New York Times obituary:
" 'He loved life in its most tangible forms, and so for him art was as physical and pleasurable as being knocked down by a wave,' said Adam Gopnik, the writer and a former student of his who collaborated on Mr. Varnedoe's first big show at the Modern, 'High & Low.' 'Art was always material first — it was never, ever bound by a thorny crown of ideas.' "
For some background on the phrase "thorny crown of ideas," see the web page
The phrase "thorny crown of ideas" is also of interest in the light of recent controversy over Mel Gibson's new film, "The Passion."
For details of the controversy, see Christopher Orlet's Aug. 14 essay at Salon.com,
For a real "thorny crown of ideas," consider the following remarks by another art historian:
"Whether or not we can follow the theorist in his demonstrations, there is one misunderstanding we must avoid at all cost. We must not confuse the analyses of geometrical symmetries with the mathematics of combination and permutation….
The earliest (and perhaps the rarest) treatise on the theory of design drives home this insight with marvellous precision."
— E. H. Gombrich, 1979, in
The Sense of Order
This is perhaps the most stupid remark I have ever read. The "treatise on the theory of design" that Gombrich refers to is
This is the title given at the web page
Truchet & Types:
Tiling Systems and Ornaments,
which gives some background.
Certain of the Truchet/Douat patterns have rather intriguing mathematical properties, sketched in my website Diamond Theory. These properties become clear if and only we we do what Gombrich moronically declares that we must not do: "confuse the analyses of geometrical symmetries with the mathematics of combination and permutation." (The verb "confuse" should, of course, be replaced by the verb "combine.")
What does all this have to do with
As jesting Pilate seems to have realized, whenever Jews (or, for that matter, Christians) tell stories, issues of truth may arise. Such issues, as shown by current events in that damned Semitic Hell-on-Earth that used to be referred to as "the Holy Land," can be of life-and-death importance.

Scene from
The Passion
The Roman soldiers may have fashioned a physical crown of thorns, but the Jews are quite capable of fashioning a very uncomfortable crown of, as Gopnik says, "ideas."
Here is an example.
"Ernst Hans Josef Gombrich, who as an author went by the name E. H. Gombrich, was born in Vienna in 1909….
The Gombrich family was Jewish, but his parents felt this had no particular relevance. In later years Mr. Gombrich said that whether someone was Jewish or not was a preoccupation for the Gestapo."
— Michael Kimmelman's obituary for Gombrich in the New York Times. Kimmelman is chief art critic for the New York Times and author of the Times's Aug. 15 Varnedoe obituary.
The web page Understanding cited above contains a link to
Pilate, Truth, and Friday the Thirteenth,
a page combining some religious remarks with a quotation of an extremely patronizing and superficial reference to my own work (and, in passing, to Truchet/Douat patterns).
This reference, and the above-quoted remark by Gombrich, constitute my own modest claim to what the Jew Gopnik jokingly calls a "thorny crown of ideas."
To me it is no joke.
This partly accounts for the rather strained quality of the attempt at humor in a web page I put together yesterday in response to Varnedoe's obituary:
Another reason for the strained quality is my being struck by the synchronicity of reading Varnedoe's obituary shortly after I had done a journal entry related to the death in July of an earlier Museum of Modern Art curator. Like Robert A. Heinlein, I think the God of the Jews is a lousy deity and an even worse father figure. I do, however, believe in synchronicity.
Powered by WordPress