Saturday, July 27, 2024
The Inscape Club
Sunday, February 4, 2024
Doily vs. Inscape: Same Abstract Structure, Different Models

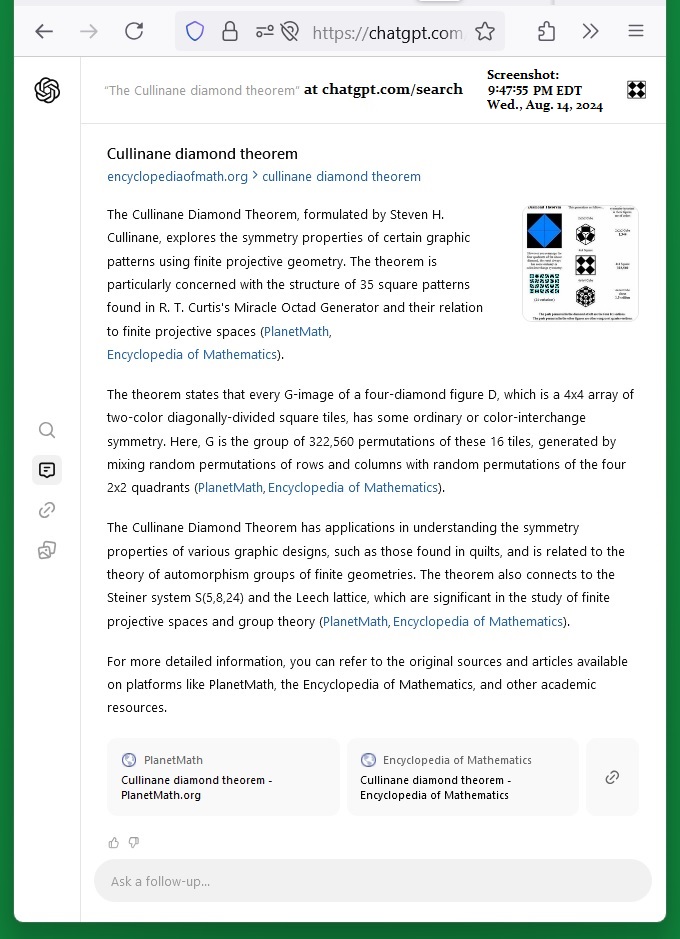
My own term "inscape" names a square incarnation of what is also
known as the "Cremona-Richmond configuration," the "generalized
quadrangle of order (2, 2)," and the "doily." —
Tuesday, July 2, 2019
Depth Psychology Meets Inscape Geometry
An illustration from the previous post may be interpreted
as an attempt to unbokeh an inscape —

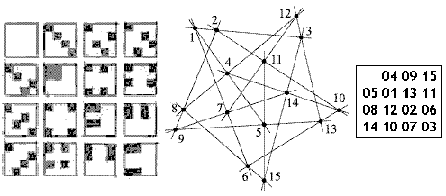
The 15 lines above are Euclidean lines based on pairs within a six-set.
For examples of Galois lines so based, see Six-Set Geometry:

Wednesday, December 12, 2018
An Inscape for Douthat
Some images, and a definition, suggested by my remarks here last night
on Apollo and Ross Douthat's remarks today on "The Return of Paganism" —
In finite geometry and combinatorics,
an inscape is a 4×4 array of square figures,
each figure picturing a subset of the overall 4×4 array:

Related material — the phrase
"Quantum Tesseract Theorem" and …
A. An image from the recent
film "A Wrinkle in Time" —

B. A quote from the 1962 book —
"There's something phoney
in the whole setup, Meg thought.
There is definitely something rotten
in the state of Camazotz."
Wednesday, March 8, 2017
Inscapes
"The particulars of attention,
whether subjective or objective,
are unshackled through form,
and offered as a relational matrix …."
— Kent Johnson in a 1993 essay
|
The 16 Dirac matrices form six anticommuting sets of five matrices each (Arfken 1985, p. 214):
1.
2.
3.
4.
5.
6. SEE ALSO: Pauli Matrices REFERENCES: Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 211-217, 1985. Berestetskii, V. B.; Lifshitz, E. M.; and Pitaevskii, L. P. "Algebra of Dirac Matrices." §22 in Quantum Electrodynamics, 2nd ed. Oxford, England: Pergamon Press, pp. 80-84, 1982. Bethe, H. A. and Salpeter, E. Quantum Mechanics of One- and Two-Electron Atoms. New York: Plenum, pp. 47-48, 1977. Bjorken, J. D. and Drell, S. D. Relativistic Quantum Mechanics. New York: McGraw-Hill, 1964. Dirac, P. A. M. Principles of Quantum Mechanics, 4th ed. Oxford, England: Oxford University Press, 1982. Goldstein, H. Classical Mechanics, 2nd ed. Reading, MA: Addison-Wesley, p. 580, 1980. Good, R. H. Jr. "Properties of Dirac Matrices." Rev. Mod. Phys. 27, 187-211, 1955. Referenced on Wolfram|Alpha: Dirac Matrices CITE THIS AS: Weisstein, Eric W. "Dirac Matrices."
From MathWorld— A Wolfram Web Resource. |
Monday, October 3, 2016
Hudson’s Inscape
Yesterday evening's post Some Old Philosophy from Rome
(a reference, of course, to a Wallace Stevens poem)
had a link to posts now tagged Wittgenstein's Pentagram.
For a sequel to those posts, see posts with the term Inscape ,
a mathematical concept related to a pentagram-like shape.
The inscape concept is also, as shown by R. W. H. T. Hudson
in 1904, related to the square array of points I use to picture
PG(3,2), the projective 3-space over the 2-element field.
Thursday, April 24, 2014
The Inscape of 24
“The more intellectual, less physical, the spell of contemplation
the more complex must be the object, the more close and elaborate
must be the comparison the mind has to keep making between
the whole and the parts, the parts and the whole.”
— The Journals and Papers of Gerard Manley Hopkins ,
edited by Humphry House, 2nd ed. (London: Oxford
University Press, 1959), p. 126, as quoted by Philip A.
Ballinger in The Poem as Sacrament
Related material from All Saints’ Day in 2012:
Sunday, April 7, 2013
Pascal Inscape
Background: Inscapes and The 2-subsets of a 6-set are the points of a PG(3,2).
Related remarks: Classical Geometry in Light of Galois Geometry.
Wednesday, April 2, 2025
Dramarama: The SIX Train
From a post of August 31, 2022 — "Release Dates: The Iceman Goeth" —
Also in May 1986 —
|
86-05-08… A linear complex related to M24 . Anatomy of the polarity pictured in the 86-04-26 note. 86-05-26… The 2-subsets of a 6-set are the points of a PG(3,2).
Beutelspacher's model of the 15 points of PG(3,2) |
More recently, Harrison Ford in the New York subway,
reportedly on Monday, March 31 —

See as well Agent Smith in Brick Space.
Wednesday, August 14, 2024
For Anne Carson
From an essay by Carson in London Review of Books,
Vol. 46 No. 16 · 15 August 2024 —
"Handwriting is a mark from inside me
that I put outside me, often with a view
to showing, telling, communicating.
It carries what Gerard Manley Hopkins
calls ‘the inscape’ out.
(Note: Hopkins meant several different things
by ‘inscape’, which I don’t know enough
about his psyche or his poetics to represent here,
but those Dublin notebooks – wow!)"
For a rather different use of "inscape," see a Log24 search.
Some related mathematics, via a beta version of ChatGPT Search —
Sunday, April 28, 2024
Minding the Gap
A design note from April 24 ten years ago —
A rather different design note from the same date ten years ago —
Wednesday, March 13, 2024
Monday, February 5, 2024
Quantum Kernel Incarnate
The "quantum kernel" of Koen Thas is a version of the incidence
structure — the Cremona-Richmond configuration — discussed
in the previous post, Doily vs. Inscape .
That post's inscape is, as noted there, an incarnation of the
abstract incidence structure. More generally, see incarnation
in this journal . . . In particular, from Michaelmas last year,
Annals of Mathematical Theology.
A somewhat more sophisticated "incarnation" example
related to the "inscape" concept —
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets

See also Numberland in this journal.
Tuesday, October 3, 2023
Tuesday… Belgium.
The inscape in the previous post suggests a review of
work by the Belgian mathematician Koen Thas on what
might be called the "quantum tesseract theorem."
Babes in Tweeland
The New Yorker yesterday on a film director —
"Lest viewers become even briefly comfortable with
the enchantments of his staging and of his actors’
performances, Anderson jolts them alert with
ever more audacious contrivances."
"As you can see, we've had our eye on you
for some time now, Mr. Anderson."
Sunday, June 11, 2023
The Dreaming*
Ask Mark Wahlberg.
* See as well the September 1982 Kate Bush album.
Addendum of 10:50 AM June 11 —
My own concerns in September 1982 were
rather different —
Thursday, May 25, 2023
Woo for Burton (Tara Isabella Burton, that is)
"William Blake's statement in The Marriage of Heaven and Hell
'Eternity is in love with the productions of time' is an adumbration
of the paradoxology of the game of hide-and-seek that Non-duality
is playing with and in celebration of itself in Ia divina commedia of
this night of its dream."
— Joseph Campbell in "The Inner Reaches of Outer Space" (©1986)
Related material from a Log24 search for "inscapes4"—
Wednesday, February 8, 2023
Local-Global lnduced Actions
See "Two Approaches to Local-Global Symmetry"
(this journal, Jan. 19, 2023), which discusses
local group actions on plane and solid graphic
patterns that induce global group actions.
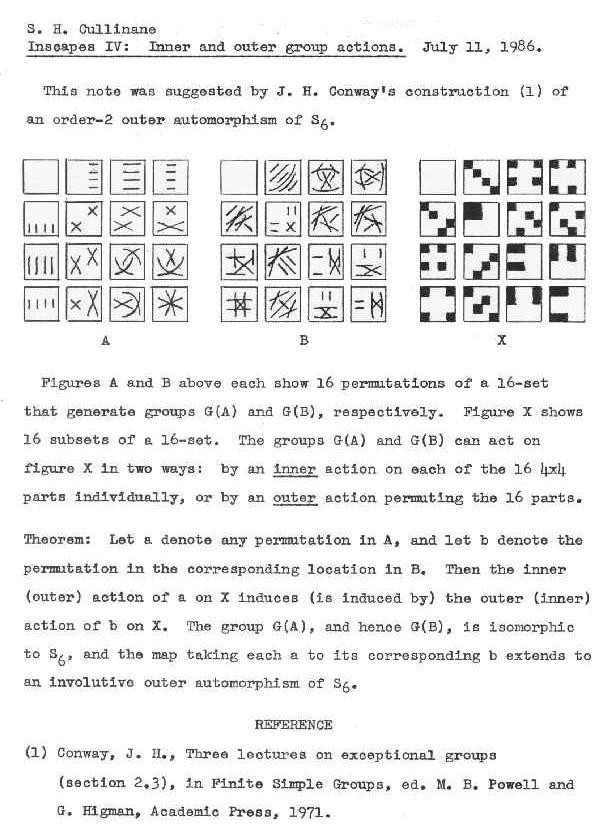
See also local and global group actions of a different sort in
the July 11, 1986, note "Inner and Outer Group Actions."
This post was suggested by some remarks of Barry Mazur,
quoted in the previous post, on " Wittgenstein's 'language game,' "
Grothendieck, global views, local views and "locales."

Further reading on "locales" — Wikipedia, Pointless topology.
The word "locale" in mathematics was apparently* introduced by Isbell —
ISBELL, JOHN R. “ATOMLESS PARTS OF SPACES.”
Mathematica Scandinavica, vol. 31, no. 1, 1972, pp. 5–32.
JSTOR, http://www.jstor.org/stable/24490585.
* According to page 841 of . . .
Johnstone, P. (2001). "Elements of the History of Locale Theory."
Pp. 835–851 in: Aull, C.E., Lowen, R. (eds) Handbook of the
History of General Topology, Vol 3. Springer, Dordrecht.
Thursday, June 9, 2022
Some Like It Hotter
The "inscape.club" of the previous post suggests Princeton's
"Triangle Club." Related material —
From the December 14, 2021, post Notes on Lines —

The triangle, a percussion instrument that was
featured prominently in the Tom Stoppard play
"Every Good Boy Deserves Favour."
Correspondence* Club
The new URL "inscape.club" forwards to …
http://m759.net/wordpress/?s=Inscape .
* For the "correspondences" of the above title, see …
http://m759.net/wordpress/?s=Correspondences+Ninth .
"He was looking at the nine engravings and at the circle,
checking strange correspondences between them."
– The Club Dumas , 1993
Friday, May 6, 2022
Interality and the Bead Game
WIkipedia on the URL suffix ".io" —
"In computer science, "IO" or "I/O" is commonly used
as an abbreviation for input/output, which makes the
.io domain desirable for services that want to be
associated with technology. .io domains are often used
for open source projects, application programming
interfaces ("APIs"), startup companies, browser games,
and other online services."
An association with the Bead Game from a post of April 7, 2018 —
|
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate A less poetic meditation on the above 4x4x4 design cube —
"I saw that in the alternation between front and back, See also a related remark by Lévi-Strauss in 1955:
"…three different readings become possible: |
The recent use by a startup company of the URL "interality.io" suggests
a fourth reading for the 1955 list of Lévi-Strauss — in and out —
i.e., inner and outer group automorphisms — from a 2011 post
on the birthday of T. S. Eliot :
A transformation:
Click on the picture for details.
Wednesday, February 23, 2022
Monday, December 27, 2021
Thursday, October 21, 2021
SIX — The Musical!
From an Instagram post today:
As for SIX — the non-musical —
For further details, see Lost in the Matrix.
Tuesday, March 30, 2021
Focus
Saturday, January 16, 2021
Dark City . . .
“Dark City is an action movie — and like all good sci-fi movies,
it has aliens in it, too. The aliens have the problem that they
do not possess individual identities or souls, and for that reason,
their race is on the brink of extinction. To prevent this from
happening they perform experiments on the inhabitants of the
city to learn the secret of individuality and to eventually acquire it.
The key ingredient is memory.”
— Chapter 13 of SHELL BEACH: The search for the final theory,
by Jesper Møller Grimstrup, published on January 10, 2021.
“She did not ask herself if the Shorter Way was really there,
did not wonder if she was easing into a delusion.
The issue was settled. Here it was.”
— Joe Hill, NOS4A2 (p. 680). William Morrow, April 30, 2013.
A different “shorter way” —
Saturday, October 24, 2020
Grids
Wikipedia on what has been called “the doily” —
“The smallest non-trivial generalized quadrangle
is GQ(2,2), whose representation* has been dubbed
‘the doily’ by Stan Payne in 1973.”
A later publication relates the doily to grids.
From Finite Generalized Quadrangles , by Stanley E. Payne
and J. A. Thas, December 1983, at researchgate.net, pp. 81-82—
“Then the lines … define a 3×3 grid G (i.e. a grid
consisting of 9 points and 6 lines).”
. . . .
“So we have shown that the grid G can completed [sic ]
in a unique way to a grid with 8 lines and 16 points.”
. . . .
“A 4×4 grid defines a linear subspace
of the 2−(64,4,1) design, i.e. a 4×4 grid
together with the affine lines on it is AG(2,4).”
A more graphic approach from this journal —
Click the image for further details.
* This wording implies that GQ(2,2) has a unique
visual representation. It does not. See inscape .
Monday, July 13, 2020
Unpoetic License

The above novel uses extensively the term “inscape.”
The term’s originator, a 19th-century Jesuit poet,
is credited . . . sort of. For other uses of the term,
search for Inscape in this journal. From that search —
A quote from a 1962 novel —
“There’s something phoney
in the whole setup, Meg thought.
There is definitely something rotten
in the state of Camazotz.”
Addendum for the Church of Synchronology —
The Joe Hill novel above was published (in hardcover)
on Walpurgisnacht —April 30, 2013. See also this journal
on that date.
Friday, November 8, 2019
Glitch
The terms glitch and cross-carrier in the previous post
suggest a review —

|
|
For some backstory, see Glitch, Gerard Manley Hopkins, Inscape —
particularly the post A Balliol Star.
Monday, October 14, 2019
Friday, August 16, 2019
Nocciolo
A revision of the above diagram showing
the Galois-addition-table structure —

Related tables from August 10 —

See "Schoolgirl Space Revisited."
Saturday, August 10, 2019
Schoolgirl Space* Revisited:
The Square "Inscape" Model of
the Generalized Quadrangle W(2)
Click image to enlarge.
* The title refers to the role of PG (3,2) in Kirkman's schoolgirl problem.
For some backstory, see my post Anticommuting Dirac Matrices as Skew Lines
and, more generally, posts tagged Dirac and Geometry.
Monday, July 29, 2019
Monday, July 1, 2019
“The Ontological Secret”
The phrase "ontological secret" is from 1927 —
" Beauty is thus 'a flashing of intelligence…
on a matter intelligibly arranged' or, as Maritain
adds in the 1927 edition of Art and Scholasticism ,
it is 'the ontological secret that [things] bear within
them[selves], their spiritual being, their operating
mystery.' "
— John G. Trapani, Jr., "'Radiance': The Metaphysical Foundations
of Maritain's Aesthetics," pp. 11-19 in Beauty, Art, and the Polis ,
ed. by Alice Ramos, publ. by American Maritain Association, 2000.
This 1927 phrase may be the source of McLuhan's 1944
"ontological secret" —

From a search in this journal for "Object of Beauty" —
“She never looked up while her mind rotated the facts,
trying to see them from all sides, trying to piece them
together into theory. All she could think was that she
was flunking an IQ test.”
— Steve Martin, An Object of Beauty
Tuesday, June 25, 2019
Rhetorical Question, Rhetorical Answer
Q —
"What kind of person
bokehs an inscape?"
A —
Robert Gorham Davis:
McLuhan's " 'mosaic' mode of presentation …
rules out discriminations, qualifications,
close reasoning, the structuring of
articulated wholes."
— Robert Gorham Davis on Marshall McLuhan.
See also Articulation in this journal.
Tuesday, June 4, 2019
Inside Out
For fans of Space Fleet and of "reclusive but gifted" programmers—
Monday, March 11, 2019
Overarching Metanarratives
See also "Overarching + Tesseract" in this journal. From the results
of that search, some context for the "inscape" of the previous post —
Sunday, March 10, 2019
Vocabulary for SXSW:
Foursquare, Inscape, Subway
Foursquare —
Inscape —
Subway —
Art installation, "Crystal Cult" by Josefine Lyche, at an Oslo subway station —
See also today's previous post.
Tuesday, February 26, 2019
Annals of Philology
"What kind of person bokehs an inscape?"
— Question adapted from the weblog Barefooted Philologists
An illustration (click to enlarge) —
Tuesday, July 3, 2018
Lost in Quantum Space
Combining concepts from earlier posts today, we have the above title.
A more concise alternative title …
Lost in the Matrix
For some related non -fiction, see posts tagged Dirac and Geometry.
Wednesday, June 20, 2018
Feature
"… what we’re witnessing is not a glitch. It’s a feature…."
— A Boston Globe columnist on June 19.
An image from this journal at the beginning of Bloomsday 2018 —
An encountered feature , from the midnight beginning of June 16 —

Literary Symbolism
"… what we’re witnessing is not a glitch. It’s a feature…."
The glitch encountered on Bloomsday by Agent Smith (who represents
the academic world) is the author of the above page, John P. Anderson.
The feature is the book that Anderson quotes, James Joyce
by Richard Ellmann (first published in 1959, revised in 1982).
Saturday, June 16, 2018
Kummer’s (16, 6) (on 6/16)
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
See too "The Ruler of Reality" in this journal.
Related material —
A more esoteric artifact: The Kummer 166 Configuration . . .
An array of Göpel tetrads appears in the background below.
"As you can see, we've had our eye on you
for some time now, Mr. Anderson."
Wednesday, June 13, 2018
Not So New
"I just found me a brand new box of matches …"
— Soundtrack of the trailer for "Ocean's 8"
"… matchwood, immortal diamond …." —
Click the above definitions for further information.
See as well Blue Diamond in this journal.
Wednesday, November 29, 2017
Definitions

See also Inscape in this journal and, for a related Chapel Hill thesis,
the post Kummer and Dirac.
Tuesday, October 10, 2017
Another 35-Year Wait
The title refers to today's earlier post "The 35-Year Wait."
A check of my activities 35 years ago this fall, in the autumn
of 1982, yields a formula I prefer to the nonsensical, but famous,
"canonical formula" of Claude Lévi-Strauss.

My "inscape" formula, from a note of Sept. 22, 1982 —
S = f ( f ( X ) ) .
Some mathematics from last year related to the 1982 formula —
See also Inscape in this journal and posts tagged Dirac and Geometry.
Tuesday, October 3, 2017
Show Us Your Wall
From Monday morning's post Advanced Study —
"Mathematical research currently relies on
a complex system of mutual trust
based on reputations."
— The late Vladimir Voevodsky,
Institute for Advanced Study, Princeton,
The Institute Letter , Summer 2014, p. 8
Related news from today's online New York Times —
A heading from the above screenshot: "SHOW US YOUR WALL."
This suggests a review of a concept from Galois geometry —
(On the wall — a Galois-geometry inscape .)
Thursday, September 28, 2017
Core
From the New York Times Wire last night —
"Mr. Hefner … styled himself as an emblem
of the sexual revolution."
From a Log24 post on September 23 —
A different emblem related to other remarks in the above Sept. 23 post —
(On the wall — a Galois-geometry inscape .)
Saturday, September 23, 2017
The Turn of the Frame
"With respect to the story's content, the frame thus acts
both as an inclusion of the exterior and as an exclusion
of the interior: it is a perturbation of the outside at the
very core of the story's inside, and as such, it is a blurring
of the very difference between inside and outside."
— Shoshana Felman on a Henry James story, p. 123 in
"Turning the Screw of Interpretation,"
Yale French Studies No. 55/56 (1977), pp. 94-207.
Published by Yale University Press.

See also the previous post and The Galois Tesseract.
Tuesday, June 6, 2017
The Table
John Horgan and James (Jim) McClellan, according to Horgan
in Scientific American on June 1, 2017 —
|
Me: "Jim, you're a scholar! Professor! Esteemed historian of science! And yet you don’t really believe science is capable of producing truth." Jim: "Science is stories we tell about nature. And some stories are better than other stories. And you can compare stories to each other on all kinds of grounds, but you have no access to"— he pauses for dramatic effect— "The Truth. Or any mode of knowing outside of your own story-telling capabilities, which include rationality, experiment, explanatory scope and the whole thing. I would love to have some means of making knowledge about the world that would allow us to say, 'This is really it. There really are goddamn electrons.'" He whacks the table. |
See also posts tagged Dirac and Geometry and Glitch.
Saturday, April 15, 2017
Pip
The title is from a poem in The New Yorker last December —
. . . pip trapped inside, god’s
knucklebone . . . .
The conclusion of yesterday's Google Image Search for Göpel Inscape —
See also "Pray to Apollo" in this journal.
Friday, April 14, 2017
For the Sunshine Girls*
Thursday, December 22, 2016
The Laugh-Hospital
See also, from the above publication date, Hudson's Inscape.
The inscape is illustrated in posts now tagged Laughing Academy.
Monday, November 21, 2016
Inner, Outer
Thursday, November 17, 2016
Rotman and the Outer Automorphism
This is a followup to Tuesday's post on the Nov. 15 American
Mathematical Society (AMS) obituary of Joseph J. Rotman.
Detail of a page in "Notes on Finite Geometry, 1978-1986,"
"An outer automorphism of S6 related to M24" —

Related work of Rotman —
"Outer Automorphisms of S6," by
Gerald Janusz and Joseph Rotman,
The American Mathematical Monthly ,
Vol. 89, No. 6 (Jun. – Jul., 1982), pp. 407-410
Some background —
"In a Nutshell: The Seed," Log24 post of Sept. 4, 2006:
Monday, September 19, 2016
Squaring the Pentagon
The "points" and "lines" of finite geometry are abstract
entities satisfying only whatever incidence requirements
yield non-contradictory and interesting results. In finite
geometry, neither the points nor the lines are required to
lie within any Euclidean (or, for that matter, non-Euclidean)
space.
Models of finite geometries may, however, embed the
points and lines within non -finite geometries in order
to aid visualization.
For instance, the 15 points and 35 lines of PG(3,2) may
be represented by subsets of a 4×4 array of dots, or squares,
located in the Euclidean plane. These "lines" are usually finite
subsets of dots or squares and not* lines of the Euclidean plane.
Example — See "4×4" in this journal.
Some impose on configurations from finite geometry
the rather artificial requirement that both points and lines
must be representable as those of a Euclidean plane.
Example: A Cremona-Richmond pentagon —
A square version of these 15 "points" —

A 1905 square version of these 15 "points"
with digits instead of letters —
See Parametrizing the 4×4 Array
(Log24 post of Sept. 13, 2016).
Update of 8 AM ET Sunday, Sept. 25, 2016 —
For more illustrations, do a Google image search
on "the 2-subsets of a 6-set." (See one such search.)
* But in some models are subsets of the grid lines
that separate squares within an array.
Monday, August 15, 2016
Google as Galatea …
Today Reviews the Concept of "Göpel Inscape ."
Shown below is a condensed version of
Google-as-Galatea's full 11.7 MB image search
based on the two words Göpel inscape .

Monday, April 4, 2016
Noon for London
(A sequel to today's earlier posts Cube for Berlin and Midnight for Paris.)
See London in this journal.
That search yields …
“The more intellectual, less physical,
the spell of contemplation
the more complex must be the object,
the more close and elaborate
must be the comparison
the mind has to keep making
between the whole and the parts,
the parts and the whole.”
— The Journals and Papers of Gerard Manley Hopkins ,
ed. by Humphry House (London: Oxford University Press, 1959),
as quoted by Philip A. Ballinger in The Poem as Sacrament
(From the post The Inscape of 24, April 24, 2014. The 14 blocks in
the design S(3, 4, 8) of today's previous post are analogous to the 759
blocks in the design S(5, 8, 24).)
Wednesday, June 17, 2015
Slow Art, Continued
The title of the previous post, "Slow Art," is a phrase
of the late art critic Robert Hughes.
Example from mathematics:
-
Göpel tetrads as subsets of a 4×4 square in the classic
1905 book Kummer's Quartic Surface by R. W. H. T. Hudson.
These subsets were constructed as helpful schematic diagrams,
without any reference to the concept of finite geometry they
were later to embody.
-
Göpel tetrads (not named as such), again as subsets of
a 4×4 square, that form the 15 isotropic projective lines of the
finite projective 3-space PG(3,2) in a note on finite geometry
from 1986 —
-
Göpel tetrads as these figures of finite geometry in a 1990
foreword to the reissued 1905 book of Hudson:
Click the Barth passage to see it with its surrounding text.
Related material:
Thursday, May 28, 2015
Show
(A sequel to the previous post, Tell )
Inscapes
An illustration (click image for further details) —

Related reading
From my JSTOR shelf —
Click the above image for a related Log24 post, Groups Acting.
Saturday, December 27, 2014
More To Be Done
The Ball-Weiner date above, 5 September 2011,
suggests a review of this journal on that date —
"Think of a DO NOT ENTER pictogram,
a circle with a diagonal slash, a type of ideogram.
It tells you what to do or not do, but not why.
The why is part of a larger context, a bigger picture."
— Customer review at Amazon.com
This passage was quoted here on August 10, 2009.
Also from that date:
The Sept. 5, 2011, Ball-Weiner paper illustrates the
"doily" view of the mathematical structure W(3,2), also
known as GQ(2,2), the Sp(4,2) generalized quadrangle.
(See Fig. 3.1 on page 33, exercise 13 on page 38, and
the answer to that exercise on page 55, illustrated by
Fig. 5.1 on page 56.)
For "another view, hidden yet true," of GQ(2,2),
see Inscape and Symplectic Polarity in this journal.
Wednesday, August 6, 2014
Symplectic Structure*
From Gotay and Isenberg, "The Symplectization of Science,"
Gazette des Mathématiciens 54, 59-79 (1992):
"… what is the origin of the unusual name 'symplectic'? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure 'line complex group' the 'symplectic group.'
… the adjective 'symplectic' means 'plaited together' or 'woven.'
This is wonderfully apt…."

The above symplectic structure** now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Some related passages from the literature:


* The title is a deliberate abuse of language .
For the real definition of "symplectic structure," see (for instance)
"Symplectic Geometry," by Ana Cannas da Silva (article written for
Handbook of Differential Geometry, vol 2.) To establish that the
above figure is indeed symplectic , see the post Zero System of
July 31, 2014.
** See Steven H. Cullinane, Inscapes III, 1986
Saturday, April 13, 2013
Princeton’s Christopher Robin
The title is that of a talk (see video) given by
George Dyson at a Princeton land preservation trust,
reportedly on March 21, 2013. The talk's subtitle was
"Oswald Veblen and the Six-hundred-acre Woods."
Meanwhile…
Thursday, March 21, 2013
|
Related material for those who prefer narrative
to mathematics:
|
Log24 on June 6, 2006:
The Omen :
|
Related material for those who prefer mathematics
to narrative:
What the Omen narrative above and the mathematics of Veblen
have in common is the number 6. Veblen, who came to
Princeton in 1905 and later helped establish the Institute,
wrote extensively on projective geometry. As the British
geometer H. F. Baker pointed out, 6 is a rather important number
in that discipline. For the connection of 6 to the Göpel tetrads
figure above from March 21, see a note from May 1986.
See also last night's Veblen and Young in Light of Galois.
"There is such a thing as a tesseract." — Madeleine L'Engle
Sunday, March 31, 2013
For Baker
Baker, Principles of Geometry, Vol. IV (1925), Title:

Baker, Principles of Geometry, Vol. IV (1925), Frontispiece:

Baker's Vol. IV frontispiece shows "The Figure of fifteen lines
and fifteen points, in space of four dimensions."
Another such figure in a vector space of four dimensions
over the two-element Galois field GF(2):

(Some background grid parts were blanked by an image resizing process.)
Here the "lines" are actually planes in the vector 4-space over GF(2),
but as planes through the origin in that space, they are projective lines .
For some background, see today's previous post and Inscapes.
Update of 9:15 PM March 31—
The following figure relates the above finite-geometry
inscape incidences to those in Baker's frontispiece. Both the inscape
version and that of Baker depict a Cremona-Richmond configuration.

For Pascal
Thursday, March 21, 2013
Geometry of Göpel Tetrads (continued)
An update to Rosenhain and Göpel Tetrads in PG(3,2)
supplies some background from
Notes on Groups and Geometry, 1978-1986,
and from a 2002 AMS Transactions paper.
Friday, December 21, 2012
Analogies*
The Moore correspondence may be regarded
as an analogy between the 35 partitions of an
8-set into two 4-sets and the 35 lines in the
finite projective space PG(3,2).
Closely related to the Moore correspondence
is a correspondence (or analogy) between the
15 2-subsets of a 6-set and the 15 points of PG(3,2).
An analogy between the two above analogies
is supplied by the exceptional outer automorphism of S6.
See…
The 2-subsets of a 6-set are the points of a PG(3,2),
Picturing outer automorphisms of S6, and
A linear complex related to M24.
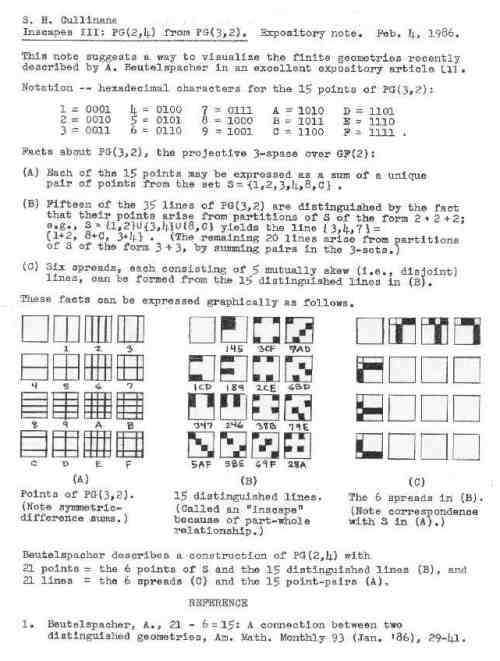
(Background: Inscapes, Inscapes III: PG(2,4) from PG(3,2),
and Picturing the smallest projective 3-space.)
* For some context, see Analogies and
"Smallest Perfect Universe" in this journal.
Monday, November 5, 2012
Sitting Specially
Some webpages at finitegeometry.org discuss
group actions on Sylvester’s duads and synthemes.
Those pages are based on the square model of
PG(3,2) described in the 1980’s by Steven H. Cullinane.
A rival tetrahedral model of PG(3,2) was described
in the 1990’s by Burkard Polster.
Polster’s tetrahedral model appears, notably, in
a Mathematics Magazine article from April 2009—
Click for a pdf of the article.
Related material:
“The Religion of Cubism” (May 9, 2003) and “Art and Lies”
(Nov. 16, 2008).
This post was suggested by following the link in yesterday’s
Sunday School post to High White Noon, and the link from
there to A Study in Art Education, which mentions the date of
Rudolf Arnheim‘s death, June 9, 2007. This journal
on that date—

The Fink-Guy article was announced in a Mathematical
Association of America newsletter dated April 15, 2009.
Those who prefer narrative to mathematics may consult
a Log24 post from a few days earlier, “Where Entertainment is God”
(April 12, 2009), and, for some backstory, The Judas Seat
(February 16, 2007).
Tuesday, February 14, 2012
The Ninth Configuration
The showmanship of Nicki Minaj at Sunday's
Grammy Awards suggested the above title,
that of a novel by the author of The Exorcist .
The Ninth Configuration —
The ninth* in a list of configurations—
"There is a (2d-1)d configuration
known as the Cox configuration."
— MathWorld article on "Configuration"
For further details on the Cox 326 configuration's Levi graph,
a model of the 64 vertices of the six-dimensional hypercube γ6 ,
see Coxeter, "Self-Dual Configurations and Regular Graphs,"
Bull. Amer. Math. Soc. Vol. 56, pages 413-455, 1950.
This contains a discussion of Kummer's 166 as it
relates to γ6 , another form of the 4×4×4 Galois cube.

See also Solomon's Cube.
* Or tenth, if the fleeting reference to 113 configurations is counted as the seventh—
and then the ninth would be a 153 and some related material would be Inscapes.
Friday, October 7, 2011
Enigma Variations
For Yom Kippur

4. Image— Argument for the Existence of Rebecca
Some literary and cinematic background—

"Are you the butterfly… ?"
Monday, September 26, 2011
Inner and Outer
For T.S. Eliot's Birthday
Last night's post "Transformation" was suggested in part
by the title of a Sunday New York Times article on
George Harrison, "Within Him, Without Him," and by
the song title "Within You Without You" in the post
Death and the Apple Tree.
Related material— "Hamlet's Transformation"—
Something have you heard
Of Hamlet’s transformation; so call it,
Sith nor the exterior nor the inward man
Resembles that it was….”
A transformation:
Click on picture for details.
See also, from this year's Feast of the Transfiguration,
Correspondences and Happy Web Day.
For those who prefer the paganism of Yeats to
the Christianity of Eliot, there is the sequel to
"Death and the Apple Tree," "Dancers and the Dance."
Wednesday, September 15, 2010
Fifteen and Other Small Numbers
Today is the birthday of mathematician Jean-Pierre Serre.
Some remarks related to today's day number within the month, "15"—
The Wikipedia article on finite geometry has the following link—
Carnahan, Scott (2007-10-27), "Small finite sets", Secret Blogging Seminar, http://sbseminar.wordpress.com/2007/10/27/small-finite-sets/, notes on a talk by Jean-Pierre Serre on canonical geometric properties of small finite sets.
From Carnahan's notes (October 27, 2007)—
Serre has been giving a series of lectures at Harvard for the last month, on finite groups in number theory. It started off with some ideas revolving around Chebotarev density, and recently moved into fusion (meaning conjugacy classes, not monoidal categories) and mod p representations. In between, he gave a neat self-contained talk about small finite groups, which really meant canonical structures on small finite sets.
He started by writing the numbers 2,3,4,5,6,7,8, indicating the sizes of the sets to be discussed, and then he tackled them in order.
Related material on finite geometry and the indicated small numbers may, with one apparent exception, be found at my own Notes on Finite Geometry.
The apparent exception is "5." See, however, the role played in finite geometry by this number (and by "15") as sketched by Robert Steinberg at Yale in 1967—

See also …
Tuesday, July 6, 2010
Thoreau on Group Theory
"Instead of a million count half a dozen." —Walden
"Of all the symmetric groups, S6 is perhaps the most remarkable."
— Notes 2 (Autumn 2008), apparently by Robert A. Wilson,
for Group Theory, MTH714U
For a connection of MTH714U with Walden, see "Window, continued."
For a connection of "Window" with the remarkable S6, see Inscapes.
For some deeper background, see Wilson's "Exceptional Simplicity."
Saturday, July 3, 2010
Beyond the Limits
"Human perception is a saga of created reality. But we were devising entities beyond the agreed-upon limits of recognition or interpretation…."
– Don DeLillo, Point Omega
Capitalized, the letter omega figures in the theology of two Jesuits, Teilhard de Chardin and Gerard Manley Hopkins. For the former, see a review of DeLillo. For the latter, see James Finn Cotter's Inscape and "Hopkins and Augustine."
The lower-case omega is found in the standard symbolic representation of the Galois field GF(4)—
A representation of GF(4) that goes beyond the standard representation—
Here the four diagonally-divided two-color squares represent the four elements of GF(4).
The graphic properties of these design elements are closely related to the algebraic properties of GF(4).
This is demonstrated by a decomposition theorem used in the proof of the diamond theorem.
To what extent these theorems are part of "a saga of created reality" may be debated.
I prefer the Platonist's "discovered, not created" side of the debate.
Friday, September 26, 2008
Friday September 26, 2008
for T.S. Eliot’s birthday
(Continued from Sept. 22–
“A Rose for Ecclesiastes.”)
From Kibler’s
“Variations on a Theme of
Heisenberg, Pauli, and Weyl,”
July 17, 2008:
“It is to be emphasized
that the 15 operators…
are underlaid by the geometry
of the generalized quadrangle
of order 2…. In this geometry,
the five sets… correspond to
a spread of this quadrangle,
i.e., to a set of 5 pairwise
skew lines….”
— Maurice R. Kibler,
July 17, 2008
For ways to visualize
this quadrangle,
see Inscapes.
|
Related material
A remark of Heisenberg “… die Schönheit… [ist] die
richtige Übereinstimmung der Teile miteinander und mit dem Ganzen.” “Beauty is the proper conformity |
Saturday, July 26, 2008
Saturday July 26, 2008
The Revelation Game
Revisited
| Lotteries on Jung’s birthday, July 26, 2008 |
Pennsylvania (No revelation) |
New York (Revelation) |
| Mid-day (No belief) |
No belief, no revelation 625 6/25 — Quine’s birthday |
Revelation without belief 003 |
| Evening (Belief) |
Belief without revelation 087 1987 — Quine publishes Quiddities |
Belief and revelation 829 |
From Josephine Klein, Jacob’s Ladder: Essays on Experiences of the Ineffable in the Context of Contemporary Psychotherapy, London, Karnac Books, 2003–
Page 14 —
Gerard Manley Hopkins
“Quiddity and haeccity were contentious topics in medieval discussions about the nature of reality, and the poet Gerard Manley Hopkins would have encountered these concepts during his Jesuit training. W. H. Gardner, who edited much of Hopkins’s work, writes that
in 1872, while studying medieval philosophy… Hopkins came across the writing of Duns Scotus, and in that subtle thinker’s Principles of Individuation and Theory of Knowledge he discovered what seemed to be a philosophical corroboration of his own private theory of inscape and instress. [Gardner, Gerard Manley Hopkins: Poems and Prose, Penguin, 1953, p. xxiii]
In this useful introduction to his selection of Hopkins’s work, Gardner writes that Hopkins was always looking for the law or principle that gave an object ‘its delicate and surprising uniqueness.’ This was for Hopkins ‘a fundamental beauty which is the active principle of all true being, the source of all true knowledge and delight.’ Clive Bell called it ‘significant form’; Hopkins called it ‘inscape’– ‘the rich and revealing oneness of the natural object’ (pp. xx-xxiv). In this chapter, I call it quiddity.”
Friday, May 2, 2008
Friday May 2, 2008
A Balliol Star
In memory of
mathematician
Graham Higman of
Balliol College and
Magdalen College,
Oxford,
Jan. 19, 1917 –
April 8, 2008
From a biography of an earlier Balliol student,
Gerard Manley Hopkins (1844-1889):
"In 1867 he won First-Class degrees in Classics
and 'Greats' (a rare 'double-first') and was
considered by Jowett to be the star of Balliol."

Hopkins, a poet who coined the term "inscape," was a member of the Society of Jesus.
According to a biography, Higman was the founder of Oxford's Invariant Society.
From a publication of that society, The Invariant, Issue 15– undated but (according to Issue 16, of 2005) from 1996 (pdf):
|
Taking the square root
of a function by Ian Collier "David Singmaster once gave a talk at the Invariants and afterwards asked this question: What is the square root of the exponential function? In other words, can you define a function f such that for all x, |
Another approach to the expression f(f(x)), by myself in 1982:

For further details,
see Inscapes.
For more about Higman, see an interview in the September 2001 newsletter of the European Mathematical Society (pdf).
of identity: what it is to give
something a name on Monday
and have it respond to
that name on Friday…."
Sunday, August 12, 2007
Sunday August 12, 2007
In the context of quantum information theory, the following structure seems to be of interest–
"… the full two-by-two matrix ring with entries in GF(2), M2(GF(2))– the unique simple non-commutative ring of order 16 featuring six units (invertible elements) and ten zero-divisors."
— "Geometry of Two-Qubits," by Metod Saniga (pdf, 17 pp.), Jan. 25, 2007

This ring is another way of looking at the 16 elements of the affine space A4(GF(2)) over the 2-element field. (Arrange the four coordinates of each element– 1's and 0's– into a square instead of a straight line, and regard the resulting squares as matrices.) (For more on A4(GF(2)), see Finite Relativity and related notes at Finite Geometry of the Square and Cube.) Using the above ring, Saniga constructs a system of 35 objects (not unlike the 35 lines of the finite geometry PG(3,2)) that he calls a "projective line" over the ring. This system of 35 objects has a subconfiguration isomorphic to the (2,2) generalized quadrangle W2 (which occurs naturally as a subconfiguration of PG(3,2)– see Inscapes.)
Saniga concludes:
"We have demonstrated that the basic properties of a system of two interacting spin-1/2 particles are uniquely embodied in the (sub)geometry of a particular projective line, found to be equivalent to the generalized quadrangle of order two. As such systems are the simplest ones exhibiting phenomena like quantum entanglement and quantum non-locality and play, therefore, a crucial role in numerous applications like quantum cryptography, quantum coding, quantum cloning/teleportation and/or quantum computing to mention the most salient ones, our discovery thus
- not only offers a principally new geometrically-underlined insight into their intrinsic nature,
- but also gives their applications a wholly new perspective
- and opens up rather unexpected vistas for an algebraic geometrical modelling of their higher-dimensional counterparts."

the physics of quantum theory.
Friday, June 15, 2007
Friday June 15, 2007
(continued from Dec. 11, 2006):
J. G. Ballard on "the architecture of death":
"… a huge system of German fortifications that included the Siegfried line, submarine pens and huge flak towers that threatened the surrounding land like lines of Teutonic knights. Almost all had survived the war and seemed to be waiting for the next one, left behind by a race of warrior scientists obsessed with geometry and death."
— The Guardian, March 20, 2006
From the previous entry, which provided a lesson in geometry related, if only by synchronicity, to the death of Jewish art theorist Rudolf Arnheim:
"We are going to keep doing this until we get it right."
Here is a lesson related, again by synchronicity, to the death of a Christian art scholar of "uncommon erudition, wit, and grace"– Robert R. Wark of the Huntington Library. Wark died on June 8, a date I think of as the feast day of St. Gerard Manley Hopkins, a Jesuit priest-poet of the nineteenth century.
From a Log24 entry on the date of Wark's death–
Samuel Pepys on a musical performance (Diary, Feb. 27, 1668):
"When the Angel comes down"
"When the Angel Comes Down, and the Soul Departs," a webpage on dance in Bali:
"Dance is also a devotion to the Supreme Being."
"I went to Bali to a remote village by a volcanic mountain…."
The above three quotations were intended to supply some background for a link to an entry on Taymor, on what Taymor has called "skewed mirrors," and on a related mathematical concept named, using a term Hopkins coined, "inscapes."
They might form part of an introductory class in mathematics and art given, like the class of the previous entry, in Purgatory.
Wark, who is now, one imagines, in Paradise, needs no such class. He nevertheless might enjoy listening in.
A guest teacher in
the purgatorial class
on mathematics
and art:


"Is it safe?"
Friday, June 8, 2007
Friday June 8, 2007
Samuel Pepys on a musical performance (Diary, Feb. 27, 1668):
"When the Angel comes down"
"When the Angel Comes Down, and the Soul Departs," a webpage on dance in Bali:
"Dance is also a devotion to the Supreme Being."
"I went to Bali to a remote village by a volcanic mountain…."
"No se puede vivir sin amar."
Log24 on St. Peter's Day, 2004:
"And so to bed."
Tuesday, April 3, 2007
Tuesday April 3, 2007
Our Judeo-Christian
Heritage –
Lottery
Hermeneutics
Part I: Judeo
| The Lottery 12/9/06 | Mid-day | Evening |
| New York | 036
See |
331
See 3/31— “square crystal” and “the symbolism could not have been more perfect.” |
| Pennsylvania | 602
See 6/02— Walter Benjamin |
111
See 1/11— “Related material:
|
Part II: Christian
| The Lottery 4/3/07 | Mid-day | Evening |
| New York | 115
See 1/15— |
017
See |
| Pennsylvania | 604
See |
714
See |
Related material:
It is perhaps relevant to
this Holy Week that the
date 6/04 (2006) above
refers to both the Christian
holy day of Pentecost and
to the day of the
facetious baccalaureate
of the Class of 2006 in
the University Chapel
at Princeton.
For further context for the
Log24 remarks of that same
date, see June 1-15, 2006.
Friday, February 2, 2007
Friday February 2, 2007
The Night Watch
For Catholic Schools Week
(continued from last year)–
Last night's Log24 Xanga
footprints from Poland:
Poland 2/2/07 1:29 AM
/446066083/item.html
2/20/06: The Past Revisited
(with link to online text of
Many Dimensions, by Charles Williams)
Poland 2/2/07 2:38 AM
/426273644/item.html
1/15/06 Inscape
(the mathematical concept, with
square and "star" diagrams)
Poland 2/2/07 3:30 AM
nextdate=2%252f8%252f20…
2/8/05 The Equation
(Russell Crowe as John Nash
with "star" diagram from a
Princeton lecture by Langlands)
Poland 2/2/07 4:31 AM
/524081776/item.html
8/29/06 Hollywood Birthday
(with link to online text of
Plato on the Human Paradox,
by a Fordham Jesuit)
Poland 2/2/07 4:43 AM
/524459252/item.html
8/30/06 Seven
(Harvard, the etymology of the
word "experience," and the
Catholic funeral of a professor's
23-year-old daughter)
Poland 2/2/07 4:56 AM
/409355167/item.html
12/19/05 Quarter to Three (cont.)
(remarks on permutation groups
for the birthday of Helmut Wielandt)
Poland 2/2/07 5:03 AM
/490604390/item.html
5/29/06 For JFK's Birthday
(The Call Girls revisited)
Poland 2/2/07 5:32 AM
/522299668/item.html
8/24/06 Beginnings
(Nasar in The New Yorker and
T. S. Eliot in Log24, both on the 2006
Beijing String Theory conference)
Poland 2/2/07 5:46 AM
/447354678/item.html
2/22/06 In the Details
(Harvard's president resigns,
with accompanying "rosebud")
Sunday, January 7, 2007
Sunday January 7, 2007
Thursday, April 7, 2005 7:26 PM
In the Details
Wallace Stevens,
An Ordinary Evening in New Haven:
Professor Eucalyptus said, "The search
For reality is as momentous as
The search for God." It is the philosopher's search
For an interior made exterior
And the poet's search for the same exterior made
Interior….
… Likewise to say of the evening star,
The most ancient light in the most ancient sky,
That it is wholly an inner light, that it shines
From the sleepy bosom of the real, re-creates,
Searches a possible for its possibleness.
Julie Taymor, "Skewed Mirrors" interview:
"… they were performing for God. Now God can mean whatever you want it to mean. But for me, I understood it so totally. The detail….
They did it from the inside to the outside. And from the outside to the in. And that profoundly moved me then. It was…it was the most important thing that I ever experienced."
Details:
The above may be of interest to students
of iconology — what Dan Brown in
The Da Vinci Code calls "symbology" —
and of redheads.
The artist of Details,
"Brenda Starr" creator
Dale Messick, died on Tuesday,
April 5, 2005, at 98.
For further details on
April 5, see
Art History:
The Pope of Hope

Wednesday, September 6, 2006
Wednesday September 6, 2006
"O God, I could be bounded in a nutshell
and count myself a king of infinite space,
were it not that I have bad dreams."
— Hamlet
Background:
- Monday's "In a Nutshell,"
- Tuesday's "The King of Infinite Space," and
- this morning's "Bad Dreams."
"… Something have you heard
Of Hamlet's transformation; so call it,
Sith nor the exterior nor the inward man
Resembles that it was…."
The transformation:
Click on picture for details.
Related material:
Figures of Speech (June 7, 2006) and
Ursprache Revisited (June 9, 2006).
Monday, September 4, 2006
Monday September 4, 2006
The Seed
"The symmetric group S6 of permutations of 6 objects is the only symmetric group with an outer automorphism….
This outer automorphism can be regarded as the seed from which grow about half of the sporadic simple groups…."
This "seed" may be pictured as
within what Burkard Polster has called "the smallest perfect universe"– PG(3,2), the projective 3-space over the 2-element field.
Related material: yesterday's entry for Sylvester's birthday.
Sunday, September 3, 2006
Sunday September 3, 2006
The following figure from a June 11, 1986, note illustrates Sylvester's "duads" and "synthemes" using the concept of an "inscape" (part B of the figure). As R. T. Curtis and Noam Elkies have explained, the duads and synthemes lead to constructions of many of the sporadic simple groups.
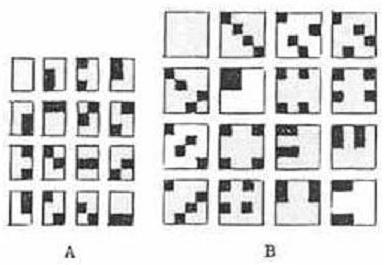
Wednesday, July 26, 2006
Thursday, March 30, 2006
Thursday March 30, 2006
| Galatians 4:4
But when the fulness |
Luke 2:13
And suddenly |
|
Inscape: The Christology and
Poetry of Gerard Manley Hopkins, by James Finn Cotter, University of Pittsburgh Press, 1972. See esp. the references to pleroma 40-48, 51, 65, 70, 81, 85, 92, 93, |
Thursday, January 19, 2006
Thursday January 19, 2006
at Breakfast
Inscapes.
For Shakespeare:
Hopkins on Inscape.
For both:
Click on the picture
for related remarks.
Sunday, January 15, 2006
Sunday January 15, 2006
Inscape
My entry for New Year's Day links to a paper by Robert T. Curtis*
from The Arabian Journal for Science and Engineering
(King Fahd University, Dhahran, Saudi Arabia),
Volume 27, Number 1A, January 2002.
From that paper:
"Combinatorially, an outer automorphism [of S6] can exist because the number of unordered pairs of 6 letters is equal to the number of ways in which 6 letters can be partitioned into three pairs. Which is to say that the two conjugacy classes of odd permutations of order 2 in S6 contain the same number of elements, namely 15. Sylvester… refers to the unordered pairs as duads and the partitions as synthemes. Certain collections of five synthemes… he refers to as synthematic totals or simply totals; each total is stabilized within S6 by a subgroup acting triply transitively on the 6 letters as PGL2(5) acts on the projective line. If we draw a bipartite graph on (15+15) vertices by joining each syntheme to the three duads it contains, we obtain the famous 8-cage (a graph of valence 3 with minimal cycles of length 8)…."
Here is a way of picturing the 8-cage and a related configuration of points and lines:
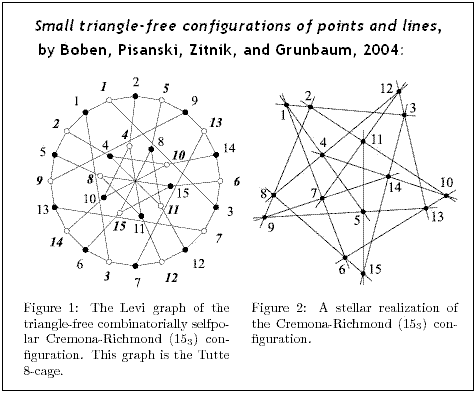
Diamond Theory shows that this structure
can also be modeled by an "inscape"
made up of subsets of a
4×4 square array:

The illustration below shows how the
points and lines of the inscape may
be identified with those of the
Cremona-Richmond configuration.

Friday, November 11, 2005
Friday November 11, 2005
(continued)

Transcendence through spelling:
Richard Gere and Flora Cross
as father and daughter
in "Bee Season."
Words Made Flesh: Code, Culture, Imagination—
… letters create things by the virtue of an algorithm…
— Sefer Yetzirah
Spelling is a sign, Elly. When you win the national bee, we'll know that you are ready to follow in Abulafia's footsteps. Once you're able to let the letters guide you through any word you are given, you will be ready to receive shefa."
In the quiet of the room, the sound of Eliza and her father breathing is everything.
"Do you mean," Eliza whispers, "that I'll be able to talk to God?"

Diamond Theory notes
of Feb. 4, 1986,
of April 26, 1986, and
of May 26, 1986,
Sacerdotal Jargon
(Log24, Dec. 5, 2002),
and 720 in the Book
(Log24, Epiphany 2004).
Thursday, April 7, 2005
Thursday April 7, 2005
In the Details
Wallace Stevens,
An Ordinary Evening in New Haven:
XXII
Professor Eucalyptus said, "The search
For reality is as momentous as
The search for God." It is the philosopher's search
For an interior made exterior
And the poet's search for the same exterior made
Interior….
… Likewise to say of the evening star,
The most ancient light in the most ancient sky,
That it is wholly an inner light, that it shines
From the sleepy bosom of the real, re-creates,
Searches a possible for its possibleness.
Julie Taymor, "Skewed Mirrors" interview:
"… they were performing for God. Now God can mean whatever you want it to mean. But for me, I understood it so totally. The detail….
They did it from the inside to the outside. And from the outside to the in. And that profoundly moved me then. It was…it was the most important thing that I ever experienced."
The above may be of interest to students
of iconology — what Dan Brown in
The Da Vinci Code calls "symbology" —
and of redheads.
The artist of Details,
"Brenda Starr" creator
Dale Messick, died on Tuesday,
April 5, 2005, at 98.
For further details on
April 5, see
Art History:
The Pope of Hope

Saturday, May 1, 2004
Saturday May 1, 2004
Honorable Bird
Tonight at 8:00 PM on BRAVO:
Black Rain
Michael Douglas and Andy Garcia are New York detectives caught up in a gang war in Japan. Masahiro: Ken Takakura.
Masahiro: "Now — music and movies are all America is good for."
From yesterday's entry Library:
"… this is the Idea that is put forward for our response. There is nothing mythological about Christian Trinitarian doctrine: it is analogical. It offers itself freely for meditation and discussion; but it is desirable that we should avoid the bewildered frame of mind of the apocryphal Japanese gentleman who complained:
'Honourable Father, very good;
Honourable Son, very good; but
Honourable Bird
I do not understand at all.' "
|
Music and |
Honorable |
See, too, Inscape (4/22/04), The Proof and the Lie (11/30/03), and Hatched (4/21/04), and recall that the theme of Black Rain is counterfeiting.
For a related meditation on the color black, see Kawabata's The Old Capital, quoted in an entry of Aug. 1, 2003.
Thursday, April 22, 2004
Thursday April 22, 2004
Inscape

Picture said to be of
a Japanese Skylark,
Hibari or Alauda japonica.
Photo: 05/2002, Nagano, Japan.
|
A false definition of “inscape”: Brad Leithauser, New York Review of Books, April 29, 2004: “Not surprisingly, most Hopkins criticism is secular at heart, though without always acknowledging just how distorted—how weirdly misguided— Hopkins himself would find all interpretations of a spiritual life that were drawn purely from the outside. For him, a failure to see how divine promptings informed his shaping internal life—his ‘inscape,’ his own term for it—was to miss everything of his life that mattered.” “By ‘inscape’ he [Hopkins] means the unified complex of characteristics that give each thing its uniqueness and that differentiate it from other things.” A false invocation of the Lord: Brad Leithauser, New York Review of Books, Sept. 26, 2002: “I’d always thought ‘Skylark’ quite appealing, but it wasn’t until I heard Helen Forrest singing it, in a 1942 recording with Harry James and his Orchestra, that it became for me something far more: one of the greatest popular songs anybody ever wrote. With her modest delivery, a voice coaxing and plaintive, Forrest is a Little Girl Lost who always finds herself coming down on exactly the right note—no easy thing with a song of such unexpected chromatic turns. On paper, the Johnny Mercer lyric looks unpromising—antiquated and clunky:
But in Helen Forrest’s performance, ‘Skylark’ turns out to be a perfect blend of pokiness and urgency, folksiness and ethereality—and all so convincing that it isn’t until the song is finished that you step back and say, ‘Good Lord, she’s singing to a bird!’ “ For Hopkins at midnight in the garden of good and evil, a truer invocation:
Friday, December 27, 2002 Saint Hoagy’s Day Today is the feast day of St. Hoagy Carmichael, who was born on the feast day of Cecelia, patron saint of music. This midnight’s site music is “Stardust,” by Carmichael (lyrics by Mitchell Parish). See also “Dead Poets Society” — my entry of Friday, December 13, on the Carmichael song “Skylark” — and the entry “Rhyme Scheme” of later that same day. |
Sunday, September 14, 2003
Sunday September 14, 2003
Skewed Mirrors
Readings on Aesthetics for the
Feast of the Triumph of the Cross
Part I —
Bill Moyers and Julie Taymor
Director Taymor on her own passion play (see previous entry), "Frida":
"We always write stories of tragedies because that's how we reach our human depth. How we get to the other side of it. We look at the cruelty, the darkness and horrific events that happened in our life whether it be a miscarriage or a husband who is not faithful. Then you find this ability to transcend. And that is called the passion, like the passion of Christ. You could call this the passion of Frida Kahlo, in a way."
— 10/25/02 interview with Bill Moyers
|
From transcript MOYERS: What happened to you in Indonesia. TAYMOR: This is probably it for me. This is the story that moves me the most…. I went to Bali to a remote village by a volcanic mountain on the lake. They were having a ceremony that only happens only every 10 years for the young men. I wanted to be alone. I was listening to this music and all of a sudden out of the darkness I could see glints of mirrors and 30 or 40 old men in full warrior costume– there was nobody in this village square. I was alone. They couldn't see me in the shadows. They came out with these spears and they started to dance. They did, I don't know, it felt like an eternity but probably a half hour dance. With these voices coming out of them. And they danced to nobody. Right after that, they and I went oh, my God. The first man came out and they were performing for God. Now God can mean whatever you want it to mean. But for me, I understood it so totally. The detail on the costumes. They didn't care if someone was paying tickets, writing reviews. They didn't care if an audience was watching. They did it from the inside to the outside. And from the outside to the in. And that profoundly moved me then. MOYERS: How did you see the world differently after you were in Indonesia? |
From transcript ….They did it from the inside to the outside. And from the outside to the in. And that profoundly moved me then. It was…it was the most important thing that I ever experienced. … ………………….. MOYERS: Now that you are so popular, now that your work is… TAYMOR: [INAUDIBLE]. MOYERS: No, I'm serious. Now that you're popular, now that your work is celebrated and people are seeking you, do you feel your creativity is threatened by that popularity or liberated by it? TAYMOR: No, I think it's neither one. I don't do things any differently now than I would before. And you think that sometimes perhaps if I get a bigger budget for a movie, then it will just be the same thing… MOYERS: Ruination. Ruination. TAYMOR: No, because LION KING is a combination of high tech and low tech. There are things up on that stage that cost 30 cents, like a little shadow puppet and a lamp, and it couldn't be any better than that. It just couldn't. Sometimes you are forced to become more creative because you have limitations. …. |
|
TAYMOR: Well I understood really the power of art to transform. I think transformation become the main word in my life. Transformation because you don't want to just put a mirror in front of people and say, here, look at yourself. What do you see? You want to have a skewed mirror. You want a mirror that says you didn't know you could see the back of your head. You didn't know that you could amount cubistic see almost all the same aspects at the same time. It allows human beings to step out of their lives and to revisit it and maybe find something different about it. |
It's not about the technology. It's about the power of art to transform. I think transformation becomes the main word in my life, transformation. Because you don't want to just put a mirror in front of people and say, here, look at yourself. What do you see? You want to have a skewed mirror. You want a mirror that says, you didn't know you could see the back of your head. You didn't know that you could…almost cubistic, see all aspects at the same time. And what that does for human beings is it allows them to step out of their lives and to revisit it and maybe find something different about it. |
Part II —
Inside and Outside: Transformation
(Research note, July 11, 1986)

|
Click on the above typewritten note to enlarge.
Summary of
Parts I and II:

See also
Geometry for Jews.
"We're not here to stick a mirror on you. Anybody can do that, We're here to give you a more cubist or skewed mirror, where you get to see yourself with fresh eyes. That's what an artist does. When you paint the Crucifixion, you're not painting an exact reproduction."
— Julie Taymor on "Frida" (AP, 10/22/02)
"She made 'real' an oxymoron,
she made mirrors, she made smoke.
She had a curve ball
that wouldn't quit,
a girlfriend for a joke."
— "Arizona Star," Guy Clark / Rich Alves
Wednesday, February 5, 2003
Wednesday February 5, 2003
Release Date
From Dr. Mac’s Cultural Calendar![]() —
—
- Novelist William S. Burroughs [of the Burroughs adding machine family], author of Naked Lunch, was born on this day in 1914.
- The Charlie Chaplin film “Modern Times“ was released on this day in 1936.
- The adding machine employing depressible keys was patented on this day in 1850.

“It all adds up.” — Saul Bellow, book title
“I see my light come shining
From the west unto the east.
Any day now, any day now,
I shall be released.”
— Bob Dylan
|
|
“The theme of the film is heavily influenced by its release date….” — Jonathan L. Bowen, review of “Modern Times” At left:
|
of 5:10 AM EST Saturday, February 1, 2003.
|
5:10 AM Feb. 1
|
9:00 AM Feb. 1
TIME |
From Robert Morris’s page on Hopkins (see note of Sunday, February 2 (Candlemas)):
“Inscape” was Gerard Manley Hopkins’s term for a special connection between the world of natural events and processes and one’s internal landscape–a frame of mind conveyed in his radical and singular poetry….
This is false, but suggestive.
![]() Checked, corrected, and annotated
Checked, corrected, and annotated
Sunday, February 2, 2003
Sunday February 2, 2003
Steering a Space-Plane
Head White House speechwriter Michael Gerson:
“In the last two weeks, I’ve been returning to Hopkins. Even in the ‘world’s wildfire,’ he asserts that ‘this Jack, joke, poor potsherd, patch, matchwood, immortal diamond,/Is immortal diamond.’ A comfort.”
— Vanity Fair, May 2002, page 162
Yesterday’s note, “Time and Eternity,” supplies the “immortal diamond” part of this meditation. For the “matchwood” part, see the cover of The New York Times Book Review of February 2 (Candlemas), 2003:
|
|
N.Y. Times Feb. 2, 2003
|
See also the Times’s excerpt from Baker‘s first chapter,
about “steering a space-plane.”
For the relationship of Hopkins to Eastern religions,
see “Out of Inscape,” by Robert Morris.
Saturday, July 20, 2002
Saturday July 20, 2002
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.

-Thas-2020.jpg)




























-embedding-in-PG(3,2)-Planat-Saniga.jpg)