"Before time began . . ." — Optimus Prime
Sunday, November 3, 2024
Sunday, October 13, 2024
Cubehenge Review
Song lyric — "Somewhere . . ."
Real estate motto— Location, Location, Location.
Illustration— The fire leap scene from Wicker Man
Comments Off on Cubehenge Review
Monday, September 23, 2024
Design History for a Guy Fawkes Day
Comments Off on Design History for a Guy Fawkes Day
Sunday, September 22, 2024
The Portable Divinity Box
In 1978, Harvard moved a structure known as the Morton Prince House
from Divinity Avenue to Prescott Street, where it occupies the former Hurlbut
Parking Lot, which was the vista from my 1960-61 freshman room.
From the Log24 post "Very Stable Kool-Aid" —
|
A Letter from Timothy Leary, Ph.D., July 17, 1961
Harvard University July 17, 1961
Dr. Thomas S. Szasz Dear Dr. Szasz: Your book arrived several days ago. I've spent eight hours on it and realize the task (and joy) of reading it has just begun. The Myth of Mental Illness is the most important book in the history of psychiatry. I know it is rash and premature to make this earlier judgment. I reserve the right later to revise and perhaps suggest it is the most important book published in the twentieth century. It is great in so many ways–scholarship, clinical insight, political savvy, common sense, historical sweep, human concern– and most of all for its compassionate, shattering honesty. . . . . |
|
Morton Prince, a Boston neurologist, founded the Journal of Abnormal Psychology in 1906 as an outlet especially for those who took a psychogenic view of neurotic disorders. Through experiments with hypnotism, he added appreciably to knowledge of subconscious and coconscious mental processes; The Dissociation of a Personality (Prince, 1905) still ranks as a classic. He early saw that studying normal people in the depth and detail with which one studied patients could make significant contributions to our whole understanding of human nature. Before his death he established and briefly directed the Harvard Psychological Clinic, devising the research environment out of which presently sprang major contributions to the study of personality.
— "Who Was Morton Prince?," by R. W. White, |
See as well Who Was R. W. White?
Comments Off on The Portable Divinity Box
Saturday, September 21, 2024
The Squarespace Gray Cube
"Before time began . . . ." — Optimus Prime
I noticed this favicon on Sept. 18 (see post) at a publisher's webpage.
It turns out that it is not specific to the publisher, but rather to sites
hosted by Squarespace.com. For instance . . .
See also a post on Christmas Day, 2013.
Related material from the Sept. 18 post mentioned above —
Comments Off on The Squarespace Gray Cube
Friday, September 20, 2024
Adapter Becomes Transformer
"When things go bonkers, you have to adapt."
— Chris Hemsworth as Dementus in "Furiosa" (2024)
"Before time began, there was the Cube."
— Optimus Prime in "Transformers" (2007)
Today, an animated Transformers opens, with
Chris Hemsworth as the voice of Optimus Prime.
Also today: The new tag "Cubehenge" in this journal.
Comments Off on Adapter Becomes Transformer
Ring and Stone
|
The Ring and the Stone
From Many Dimensions, a novel "This Holy Thing has been kept in seclusion," Ibrahim answered, "through many centuries, and in all that time none of its keepers have approached or touched it. And since Giles Tumulty stole it men have grasped at it in their own wisdom. But this woman has put her will at its disposal, and between it and her the union may be achieved by which the other Hiddenness is made manifest." "What is the other Hiddenness?" Lord Arglay asked. The Hajji hesitated, then he turned his eyes back to Chloe and seemed to ask a question of her. What answer he saw on the forehead at which he gazed she could not guess, but he spoke then in a low and careful voice. "In the Crown of Suleiman the Wise-the Peace be upon him!-" he said, "there was a Stone, and this Stone was that which is the First Matter of Creation, holy and terrible. But on the hand of the King there was a Ring and in the Ring was another secret, more holy and terrible than the Stone. For within the Ring there was a point of that Light which is the Spirit of Creation, the Adornment of the Unity, the Knowledge of the Loveliness, the Divine Image in the mirror of the worlds just and true. This was the justice and the Wisdom of Suleiman, by which all souls were made manifest to him and all causes rightly determined. Also when within the Holy of Holies in the Temple that the King made he laid his crown upon the Ark and between the wings of the Cherubim, and held his hand over it, the Light of the Ring shone upon the Stone and all things had peace. But when the King erred, building altars to strange gods, he dared no longer let the Light fall upon the Stone; also he put aside the Ring and it is told that Asmodeus sat upon his throne seven years. But I think that perhaps the King himself had not all that time parted from his throne, how closely soever Asmodeus dwelt within his soul. And of the hiding place of the Ring I do not know, nor any of my house; if it is on earth it is very secret. But the Light of it is in the Stone and all the Types of the Stone-and the Power of it is in the soul and body of any who have sought the union with the Stone, so that whoever touches them in anger or hatred or evil desire is subjected to the Light and Power of the Adornment of the Unity. And this I think my nephew did, and this is the cause of his blasting and hurling out." He looked straight at Chloe. "But woe, woe, woe to you," he said, "if from this time forth for ever you forget that you gave your will to the Will of That which is behind the Stone." |
This post was suggested by a Friday the Thirteenth death.
Comments Off on Ring and Stone
Thursday, September 19, 2024
For Case Study Films: Compare and Contrast
From August 10 —
Earlier . . .
Still earlier . . .
Comments Off on For Case Study Films: Compare and Contrast
Verhexung Illustrated
"Die Philosophie ist ein Kampf gegen die Verhexung
unsres Verstandes durch die Mittel unserer Sprache."
— Wittgenstein, Philosophical Investigations (1953), Section 109
Comments Off on Verhexung Illustrated
Wednesday, September 18, 2024
Flatiron Building Timelessness . . .
Directed by Quine, not Hitchcock
Click the "timelessness" quote below for the "Bell, Book and Candle" scene
with Kim Novak and James Stewart atop the Flatiron Building.
"Before time began . . . ." — Optimus Prime
Comments Off on Flatiron Building Timelessness . . .
Directed by Quine, not Hitchcock
Directed by Quine, not Hitchcock
Saturday, September 14, 2024
Notes on a Friday the 13th Death
A passage accessed via the new URL Starbrick.art* —
Thursday, February 25, 2021
|
A related cultural note suggested by the New York Times obituary today
of fashion designer Mary McFadden, who reportedly died yesterday
(a Friday the Thirteenth) and is described by the Times as a late-life
partner of "eightfold-way" physicist Murray Gell-Mann —
* A reference to the 2-column 4-row matrix (a "brick") that underlies
the patterns in the Miracle Octad Generator of R. T. Curtis. The only
connection of this eight-part matrix to Gell-Mann's "Eightfold Way"
that I know of is simply the number 8 itself.
Comments Off on Notes on a Friday the 13th Death
Tuesday, August 27, 2024
For Rubik Worshippers
The above is six-dimensional as an affine space, but only five-dimensional
as a projective space . . . the space PG(5, 2).
As the domain of the smallest model of the Klein correspondence and the
Klein quadric, PG (5,2) is not without mathematical importance.
See Chess Bricks and Ovid.group.
This post was suggested by the date July 6, 2024 in a Warren, PA obituary
and by that date in this journal.
Comments Off on For Rubik Worshippers
Wednesday, May 15, 2024
Transformer Problems (Before the Pretrained Ones)
Comments Off on Transformer Problems (Before the Pretrained Ones)
Thursday, April 18, 2024
Summer Solstice Entertainment, 2019
Related Helen Mirren image . . .
Comments Off on Summer Solstice Entertainment, 2019
Wednesday, April 17, 2024
Thursday, March 21, 2024
Library Note: Chicago Exposition
|
"Chang noted that 'the story starts slowly, for
1. Chang, Margaret A. "The King in the Window". |
Some will prefer exposition more closely related to Chicago.
From a Log24 search for that word . . .
The above phrase "the intersection of storytelling and visual arts"
suggests a review . . .
Some exposition that does not go back thousands of years —
Comments Off on Library Note: Chicago Exposition
The Cross Section
Addendum for Christopher Nolan — Dice and the Eightfold Cube.
Comments Off on The Cross Section
Tuesday, March 19, 2024
Belgian Puzzle Art
From the Belgian artist of the March 25 New Yorker cover —
“There comes a time when the learner has identified
the abstract content of a number of different games
and is practically crying out for some sort of picture
by means of which to represent that which has been
gleaned as the common core of the various activities.”
— Article at Zoltan Dienes’s website
Comments Off on Belgian Puzzle Art
Monday, January 29, 2024
Self as Imago Dei: Hofstadter vs. Valéry
|
Google search result: Saint Anselm College https://www.anselm.edu › Documents › Brown by M Brown · 2014 · Cited by 14 — Thomas insists that the image of God exists most perfectly in the acts of the soul, for the soul is that which is most perfect in us and so best images God, and … 11 pages |
For a Douglas Hofstadter version of the Imago Dei , see the
"Gödel, Escher, Bach" illustration in the Jan. 15 screenshot below —
Tuesday, June 15, 2010
|
Comments Off on Self as Imago Dei: Hofstadter vs. Valéry
Tuesday, October 24, 2023
A Bond with Reality: The Geometry of Cuts
Illustrations of object and gestures
from finitegeometry.org/sc/ —
Object
Gestures
An earlier presentation of the above
seven partitions of the eightfold cube:
|
|
Related mathematics:
|
The use of binary coordinate systems
Natural physical transformations of square or cubical arrays See "The Thing and I." |
and . . .
Related entertainment:
Or Matt Helm by way of a Jedi cube.
Comments Off on A Bond with Reality: The Geometry of Cuts
Sunday, June 4, 2023
The Galois Core
Comments Off on The Galois Core
Wednesday, May 10, 2023
Saving the Appearances
“… I realized that to me,
Gödel and Escher and Bach
were only shadows
cast in different directions by
some central solid essence.
I tried to reconstruct
the central object, and
came up with this book.”
Related images —
Comments Off on Saving the Appearances
Friday, January 27, 2023
The Stone
Here stands the mean, uncomely stone,
’Tis very cheap in price!
The more it is despised by fools,
The more loved by the wise.
— https://jungcurrents.com/
the-story-of-the-stone-at-bollingen
Not so cheap:
Identical copies of the above image are being offered for sale
on three websites as representing a Masonic "cubic stone."
None of the three sites say where, exactly, the image originated.
Image searches for "Masonic stone," "Masonic cube," etc.,
fail to yield any other pictures that look like the above image —
that of a 2x2x2 array of eight identical subcubes.
For purely mathematical — not Masonic — properties of such
an array, see "eightfold cube" in this journal.
The websites offering to sell the questionable image —
|
Getty —
|
|
Alamy —
https://www.alamy.com/
|
|
Photo12 —
https://www.photo12.com/en/image/
No price quoted on public page:
|
Comments Off on The Stone
Monday, November 28, 2022
Groups, Spaces, and Ripoffs
"Rubik's Cube, and the simpler [2x2x2] Super Cube, represent
one form of mathematical and physical reality."
— Solomon W. Golomb, "Rubik's Cube and Quarks:
Twists on the eight corner cells of Rubik's Cube
provide a model for many aspects of quark behavior,"
American Scientist , Vol. 70, No. 3 (May-June 1982), pp. 257-259
From the last (Nov. 14, 2022) of the Log24 posts now tagged Groups and Spaces —
From the first (June 21, 2010) of the Log24 posts now tagged Groups and Spaces —
Comments Off on Groups, Spaces, and Ripoffs
Saturday, October 8, 2022
For Fans of Religious Lunacy … The Firebird Date
("Raiders of the Lost Spell" continues.)
The above flashback to a 2002 post was suggested by a search in
this journal for "Firebird" that yielded, as the only result . . .
http://www.amazon.com/
Witch-Seldom-Firebird-Nancy-Springer/dp/0142302201/.
That URL connects to The Hex Witch of Seldom at Amazon.com.
That book was reportedly published by Firebird on September 16, 2002,
the date of the above Log24 post.
Comments Off on For Fans of Religious Lunacy … The Firebird Date
Saturday, March 26, 2022
Box Geometry: Space, Group, Art (Work in Progress)
| Name Tag | .Space | .Group | .Art |
|---|---|---|---|
| Box4 |
2×2 square representing the four-point finite affine geometry AG(2,2). (Box4.space) |
S4 = AGL(2,2) (Box4.group) |
(Box4.art) |
| Box6 |
3×2 (3-row, 2-column) rectangular array representing the elements of an arbitrary 6-set. |
S6 | |
| Box8 | 2x2x2 cube or 4×2 (4-row, 2-column) array. | S8 or A8 or AGL(3,2) of order 1344, or GL(3,2) of order 168 | |
| Box9 | The 3×3 square. | AGL(2,3) or GL(2,3) | |
| Box12 | The 12 edges of a cube, or a 4×3 array for picturing the actions of the Mathieu group M12. | Symmetries of the cube or elements of the group M12 | |
| Box13 | The 13 symmetry axes of the cube. | Symmetries of the cube. | |
| Box15 |
The 15 points of PG(3,2), the projective geometry of 3 dimensions over the 2-element Galois field. |
Collineations of PG(3,2) | |
| Box16 |
The 16 points of AG(4,2), the affine geometry of 4 dimensions over the 2-element Galois field. |
AGL(4,2), the affine group of |
|
| Box20 | The configuration representing Desargues's theorem. | ||
| Box21 | The 21 points and 21 lines of PG(2,4). | ||
| Box24 | The 24 points of the Steiner system S(5, 8, 24). | ||
| Box25 | A 5×5 array representing PG(2,5). | ||
| Box27 |
The 3-dimensional Galois affine space over the 3-element Galois field GF(3). |
||
| Box28 | The 28 bitangents of a plane quartic curve. | ||
| Box32 |
Pair of 4×4 arrays representing orthogonal Latin squares. |
Used to represent elements of AGL(4,2) |
|
| Box35 |
A 5-row-by-7-column array representing the 35 lines in the finite projective space PG(3,2) |
PGL(3,2), order 20,160 | |
| Box36 | Eurler's 36-officer problem. | ||
| Box45 | The 45 Pascal points of the Pascal configuration. | ||
| Box48 | The 48 elements of the group AGL(2,3). | AGL(2,3). | |
| Box56 |
The 56 three-sets within an 8-set or |
||
| Box60 | The Klein configuration. | ||
| Box64 | Solomon's cube. |
— Steven H. Cullinane, March 26-27, 2022
Comments Off on Box Geometry: Space, Group, Art (Work in Progress)
Friday, February 11, 2022
De Beer’s Consolidated Mine
The misleading image at right above is from the cover of
an edition of Charles Williams's classic 1931 novel
Many Dimensions published in 1993 by Wm. B. Eerdmans.
But seriously . . .
Comments Off on De Beer’s Consolidated Mine
Friday, December 31, 2021
Aesthetics in Academia
Related art — The non-Rubik 3x3x3 cube —
The above structure illustrates the affine space of three dimensions
over the three-element finite (i.e., Galois) field, GF(3). Enthusiasts
of Judith Brown's nihilistic philosophy may note the "radiance" of the
13 axes of symmetry within the "central, structuring" subcube.
I prefer the radiance (in the sense of Aquinas) of the central, structuring
eightfold cube at the center of the affine space of six dimensions over
the two-element field GF(2).
Comments Off on Aesthetics in Academia
Saturday, August 28, 2021
Solomon’s Super* Cube…
Geometry for Jews continues.
The conclusion of Solomon Golomb's
"Rubik's Cube and Quarks,"
American Scientist , May-June 1982 —
Related geometric meditation —
Archimedes at Hiroshima
in posts tagged Aitchison.
* As opposed to Solomon's Cube .
Comments Off on Solomon’s Super* Cube…
Wednesday, January 27, 2021
Adoration of the Cube . . .
Related vocabulary —
See as well the word facet in this journal.
Analogously, one might write . . .
A Hiroshima cube consists of 6 faces ,
each with 4 squares called facets ,
for a total of 24 facets. . . ."
(See Aitchison's Octads , a post of Feb. 19, 2020.)
Click image to enlarge. Background: Posts tagged 'Aitchison.'"
Comments Off on Adoration of the Cube . . .
Sunday, October 11, 2020
The Weisheit Weekend
Comments Off on The Weisheit Weekend
Sunday, September 20, 2020
Epistemological Metaphor
Comments Off on Epistemological Metaphor
Friday, February 7, 2020
Correspondences
The 15 2-subsets of a 6-set correspond to the 15 points of PG(3,2).
(Cullinane, 1986*)
The 35 3-subsets of a 7-set correspond to the 35 lines of PG(3,2).
(Conwell, 1910)
The 56 3-subsets of an 8-set correspond to the 56 spreads of PG(3,2).
(Seidel, 1970)
Each correspondence above may have been investigated earlier than
indicated by the above dates , which are the earliest I know of.
See also Correspondences in this journal.
* The above 1986 construction of PG(3,2) from a 6-set also appeared
in the work of other authors in 1994 and 2002 . . .
-
Gonzalez-Dorrego, Maria R. (Maria del Rosario),
(16,6) Configurations and Geometry of Kummer Surfaces in P3.
American Mathematical Society, Providence, RI, 1994. -
Dolgachev, Igor, and Keum, JongHae,
"Birational Automorphisms of Quartic Hessian Surfaces."
Trans. Amer. Math. Soc. 354 (2002), 3031-3057.
Addendum at 5:09 PM suggested by an obituary today for Stephen Joyce:
See as well the word correspondences in
"James Joyce and the Hermetic Tradition," by William York Tindall
(Journal of the History of Ideas , Jan. 1954).
Comments Off on Correspondences
Wednesday, February 5, 2020
Appropriation Appropriates*
* A weblog motto. See …
http://enowning.blogspot.com/
2007/07/alfred-denkers-dictionary-on-ereignis.html.
That Ereignis post is dated July 3, 2007.
Related material for the Church of Synchronology —
"The deepest strain in a religion is the particular
and particularistic doctrine it asserts at its heart,
in the company of such pronouncements as
‘Thou shalt have no other Gods before me.’
Take the deepest strain of religion away…
and what remains are the surface pieties —
abstractions without substantive bite —
to which everyone will assent
because they are empty, insipid, and safe."
— Stanley Fish, quoted here on July 3, 2007…
The opening date of the film "Transformers."
The opening pronouncement of "Transformers" —
Comments Off on Appropriation Appropriates*
Sunday, December 22, 2019
M24 from the Eightfold Cube
Exercise: Use the Guitart 7-cycles below to relate the 56 triples
in an 8-set (such as the eightfold cube) to the 56 triangles in
a well-known Klein-quartic hyperbolic-plane tiling. Then use
the correspondence of the triples with the 56 spreads of PG(3,2)
to construct M24.
Click image below to download a Guitart PowerPoint presentation.
See as well earlier posts also tagged Triangles, Spreads, Mathieu.
Comments Off on M24 from the Eightfold Cube
Sunday, December 8, 2019
Geometry of 6 and 8
Just as
the finite space PG(3,2) is
the geometry of the 6-set, so is
the finite space PG(5,2)
the geometry of the 8-set.*
Selah.
* Consider, for the 6-set, the 32
(16, modulo complementation)
0-, 2-, 4-, and 6-subsets,
and, for the 8-set, the 128
(64, modulo complementation)
0-, 2-, 4-, 6-, and 8-subsets.
Update of 11:02 AM ET the same day:
See also Eightfold Geometry, a note from 2010.
Comments Off on Geometry of 6 and 8
Monday, November 11, 2019
Time and Chance
The misleading image at right above is from the cover of
an edition of Charles Williams's classic 1931 novel
Many Dimensions published in 1993 by Wm. B. Eerdmans.
Compare and constrast —
Cover of a book by Douglas Hofstadter
Comments Off on Time and Chance
Friday, November 1, 2019
Hofstadter’s Shadowland
“… I realized that to me,
Gödel and Escher and Bach
were only shadows
cast in different directions by
some central solid essence.
I tried to reconstruct
the central object, and
came up with this book.”
See also a search for Gresham Alley.
Comments Off on Hofstadter’s Shadowland
Thursday, October 31, 2019
56 Triangles
The post "Triangles, Spreads, Mathieu" of October 29 has been
updated with an illustration from the Curtis Miracle Octad Generator.
Related material — A search in this journal for "56 Triangles."
Comments Off on 56 Triangles
Tuesday, October 29, 2019
Triangles, Spreads, Mathieu
There are many approaches to constructing the Mathieu
group M24. The exercise below sketches an approach that
may or may not be new.
Exercise:
It is well-known that …
There are 56 triangles in an 8-set.
There are 56 spreads in PG(3,2).
The alternating group An is generated by 3-cycles.
The alternating group A8 is isomorphic to GL(4,2).
Use the above facts, along with the correspondence
described below, to construct M24.
Some background —
A Log24 post of May 19, 2013, cites …
Peter J. Cameron in a 1976 Cambridge U. Press
book — Parallelisms of Complete Designs .
See the proof of Theorem 3A.13 on pp. 59 and 60.
See also a Google search for "56 triangles" "56 spreads" Mathieu.
Update of October 31, 2019 — A related illustration —
Update of November 2, 2019 —
See also p. 284 of Geometry and Combinatorics:
Selected Works of J. J. Seidel (Academic Press, 1991).
That page is from a paper published in 1970.
Update of December 20, 2019 —
Comments Off on Triangles, Spreads, Mathieu
Sunday, September 29, 2019
Spiritual Kin
"The 15 Puzzle and the Magic Cube
are spiritual kin …."
— "Metamagical Themas" column,
Douglas R. Hofstadter, Scientific American ,
Vol. 244, No. 3 (March 1981), pp. 20-39
As are the 15 Schoolgirls and the Eightfold Cube.
Comments Off on Spiritual Kin
Stage Direction: “Comments Off.”
The previous post dealt with "magic" cubes, so called because of the
analogous "magic" squares. Douglas Hofstadter has written about a
different, physical , object, promoted as "the Magic Cube," that Hofstadter
felt embodied "a deep invariant":
Comments Off on Stage Direction: “Comments Off.”
Thursday, June 27, 2019
Group Actions on the 4x4x4 Cube
For affine group actions, see Ex Fano Appollinis (June 24)
and Solomon's Cube.
For one approach to Mathieu group actions on a 24-cube subset
of the 4x4x4 cube, see . . .
For a different sort of Mathieu cube, see Aitchison.
Comments Off on Group Actions on the 4x4x4 Cube
Sunday, June 23, 2019
Verbum Sat
Vinnie Mancuso, in an article now dated December 25, 2018 —
Related art —
Click image for further details.
Comments Off on Verbum Sat
Friday, June 21, 2019
Cube Tales for Solstice Day
Comments Off on Cube Tales for Solstice Day
Thursday, December 6, 2018
The Mathieu Cube of Iain Aitchison
This journal ten years ago today —
Surprise Package
From a talk by a Melbourne mathematician on March 9, 2018 —
The source — Talk II below —
Search Results
|
Related material —
The 56 triangles of the eightfold cube . . .
- in Aitchison's March 9, 2018, talk (slides 32-34), and
- in this journal on July 25, 2008, and later.
Image from Christmas Day 2005.
Comments Off on The Mathieu Cube of Iain Aitchison
Friday, October 19, 2018
Kaleidoscope and Old Lace
See posts now tagged "Kaleidoscope Society" and, more generally,
a search in this journal for "Kaleidoscope."
Related material —
Photo caption in a news story today:
"Father Gary Thomas attends the premiere of Warner Brothers’
'The Rite' at Grauman’s Chinese Theatre, in Los Angeles,
on January 26, 2011. Thomas is holding a special Mass
on Thursday and Saturday [Oct. 18 and 20] to counter
a planned hex on Supreme Court Justice Brett Kavanaugh."
See as well posts tagged "Rubik Exorcism."
Comments Off on Kaleidoscope and Old Lace
Wednesday, August 1, 2018
Abschattungen*
“… I realized that to me, Gödel and Escher and Bach
were only shadows cast in different directions
by some central solid essence.
I tried to reconstruct the central object . . . ."
— Douglas Hofstadter (1979)
See also posts of July 23, 2007, and April 7, 2018.
* Term from a visual-culture lexicon —
Comments Off on Abschattungen*
Sunday, July 15, 2018
Jewish Oases
"… Lincoln Plaza Cinemas, the Juilliard String Quartet,
and the Strand Book Store remained oases
for cultural and intellectual stimulation."
— John S. Friedman in The Forward , Jan. 21, 2018
Read more:
https://forward.com/culture/392483/
how-fred-bass-dan-talbot-robert-mann
-shaped-new-york-culture/
From the Oasis in Steven Spielberg's "Ready Player One" (2018) —
I prefer, from a Log24 search for Flux Capacitor …
From "Raiders of the Lost Images" —
"The cube shape of the lost Mother Box,
also known as the Change Engine,
is shared by the Stone in a novel by
Charles Williams, Many Dimensions .
See the Solomon's Cube webpage."
Comments Off on Jewish Oases
Saturday, March 31, 2018
Illusion
“The greatest obstacle to discovery
is not ignorance —
it is the illusion of knowledge.”
— Daniel J. Boorstin,
Librarian of Congress,
quoted here in 2006.
Related material —
Remarks on Rubik's Cube from June 13, 2014 and . . .
See as well a different Gresham, author of Nightmare Alley ,
and Log24 posts on that book and the film of the same name .
Comments Off on Illusion
Thursday, March 29, 2018
“Before Creation Itself . . .”
From the Diamond Theorem Facebook page —
A question three hours ago at that page —
"Is this Time Cube?"
Notes toward an answer —
And from Six-Set Geometry in this journal . . .
Comments Off on “Before Creation Itself . . .”
Tuesday, March 27, 2018
Compare and Contrast
Related material on automorphism groups —
The "Eightfold Cube" structure shown above with Weyl
competes rather directly with the "Eightfold Way" sculpture
shown above with Bryant. The structure and the sculpture
each illustrate Klein's order-168 simple group.
Perhaps in part because of this competition, fans of the Mathematical
Sciences Research Institute (MSRI, pronounced "Misery') are less likely
to enjoy, and discuss, the eight-cube mathematical structure above
than they are an eight-cube mechanical puzzle like the one below.
Note also the earlier (2006) "Design Cube 2x2x2" webpage
illustrating graphic designs on the eightfold cube. This is visually,
if not mathematically, related to the (2010) "Expert's Cube."
Comments Off on Compare and Contrast
Tuesday, February 27, 2018
Raiders of the Lost Images
On the recent film "Justice League" —
From DC Extended Universe Wiki, "Mother Box" —
"However, during World War I, the British rediscovered
mankind's lost Mother Box. They conducted numerous studies
but were unable to date it due to its age. The Box was then
shelved in an archive, up until the night Superman died,
where it was then sent to Doctor Silas Stone, who
recognized it as a perpetual energy matrix. . . ." [Link added.]
The cube shape of the lost Mother Box, also known as the
Change Engine, is shared by the Stone in a novel by Charles Williams,
Many Dimensions . See the Solomon's Cube webpage.
See too the matrix of Claude Lévi-Strauss in posts tagged
Verwandlungslehre .
Some literary background:
Who speaks in primordial images speaks to us
as with a thousand trumpets, he grips and overpowers,
and at the same time he elevates that which he treats
out of the individual and transitory into the sphere of
the eternal. — C. G. JUNG
"In the conscious use of primordial images—
the archetypes of thought—
one modern novelist stands out as adept and
grand master: Charles Williams.
In The Place of the Lion he incarnates Plato’s
celestial archetypes with hair-raising plausibility.
In Many Dimensions he brings a flock of ordinary
mortals face to face with the stone bearing
the Tetragrammaton, the Divine Name, the sign of Four.
Whether we understand every line of a Williams novel
or not, we feel something deep inside us quicken
as Williams tells the tale.
Here, in The Greater Trumps , he has turned to
one of the prime mysteries of earth . . . ."
— William Lindsay Gresham, Preface (1950) to
Charles Williams's The Greater Trumps (1932)
For fans of what the recent series Westworld called "bulk apperception" —
Comments Off on Raiders of the Lost Images
Tuesday, July 11, 2017
Dialogue from Plato’s Cave
Why was the Cosmic Cube named the Tesseract
in the Marvel movie series? Is there any specific reason
for the name change? According to me, Cosmic Cube
seems a nice and cooler name.
— Asked March 14, 2013, by Dhwaneet Bhatt
At least it wasn't called 'The AllSpark.'
It's not out of the realm of possibility.
— Solemnity, March 14, 2013
Comments Off on Dialogue from Plato’s Cave
Wednesday, April 26, 2017
Again
See also "Cornerstone" in this journal and …
A sidebar from a Google search today —
This suggests a review of posts now tagged Obelisk,
which include …
Comments Off on Again
Saturday, February 18, 2017
Verbum
The Log24 version (Nov. 9, 2005, and later posts) —
|
VERBUM
|
See also related material in the previous post, Transformers.
Comments Off on Verbum
Thursday, June 30, 2016
Rubik vs. Galois: Preconception vs. Pre-conception
From Psychoanalytic Aesthetics: The British School ,
by Nicola Glover, Chapter 4 —
|
In his last theoretical book, Attention and Interpretation (1970), Bion has clearly cast off the mathematical and scientific scaffolding of his earlier writings and moved into the aesthetic and mystical domain. He builds upon the central role of aesthetic intuition and the Keats's notion of the 'Language of Achievement', which
… includes language that is both Bion distinguishes it from the kind of language which is a substitute for thought and action, a blocking of achievement which is lies [sic ] in the realm of 'preconception' – mindlessness as opposed to mindfulness. The articulation of this language is possible only through love and gratitude; the forces of envy and greed are inimical to it.. This language is expressed only by one who has cast off the 'bondage of memory and desire'. He advised analysts (and this has caused a certain amount of controversy) to free themselves from the tyranny of the past and the future; for Bion believed that in order to make deep contact with the patient's unconscious the analyst must rid himself of all preconceptions about his patient – this superhuman task means abandoning even the desire to cure . The analyst should suspend memories of past experiences with his patient which could act as restricting the evolution of truth. The task of the analyst is to patiently 'wait for a pattern to emerge'. For as T.S. Eliot recognised in Four Quartets , 'only by the form, the pattern / Can words or music reach/ The stillness'.30. The poet also understood that 'knowledge' (in Bion's sense of it designating a 'preconception' which blocks thought, as opposed to his designation of a 'pre -conception' which awaits its sensory realisation), 'imposes a pattern and falsifies'
For the pattern is new in every moment The analyst, by freeing himself from the 'enchainment to past and future', casts off the arbitrary pattern and waits for new aesthetic form to emerge, which will (it is hoped) transform the content of the analytic encounter. 29. Attention and Interpretation (Tavistock, 1970), p. 125 30. Collected Poems (Faber, 1985), p. 194. 31. Ibid., p. 199. |
See also the previous posts now tagged Bion.
Preconception as mindlessness is illustrated by Rubik's cube, and
"pre -conception" as mindfulness is illustrated by n×n×n Froebel cubes
for n= 1, 2, 3, 4.
Suitably coordinatized, the Froebel cubes become Galois cubes,
and illustrate a new approach to the mathematics of space .
Comments Off on Rubik vs. Galois: Preconception vs. Pre-conception
Sunday, June 26, 2016
Common Core versus Central Structure
Rubik's Cube Core Assembly — Swarthmore Cube Project, 2008 —
"Children of the Common Core" —
There is also a central structure within Solomon's Cube —
For a more elaborate entertainment along these lines, see the recent film
"Midnight Special" —
Comments Off on Common Core versus Central Structure
Monday, April 25, 2016
Peirce’s Accounts of the Universe
Compare and contrast Peirce's seven systems of metaphysics with
the seven projective points in a post of March 1, 2010 —
From my commentary on Carter's question —
Comments Off on Peirce’s Accounts of the Universe
Monday, April 4, 2016
Cube for Berlin
Foreword by Sir Michael Atiyah —
"Poincaré said that science is no more a collection of facts
than a house is a collection of bricks. The facts have to be
ordered or structured, they have to fit a theory, a construct
(often mathematical) in the human mind. . . .
… Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to
explain how art fits into our subject and what we mean by beauty.
In attempting to bridge this divide I have always found that
architecture is the best of the arts to compare with mathematics.
The analogy between the two subjects is not hard to describe
and enables abstract ideas to be exemplified by bricks and mortar,
in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics"
in the AMS Notices , January 2010
Judy Bass, Los Angeles Times , March 12, 1989 —
"Like Rubik's Cube, The Eight demands to be pondered."
As does a figure from 1984, Cullinane's Cube —
For natural group actions on the Cullinane cube,
see "The Eightfold Cube" and
"A Simple Reflection Group of Order 168."
See also the recent post Cube Bricks 1984 —
Related remark from the literature —
Note that only the static structure is described by Felsner, not the
168 group actions discussed by Cullinane. For remarks on such
group actions in the literature, see "Cube Space, 1984-2003."
(From Anatomy of a Cube, Sept. 18, 2011.)
Comments Off on Cube for Berlin
Sunday, March 27, 2016
Friday, November 13, 2015
A Connection between the 16 Dirac Matrices and the Large Mathieu Group
Note that the six anticommuting sets of Dirac matrices listed by Arfken
correspond exactly to the six spreads in the above complex of 15 projective
lines of PG(3,2) fixed under a symplectic polarity (the diamond theorem
correlation ). As I noted in 1986, this correlation underlies the Miracle
Octad Generator of R. T. Curtis, hence also the large Mathieu group.
References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Related material:
The 6-set in my 1986 note above also appears in a 1996 paper on
the sixteen Dirac matrices by David M. Goodmanson —
Background reading:
Ron Shaw on finite geometry, Clifford algebras, and Dirac groups
(undated compilation of publications from roughly 1994-1995)—
Comments Off on A Connection between the 16 Dirac Matrices and the Large Mathieu Group
Sunday, August 2, 2015
Symplectic
See "Symplectic" in this journal. Some illustrations —
Midrash —
"Adorned with cryptic stones and sliding shines,
An immaculate personage in nothingness,
With the whole spirit sparkling in its cloth,
Generations of the imagination piled
In the manner of its stitchings, of its thread,
In the weaving round the wonder of its need,
And the first flowers upon it, an alphabet
By which to spell out holy doom and end,
A bee for the remembering of happiness."
— Wallace Stevens, "The Owl in the Sarcophagus"
Comments Off on Symplectic
Saturday, October 18, 2014
Educational Series
Barron's Educational Series (click to enlarge):
The Tablet of Ahkmenrah:
"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
Another educational series (this journal):
Art theorist Rosalind Krauss and The Ninefold Square
Comments Off on Educational Series
Tuesday, July 8, 2014
Toward Freedom
A search for "Dark Fields of the Republic,"
an F. Scott Fitzgerald phrase mentioned in
the previous post, yields a book by that title.
"When does a life bend toward freedom?
grasp its direction?"
— Adrienne Rich on page 275 of
Later Poems Selected and New: 1971-2012
The book's author, Adrienne Rich, died at 82 on
March 27, 2012. See that date in this journal.
See also the following:
|
The Diamond Cutters by Adrienne Rich (1955)
However legendary,
Now, you intelligence
Be serious, because
Be hard of heart, because
Be proud, when you have set |
Comments Off on Toward Freedom
Friday, June 13, 2014
It’s 10 PM
"The wind of change is blowing throughout the continent.
Whether we like it or not, this growth of national consciousness
is a political fact."— Prime Minister Harold Macmillan,
South Africa, 1960
"Lord knows when the cold wind blows
it'll turn your head around." — James Taylor
From a Log24 post of August 27, 2011:
For related remarks on "national consciousness," see Frantz Fanon.
Comments Off on It’s 10 PM
Monday, May 19, 2014
Cube Space
A sequel to this afternoon's Rubik Quote:
"The Cube was born in 1974 as a teaching tool
to help me and my students better understand
space and 3D. The Cube challenged us to find
order in chaos."
— Professor Ernő Rubik at Chrome Cube Lab
(Click image below to enlarge.)
Comments Off on Cube Space
Rubik Quote
“The Cube was born in 1974 as a teaching tool
to help me and my students better understand
space and 3D. The Cube challenged us to find
order in chaos."
— Professor Ernő Rubik at Chrome Cube Lab
For a Chinese approach to order and chaos,
see I Ching Cube in this journal.
Comments Off on Rubik Quote
Sunday, April 27, 2014
Sunday School
Galois and Abel vs. Rubik
"Abel was done to death by poverty, Galois by stupidity.
In all the history of science there is no completer example
of the triumph of crass stupidity…."
— Eric Temple Bell, Men of Mathematics
Gray Space (Continued)
… For The Church of Plan 9.
Comments Off on Sunday School
Saturday, April 26, 2014
For Two Artists of Norway
See also LYNX 760 , Rubik vs. Abel, and Toying.
Comments Off on For Two Artists of Norway
Wednesday, December 25, 2013
Rotating the Facts
"She never looked up while her mind rotated the facts,
trying to see them from all sides, trying to piece them
together into theory. All she could think was that she
was flunking an IQ test."
— Steve Martin, An Object of Beauty
"So you should not feel so all alone…"
— Adapted song lyric
Comments Off on Rotating the Facts
Thursday, June 20, 2013
ART WARS: Chesterton Thursday
The New York Times philosophy column "The Stone"
last evening had an essay on art by a sarcastic anarchist,
one Crispin Sartwell—
"… whole generations of art lovers have been
trained in modernist dogma, and arts institutions’
access to various forms of state or foundation
support depend on it completely. One goes to
the museum to gasp at stunning works of
incomparable, super-human genius by beings
who are infinitely more exalted and important
than the mere humans staring at their paintings.
That’s why ordinary people staring at a Picasso
(allegedly) experience a kind of transcendence
or re-articulation of their lives and world."
Cubism Re-Articulated:
Click image for some backstory.
(IMAGE: Walter Gropius and Froebel's Third Gift,
from a Google image search today)
Background: Cubism in this journal and
Pilate Goes to Kindergarten.
Related material: Chesterton + Thursday in this journal.
Comments Off on ART WARS: Chesterton Thursday
Saturday, May 11, 2013
Core
Promotional description of a new book:
"Like Gödel, Escher, Bach before it, Surfaces and Essences will profoundly enrich our understanding of our own minds. By plunging the reader into an extraordinary variety of colorful situations involving language, thought, and memory, by revealing bit by bit the constantly churning cognitive mechanisms normally completely hidden from view, and by discovering in them one central, invariant core— the incessant, unconscious quest for strong analogical links to past experiences— this book puts forth a radical and deeply surprising new vision of the act of thinking."
"Like Gödel, Escher, Bach before it…."
Or like Metamagical Themas .
Rubik core:
Non- Rubik cores:
|
Of the odd nxnxn cube:
|
Of the even nxnxn cube:
|
Related material: The Eightfold Cube and…
"A core component in the construction
is a 3-dimensional vector space V over F2 ."
— Page 29 of "A twist in the M24 moonshine story,"
by Anne Taormina and Katrin Wendland.
(Submitted to the arXiv on 13 Mar 2013.)
Comments Off on Core
Monday, November 5, 2012
Design Cubes
Continued from April 2, 2012.
Some predecessors of the Cullinane design cubes of 1984
that lack the Cullinane cubes' symmetry properties—
Kohs cubes (see 1920 article)
Wechsler cubes (see Wechsler in this journal), and
Horowitz cubes (see links below).
Comments Off on Design Cubes
Thursday, July 26, 2012
Solomon’s Seal
(Mathematics and Narrative, continued)
Narrative—
The Ring and The Stone from yesterday's post, and…
"In Medieval Jewish, Christian and Islamic legends,
the Seal of Solomon was a magical signet ring
said to have been possessed by King Solomon…."
— Wikipedia article, Seal of Solomon
Mathematics—
A fact related to the mathematical
"Solomon's seal" described above by Bell:
The reference to Edge is as follows—
[3] Edge, W. L., Quadrics over GF(2) and
their relevance for the cubic surface group,
Canadian J. Maths. 11 (1959) ….
(This reference relates Hirschfeld's remarks
quoted above to the 64-point affine space
illustrated below (via the associated
63-point projective space PG (5, 2)).
As for the narrative's Stone…
See Solomon's Cube.
Comments Off on Solomon’s Seal
Sunday, January 22, 2012
Pound Sign
Comments Off on Pound Sign
Saturday, January 14, 2012
Damnation Morning*
The following is adapted from a 2011 post—
* The title, that of a Fritz Leiber story, is suggested by
the above picture of the symmetry axes of the square.
Click "Continued" above for further details. See also
last Wednesday's Cuber.
Comments Off on Damnation Morning*
Wednesday, January 11, 2012
Cuber
"Examples galore of this feeling must have arisen in the minds of the people who extended the Magic Cube concept to other polyhedra, other dimensions, other ways of slicing. And once you have made or acquired a new 'cube'… you will want to know how to export a known algorithm , broken up into its fundamental operators , from a familiar cube. What is the essence of each operator? One senses a deep invariant lying somehow 'down underneath' it all, something that one can’t quite verbalize but that one recognizes so clearly and unmistakably in each new example, even though that example might violate some feature one had thought necessary up to that very moment. In fact, sometimes that violation is what makes you sure you’re seeing the same thing , because it reveals slippabilities you hadn’t sensed up till that time….
… example: There is clearly only one sensible 4 × 4 × 4 Magic Cube. It is the answer; it simply has the right spirit ."
— Douglas R. Hofstadter, 1985, Metamagical Themas: Questing for the Essence of Mind and Pattern (Kindle edition, locations 11557-11572)
See also Many Dimensions in this journal and Solomon's Cube.
Comments Off on Cuber
Saturday, November 5, 2011
Shadows
Between the idea
And the reality
Between the motion
And the act
Falls the Shadow
— T. S. Eliot, "The Hollow Men"
A passage quoted here on this date in 2005—
Douglas Hofstadter on his magnum opus:
“… I realized that to me,
Gödel and Escher and Bach
were only shadows
cast in different directions
by some central solid essence."
This refers to Hofstadter's cover image:
Also from this date in 2005:
Midrash: Hearts of Darkness.
Comments Off on Shadows
Sunday, September 18, 2011
Anatomy of a Cube
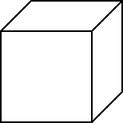
R.D. Carmichael's seminal 1931 paper on tactical configurations suggests
a search for later material relating such configurations to block designs.
Such a search yields the following—
"… it seems that the relationship between
BIB [balanced incomplete block ] designs
and tactical configurations, and in particular,
the Steiner system, has been overlooked."
— D. A. Sprott, U. of Toronto, 1955
The figure by Cullinane included above shows a way to visualize Sprott's remarks.
For the group actions described by Cullinane, see "The Eightfold Cube" and
"A Simple Reflection Group of Order 168."
Update of 7:42 PM Sept. 18, 2011—
From a Summer 2011 course on discrete structures at a Berlin website—
A different illustration of the eightfold cube as the Steiner system S(3, 4, 8)—
Note that only the static structure is described by Felsner, not the
168 group actions discussed (as above) by Cullinane. For remarks on
such group actions in the literature, see "Cube Space, 1984-2003."
Comments Off on Anatomy of a Cube
Friday, September 9, 2011
Galois vs. Rubik
(Continued from Abel Prize, August 26)
The situation is rather different when the
underlying Galois field has two rather than
three elements… See Galois Geometry.
The coffee scene from "Bleu"
Related material from this journal:
The Dream of
the Expanded Field
Comments Off on Galois vs. Rubik
Monday, August 29, 2011
Many = Six.
A comment today on yesterday's New York Times philosophy column "The Stone"
notes that "Augustine… incorporated Greek ideas of perfection into Christianity."
Yesterday's post here for the Feast of St. Augustine discussed the 2×2×2 cube.
Today's Augustine comment in the Times reflects (through a glass darkly)
a Log24 post from Augustine's Day, 2006, that discusses the larger 4×4×4 cube.
For related material, those who prefer narrative to philosophy may consult
Charles Williams's 1931 novel Many Dimensions . Those who prefer mathematics
to either may consult an interpretation in which Many = Six.
Click image for some background.
Comments Off on Many = Six.
Sunday, August 28, 2011
The Cosmic Part
Yesterday's midday post, borrowing a phrase from the theology of Marvel Comics,
offered Rubik's mechanical contrivance as a rather absurd "Cosmic Cube."
A simpler candidate for the "Cube" part of that phrase:
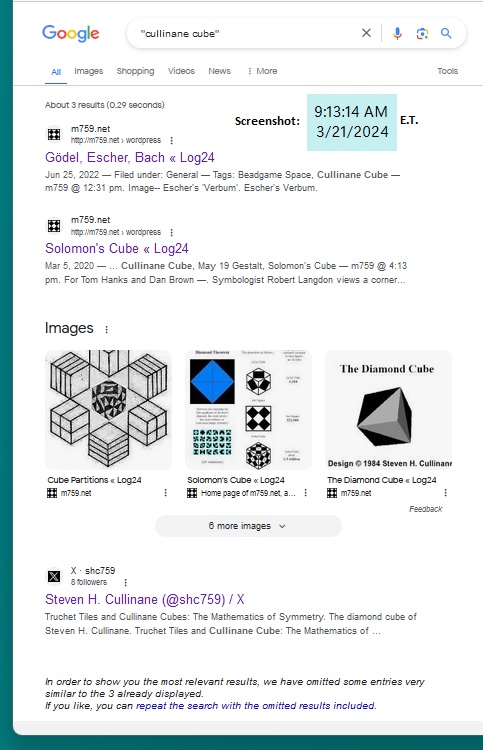
The Eightfold Cube
As noted elsewhere, a simple reflection group* of order 168 acts naturally on this structure.
"Because of their truly fundamental role in mathematics,
even the simplest diagrams concerning finite reflection groups
(or finite mirror systems, or root systems—
the languages are equivalent) have interpretations
of cosmological proportions."
— Alexandre V. Borovik in "Coxeter Theory: The Cognitive Aspects"
Borovik has a such a diagram—
The planes in Borovik's figure are those separating the parts of the eightfold cube above.
In Coxeter theory, these are Euclidean hyperplanes. In the eightfold cube, they represent three of seven projective points that are permuted by the above group of order 168.
In light of Borovik's remarks, the eightfold cube might serve to illustrate the "Cosmic" part of the Marvel Comics phrase.
For some related theological remarks, see Cube Trinity in this journal.
Happy St. Augustine's Day.
* I.e., one generated by reflections : group actions that fix a hyperplane pointwise. In the eightfold cube, viewed as a vector space of 3 dimensions over the 2-element Galois field, these hyperplanes are certain sets of four subcubes.
Comments Off on The Cosmic Part
Saturday, August 27, 2011
Cosmic Cube*
Prequel — (Click to enlarge)
Background —
See also Rubik in this journal.
* For the title, see Groups Acting.
Comments Off on Cosmic Cube*
Tuesday, June 28, 2011
ART WARS continued
See the signature link in last night's post for a representation of Madison Avenue.
For a representation by Madison Avenue, see today's New York Times—
"As a movement Pop Art came and went in a flash, but it was the kind of flash that left everything changed. The art public was now a different public— larger, to be sure, but less serious, less introspective, less willing or able to distinguish between achievement and its trashy simulacrum. Moreover, everything connected with the life of art— everything, anyway, that might have been expected to offer some resistance to this wholesale vulgarization and demoralization— was now cheapened and corrupted. The museums began their rapid descent into show biz and the retail trade. Their exhibitions were now mounted like Broadway shows, complete with set designers and lighting consultants, and their directors pressed into service as hucksters, promoting their wares in radio and television spots and selling their facilities for cocktail parties and other entertainments, while their so-called education programs likewise degenerated into sundry forms of entertainment and promotion. The critics were co-opted, the art magazines commercialized, and the academy, which had once taken a certain pride in remaining aloof from the blandishments of the cultural marketplace, now proved eager to join the crowd— for there was no longer any standard in the name of which a sellout could be rejected. When the boundary separating art and fashion was breached, so was the dividing line between high art and popular culture, and upon all those institutions and professions which had been painstakingly created to preserve high art from the corruptions of popular culture. The effect was devastating. Some surrendered their standards with greater alacrity than others, but the drift was unmistakable and all in the same direction— and the momentum has only accelerated with the passage of time."
— Hilton Kramer, The Triumph of Modernism: The Art World, 1985-2005 , publ. by Ivan R. Dee on Oct. 26, 2006, pp. 146-147
Related material— Rubik in this journal, Exorcist in this journal, and For the Class of '11.
Comments Off on ART WARS continued
Monday, June 27, 2011
Galois Cube Revisited
The 3×3×3 Galois Cube
This cube, unlike Rubik's, is a
purely mathematical structure.
Its properties may be compared
with those of the order-2 Galois
cube (of eight subcubes, or
elements ) and the order-4 Galois
cube (of 64 elements). The
order-3 cube (of 27 elements)
lacks, because it is based on
an odd prime, the remarkable
symmetry properties of its smaller
and larger cube neighbors.
Comments Off on Galois Cube Revisited
Thursday, May 26, 2011
For the Class of ’11
But leave the wise to wrangle, and with me
the quarrel of the universe let be;
and, in some corner of the hubbub couched,
make game of that which makes as much of thee.
Related material: Harvard Treasure, Favicon, and Crimson Tide.
Comments Off on For the Class of ’11
Tuesday, May 10, 2011
Groups Acting
The LA Times on last weekend's film "Thor"—
"… the film… attempts to bridge director Kenneth Branagh's high-minded Shakespearean intentions with Marvel Entertainment's bottom-line-oriented need to crank out entertainment product."
Those averse to Nordic religion may contemplate a different approach to entertainment (such as Taymor's recent approach to Spider-Man).
A high-minded— if not Shakespearean— non-Nordic approach to groups acting—
"What was wrong? I had taken almost four semesters of algebra in college. I had read every page of Herstein, tried every exercise. Somehow, a message had been lost on me. Groups act . The elements of a group do not have to just sit there, abstract and implacable; they can do things, they can 'produce changes.' In particular, groups arise naturally as the symmetries of a set with structure. And if a group is given abstractly, such as the fundamental group of a simplical complex or a presentation in terms of generators and relators, then it might be a good idea to find something for the group to act on, such as the universal covering space or a graph."
— Thomas W. Tucker, review of Lyndon's Groups and Geometry in The American Mathematical Monthly , Vol. 94, No. 4 (April 1987), pp. 392-394
"Groups act "… For some examples, see
- The 2×2×2 Cube,
- The Diamond 16 Puzzle,
- The Diamond Theorem, and
- Finite Geometry of the Square and Cube.
Related entertainment—
High-minded— Many Dimensions—
Not so high-minded— The Cosmic Cube—
One way of blending high and low—
The high-minded Charles Williams tells a story
in his novel Many Dimensions about a cosmically
significant cube inscribed with the Tetragrammaton—
the name, in Hebrew, of God.
The following figure can be interpreted as
the Hebrew letter Aleph inscribed in a 3×3 square—
The above illustration is from undated software by Ed Pegg Jr.
For mathematical background, see a 1985 note, "Visualizing GL(2,p)."
For entertainment purposes, that note can be generalized from square to cube
(as Pegg does with his "GL(3,3)" software button).
For the Nordic-averse, some background on the Hebrew connection—
Comments Off on Groups Acting
Thursday, May 5, 2011
Beyond Forgetfulness
From this journal on July 23, 2007—
|
It is not enough to cover the rock with leaves. We must be cured of it by a cure of the ground Or a cure of ourselves, that is equal to a cure
Of the ground, a cure beyond forgetfulness.
And if we ate the incipient colorings – Wallace Stevens, "The Rock" |
This quotation from Stevens (Harvard class of 1901) was posted here on when Daniel Radcliffe (i.e., Harry Potter) turned 18 in July 2007.
Other material from that post suggests it is time for a review of magic at Harvard.
On September 9, 2007, President Faust of Harvard
"encouraged the incoming class to explore Harvard’s many opportunities.
'Think of it as a treasure room of hidden objects Harry discovers at Hogwarts,' Faust said."
That class is now about to graduate.
It is not clear what "hidden objects" it will take from four years in the Harvard treasure room.
Perhaps the following from a book published in 1985 will help…
The March 8, 2011, Harvard Crimson illustrates a central topic of Metamagical Themas , the Rubik's Cube—
Hofstadter in 1985 offered a similar picture—
Hofstadter asks in his Metamagical introduction, "How can both Rubik's Cube and nuclear Armageddon be discussed at equal length in one book by one author?"
For a different approach to such a discussion, see Paradigms Lost, a post made here a few hours before the March 11, 2011, Japanese earthquake, tsunami, and nuclear disaster—
Whether Paradigms Lost is beyond forgetfulness is open to question.
Perhaps a later post, in the lighthearted spirit of Faust, will help. See April 20th's "Ready When You Are, C.B."
Comments Off on Beyond Forgetfulness
Thursday, April 28, 2011
Crimson Tide…
A sequel to Wednesday afternoon's post on The Harvard Crimson ,
Atlas Shrugged (illustrated below) —
Related material found today in Wikipedia—
|
See also Savage Logic (Oct. 19, 2010), as well as
Stellan Skarsgård in Lie Groups for Holy Week (March 30, 2010)
and in Exorcist: The Beginning (2004).
Comments Off on Crimson Tide…
Monday, April 18, 2011
Romancing the Junction
From Thomas Pynchon's 1997 Introduction to Stone Junction—
"He takes the Diamond, and then the Diamond takes him. For it turns out to be a gateway to elsewhere, and Daniel's life's tale an account of the incarnation of a god, not the usual sort that ends up bringing aid and comfort to earthly powers, but that favorite of writers, the incorruptible wiseguy known to anthropologists as the Trickster, to working alchemists as Hermes, to card-players everywhere as the Joker. We don't learn this till the end of the story, by which point, knowing Daniel as we've come to, we are free to take it literally as a real transfiguration, or as a metaphor of spiritual enlightenment, or as a description of Daniel's unusually exalted state of mind as he prepares to cross, forever, the stone junction between Above and Below— by this point, all of these possibilities have become equally true, for we have been along on one of those indispensable literary journeys, taken nearly as far as Daniel— though it is for him to slip along across the last borderline, into what Wittgenstein once supposed cannot be spoken of, and upon which, as Eliphaz Levi advised us— after 'To know, to will, to dare' as the last and greatest of the rules of Magic— we must keep silent."
"The devil likes metamorphoses." —The Club Dumas
Comments Off on Romancing the Junction
Thursday, March 10, 2011
Paradigms Lost
(Continued from February 19)
The cover of the April 1, 1970 second edition of The Structure of Scientific Revolutions , by Thomas S. Kuhn—
This journal on January 19, 2011—
If Galois geometry is thought of as a paradigm shift from Euclidean geometry,
both images above— the Kuhn cover and the nine-point affine plane—
may be viewed, taken together, as illustrating the shift. The nine subcubes
of the Euclidean 3x3x3 cube on the Kuhn cover do not form an affine plane
in the coordinate system of the Galois cube in the second image, but they
at least suggest such a plane. Similarly, transformations of a
non-mathematical object, the 1974 Rubik cube, are not Galois transformations,
but they at least suggest such transformations.
See also today's online Harvard Crimson illustration of problems of translation—
not unrelated to the problems of commensurability discussed by Kuhn.
Comments Off on Paradigms Lost
Thursday, December 2, 2010
Caesarian
The Dreidel Is Cast
The Nietzschean phrase "ruling and Caesarian spirits" occurred in yesterday morning's post "Novel Ending."
That post was followed yesterday morning by a post marking, instead, a beginning— that of Hanukkah 2010. That Jewish holiday, whose name means "dedication," commemorates the (re)dedication of the Temple in Jerusalem in 165 BC.
The holiday is celebrated with, among other things, the Jewish version of a die— the dreidel . Note the similarity of the dreidel to an illustration of The Stone* on the cover of the 2001 Eerdmans edition of Charles Williams's 1931 novel Many Dimensions—
For mathematics related to the dreidel , see Ivars Peterson's column on this date fourteen years ago.
For mathematics related (if only poetically) to The Stone , see "Solomon's Cube" in this journal.
Here is the opening of Many Dimensions—
For a fanciful linkage of the dreidel 's concept of chance to The Stone 's concept of invariant law, note that the New York Lottery yesterday evening (the beginning of Hanukkah) was 840. See also the number 840 in the final post (July 20, 2002) of the "Solomon's Cube" search.
Some further holiday meditations on a beginning—
Today, on the first full day of Hanukkah, we may or may not choose to mark another beginning— that of George Frederick James Temple, who was born in London on this date in 1901. Temple, a mathematician, was President of the London Mathematical Society in 1951-1953. From his MacTutor biography—
"In 1981 (at the age of 80) he published a book on the history of mathematics. This book 100 years of mathematics (1981) took him ten years to write and deals with, in his own words:-
those branches of mathematics in which I had been personally involved.
He declared that it was his last mathematics book, and entered the Benedictine Order as a monk. He was ordained in 1983 and entered Quarr Abbey on the Isle of Wight. However he could not stop doing mathematics and when he died he left a manuscript on the foundations of mathematics. He claims:-
The purpose of this investigation is to carry out the primary part of Hilbert's programme, i.e. to establish the consistency of set theory, abstract arithmetic and propositional logic and the method used is to construct a new and fundamental theory from which these theories can be deduced."
For a brief review of Temple's last work, see the note by Martin Hyland in "Fundamental Mathematical Theories," by George Temple, Philosophical Transactions of the Royal Society, A, Vol. 354, No. 1714 (Aug. 15, 1996), pp. 1941-1967.
The following remarks by Hyland are of more general interest—
"… one might crudely distinguish between philosophical and mathematical motivation. In the first case one tries to convince with a telling conceptual story; in the second one relies more on the elegance of some emergent mathematical structure. If there is a tradition in logic it favours the former, but I have a sneaking affection for the latter. Of course the distinction is not so clear cut. Elegant mathematics will of itself tell a tale, and one with the merit of simplicity. This may carry philosophical weight. But that cannot be guaranteed: in the end one cannot escape the need to form a judgement of significance."
— J. M. E. Hyland. "Proof Theory in the Abstract." (pdf)
Annals of Pure and Applied Logic 114, 2002, 43-78.
Here Hyland appears to be discussing semantic ("philosophical," or conceptual) and syntactic ("mathematical," or structural) approaches to proof theory. Some other remarks along these lines, from the late Gian-Carlo Rota—
See also "Galois Connections" at alpheccar.org and "The Galois Connection Between Syntax and Semantics" at logicmatters.net.
* Williams's novel says the letters of The Stone are those of the Tetragrammaton— i.e., Yod, He, Vau, He (cf. p. 26 of the 2001 Eerdmans edition). But the letters on the 2001 edition's cover Stone include the three-pronged letter Shin , also found on the dreidel . What esoteric religious meaning is implied by this, I do not know.
Comments Off on Caesarian
Saturday, November 27, 2010
Simplex Sigillum Veri
An Adamantine View of "The [Philosophers'] Stone"
The New York Times column "The Stone" on Sunday, Nov. 21 had this—
"Wittgenstein was formally presenting his Tractatus Logico-Philosophicus , an already well-known work he had written in 1921, as his doctoral thesis. Russell and Moore were respectfully suggesting that they didn’t quite understand proposition 5.4541 when they were abruptly cut off by the irritable Wittgenstein. 'I don’t expect you to understand!' (I am relying on local legend here….)"
Proposition 5.4541*—
Related material, found during a further search—
A commentary on "simplex sigillum veri" leads to the phrase "adamantine crystalline structure of logic"—
For related metaphors, see The Diamond Cube, Design Cube 2x2x2, and A Simple Reflection Group of Order 168.
Here Łukasiewicz's phrase "the hardest of materials" apparently suggested the commentators' adjective "adamantine." The word "diamond" in the links above refers of course not to a material, but to a geometric form, the equiangular rhombus. For a connection of this sort of geometry with logic, see The Diamond Theorem and The Geometry of Logic.
For more about God, a Stone, logic, and cubes, see Tale (Nov. 23).
* 5.4541 in the German original—
Die Lösungen der logischen Probleme müssen einfach sein,
denn sie setzen den Standard der Einfachheit.
Die Menschen haben immer geahnt, dass es
ein Gebiet von Fragen geben müsse, deren Antworten—
a priori—symmetrisch, und zu einem abgeschlossenen,
regelmäßigen Gebilde vereint liegen.
Ein Gebiet, in dem der Satz gilt: simplex sigillum veri.
Here "einfach" means "simple," not "neat," and "Gebiet" means
"area, region, field, realm," not (except metaphorically) "sphere."
Comments Off on Simplex Sigillum Veri
Saturday, June 19, 2010
Imago Creationis
In the above view, four of the tesseract's 16
vertices are overlaid by other vertices.
For views that are more complete and
moveable, see Smith's tesseract page.
Four-Part Tesseract Divisions—
The above figure shows how four-part partitions
of the 16 vertices of a tesseract in an infinite
Euclidean space are related to four-part partitions
of the 16 points in a finite Galois space
|
Euclidean spaces versus Galois spaces in a larger context— Infinite versus Finite The central aim of Western religion —
"Each of us has something to offer the Creator...
the bridging of
masculine and feminine,
life and death.
It's redemption.... nothing else matters."
-- Martha Cooley in The Archivist (1998)
The central aim of Western philosophy —
Dualities of Pythagoras
as reconstructed by Aristotle:
Limited Unlimited
Odd Even
Male Female
Light Dark
Straight Curved
... and so on ....
"Of these dualities, the first is the most important; all the others may be seen as different aspects of this fundamental dichotomy. To establish a rational and consistent relationship between the limited [man, etc.] and the unlimited [the cosmos, etc.] is… the central aim of all Western philosophy." |
Another picture related to philosophy and religion—
Jung's Four-Diamond Figure from Aion—
This figure was devised by Jung
to represent the Self. Compare the
remarks of Paul Valéry on the Self—
|
Flight from Eden: The Origins of Modern Literary Criticism and Theory, by Steven Cassedy, U. of California Press, 1990, pages 156-157—
Valéry saw the mind as essentially a relational system whose operation he attempted to describe in the language of group mathematics. "Every act of understanding is based on a group," he says (C, 1:331). "My specialty— reducing everything to the study of a system closed on itself and finite" (C, 19: 645). The transformation model came into play, too. At each moment of mental life the mind is like a group, or relational system, but since mental life is continuous over time, one "group" undergoes a "transformation" and becomes a different group in the next moment. If the mind is constantly being transformed, how do we account for the continuity of the self? Simple; by invoking the notion of the invariant. And so we find passages like this one: "The S[elf] is invariant, origin, locus or field, it's a functional property of consciousness" (C, 15:170 [2:315]). Just as in transformational geometry, something remains fixed in all the projective transformations of the mind's momentary systems, and that something is the Self (le Moi, or just M, as Valéry notates it so that it will look like an algebraic variable). Transformation theory is all over the place. "Mathematical science… reduced to algebra, that is, to the analysis of the transformations of a purely differential being made up of homogeneous elements, is the most faithful document of the properties of grouping, disjunction, and variation in the mind" (O, 1:36). "Psychology is a theory of transformations, we just need to isolate the invariants and the groups" (C, 1:915). "Man is a system that transforms itself" (C, 2:896). O Paul Valéry, Oeuvres (Paris: Pléiade, 1957-60) C Valéry, Cahiers, 29 vols. (Paris: Centre National de le Recherche Scientifique, 1957-61) |
Note also the remarks of George David Birkhoff at Rice University
in 1940 (pdf) on Galois's theory of groups and the related
"theory of ambiguity" in Galois's testamentary letter—
|
… metaphysical reasoning always relies on the Principle of Sufficient Reason, and… the true meaning of this Principle is to be found in the “Theory of Ambiguity” and in the associated mathematical “Theory of Groups.” If I were a Leibnizian mystic, believing in his “preestablished harmony,” and the “best possible world” so satirized by Voltaire in “Candide,” I would say that the metaphysical importance of the Principle of Sufficient Reason and the cognate Theory of Groups arises from the fact that God thinks multi-dimensionally* whereas men can only think in linear syllogistic series, and the Theory of Groups is the appropriate instrument of thought to remedy our deficiency in this respect. * That is, uses multi-dimensional symbols beyond our grasp. |
Related material:
A medal designed by Leibniz to show how
binary arithmetic mirrors the creation by God
of something (1) from nothing (0).
Another array of 16 strings of 0's and 1's, this time
regarded as coordinates rather than binary numbers—
Some context by a British mathematician —
|
Imago by Wallace Stevens Who can pick up the weight of Britain, Who can move the German load Or say to the French here is France again? Imago. Imago. Imago. It is nothing, no great thing, nor man Of ten brilliancies of battered gold And fortunate stone. It moves its parade Of motions in the mind and heart, A gorgeous fortitude. Medium man In February hears the imagination's hymns And sees its images, its motions And multitudes of motions And feels the imagination's mercies, In a season more than sun and south wind, Something returning from a deeper quarter, A glacier running through delirium, Making this heavy rock a place, Which is not of our lives composed . . . Lightly and lightly, O my land, Move lightly through the air again. |
Comments Off on Imago Creationis
Monday, June 7, 2010
Inspirational Combinatorics
According to the Mathematical Association of America this morning, one purpose of the upcoming June/July issue of the Notices of the American Mathematical Society is
"…to stress the inspirational role of combinatorics…."
Here is another contribution along those lines—
Eidetic Variation
from page 244 of
From Combinatorics to Philosophy: The Legacy of G.-C. Rota,
hardcover, published by Springer on August 4, 2009
(Edited by Ernesto Damiani, Ottavio D'Antona, Vincenzo Marra, and Fabrizio Palombi)
"Rota's Philosophical Insights," by Massimo Mugnai—
"… In other words, 'objectivism' is the attitude [that tries] to render a particular aspect absolute and dominant over the others; it is a kind of narrow-mindedness attempting to reduce to only one the multiple layers which constitute what we call 'reality.' According to Rota, this narrow-mindedness limits in an essential way even of [sic ] the most basic facts of our cognitive activity, as, for example, the understanding of a simple declarative sentence: 'So objectivism is the error we [make when we] persist in believing that we can understand what a declarative sentence means without a possible thematization of this declarative sentence in one of [an] endless variety of possible contexts' (Rota, 1991*, p. 155). Rota here implicitly refers to what, amongst phenomenologists is known as eidetic variation, i.e. the change of perspective, imposed by experience or performed voluntarily, from which to look at things, facts or sentences of the world. A typical example, proposed by Heidegger, in Sein und Zeit (1927) and repeated many times by Rota, is that of the hammer."
* Rota, G.-C. (1991), The End of Objectivity: The Legacy of Phenomenology. Lectures at MIT, Cambridge, MA, MIT Mathematics Department
The example of the hammer appears also on yesterday's online New York Times front page—
Related material:
From The Blackwell Dictionary of Western Philosophy—
Eidetic variation — an alternative expression for eidetic reduction
Husserl's term for an intuitive act toward an essence or universal, in contrast to an empirical intuition or perception. He also called this act an essential intuition, eidetic intuition, or eidetic variation. In Greek, eideo means “to see” and what is seen is an eidos (Platonic Form), that is, the common characteristic of a number of entities or regularities in experience. For Plato, eidos means what is seen by the eye of the soul and is identical with essence. Husserl also called this act “ideation,” for ideo is synonymous with eideo and also means “to see” in Greek. Correspondingly, idea is identical to eidos.
An example of eidos— Plato's diamond (from the Meno )—
For examples of variation of this eidos, see the diamond theorem.
See also Blockheads (8/22/08).
Related poetic remarks— The Trials of Device.
Comments Off on Inspirational Combinatorics
Saturday, February 27, 2010
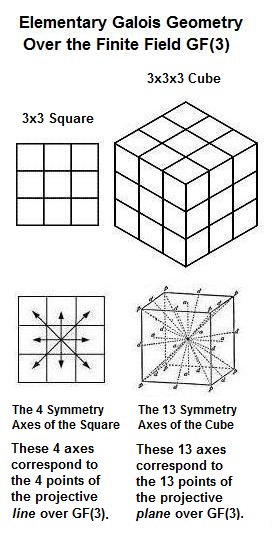
Cubist Geometries
"The cube has…13 axes of symmetry:
6 C2 (axes joining midpoints of opposite edges),
4 C3 (space diagonals), and
3C4 (axes joining opposite face centroids)."
–Wolfram MathWorld article on the cube
These 13 symmetry axes can be used to illustrate the interplay between Euclidean and Galois geometry in a cubic model of the 13-point Galois plane.
The geometer's 3×3×3 cube–
27 separate subcubes unconnected
by any Rubik-like mechanism–
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
A closely related structure–
the finite projective plane
with 13 points and 13 lines–
A later version of the 13-point plane
by Ed Pegg Jr.–
A group action on the 3×3×3 cube
as illustrated by a Wolfram program
by Ed Pegg Jr. (undated, but closely
related to a March 26, 1985 note
by Steven H. Cullinane)–
The above images tell a story of sorts.
The moral of the story–
Galois projective geometries can be viewed
in the context of the larger affine geometries
from which they are derived.
The standard definition of points in a Galois projective plane is that they are lines through the (arbitrarily chosen) origin in a corresponding affine 3-space converted to a vector 3-space.
If we choose the origin as the center cube in coordinatizing the 3×3×3 cube (See Weyl's relativity problem ), then the cube's 13 axes of symmetry can, if the other 26 cubes have properly (Weyl's "objectively") chosen coordinates, illustrate nicely the 13 projective points derived from the 27 affine points in the cube model.
The 13 lines of the resulting Galois projective plane may be derived from Euclidean planes through the cube's center point that are perpendicular to the cube's 13 Euclidean symmetry axes.
The above standard definition of points in a Galois projective plane may of course also be used in a simpler structure– the eightfold cube.
(The eightfold cube also allows a less standard way to picture projective points that is related to the symmetries of "diamond" patterns formed by group actions on graphic designs.)
See also Ed Pegg Jr. on finite geometry on May 30, 2006
at the Mathematical Association of America.
Comments Off on Cubist Geometries
Saturday, November 14, 2009
Mathematics and Narrative, continued:
A graphic novel reviewed in the current Washington Post features Alfred North Whitehead and Bertrand Russell–
Related material:
Whitehead on Fano's finite projective three-space:
"This is proved by the consideration of a three dimensional geometry in which there are only fifteen points."
—The Axioms of Projective Geometry , Cambridge University Press, 1906
Further reading:
See Solomon's Cube and the link at the end of today's previous entry, then compare and contrast the above portraits of Whitehead and Russell with Charles Williams's portraits of Sir Giles Tumulty and Lord Arglay in the novel Many Dimensions .
Comments Off on Mathematics and Narrative, continued:
Thursday, September 17, 2009
Thursday September 17, 2009
Jennifer's Body
The following remark this evening by Ann Hornaday of The Washington Post serves as an instant review of today's previous cinematic Log24 offering starring the late Patrick Swayze:
"Watch it, forget it, move on."
A perhaps more enduring tribute:
Comments Off on Thursday September 17, 2009
Wednesday, May 6, 2009
Wednesday May 6, 2009
Joke
“My pursuits are a joke
in that the universe is a joke.
One has to reflect
the universe faithfully.”
— John Frederick Michell
Feb. 9, 1933 –
April 24, 2009
“I laugh because I dare not cry.
This is a crazy world and
the only way to enjoy it
is to treat it as a joke.”
This is a crazy world and
the only way to enjoy it
is to treat it as a joke.”
— Robert A. Heinlein,
The Number of the Beast
For Marisa Tomei
(born Dec. 4, 1964) —
on the day that
Bob Seger turns 64 —
A Joke:
Points All Her Own
Points All Her Own,
Part I:
(For the backstory, see
the Log24 entries and links
on Marisa Tomei’s birthday
last year.)
Points All Her Own,
Part II:
(For the backstory, see
Galois Geometry:
The Simplest Examples.)
Points All Her Own,
Part III:
(For the backstory, see
Geometry of the I Ching
and the history of
Chinese philosophy.)
In simpler terms:
Comments Off on Wednesday May 6, 2009
Tuesday, February 24, 2009
Tuesday February 24, 2009
Hollywood Nihilism
Meets
Pantheistic Solipsism
Meets
Pantheistic Solipsism
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."
|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer… A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. |
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
This interpretation might appeal to Joan Didion, who, as author of the classic novel Play It As It Lays, is perhaps the world's leading expert on Hollywood nihilism.
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)
over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Heinlein should perhaps have had in mind the Klein correspondence when he discussed "some way to project six dimensions into three." While such a projection is of course trivial for anyone who has taken an undergraduate course in linear algebra, the following remarks by Philippe Cara present a much more meaningful mapping, using the Klein correspondence, of structures in six (affine) dimensions to structures in three.
Cara:
Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Comments Off on Tuesday February 24, 2009
Friday, December 12, 2008
Friday December 12, 2008
On the Symmetric Group S8
Wikipedia on Rubik's 2×2×2 "Pocket Cube"–
"Any permutation of the 8 corner cubies is possible (8! positions)."
Some pages related to this claim–
Analyzing Rubik's Cube with GAP
Online JavaScript Pocket Cube.
The claim is of course trivially true for the unconnected subcubes of Froebel's Third Gift:
© 2005 The Institute for Figuring
Photo by Norman Brosterman
fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
See also:
and
"Ad Reinhardt and Tony Smith:
A Dialogue,"
an exhibition opening today
at Pace Wildenstein.
For a different sort
of dialogue, click on the
artists' names above.
For a different
approach to S8,
see Symmetries.
"With humor, my dear Zilkov.
Always with a little humor."
-- The Manchurian Candidate
Comments Off on Friday December 12, 2008
Sunday, August 3, 2008
Sunday August 3, 2008
Kindergarten
Geometry
Geometry
Preview of a Tom Stoppard play presented at Town Hall in Manhattan on March 14, 2008 (Pi Day and Einstein's birthday):
The play's title, "Every Good Boy Deserves Favour," is a mnemonic for the notes of the treble clef EGBDF.
The place, Town Hall, West 43rd Street. The time, 8 p.m., Friday, March 14. One single performance only, to the tinkle– or the clang?– of a triangle. Echoing perhaps the clang-clack of Warsaw Pact tanks muscling into Prague in August 1968.
The “u” in favour is the British way, the Stoppard way, "EGBDF" being "a Play for Actors and Orchestra" by Tom Stoppard (words) and André Previn (music).
And what a play!– as luminescent as always where Stoppard is concerned. The music component of the one-nighter at Town Hall– a showcase for the Boston University College of Fine Arts– is by a 47-piece live orchestra, the significant instrument being, well, a triangle.
When, in 1974, André Previn, then principal conductor of the London Symphony, invited Stoppard "to write something which had the need of a live full-time orchestra onstage," the 36-year-old playwright jumped at the chance.
One hitch: Stoppard at the time knew "very little about 'serious' music… My qualifications for writing about an orchestra," he says in his introduction to the 1978 Grove Press edition of "EGBDF," "amounted to a spell as a triangle player in a kindergarten percussion band."
Review of the same play as presented at Chautauqua Institution on July 24, 2008:
"Stoppard's modus operandi– to teasingly introduce numerous clever tidbits designed to challenge the audience."
— Jane Vranish, Pittsburgh Post-Gazette, Saturday, August 2, 2008
"The leader of the band is tired
And his eyes are growing old
But his blood runs through
My instrument
And his song is in my soul."
— Dan Fogelberg
"He's watching us all the time."
|
Finnegans Wake, Book II, Episode 2, pp. 296-297:
I'll make you to see figuratleavely the whome of your eternal geomater. And if you flung her headdress on her from under her highlows you'd wheeze whyse Salmonson set his seel on a hexengown.1 Hissss!, Arrah, go on! Fin for fun! 1 The chape of Doña Speranza of the Nacion. |
|
Reciprocity From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity…. … E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies." — William Boyd, review of Himmelfarb, a novel by Michael Kruger, in The New York Times Book Review, October 30, 1994 Last year's entry on this date:
The picture above is of the complete graph K6 … Six points with an edge connecting every pair of points… Fifteen edges in all. Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24. If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See Reciprocity and Reversal in Lao Tzu. For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the K6 graph above, expresses the reciprocity of male and female, as my May 2003 archives illustrate. The star of David also appears as part of a graphic design for cubes that illustrate the concepts of diamond theory:
Click on the design for details. Those who prefer a Jewish approach to physics can find the star of David, in the form of K6, applied to the sixteen 4×4 Dirac matrices, in
A Graphical Representation The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See |
"Finn MacCool ate the Salmon of Knowledge."
Wikipedia:
"George Salmon spent his boyhood in Cork City, Ireland. His father was a linen merchant. He graduated from Trinity College Dublin at the age of 19 with exceptionally high honours in mathematics. In 1841 at age 21 he was appointed to a position in the mathematics department at Trinity College Dublin. In 1845 he was appointed concurrently to a position in the theology department at Trinity College Dublin, having been confirmed in that year as an Anglican priest."
Related material:
Arrangements for
56 Triangles.
For more on the
arrangement of
triangles discussed
in Finnegans Wake,
see Log24 on Pi Day,
March 14, 2008.
Happy birthday,
Martin Sheen.
Comments Off on Sunday August 3, 2008
Friday, July 25, 2008
Friday July 25, 2008
56 Triangles
"This wonderful picture was drawn by Greg Egan with the help of ideas from Mike Stay and Gerard Westendorp. It's probably the best way for a nonmathematician to appreciate the symmetry of Klein's quartic. It's a 3-holed torus, but drawn in a way that emphasizes the tetrahedral symmetry lurking in this surface! You can see there are 56 triangles: 2 for each of the tetrahedron's 4 corners, and 8 for each of its 6 edges."
Exercise:
Click on image for further details.
Note that if eight points are arranged
in a cube (like the centers of the
eight subcubes in the figure above),
there are 56 triangles formed by
the 8 points taken 3 at a time.
Baez's discussion says that the Klein quartic's 56 triangles can be partitioned into 7 eight-triangle Egan "cubes" that correspond to the 7 points of the Fano plane in such a way that automorphisms of the Klein quartic correspond to automorphisms of the Fano plane. Show that the 56 triangles within the eightfold cube can also be partitioned into 7 eight-triangle sets that correspond to the 7 points of the Fano plane in such a way that (affine) transformations of the eightfold cube induce (projective) automorphisms of the Fano plane.
Comments Off on Friday July 25, 2008
Monday, July 23, 2007
Monday July 23, 2007
Daniel Radcliffe
is 18 today.
is 18 today.
Greetings.
“The greatest sorcerer (writes Novalis memorably)
would be the one who bewitched himself to the point of
taking his own phantasmagorias for autonomous apparitions.
Would not this be true of us?”
–Jorge Luis Borges, “Avatars of the Tortoise”
“El mayor hechicero (escribe memorablemente Novalis)
sería el que se hechizara hasta el punto de
tomar sus propias fantasmagorías por apariciones autónomas.
¿No sería este nuestro caso?”
–Jorge Luis Borges, “Los Avatares de la Tortuga“
Autonomous Apparition
At Midsummer Noon:
|
|
It is not enough to cover the rock with leaves. We must be cured of it by a cure of the ground Or a cure of ourselves, that is equal to a cure
Of the ground, a cure beyond forgetfulness.
And if we ate the incipient colorings – Wallace Stevens, “The Rock” |
See also
“… I realized that to me,
Gödel and Escher and Bach
were only shadows
cast in different directions by
some central solid essence.
I tried to reconstruct
the central object, and
came up with this book.”
Gödel and Escher and Bach
were only shadows
cast in different directions by
some central solid essence.
I tried to reconstruct
the central object, and
came up with this book.”
Hofstadter’s cover.
Here are three patterns,
“shadows” of a sort,
derived from a different
“central object”:
“shadows” of a sort,
derived from a different
“central object”:
Click on image for details.
Comments Off on Monday July 23, 2007
Friday, February 2, 2007
Friday February 2, 2007
The Night Watch
For Catholic Schools Week
(continued from last year)–
Last night's Log24 Xanga
footprints from Poland:
Poland 2/2/07 1:29 AM
/446066083/item.html
2/20/06: The Past Revisited
(with link to online text of
Many Dimensions, by Charles Williams)
Poland 2/2/07 2:38 AM
/426273644/item.html
1/15/06 Inscape
(the mathematical concept, with
square and "star" diagrams)
Poland 2/2/07 3:30 AM
nextdate=2%252f8%252f20…
2/8/05 The Equation
(Russell Crowe as John Nash
with "star" diagram from a
Princeton lecture by Langlands)
Poland 2/2/07 4:31 AM
/524081776/item.html
8/29/06 Hollywood Birthday
(with link to online text of
Plato on the Human Paradox,
by a Fordham Jesuit)
Poland 2/2/07 4:43 AM
/524459252/item.html
8/30/06 Seven
(Harvard, the etymology of the
word "experience," and the
Catholic funeral of a professor's
23-year-old daughter)
Poland 2/2/07 4:56 AM
/409355167/item.html
12/19/05 Quarter to Three (cont.)
(remarks on permutation groups
for the birthday of Helmut Wielandt)
Poland 2/2/07 5:03 AM
/490604390/item.html
5/29/06 For JFK's Birthday
(The Call Girls revisited)
Poland 2/2/07 5:32 AM
/522299668/item.html
8/24/06 Beginnings
(Nasar in The New Yorker and
T. S. Eliot in Log24, both on the 2006
Beijing String Theory conference)
Poland 2/2/07 5:46 AM
/447354678/item.html
2/22/06 In the Details
(Harvard's president resigns,
with accompanying "rosebud")
Comments Off on Friday February 2, 2007
Wednesday, July 5, 2006
Wednesday July 5, 2006
And now, from
the author of Sphere…
CUBE
He beomes aware of something else… some other presence.
"Anybody here?" he says.
I am here.
He almost jumps, it is so loud. Or it seems loud. Then he wonders if he has heard anything at all.
"Did you speak?"
No.
How are we communicating? he wonders.
The way everything communicates with everything else.
Which way is that?
Why do you ask if you already know the answer?
— Sphere, by Michael Crichton, Harvard '64
"… when I went to Princeton things were completely different. This chapel, for instance– I remember when it was just a clearing, cordoned off with sharp sticks. Prayer was compulsory back then, and you couldn't just fake it by moving your lips; you had to know the words, and really mean them. I'm dating myself, but this was before Jesus Christ."
— Baccalaureate address at Princeton, Pentecost 2006, reprinted in The New Yorker, edited by David Remnick, Princeton '81
Related figures:
For further details,
see Solomon's Cube
and myspace.com/affine.
For further details,
see Jews on Buddhism
and
Adventures in Group Theory.
"In this way we are offered
a formidable lesson
for every Christian community."
Pope Benedict XVI
on Pentecost,
June 4, 2006,
St. Peter's Square.
Comments Off on Wednesday July 5, 2006
Wednesday, June 21, 2006
Wednesday June 21, 2006
Go with the Flow
The previous entry links to a document that discusses the mathematical concept of "Ricci flow (pdf)."
Though the concept was not named for him, this seems as good a time as any to recall the virtues of St. Matteo Ricci, a Jesuit who died in Beijing on May 11, 1610. (The Church does not yet recognize him as a saint; so much the worse for the Church.)
There was no Log24 entry on Ricci's saint's day, May 11, this year, but an entry for 4:29 PM May 10, 2006, seems relevant, since Beijing is 12 hours ahead of my local (Eastern US) time.
The relevance of this structure
to memory and to Chinese culture
is given in Dragon School and in
Geometry of the 4x4x4 Cube.
For some related remarks on
the colloquial, rather than the
mathematical, concept of flow,
see
Philosophy, Religion, and Science
as well as Crystal and Dragon.
Yesterday's entry on the 1865
remarks on aesthetics of
Gerard Manley Hopkins,
who later became a Jesuit,
may also have some relevance.
Comments Off on Wednesday June 21, 2006
Sunday, February 20, 2005
Sunday February 20, 2005
Relativity Blues
Today, February 20, is the 19th anniversary of my note The Relativity Problem in Finite Geometry. Here is some related material.
In 1931, the Christian writer Charles Williams grappled with the theology of time, space, free will, and the many-worlds interpretation of quantum mechanics (anticipating by many years the discussion of this topic by physicists beginning in the 1950's).
(Some pure mathematics — untainted by physics or theology — that is nevertheless related, if only by poetic analogy, to Williams's 1931 novel, Many Dimensions, is discussed in the above-mentioned note and in a generalization, Solomon's Cube.)
On the back cover of Williams's 1931 novel, the current publisher, William B. Eerdmans Publishing Company of Grand Rapids, Michigan, makes the following statement:
"Replete with rich religious imagery, Many Dimensions explores the relation between predestination and free will as it depicts different human responses to redemptive transcendence."
One possible response to such statements was recently provided in some detail by a Princeton philosophy professor. See On Bullshit, by Harry G. Frankfurt, Princeton University Press, 2005.
A more thoughtful response would take into account the following:
1. The arguments presented in favor of philosopher John Calvin, who discussed predestination, in The Death of Adam: Essays on Modern Thought, by Marilynne Robinson
2. The physics underlying Einstein's remarks on free will, God, and dice
3. The physics underlying Rebecca Goldstein's novel Properties of Light and Paul Preuss's novels Secret Passages and Broken Symmetries
4. The physics underlying the recent so-called "free will theorem" of John Conway and Simon Kochen of Princeton University
5. The recent novel Gilead, by Marilynne Robinson, which deals not with philosophy, but with lives influenced by philosophy — indirectly, by the philosophy of the aforementioned John Calvin.
From a review of Gilead by Jane Vandenburgh:
"In The Death of Adam, Robinson shows Jean Cauvin to be the foremost prophet of humanism whose Protestant teachings against the hierarchies of the Roman church set in motion the intellectual movements that promoted widespread literacy among the middle and lower classes, led to both the American and French revolutions, and not only freed African slaves in the United States but brought about suffrage for women. It's odd then that through our culture's reverse historicism, the term 'Calvinism' has come to mean 'moralistic repression.'"
For more on what the Calvinist publishing firm Eerdmans calls "redemptive transcendence," see various July 2003 Log24.net entries. If these entries include a fair amount of what Princeton philosophers call bullshit, let the Princeton philosophers meditate on the summary of Harvard philosophy quoted here on November 5 of last year, as well as the remarks of November 5, 2003, and those of November 5, 2002.
From Many Dimensions (Eerdmans paperback, 1963, page 53):
"Lord Arglay had a suspicion that the Stone would be purely logical. Yes, he thought, but what, in that sense, were the rules of its pure logic?"
A recent answer:
Modal Theology
"We symbolize logical necessity
with the box 
)
and logical possibility
with the diamond 
).
— Keith Allen Korcz,
(Log24.net, 1/25/05)
And what do we
symbolize by 
?
"The possibilia that exist,
and out of which
the Universe arose,
are located in
a necessary being…."
— Michael Sudduth,
Notes on
God, Chance, and Necessity
by Keith Ward,
Regius Professor of Divinity
at Christ Church College, Oxford
(the home of Lewis Carroll)
Comments Off on Sunday February 20, 2005
Wednesday, September 3, 2003
Wednesday September 3, 2003
Reciprocity
From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity….
… E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies."
— William Boyd, review of Himmelfarb, New York Times Book Review, October 30, 1994
Last year's entry on this date:
|
Today's birthday:
"Mathematics is the music of reason."
Sylvester, a nineteenth-century mathematician, coined the phrase "synthematic totals" to describe some structures based on 6-element sets that R. T. Curtis has called "rather unwieldy objects." See Curtis's abstract, Symmetric Generation of Finite Groups, John Baez's essay, Some Thoughts on the Number 6, and my website, Diamond Theory. |
The picture above is of the complete graph
Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24.
If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See
Reciprocity and Reversal in Lao Tzu.
For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in
Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the
Click on the design for details.
Those who prefer a Jewish approach to physics can find the star of David, in the form of
A Graphical Representation
of the Dirac Algebra.
The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See
Thursday, December 5, 2002
Thursday December 5, 2002
Sacerdotal Jargon
From the website
Abstracts and Preprints in Clifford Algebra [1996, Oct 8]:
Paper: clf-alg/good9601
From: David M. Goodmanson
Address: 2725 68th Avenue S.E., Mercer Island, Washington 98040
Title: A graphical representation of the Dirac Algebra
Abstract: The elements of the Dirac algebra are represented by sixteen 4×4 gamma matrices, each pair of which either commute or anticommute. This paper demonstrates a correspondence between the gamma matrices and the complete graph on six points, a correspondence that provides a visual picture of the structure of the Dirac algebra. The graph shows all commutation and anticommutation relations, and can be used to illustrate the structure of subalgebras and equivalence classes and the effect of similarity transformations….
Published: Am. J. Phys. 64, 870-880 (1996)
The following is a picture of K6, the complete graph on six points. It may be used to illustrate various concepts in finite geometry as well as the properties of Dirac matrices described above.
From
"The Relations between Poetry and Painting,"
by Wallace Stevens:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color. . . . The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space—which he calls the mind or heart of creation— determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
Comments Off on Thursday December 5, 2002
Monday, September 16, 2002
Monday September 16, 2002
A Time to Gather Stones Together
(Ecclesiastes 3:5)
Readings for Yom Kippur:
- "Stones," from Jewish Heritage Online Magazine
- "The Foundation Stone: The Center of Creation," from Jewish Heritage Online Magazine
- Many Dimensions, a novel by Charles Williams
Comments Off on Monday September 16, 2002
Sunday, August 4, 2002
Sunday August 4, 2002
versus
One year ago today, Lorenzo Music, the voice of Carlton the doorman on Rhoda, died. His eulogy from Valerie Harper:
"Valerie's heart is breaking, but Rhoda is certain that Carlton the doorman is giving St. Peter at the gate a run for his money."
Today's birthday: Logician John Venn.
Appearing for the story theory…
Flannery O'Connor:
"In the long run, a people is known, not by its statements or statistics, but by the stories it tells. Fiction is the most impure and the most modest and the most human of the arts."
Appearing for the diamond theory…
Mary McCarthy and G. H. Hardy:
From the Hollywood Investigator:
On October 18, 1979, Mary McCarthy said on PBS's Dick Cavett Show: "Every word she writes is a lie, including 'and' and 'the.'"
Don't forget "a," as in "a people is known" —
"Greek mathematics is permanent, more permanent even than Greek literature. Archimedes will be remembered when Aeschylus is forgotten, because languages die and mathematical ideas do not."
— G. H. Hardy in A Mathematician's Apology
And a closing rebuttal from the story theory…
Martin Heidegger and Dean Martin:
Words of wisdom from Martin Heidegger, Catholic Nazi:
"The nature of art is poetry. The nature of poetry, in turn, is the founding of truth…. In the work, truth is thrown toward… an historical group of men."
— Poetry, Language, Thought, page 75, translated by Albert Hofstadter, Harper & Row paperback, 1975
And from Dean Martin, avatar of anti-art :
– Artist: Dean Martin as sung on "Dean Martin's Greatest Hits"
– Capitol 4XL-9389
– peak Billboard position # 2 in 1953
– from the movie "the Caddy" starring Dean, Jerry Lewis, and Donna Reed
– Words and Music by Harry Warren and Jack Brooks(In Napoli where love is King, when boy meets girl, here's what they say)
When the moon hits your eye like a big-a pizza pie,
That's amore!
When the world seems to shine like you've had too much wine,
That's amore!
Comments Off on Sunday August 4, 2002