Monday, April 8, 2024
Saturday, April 6, 2024
Thursday, April 4, 2024
Figurate Geometry: Order-5 Triangle Labelings
See also Figurate Geometry at Zenodo —
Comments Off on Figurate Geometry: Order-5 Triangle Labelings
Tuesday, May 23, 2023
“A Mad Day’s Work” (Hat tip to Pierre Cartier)*
Comments Off on “A Mad Day’s Work” (Hat tip to Pierre Cartier)*
Monday, May 22, 2023
Triangular Hyperplane Arrangement
Abstract: Boolean functions on a triangular grid.
Note: It seems that the above rearrangement of a square array
of hyperplanes to a triangular array of hyperplanes, which was
rather arbitrarily constructed to have nice symmetries, will
answer a question posed here on Dec. 15, 2015.
Comments Off on Triangular Hyperplane Arrangement
Wednesday, February 1, 2023
Variations in Memory of a Designer
Last updated at 22:46 PM ET on 1 February 2023.
Click for a designer's obituary.
Paraphrase for a road-sign collector:
See as well … Today's New York Times obituary
of the Harvard Business School Publishing
Director of Intellectual Property.
Comments Off on Variations in Memory of a Designer
Thursday, January 5, 2023
Saturday, September 10, 2022
Orthogonal Latin Triangles
From a 1964 recreational-mathematics essay —
Note that the first two triangle-dissections above are analogous to
mutually orthogonal Latin squares . This implies a connection to
affine transformations within Galois geometry. See triangle graphics
in this journal.
Update of 4:40 AM ET —
Other mystical figures —
"Before time began, there was the Cube."
— Optimus Prime in "Transformers" (Paramount, 2007)
Comments Off on Orthogonal Latin Triangles
Thursday, July 21, 2022
Siamese Combinatorial Remarks
Further combinatorial properties* of 24261120 may
be investigated with the aid of a 9×9 square grid, and
perhaps (eventually) also with its triangular counterpart —
.
* Cap sets, gerechte designs, etc.
Comments Off on Siamese Combinatorial Remarks
Thursday, July 7, 2022
Square-Triangle Geometry
Each of the above mappings is, in some sense, "natural."
Is there any general order-n natural square-to-triangle mapping?
Comments Off on Square-Triangle Geometry
Wednesday, June 22, 2022
Occupy Space Continues.
Alternate Title —
Types of Ambiguity:
The Circle in the Triangle,
the Singer in the Song.
From an excellent June 17 Wall Street Journal review of a new
Isaac Bashevis Singer book from Princeton University Press —
" 'Old Truths and New Clichés,' a collection of 19
prose articles, most appearing in English for the
first time, reveals that Singer was as consummate
an essayist as he was a teller of tales." — Benjamin Balint
From a search in this journal for Singer —
Related material —
From a post of June 2, "Self-Enclosing" —
|
"… the self-enclosing processes by which late 20th-century
— Colin Burrow in the June 9, 2022 issue |
From the December 14, 2021, post Notes on Lines —
The triangle, a percussion instrument that was
featured prominently in the Tom Stoppard play
"Every Good Boy Deserves Favour."
Comments Off on Occupy Space Continues.
Sunday, June 12, 2022
Vocabulary: Trisquare Theorem
See also trisquare.space.
Comments Off on Vocabulary: Trisquare Theorem
Thursday, May 26, 2022
A Mad Day’s Work*
Comments Off on A Mad Day’s Work*
Friday, May 20, 2022
Squares to Triangles
Comments Off on Squares to Triangles
Monday, May 16, 2022
Sketch for a Magic Triangle
Updates from later the same day —
Related affine structures —
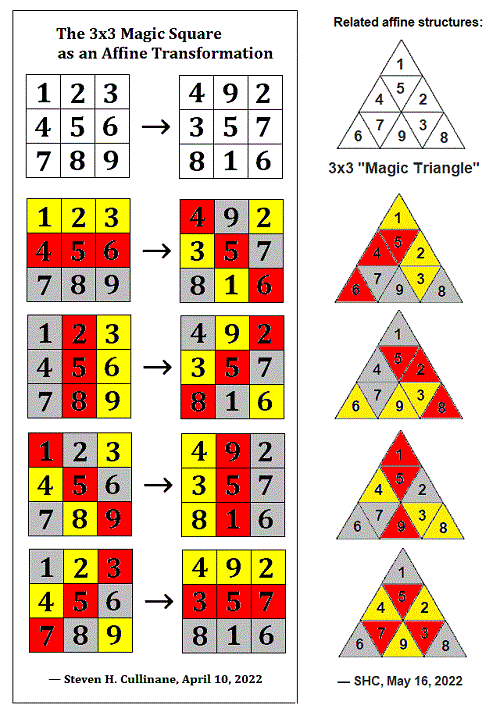
See also "Square+Triangles" in this journal.
The fishlike shapes within three of the above
ninefold colored triangles suggest some . . .
Related Entertainment —
Comments Off on Sketch for a Magic Triangle
Wednesday, November 27, 2019
A Companion-Piece for the Circular Rectangle:
For the circular rectangle, see today's earlier post "Enter Jonathan Miller…."
A recent view of the above address —
Comments Off on A Companion-Piece for the Circular Rectangle:
Tuesday, September 10, 2019
Tuesday, December 15, 2015
Square Triangles
Click image for some background.
Exercise: Note that, modulo color-interchange, the set of 15 two-color
patterns above is invariant under the group of six symmetries of the
equilateral triangle. Are there any other such sets of 15 two-color triangular
patterns that are closed as sets , modulo color-interchange, under the six
triangle symmetries and under the 322,560 permutations of the 16
subtriangles induced by actions of the affine group AGL(4,2)
on the 16 subtriangles' centers , given a suitable coordinatization?
Comments Off on Square Triangles
Tuesday, March 17, 2015
Saturday, January 18, 2014
The Triangle Relativity Problem
A sequel to last night's post The 4×4 Relativity Problem —
In other words, how should the triangle corresponding to
the above square be coordinatized ?
See also a post of July 8, 2012 — "Not Quite Obvious."
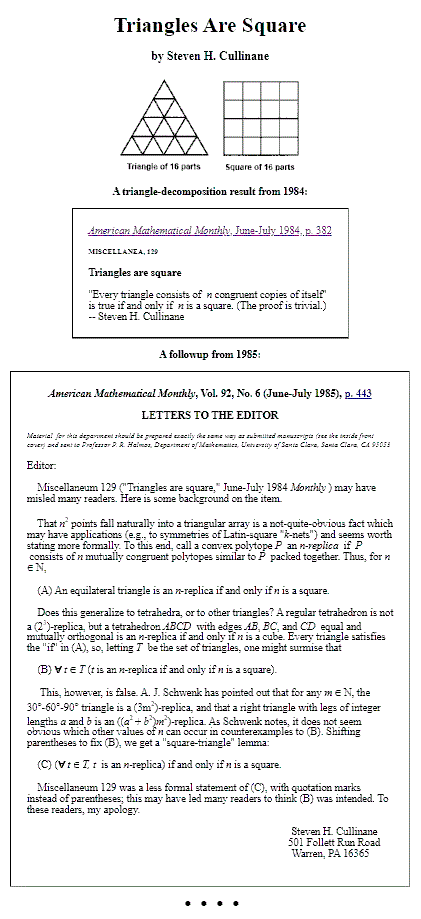
Context — "Triangles Are Square," a webpage stemming
from an American Mathematical Monthly item published
in 1984.
Comments Off on The Triangle Relativity Problem
Thursday, November 22, 2012
Finite Relativity
(Continued from 1986)
|
S. H. Cullinane This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.
— H. Weyl, The Classical Groups , In finite geometry "points" are often defined as ordered n-tuples of a finite (i.e., Galois) field GF(q). What geometric structures ("frames of reference," in Weyl's terms) are coordinatized by such n-tuples? Weyl's use of "objectively" seems to mean that such structures should have certain objective— i.e., purely geometric— properties invariant under each S. This note suggests such a frame of reference for the affine 4-space over GF(2), and a class of 322,560 equivalent coordinatizations of the frame. The frame: A 4×4 array. The invariant structure: The following set of 15 partitions of the frame into two 8-sets.
A representative coordinatization:
0000 0001 0010 0011
The group: The group AGL(4,2) of 322,560 regular affine transformations of the ordered 4-tuples over GF(2). |
S. H. Cullinane This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.
— H. Weyl, The Classical Groups , In finite geometry "points" are often defined as ordered n-tuples of a finite (i.e., Galois) field GF(q). What geometric structures ("frames of reference," in Weyl's terms) are coordinatized by such n-tuples? Weyl's use of "objectively" seems to mean that such structures should have certain objective— i.e., purely geometric— properties invariant under each S. This note suggests such a frame of reference for the affine 4-space over GF(2), and a class of 322,560 equivalent coordinatizations of the frame. The frame: An array of 16 congruent equilateral subtriangles that make up a larger equilateral triangle. The invariant structure: The following set of 15 partitions of the frame into two 8-sets.
The group: The group AGL(4,2) of 322,560 regular affine transformations of the ordered 4-tuples over GF(2). |
For some background on the triangular version,
see the Square-Triangle Theorem,
noting particularly the linked-to coordinatization picture.
Comments Off on Finite Relativity
Sunday, July 15, 2012
Squares Are Triangular
"A figurate number… is a number
that can be represented by
a regular geometrical arrangement
of equally spaced points."
— Eric W. Weisstein at Wolfram MathWorld
For example—
Call a convex polytope P an n-replica if P consists of n
mutually congruent polytopes similar to P packed together.
The square-triangle theorem (or lemma) says that
"Every triangle is an n-replica"
is true if and only if n is a square.
Equivalently,
The positive integer n is a square
if and only if every triangle is an n-replica.
(I.e., squares are triangular.)
This supplies the converse to the saying that
Comments Off on Squares Are Triangular
Sunday, July 8, 2012
Not Quite Obvious
"That n 2 points fall naturally into a triangular array
is a not-quite-obvious fact which may have applications…
and seems worth stating more formally."
— Steven H. Cullinane, letter in the
American Mathematical Monthly 1985 June-July issue
If the ancient Greeks had not been distracted by
investigations of triangular (as opposed to square )
numbers, they might have done something with this fact.
A search for occurrences of the phrase
"n2 [i.e., n 2 ] congruent triangles"
indicates only fairly recent (i.e., later than 1984) results.*
Some related material, updated this morning—
|
This suggests a problem—
What mappings of a square array of n 2 points to
In the figure above, whether |
* Update of July 15, 2012 (11:07 PM ET)—
Theorem on " rep-n 2 " (Golomb's terminology)
triangles from a 1982 book—
Comments Off on Not Quite Obvious