Wednesday, December 24, 2025
Thursday, February 3, 2022
Through the Asian Looking Glass
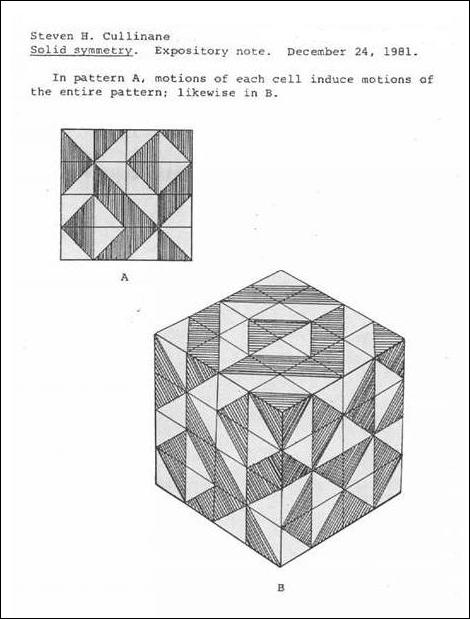
The Miracle Octad Generator (MOG) —
The Conway-Sloane version of 1988:
See also the 1976 R. T. Curtis version, of which the Conway-Sloane version
is a mirror reflection —
“There is a correspondence between the two systems
of 35 groups, which is illustrated in Fig. 4 (the MOG or
Miracle Octad Generator).”
—R.T. Curtis, “A New Combinatorial Approach to M24,”
Mathematical Proceedings of the Cambridge Philosophical
Society (1976), 79: 25-42
Curtis’s 1976 Fig. 4. (The MOG.)
The Guy Embedding (named for M.J.T., not Richard K., Guy) states that
the MOG is naturally embedded in the codewords of the extended binary
Golay code, if those codewords are generated in lexicographic order.
The above reading order for the MOG 4×6 array —
down the columns, from left to right — yields the Conway-Sloane MOG.
Since that is a mirror image of the original Curtis MOG, the reading order
yielding that MOG is down the columns, from right to left.
"Traditionally, Chinese, Japanese, Vietnamese and Korean are written vertically
in columns going from top to bottom and ordered from right to left, with each
new column starting to the left of the preceding one." — Wikipedia
The Asian reading order has certain artistic advantages:
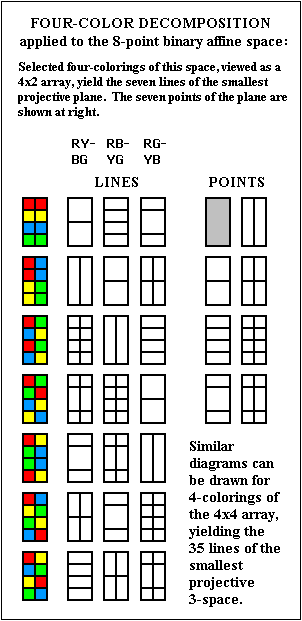
Comments Off on Through the Asian Looking Glass
Four-Color Structures (Review)
Comments Off on Four-Color Structures (Review)
Thursday, January 27, 2022
The Lexicographic Octad Generator
A Lexicographic Basis for the Binary Golay Code:
Brouwer and Guven — "Long ago," in
"The generating rank of the space of short vectors
in the Leech lattice mod 2," by
Andries Brouwer & Cicek Guven,
https://www.win.tue.nl/~aeb/preprints/udim24a.pdf —
"One checks by computer" that this is a basis:
000000000000000011111111
000000000000111100001111
000000000011001100110011
000000000101010101010101
000000001001011001101001
000000110000001101010110
000001010000010101100011
000010010000011000111010
000100010001000101111000
001000010001001000011101
010000010001010001001110
100000010001011100100100
(Copied from the Brouwer-Guven paper)
_________________________________________________
Adlam at Harvard —
"Constructing the Extended Binary Golay Code,"
by Ben Adlam, Harvard University, August 9, 2011,
https://fliphtml5.com/llqx/wppz/basic —
Adlam also asserts, citing a reference, that this same
set of twelve vectors is a basis:
000000000000000011111111
000000000000111100001111
000000000011001100110011
000000000101010101010101
000000001001011001101001
000000110000001101010110
000001010000010101100011
000010010000011000111010
000100010001000101111000
001000010001001000011101
010000010001010001001110
100000010001011100100100
(Copied from the Adlam paper)
__________________________________________________
Sources —
"One checks by computer" —
At http://magma.maths.usyd.edu.au/calc/ —
|
> V24 := VectorSpace(FiniteField(2), 24); > G := sub< V24 | > [0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,0,1,1,1,1,1,1,1,1], > [0,0,0,0,0,0,0,0,0,0,0,0,1,1,1,1,0,0,0,0,1,1,1,1], > [0,0,0,0,0,0,0,0,0,0,1,1,0,0,1,1,0,0,1,1,0,0,1,1], > [0,0,0,0,0,0,0,0,0,1,0,1,0,1,0,1,0,1,0,1,0,1,0,1], > [0,0,0,0,0,0,0,0,1,0,0,1,0,1,1,0,0,1,1,0,1,0,0,1], > [0,0,0,0,0,0,1,1,0,0,0,0,0,0,1,1,0,1,0,1,0,1,1,0], > [0,0,0,0,0,1,0,1,0,0,0,0,0,1,0,1,0,1,1,0,0,0,1,1], > [0,0,0,0,1,0,0,1,0,0,0,0,0,1,1,0,0,0,1,1,1,0,1,0], > [0,0,0,1,0,0,0,1,0,0,0,1,0,0,0,1,0,1,1,1,1,0,0,0], > [0,0,1,0,0,0,0,1,0,0,0,1,0,0,1,0,0,0,0,1,1,1,0,1], > [0,1,0,0,0,0,0,1,0,0,0,1,0,1,0,0,0,1,0,0,1,1,1,0], > [1,0,0,0,0,0,0,1,0,0,0,1,0,1,1,1,0,0,1,0,0,1,0,0]>; > Dimension(G); 12 |
Comments Off on The Lexicographic Octad Generator
Sunday, January 2, 2022
Annals of Modernism: UR–Grid
The above New Yorker art illustrates the 2×4 structure of
an octad in the Miracle Octad Generator of R. T. Curtis.
Enthusiasts of simplicity may note how properties of this eight-cell
2×4 grid are related to those of the smaller six-cell 3×2 grid:
See Nocciolo in this journal and . . .
Further reading on the six-set – eight-set relationship:
Comments Off on Annals of Modernism: UR–Grid
Thursday, December 30, 2021
Antidote to Chaos?
Some formal symmetry —
|
"… each 2×4 "brick" in the 1974 Miracle Octad Generator
Folding a 2×4 Curtis array yet again yields — Steven H. Cullinane on April 19, 2016 — The Folding. |
Related art-historical remarks:
The Shape of Time (Kubler, Yale U.P., 1962).
See yesterday's post The Thing .
Comments Off on Antidote to Chaos?
Friday, October 8, 2021
Sunday, May 30, 2021
Framed
Related reading: "Frame Analysis" in this journal.
Update of Monday, May 31, 2021 —
For connoisseurs of bullshit —
"… it would have made for a memorable
photograph, the image preserved within
he confines of a four-by-six-inch print."
— Lincoln Perry on a remembered scene
in "If You Frame It Like That," an essay in
The American Scholar dated March 2, 2020,
linked to today at Arts & Letters Daily
(A website whose motto is VERITAS ODIT
MORAS , "Truth hates delay").
And then there is non-bullshit about a
four-by-six frame —
Bullshit addicts pondering the meaning of the letter "Q" may consult
"Q is for Quelle ," "Q is for Quality," and this journal on the above
American Scholar date — March 2, 2020.
Comments Off on Framed
Saturday, May 8, 2021
A Tale of Two Omegas
The Greek capital letter Omega, Ω, is customarily
used to denote a set that is acted upon by a group.
If the group is the affine group of 322,560
transformations of the four-dimensional
affine space over the two-element Galois field,
the appropriate Ω is the 4×4 grid above.
See the Cullinane diamond theorem .
If the group is the large Mathieu group of
244,823,040 permutations of 24 things,
the appropriate Ω is the 4×6 grid below.
See the Miracle Octad Generator of R. T. Curtis.
Comments Off on A Tale of Two Omegas
Sunday, March 21, 2021
Mathematics and Narrative: The Unity
“To conquer, three boxes* have to synchronize and join together into the Unity.”
―Wonder Woman in Zack Snyder’s Justice League
See also The Unity of Combinatorics and The Miracle Octad Generator.
* Cf. Aitchison’s Octads —
Comments Off on Mathematics and Narrative: The Unity
Thursday, February 25, 2021
Compare and Contrast
“… What is your dream—your ideal? What is your News from Nowhere,
or, rather, What is the result of the little shake your hand has given to
the old pasteboard toy with a dozen bits of colored glass for contents?
And, most important of all, can you present it in a narrative or romance
which will enable me to pass an idle hour not disagreeably? How, for instance,
does it compare in this respect with other prophetic books on the shelf?”
— Hudson, W. H.. A Crystal Age (p. 2). Open Road Media. Kindle Edition.
See as well . . .
The lexicographic Golay code
contains, embedded within it,
the Miracle Octad Generator.
Comments Off on Compare and Contrast
Wednesday, January 27, 2021
Adoration of the Cube . . .
Related vocabulary —
See as well the word facet in this journal.
Analogously, one might write . . .
A Hiroshima cube consists of 6 faces ,
each with 4 squares called facets ,
for a total of 24 facets. . . ."
(See Aitchison's Octads , a post of Feb. 19, 2020.)
Click image to enlarge. Background: Posts tagged 'Aitchison.'"
Comments Off on Adoration of the Cube . . .
Thursday, December 3, 2020
Brick Joke
The "bricks" in posts tagged Octad Group suggest some remarks
from last year's HBO "Watchmen" series —
Related material — The two bricks constituting a 4×4 array, and . . .
"(this is the famous Kummer abstract configuration )"
— Igor Dolgachev, ArXiv, 16 October 2019.
As is this —
.
The phrase "octad group" does not, as one might reasonably
suppose, refer to symmetries of an octad (a "brick"), but
instead to symmetries of the above 4×4 array.
A related Broomsday event for the Church of Synchronology —
Comments Off on Brick Joke
Wednesday, October 7, 2020
Between Pyramid and Pentagon
The 35 small squares between the pyramid and the pentagon
in the above search result illustrate the role of finite geometry
in the Miracle Octad Generator of R. T. Curtis.
Comments Off on Between Pyramid and Pentagon
Wednesday, September 2, 2020
Space Wars: Sith Pyramid vs. Jedi Cube
For the Sith Pyramid, see posts tagged Pyramid Game.
For the Jedi Cube, see posts tagged Enigma Cube
and cube-related remarks by Aitchison at Hiroshima.
This post was suggested by two events of May 16, 2019 —
A weblog post by Frans Marcelis on the Miracle Octad
Generator of R. T. Curtis (illustrated with a pyramid),
and the death of I. M. Pei, architect of the Louvre pyramid.
That these events occurred on the same date is, of course,
completely coincidental.
Perhaps Dan Brown can write a tune to commemorate
the coincidence.
Comments Off on Space Wars: Sith Pyramid vs. Jedi Cube
Thursday, August 27, 2020
The Complete Extended Binary Golay Code
All 4096 vectors in the code are at . . .
http://neilsloane.com/oadir/oa.4096.12.2.7.txt.
Sloane’s list* contains the 12 generating vectors
listed in 2011 by Adlam —
As noted by Conway in Sphere Packings, Lattices and Groups ,
these 4096 vectors, constructed lexicographically, are exactly
the same vectors produced by using the Conway-Sloane version
of the Curtis Miracle Octad Generator (MOG). Conway says this
lexico-MOG equivalence was first discovered by M. J. T. Guy.
(Of course, any permutation of the 24 columns above produces
a version of the code qua code. But because the lexicographic and
the MOG constructions yield the same result, that result is in
some sense canonical.)
See my post of July 13, 2020 —
The lexicographic Golay code
contains, embedded within it,
the Miracle Octad Generator.
For some related results, Google the twelfth generator:
* Sloane’s list is of the codewords as the rows of an orthogonal array —
See also http://neilsloane.com/oadir/.
Comments Off on The Complete Extended Binary Golay Code
Tuesday, July 28, 2020
Friday, July 17, 2020
Theoretical as Well as Practical Value
See also The Lexicographic Octad Generator (LOG) (July 13, 2020)
and Octads and Geometry (April 23, 2020).
Comments Off on Theoretical as Well as Practical Value
Monday, July 13, 2020
The Lexicographic Octad Generator (LOG)*
The lexicographic Golay code
contains, embedded within it,
the Miracle Octad Generator.
By Steven H. Cullinane, July 13, 2020
Background —
The Miracle Octad Generator (MOG)
of R. T. Curtis (Conway-Sloane version) —
A basis for the Golay code, excerpted from a version of
the code generated in lexicographic order, in
"Constructing the Extended Binary Golay Code"
Ben Adlam
Harvard University
August 9, 2011:
000000000000000011111111
000000000000111100001111
000000000011001100110011
000000000101010101010101
000000001001011001101001
000000110000001101010110
000001010000010101100011
000010010000011000111010
000100010001000101111000
001000010001001000011101
010000010001010001001110
100000010001011100100100
Below, each vector above has been reordered within
a 4×6 array, by Steven H. Cullinane, to form twelve
independent Miracle Octad Generator vectors
(as in the Conway-Sloane SPLAG version above, in
which Curtis's earlier heavy bricks are reflected in
their vertical axes) —
01 02 03 04 05 . . . 20 21 22 23 24 --> 01 05 09 13 17 21 02 06 10 14 18 22 03 07 11 15 19 23 04 08 12 16 20 24 0000 0000 0000 0000 1111 1111 --> 0000 11 0000 11 0000 11 0000 11 as in the MOG. 0000 0000 0000 1111 0000 1111 --> 0001 01 0001 01 0001 01 0001 01 as in the MOG. 0000 0000 0011 0011 0011 0011 --> 0000 00 0000 00 0011 11 0011 11 as in the MOG. 0000 0000 0101 0101 0101 0101 --> 0000 00 0011 11 0000 00 0011 11 as in the MOG. 0000 0000 1001 0110 0110 1001 --> 0010 01 0001 10 0001 10 0010 01 as in the MOG. 0000 0011 0000 0011 0101 0110 --> 0000 00 0000 11 0101 01 0101 10 as in the MOG. 0000 0101 0000 0101 0110 0011 --> 0000 00 0101 10 0000 11 0101 01 as in the MOG. 0000 1001 0000 0110 0011 1010 --> 0100 01 0001 00 0001 11 0100 10 as in the MOG. 0001 0001 0001 0001 0111 1000 --> 0000 01 0000 10 0000 10 1111 10 as in the MOG. 0010 0001 0001 0010 0001 1101 --> 0000 01 0000 01 1001 00 0110 11 as in the MOG. 0100 0001 0001 0100 0100 1110 --> 0000 01 1001 11 0000 01 0110 00 as in the MOG. 1000 0001 0001 0111 0010 0100 --> 10 00 00 00 01 01 00 01 10 01 11 00 as in the MOG (heavy brick at center).
Update at 7:41 PM ET the same day —
A check of SPLAG shows that the above result is not new:
And at 7:59 PM ET the same day —
Conway seems to be saying that at some unspecified point in the past,
M.J.T. Guy, examining the lexicographic Golay code, found (as I just did)
that weight-8 lexicographic Golay codewords, when arranged naturally
in 4×6 arrays, yield certain intriguing visual patterns. If the MOG existed
at the time of his discovery, he would have identified these patterns as
those of the MOG. (Lexicographic codes have apparently been
known since 1960, the MOG since the early 1970s.)
* Addendum at 4 AM ET the next day —
See also Logline (Walpurgisnacht 2013).
Comments Off on The Lexicographic Octad Generator (LOG)*
Saturday, May 2, 2020
Turyn’s Octad Theorem: The Next Level*
From the obituary of a game inventor who reportedly died
on Monday, February 25, 2013 —
” ‘He was hired because of the game,’ Richard Turyn,
a mathematician who worked at Sylvania, told
the Washington Post in a 2004 feature on Diplomacy.”
* For the theorem, see Wolfram Neutsch, Coordinates .
(Published by de Gruyter, 1996. See pp. 761-766.)
Having defined (pp. 751-752) the Miracle Octad Generator (MOG)
as a 4×6 array to be used with Conway’s “hexacode,” Neutsch says . . .
“Apart from the three constructions of the Golay codes
discussed at length in this book (lexicographic and via
the MOG or the projective line), there are literally
dozens of alternatives. For lack of space, we have to
restrict our attention to a single example. It has been
discovered by Turyn and can be connected in a very
beautiful way with the Miracle Octad Generator….
To this end, we consider the natural splitting of the MOG into
three disjoint octads L, M, R (‘left’, ‘middle’, and ‘right’ octad)….”
— From page 761
“The theorem of Turyn” is on page 764 —
Comments Off on Turyn’s Octad Theorem: The Next Level*
Wednesday, April 29, 2020
Curtis at Pilsen, Thursday, July 5, 2018
For an account by R. T. Curtis of how he discovered the Miracle Octad Generator,
see slides by Curtis, "Graphs and Groups," from his talk on July 5, 2018, at the
Pilsen conference on algebraic graph theory, "Symmetry vs. Regularity: The first
50 years since Weisfeiler-Leman stabilization" (WL2018).
See also "Notes to Robert Curtis’s presentation at WL2018," by R. T. Curtis.
Meanwhile, here on July 5, 2018 —
|
Simultaneous perspective does not look upon language as a path because it is not the search for meaning that orients it. Poetry does not attempt to discover what there is at the end of the road; it conceives of the text as a series of transparent strata within which the various parts—the different verbal and semantic currents—produce momentary configurations as they intertwine or break apart, as they reflect each other or efface each other. Poetry contemplates itself, fuses with itself, and obliterates itself in the crystallizations of language. Apparitions, metamorphoses, volatilizations, precipitations of presences. These configurations are crystallized time: although they are perpetually in motion, they always point to the same hour—the hour of change. Each one of them contains all the others, each one is inside the others: change is only the oft-repeated and ever-different metaphor of identity.
— Paz, Octavio. The Monkey Grammarian |
he 2018 Log24 post containing the above Paz quote goes on to quote
remarks by Lévi-Strauss. Paz's phrase "series of transparent strata"
suggests a review of other remarks by Lévi-Strauss in the 2016 post
"Key to All Mythologies."
Comments Off on Curtis at Pilsen, Thursday, July 5, 2018
Thursday, April 23, 2020
Octads and Geometry
See the web pages octad.group and octad.us.
Related geometry (not the 759 octads, but closely related to them) —
#/media/File:150614-PG-3-2-schoolgirls-arrangement.png)
The 4×6 rectangle of R. T. Curtis
illustrates the geometry of octads —
Curtis splits the 4×6 rectangle into three 4×2 "bricks" —
.
"In fact the construction enables us to describe the octads
in a very revealing manner. It shows that each octad,
other than Λ1, Λ2, Λ3, intersects at least one of these ' bricks' —
the 'heavy brick' – in just four points." . . . .
— R. T. Curtis (1976). "A new combinatorial approach to M24,"
Mathematical Proceedings of the Cambridge Philosophical Society ,
79, pp 25-42.
Comments Off on Octads and Geometry
Sunday, March 15, 2020
The “Octad Group”
The phrase “octad group” discussed here in a post
of March 7 is now a domain name, “octad.group,”
that leads to that post. Remarks by Conway and
Sloane now quoted there indicate how the group
that I defined in 1979 is embedded in the large
Mathieu group M24.
Related literary notes — Watson + Embedding.
Comments Off on The “Octad Group”
Saturday, March 7, 2020
The “Octad Group” as Symmetries of the 4×4 Square
From "Mathieu Moonshine and Symmetry Surfing" —
(Submitted on 29 Sep 2016, last revised 22 Jan 2018)
by Matthias R. Gaberdiel (1), Christoph A. Keller (2),
and Hynek Paul (1)
(1) Institute for Theoretical Physics, ETH Zurich
(2) Department of Mathematics, ETH Zurich
https://arxiv.org/abs/1609.09302v2 —
"This presentation of the symmetry groups Gi is
particularly well-adapted for the symmetry surfing
philosophy. In particular it is straightforward to
combine them into an overarching symmetry group G
by combining all the generators. The resulting group is
the so-called octad group
G = (Z2)4 ⋊ A8 .
It can be described as a maximal subgroup of M24
obtained by the setwise stabilizer of a particular
'reference octad' in the Golay code, which we take
to be O9 = {3,5,6,9,15,19,23,24} ∈ 𝒢24. The octad
subgroup is of order 322560, and its index in M24
is 759, which is precisely the number of
different reference octads one can choose."
This "octad group" is in fact the symmetry group of the affine 4-space over GF(2),
so described in 1979 in connection not with the Golay code but with the geometry
of the 4×4 square.* Its nature as an affine group acting on the Golay code was
known long before 1979, but its description as an affine group acting on
the 4×4 square may first have been published in connection with the
Cullinane diamond theorem and Abstract 79T-A37, "Symmetry invariance in a
diamond ring," by Steven H. Cullinane in Notices of the American Mathematical
Society , February 1979, pages A-193, 194.
* The Galois tesseract .
Update of March 15, 2020 —
Conway and Sloane on the "octad group" in 1993 —
Comments Off on The “Octad Group” as Symmetries of the 4×4 Square
Wednesday, February 19, 2020
Aitchison’s Octads
The 759 octads of the Steiner system S(5,8,24) are displayed
rather neatly in the Miracle Octad Generator of R. T. Curtis.
A March 9, 2018, construction by Iain Aitchison* pictures the
759 octads on the faces of a cube , with octad elements the
24 edges of a cuboctahedron :
The Curtis octads are related to symmetries of the square.
See my webpage "Geometry of the 4×4 square" from March 2004.
Aitchison's p. 42 slide includes an illustration from that page —
Aitchison's octads are instead related to symmetries of the cube.
Note that essentially the same model as Aitchison's can be pictured
by using, instead of the 24 edges of a cuboctahedron, the 24 outer
faces of subcubes in the eightfold cube .
Image from Christmas Day 2005.
* http://www.math.sci.hiroshima-u.ac.jp/branched/files/2018/
presentations/Aitchison-Hiroshima-2-2018.pdf.
See also Aitchison in this journal.
Comments Off on Aitchison’s Octads
Saturday, December 14, 2019
Colorful Tale
The above image is from
"A Four-Color Theorem:
Function Decomposition Over a Finite Field,"
http://finitegeometry.org/sc/gen/mapsys.html.
These partitions of an 8-set into four 2-sets
occur also in Wednesday night's post
Miracle Octad Generator Structure.
This post was suggested by a Daily News
story from August 8, 2011, and by a Log24
post from that same date, "Organizing the
Mine Workers" —
Comments Off on Colorful Tale
Wednesday, December 11, 2019
Miracle Octad Generator Structure
(Adapted from Eightfold Geometry, a note of April 28, 2010.
See also the recent post Geometry of 6 and 8.)
Comments Off on Miracle Octad Generator Structure
Thursday, October 31, 2019
56 Triangles
The post "Triangles, Spreads, Mathieu" of October 29 has been
updated with an illustration from the Curtis Miracle Octad Generator.
Related material — A search in this journal for "56 Triangles."
Comments Off on 56 Triangles
Saturday, September 21, 2019
Annals of Random Fandom
For Dan Brown fans …
… and, for fans of The Matrix, another tale
from the above death date: May 16, 2019 —
An illustration from the above
Miracle Octad Generator post:
Related mathematics — Tetrahedron vs. Square.
Comments Off on Annals of Random Fandom
Thursday, July 25, 2019
Simple
From "110 in the Shade" —
A quote from "Marshall, Meet Bagger," July 29, 2011:
"Time for you to see the field."
_________________________________________________________
From a Log24 search for "To See the Field" —
For further details, see the 1985 note
"Generating the Octad Generator."
Comments Off on Simple