Tuesday, June 15, 2010
|
Friday, April 25, 2025
Imago Review: “The Thing and I” Continues.
Sunday, November 18, 2012
Sermon
Happy birthday to…

Today's sermon, by Marie-Louise von Franz—
For more on the modern physicist analyzed by von Franz,
see The Innermost Kernel , by Suzanne Gieser.
Another modern physicist, Niels Bohr, died
on this date in 1962…
|
The circle above is marked with a version For the square, see the diamond theorem. "Two things of opposite natures seem to depend — Wallace Stevens, |
Saturday, November 5, 2011
Shadows
Between the idea
And the reality
Between the motion
And the act
Falls the Shadow
— T. S. Eliot, "The Hollow Men"
A passage quoted here on this date in 2005—
Douglas Hofstadter on his magnum opus:
“… I realized that to me,
Gödel and Escher and Bach
were only shadows
cast in different directions
by some central solid essence."
This refers to Hofstadter's cover image:
Also from this date in 2005:

Friday, November 4, 2011
Logos
Continued from All Hallows Eve…

The Belgian Lottery was a sponsor of
last month's 25th Solvay Conference —
"The Theory of the Quantum World,"
Brussels, October 19-22, 2011.
See also this journal in October and Change Logos—

(Physicists will recognize the kinship
with the coat of arms of Niels Bohr.)
Friday, July 1, 2011
Symmetry Review
Popular novelist Dan Brown is to speak at Chautauqua Institution on August 1.
This suggests a review of some figures discussed here in a note on Brown from February 20, 2004—

Related material: Notes from Nov. 5, 1981, and from Dec. 24, 1981.
For the lower figure in context, see the diamond theorem.
Saturday, May 28, 2011
Savage Detectives
|
From Savage Logic— Sunday, March 15, 2009 5:24 PM The Origin of Change A note on the figure "Two things of opposite natures seem to depend — Wallace Stevens, |
Saturday, January 22, 2011
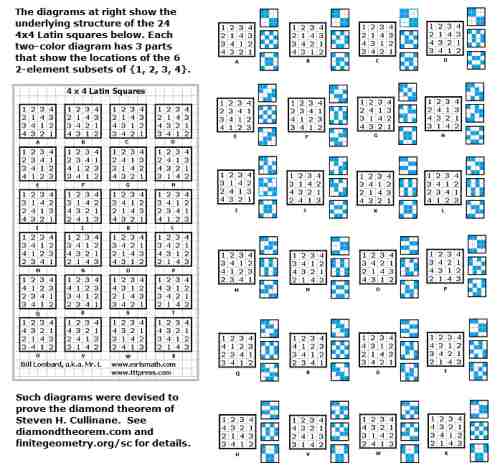
High School Squares*
The following is from the weblog of a high school mathematics teacher—

This is related to the structure of the figure on the cover of the 1976 monograph Diamond Theory—

Each small square pattern on the cover is a Latin square,
with elements that are geometric figures rather than letters or numerals.
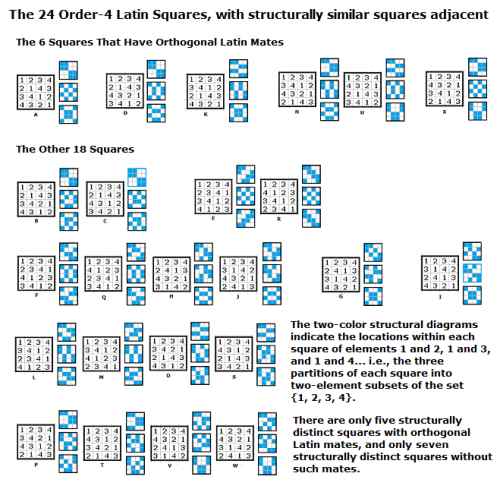
All order-four Latin squares are represented.
For a deeper look at the structure of such squares, let the high-school
chart above be labeled with the letters A through X, and apply the
four-color decomposition theorem. The result is 24 structural diagrams—
Some of the squares are structurally congruent under the group of 8 symmetries of the square.
This can be seen in the following regrouping—
(Image corrected on Jan. 25, 2011– "seven" replaced "eight.")
* Retitled "The Order-4 (i.e., 4×4) Latin Squares" in the copy at finitegeometry.org/sc.
Tuesday, October 19, 2010
Savage Logic…
and the New York Lottery

A search in this journal for yesterday's evening number in the New York Lottery, 359, leads to…
The Cerebral Savage:
On the Work of Claude Lévi-Strauss
by Clifford Geertz
Shown below is 359, the final page of Chapter 13 in
The Interpretation of Cultures: Selected Essays by Clifford Geertz,
New York, 1973: Basic Books, pp. 345-359 —
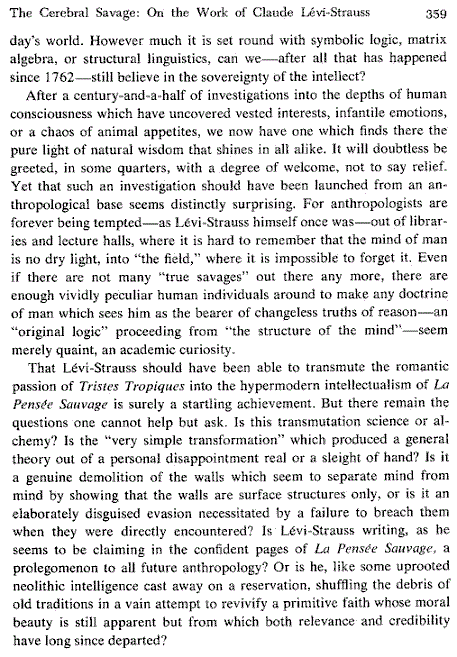
This page number 359 also appears in this journal in an excerpt from Dan Brown's novel Angels & Demons—
See this journal's entries for March 1-15, 2009, especially…
| Sunday, March 15, 2009 5:24 PM
Philosophy and Poetry: The Origin of Change A note on the figure "Two things of opposite natures seem to depend On one another, as a man depends On a woman, day on night, the imagined On the real. This is the origin of change. Winter and spring, cold copulars, embrace And forth the particulars of rapture come." -- Wallace Stevens, "Notes Toward a Supreme Fiction," Canto IV of "It Must Change" Sunday, March 15, 2009 11:00 AM Ides of March Sermon: Angels, Demons,
"Symbology" "On Monday morning, 9 March, after visiting the Mayor of Rome and the Municipal Council on the Capitoline Hill, the Holy Father spoke to the Romans who gathered in the square outside the Senatorial Palace…
'… a verse by Ovid, the great Latin poet, springs to mind. In one of his elegies he encouraged the Romans of his time with these words: "Perfer et obdura: multo graviora tulisti." "Hold out and persist: (Tristia, Liber V, Elegia XI, verse 7).'" This journal
on 9 March: Note the color-interchange Related material: |
The symmetry of the yin-yang symbol, of the diamond-theorem symbol, and of Brown's Illuminati Diamond is also apparent in yesterday's midday New York lottery number (see above).
"Savage logic works like a kaleidoscope…." — Clifford Geertz on Lévi-Strauss
Tuesday, June 15, 2010
Imago, Imago, Imago
Recommended— an online book—
Flight from Eden: The Origins of Modern Literary Criticism and Theory,
by Steven Cassedy, U. of California Press, 1990.
See in particular
Valéry and the Discourse On His Method.
Pages 156-157—
Valéry saw the mind as essentially a relational system whose operation he attempted to describe in the language of group mathematics. "Every act of understanding is based on a group," he says (C, 1:331). "My specialty—reducing everything to the study of a system closed on itself and finite" (C, 19: 645). The transformation model came into play, too. At each moment of mental life the mind is like a group, or relational system, but since mental life is continuous over time, one "group" undergoes a "transformation" and becomes a different group in the next moment. If the mind is constantly being transformed, how do we account for the continuity of the self? Simple; by invoking the notion of the invariant. And so we find passages like this one: "The S[elf] is invariant, origin, locus or field, it's a functional property of consciousness" (C, 15:170 [2: 315]). Just as in transformational geometry, something remains fixed in all the projective transformations of the mind's momentary systems, and that something is the Self (le Moi, or just M, as Valéry notates it so that it will look like an algebraic variable). Transformation theory is all over the place. "Mathematical science . . . reduced to algebra, that is, to the analysis of the transformations of a purely differential being made up of homogeneous elements, is the most faithful document of the properties of grouping, disjunction, and variation in the mind" (O, 1:36). "Psychology is a theory of transformations, we just need to isolate the invariants and the groups" (C, 1:915). "Man is a system that transforms itself" (C, 2:896).
O Paul Valéry, Oeuvres (Paris: Pléiade, 1957-60)
C Valéry, Cahiers, 29 vols. (Paris: Centre National de le Recherche Scientifique, 1957-61)
Compare Jung's image in Aion of the Self as a four-diamond figure:

and Cullinane's purely geometric four-diamond figure:

For a natural group of 322,560 transformations acting on the latter figure, see the diamond theorem.
What remains fixed (globally, not pointwise) under these transformations is the system of points and hyperplanes from the diamond theorem. This system was depicted by artist Josefine Lyche in her installation "Theme and Variations" in Oslo in 2009. Lyche titled this part of her installation "The Smallest Perfect Universe," a phrase used earlier by Burkard Polster to describe the projective 3-space PG(3,2) that contains these points (at right below) and hyperplanes (at left below).
Although the system of points (at right above) and hyperplanes (at left above) exemplifies Valéry's notion of invariant, it seems unlikely to be the sort of thing he had in mind as an image of the Self.
Sunday, March 29, 2009
Sunday March 29, 2009
Getting All
the Meaning In
Webpage heading for the
2009 meeting of the
American Comparative
Literature Association:
The mysterious symbols on
the above map suggest the
following reflections:
From A Cure of the Mind: The Poetics of Wallace Stevens, by Theodore Sampson, published by Black Rose Books Ltd., 2000–
Page x:
"… if what he calls 'the spirit's alchemicana' (CP [Collected Poems] 471) addresses itself to the irrational element in poetry, to what extent is such an element dominant in his theory and practice of poetry, and therefore in what way is Stevens' intricate verbal music dependent on his irrational use of language– a 'pure rhetoric of a language without words?' (CP 374)?"
| From "'When Novelists Become Cubists:' The Prose Ideograms of Guy Davenport," by Andre Furlani:
Laurence Zachar argues that Davenport's writing is situated "aux frontieres intergeneriques" where manifold modes are brought into concord: "L'etonnant chez Davenport est la facon don't ce materiau qui parait l'incarnation meme du chaos– hermetique, enigmatique, obscur, avec son tropplein de references– se revele en fait etre construit, ordonne, structure. Plus l'on s'y plonge, et plus l'on distingue de cohesion dans le texte." 'What astonishes in Davenport is the way in which material that seems the very incarnation of chaos– hermetic, enigmatic, obscure, with its proliferation of allusions– in fact reveals itself to be constructed, organized, structured. The more one immerses oneself in them the more one discerns the texts' cohesion.' (62). Davenport also works along the intergeneric border between text and graphic, for he illustrates many of his texts. (1) "The prime use of words is for imagery: my writing is drawing," he states in an interview (Hoeppfner 123). Visual imagery is not subordinated to writing in Davenport, who draws on the assemblage practice of superimposing image and writing. "I trust the image; my business is to get it onto the page," he writes in the essay "Ernst Machs Max Ernst." "A page, which I think of as a picture, is essentially a texture of images. […] The text of a story is therefore a continuous graph, kin to the imagist poem, to a collage (Ernst, Willi Baumeister, El Lissitzky), a page of Pound, a Brakhage film" (Geography 374-75). Note: (1.) Davenport is an illustrator of books (such as Hugh Kenner's The Stoic Comedians and The Counterfeiters) and journals (such as The Kenyon Review, Parnassus, and Paideuma). His art is the subject of Erik Anderson Reece's monograph, A Balance of Quinces, which reveals the inseparable relationship between Davenport's literary and pictorial work. References: Davenport, Guy. The Geography of the Imagination. San Francisco: North Point Press, 1981. Rpt. New York: Pantheon, 1992. Hoepffner, Bernard. "Pleasant Hill: An Interview with Guy Davenport." Conjunctions 24 (1995): 118-24. Reece, Erik Anderson. A Balance of Quinces: The Paintings and Drawings of Guy Davenport. New York: New Directions, 1996. Zachar, Laurence. "Guy Davenport: Une Mosaique du genres." Recherches Anglaises et Nord-Americaines 21 (1994): 51-63. |
"… when novelists become Cubists; that is, when they see the possibilities of making a hieroglyph, a coherent symbol, an ideogram of the total work. A symbol comes into being when an artist sees that it is the only way to get all the meaning in."
— Guy Davenport, The Geography of the Imagination
Sunday, March 15, 2009
Sunday March 15, 2009
The Origin of Change
A note on the figure
from this morning's sermon:
"Two things of opposite natures seem to depend On one another, as a man depends On a woman, day on night, the imagined On the real. This is the origin of change. Winter and spring, cold copulars, embrace And forth the particulars of rapture come." -- Wallace Stevens, "Notes Toward a Supreme Fiction," Canto IV of "It Must Change"
Sunday March 15, 2009
Angels, Demons,
"Symbology"
"On Monday morning, 9 March, after visiting the Mayor of Rome and the Municipal Council on the Capitoline Hill, the Holy Father spoke to the Romans who gathered in the square outside the Senatorial Palace…
'… a verse by Ovid, the great Latin poet, springs to mind. In one of his elegies he encouraged the Romans of his time with these words:
"Perfer et obdura: multo graviora tulisti."
"Hold out and persist:
you have got through
far more difficult situations."
(Tristia, Liber V, Elegia XI, verse 7).'"
on 9 March:
Note the color-interchange
symmetry of each symbol
under 180-degree rotation.
Related material:
The Illuminati Diamond:


Tom Hanks as Robert Langdon
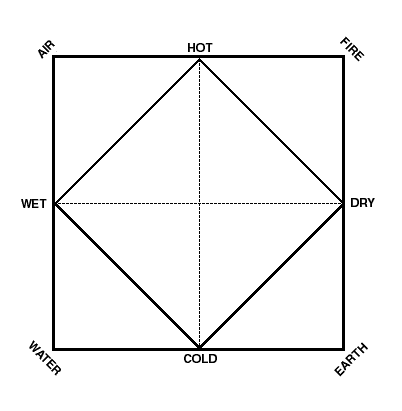
A possible source for Brown's term "symbology" is a 1995 web page, "The Rotation of the Elements," by one "John Opsopaus." (Cf. Art History Club.)
"The four qualities are the key to understanding the rotation of the elements and many other applications of the symbology of the four elements." –John Opsopaus
* "…ambigrams were common in symbology…." —Angels & Demons
Monday, March 9, 2009
Monday March 9, 2009
Humorism

"Always with a
little humor."
— Dr. Yen Lo

From Temperament: A Brief Survey
For other interpretations
of the above shape, see
The Illuminati Diamond.
from Jung's Aion:
As for rotation, see the ambigrams in Dan Brown's Angels & Demons (to appear as a film May 15) and the following figures:

Click on image
for a related puzzle.
For a solution, see
The Diamond Theorem.
A related note on
"Angels & Demons"
director Ron Howard:
Monday, March 2, 2009
Monday March 2, 2009
Joyce's Nightmare
continues
Today in History – March 2
|

|
From Gravity's Rainbow (Penguin Classics, 1995), page 563:
"He brings out the mandala he found.
Slothrop gives him the mandala. He hopes it will work like the mantra that Enzian told him once, mba-kayere (I am passed over), mba-kayere… a spell […]. A mezuzah. Safe passage through a bad night…."
In lieu of Slothrop's mandala, here is another…
Christ and the Four Elements
This 1495 image is found in
For further details,
click on any of the three mandalas above. |

Happy birthday to
Tom Wolfe, author of
The Painted Word.
Friday, May 25, 2007
Friday May 25, 2007
From Log24 on
this date last year:
"May there be an ennui
of the first idea?
What else,
prodigious scholar,
should there be?"
— Wallace Stevens,
"Notes Toward a
Supreme Fiction"
The Associated Press,
May 25, 2007–
Thought for Today:
"I hate quotations.
Tell me what you know."
— Ralph Waldo Emerson

This "telling of what
I know" will of course
mean little to those
who, like Emerson,
have refused to learn
through quotations.
For those less obdurate
than Emerson —Harold Bloom
on Wallace Stevens
and Paul Valery's
"Dance and the Soul"–
"Stevens may be playful, yet seriously so, in describing desire, at winter's end, observing not only the emergence of the blue woman of early spring, but seeing also the myosotis, whose other name is 'forget-me-not.' Desire, hearing the calendar hymn, repudiates the negativity of the mind of winter, unable to bear what Valery's Eryximachus had called 'this cold, exact, reasonable, and moderate consideration of human life as it is.' The final form of this realization in Stevens comes in 1950, in The Course of a Particular, in the great monosyllabic line 'One feels the life of that which gives life as it is.' But even Stevens cannot bear that feeling for long. As Eryximachus goes on to say in Dance and the Soul:
A cold and perfect clarity is a poison impossible to combat. The real, in its pure state, stops the heart instantaneously….[…] To a handful of ashes is the past reduced, and the future to a tiny icicle. The soul appears to itself as an empty and measurable form. –Here, then, things as they are come together, limit one another, and are thus chained together in the most rigorous and mortal* fashion….
O Socrates, the universe cannot for one instant endure to be only what it is.
Valery's formula for reimagining the First Idea is, 'The idea introduces into what is, the leaven of what is not.' This 'murderous lucidity' can be cured only by what Valery's Socrates calls 'the intoxication due to act,' particularly Nietzschean or Dionysiac dance, for this will rescue us from the state of the Snow Man, 'the motionless and lucid observer.'" —Wallace Stevens: The Poems of Our Climate
* "la sorte… la plus mortelle":
mortal in the sense
"deadly, lethal"
Other quotations
(from March 28,
the birthday of
Reba McEntire):
Logical Songs

Logical Song I
(Supertramp)
"When I was young, it seemed that
Life was so wonderful, a miracle,
Oh it was beautiful, magical
And all the birds in the trees,
Well they'd be singing so happily,
Joyfully, playfully watching me"
Logical Song II
(Sinatra)
"You make me feel so young,
You make me feel like
Spring has sprung
And every time I see you grin
I'm such a happy in-
dividual….
You and I are
Just like a couple of tots
Running across the meadow
Picking up lots
Of forget-me-nots"
Tuesday, August 10, 2004
Tuesday August 10, 2004
Battle of Gods and Giants
In checking the quotations from Dante in the previous entry, I came across the intriguing site Gigantomachia:
"A gigantomachia or primordial battle between the gods has been retold in myth, cult, art and theory for thousands of years, from the Egyptians to Heidegger. This site will present the history of the theme. But it will do so in an attempt to raise the question of the contemporary relevance of it. Does the gigantomachia take place today? Where? When? In what relation to you and me?"
Perhaps atop the Empire State Building?
(See An Affair to Remember and Empire State Building to Honor Fay Wray.)
Perhaps in relation to what the late poet Donald Justice called "the wood within"?
Perhaps in relation to T. S. Eliot's "The Waste Land" and the Feast of the Metamorphosis?
Or perhaps not.
Perhaps at Pergamon:
Perhaps at Pergamon Press:
"What modern painters are trying to do,
if they only knew it, is paint invariants."
— James J. Gibson in Leonardo
(Vol. 11, pp. 227-235.
Pergamon Press Ltd., 1978)
An example of invariant structure:

The three line diagrams above result from the three partitions, into pairs of 2-element sets, of the 4-element set from which the entries of the bottom colored figure are drawn. Taken as a set, these three line diagrams describe the structure of the bottom colored figure. After coordinatizing the figure in a suitable manner, we find that this set of three line diagrams is invariant under the group of 16 binary translations acting on the colored figure.
A more remarkable invariance — that of symmetry
This sort of mathematics illustrates the invisible "form" or "idea" behind the visible two-color pattern. Hence it exemplifies, in a way, the conflict described by Plato between those who say that "real existence belongs only to that which can be handled" and those who say that "true reality consists in certain intelligible and bodiless forms."
For further details, see a section on Plato in the Gigantomachia site.
Monday, April 5, 2004
Monday April 5, 2004
Ideas and Art

— Motto of
Plato's Academy
|
From Minimalist Fantasies,
All I want anyone to get out of my paintings, and all I ever get out of them, is the fact that you can see the whole idea without any confusion. … What you see is what you see.
Minimal Art remains too much a feat of ideation, and not enough anything else. Its idea remains an idea, something deduced instead of felt and discovered.
The artists even questioned whether art needed to be a tangible object. Minimalism … Conceptualism — suddenly art could be nothing more than an idea, a thought on a piece of paper….
There was a period, a decade or two ago, when you could hardly open an art journal without encountering the quotation from Frank Stella I used as an epigraph. The bit about “what you see is what you see” was reproduced ad nauseam. It was thought by some to be very deep. In fact, Stella’s remarks—from a joint interview with him and Donald Judd—serve chiefly to underscore the artistic emptiness of the whole project of minimalism. No one can argue with the proposition that “what you see is what you see,” but there’s a lot to argue with in what he calls “the fact that you can see the whole idea without any confusion.” We do not, of course, see ideas. Stella’s assertion to the contrary might be an instance of verbal carelessness, but it is not merely verbal carelessness. At the center of minimalism, as Clement Greenberg noted, is the triumph of ideation over feeling and perception, over aesthetics. |
|
From How Not Much Is a Whole World, Decades on, it's curious how much Minimalism, the last great high modern movement, still troubles people who just can't see why … a plain white canvas with a line painted across it
should be considered art. That line might as well be in the sand: on this side is art, it implies. Go ahead. Cross it. …. The tug of an art that unapologetically sees itself as on a par with science and religion is not to be underestimated, either. Philosophical ambition and formal modesty still constitute Minimalism's bottom line. If what results can sometimes be more fodder for the brain than exciting to look at, it can also have a serene and exalted eloquence…. That line in the sand doesn't separate good art from bad, or art from nonart, but a wide world from an even wider one. |
I maintain that of course
we can see ideas.
Example: the idea of
invariant structure.

"What modern painters
are trying to do,
if they only knew it,
is paint invariants."
— James J. Gibson, Leonardo,
Vol. 11, pp. 227-235.
Pergamon Press Ltd., 1978
For a discussion
of how this works, see
Block Designs,
4×4 Geometry, and
Diamond Theory.
Incidentally, structures like the one shown above are invariant under an important subgroup of the affine group AGL(4,2)… That is to say, they are not lost in translation. (See previous entry.)
Tuesday, December 3, 2002
Tuesday December 3, 2002
Symmetry, Invariance, and Objectivity
The book Invariances: The Structure of the Objective World, by Harvard philosopher Robert Nozick, was reviewed in the New York Review of Books issue dated June 27, 2002.
On page 76 of this book, published by Harvard University Press in 2001, Nozick writes:
"An objective fact is invariant under various transformations. It is this invariance that constitutes something as an objective truth…."
Compare this with Hermann Weyl's definition in his classic Symmetry (Princeton University Press, 1952, page 132):
"Objectivity means invariance with respect to the group of automorphisms."
It has finally been pointed out in the Review, by a professor at Göttingen, that Nozick's book should have included Weyl's definition.
I pointed this out on June 10, 2002.
For a survey of material on this topic, see this Google search on "nozick invariances weyl" (without the quotes).
Nozick's omitting Weyl's definition amounts to blatant plagiarism of an idea.
Of course, including Weyl's definition would have required Nozick to discuss seriously the concept of groups of automorphisms. Such a discussion would not have been compatible with the current level of philosophical discussion at Harvard, which apparently seldom rises above the level of cocktail-party chatter.
A similarly low level of discourse is found in the essay "Geometrical Creatures," by Jim Holt, also in the issue of the New York Review of Books dated December 19, 2002. Holt at least writes well, and includes (if only in parentheses) a remark that is highly relevant to the Nozick-vs.-Weyl discussion of invariance elsewhere in the Review:
"All the geometries ever imagined turn out to be variations on a single theme: how certain properties of a space remain unchanged when its points get rearranged." (p. 69)
This is perhaps suitable for intelligent but ignorant adolescents; even they, however, should be given some historical background. Holt is talking here about the Erlangen program of Felix Christian Klein, and should say so. For a more sophisticated and nuanced discussion, see this web page on Klein's Erlangen Program, apparently by Jean-Pierre Marquis, Département de Philosophie, Université de Montréal. For more by Marquis, see my later entry for today, "From the Erlangen Program to Category Theory."
Saturday, July 20, 2002
Saturday July 20, 2002
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.