
Illustration from other posts now tagged
Cervantes Meets Pascal.
See as well Verhexung in this journal.
This post was suggested by . . .

Illustration from other posts now tagged
Cervantes Meets Pascal.
See as well Verhexung in this journal.
This post was suggested by . . .
Last midnight's post quoted poet John Hollander
on Cervantes—
"… the Don’s view of the world is correct at midnight,
and Sancho’s at noon."
The post concluded with a figure that might, if
rotated slightly, be regarded as a sort of Star of
David or Solomon's Seal. The figure's six vertices
may be viewed as an illustration of Pascal's
"mystic hexagram."

Pacal's hexagram is usually described
as a hexagon inscribed in a conic
(such as a circle). Clearly the hexagon
above may be so inscribed.
The figure suggests that last midnight's Don be
played by the nineteenth-century mathematician
James Joseph Sylvester. His 1854 remarks on
the nature of geometry describe a different approach
to the Pascal hexagram—
| "… the celebrated theorem of Pascal known under the name of the Mystic Hexagram, which is, that if you take two straight lines in a plane, and draw at random other straight lines traversing in a zigzag fashion between them, from A in the first to B in the second, from B in the second to C in the first, from C in the first to D in the second, from D in the second to E in the first, from E in the first to F in the second and finally from F in the second back again to A the starting point in the first, so as to obtain ABCDEF a twisted hexagon, or sort of cat's-cradle figure and if you arrange the six lines so drawn symmetrically in three couples: viz. the 1st and 4th in one couple, the 2nd and 5th in a second couple, the 3rd and 6th in a third couple; then (no matter how the points ACE have been selected upon one of the given lines, and BDF upon the other) the three points through which these three couples of lines respectively pass, or to which they converge (as the case may be) will lie all in one and the same straight line." |
For a Sancho view of Sylvester's "cat's cradle," see some twentieth-century
remarks on "the most important configuration of all geometry"—
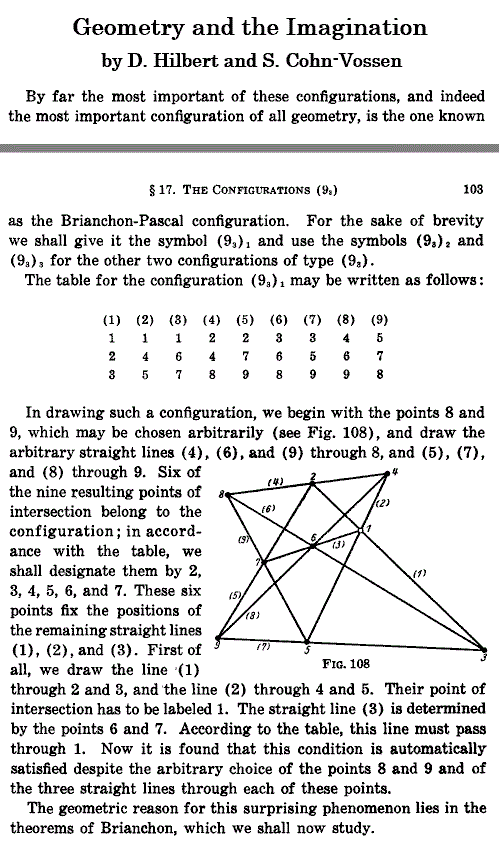
"Now look, your grace," said Sancho,
"what you see over there aren't giants,
but windmills, and what seems to be arms
are just their sails, that go around in the wind
and turn the millstone."
"Obviously," replied Don Quijote,
"you don't know much about adventures.”
From a 2003 interview by Paul Devlin (PD) with poet John Hollander (JH),
who reportedly died Saturday—
|
PD: You wrote in the introduction to the new edition of Reflections on Espionage that whenever you have been "free of political callowness" it was partly as a result of reading W.H. Auden, George Orwell, and George Bernard Shaw. Do you think these writers might possibly be an antidote to political callowness that exists in much contemporary literary criticism? JH: If not they, then some other writers who can help one develop within one a skepticism strongly intertwined with passion, so that each can simultaneously check and reinforce the other. It provides great protection from being overcome by blind, true-believing zeal and corrupting cynicism (which may be two sides of the same false coin). Shaw was a great teacher for many in my generation. I started reading him when I was in sixth grade, and I responded strongly not only to the wit but to various modes, scene and occasions of argument and debate as they were framed by various kinds of dramatic situation. I remember being electrified when quite young by the moment in the epilogue scene of Saint Joan when the English chaplain, De Stogumber, who had been so zealous in urging for Joan’s being burned at the stake, returns to testify about how seeing her suffering the flames had made a changed man of him. The Inquisitor, Peter Cauchon, calls out (with what I imagined was a kind of moral distaste I’d never been aware of before), "Must then a Christ perish in torment in every age to save those who have no imagination?" It introduced me to a skepticism about the self-satisfaction of the born-again, of any persuasion. With Auden and Orwell, much later on and after my mental world had become more complicated, it was education in negotiating a living way between a destructively naïve idealism and the crackpot realism—equally inimical to the pragmatic. PD: Would you consider yourself a "formal" pragmatist, i.e., a student of Peirce, James, Dewey, Mead (etc.) or an "informal" pragmatist – someone taking the common-sense position on events…or someone who refuses to be pigeon-holed politically? JH: "Informal" – of the sort that often leads me to ask of theoretical formulations, "Yes, but what’s it for ?" PD: Which other authors do you think might help us negotiate between "naïve idealism" and "crackpot realism"? I think of Joyce, Wallace Stevens, perhaps Faulkner? JH: When I was in college, a strong teacher for just this question was Cervantes. One feels, in an Emersonian way, that the Don’s view of the world is correct at midnight, and Sancho’s at noon. |
Then there is mathematical realism.
A post in this journal on Saturday, the reported date of Hollander's death,
discussed a possible 21st-century application of 19th-century geometry.
For some background, see Peter J. Cameron's May 11, 2010, remarks
on Sylvester's duads and synthemes . The following figure from the
paper discussed here Saturday is related to figures in Cameron's remarks.

The following excerpt from a January 20, 2013, preprint shows that
a Galois-geometry version of the large Desargues 154203 configuration,
although based on the nineteenth-century work of Galois* and of Fano,**
may at times have twenty-first-century applications.

Atkinson's paper does not use the square model of PG(3,2), which later
in 2013 provided a natural view of the large Desargues 154203 configuration.
See my own Classical Geometry in Light of Galois Geometry. Atkinson's
"subset of 20 lines" corresponds to 20 of the 80 Rosenhain tetrads
mentioned in that later article and pictured within 4×4 squares in Hudson's
1905 classic Kummer's Quartic Surface.
* E. Galois, definition of finite fields in "Sur la Théorie des Nombres,"
Bulletin des Sciences Mathématiques de M. Férussac,
Vol. 13, 1830, pp. 428-435.
** G. Fano, definition of PG(3,2) in "Sui Postulati Fondamentali…,"
Giornale di Matematiche, Vol. 30, 1892, pp. 106-132.
Powered by WordPress