Friday, May 31, 2019
Bulk Apperception
Comments Off on Bulk Apperception
Tuesday, April 23, 2019
Critical Invisibility
From Gotay and Isenberg, "The Symplectization of Science,"
Gazette des Mathématiciens 54, 59-79 (1992):
"… what is the origin of the unusual name 'symplectic'? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure 'line complex group' the 'symplectic group.'
… the adjective 'symplectic' means 'plaited together' or 'woven.'
This is wonderfully apt…."
On "The Emperor's New Clothes" —
|
Andersen’s weavers, as one commentator points out, are merely insisting that “the value of their labor be recognized apart from its material embodiment.” The invisible cloth they weave may never manifest itself in material terms, but the description of its beauty (“as light as spiderwebs” and “exquisite”) turns it into one of the many wondrous objects found in Andersen’s fairy tales. It is that cloth that captivates us, making us do the imaginative work of seeing something beautiful even when it has no material reality. Deeply resonant with meaning and of rare aesthetic beauty—even if they never become real—the cloth and other wondrous objets d’art have attained a certain degree of critical invisibility. — Maria Tatar, The Annotated Hans Christian Andersen (W. W. Norton & Company, 2007). Kindle Edition. |
Comments Off on Critical Invisibility
A Certain Dramatic Artfulness
Comments Off on A Certain Dramatic Artfulness
Thursday, March 28, 2019
Culture
The previous post, "Dream of Plenitude," suggests . . .
"So here's to you, Nordstrom-Robinson . . . ."
Comments Off on Culture
Tuesday, March 12, 2019
Wednesday, March 6, 2019
The Relativity Problem and Burkard Polster
|
From some 1949 remarks of Weyl— "The relativity problem is one of central significance throughout geometry and algebra and has been recognized as such by the mathematicians at an early time." — Hermann Weyl, "Relativity Theory as a Stimulus in Mathematical Research," Proceedings of the American Philosophical Society , Vol. 93, No. 7, Theory of Relativity in Contemporary Science: Papers Read at the Celebration of the Seventieth Birthday of Professor Albert Einstein in Princeton, March 19, 1949 (Dec. 30, 1949), pp. 535-541 Weyl in 1946—: "This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them." — Hermann Weyl, The Classical Groups , Princeton University Press, 1946, p. 16 |
For some context, see Relativity Problem in this journal.
In the case of PG(3,2), there is a choice of geometric models
to be coordinatized: two such models are the traditional
tetrahedral model long promoted by Burkard Polster, and
the square model of Steven H. Cullinane.
The above Wikipedia section tacitly (and unfairly) assumes that
the model being coordinatized is the tetrahedral model. For
coordinatization of the square model, see (for instance) the webpage
Finite Relativity.
For comparison of the two models, see a figure posted here on
May 21, 2014 —
Labeling the Tetrahedral Model (Click to enlarge) —
"Citation needed" —
The anonymous characters who often update the PG(3,2) Wikipedia article
probably would not consider my post of 2014, titled "The Tetrahedral
Model of PG(3,2)," a "reliable source."
Comments Off on The Relativity Problem and Burkard Polster
Tuesday, March 5, 2019
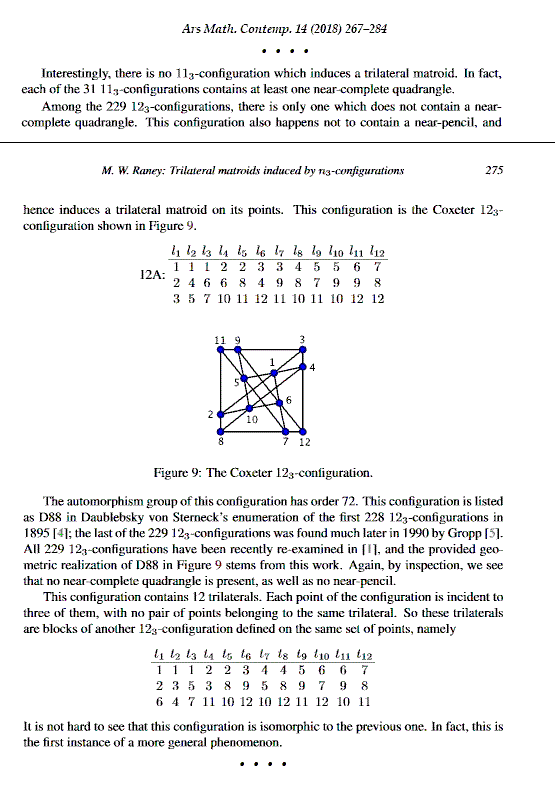
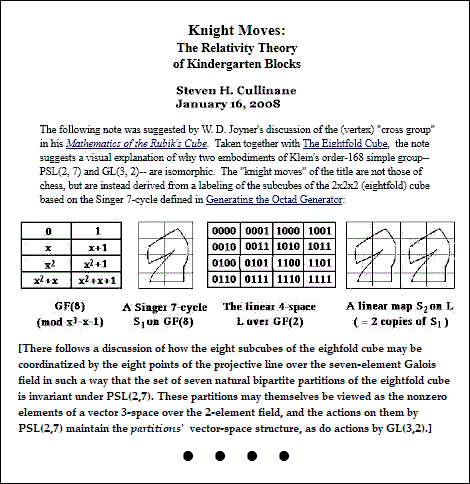
The Eightfold Cube and PSL(2,7)
For PSL(2,7), this is ((49-1)(49-7))/((7-1)(2))=168.
The group GL(3,2), also of order 168, acts naturally
on the set of seven cube-slicings below —
Another way to picture the seven natural slicings —
Application of the above images to picturing the
isomorphism of PSL(2,7) with GL(3,2) —
.gif)
For a more detailed proof, see . . .
Comments Off on The Eightfold Cube and PSL(2,7)
Wednesday, February 27, 2019
Construction of PG(3,2) from K6
From this journal on April 23, 2013 —
From this journal in 2003 —
From Wikipedia on Groundhog Day, 2019 —
Comments Off on Construction of PG(3,2) from K6
Monday, February 25, 2019
Sunday, February 24, 2019
Monday, February 18, 2019
The Joy of Six
__________________________________________________________________________
See also the previous post.
I prefer the work of Josefine Lyche on the smallest perfect number/universe.
Context —
Lyche's Lynx760 installations and Vigeland's nearby Norwegian clusterfuck.
Comments Off on The Joy of Six
Sacerdotal K6, Continued
Comments Off on Sacerdotal K6, Continued
Sunday, February 17, 2019
Sacerdotal K6
Comments Off on Sacerdotal K6
Wednesday, February 13, 2019
April 18, 2003 (Good Friday), Continued
"The purpose of mathematics cannot be derived from an activity
inferior to it but from a higher sphere of human activity, namely,
religion."
— Igor Shafarevitch, 1973 remark published as above in 1982.
"Perhaps."
— Steven H. Cullinane, February 13, 2019
|
From Log24 on Good Friday, April 18, 2003 — . . . What, indeed, is truth? I doubt that the best answer can be learned from either the Communist sympathizers of MIT or the “Red Mass” leftists of Georgetown. For a better starting point than either of these institutions, see my note of April 6, 2001, Wag the Dogma. See, too, In Principio Erat Verbum , which notes that “numbers go to heaven who know no more of God on earth than, as it were, of sun in forest gloom.” Since today is the anniversary of the death of MIT mathematics professor Gian-Carlo Rota, an example of “sun in forest gloom” seems the best answer to Pilate’s question on this holy day. See
“Examples are the stained glass windows Motto of Plato’s Academy † The Exorcist, 1973 |
Detail from an image linked to in the above footnote —
"And the darkness comprehended it not."
Id est :
A Good Friday, 2003, article by
a student of Shafarevitch —
"… there are 25 planes in W . . . . Of course,
replacing {a,b,c} by the complementary set
does not change the plane. . . ."
Of course.
See. however, Six-Set Geometry in this journal.
Comments Off on April 18, 2003 (Good Friday), Continued
Saturday, January 26, 2019
In Memory of a Composer
who reportedly died early today in Paris, a tribute from
those who wrote the English lyrics for "Windmills of Your Mind" —
Comments Off on In Memory of a Composer
Installasjon
The above cryptic search result indicates that there may
soon be a new Norwegian art installation based on this page
of Eddington (via Log24) —
See also other posts tagged Kummerhenge.
Comments Off on Installasjon
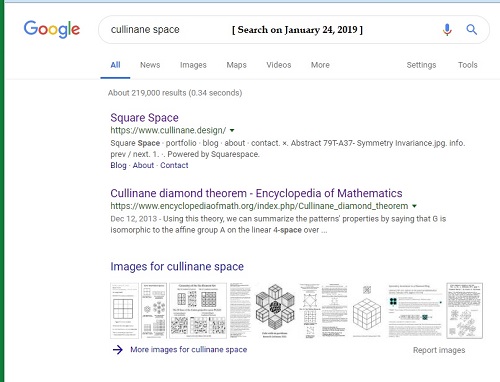
Thursday, January 24, 2019
Name Space
A correction at Wikipedia (Click to enlarge.) —
That this correction is needed indicates that the phrase
"Cullinane space" might be useful. (Click to enlarge.)
Comments Off on Name Space
Wednesday, January 23, 2019
Decorated
For those who prefer more elaborate decorations —
1. A Facebook image from last August …
2. The Facebook glider suggests a tune from "The Thomas Crown Affair"
(1968) that appeared in a Dec. 16, 2018 post on Christianity and
"interlocking names"—
The revised lyrics describe a square space.
3. An even more elaborate square space:
the Dance of the Snowflakes from
Balanchine's version of The Nutcracker —
Comments Off on Decorated
Thursday, January 10, 2019
Archimedes at Hiroshima
Two examples from the Wikipedia article "Archimedean solid" —
Iain Aitchison said in a talk last year at Hiroshima that
the Mathieu group M24 can be represented as permuting
naturally the 24 edges of the cuboctahedron.
The 24 vertices of the truncated octahedron are labeled
naturally by the 24 elements of S4 in a permutahedron —
Can M24 be represented as permuting naturally
the 24 vertices of the truncated octahedron?
Comments Off on Archimedes at Hiroshima
Sunday, January 6, 2019
For Broom Bridge*
GL(2,3) is not unrelated to GL(3,2).
See Quaternion Automorphisms
and Spinning in Infinity.
* See Wikipedia.
Comments Off on For Broom Bridge*
Wednesday, January 2, 2019
Wolf as Lamb
The above graphic design is by Noma Bar.
See as well the lamb-in-triangle of the Dec. 27 post
A Candle for Lily —
Related material —
Remarks by Evelyn Lamb on the Deathly Hallows symbol.
Comments Off on Wolf as Lamb
Saturday, December 22, 2018
Cremona-Richmond
The following are some notes on the history of Clifford algebras
and finite geometry suggested by the "Clifford Modules" link in a
Log24 post of March 12, 2005 —
A more recent appearance of the configuration —
Comments Off on Cremona-Richmond
Sunday, December 16, 2018
Friday, December 14, 2018
Small Space Odyssey
References in recent posts to physical space and
to mathematical space suggest a comparison.
Physical space is well known, at least in the world
of mass entertainment.
Mathematical space, such as the 12-dimensional
finite space of the Golay code, is less well known.
A figure from each space —
The source of the Conway-Sloane brick —
Quote from a mathematics writer —
“Looking carefully at Golay’s code is like staring into the sun.”
The former practice yields reflections like those of Conway and Sloane.
The latter practice is not recommended.
Comments Off on Small Space Odyssey
Wednesday, December 12, 2018
Kummerhenge Continues.
Those pleased by what Ross Douthat today called
"The Return of Paganism" are free to devise rituals
involving what might be called "the sacred geometry
of the Kummer 166 configuration."
As noted previously in this journal,
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
See also earlier posts also tagged "Kummerhenge" and
another property of the remarkable Kummer 166 —
For some related literary remarks, see "Transposed" in this journal.
Some background from 2001 —
Comments Off on Kummerhenge Continues.
An Inscape for Douthat
Some images, and a definition, suggested by my remarks here last night
on Apollo and Ross Douthat's remarks today on "The Return of Paganism" —
In finite geometry and combinatorics,
an inscape is a 4×4 array of square figures,
each figure picturing a subset of the overall 4×4 array:
Related material — the phrase
"Quantum Tesseract Theorem" and …
A. An image from the recent
film "A Wrinkle in Time" —
B. A quote from the 1962 book —
"There's something phoney
in the whole setup, Meg thought.
There is definitely something rotten
in the state of Camazotz."
Comments Off on An Inscape for Douthat
Sunday, December 9, 2018
Quaternions in a Small Space
The previous post, on the 3×3 square in ancient China,
suggests a review of group actions on that square
that include the quaternion group.
Click to enlarge —
Three links from the above finitegeometry.org webpage on the
quaternion group —
-
Visualizing GL(2,p) — A 1985 note illustrating group actions
on the 3×3 (ninefold) square. -
Another 1985 note showing group actions on the 3×3 square
transferred to the 2x2x2 (eightfold) cube. - Quaternions in an Affine Galois Plane — A webpage from 2010.
Related material —
See as well the two Log24 posts of December 1st, 2018 —
Character and In Memoriam.
Comments Off on Quaternions in a Small Space
Friday, December 7, 2018
The Angel Particle
(Continued from this morning)
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
See also other Log24 posts tagged Kummerhenge.
Comments Off on The Angel Particle
Tuesday, December 4, 2018
Melbourne Noir
Comments Off on Melbourne Noir
Monday, December 3, 2018
The Relativity Problem at Hiroshima
“This is the relativity problem: to fix objectively a class of
equivalent coordinatizations and to ascertain the group of
transformations S mediating between them.”
— Hermann Weyl, The Classical Groups ,
Princeton University Press, 1946, p. 16
See also Relativity Problem and Diamonds and Whirls.
Comments Off on The Relativity Problem at Hiroshima
Sunday, December 2, 2018
Symmetric Generation …
Comments Off on Symmetric Generation …
Symmetry at Hiroshima
A search this morning for articles mentioning the Miracle Octad Generator
of R. T. Curtis within the last year yielded an abstract for two talks given
at Hiroshima on March 8 and 9, 2018 —
|
http://www.math.sci.hiroshima-u.ac.jp/ branched/files/2018/abstract/Aitchison.txt
Iain AITCHISON Title: Construction of highly symmetric Riemann surfaces, related manifolds, and some exceptional objects, I, II Abstract: Since antiquity, some mathematical objects have played a special role, underpinning new mathematics as understanding deepened. Perhaps archetypal are the Platonic polyhedra, subsequently related to Platonic idealism, and the contentious notion of existence of mathematical reality independent of human consciousness. Exceptional or unique objects are often associated with symmetry – manifest or hidden. In topology and geometry, we have natural base points for the moduli spaces of closed genus 2 and 3 surfaces (arising from the 2-fold branched cover of the sphere over the 6 vertices of the octahedron, and Klein's quartic curve, respectively), and Bring's genus 4 curve arises in Klein's description of the solution of polynomial equations of degree greater than 4, as well as in the construction of the Horrocks-Mumford bundle. Poincare's homology 3-sphere, and Kummer's surface in real dimension 4 also play special roles. In other areas: we have the exceptional Lie algebras such as E8; the sporadic finite simple groups; the division algebras: Golay's binary and ternary codes; the Steiner triple systems S(5,6,12) and S(5,8,24); the Leech lattice; the outer automorphisms of the symmetric group S6; the triality map in dimension 8; and so on. We also note such as: the 27 lines on a cubic, the 28 bitangents of a quartic curve, the 120 tritangents of a sextic curve, and so on, related to Galois' exceptional finite groups PSL2(p) (for p= 5,7,11), and various other so-called `Arnol'd Trinities'. Motivated originally by the `Eightfold Way' sculpture at MSRI in Berkeley, we discuss inter-relationships between a selection of these objects, illustrating connections arising via highly symmetric Riemann surface patterns. These are constructed starting with a labeled polygon and an involution on its label set. Necessarily, in two lectures, we will neither delve deeply into, nor describe in full, contexts within which exceptional objects arise. We will, however, give sufficient definition and detail to illustrate essential inter-connectedness of those exceptional objects considered. Our starting point will be simplistic, arising from ancient Greek ideas underlying atomism, and Plato's concepts of space. There will be some overlap with a previous talk on this material, but we will illustrate with some different examples, and from a different philosophical perspective. Some new results arising from this work will also be given, such as an alternative graphic-illustrated MOG (Miracle Octad Generator) for the Steiner system S(5,8,24), and an alternative to Singerman – Jones' genus 70 Riemann surface previously proposed as a completion of an Arnol'd Trinity. Our alternative candidate also completes a Trinity whose two other elements are Thurston's highly symmetric 6- and 8-component links, the latter related by Thurston to Klein's quartic curve. |
See also yesterday morning's post, "Character."
Update: For a followup, see the next Log24 post.
Comments Off on Symmetry at Hiroshima
Friday, November 30, 2018
Latin-Square Structure
Continued from March 13, 2011 —
"…as we saw, there are two different Latin squares of order 4…."
— Peter J. Cameron, "The Shrikhande Graph," August 26, 2010
Cameron counts Latin squares as the same if they are isotopic .
Some further context for Cameron's remark—
A new website illustrates a different approach to Latin squares of order 4 —
Comments Off on Latin-Square Structure
Thursday, November 29, 2018
The White Cube
Clicking on Zong in the above post leads to a 2005 article
in the Bulletin of the American Mathematical Society .
See also the eightfold cube and interality .
Comments Off on The White Cube
Wednesday, November 28, 2018
Geometry and Experience
Einstein, "Geometry and Experience," lecture before the
Prussian Academy of Sciences, January 27, 1921–
|
… This view of axioms, advocated by modern axiomatics, purges mathematics of all extraneous elements, and thus dispels the mystic obscurity, which formerly surrounded the basis of mathematics. But such an expurgated exposition of mathematics makes it also evident that mathematics as such cannot predicate anything about objects of our intuition or real objects. In axiomatic geometry the words "point," "straight line," etc., stand only for empty conceptual schemata. That which gives them content is not relevant to mathematics. Yet on the other hand it is certain that mathematics generally, and particularly geometry, owes its existence to the need which was felt of learning something about the behavior of real objects. The very word geometry, which, of course, means earth-measuring, proves this. For earth-measuring has to do with the possibilities of the disposition of certain natural objects with respect to one another, namely, with parts of the earth, measuring-lines, measuring-wands, etc. It is clear that the system of concepts of axiomatic geometry alone cannot make any assertions as to the behavior of real objects of this kind, which we will call practically-rigid bodies. To be able to make such assertions, geometry must be stripped of its merely logical-formal character by the coordination of real objects of experience with the empty conceptual schemata of axiomatic geometry. To accomplish this, we need only add the proposition: solid bodies are related, with respect to their possible dispositions, as are bodies in Euclidean geometry of three dimensions. Then the propositions of Euclid contain affirmations as to the behavior of practically-rigid bodies. Geometry thus completed is evidently a natural science; we may in fact regard it as the most ancient branch of physics. Its affirmations rest essentially on induction from experience, but not on logical inferences only. We will call this completed geometry "practical geometry," and shall distinguish it in what follows from "purely axiomatic geometry." The question whether the practical geometry of the universe is Euclidean or not has a clear meaning, and its answer can only be furnished by experience. …. |
Later in the same lecture, Einstein discusses "the theory of a finite
universe." Of course he is not using "finite" in the sense of the field
of mathematics known as "finite geometry " — geometry with only finitely
many points.
Nevertheless, his remarks seem relevant to the Fano plane , an
axiomatically defined entity from finite geometry, and the eightfold cube ,
a physical object embodying the properties of the Fano plane.
|
I want to show that without any extraordinary difficulty we can illustrate the theory of a finite universe by means of a mental picture to which, with some practice, we shall soon grow accustomed. First of all, an observation of epistemological nature. A geometrical-physical theory as such is incapable of being directly pictured, being merely a system of concepts. But these concepts serve the purpose of bringing a multiplicity of real or imaginary sensory experiences into connection in the mind. To "visualize" a theory therefore means to bring to mind that abundance of sensible experiences for which the theory supplies the schematic arrangement. In the present case we have to ask ourselves how we can represent that behavior of solid bodies with respect to their mutual disposition (contact) that corresponds to the theory of a finite universe. |
Comments Off on Geometry and Experience
Saturday, November 24, 2018
Thursday, November 22, 2018
Rosenhain and Göpel Meet Kummer in Projective 3-Space
For further details, see finitegeometry.org/sc/35/hudson.html.
Comments Off on Rosenhain and Göpel Meet Kummer in Projective 3-Space
Geometric Incarnation
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
Note also the four 4×4 arrays surrounding the central diamond
in the chi of the chi-rho page of the Book of Kells —
From a Log24 post
of March 17, 2012
"Interlocking, interlacing, interweaving"
— Condensed version of page 141 in Eddington's
1939 Philosophy of Physical Science
Comments Off on Geometric Incarnation
Wednesday, November 21, 2018
Opening Credits
From Log24 on Friday, Nov. 16 —
This evening's New York Times on an opening-credits designer,
Pablo Ferro, who reportedly died at 83 on Friday, Nov. 16 —
An example of Ferro's later work in film —
Musical accompaniment from Sunday morning —
Comments Off on Opening Credits
Tuesday, November 20, 2018
Logos
Musical accompaniment from Sunday morning —
Update of Nov. 21 —
The reader may contrast the above Squarespace.com logo
(a rather serpentine version of the acronym SS) with a simpler logo
for a square space (the Galois window ):
Comments Off on Logos
Sunday, November 18, 2018
Space Music
Comments Off on Space Music
Diamond Theorem Symmetry
The title is a useful search phrase:
Comments Off on Diamond Theorem Symmetry
Saturday, November 17, 2018
Representation
See as well . . .
. . . and posts tagged Alperin.
On its current homepage, the American Mathematical Society
links to a Nov. 15 blog post illustrating the Stan Lee approach
to mathematics:
Stories: "Math needs more stories. All kinds of stories…" —
See too Mathematics and Narrative in this journal.
Comments Off on Representation
Friday, November 16, 2018
Thursday, November 15, 2018
Mathematics and Narrative
Mathematics —
See (for instance) a research group at Ghent University.
For those who prefer narrative . . .
See also . . .
Comments Off on Mathematics and Narrative
Wednesday, November 14, 2018
Tuesday, November 13, 2018
Blackboard Jungle Continues.
From the 1955 film "Blackboard Jungle" —
From a trailer for the recent film version of A Wrinkle in Time —
Detail of the phrase "quantum tesseract theorem":
From the 1962 book —
"There's something phoney
in the whole setup, Meg thought.
There is definitely something rotten
in the state of Camazotz."
Related mathematics from Koen Thas that some might call a
"quantum tesseract theorem" —
Some background —
See also posts tagged Dirac and Geometry. For more
background on finite geometry, see a web page
at Thas's institution, Ghent University.
Comments Off on Blackboard Jungle Continues.
Thursday, November 8, 2018
Reality vs. Axiomatic Thinking
From https://blogs.scientificamerican.com/…
|
A Few of My Favorite Spaces:
The intuition-challenging Fano plane may be By Evelyn Lamb on October 24, 2015
"…finite projective planes seem like |
For Fano's axiomatic approach, see the Nov. 3 Log24 post
"Foundations of Geometry."
For the Fano plane's basis in reality , see the eightfold cube
at finitegeometry.org/sc/ and in this journal.
See as well "Two Views of Finite Space" (in this journal on the date
of Lamb's remarks — Oct. 24, 2015).
Some context: Gödel's Platonic realism vs. Hilbert's axiomatics
in remarks by Manuel Alfonseca on June 7, 2018. (See too remarks
in this journal on that date, in posts tagged "Road to Hell.")
Comments Off on Reality vs. Axiomatic Thinking
Geometry Lesson
From "The Trials of Device" (April 24, 2017) —
See also Wittgenstein in a search for "Ein Kampf " in this journal.
Comments Off on Geometry Lesson
Tuesday, November 6, 2018
On Mathematical Beauty
A phrase from the previous post —
"a size-eight dame in a size-six dress" —
suggests a review . . .
See as well the diamond-theorem correlation and . . .
Comments Off on On Mathematical Beauty
Saturday, November 3, 2018
Foundations of Geometry
"costruire (o, dirò meglio immaginare) un ente" — Fano, 1892
"o, dirò meglio, costruire" — Cullinane, 2018
Comments Off on Foundations of Geometry
Thursday, November 1, 2018
Formation, Transformation . . . . Solution, Dissolution
Comments Off on Formation, Transformation . . . . Solution, Dissolution
Tuesday, October 23, 2018
Saturday, October 20, 2018
Study in Blue and Pink
Comments Off on Study in Blue and Pink
Wednesday, October 17, 2018
Breakthrough Prize
"… what once seemed pure abstractions have turned out to
underlie real physical processes."
— https://breakthroughprize.org/Prize/3
Related material from the current New Yorker —
Comments Off on Breakthrough Prize
Aesthetics
|
From "The Phenomenology of Mathematical Beauty," The Lightbulb Mistake . . . . Despite the fact that most proofs are long, and despite our need for extensive background, we think back to instances of appreciating mathematical beauty as if they had been perceived in a moment of bliss, in a sudden flash like a lightbulb suddenly being lit. The effort put into understanding the proof, the background material, the difficulties encountered in unraveling an intricate sequence of inferences fade and magically disappear the moment we become aware of the beauty of a theorem. The painful process of learning fades from memory, and only the flash of insight remains. We would like mathematical beauty to consist of this flash; mathematical beauty should be appreciated with the instantaneousness of a lightbulb being lit. However, it would be an error to pretend that the appreciation of mathematical beauty is what we vaingloriously feel it should be, namely, an instantaneous flash. Yet this very denial of the truth occurs much too frequently. The lightbulb mistake is often taken as a paradigm in teaching mathematics. Forgetful of our learning pains, we demand that our students display a flash of understanding with every argument we present. Worse yet, we mislead our students by trying to convince them that such flashes of understanding are the core of mathematical appreciation. Attempts have been made to string together beautiful mathematical results and to present them in books bearing such attractive titles as The One Hundred Most Beautiful Theorems of Mathematics . Such anthologies are seldom found on a mathematician’s bookshelf. The beauty of a theorem is best observed when the theorem is presented as the crown jewel within the context of a theory. But when mathematical theorems from disparate areas are strung together and presented as “pearls,” they are likely to be appreciated only by those who are already familiar with them. The Concept of Mathematical Beauty The lightbulb mistake is our clue to understanding the hidden sense of mathematical beauty. The stark contrast between the effort required for the appreciation of mathematical beauty and the imaginary view mathematicians cherish of a flashlike perception of beauty is the Leitfaden that leads us to discover what mathematical beauty is. Mathematicians are concerned with the truth. In mathematics, however, there is an ambiguity in the use of the word “truth.” This ambiguity can be observed whenever mathematicians claim that beauty is the raison d’être of mathematics, or that mathematical beauty is what gives mathematics a unique standing among the sciences. These claims are as old as mathematics and lead us to suspect that mathematical truth and mathematical beauty may be related. Mathematical beauty and mathematical truth share one important property. Neither of them admits degrees. Mathematicians are annoyed by the graded truth they observe in other sciences. Mathematicians ask “What is this good for?” when they are puzzled by some mathematical assertion, not because they are unable to follow the proof or the applications. Quite the contrary. Mathematicians have been able to verify its truth in the logical sense of the term, but something is still missing. The mathematician who is baffled and asks “What is this good for?” is missing the sense of the statement that has been verified to be true. Verification alone does not give us a clue as to the role of a statement within the theory; it does not explain the relevance of the statement. In short, the logical truth of a statement does not enlighten us as to the sense of the statement. Enlightenment , not truth, is what the mathematician seeks when asking, “What is this good for?” Enlightenment is a feature of mathematics about which very little has been written. The property of being enlightening is objectively attributed to certain mathematical statements and denied to others. Whether a mathematical statement is enlightening or not may be the subject of discussion among mathematicians. Every teacher of mathematics knows that students will not learn by merely grasping the formal truth of a statement. Students must be given some enlightenment as to the sense of the statement or they will quit. Enlightenment is a quality of mathematical statements that one sometimes gets and sometimes misses, like truth. A mathematical theorem may be enlightening or not, just as it may be true or false. If the statements of mathematics were formally true but in no way enlightening, mathematics would be a curious game played by weird people. Enlightenment is what keeps the mathematical enterprise alive and what gives mathematics a high standing among scientific disciplines. Mathematics seldom explicitly acknowledges the phenomenon of enlightenment for at least two reasons. First, unlike truth, enlightenment is not easily formalized. Second, enlightenment admits degrees: some statements are more enlightening than others. Mathematicians dislike concepts admitting degrees and will go to any length to deny the logical role of any such concept. Mathematical beauty is the expression mathematicians have invented in order to admit obliquely the phenomenon of enlightenment while avoiding acknowledgment of the fuzziness of this phenomenon. They say that a theorem is beautiful when they mean to say that the theorem is enlightening. We acknowledge a theorem’s beauty when we see how the theorem “fits” in its place, how it sheds light around itself, like Lichtung — a clearing in the woods. We say that a proof is beautiful when it gives away the secret of the theorem, when it leads us to perceive the inevitability of the statement being proved. The term “mathematical beauty,” together with the lightbulb mistake, is a trick mathematicians have devised to avoid facing up to the messy phenomenon of enlightenment. The comfortable one-shot idea of mathematical beauty saves us from having to deal with a concept that comes in degrees. Talk of mathematical beauty is a cop-out to avoid confronting enlightenment, a cop-out intended to keep our description of mathematics as close as possible to the description of a mechanism. This cop-out is one step in a cherished activity of mathematicians, that of building a perfect world immune to the messiness of the ordinary world, a world where what we think should be true turns out to be true, a world that is free from the disappointments, ambiguities, and failures of that other world in which we live. |
How many mathematicians does it take to screw in a lightbulb?
Comments Off on Aesthetics
Monday, October 15, 2018
History at Bellevue
The previous post, "Tesserae for a Tesseract," contains the following
passage from a 1987 review of a book about Finnegans Wake —
"Basically, Mr. Bishop sees the text from above
and as a whole — less as a sequential story than
as a box of pied type or tesserae for a mosaic,
materials for a pattern to be made."
A set of 16 of the Wechsler cubes below are tesserae that
may be used to make patterns in the Galois tesseract.
Another Bellevue story —
“History, Stephen said, is a nightmare
from which I am trying to awake.”
— James Joyce, Ulysses
Comments Off on History at Bellevue
For Zingari Shoolerim*
The structure at top right is that of the
ROMA-ORAM-MARO-AMOR square
in the previous post.
* "Zingari shoolerim" is from
Finnegans Wake .
Comments Off on For Zingari Shoolerim*
Friday, October 5, 2018
The “Ignotiles” of Paul Hertz
The above figure illustrating 24 permutations is dated "2007."
An earlier permutations figure, archived on June 17, 2006 —
An illustration of the above title "I Want To Be a Mathematician" —
Wanting does not make it so.
Comments Off on The “Ignotiles” of Paul Hertz
Wednesday, October 3, 2018
Sunday, September 30, 2018
Iconology of the Eightfold Cube
Found today in an Internet image search, from the website of
an anonymous amateur mathematics enthusiast —
Forming Gray codes in the eightfold cube with the eight
I Ching trigrams (bagua ) —
This journal on Nov. 7, 2016 —
A different sort of cube, from the makers of the recent
Netflix miniseries "Maniac" —
See also Rubik in this journal.
Comments Off on Iconology of the Eightfold Cube
Saturday, September 29, 2018
“Ikonologie des Zwischenraums”
The title is from Warburg. The Zwischenraum lines and shaded "cuts"
below are to be added together in characteristic two, i.e., via the
set-theoretic symmetric difference operator.
Comments Off on “Ikonologie des Zwischenraums”
Sunday, September 23, 2018
Three Times Eight
The New York Times 's Sunday School today —
I prefer the three bricks of the Miracle Octad Generator —
Comments Off on Three Times Eight
Saturday, September 22, 2018
Minimalist Configuration
Comments Off on Minimalist Configuration
The Venturi Manifesto
Venturi reportedly died on Tuesday, September 18.*
See also this journal on that date.
* Fact check:
Comments Off on The Venturi Manifesto
Symmetric Generation, by Curtis
Norwegian artist Josefine Lyche —
Lyche's shirt honors the late Kurt Cobain.
"Here we are now, entertain us."
Comments Off on Symmetric Generation, by Curtis
Friday, September 21, 2018
Monday, September 17, 2018
Lying at the Axis
Or: Zero Dark Zero
|
" Lying at the axis of everything, zero is both real and imaginary. Lovelace was fascinated by zero; as was Gottfried Leibniz, for whom, like mathematics itself, it had a spiritual dimension. It was this that let him to imagine the binary numbers that now lie at the heart of computers: 'the creation of all things out of nothing through God's omnipotence, it might be said that nothing is a better analogy to, or even demonstration of such creation than the origin of numbers as here represented, using only unity and zero or nothing.' He also wrote, 'The imaginary number is a fine and wonderful recourse of the divine spirit, almost an amphibian between being and nonbeing.' "
— A footnote from page 229 of Sydney Padua's |
Comments Off on Lying at the Axis
Saturday, September 15, 2018
Axioms
Update of 10:18 AM the same day —
See also Logicomix in this journal and, at Harvard,
http://www.math.harvard.edu/~mazur/ —
-
September 6, 2018: Eric Maskin, Amartya Sen and I
are giving a course this semester: 'Axiomatic Reasoning'
(PHIL 273B). Introduction to Axiomatic Reasoning gives a
general sense of what we intend to cover.
Update of 10:48 AM the same day —
See Log24 on the date of Tieszen's death.
Comments Off on Axioms
Eidetic Reduction in Geometry
|
"Husserl is not the greatest philosopher of all times. — Kurt Gödel as quoted by Gian-Carlo Rota Some results from a Google search — Eidetic reduction | philosophy | Britannica.com Eidetic reduction, in phenomenology, a method by which the philosopher moves from the consciousness of individual and concrete objects to the transempirical realm of pure essences and thus achieves an intuition of the eidos (Greek: “shape”) of a thing—i.e., of what it is in its invariable and essential structure, apart … Phenomenology Online » Eidetic Reduction
The eidetic reduction: eidos. Method: Bracket all incidental meaning and ask: what are some of the possible invariate aspects of this experience? The research Eidetic reduction – New World Encyclopedia Sep 19, 2017 – Eidetic reduction is a technique in Husserlian phenomenology, used to identify the essential components of the given phenomenon or experience. |
For example —
The reduction of two-colorings and four-colorings of a square or cubic
array of subsquares or subcubes to lines, sets of lines, cuts, or sets of
cuts between* the subsquares or subcubes.
See the diamond theorem and the eightfold cube.
* Cf. posts tagged Interality and Interstice.
Comments Off on Eidetic Reduction in Geometry
Friday, September 14, 2018
Denkraum
Underlying the I Ching structure is the finite affine space
of six dimensions over the Galois field with two elements.
In this field, "1 + 1 = 0," as noted here Wednesday.
See also other posts now tagged Interstice.
Comments Off on Denkraum
Thursday, September 13, 2018
Iconology of the Interstice
The title is from the 2013 paper by Latsis in the previous post.
The symmetries of the interstices at right underlie
the symmetries of the images at left.
Comments Off on Iconology of the Interstice
Godard and Interality
The previous post, "One Plus One," suggests some further
art-historical remarks on interality —
|
From Third Text , 2013, Vol. 27, No. 6, pp. 774–785 — "Genealogy of the Image in Histoire(s) du Cinéma : Godard, Warburg and the Iconology of the Interstice" * * * * P. 775 — My discussion will focus on the significance of the concept of the ‘space in-between,’ its importance for Godard’s work and its role in a relational historiography of images more broadly. I hope to corroborate how Godard functions as a twenty-first century archaeologist of the moving image, constructing a meta-cinematic collage that, while consisting of an indexing of (almost exclusively) pre-existing filmic samples, ends up becoming a hybrid work of art in its own right. Godard, in the final analysis, expands the Warburgian programme of iconology into that of a cinematographic iconology of the interstice. * * * * P. 777 — Godard conceives of the image only in the plural, in the intermediate space between two images, be it a prolonged one (in Histoire(s) there are frequent instances of black screens) or a non-existent one (superimposition, co-presence of two images on screen). He comments: ‘[For me] it’s always two, begin by showing two images rather than one, that’s what I call image, the one made up of two’ [18] and elsewhere, ‘I perceived . . . cinema is that which is between things, not things [themselves] but between one and another.’ [19] 18. Jean-Luc Godard and Youssef Ishaghpour, "Archéologie du cinéma et mémoire du siècle," Farrago ,Tours, 2000, p. 27. The title of this work is reflective of the Godardian agenda that permeates Histoire(s) . 19. Jean-Luc Godard, "Introduction à une véritable histoire du cinéma," Albatros , Paris,1980, p. 145 * * * * P. 783 — If it is in ‘the in-between’ that thought is born, then for Godard cinematography as ‘a form that thinks . . . was born with the advent of modern painting.’ [62] 62. Godard and Ishaghpour, op. cit., pp 45–46. * * * * P. 785 — Warburg commented on the signification of the black spaces that he placed between images in his analysis of the network of intervals in Mnemosyne , by quoting Johann Wolfgang Goethe’s dictum ‘the truth inhabits the middle space.’ [68] This citation induces a feeling of déjà-vu for the viewer of Histoire(s). The link was not missed by Warburg himself, as one of his diary entries testifies: ‘We can compare this phenomenon [the iconology of the interval] to that of the cinematic montage, the domain of the interpretation is an intervallic one.’ [69] 68. Warburg, Mnemosyne , pp 135–146. 69. Warburg is quoted in Didi-Huberman, L’image survivante, p. 503. (Georges Didi-Huberman, L’image survivante. Histoire de l’art et temps des fantômes selon Aby Warburg , Minuit, Paris, 2002) |
Comments Off on Godard and Interality
Sunday, September 9, 2018
Plan 9 Continues.
"The role of Desargues's theorem was not understood until
the Desargues configuration was discovered. For example,
the fundamental role of Desargues's theorem in the coordinatization
of synthetic projective geometry can only be understood in the light
of the Desargues configuration.
Thus, even as simple a formal statement as Desargues's theorem
is not quite what it purports to be. The statement of Desargues's theorem
pretends to be definitive, but in reality it is only the tip of an iceberg
of connections with other facts of mathematics."
— From p. 192 of "The Phenomenology of Mathematical Proof,"
by Gian-Carlo Rota, in Synthese , Vol. 111, No. 2, Proof and Progress
in Mathematics (May, 1997), pp. 183-196. Published by: Springer.
Stable URL: https://www.jstor.org/stable/20117627.
Related figures —
Note the 3×3 subsquare containing the triangles ABC, etc.
"That in which space itself is contained" — Wallace Stevens
Comments Off on Plan 9 Continues.
Saturday, September 8, 2018
Wednesday, September 5, 2018
Multifaceted Narrative
See also, in this journal, 23-cycle.
Update of Sept. 6, 2018, 9:05 AM ET: "The Cubist Method" —
Multifaceted narrative by James Joyce —
Multifaceted structures in pure mathematics, from Plato and R. T. Curtis —
Comments Off on Multifaceted Narrative
Saturday, September 1, 2018
Ron Shaw — D. 21 June 2016
The date of Ron Shaw's 2016 death appears to be June 21:
All other Internet sources I have seen omit the June 21 date.
This journal on that date —
Comments Off on Ron Shaw — D. 21 June 2016
Friday, August 31, 2018
Perception of Number
Review of yesterday's post Perception of Space —
From Harry Potter and the Philosopher's Stone (1997),
republished as "… and the Sorcerer's Stone ," Kindle edition:
In a print edition from Bloomsbury (2004), and perhaps in the
earliest editions, the above word "movements" is the first word
on page 168:
Click the above ellipse for some Log24 posts on the eightfold cube,
the source of the 168 automorphisms ("movements") of the Fano plane.
"Refined interpretation requires that you know that
someone once said the offspring of reality and illusion
is only a staggering confusion."
— Poem, "The Game of Roles," by Mary Jo Bang
Related material on reality and illusion —
an ad on the back cover of the current New Yorker —
"Hey, the stars might lie, but the numbers never do." — Song lyric
Comments Off on Perception of Number
Thursday, August 30, 2018
Perception* of Space
* A footnote in memory of a dancer who reportedly died
yesterday, August 29 — See posts tagged Paradigm Shift.
"Birthday, death-day — what day is not both?" — John Updike
Comments Off on Perception* of Space
Monday, August 27, 2018
Geometry and Simplicity
From …
Thinking in Four Dimensions
By Dusa McDuff
"I’ve got the rather foolhardy idea of trying to explain
to you the kind of mathematics I do, and the kind of
ideas that seem simple to me. For me, the search
for simplicity is almost synonymous with the search
for structure.
I’m a geometer and topologist, which means that
I study the structure of space …
. . . .
In each dimension there is a simplest space
called Euclidean space … "
— In Roman Kossak, ed.,
Simplicity: Ideals of Practice in Mathematics and the Arts
(Kindle Locations 705-710, 735). Kindle Edition.
For some much simpler spaces of various
dimensions, see Galois Space in this journal.
Comments Off on Geometry and Simplicity
Children of the Six Sides
From the former date above —
|
Saturday, September 17, 2016 |
From the latter date above —
|
Tuesday, October 18, 2016
Parametrization
|
From March 2018 —
Comments Off on Children of the Six Sides
Saturday, August 25, 2018
“Waugh, Orwell. Orwell, Waugh.”
Suggested by a review of Curl on Modernism —
Related material —
Waugh + Orwell in this journal and …
Comments Off on “Waugh, Orwell. Orwell, Waugh.”
Sunday, August 19, 2018
Possible Permutations
John Calder, an independent British publisher who built a prestigious list
of authors like Samuel Beckett and Heinrich Böll and spiritedly defended
writers like Henry Miller against censorship, died on Aug. 13 in Edinburgh.
He was 91.
— Richard Sandomir in the online New York Times this evening
On Beckett —
Also on August 13th —
Comments Off on Possible Permutations
Thursday, August 16, 2018
Mathematics and Narrative
Comments Off on Mathematics and Narrative
Wednesday, August 15, 2018
An Illusion of Brilliance
” . . . the 3 by 3, the six-sided, three-layer configuration of
the original Rubik’s Cube, which bestows an illusion of brilliance
on those who can solve it.”
— John Branch in the online New York Times today,
“Children of the Cube”:
https://www.nytimes.com/2018/08/15/sports/
cubing-usa-nationals-max-park.html
Cube-solving, like other sports, allows for displays of
impressive and admirable skill, if not “brilliance.”
The mathematics — group theory — that is sometimes associated
with Rubik’s Cube is, however, not a sport. See Rubik + Group
in this journal.
Comments Off on An Illusion of Brilliance
An Exceptional Isomorphism
From previous posts on this topic —
Comments Off on An Exceptional Isomorphism
Tuesday, August 14, 2018
-GL(3,2)-Oct-2009.jpg)
Monday, August 13, 2018
Trojan Horsitude
|
"It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy. It structures Plato's (realist) reaction to the sophists (nominalists). What is often called 'postmodernism' is really just nominalism, colourfully presented as the doctrine that there is nothing except texts. It is the variety of nominalism represented in many modern humanities, paralysing appeals to reason and truth."
— Simon Blackburn, Think, |
". . . a perfect triptych of horsitude"
— James Parker on the 2007 film "Michael Clayton"
Related material —
Horsitude in the 4×2 grid, and
Comments Off on Trojan Horsitude
Friday, August 10, 2018
Thursday, August 9, 2018
True Grids
From a search in this journal for "True Grid,"
a fanciful description of the 3×3 grid —
"This is the garden of Apollo,
the field of Reason…."
– John Outram, architect
A fanciful instance of the 4×2 grid in
a scene from the film "The Master" —
A fanciful novel referring to the number 8,
and a not -so-fanciful reference:
-actions-500w.jpg)
Illustrated above are Katherine Neville's novel The Eight and the
"knight" coordinatization of the 4×2 grid from a page on the exceptional
isomorphism between PSL(3,2) (alias GL(3,2)) and PSL(2,7) — groups
of, respectively, degree 7 and degree 8.
Literature related to the above remarks on grids:
Ross Douthat's New York Times column yesterday purported, following
a 1946 poem by Auden, to contrast students of the humanities with
technocrats by saying that the former follow Hermes, the latter Apollo.
I doubt that Apollo would agree.
Comments Off on True Grids
Wednesday, August 8, 2018
8/8
From mathoverflow.net on Dec. 7, 2016 —
The exceptional isomorphism between
PGL(3,2) and PSL(2,7): geometric origin?
Essentially the same question was asked earlier at
math.stackexchange.com on Aug. 2, 2010.
See also this journal in November 2017 —
"Read something that means something."
— New Yorker ad
.
Background — Relativity Problem in Log24.
Comments Off on 8/8
Monday, August 6, 2018
The Girl with Kaleidoscope Eyes
|
“All right, Jessshica. It’s time to open the boxsssschhh.” “Gahh,” she said. She began to walk toward the box, but her heart failed her and she retreated back to the chair. “Fuck. Fuck.” Something mechanical purred. The seam she had found cracked open and the top of the box began to rise. She squeezed shut her eyes and groped her way into a corner, curling up against the concrete and plugging her ears with her fingers. That song she’d heard the busker playing on the train platform with Eliot, “Lucy in the Sky with Diamonds”; she used to sing that. Back in San Francisco, before she learned card tricks. It was how she’d met Benny: He played guitar. Lucy was the best earner, Benny said, so that was mainly what she sang. She must have sung it five times an hour, day after day. At first she liked it but then it was like an infection, and there was nothing she could do and nowhere she could go without it running across her brain or humming on her lips, and God knew she tried; she was smashing herself with sex and drugs but the song began to find its way even there. One day, Benny played the opening chord and she just couldn’t do it. She could not sing that fucking song. Not again. She broke down, because she was only fifteen, and Benny took her behind the mall and told her it would be okay. But she had to sing. It was the biggest earner. She kind of lost it and then so did Benny and that was the first time he hit her. She ran away for a while. But she came back to him, because she had nothing else, and it seemed okay. It seemed like they had a truce: She would not complain about her bruised face and he would not ask her to sing “Lucy.” She had been all right with this. She had thought that was a pretty good deal. Now there was something coming out of a box, and she reached for the most virulent meme she knew. “Lucy in the sky!” she sang. “With diamonds!” • • •
Barry, Max. Lexicon: A Novel (pp. 247-248). |
Related material from Log24 on All Hallows' Eve 2013 —
"Just another shake of the kaleidoscope" —
Related material:
Kaleidoscope Puzzle,
Design Cube 2x2x2, and
Through the Looking Glass: A Sort of Eternity.
Comments Off on The Girl with Kaleidoscope Eyes
Sunday, August 5, 2018
Saturday, August 4, 2018
The Secret Life of Harry Albers
A novel by Harry Albers featuring his fictional Pacific Science Institute:
See the real Pacific Science Institute (PSI) in the previous post.
Synchronology check —
Related literary remarks —
— Cloud Atlas , by David Mitchell (2004).
Comments Off on The Secret Life of Harry Albers
Manifestations of Exquisite Geometry
An alleged manifestation in physics, from Scientific American —
Manifestations in pure mathematics, from Plato and R. T. Curtis —
For some entertaining literary manifestations, see Wrinkle.
Comments Off on Manifestations of Exquisite Geometry
Tuesday, July 31, 2018
Easter Storytelling
From the Wikipedia article on the 1994 film "North" —
"North is forced to ship himself home in a FedEx box.
He reaches his house … but as he runs toward his parents,
an assassin takes aim. As he squeezes the trigger,
North awakens in the mall, now empty. The Easter Bunny
takes him home . . . ."
The film's author —
Zweibel's FedEx box suggests a review of
the post Geometry for Goyim (June 6, 2018).
Comments Off on Easter Storytelling
Sunday, July 29, 2018
The Materialization
McCarthy's "materialization of plot and character" does not,
for me, constitute a proof that "there is being, after all,
beyond the arbitrary flux of existence."
Neither does the above materialization of 281 as the page
number of her philosophical remark.
See also the materialization of 281 as a page number in
the book Witchcraft by Charles Williams —
The materialization of 168 as a page number in a
Stephen King novel is somewhat more convincing,
but less convincing than the materialization of Klein's
simple group of of 168 elements in the eightfold cube.
Comments Off on The Materialization
Saturday, July 28, 2018
Angle
Floyd: "You're trying to figure out this length.
That's the hypotenuse. So you have to
know this angle."
Comments Off on Angle
Wednesday, July 25, 2018
Bucharest Physics
Update of 10:30 PM ET the same day —
For some philosophical background, including an I Ching diagram, see . . .
See as well my own 8×8 diagram (1989) related to the I Ching .
Comments Off on Bucharest Physics
Tuesday, July 24, 2018
Monday, July 23, 2018
Eightfold Cube for Furey*
Click to enlarge:
Above are the 7 frames of an animated gif from a Wikipedia article.
* For the Furey of the title, see a July 20 Quanta Magazine piece —
See also the eightfold cube in this journal.
"Before time began . . . ." — Optimus Prime
Comments Off on Eightfold Cube for Furey*
Space 101
From the April 1st publication date of "Interality Shows Through,"
by Geling Shang —
See too yesterday's post Space.
Comments Off on Space 101
Sunday, July 22, 2018
Saturday, July 21, 2018
Comic-Con 2018
|
"For many of us, the geometry course sounded the death knell — "Shape and Space in Geometry"
© 1997-2003 Annenberg/CPB. All rights reserved. |
Comments Off on Comic-Con 2018
Building-Block Theory
(A sequel to yesterday’s Geometry for Jews)
From this journal on the above UCI posting date — April 6, 2018 —
From this journal on the above lecture date — April 26, 2018 —
illustrations in a post titled Defining Form —
For the relevance of the above material to building blocks,
see Eightfold Cube in this journal.
Comments Off on Building-Block Theory
Friday, July 20, 2018
Geometry for Jews
Click image to enlarge —
A portrait from the home page of David Eppstein,
a professor at the University of California, Irvine
“… how can an image with 8 points and 8 lines
possibly represent a space with 7 points and 7 lines???“
— David Eppstein, 21 December 2015
See ” Projective spaces as ‘collapsed vector spaces,’ ”
page 203 in Geometry and Symmetry by Paul B. Yale,
published by Holden-Day in 1968.
Comments Off on Geometry for Jews
Wednesday, July 18, 2018
Doodle
From "The Educated Imagination: A Website Dedicated
to Northrop Frye" —
"In one of the notebooks for his first Bible book Frye writes,
'For at least 25 years I’ve been preoccupied by
the notion of a key to all mythologies.' . . . .
Frye made a valiant effort to provide a key to all mythology,
trying to fit everything into what he called the Great Doodle. . . ."
From a different page at the same website —
Here Frye provides a diagram of four sextets.
I prefer the Miracle Octad Generator of R. T. Curtis —
.
Comments Off on Doodle
Monday, July 16, 2018
Greatly Exaggerated Report
"The novel has a parallel narrative that eventually
converges with the main story."
— Wikipedia on a book by Foer's novelist brother
Public Squares
An image from the online New York Times
on the date, July 6,
of the above Atlantic article —
An image from "Blackboard Jungle," 1955 —
"Through the unknown, remembered gate . . . ."
Comments Off on Greatly Exaggerated Report
Sunday, July 15, 2018
Jewish Oases
"… Lincoln Plaza Cinemas, the Juilliard String Quartet,
and the Strand Book Store remained oases
for cultural and intellectual stimulation."
— John S. Friedman in The Forward , Jan. 21, 2018
Read more:
https://forward.com/culture/392483/
how-fred-bass-dan-talbot-robert-mann
-shaped-new-york-culture/
From the Oasis in Steven Spielberg's "Ready Player One" (2018) —
I prefer, from a Log24 search for Flux Capacitor …
From "Raiders of the Lost Images" —
"The cube shape of the lost Mother Box,
also known as the Change Engine,
is shared by the Stone in a novel by
Charles Williams, Many Dimensions .
See the Solomon's Cube webpage."
Comments Off on Jewish Oases
Saturday, July 14, 2018
Expanding the Spiel
The walkerart.org passage above is from Feb. 17, 2011.
See also this journal on Feb. 17, 2011 —
"… Only by the form, the pattern,
Can words or music reach
The stillness…."
— T. S. Eliot,
Four Quartets
For further details, see Time Fold.
Comments Off on Expanding the Spiel
Friday, July 13, 2018
Segue for Harlan Ellison
From a Log24 post of March 13, 2003 —
|
"For many of us, the geometry course sounded the death knell — "Shape and Space in Geometry"
© 1997-2003 Annenberg/CPB. All rights reserved. |
Comments Off on Segue for Harlan Ellison
Thursday, July 12, 2018
Kummerhenge Illustrated
“… the utterly real thing in writing is the only thing that counts…."
— Maxwell Perkins to Ernest Hemingway, Aug. 30, 1935
"Omega is as real as we need it to be."
— Burt Lancaster in "The Osterman Weekend"
Comments Off on Kummerhenge Illustrated
Wednesday, July 11, 2018
Clarity and Precision
"The whole meaning of the word is
looking into something with clarity and precision,
seeing each component as distinct,
and piercing all the way through
so as to perceive the most fundamental reality
of that thing."
For the word itself, try a Web search on
noteworthy phrases above.
“. . . the utterly real thing in writing is
the only thing that counts . . . ."
— Maxwell Perkins to Ernest Hemingway, Aug. 30, 1935
"168"
— Page number in a 2016 Scribner edition
of Stephen King's IT
Comments Off on Clarity and Precision