Also on April 1, 2015 —
From the essay cited above —
For the formula of building blocks , see Walsh series.
* See Google.
Thursday, July 14, 2022
Tuesday, November 24, 2020
Monday, November 16, 2020
Physics Jeopardy: “What Is a Particle?”
Comments Off on Physics Jeopardy: “What Is a Particle?”
Sunday, January 26, 2020
Harmonic-Analysis Building Blocks
Comments Off on Harmonic-Analysis Building Blocks
Duke Blocks
The Wall Street Journal Jan. 24 on a Duke University professor —
"Dr. Daubechies is best known for her work on mathematical structures
called wavelets; her discoveries have been so influential, in fact, that
these are referred to in the field as Daubechies wavelets. She describes
them as 'mathematical building blocks' that can be used to extract the
essential elements of images or signals without losing their quality—
in effect, a new universal language for scientists and researchers."
See also this journal on January 20-21, and …
Comments Off on Duke Blocks
Monday, January 20, 2020
Dyadic Harmonic Analysis:
The Fourfold Square and Eightfold Cube
Related material: A Google image search for “field dream” + log24.
Comments Off on Dyadic Harmonic Analysis:
Thursday, October 3, 2019
Quarks for Poets
The title was suggested by a recent New Yorker poem.
Related material: The remarks of Mysterio in "Spider-Man: Far From Home."
Comments Off on Quarks for Poets
Monday, September 16, 2019
Emergence
"Elementary particles are the most fundamental building blocks
of nature, and their study would seem to be an expression of
simplification in its purest form. The essence of complexity
research, by contrast, is the emergence of new kinds of order
that are only manifest when systems are large and messy."
— Sean Carroll in an opinion piece that concludes as follows:
The above plug for Sean Carroll's book
The Big Picture : On the Origins of
Life, Meaning, and the Universe Itself suggests…
Comments Off on Emergence
Sunday, May 26, 2019
Burning Bright
Compare and contrast with . . .
The Brightburn Logo:
Related material from the May 12 post
"The Collective Unconscious
in a Cartoon Graveyard" —
|
"When they all finally reach their destination — " When asked about the film's similarities to the 2015 Disney movie Tomorrowland , which also posits a futuristic world that exists in an alternative dimension, Nichols sighed. 'I was a little bummed, I guess,' he said of when he first learned about the project. . . . 'Our die was cast. Sometimes this kind of collective unconscious that we're all dabbling in, sometimes you're not the first one out of the gate.' " |
Comments Off on Burning Bright
Sunday, May 19, 2019
The Building Blocks of Geometry
From "On the life and scientific work of Gino Fano"
by Alberto Collino, Alberto Conte, and Alessandro Verra,
ICCM Notices , July 2014, Vol. 2 No. 1, pp. 43-57 —
|
" Indeed, about the Italian debate on foundations of Geometry, it is not rare to read comments in the same spirit of the following one, due to Jeremy Gray13. He is essentially reporting Hans Freudenthal’s point of view: ' When the distinguished mathematician and historian of mathematics Hans Freudenthal analysed Hilbert’s Grundlagen he argued that the link between reality and geometry appears to be severed for the first time in Hilbert’s work. However, he discovered that Hilbert had been preceded by the Italian mathematician Gino Fano in 1892. . . .' " 13 J. Gray, "The Foundations of Projective Geometry in Italy," Chapter 24 (pp. 269–279) in his book Worlds Out of Nothing , Springer (2010). |
Restoring the severed link —
See also Espacement and The Thing and I.
Related material —
Comments Off on The Building Blocks of Geometry
Monday, May 6, 2019
One Stuff
Comments Off on One Stuff
Monday, March 25, 2019
Espacement
(Continued from the previous post.)
|
In-Between "Spacing" and the "Chôra " (Ch. 2 in Henk Oosterling & Ewa Plonowska Ziarek (Eds.), Intermedialities: Philosophy, Arts, Politics , Lexington Books, October 14, 2010) "The term 'spacing' ('espacement ') is absolutely central to Derrida's entire corpus, where it is indissociable from those of différance (characterized, in the text from 1968 bearing this name, as '[at once] spacing [and] temporizing' 1), writing (of which 'spacing' is said to be 'the fundamental property' 2) and deconstruction (with one of Derrida's last major texts, Le Toucher: Jean-Luc Nancy , specifying 'spacing ' to be 'the first word of any deconstruction' 3)." 1 Jacques Derrida, “La Différance,” in Marges – de la philosophie (Paris: Minuit, 1972), p. 14. Henceforth cited as D . 2 Jacques Derrida, “Freud and the Scene of Writing,” trans. A. Bass, in Writing and Difference (Chicago: University of Chicago Press, 1978), p. 217. Henceforth cited as FSW . 3 Jacques Derrida, Le Toucher, Jean-Luc Nancy (Paris: Galilée, 2000), p. 207. . . . . "… a particularly interesting point is made in this respect by the French philosopher, Michel Haar. After remarking that the force Derrida attributes to différance consists simply of the series of its effects, and is, for this reason, 'an indefinite process of substitutions or permutations,' Haar specifies that, for this process to be something other than a simple 'actualisation' lacking any real power of effectivity, it would need “a soubassement porteur ' – let’s say a 'conducting underlay' or 'conducting medium' which would not, however, be an absolute base, nor an 'origin' or 'cause.' If then, as Haar concludes, différance and spacing show themselves to belong to 'a pure Apollonism' 'haunted by the groundless ground,' which they lack and deprive themselves of,16 we can better understand both the threat posed by the 'figures' of space and the mother in the Timaeus and, as a result, Derrida’s insistent attempts to disqualify them. So great, it would seem, is the menace to différance that Derrida must, in a 'properly' apotropaic gesture, ward off these 'figures' of an archaic, chthonic, spatial matrix in any and all ways possible…." 16 Michel Haar, “Le jeu de Nietzsche dans Derrida,” Revue philosophique de la France et de l’Etranger 2 (1990): 207-227. . . . . … "The conclusion to be drawn from Democritus' conception of rhuthmos , as well as from Plato's conception of the chôra , is not, therefore, as Derrida would have it, that a differential field understood as an originary site of inscription would 'produce' the spatiality of space but, on the contrary, that 'differentiation in general' depends upon a certain 'spatial milieu' – what Haar would name a 'groundless ground' – revealed as such to be an 'in-between' more 'originary' than the play of differences it in-forms. As such, this conclusion obviously extends beyond Derrida's conception of 'spacing,' encompassing contemporary philosophy's continual privileging of temporization in its elaboration of a pre-ontological 'opening' – or, shall we say, 'in-between.' |
For permutations and a possible "groundless ground," see
the eightfold cube and group actions both on a set of eight
building blocks arranged in a cube (a "conducting base") and
on the set of seven natural interstices (espacements ) between
the blocks. Such group actions provide an elementary picture of
the isomorphism between the groups PSL(2,7) (acting on the
eight blocks) and GL(3,2) (acting on the seven interstices).
Espacements
For the Church of Synchronology —
See also, from the reported publication date of the above book
Intermedialities , the Log24 post Synchronicity.
Comments Off on Espacement
Sunday, March 17, 2019
Just Another Block in the Wall
Yesterday's post Grundlagen —
Midrash on yesterday's Grundlagen —
A poem linked to here on the above "building blocks" date, in the
Log24 post Sermon of 11 AM ET Sunday, 15 September 2013 —
Comments Off on Just Another Block in the Wall
Saturday, March 16, 2019
Thursday, February 21, 2019
A Tale of Eight Building Blocks*
* For another such tale, see Eightfold Cube in this journal.
Comments Off on A Tale of Eight Building Blocks*
Saturday, July 21, 2018
Building-Block Theory
(A sequel to yesterday’s Geometry for Jews)
From this journal on the above UCI posting date — April 6, 2018 —
From this journal on the above lecture date — April 26, 2018 —
illustrations in a post titled Defining Form —
For the relevance of the above material to building blocks,
see Eightfold Cube in this journal.
Comments Off on Building-Block Theory
Thursday, July 28, 2016
The Giglmayr Foldings
Giglmayr's transformations (a), (c), and (e) convert
his starting pattern
1 2 5 6
3 4 7 8
9 10 13 14
11 12 15 16
to three length-16 sequences. Putting these resulting
sequences back into the 4×4 array in normal reading
order, we have
1 2 3 4 1 2 4 3 1 4 2 3
5 6 7 8 5 6 8 7 7 6 8 5
9 10 11 12 13 14 16 15 15 14 16 13
13 14 15 16 9 10 12 11 9 12 10 11
(a) (c) (e)
Four length-16 basis vectors for a Galois 4-space consisting
of the origin and 15 weight-8 vectors over GF(2):
0 0 0 0 0 0 0 0 0 0 1 1 0 1 0 1
0 0 0 0 1 1 1 1 0 0 1 1 0 1 0 1
1 1 1 1 0 0 0 0 0 0 1 1 0 1 0 1
1 1 1 1 1 1 1 1 0 0 1 1 0 1 0 1 .
(See "Finite Relativity" at finitegeometry.org/sc.)
The actions of Giglmayr's transformations on the above
four basis vectors indicate the transformations are part of
the affine group (of order 322,560) on the affine space
corresponding to the above vector space.
For a description of such transformations as "foldings,"
see a search for Zarin + Folded in this journal.
Comments Off on The Giglmayr Foldings
Tuesday, November 17, 2015
The Physics and Theology of Building Blocks
Physics:
Theology:
Neither of the above prose passages inspires confidence, since
building blocks are, by their very nature, not infinitesimal.
See the post Being Interpreted of August 14, 2015 —
Comments Off on The Physics and Theology of Building Blocks
Saturday, September 5, 2015
Operation Blockhead
A New Yorker writer on the new parent corporation of
Google, named Alphabet:
"In Larry Page’s letter explaining it to us, Alphabet
is illustrated with a bunch of kids’ building blocks.
Operation Childlike Innocence, Phase One."
— Sarah Larson
Building blocks, Sarah, are not the same thing
as alphabet blocks. For the distinction, see a
Log24 post of August 14, 2015, "Being Interpreted."
The New Yorker apparently also has another fact wrong.
The official version of Page's letter is not "illustrated."
Perhaps, Sarah, you mistook the new Alphabet website
abc.xyz, which did show alphabet blocks and quoted
Page's letter, for the letter itself.
Comments Off on Operation Blockhead
Blockheads
Cartoon from the current (Sept. 7, 2015) New Yorker , p. 25 —
See as well searches in this journal for Montessori and Machiavelli.
Midrash from Sept. 3 at the online New Yorker —
"We don’t instinctively care about the brand unity
Google wants to achieve with its new mega-company,
Alphabet, of which it is now a part. Especially because
Alphabet takes our most elementally wonderful
general-use word—the name of the components of
language itself—and reassigns it, like the words tweet,
twitter, vine, facebook, friend, and so on, into a branded
realm. In Larry Page’s letter explaining it to us,
Alphabet is illustrated with a bunch of kids’ building blocks.
Operation Childlike Innocence, Phase One."
— Sarah Larson
Comments Off on Blockheads
Saturday, April 11, 2015
The Starbird Manifesto
"But what was supposed to be the source of a compound's
authority? Why, the same as that of all new religious movements:
direct access to the godhead, which in this case was Creativity."
— Tom Wolfe, From Bauhaus to Our House
"Creativity is not a matter of magical inspiration."
— Burger and Starbird, The 5 Elements of Effective Thinking (2012)
Video published on Oct 19, 2012
"In this fifth of five videos, mathematics professor
Michael Starbird talks about the fifth element
in his new book, The 5 Elements of Effective Thinking ,
co-authored with Williams College professor
Edward B. Burger."
For more on the Starbird manifesto, see Princeton University Press.
An excerpt —
See also a post for Abel's Birthday, 2011 —
Midnight in Oslo — and a four-elements image from
the Jan. 26, 2010, post Symbology —
.
Comments Off on The Starbird Manifesto
Thursday, December 27, 2012
Object Lesson
Yesterday's post on the current Museum of Modern Art exhibition
"Inventing Abstraction: 1910-1925" suggests a renewed look at
abstraction and a fundamental building block: the cube.
From a recent Harvard University Press philosophical treatise on symmetry—
The treatise corrects Nozick's error of not crediting Weyl's 1952 remarks
on objectivity and symmetry, but repeats Weyl's error of not crediting
Cassirer's extensive 1910 (and later) remarks on this subject.
For greater depth see Cassirer's 1910 passage on Vorstellung :
This of course echoes Schopenhauer, as do discussions of "Will and Idea" in this journal.
For the relationship of all this to MoMA and abstraction, see Cube Space and Inside the White Cube.
"The sacramental nature of the space becomes clear…." — Brian O'Doherty
Comments Off on Object Lesson
Thursday, March 1, 2012
Block That Metaphor:
The Cube Model and Peano Arithmetic
The eightfold cube model of the Fano plane may or may not have influenced a new paper (with the date Feb. 10, 2011, in its URL) on an attempted consistency proof of Peano arithmetic—
The Consistency of Arithmetic, by Storrs McCall
"Is Peano arithmetic (PA) consistent? This paper contains a proof that it is. …
Axiomatic proofs we may categorize as 'syntactic', meaning that they concern only symbols and the derivation of one string of symbols from another, according to set rules. 'Semantic' proofs, on the other hand, differ from syntactic proofs in being based not only on symbols but on a non-symbolic, non-linguistic component, a domain of objects. If the sole paradigm of 'proof ' in mathematics is 'axiomatic proof ', in which to prove a formula means to deduce it from axioms using specified rules of inference, then Gödel indeed appears to have had the last word on the question of PA-consistency. But in addition to axiomatic proofs there is another kind of proof. In this paper I give a proof of PA's consistency based on a formal semantics for PA. To my knowledge, no semantic consistency proof of Peano arithmetic has yet been constructed.
The difference between 'semantic' and 'syntactic' theories is described by van Fraassen in his book The Scientific Image :
"The syntactic picture of a theory identifies it with a body of theorems, stated in one particular language chosen for the expression of that theory. This should be contrasted with the alternative of presenting a theory in the first instance by identifying a class of structures as its models. In this second, semantic, approach the language used to express the theory is neither basic nor unique; the same class of structures could well be described in radically different ways, each with its own limitations. The models occupy centre stage." (1980, p. 44)
Van Fraassen gives the example on p. 42 of a consistency proof in formal geometry that is based on a non-linguistic model. Suppose we wish to prove the consistency of the following geometric axioms:
A1. For any two lines, there is at most one point that lies on both.
A2. For any two points, there is exactly one line that lies on both.
A3. On every line there lie at least two points.
The following diagram shows the axioms to be consistent:
Figure 1
The consistency proof is not a 'syntactic' one, in which the consistency of A1-A3 is derived as a theorem of a deductive system, but is based on a non-linguistic structure. It is a semantic as opposed to a syntactic proof. The proof constructed in this paper, like van Fraassen's, is based on a non-linguistic component, not a diagram in this case but a physical domain of three-dimensional cube-shaped blocks. ….
… The semantics presented in this paper I call 'block semantics', for reasons that will become clear…. Block semantics is based on domains consisting of cube-shaped objects of the same size, e.g. children's wooden building blocks. These can be arranged either in a linear array or in a rectangular array, i.e. either in a row with no space between the blocks, or in a rectangle composed of rows and columns. A linear array can consist of a single block, and the order of individual blocks in a linear or rectangular array is irrelevant. Given three blocks A, B and C, the linear arrays ABC and BCA are indistinguishable. Two linear arrays can be joined together or concatenated into a single linear array, and a rectangle can be re-arranged or transformed into a linear array by successive concatenation of its rows. The result is called the 'linear transformation' of the rectangle. An essential characteristic of block semantics is that every domain of every block model is finite. In this respect it differs from Tarski’s semantics for first-order logic, which permits infinite domains. But although every block model is finite, there is no upper limit to the number of such models, nor to the size of their domains.
It should be emphasized that block models are physical models, the elements of which can be physically manipulated. Their manipulation differs in obvious and fundamental ways from the manipulation of symbols in formal axiomatic systems and in mathematics. For example the transformations described above, in which two linear arrays are joined together to form one array, or a rectangle of blocks is re-assembled into a linear array, are physical transformations not symbolic transformations. …"
— Storrs McCall, Department of Philosophy, McGill University
See also…
- Finite Geometry and Physical Space,
- McCall in the book search of this morning's Salvation for Gopnik, and
- the post Declarations of Jan. 29, 2012.
Comments Off on Block That Metaphor:
Saturday, December 3, 2011
Blockheads
(Continued from earlier posts.)
See the online New York Times on November 27—
With Blocks, Educators Go Back to Basics
— and related letters, online today—
The Building Blocks of Education
Another back-to-basics illustration—
"Design is how it works."
— Steve Jobs
See also the designer of the above Big apple—
“I’m fascinated with how past designers
had to come up with ideas
and solve problems using limited resources.”
Comments Off on Blockheads
Wednesday, April 27, 2011
Block That Metaphor–
A Note on Galois Geometry
|
Simple groups as the (Click image to enlarge.)
|
Points, lines, etc., as the
|
Related material —
(Click images for some background.)
|
Building blocks and
|
Building blocks and |
Comments Off on Block That Metaphor–
Saturday, July 24, 2010
Thursday, April 2, 2009
Thursday April 2, 2009
In memory of
physics historian
Martin J. Klein,
(June 25, 1924-
March 28, 2009)
"… in physics itself, there was what appeared, briefly, to be an ending, which then very quickly gave way to a new beginning: The quest for the ultimate building-blocks of the universe had been taken down to the molecular level in nineteenth-century kinetic theory… and finally to the nuclear level in the second and third decades of the twentieth century. For a moment in the 1920s the quest appeared to have ended…. However… this paradise turned out to be, if not exactly a fool's paradise, then perhaps an Eden lost."
— No Truth Except in the Details: Essays in Honor of Martin J. Klein, introduction by A.J. Kox and Daniel Siegel, June 25, 1994
New York Times obituary dated April 1, 2009:
"Martin J. Klein, a historian of modern physics…. died Saturday, [March 28, 2009] in Chapel Hill, N.C. He was 84 and lived in Chapel Hill."
Klein edited, among other things, Paul Ehrenfest: Collected Scientific Papers (publ. by North-Holland, Amsterdam, 1959).
|
Related material:
"Almost every famous chess game
is a well-wrought urn
in Cleanth Brooks’ sense."
— John Holbo,
Now We See
Wherein Lies the Pleasure
"The entire sequence of moves in these… chapters reminds one– or should remind one– of a certain type of chess problem where the point is not merely the finding of a mate in so many moves, but what is termed 'retrograde analysis'…."
— Vladimir Nabokov, foreword to The Defense
Comments Off on Thursday April 2, 2009
Saturday, May 10, 2008
Saturday May 10, 2008
MoMA Goes to
Kindergarten
Kindergarten
"… the startling thesis of Mr. Brosterman's new book, 'Inventing Kindergarten' (Harry N. Abrams, $39.95): that everything the giants of modern art and architecture knew about abstraction they learned in kindergarten, thanks to building blocks and other educational toys designed by Friedrich Froebel, a German educator, who coined the term 'kindergarten' in the 1830's."
— "Was Modernism Born
in Toddler Toolboxes?"
by Trip Gabriel, New York Times,
April 10, 1997
RELATED MATERIAL
Figure 1 —
Concept from 1819:
(Footnotes 1 and 2)
Figure 2 —
The Third Gift, 1837:
Froebel, the inventor of
kindergarten, worked as
an assistant to the
crystallographer Weiss
mentioned in Fig. 1.
(Footnote 3)
Figure 3 —
The Third Gift, 1906:
Figure 4 —
Solomon's Cube,
1981 and 1983:
Figure 5 —
Design Cube, 2006:
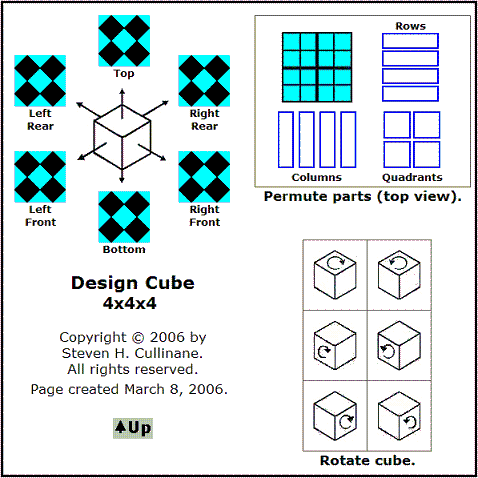
The above screenshot shows a
moveable JavaScript display
of a space of six dimensions
(over the two-element field).
(To see how the display works,
try the Kaleidoscope Puzzle first.)
For some mathematical background, see
Footnotes:
1. Image said to be after Holden and Morrison, Crystals and Crystal Growing, 1982
2. Curtis Schuh, "The Library: Biobibliography of Mineralogy," article on Mohs
3. Bart Kahr, "Crystal Engineering in Kindergarten" (pdf), Crystal Growth & Design, Vol. 4 No. 1, 2004, 3-9
2. Curtis Schuh, "The Library: Biobibliography of Mineralogy," article on Mohs
3. Bart Kahr, "Crystal Engineering in Kindergarten" (pdf), Crystal Growth & Design, Vol. 4 No. 1, 2004, 3-9
Comments Off on Saturday May 10, 2008
Monday, August 14, 2006
Monday August 14, 2006
| Cleavage Term
“… a point of common understanding between the classic and romantic worlds. Quality, the cleavage term between hip and square, seemed to be it. Both worlds used the term. Both knew what it was. It was just that the romantic left it alone and appreciated it for what it was and the classic tried to turn it into a set of intellectual building blocks for other purposes.”
For such building blocks, see
A Trinity for Rebecca (4/25/06) and yesterday’s lottery |
|
| In memory of Kermit Hall, college president, who died Sunday, August 13, 2006: Square
|
In memory of Duke Jordan, jazz pianist, who died Tuesday, August 8, 2006:
|
Square and hip may each have a place
in heaven; for a less pleasant destination,
see the previous entry.
__________________________________
* Update of 3 PM 8/14/06:
See Forrest Gump on God
in an Aug. 11 entry and
the related paper
| Renegotiating Chinese Identity: Between Local Group and National Ideology, by Kristen Parris:
The paper is found in Related material |
Comments Off on Monday August 14, 2006
Thursday, July 13, 2006
Thursday July 13, 2006
Today's birthday:
Harrison Ford
"The forest here at the bottom of the canyon is mostly pine, with a few aspen and broad-leafed shrubs. Steep canyon walls rise way above us on both sides. Occasionally the trail opens into a patch of sunlight and grass that edges the canyon stream, but soon it reenters the deep shade of the pines. The earth of the trail is covered with a soft springy duff of pine needles. It is very quiet here.
Mountains like these and travelers in the mountains and events that happen to them here are found not only in Zen literature but in the tales of every major religion."– Robert Pirsig
Related material:
"Canyon Breeze" as played at
myspace.com/montanaskies
"… a point of common understanding between the classic and romantic worlds. Quality, the cleavage term between hip and square, seemed to be it. Both worlds used the term. Both knew what it was. It was just that the romantic left it alone and appreciated it for what it was and the classic tried to turn it into a set of intellectual building blocks for other purposes."– Robert Pirsig
For such building blocks, see
myspace.com/affine.
myspace.com/affine.
The background music there
is the same, by Montana Skies.
Comments Off on Thursday July 13, 2006