(29 January 1810 – 14 May 1893)
See as well some earlier references to diamond signs here .
The proper context for some diamond figures that I am interested in
is the 4×4 array that appears, notably, in Hudson's 1905 classic
Kummer's Quartic Surface . Hence this post's "Kummerhenge" tag,
suggested also by some monumental stonework at Tufte's site.
http://www.math.lsa.umich.edu/~idolga/KummerOliver.pdf
is a preprint of an Oct. 10, 2019, talk by Igor Dolgachev —
Kummer Surfaces: 200 Years of Study.
The preprint is also available on the arXiv:
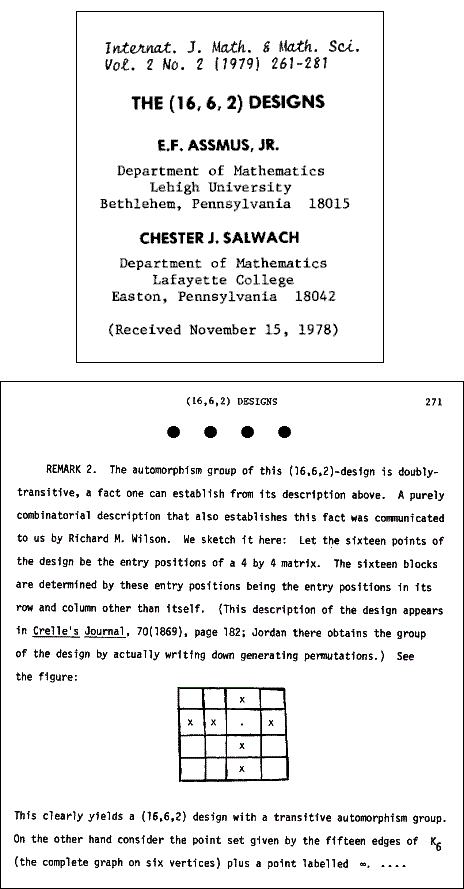
The Hudson array mentioned above is as follows —
See also Whitehead and the
Relativity Problem (Sept. 22).
For coordinatization of a 4×4
array, see a note from 1986
in the Feb. 26 post Citation.
(Continued.)
The previous post suggests a review of
the following mathematical landmark —
The cited article by Kummer is at . . .
https://archive.org/details/monatsberichtede1864kn/page/246 .
From the series of posts tagged Kummerhenge —
A Wikipedia article relating the above 4×4 square to the work of Kummer —
A somewhat more interesting aspect of the geometry of the 4×4 square
is its relationship to the 4×6 grid underlying the Miracle Octad Generator
(MOG) of R. T. Curtis. Hudson's 1905 classic Kummer's Quartic Surface
deals with the Kummer properties above and also foreshadows, without
explicitly describing, the finite-geometry properties of the 4×4 square as
a finite affine 4-space — properties that are of use in studying the Mathieu
group M24 with the aid of the MOG.
Those pleased by what Ross Douthat today called
"The Return of Paganism" are free to devise rituals
involving what might be called "the sacred geometry
of the Kummer 166 configuration."
As noted previously in this journal,
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
See also earlier posts also tagged "Kummerhenge" and
another property of the remarkable Kummer 166 —
For some related literary remarks, see "Transposed" in this journal.
Some background from 2001 —
For further details, see finitegeometry.org/sc/35/hudson.html.
“… the utterly real thing in writing is the only thing that counts…."
— Maxwell Perkins to Ernest Hemingway, Aug. 30, 1935
"Omega is as real as we need it to be."
— Burt Lancaster in "The Osterman Weekend"
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
See too "The Ruler of Reality" in this journal.
Related material —
A more esoteric artifact: The Kummer 166 Configuration . . .
An array of Göpel tetrads appears in the background below.
"As you can see, we've had our eye on you
for some time now, Mr. Anderson."
The previous post quoted Tom Wolfe on Chomsky's use of
the word "array."
An example of particular interest is the 4×4 array
(whether of dots or of unit squares) —
.
Some context for the 4×4 array —
The following definition indicates that the 4×4 array, when
suitably coordinatized, underlies the Kummer lattice .
Further background on the Kummer lattice:
Alice Garbagnati and Alessandra Sarti,
"Kummer Surfaces and K3 surfaces
with $(Z/2Z)^4$ symplectic action."
To appear in Rocky Mountain J. Math. —
The above article is written from the viewpoint of traditional
algebraic geometry. For a less traditional view of the underlying
affine 4-space from finite geometry, see the website
Finite Geometry of the Square and Cube.
Some further context …
"To our knowledge, the relation of the Golay code
to the Kummer lattice … is a new observation."
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of
Kummer surfaces in the Mathieu group M24 "
As noted earlier, Taormina and Wendland seem not to be aware of
R. W. H. T. Hudson's use of the (uncoordinatized*) 4×4 array in his
1905 book Kummer's Quartic Surface. The array was coordinatized,
i.e. given a "vector space structure," by Cullinane eight years prior to
the cited remarks of Curtis.
* Update of Sept. 14: "Uncoordinatized," but parametrized by 0 and
the 15 two-subsets of a six-set. See the post of Sept. 13.
Further details from Edmund Hess in 1889* related to
last night's remarks on the Klein 6015 configuration
and the Kummer 166 configuration —
* Edmund Hess, "Beiträge zur Theorie der räumlichen Configurationen.
Ueber die Klein'sche Configuration Cf. (60₁₅, 30₆) und einige
bemerkenswerthe aus dieser ableitbare räumliche Configurationen."
From "Projective Geometry and PT-Symmetric Dirac Hamiltonian,"
Y. Jack Ng and H. van Dam,
Physics Letters B , Volume 673, Issue 3,
23 March 2009, Pages 237–239
(http://arxiv.org/abs/0901.2579v2, last revised Feb. 20, 2009)
" Studies of spin-½ theories in the framework of projective geometry
have been undertaken before. See, e.g., Ref. [4]. 1 "
" 1 These papers are rather mathematical and technical.
The authors of the first two papers discuss the Dirac equation
in terms of the Plucker-Klein correspondence between lines of
a three-dimensional projective space and points of a quadric
in a five-dimensional projective space. The last paper shows
that the Dirac equation bears a certain relation to Kummer’s
surface, viz., the structure of the Dirac ring of matrices is
related to that of Kummer’s 166 configuration . . . ."
[4]
O. Veblen
Proc. Natl. Acad. Sci. USA , 19 (1933), p. 503
Full Text via CrossRef
E.M. Bruins
Proc. Nederl. Akad. Wetensch. , 52 (1949), p. 1135
F.C. Taylor Jr., Master thesis, University of North Carolina
at Chapel Hill (1968), unpublished
A remark of my own on the structure of Kummer’s 166 configuration . . . .
See as well yesterday morning's post.
The Dream of the Expanded Field continues…
From Klein's 1893 Lectures on Mathematics —
"The varieties introduced by Wirtinger may be called Kummer varieties…."
— E. Spanier, 1956
From this journal on March 10, 2013 —
From a recent paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
"The Universal Kummer Threefold," by
Qingchun Ren, Steven V Sam, Gus Schrader, and Bernd Sturmfels —
Two such considerations —
Update of 10 PM ET March 7, 2014 —
The following slides by one of the "Kummer Threefold" authors give
some background related to the above 64-point vector space and
to the Weyl group of type E7, W (E7):
The Cayley reference is to "Algorithm for the characteristics of the
triple ϑ-functions," Journal für die Reine und Angewandte
Mathematik 87 (1879): 165-169. <http://eudml.org/doc/148412>.
To read this in the context of Cayley's other work, see pp. 441-445
of Volume 10 of his Collected Mathematical Papers .
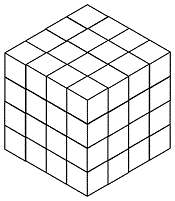
Denote the d-dimensional hypercube by
"… after coloring the sixty-four vertices of
alternately red and blue, we can say that
the sixteen pairs of opposite red vertices represent
the sixteen nodes of Kummer's surface, while
the sixteen pairs of opposite blue vertices
represent the sixteen tropes."
— From "Kummer's 166 ," section 12 of Coxeter's 1950
"Self-dual Configurations and Regular Graphs"
Just as the 4×4 square represents the 4-dimensional
hypercube
so the 4x4x4 cube represents the 6-dimensional
hypercube
For religious interpretations, see
Nanavira Thera (Indian) and
I Ching geometry (Chinese).
See also two professors in The New York Times
discussing images of the sacred in an op-ed piece
dated Sept. 26 (Yom Kippur).
… in posts tagged Kummer-Sept-2013.
Musical accompaniment suggested by
tonight's earlier Paradise Dreams —
A New Yorker piece from October 7th, 2023 —
"Terry Bisson's History of the Future" . . .
The "May 19th" name "was derived from the birthdays
of Ho Chi Minh and Malcolm X." — Wikipedia
And then there is the May 19 Gestalt . . .
For a prequel of sorts, see a May 19, 2023, arXiv paper —
Related Log24 reading: Other posts tagged Kummerhenge.
See also https://m759.net/wordpress/?s=Dirac+skew+anticommuting.
For fans of a different sort of space . . .
See also the Wikipedia article on Bloom.
See as well "The Thing and I."
See also a Log24 search for "The Path."
Related material from a similar search
for "Nanavira Thera" —
"I am glad you have discovered that the situation is comical:
ever since studying Kummer I have been, with some difficulty,
refraining from making that remark."
— Nanavira Thera, Seeking the Path [Early Letters, 17 July 1958].
From "A special configuration of 12 conics and generalized Kummer surfaces,"
by David Kohel, Xavier Roulleau, and Alessandra Sarti.
(arXiv:2004.11421 (math), submitted on 23 Apr 2020 (v1),
last revised 17 May 2021 (this version, v2)) —
"… we study the set C12 of conics that contain at least 6 points in P9. One has
Theorem 1. The set C12 has cardinality 12. Each conic in C12 contains exactly
6 points in P9 and through each point in P9 there are 8 conics. The sets (P9, C12)
form therefore a (98, 126)-configuration.
The configuration (P9, C12) has interesting symmetries, e.g. there are 8 conics
among the 12 passing through a fixed point q in P9 and the 8 points in P9 \ {q},
which form a 85 point-conic configuration. The freeness of the arrangement of
curves C12 is studied in [19], where we learned that this configuration has been
also independently discovered in [11]."
[11] Dolgachev I., Laface A., Persson U., Urzúa G.,
"Chilean configuration of conics, lines and points," preprint.
(arXiv:2008.09627 (math), submitted on 21 Aug 2020)
[19] Pokora P., Szemberg T.,
"Conic-line arrangements in the complex projective plane," preprint
(arXiv:2002.01760 (math), submitted on 5 Feb 2020 (v1),
last revised 10 Feb 2022 (this version, v3))
From Log24 posts tagged Art Space —
From a paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
“The Universal Kummer Threefold,” by
Qingchun Ren, Steven V Sam, Gus Schrader,
and Bernd Sturmfels —
Two such considerations —
The geometry of the 4×4 square may be associated with the name
Galois, as in "the Galois tesseract," or similarly with the name Kummer.
Here is a Google image search using the latter name —
(Click to enlarge.)
"Consider the six-dimensional vector space ( 𝔽2 )6
over the two-element field 𝔽2 ."
— Page 23 of "The Universal Kummer Threefold,"
arXiv:1208.1229v3, 12 June 2013, by Qingchun Ren,
Steven V. Sam, Gus Schrader, and Bernd Sturmfels.
An illustration of that space from 1981 —
The above recollection of the Kummer Threefold remark was suggested by
recent posts now tagged Smallfield . . .
|
"Third Man – an elderly American railway bum, "Art to which I fix my celebrated signature." — "Third Man" in Victor Snaith's play "Changing Stations"
|
If we read the above "art" as a scythe blade to which the "signature" —
Snaith ("the crooked handle or shaft of a scythe") — is attached,
an image of the late art critic Robert Hughes comes to mind:
That image of Hughes appeared here in a post of June 17, 2015 —
"Slow Art, Continued" — that also referenced the Kummer Threefold
paper above.
"My little horse must think it queer" — Robert Frost.
This is from a poem mentioned here on December 22, 2004,
in a post titled "The Longest Night."
Related material from December 21, 2004 —
And then there is the Timeless Square . . .
See "Framed" (May 30) and "In Memory of Ernst Eduard Kummer" (May 14).
The Importance of Being Ernst —
In the above Wikipedia revision today, the anonymous user “Redactedentity”
found that the article Kummer surface omitted Kummer’s first name
and so changed “authorlink=Ernst Kummer|last=Kummer” to
“authorlink=Ernst Kummer|last=Ernst Kummer.” This fixed the
omission but makes no sense as a statement of parameters.
“Redactedentity” was apparently unable to read the following page,
which explains that “last=” is for the author’s last name —
Of course, this revision may be merely an instance of trolling or of
the sort of humor sometimes found among people with the following
interests:
See also Pazouzou.
The "bricks" in posts tagged Octad Group suggest some remarks
from last year's HBO "Watchmen" series —
Related material — The two bricks constituting a 4×4 array, and . . .
"(this is the famous Kummer abstract configuration )"
— Igor Dolgachev, ArXiv, 16 October 2019.
As is this —
.
The phrase "octad group" does not, as one might reasonably
suppose, refer to symmetries of an octad (a "brick"), but
instead to symmetries of the above 4×4 array.
A related Broomsday event for the Church of Synchronology —
The 15 2-subsets of a 6-set correspond to the 15 points of PG(3,2).
(Cullinane, 1986*)
The 35 3-subsets of a 7-set correspond to the 35 lines of PG(3,2).
(Conwell, 1910)
The 56 3-subsets of an 8-set correspond to the 56 spreads of PG(3,2).
(Seidel, 1970)
Each correspondence above may have been investigated earlier than
indicated by the above dates , which are the earliest I know of.
See also Correspondences in this journal.
* The above 1986 construction of PG(3,2) from a 6-set also appeared
in the work of other authors in 1994 and 2002 . . .
Addendum at 5:09 PM suggested by an obituary today for Stephen Joyce:
See as well the word correspondences in
"James Joyce and the Hermetic Tradition," by William York Tindall
(Journal of the History of Ideas , Jan. 1954).
James R. Flynn (born in 1934), "is famous for his discovery of
the Flynn effect, the continued year-after-year increase of IQ
scores in all parts of the world." —Wikipedia
His son Eugene Victor Flynn is a mathematician, co-author
of the following chapter on the Kummer surface—
From "Six Significant Landscapes," by Wallace Stevens (1916) —
VI
Rationalists, wearing square hats,
Think, in square rooms,
Looking at the floor,
Looking at the ceiling.
They confine themselves
To right-angled triangles.
If they tried rhomboids,
Cones, waving lines, ellipses —
As, for example, the ellipse of the half-moon —
Rationalists would wear sombreros.
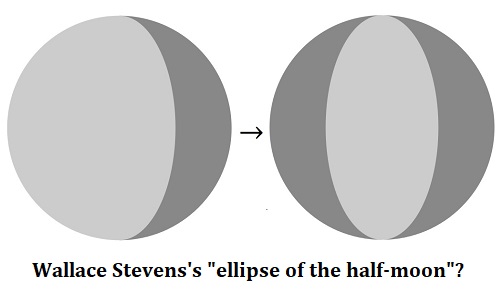
But see "cones, waving lines, ellipses" in Kummer's Quartic Surface
(by R. W. H. T. Hudson, Cambridge University Press, 1905) and their
intimate connection with the geometry of the 4×4 square.
The title refers to Calabi-Yau spaces.
Four Quartets
. . . Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness.
A less "cosmic" but still noteworthy code — The Golay code.
This resides in a 12-dimensional space over GF(2).
Related material from Plato and R. T. Curtis —
A related Calabi-Yau "Chinese jar" first described in detail in 1905 —
A figure that may or may not be related to the 4x4x4 cube that
holds the classical Chinese "cosmic code" — the I Ching —
ftp://ftp.cs.indiana.edu/pub/hanson/forSha/AK3/old/K3-pix.pdf
"This outer automorphism can be regarded as
the seed from which grow about half of the
sporadic simple groups…." — Noam Elkies
Closely related material —
The top two cells of the Curtis "heavy brick" are also
the key to the diamond-theorem correlation.
The previous post, "Dream of Plenitude," suggests . . .
"So here's to you, Nordstrom-Robinson . . . ."
Some related material in this journal — See a search for k6.gif.
Some related material from Harvard —
Elkies's "15 simple transpositions" clearly correspond to the 15 edges of
the complete graph K6 and to the 15 2-subsets of a 6-set.
For the connection to PG(3,2), see Finite Geometry of the Square and Cube.
The following "manifestation" of the 2-subsets of a 6-set might serve as
the desired Wikipedia citation —
See also the above 1986 construction of PG(3,2) from a 6-set
in the work of other authors in 1994 and 2002 . . .
This journal on the above date, October 17, 2008 —
“Every musician wants to do something of lasting quality,
something which will hold up for a long time, and
I guess we did it with ‘Stairway.'”
— Jimmy Page on “Stairway to Heaven“
Scholium —
"Kummer " in German means "sorrow."
Related material —
Other posts now tagged Dolmen.
The above cryptic search result indicates that there may
soon be a new Norwegian art installation based on this page
of Eddington (via Log24) —
See also other posts tagged Kummerhenge.
For Oslo artist Josefine Lyche, excerpts
from a Google image search today —
Material related to Lyche's experience as an adolescent with a ZX Spectrum computer —
Click "Hello World" for a larger image.
(Continued from this morning)
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
See also other Log24 posts tagged Kummerhenge.
A search this morning for articles mentioning the Miracle Octad Generator
of R. T. Curtis within the last year yielded an abstract for two talks given
at Hiroshima on March 8 and 9, 2018 —
|
http://www.math.sci.hiroshima-u.ac.jp/ branched/files/2018/abstract/Aitchison.txt
Iain AITCHISON Title: Construction of highly symmetric Riemann surfaces, related manifolds, and some exceptional objects, I, II Abstract: Since antiquity, some mathematical objects have played a special role, underpinning new mathematics as understanding deepened. Perhaps archetypal are the Platonic polyhedra, subsequently related to Platonic idealism, and the contentious notion of existence of mathematical reality independent of human consciousness. Exceptional or unique objects are often associated with symmetry – manifest or hidden. In topology and geometry, we have natural base points for the moduli spaces of closed genus 2 and 3 surfaces (arising from the 2-fold branched cover of the sphere over the 6 vertices of the octahedron, and Klein's quartic curve, respectively), and Bring's genus 4 curve arises in Klein's description of the solution of polynomial equations of degree greater than 4, as well as in the construction of the Horrocks-Mumford bundle. Poincare's homology 3-sphere, and Kummer's surface in real dimension 4 also play special roles. In other areas: we have the exceptional Lie algebras such as E8; the sporadic finite simple groups; the division algebras: Golay's binary and ternary codes; the Steiner triple systems S(5,6,12) and S(5,8,24); the Leech lattice; the outer automorphisms of the symmetric group S6; the triality map in dimension 8; and so on. We also note such as: the 27 lines on a cubic, the 28 bitangents of a quartic curve, the 120 tritangents of a sextic curve, and so on, related to Galois' exceptional finite groups PSL2(p) (for p= 5,7,11), and various other so-called `Arnol'd Trinities'. Motivated originally by the `Eightfold Way' sculpture at MSRI in Berkeley, we discuss inter-relationships between a selection of these objects, illustrating connections arising via highly symmetric Riemann surface patterns. These are constructed starting with a labeled polygon and an involution on its label set. Necessarily, in two lectures, we will neither delve deeply into, nor describe in full, contexts within which exceptional objects arise. We will, however, give sufficient definition and detail to illustrate essential inter-connectedness of those exceptional objects considered. Our starting point will be simplistic, arising from ancient Greek ideas underlying atomism, and Plato's concepts of space. There will be some overlap with a previous talk on this material, but we will illustrate with some different examples, and from a different philosophical perspective. Some new results arising from this work will also be given, such as an alternative graphic-illustrated MOG (Miracle Octad Generator) for the Steiner system S(5,8,24), and an alternative to Singerman – Jones' genus 70 Riemann surface previously proposed as a completion of an Arnol'd Trinity. Our alternative candidate also completes a Trinity whose two other elements are Thurston's highly symmetric 6- and 8-component links, the latter related by Thurston to Klein's quartic curve. |
See also yesterday morning's post, "Character."
Update: For a followup, see the next Log24 post.
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
Note also the four 4×4 arrays surrounding the central diamond
in the chi of the chi-rho page of the Book of Kells —
From a Log24 post
of March 17, 2012
"Interlocking, interlacing, interweaving"
— Condensed version of page 141 in Eddington's
1939 Philosophy of Physical Science
The phrase "Blue Dream" in the previous post
suggests a Web search for Traumnovelle .
That search yields an interesting weblog post
from 2014 commemorating the 1999 dies natalis
(birth into heaven) of St. Stanley Kubrick.
Related material from March 7, 2014,
in this journal —
That 2014 post was titled "Kummer Varieties." It is now tagged
"Kummerhenge." For some backstory, see other posts so tagged.
"Summerfield, Kummerhenge. Kummerhenge, Summerfield."
A clue to the relationship between the Kummer (16, 6)
configuration and the large Mathieu group M24 —
Related material —
See too the diamond-theorem correlation.
A Buddhist view —
“Just fancy a scale model of Being
made out of string and cardboard.”
— Nanavira Thera, 1 October 1957,
on a model of Kummer’s Quartic Surface
mentioned by Eddington
A Christian view —
A formal view —
From a Log24 search for High Concept:
See also Galois Tesseract.
"Just fancy a scale model of Being
made out of string and cardboard."
— Nanavira Thera, 1 October 1957,
on a model of Kummer's Quartic Surface
mentioned by Eddington
"… a treatise on Kummer's quartic surface."
The "super-mathematician" Eddington did not see fit to mention
the title or the author of the treatise he discussed.
See Hudson + Kummer in this journal.
See also posts tagged Dirac and Geometry.
The Institute for Advanced Study (IAS) at Princeton in its Fall 2015 Letter
revived "Beautiful Mathematics" as a title:
This ugly phrase was earlier used by Truman State University
professor Martin Erickson as a book title. See below.
In the same IAS Fall 2015 Letter appear the following remarks
by Freeman Dyson —
". . . a special case of a much deeper connection that Ian Macdonald
discovered between two kinds of symmetry which we call modular and affine.
The two kinds of symmetry were originally found in separate parts of science,
modular in pure mathematics and affine in physics. Modular symmetry is
displayed for everyone to see in the drawings of flying angels and devils
by the artist Maurits Escher. Escher understood the mathematics and got the
details right. Affine symmetry is displayed in the peculiar groupings of particles
created by physicists with high-energy accelerators. The mathematician
Robert Langlands was the first to conjecture a connection between these and
other kinds of symmetry. . . ." (Wikipedia link added.)
The adjective "modular" might aptly be applied to . . .
The adjective "affine" might aptly be applied to . . .
The geometry of the 4×4 square combines modular symmetry
(i.e., related to theta functions) with the affine symmetry above.
Hudson's 1905 discussion of modular symmetry (that of Rosenhain
tetrads and Göpel tetrads) in the 4×4 square used a parametrization
of that square by the digit 0 and the fifteen 2-subsets of a 6-set, but
did not discuss the 4×4 square as an affine space.
For the connection of the 15 Kummer modular 2-subsets with the 16-
element affine space over the two-element Galois field GF(2), see my note
of May 26, 1986, "The 2-subsets of a 6-set are the points of a PG(3,2)" —
— and the affine structure in the 1979 AMS abstract
"Symmetry invariance in a diamond ring" —
For some historical background on the symmetry investigations by
Dyson and Macdonald, see Dyson's 1972 article "MIssed Opportunities."
For Macdonald's own use of the words "modular" and "affine," see
Macdonald, I. G., "Affine Lie algebras and modular forms,"
Séminaire N. Bourbaki , Vol. 23 (1980-1981), Talk no. 577, pp. 258-276.
Scholia on the title — See Quantum + Mystic in this journal.
"In Vol. I of Structural Anthropology , p. 209, I have shown that
this analysis alone can account for the double aspect of time
representation in all mythical systems: the narrative is both
'in time' (it consists of a succession of events) and 'beyond'
(its value is permanent)." — Claude Lévi-Strauss, 1976
I prefer the earlier, better-known, remarks on time by T. S. Eliot
in Four Quartets , and the following four quartets (from
The Matrix Meets the Grid) —
.
From a Log24 post of June 26-27, 2017:
A work of Eddington cited in 1974 by von Franz —
See also Dirac and Geometry and Kummer in this journal.
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
See also Inscape in this journal and, for a related Chapel Hill thesis,
the post Kummer and Dirac.
https://www.shmoop.com/no-country-for-old-men/coin-symbol.html —
"You know the date on this coin?"
Related material —
This journal on March 7, 2014 —
From Klein’s 1893 Lectures on Mathematics —
“The varieties introduced by Wirtinger may be called
Kummer varieties….” — E. Spanier, 1956
From the "varieties introduced by Wirtinger" link above —
.
"For use of the Kummer surface in Buddhist metaphysics . . ."
is a phrase from a search in this journal for Nanavira.
See as well Buddhism in the previous post.
This post was suggested by the names* (if not the very abstruse
concepts ) in the Aug. 20, 2013, preprint "A Panoramic Overview
of Inter-universal Teichmuller Theory," by S. Mochizuki.
* Specifically, Jacobi and Kummer (along with theta functions).
I do not know of any direct connection between these names'
relevance to the writings of Mochizuki and their relevance
(via Hudson, 1905) to my own much more elementary studies of
the geometry of the 4×4 square.
For the title, see Icon Parking in a search for 54th in this journal.
For related iconic remarks, click on either image below.
.
This post was suggested by the Dec. 30, 2016, date of the
death in Nuremberg of mathematician Wolf Barth. The first
image above is from a mathematics-related work by
John von Neumann discussed here on that date.
See also Wolf Barth in this journal for posts that largely
concern not the above Barth, but an artist of the same name.
For posts on the mathematician only, see Barth + Kummer.
This post was suggested by the previous post — Four Dots —
and by the phrase "smallest perfect" in this journal.
Related material (click to enlarge) —
Detail —
From the work of Eddington cited in 1974 by von Franz —
See also Dirac and Geometry and Kummer in this journal.
Updates from the morning of June 27 —
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
The above four-element sets of black subsquares of a 4×4 square array
are 15 of the 60 Göpel tetrads , and 20 of the 80 Rosenhain tetrads , defined
by R. W. H. T. Hudson in his 1905 classic Kummer's Quartic Surface .
Hudson did not view these 35 tetrads as planes through the origin in a finite
affine 4-space (or, equivalently, as lines in the corresponding finite projective
3-space).
In order to view them in this way, one can view the tetrads as derived,
via the 15 two-element subsets of a six-element set, from the 16 elements
of the binary Galois affine space pictured above at top left.
This space is formed by taking symmetric-difference (Galois binary)
sums of the 15 two-element subsets, and identifying any resulting four-
element (or, summing three disjoint two-element subsets, six-element)
subsets with their complements. This process was described in my note
"The 2-subsets of a 6-set are the points of a PG(3,2)" of May 26, 1986.
The space was later described in the following —
"And as the characters in the meme twitch into the abyss
that is the sky, this meme will disappear into whatever
internet abyss swallowed MySpace."
—Staff writer Kamila Czachorowski, Harvard Crimson today
From Log24 posts tagged Art Space —
From a recent paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
“The Universal Kummer Threefold,” by
Qingchun Ren, Steven V Sam, Gus Schrader, and
Bernd Sturmfels —
Two such considerations —
*The Hudson of the title is the author of Kummer's Quartic Surface (1905).
The Rosenhain of the title is the author for whom Hudson's 4×4 diagrams
of "Rosenhain tetrads" are named. For the "complexity to simplicity" of
the title, see Roger Fry in the previous post.
(Continued … See the title in this journal, as well as Cube Bricks.)
Cube Bricks 1984 —
Related material —
Dirac and Geometry in this journal,
Kummer's Quartic Surface in this journal,
Nanavira Thera in this journal, and
The Razor's Edge and Nanavira Thera.
See as well Bill Murray's 1984 film "The Razor's Edge" …
Movie poster from 1984 —
"A thin line separates
love from hate,
success from failure,
life from death."
Three other dualities, from Nanavira Thera in 1959 —
"I find that there are, in every situation,
three independent dualities…."
(Click to enlarge.)
The previous post discussed the parametrization of
the 4×4 array as a vector 4-space over the 2-element
Galois field GF(2).
The 4×4 array may also be parametrized by the symbol
0 along with the fifteen 2-subsets of a 6-set, as in Hudson's
1905 classic Kummer's Quartic Surface —
Hudson in 1905:
These two ways of parametrizing the 4×4 array — as a finite space
and as an array of 2-element sets — were related to one another
by Cullinane in 1986 in describing, in connection with the Curtis
"Miracle Octad Generator," what turned out to be 15 of Hudson's
1905 "Göpel tetrads":
A recap by Cullinane in 2013:
Click images for further details.
The following passage by Igor Dolgachev (Good Friday, 2003)
seems somewhat relevant (via its connection to Kummer's 166 )
to previous remarks here on Dirac matrices and geometry —
Note related remarks from E. M. Bruins in 1959 —
A review of some recent posts on Dirac and geometry,
each of which mentions the late physicist Hendrik van Dam:
The first of these posts mentions the work of E. M. Bruins.
Some earlier posts that cite Bruins:
The authors Taormina and Wendland in the previous post
discussed some mathematics they apparently did not know was
related to a classic 1905 book by R. W. H. T. Hudson, Kummer's
Quartic Surface .
"This famous book is a prototype for the possibility
of explaining and exploring a many-faceted topic of
research, without focussing on general definitions,
formal techniques, or even fancy machinery. In this
regard, the book still stands as a highly recommendable,
unparalleled introduction to Kummer surfaces, as a
permanent source of inspiration and, last but not least,
as an everlasting symbol of mathematical culture."
— Werner Kleinert, Mathematical Reviews ,
as quoted at Amazon.com
Some 4×4 diagrams from that book are highly relevant to the
discussion by Taormina and Wendland of the 4×4 squares within
the 1974 Miracle Octad Generator of R. T. Curtis that were later,
in 1987, described by Curtis as pictures of the vector 4-space over
the two-element Galois field GF(2).
Hudson did not think of his 4×4 diagrams as illustrating a vector space,
but he did use them to picture certain subsets of the 16 cells in each
diagram that he called Rosenhain and Göpel tetrads .
Some related work of my own (click images for related posts)—
Rosenhain tetrads as 20 of the 35 projective lines in PG(3,2)
Göpel tetrads as 15 of the 35 projective lines in PG(3,2)
Related terminology describing the Göpel tetrads above
The hexagons above appear also in Gary W. Gibbons,
"The Kummer Configuration and the Geometry of Majorana Spinors,"
1993, in a cube model of the Kummer 166 configuration —
Related material — The Religion of Cubism (May 9, 2003).
From p. 34 of the preprint "Snapshots of Conformal Field Theory,"
by Katrin Wendland, arXiv, 11 April 2014 —
50. Gannon, T.: Much ado about Mathieu (arXiv:1211.5531 [math.RT])
85. Taormina, A., Wendland, K.: The overarching finite symmetry group
of Kummer surfaces in the Mathieu group M24. JHEP 08, 125 (2013)
86. Taormina, A., Wendland, K.: Symmetry-surfing the moduli space
of Kummer K3s (arXiv:1303.2931 [hep-th])
87. Taormina, A., Wendland, K.: A twist in the M24 moonshine story
(arXiv:1303.3221 [hep-th])
The Wendland paper was published on Jan. 7, 2015, in
Mathematical Aspects of Quantum Field Theories ,
edited by Damien Calaque and Thomas Strobl
(Springer Mathematical Physics Studies), pages 89-129.
Some background for my post of Nov. 20,
"Anticommuting Dirac Matrices as Skew Lines" —
His earlier paper that Bruins refers to, "Line Geometry
and Quantum Mechanics," is available in a free PDF.
For a biography of Bruins translated by Google, click here.
For some additional historical background going back to
Eddington, see Gary W. Gibbons, "The Kummer
Configuration and the Geometry of Majorana Spinors,"
pages 39-52 in Oziewicz et al., eds., Spinors, Twistors,
Clifford Algebras, and Quantum Deformations:
Proceedings of the Second Max Born Symposium held
near Wrocław, Poland, September 1992 . (Springer, 2012,
originally published by Kluwer in 1993.)
For more-recent remarks on quantum geometry, see a
paper by Saniga cited in today's update to my Nov. 20 post.
|
Saturday, March 14, 2015
Introduction to Yau
|
A note on the somewhat distant relation —
See also Kummer in this journal.
Some context for yesterday's post on a symplectic polarity —
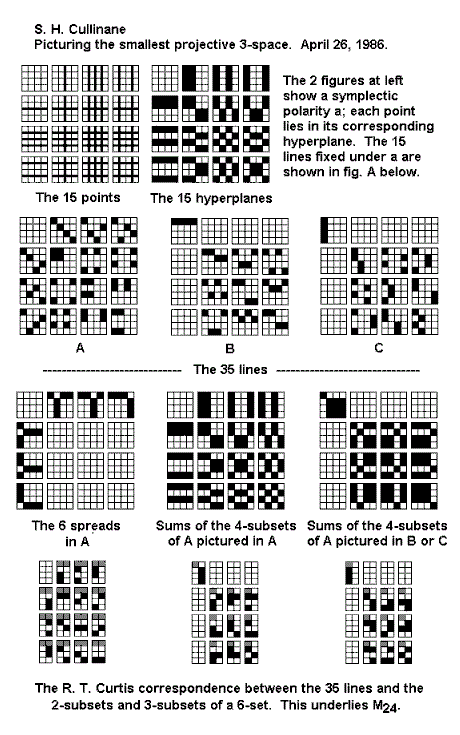
This 1986 note may or may not have inspired some remarks
of Wolf Barth in his foreword to the 1990 reissue of Hudson's
1905 Kummer's Quartic Surface .
See also the diamond-theorem correlation.
The title of the previous post, "Slow Art," is a phrase
of the late art critic Robert Hughes.
Example from mathematics:
Click the Barth passage to see it with its surrounding text.
Related material:
A short poem by several authors:
"The role of
the 16 singular points
on the Kummer surface
is now played by
the 64 singular points
on the Kummer threefold."
— From Remark 2.4 on page 9 of
"The Universal Kummer Threefold,"
by Qingchun Ren, Steven V Sam,
Gus Schrader, and Bernd Sturmfels,
http://arxiv.org/abs/1208.1229v3,
August 6, 2012 — June 12, 2013.
See also "Expanded Field" in this journal.
Illustration from "Sunday School," July 20, 2014.
Robin Williams and the Stages of Grief
A weblog post from Jan. 25, 2014 (click image to enlarge)—
Clues for a Mystery (Click links for more details)—
Clue 1: A June 11,* 2014, math death.
Clue 2: The answer is a surname.
Midrash for Will Hunting:
See Nanavira Thera at Wikipedia and space notes from September 2012.
* According to a (perhaps inaccurate) math department.
June 10, according to other sources cited by the department.
"Richard Hughes’s celebrated short novel is
a masterpiece of concentrated narrative."
— New York Review of Books on
A High Wind in Jamaica
As perhaps were, in their way, parts of the life
of the late Patrice Wymore Flynn, who reportedly
died at 87 on Saturday.
Deep backstory: See Colony of Santiago (Jamaica).
For the "mathematics" part of this post's title, see
Saturday's Log24 post on Kummer-surface terms
and a post of September 23, 2012.
Mathematical
Literary (also from May 18, 2010)
“Charting the Real Four-Qubit Pauli Group
via Ovoids of a Hyperbolic Quadric of PG(7,2),”
by Metod Saniga, Péter Lévay and Petr Pracna,
arXiv:1202.2973v2 [math-ph] 26 Jun 2012 —
P. 4— “It was found that Q +(5,2) (the Klein quadric)
has, up to isomorphism, a unique one — also known,
after its discoverer, as a Conwell heptad [18].
The set of 28 points lying off Q +(5,2) comprises
eight such heptads, any two having exactly one
point in common.”
P. 11— “This split reminds us of a similar split of
63 points of PG(5,2) into 35/28 points lying on/off
a Klein quadric Q +(5,2).”
[18] G. M. Conwell, Ann. Math. 11 (1910) 60–76
A similar split occurs in yesterday’s Kummer Varieties post.
See the 63 = 28 + 35 vectors of R8 discussed there.
For more about Conwell heptads, see The Klein Correspondence,
Penrose Space-Time, and a Finite Model.
For my own remarks on the date of the above arXiv paper
by Saniga et. al., click on the image below —
Walter Gropius
See also Rosenhain and Göpel in the Wikipedia
article Kummer surface, and in this journal.
Related material: user @hyperelliptic on Twitter.
(Continued from Mystery Box, Feb. 4, and Mystery Box II, Feb. 5.)
The Box
Inside the Box
Outside the Box
For the connection of the inside notation to the outside geometry,
see Desargues via Galois.
(For a related connection to curves and surfaces in the outside
geometry, see Hudson's classic Kummer's Quartic Surface and
Rosenhain and Göpel Tetrads in PG(3,2).)
"Let X equal the month of full bookstores."
— Proof by David Auburn
|
"Harvard Book Store is thrilled to welcome Stephen King for a special event to celebrate the release of Doctor Sleep , the long-anticipated sequel to 1977’s The Shining . Mr. King will read from and discuss the new book, followed by a Q&A with the audience at Memorial Church in Harvard Yard on September 27." |
See as well Corpse + Eliot and some remarks
on the mathematics of Kummer
from this September and from last September.
"… a list of group theoretic invariants
and their geometric incarnation…"
— David Lehavi on the Kummer 166 configuration in 2007
Related material —
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
"This is not theology; this is mathematics."
— Steven H. Cullinane on four quartets
To wit:
The Kummer 166 configuration is the configuration of sixteen
6-sets within a 4×4 square array of points in which each 6-set
is determined by one of the 16 points of the array and
consists of the 3 other points in that point's row and the
3 other points in that point's column.
See Configurations and Squares.
The Wikipedia article Kummer surface uses a rather poetic
phrase* to describe the relationship of the 166 to a number
of other mathematical concepts — "geometric incarnation."
Related material from finitegeometry.org —
* Apparently from David Lehavi on March 18, 2007, at Citizendium .
Mathematics:
A review of posts from earlier this month —
Wednesday, September 4, 2013
|
Narrative:
Aooo.
Happy birthday to Stephen King.
(Continued from yesterday)
The foreword by Wolf Barth in the 1990 Cambridge U. Press
reissue of Hudson's 1905 classic Kummer's Quartic Surface
covers some of the material in yesterday's post Moonshine.
The distinction that Barth described in 1990 was also described, and illustrated,
in my 1986 note "Picturing the smallest projective 3-space." The affine 4-space
over the the finite Galois field GF(2) that Barth describes was earlier described—
within a 4×4 array like that pictured by Hudson in 1905— in a 1979 American
Mathematical Society abstract, "Symmetry invariance in a diamond ring."
"The distinction between Rosenhain and Goepel tetrads
is nothing but the distinction between isotropic and
non-isotropic planes in this affine space over the finite field."
The 1990 paragraph of Barth quoted above may be viewed as a summary
of these facts, and also of my March 17, 2013, note "Rosenhain and Göpel
Tetrads in PG(3,2)."
The following excerpt from a January 20, 2013, preprint shows that
a Galois-geometry version of the large Desargues 154203 configuration,
although based on the nineteenth-century work of Galois* and of Fano,**
may at times have twenty-first-century applications.
Atkinson's paper does not use the square model of PG(3,2), which later
in 2013 provided a natural view of the large Desargues 154203 configuration.
See my own Classical Geometry in Light of Galois Geometry. Atkinson's
"subset of 20 lines" corresponds to 20 of the 80 Rosenhain tetrads
mentioned in that later article and pictured within 4×4 squares in Hudson's
1905 classic Kummer's Quartic Surface.
* E. Galois, definition of finite fields in "Sur la Théorie des Nombres,"
Bulletin des Sciences Mathématiques de M. Férussac,
Vol. 13, 1830, pp. 428-435.
** G. Fano, definition of PG(3,2) in "Sui Postulati Fondamentali…,"
Giornale di Matematiche, Vol. 30, 1892, pp. 106-132.
Today's previous post on coordinate systems
suggests a look at the phrase "Galois coordinates."
A search shows that the phrase, though natural,
has apparently not been used before 2011* for solutions
to what Hermann Weyl called "the relativity problem."
A thorough historical essay on Galois coordinatization
in this sense would require more academic resources
than I have available. It would likely describe a number
of applications of Galois-field coordinates to square
(and perhaps to cubical) arrays that were studied before
1976, the date of my Diamond Theory monograph.
But such a survey might not find any such pre-1976
coordinatization of a 4×4 array by the 16 elements
of the vector 4-space over the Galois field with two
elements, GF(2).
Such coordinatizations are important because of their
close relationship to the Mathieu group M 24 .
See a preprint by Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ," with its remark
denying knowledge of any such coordinatization
prior to a 1989 paper by R. T. Curtis.
Related material:
Some images related to Galois coordinates, excerpted
from a Google search today (click to enlarge)—
* A rather abstract 2011 paper that uses the phrase
"Galois coordinates" may have some implications
for the naive form of the relativity problem
related to square and cubical arrays.
From an arXiv preprint submitted July 18, 2011,
and last revised on March 11, 2013 (version 4):
"By our construction, this vector space is the dual
of our hypercube F24 built on I \ O9. The vector space
structure of the latter, to our knowledge, is first
mentioned by Curtis in [Cur89]. Hence altogether
our proposition 2.3.4 gives a novel geometric
meaning in terms of Kummer geometry to the known
vector space structure on I \ O9."
[Cur89] reference:
R. T. Curtis, "Further elementary techniques using
the miracle octad generator," Proc. Edinburgh
Math. Soc. 32 (1989), 345-353 (received on
July 20, 1987).
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ,"
arXiv.org > hep-th > arXiv:1107.3834
"First mentioned by Curtis…."
No. I claim that to the best of my knowledge, the
vector space structure was first mentioned by me,
Steven H. Cullinane, in an AMS abstract submitted
in October 1978, some nine years before the
Curtis article.
|
Update of the above paragraph on July 6, 2013—
No. The vector space structure was described by
The vector space structure as it occurs in a 4×4 array |
See Notes on Finite Geometry for some background.
See in particular The Galois Tesseract.
For the relationship of the 1978 abstract to Kummer
geometry, see Rosenhain and Göpel Tetrads in PG(3,2).
Some historical background for today's note on the geometry
underlying the Curtis Miracle Octad Generator (MOG):
The above incidence diagram recalls those in today's previous post
on the MOG, which is used to construct the large Mathieu group M24.
For some related material that is more up-to-date, search the Web
for Mathieu + Kummer .
Desargues' theorem according to a standard textbook:
"If two triangles are perspective from a point
they are perspective from a line."
The converse, from the same book:
"If two triangles are perspective from a line
they are perspective from a point."
Desargues' theorem according to Wikipedia
combines the above statements:
"Two triangles are in perspective axially [i.e., from a line]
if and only if they are in perspective centrally [i.e., from a point]."
A figure often used to illustrate the theorem,
the Desargues configuration , has 10 points and 10 lines,
with 3 points on each line and 3 lines on each point.
A discussion of the "if and only if" version of the theorem
in light of Galois geometry requires a larger configuration—
15 points and 20 lines, with 3 points on each line
and 4 lines on each point.
This large Desargues configuration involves a third triangle,
needed for the proof (though not the statement ) of the
"if and only if" version of the theorem. Labeled simply
"Desargues' Theorem," the large configuration is the
frontispiece to Volume I (Foundations) of Baker's 6-volume
Principles of Geometry .
Point-line incidence in this larger configuration is,
as noted in a post of April 1, 2013, described concisely
by 20 Rosenhain tetrads (defined in 1905 by
R. W. H. T. Hudson in Kummer's Quartic Surface ).
The third triangle, within the larger configuration,
is pictured below.
Background: Rosenhain and Göpel Tetrads in PG(3,2)
|
Introduction: The Large Desargues Configuration Added by Steven H. Cullinane on Friday, April 19, 2013 Desargues' theorem according to a standard textbook:
"If two triangles are perspective from a point The converse, from the same book:
"If two triangles are perspective from a line
Desargues' theorem according to Wikipedia
"Two triangles are in perspective axially [i.e., from a line]
A figure often used to illustrate the theorem,
A discussion of the "if and only if" version of the theorem
This large Desargues configuration involves a third triangle,
Point-line incidence in this larger configuration is,
The third triangle, within the larger configuration,
|
A connection discovered today (April 1, 2013)—
(Click to enlarge the image below.)
Update of April 18, 2013
Note that Baker's Desargues-theorem figure has three triangles,
ABC, A'B'C', A"B"C", instead of the two triangles that occur in
the statement of the theorem. The third triangle appears in the
course of proving, not just stating, the theorem (or, more precisely,
its converse). See, for instance, a note on a standard textbook for
further details.
(End of April 18, 2013 update.)
Update of April 14, 2013
See Baker's Proof (Edited for the Web) for a detailed explanation
of the above picture of Baker's Desargues-theorem frontispiece.
(End of April 14, 2013 update.)
Update of April 12, 2013
A different figure, from a site at National Tsing Hua University,
shows the three triangles of Baker's figure more clearly:
(End of update of April 12, 2013)
Update of April 13, 2013
Another in a series of figures illustrating
Desargues's theorem in light of Galois geometry:
See also the original Veblen-Young figure in context.
(End of update of April 13, 2013)
Rota's remarks, while perhaps not completely accurate, provide some context
for the above Desargues-Rosenhain connection. For some other context,
see the interplay in this journal between classical and finite geometry, i.e.
between Euclid and Galois.
For the recent context of the above finite-geometry version of Baker's Vol. I
frontispiece, see Sunday evening's finite-geometry version of Baker's Vol. IV
frontispiece, featuring the Göpel, rather than the Rosenhain, tetrads.
For a 1986 illustration of Göpel and Rosenhain tetrads (though not under
those names), see Picturing the Smallest Projective 3-Space.
In summary… the following classical-geometry figures
are closely related to the Galois geometry PG(3,2):
|
Volume I of Baker's Principles has a cover closely related to the Rosenhain tetrads in PG(3,2) |
Volume IV of Baker's Principles has a cover closely related to the Göpel tetrads in PG(3,2) |
|
Foundations (click to enlarge)
|
Higher Geometry (click to enlarge)
|
The 16-point affine Galois space:
Further properties of this space:
In Configurations and Squares, see the
discusssion of the Kummer 166 configuration.
Some closely related material:
For the first two pages, click here.
Yesterday's post Permanence dealt with the cube
as a symmetric model of the finite projective plane
PG(2,3), which has 13 points and 13 lines. The points
and lines of the finite geometry occur in the cube as
the 13 axes of symmetry and the 13 planes through
the center perpendicular to those axes. If the three
axes lying in a plane that cuts the cube in a hexagon
are supplemented by the axis perpendicular to that
plane, each plane is associated with four axes and,
dually, each axis is associated with four planes.
My web page on this topic, Cubist Geometries, was
written on February 27, 2010, and first saved to the
Internet Archive on Oct. 4, 2010.
For a more recent treatment of this topic that makes
exactly the same points as the 2010 page, see p. 218
of Configurations from a Graphical Viewpoint , by
Tomaž Pisanski and Brigitte Servatius, published by
Springer on Sept. 23, 2012 (date from both Google
Books and Amazon.com):
For a similar 1998 treatment of the topic, see Burkard Polster's
A Geometrical Picture Book (Springer, 1998), pp. 103-104.
The Pisanski-Servatius book reinforces my argument of Jan. 13, 2013,
that the 13 planes through the cube's center that are perpendicular
to the 13 axes of symmetry of the cube should be called the cube's
symmetry planes , contradicting the usual use of of that term.
That argument concerns the interplay between Euclidean and
Galois geometry. Pisanski and Servatius (and, in 1998, Polster)
emphasize the Euclidean square and cube as guides* to
describing the structure of a Galois space. My Jan. 13 argument
uses Galois structures as a guide to re-describing those of Euclid .
(For a similar strategy at a much more sophisticated level,
see a recent Harvard Math Table.)
Related material: Remarks on configurations in this journal
during the month that saw publication of the Pisanski-Servatius book.
* Earlier guides: the diamond theorem (1978), similar theorems for
2x2x2 (1984) and 4x4x4 cubes (1983), and Visualizing GL(2,p)
(1985). See also Spaces as Hypercubes (2012).
Last Wednesday's 11 PM post mentioned the
adjacency-isomorphism relating the 4-dimensional
hypercube over the 2-element Galois field GF(2) to
the 4×4 array made up of 16 square cells, with
opposite edges of the 4×4 array identified.
A web page illustrates this property with diagrams that
enjoy the Karnaugh property— adjacent vertices, or cells,
differ in exactly one coordinate. A brief paper by two German
authors relates the Karnaugh property to the construction
of a magic square like that of Dürer (see last Wednesday).
In a similar way (search the Web for Karnaugh + cube ),
vertex adjacency in the 6-dimensional hypercube over GF(2)
is isomorphic to cell adjacency in the 4x4x4 cube, with
opposite faces of the 4x4x4 cube identified.
The above cube may be used to illustrate some properties
of the 64-point Galois 6-space that are more advanced
than those studied by enthusiasts of "magic" squares
and cubes.
See
Those who prefer narrative to mathematics may
consult posts in this journal containing the word "Cuber."
In Like Flynn
From the Wall Street Journal site Friday evening—
| ESSAY September 21, 2012, 9:10 p.m. ET
Are We Really Getting Smarter? Americans’ IQ scores have risen steadily over the past century.
|
No, thank you. I prefer the ninth configuration as is—
Why? See Josefine Lyche’s art installation “Grids, you say?“
Her reference there to “High White Noon” is perhaps
related to the use of that phrase in this journal.
The phrase is from a 2010 novel by Don DeLillo.
See “Point Omega,” as well as Lyche’s “Omega Point,”
in this journal.
The Wall Street Journal author above, James R. Flynn (born in 1934),
“is famous for his discovery of the Flynn effect, the continued
year-after-year increase of IQ scores in all parts of the world.”
—Wikipedia
His son Eugene Victor Flynn is a mathematician, co-author
of the following chapter on the Kummer surface—
For use of the Kummer surface in Buddhist metaphysics, see last night’s
post “Occupy Space (continued)” and the letters of Nanavira Thera from the
late 1950s at nanavira.blogspot.com.
These letters, together with Lyche’s use of the phrase “high white noon,”
suggest a further quotation—
You know that it would be untrue
You know that I would be a liar
If I was to say to you
Girl, we couldn’t get much higher
See also the Kummer surface at the web page Configurations and Squares.
"The word 'space' has, as you suggest, a large number of different meanings."
— Nanavira Thera in [Early Letters. 136] 10.xii.1958
From that same letter (links added to relevant Wikipedia articles)—
|
Space (ākāsa) is undoubtedly used in the Suttas
Your second letter seems to suggest that the space |
A simpler metaphysical system along the same lines—
|
The theory, he had explained, was that the persona
— The Gameplayers of Zan , |
"I am glad you have discovered that the situation is comical:
ever since studying Kummer I have been, with some difficulty,
refraining from making that remark."
— Nanavira Thera, [Early Letters, 131] 17.vii.1958
The showmanship of Nicki Minaj at Sunday's
Grammy Awards suggested the above title,
that of a novel by the author of The Exorcist .
The Ninth Configuration —
The ninth* in a list of configurations—
"There is a (2d-1)d configuration
known as the Cox configuration."
— MathWorld article on "Configuration"
For further details on the Cox 326 configuration's Levi graph,
a model of the 64 vertices of the six-dimensional hypercube γ6 ,
see Coxeter, "Self-Dual Configurations and Regular Graphs,"
Bull. Amer. Math. Soc. Vol. 56, pages 413-455, 1950.
This contains a discussion of Kummer's 166 as it
relates to γ6 , another form of the 4×4×4 Galois cube.
See also Solomon's Cube.
* Or tenth, if the fleeting reference to 113 configurations is counted as the seventh—
and then the ninth would be a 153 and some related material would be Inscapes.
"Total grandeur of a total edifice,
Chosen by an inquisitor of structures
For himself. He stops upon this threshold,
As if the design of all his words takes form
And frame from thinking and is realized."
— Wallace Stevens, "To an Old Philosopher in Rome"
The following edifice may be lacking in grandeur,
and its properties as a configuration were known long
before I stumbled across a description of it… still…
"What we do may be small, but it has
a certain character of permanence…."
— G.H. Hardy, A Mathematician's Apology
The Kummer 166 Configuration
as seen by Kantor in 1969— (pdf, 2.5 MB)
For some background, see Configurations and Squares.
For some quite different geometry of the 4×4 square that is
original with me, see a page with that title. (The geometry's
importance depends in part on its connection with the
Miracle Octad Generator (MOG) of R.T. Curtis. I of course
had nothing to do with the MOG's discovery, but I do claim credit
for discovering some geometric properties of the 4×4 square
that constitutes two-thirds of the MOG as originally defined .)
Related material— The Schwartz Notes of June 1.
On this date 106 years ago…
Prefatory note from Hudson's classic Kummer's Quartic Surface ,
Cambridge University Press, 1905—
RONALD WILLIAM HENRY TURNBULL HUDSON would have
been twenty-nine years old in July of this year; educated at
St Paul's School, London, and at St John's College, Cambridge,
he obtained the highest honours in the public examinations of the
University, in 1898, 1899, 1900; was elected a Fellow of St John's
College in 1900; became a Lecturer in Mathematics at University
College, Liverpool, in 1902; was D.Sc. in the University of London
in 1903; and died, as the result of a fall while climbing in Wales,
in the early autumn of 1904….
A many-sided theory such as that of this volume is
generally to be won only by the work of many lives;
one who held so firmly the faith that the time is well spent
could ill be spared.
— H. F. Baker, 27 March 1905
For some more recent remarks related to the theory, see
Defining Configurations and its updates, March 20-27, 2011.
The On-Line Encyclopedia of Integer Sequences has an article titled "Number of combinatorial configurations of type (n_3)," by N.J.A. Sloane and D. Glynn.
From that article:
The following corrects the word "unique" in the example.
* This post corrects an earlier post, also numbered 14660 and dated 7 PM March 18, 2011, that was in error.
The correction was made at about 11:50 AM on March 20, 2011.
_____________________________________________________________
Update of March 21
The problem here is of course with the definition. Sloane and Glynn failed to include in their definition a condition that is common in other definitions of configurations, even abstract or purely "combinatorial" configurations. See, for instance, Configurations of Points and Lines , by Branko Grunbaum (American Mathematical Society, 2009), p. 17—
In the most general sense we shall consider combinatorial (or abstract) configurations; we shall use the term set-configurations as well. In this setting "points" are interpreted as any symbols (usually letters or integers), and "lines" are families of such symbols; "incidence" means that a "point" is an element of a "line". It follows that combinatorial configurations are special kinds of general incidence structures. Occasionally, in order to simplify and clarify the language, for "points" we shall use the term marks, and for "lines" we shall use blocks. The main property of geometric configurations that is preserved in the generalization to set-configurations (and that characterizes such configurations) is that two marks are incident with at most one block, and two blocks with at most one mark.
Whether or not omitting this "at most one" condition from the definition is aesthetically the best choice, it dramatically changes the number of configurations in the resulting theory, as the above (8_3) examples show.
Update of March 22 (itself updated on March 25)
For further background on configurations, see Dolgachev—
Note that the two examples Dolgachev mentions here, with 16 points and 9 points, are not unrelated to the geometry of 4×4 and 3×3 square arrays. For the Kummer and related 16-point configurations, see section 10.3, "The Three Biplanes of Order 4," in Burkard Polster's A Geometrical Picture Book (Springer, 1998). See also the 4×4 array described by Gordon Royle in an undated web page and in 1980 by Assmus and Sardi. For the Hesse configuration, see (for instance) the passage from Coxeter quoted in Quaternions in an Affine Galois Plane.
Update of March 27
See the above link to the (16,6) 4×4 array and the (16,6) exercises using this array in R.D. Carmichael's classic Introduction to the Theory of Groups of Finite Order (1937), pp. 42-43. For a connection of this sort of 4×4 geometry to the geometry of the diamond theorem, read "The 2-subsets of a 6-set are the points of a PG(3,2)" (a note from 1986) in light of R.W.H.T. Hudson's 1905 classic Kummer's Quartic Surface , pages 8-9, 16-17, 44-45, 76-77, 78-79, and 80.
Powered by WordPress
