For related material on the geometry of a 2×3 rectangular array —
a domino — see the previous post and also a search in this journal for attic .
Friday, July 21, 2023
The Domino Model: Tricksters in the Attic
Comments Off on The Domino Model: Tricksters in the Attic
Wednesday, June 29, 2022
The Battle of Pergamon Press
See as well Buranyi in the previous post and Pergamon in this journal.
Comments Off on The Battle of Pergamon Press
Thursday, June 2, 2022
The Separatrix: 6/2
Comments Off on The Separatrix: 6/2
Friday, April 29, 2022
Metamorphosis: Seed to Flower in New Yorker Propaganda
Illustration by Nicholas Konrad / The New Yorker April 28, 2022
Some related material from Harvard —
Comments Off on Metamorphosis: Seed to Flower in New Yorker Propaganda
Tuesday, April 26, 2022
The Nocciolo
Comments Off on The Nocciolo
Friday, June 30, 2017
Hurriedly Put Together
The previous post quoted one theologian on a book
by another theologian, saying its tone "is patronizing
and its arguments are hurriedly put together."
For a more leisurely sort of argument, see a 1995* remark
by a mathematician, Ronald Shaw, quoted here on the morning
of Tuesday, June 27, in an update at the end of the previous day's
post "Upgrading to Six" —
". . . recall the notions of Eddington (1936) . . . ."
* In "Finite Geometry, Dirac Groups and the
Table of Real Clifford Algebras," pages 59-99 of
R. Ablamowicz and P. Lounesto (eds.),
Clifford Algebras and Spinor Structures ,
Kluwer Academic Publishers, 1995.
Comments Off on Hurriedly Put Together
Tuesday, June 27, 2017
Monday, June 26, 2017
Upgrading to Six
This post was suggested by the previous post — Four Dots —
and by the phrase "smallest perfect" in this journal.
Related material (click to enlarge) —
Detail —
From the work of Eddington cited in 1974 by von Franz —
See also Dirac and Geometry and Kummer in this journal.
Updates from the morning of June 27 —
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
Comments Off on Upgrading to Six
Monday, November 11, 2013
The Mystic Hexastigm…
Or: The Nutshell
What about Pascal?
For some background on Pascal's mathematics,
not his wager, see…
Richmond, H. W.,
"On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics ,
Volume 31 (1900), pp. 125-160,
dated by Richmond March 30,1899
Richmond, H. W.,
"The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen ,
Volume 53 (1900), Issue 1-2, pp 161-176,
dated by Richmond February 1, 1899
See also Nocciolo in this journal.
Recall as well that six points in space may,
if constrained to lie on a circle, be given
a religious interpretation. Richmond's
six points are secular and more general.
Comments Off on The Mystic Hexastigm…
Tuesday, May 14, 2013
Raiders of the Lost Aleph
See Coxeter + Aleph in this journal.
Epigraph to "The Aleph," a 1945 story by Borges:
"O God! I could be bounded in a nutshell,
and count myself a King of infinite space…"
– Hamlet, II, 2
Comments Off on Raiders of the Lost Aleph
Tuesday, April 2, 2013
Rota in a Nutshell
"The proof of Desargues' theorem of projective geometry
comes as close as a proof can to the Zen ideal.
It can be summarized in two words: 'I see!' "
— Gian-Carlo Rota in Indiscrete Thoughts (1997)
Also in that book, originally from a review in Advances in Mathematics,
Vol. 84, Number 1, Nov. 1990, p. 136:
Related material:
Pascal and the Galois nocciolo ,
Conway and the Galois tesseract,
Gardner and Galois.
See also Rota and Psychoshop.
Comments Off on Rota in a Nutshell
Baker on Configurations
The geometry posts of Sunday and Monday have been
placed in finitegeometry.org as
Classical Geometry in Light of Galois Geometry.
Some background:
See Baker, Principles of Geometry , Vol. II, Note I
(pp. 212-218)—
On Certain Elementary Configurations, and
on the Complete Figure for Pappus's Theorem
and Vol. II, Note II (pp. 219-236)—
On the Hexagrammum Mysticum of Pascal.
Monday's elucidation of Baker's Desargues-theorem figure
treats the figure as a 154203 configuration (15 points,
4 lines on each, and 20 lines, 3 points on each).
Such a treatment is by no means new. See Baker's notes
referred to above, and
"The Complete Pascal Figure Graphically Presented,"
a webpage by J. Chris Fisher and Norma Fuller.
What is new in the Monday Desargues post is the graphic
presentation of Baker's frontispiece figure using Galois geometry :
specifically, the diamond theorem square model of PG(3,2).
See also Cremona's kernel, or nocciolo :
Baker on Cremona's approach to Pascal—
"forming, in Cremona's phrase, the nocciolo of the whole."
A related nocciolo :
Click on the nocciolo for some
geometric background.
Comments Off on Baker on Configurations
Thursday, March 14, 2013
Nutshell continued
For the new Jesuit pope (see previous post)
Now among Log24 posts tagged "Khora" is one
from July 15, 2010, dealing with a book called
Deconstruction in a Nutshell: A Conversation with
Jacques Derrida , edited and with a commentary by
John D. Caputo (Fordham University Press, 1997).
Related material:
"Khora is the felix culpa of a passion for the impossible,
the happy fault of a poetics of the possible, the heartless
heart of an ethical and religious eschatology.
Khora is the devil that justice demands we give his due."
— John D. Caputo, conclusion of "Abyssus Abyssum Invocat :
A Response to Kearney." Caputo's remarks followed
Richard Kearney's "Khora or God?," pp. 107-122 in
A Passion for the Impossible: John D. Caputo in Focus ,
edited by Mark Dooley, State University of New York Press,
Albany, 2003. See "Abyssus " on pp. 123-127.
See also other uses here of the phrase "In a Nutshell."
Comments Off on Nutshell continued
Friday, January 7, 2011
Coxeter and the Aleph
In a nutshell —
Epigraph to "The Aleph," a 1945 story by Borges:
O God! I could be bounded in a nutshell,
and count myself a King of infinite space…
— Hamlet, II, 2
The story in book form, 1949
A 2006 biography of geometer H.S.M. Coxeter:
The Aleph (implicit in a 1950 article by Coxeter):
The details:
Related material: Group Actions, 1984-2009.
Comments Off on Coxeter and the Aleph
Thursday, July 15, 2010
Brightness at Noon, continued
"What exactly was Point Omega?"
This is Robert Wright in Nonzero: The Logic of Human Destiny.
Wright is discussing not the novel Point Omega by Don DeLillo,
but rather a (related) concept of the Jesuit philosopher Pierre Teilhard de Chardin.
My own idiosyncratic version of a personal "point omega"—
The circular sculpture in the foreground
is called by the artist "The Omega Point."
This has been described as
"a portal that leads in or out of time and space."
For some other sorts of points, see the drawings
on the wall and Geometry Simplified—
The two points of the trivial affine space are represented by squares,
and the one point of the trivial projective space is represented by
a line segment separating the affine-space squares.
For related darkness at noon, see Derrida on différance
as a version of Plato's khôra—
The above excerpts are from a work on and by Derrida
published in 1997 by Fordham University,
a Jesuit institution— Deconstruction in a Nutshell—
For an alternative to the Villanova view of Derrida,
see Angels in the Architecture.
Comments Off on Brightness at Noon, continued
Sunday, February 21, 2010
Reflections, continued
"The eye you see him with is the same
eye with which he sees you."
– Father Egan on page 333
of Robert Stone's A Flag for Sunrise
(Knopf hardcover, 1981)
Part I– Bounded in a Nutshell
Ian McKellen at a mental hospital's diamond-shaped window in "Neverwas"
Part II– The Royal Castle
Ian McKellen at his royal castle's diamond-shaped window in "Neverwas"
Part III– King of Infinite Space
H.S.M. Coxeter crowns himself "King of Infinite Space"
Related material:
See Coxeter in this journal.
Comments Off on Reflections, continued
Wednesday, September 6, 2006
Wednesday September 6, 2006
Hamlet's Transformation
"O God, I could be bounded in a nutshell
and count myself a king of infinite space,
were it not that I have bad dreams."
— Hamlet
Background:
- Monday's "In a Nutshell,"
- Tuesday's "The King of Infinite Space," and
- this morning's "Bad Dreams."
"… Something have you heard
Of Hamlet's transformation; so call it,
Sith nor the exterior nor the inward man
Resembles that it was…."
The transformation:
Click on picture for details.
Related material:
Figures of Speech (June 7, 2006) and
Ursprache Revisited (June 9, 2006).
Comments Off on Wednesday September 6, 2006
Monday, September 4, 2006
Monday September 4, 2006
In a Nutshell:
The Seed
The Seed
"The symmetric group S6 of permutations of 6 objects is the only symmetric group with an outer automorphism….
This outer automorphism can be regarded as the seed from which grow about half of the sporadic simple groups…."
This "seed" may be pictured as
within what Burkard Polster has called "the smallest perfect universe"– PG(3,2), the projective 3-space over the 2-element field.
Related material: yesterday's entry for Sylvester's birthday.
Comments Off on Monday September 4, 2006
Sunday, September 3, 2006
Sunday September 3, 2006
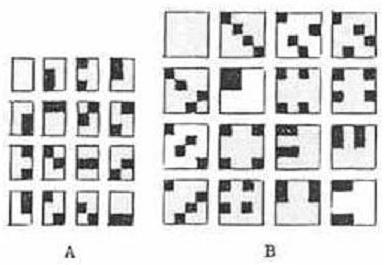
The following figure from a June 11, 1986, note illustrates Sylvester's "duads" and "synthemes" using the concept of an "inscape" (part B of the figure). As R. T. Curtis and Noam Elkies have explained, the duads and synthemes lead to constructions of many of the sporadic simple groups.
Comments Off on Sunday September 3, 2006
Sunday, January 15, 2006
Sunday January 15, 2006
Inscape
My entry for New Year's Day links to a paper by Robert T. Curtis*
from The Arabian Journal for Science and Engineering
(King Fahd University, Dhahran, Saudi Arabia),
Volume 27, Number 1A, January 2002.
From that paper:
"Combinatorially, an outer automorphism [of S6] can exist because the number of unordered pairs of 6 letters is equal to the number of ways in which 6 letters can be partitioned into three pairs. Which is to say that the two conjugacy classes of odd permutations of order 2 in S6 contain the same number of elements, namely 15. Sylvester… refers to the unordered pairs as duads and the partitions as synthemes. Certain collections of five synthemes… he refers to as synthematic totals or simply totals; each total is stabilized within S6 by a subgroup acting triply transitively on the 6 letters as PGL2(5) acts on the projective line. If we draw a bipartite graph on (15+15) vertices by joining each syntheme to the three duads it contains, we obtain the famous 8-cage (a graph of valence 3 with minimal cycles of length 8)…."
Here is a way of picturing the 8-cage and a related configuration of points and lines:
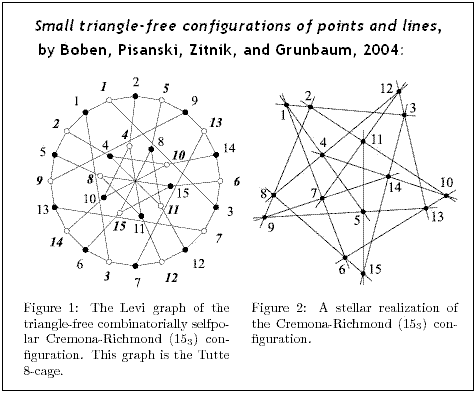
Diamond Theory shows that this structure
can also be modeled by an "inscape"
made up of subsets of a
4×4 square array:
The illustration below shows how the
points and lines of the inscape may
be identified with those of the
Cremona-Richmond configuration.
* "A fresh approach to the exceptional automorphism and covers of the symmetric groups"
Comments Off on Sunday January 15, 2006
Friday, September 24, 2004
Friday September 24, 2004
Readings for
Yom Kippur
The film Pi is, in part, about an alleged secret name of God that can be uttered only on Yom Kippur. This is my personal version of such a name– not an utterance, but instead a picture:
6:49:32 PM
Sept. 24, 2004
The Details:
Synthemes and Spreads (pdf)
(Appendix A of
"Classification of
Partial Spreads in PG(4,2),"
by Leonard H. Soicher et al.)
Comments Off on Friday September 24, 2004
Wednesday, September 3, 2003
Wednesday September 3, 2003
Reciprocity
From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity….
… E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies."
— William Boyd, review of Himmelfarb, New York Times Book Review, October 30, 1994
Last year's entry on this date:
|
Today's birthday:
"Mathematics is the music of reason."
Sylvester, a nineteenth-century mathematician, coined the phrase "synthematic totals" to describe some structures based on 6-element sets that R. T. Curtis has called "rather unwieldy objects." See Curtis's abstract, Symmetric Generation of Finite Groups, John Baez's essay, Some Thoughts on the Number 6, and my website, Diamond Theory. |
The picture above is of the complete graph
Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24.
If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See
Reciprocity and Reversal in Lao Tzu.
For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in
Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the
Click on the design for details.
Those who prefer a Jewish approach to physics can find the star of David, in the form of
A Graphical Representation
of the Dirac Algebra.
The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See
Tuesday, September 3, 2002
Tuesday September 3, 2002
Today's birthday: James Joseph Sylvester
"Mathematics is the music of reason." — J. J. Sylvester
Sylvester, a nineteenth-century mathematician, coined the phrase "synthematic totals" to describe some structures based on 6-element sets that R. T. Curtis has called "rather unwieldy objects." See Curtis's abstract, Symmetric Generation of Finite Groups, John Baez's essay, Some Thoughts on the Number 6, and my website, Diamond Theory. See also the abstract of a December 7, 2000, talk, Mathematics and the Art of M. C. Escher, in which Curtis notes that graphic designs can "often convey a mathematical idea more eloquently than pages of symbolism."
Comments Off on Tuesday September 3, 2002