Log24

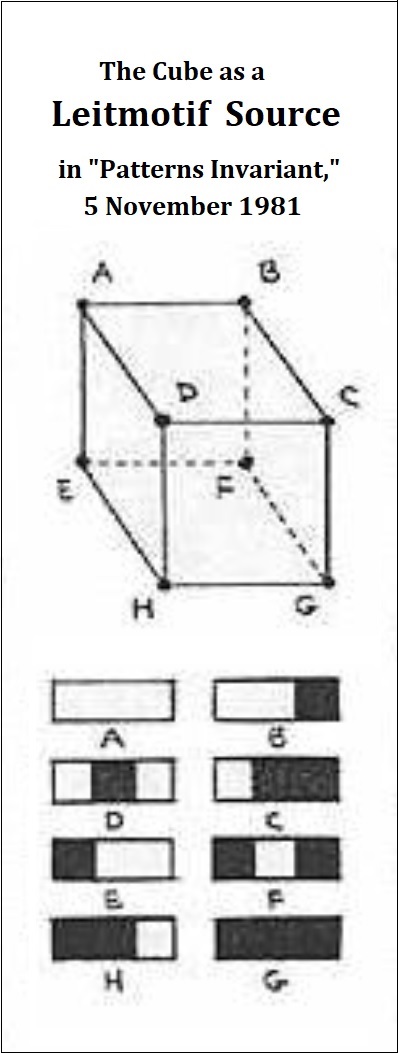
A 2008 statement on the order of the automorphism group of the Nordstrom-Robinson code—

"The Nordstrom-Robinson code has an unusually large group of automorphisms (of order 8! = 40,320) and is optimal in many respects. It can be found inside the binary Golay code."

— Jürgen Bierbrauer and Jessica Fridrich, preprint of "Constructing Good Covering Codes for Applications in Steganography," Transactions on Data Hiding and Multimedia Security III, Springer Lecture Notes in Computer Science, 2008, Volume 4920/2008, 1-22

A statement by Bierbrauer from 2004 has an error that doubles the above figure—

The automorphism group of the binary Golay code G is the simple Mathieu group M24 of order |M24| = 24 × 23 × 22 × 21 × 20 × 48 in its 5-transitive action on the 24 coordinates. As M24 is transitive on octads, the stabilizer of an octad has order |M24|/759 [=322,560]. The stabilizer of NR has index 8 in this group. It follows that NR admits an automorphism group of order |M24| / (759 × 8 ) = [?] 16 × 7! [=80,640]. This is a huge symmetry group. Its structure can be inferred from the embedding in G as well. The automorphism group of NR is a semidirect product of an elementary abelian group of order 16 and the alternating group A₇.

— Jürgen Bierbrauer, "Nordstrom-Robinson Code and A₇-Geometry," preprint dated April 14, 2004, published in Finite Fields and Their Applications , Volume 13, Issue 1, January 2007, Pages 158-170

The error is corrected (though not detected) later in the same 2004 paper—

In fact the symmetry group of the octacode is a semidirect product of an elementary abelian group of order 16 and the simple group GL(3, 2) of order 168. This constitutes a large automorphism group (of order 2688), but the automorphism group of NR is larger yet as we saw earlier (order 40,320).

For some background, see a well-known construction of the code from the Miracle Octad Generator of R.T. Curtis—

Click to enlarge:

For some context, see the group of order 322,560 in Geometry of the 4×4 Square.