The "Change Arises" phrase in the previous post suggests a sort of
architectural plan and elevation for the structure of the I Ching —
Saturday, June 8, 2024
Paradigms of Geometry
Comments Off on Paradigms of Geometry
Thursday, October 13, 2022
Prickly Paradigm Press
A search for background on the previous post's Eliot Weinberger yields,
from Berlin . . .
"In 2000 he was the first US writer to be honoured with
the Order of the Aztec Eagle from the Mexican government."
— "2000 zeichnete ihn die mexikanische Regierung als ersten
US-amerikanischen Autor mit dem Order of the Aztec Eagle aus."
The Aztec Eagle with a serpent in its beak, landing on a prickly pear,
is pictured on the flag of Mexico.
See also Weinberger's work at Prickly Paradigm Press.
Related material: Other Log24 posts tagged Prickly.
Comments Off on Prickly Paradigm Press
Wednesday, January 15, 2020
Paradigm Shift
Illustration, from a search in this journal for “Symplectic” —
.
Some background: Rift-design in this journal and …
Comments Off on Paradigm Shift
Wednesday, July 11, 2018
Plato and Paradigms, Revisited
"Plato thought nature but a spume that plays
Upon a ghostly paradigm of things"
— W. B. Yeats, "Among School Children"
Comments Off on Plato and Paradigms, Revisited
Sunday, April 16, 2017
Art Space Paradigm Shift
This post’s title is from the tags of the previous post —
The title’s “shift” is in the combined concepts of …
Space and Number
From Finite Jest (May 27, 2012):
The books pictured above are From Discrete to Continuous ,
by Katherine Neal, and Geometrical Landscapes , by Amir Alexander.
For some details of the shift, see a Log24 search for Boole vs. Galois.
From a post found in that search —
“Benedict Cumberbatch Says
a Journey From Fact to Faith
Is at the Heart of Doctor Strange“
— io9 , July 29, 2016
” ‘This man comes from a binary universe
where it’s all about logic,’ the actor told us
at San Diego Comic-Con . . . .
‘And there’s a lot of humor in the collision
between Easter [ sic ] mysticism and
Western scientific, sort of logical binary.’ “
[Typo now corrected, except in a comment.]
Comments Off on Art Space Paradigm Shift
Thursday, May 7, 2015
Paradigm for Pedagogues
Illustrations from a post of Feb. 17, 2011:
Plato’s paradigm in the Meno —
Changed paradigm in the diamond theorem (2×2 case) —
Comments Off on Paradigm for Pedagogues
Thursday, July 17, 2014
Paradigm Shift:
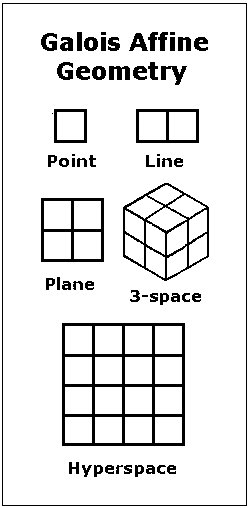
Continuous Euclidean space to discrete Galois space*
Euclidean space:
Counting symmetries in Euclidean space:
Galois space:
Counting symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
* For related remarks, see posts of May 26-28, 2012.
Comments Off on Paradigm Shift:
Friday, April 27, 2012
Paradigms Lost continues…
This post was suggested by Paradigms Lost
(a post cited here a year ago today),
by David Weinberger's recent essay "Shift Happens,"
and by today's opening of "The Raven."
David Weinberger in The Chronicle of Higher Education , April 22—
"… Kuhn was trying to understand how Aristotle could be such a brilliant natural scientist except when it came to understanding motion. Aristotle's idea that stones fall and fire rises because they're trying to get to their natural places seems like a simpleton's animism.
Then it became clear to Kuhn all at once. Ever since Newton, we in the West have thought movement changes an object's position in neutral space but does not change the object itself. For Aristotle, a change in position was a change in a quality of the object, and qualitative change tended toward an asymmetric actualization of potential: an acorn becomes an oak, but an oak never becomes an acorn. Motion likewise expressed a tendency for things to actualize their essence by moving to their proper place. With that, 'another initially strange part of Aristotelian doctrine begins to fall into place,' Kuhn wrote in The Road Since Structure ."
Dr. John Raven (of Raven's Progressive Matrices)—
"… these tools cannot be immediately applied within our current workplaces, educational systems, and public management systems because the operation of these systems is determined, not by personal developmental or societal needs, but by a range of latent, rarely discussed, and hard to influence sociological forces.
But this is not a cry of despair: It points to another topic which has been widely neglected by psychologists: It tells us that human behaviour is not mainly determined by internal properties— such as talents, attitudes, and values— but by external social forces. Such a transformation in psychological thinking and theorising is as great as the transformation Newton introduced into physics by noting that the movement of inanimate objects is not determined by internal, 'animistic,' properties of the objects but by invisible external forces which act upon them— invisible forces that can nevertheless be mapped, measured, and harnessed to do useful work for humankind.
So this brings us to our fourth conceptualisation and measurement topic: How are these social forces to be conceptualised, mapped, measured, and harnessed in a manner analogous to the way in which Newton made it possible to harness the destructive forces of the wind and the waves to enable sailing boats to get to their destinations?"
Before Newton, boats never arrived?
Comments Off on Paradigms Lost continues…
Sunday, June 26, 2011
Paradigms Lost
Continued from March 10, 2011 — A post that says
"If Galois geometry is thought of as a paradigm shift
from Euclidean geometry, both… the Kuhn cover
and the nine-point affine plane may be viewed…
as illustrating the shift."
Yesterday's posts The Fano Entity and Theology for Antichristmas,
together with this morning's New York Times obituaries (below)—
—suggest a Sunday School review from last year's
Devil's Night (October 30-31, 2010)—
|
Sunday, October 31, 2010 ART WARS – m759 @ 2:00 AM … There is a Cave – Paradise Lost , by John Milton
|
See also Ash Wednesday Surprise and Geometry for Jews.
Comments Off on Paradigms Lost
Thursday, March 10, 2011
Paradigms Lost
(Continued from February 19)
The cover of the April 1, 1970 second edition of The Structure of Scientific Revolutions , by Thomas S. Kuhn—
This journal on January 19, 2011—
If Galois geometry is thought of as a paradigm shift from Euclidean geometry,
both images above— the Kuhn cover and the nine-point affine plane—
may be viewed, taken together, as illustrating the shift. The nine subcubes
of the Euclidean 3x3x3 cube on the Kuhn cover do not form an affine plane
in the coordinate system of the Galois cube in the second image, but they
at least suggest such a plane. Similarly, transformations of a
non-mathematical object, the 1974 Rubik cube, are not Galois transformations,
but they at least suggest such transformations.
See also today's online Harvard Crimson illustration of problems of translation—
not unrelated to the problems of commensurability discussed by Kuhn.
Comments Off on Paradigms Lost
Saturday, February 19, 2011
Paradigms Lost
Harvard Science Review (Winter 1997) on Thomas Kuhn's
The Structure of Scientific Revolutions —
"…his language often portrays paradigms as cults
and the battle between paradigms as quasi-religious wars."
Related material: This journal's "Paradigms" on February 17th
and the following notes—
Comments Off on Paradigms Lost
Thursday, February 17, 2011
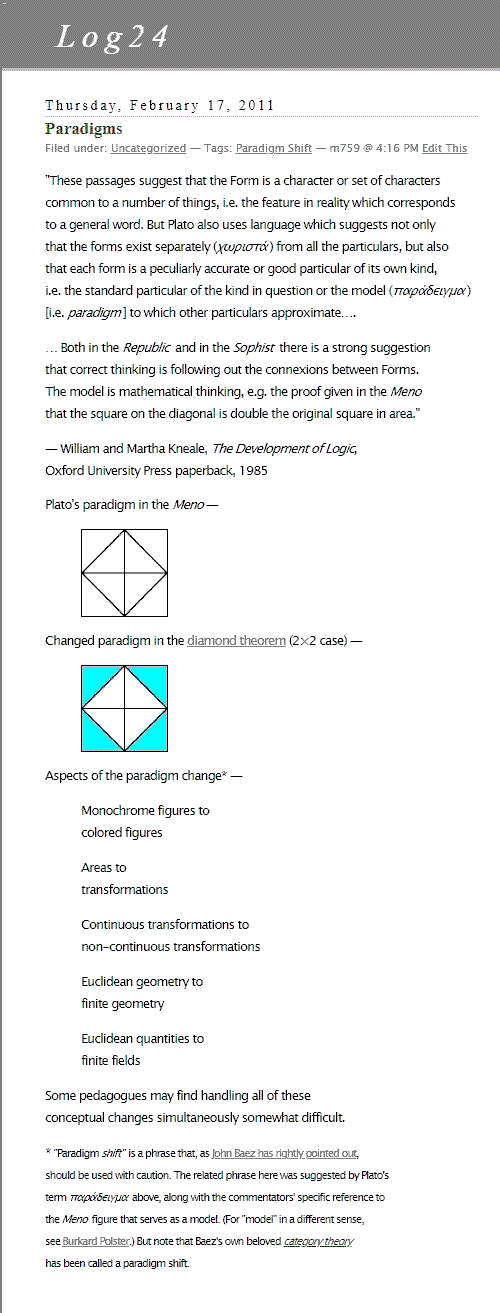
Paradigms
"These passages suggest that the Form is a character or set of characters
common to a number of things, i.e. the feature in reality which corresponds
to a general word. But Plato also uses language which suggests not only
that the forms exist separately (χωριστά ) from all the particulars, but also
that each form is a peculiarly accurate or good particular of its own kind,
i.e. the standard particular of the kind in question or the model (παράδειγμα )
[i.e. paradigm ] to which other particulars approximate….
… Both in the Republic and in the Sophist there is a strong suggestion
that correct thinking is following out the connexions between Forms.
The model is mathematical thinking, e.g. the proof given in the Meno
that the square on the diagonal is double the original square in area."
— William and Martha Kneale, The Development of Logic,
Oxford University Press paperback, 1985
Plato's paradigm in the Meno —
Changed paradigm in the diamond theorem (2×2 case) —
Aspects of the paradigm change* —
Monochrome figures to
colored figures
Areas to
transformations
Continuous transformations to
non-continuous transformations
Euclidean geometry to
finite geometry
Euclidean quantities to
finite fields
Some pedagogues may find handling all of these
conceptual changes simultaneously somewhat difficult.
* "Paradigm shift " is a phrase that, as John Baez has rightly pointed out,
should be used with caution. The related phrase here was suggested by Plato's
term παράδειγμα above, along with the commentators' specific reference to
the Meno figure that serves as a model. (For "model" in a different sense,
see Burkard Polster.) But note that Baez's own beloved category theory
has been called a paradigm shift.
Comments Off on Paradigms
Monday, June 17, 2024
Culture Note: Thinking Inside the Box
A more specific account of
Outside/Inside box-thinking . . .
|
A connection discovered on April 1, 2013 — (Click to enlarge the image below.)
|
Comments Off on Culture Note: Thinking Inside the Box
Sunday, July 16, 2023
Getting to Wow
A screenshot today of a May 31 NY Times review of a book on hacking —
Comments Off on Getting to Wow
Monday, February 6, 2023
Interality Studies
|
You, Xi-lin; Zhang, Peter. "Interality in Heidegger."
The term "interology" is meant as an interventional alternative to traditional Western ontology. The idea is to help shift people's attention and preoccupation from subjects, objects, and entities to the interzones, intervals, voids, constitutive grounds, relational fields, interpellative assemblages, rhizomes, and nothingness that lie between, outside, or beyond the so-called subjects, objects, and entities; from being to nothing, interbeing, and becoming; from self-identicalness to relationality, chance encounters, and new possibilities of life; from "to be" to "and … and … and …" (to borrow Deleuze's language); from the actual to the virtual; and so on. As such, the term wills nothing short of a paradigm shift. Unlike other "logoi," which have their "objects of study," interology studies interality, which is a non-object, a no-thing that in-forms and constitutes the objects and things studied by other logoi. |
Some remarks from this journal on April 1, 2015 —
Manifest O
|
| 83-06-21 | An invariance of symmetry The diamond theorem on a 4x4x4 cube, and a sketch of the proof. |
| 83-10-01 | Portrait of O A table of the octahedral group O using the 24 patterns from the 2×2 case of the diamond theorem. |
| 83-10-16 | Study of O A different way of looking at the octahedral group, using cubes that illustrate the 2x2x2 case of the diamond theorem. |
| 84-09-15 | Diamonds and whirls Block designs of a different sort — graphic figures on cubes. See also the University of Exeter page on the octahedral group O. |
The above site, finitegeometry.org/sc, illustrates how the symmetry
of various visual patterns is explained by what Zhang calls "interality."
Comments Off on Interality Studies
Sunday, April 17, 2022
Annals of Iconic Simplicity
The New York Times today has an obituary for
Kevin Lippert, the founder and publisher of
Princeton Architectural Press, who reportedly
died at 63 on March 29, 2022.
“'There was a space between the academic,
theory-heavy M.I.T. Press and the coffeetableism
of Rizzoli,' Mr. Lamster wrote, adding that
Princeton Architectural Press would fill the gap
with 'the voice of the young practitioner.'
Mr. Lippert championed emerging architects.
He published Steven Holl’s seminal architectural
manifesto, 'Anchoring,' in 1989, and wrote the
introduction to the book of the same name.
Mr. Holl, in a tribute to Mr. Lippert on his website,
called him 'a committed intellectual and impresario
for the culture of architecture.'”
— Katharine Q. Seelye, April 17, 2022, 2:21 p.m. ET
From the cited tribute to Lippert on Holl's website —
"An excerpt from his publisher’s foreword to Anchoring :
In its iconic simplicity, his work seems to be about
the language of architecture, not in the allusive sense
used by postmodernists nor in the paradigmatic sense
used by so-called 'deconstructivists' but at the level of
essences of tropes and morphs… He is the only
American architect of his generation to be directly
influenced by the main lines in modern philosophy and
music, that is to say, by the line leading from Husserl
through to Heidegger and by separate achievements
of Bartok and Schonberg ."
Actually, although the above "iconic simplicity" passage,
up to the ellipsis after "morphs," is from the foreword
by Lippert, the references that follow the ellipsis — to
Husserl, Heidegger, Bartok, and Schonberg — are not
from Lippert's foreword, but from the introduction by
one Kenneth Frampton —
From Google Books:
Bibliographic data —
Another architectural memorial, from the reported date of Lippert's death —
Comments Off on Annals of Iconic Simplicity
Saturday, March 26, 2022
Saturday, October 16, 2021
Prose for Pedagogues*
Comments Off on Prose for Pedagogues*
Monday, February 3, 2020
A Kuhnian Register
Ereignis in the Stanford Encyclopedia of Philosophy —
|
Further aspects of the essential unfolding of Being are revealed by what is perhaps the key move in the Contributions—a rethinking of Being in terms of the notion of Ereignis, a term translated variously as ‘event’ (most closely reflecting its ordinary German usage), ‘appropriation’, ‘appropriating event’, ‘event of appropriation’ or ‘enowning’. (For an analysis which tracks Heidegger's use of the term Ereignis at various stages of his thought, see Vallega-Neu 2010). The history of Being is now conceived as a series of appropriating events in which the different dimensions of human sense-making—the religious, political, philosophical (and so on) dimensions that define the culturally conditioned epochs of human history—are transformed. Each such transformation is a revolution in human patterns of intelligibility, so what is appropriated in the event is Dasein and thus the human capacity for taking-as (see e.g., Contributions 271: 343). Once appropriated in this way, Dasein operates according to a specific set of established sense-making practices and structures. In a Kuhnian register, one might think of this as the normal sense-making that follows a paradigm-shift. — Michael Wheeler, 2011 |
See as well "reordering" in Sunday evening's post Tetrads for McLuhan
and in a Log24 search for Reordering + Steiner.
Comments Off on A Kuhnian Register
Thursday, December 13, 2018
Space Art
For Oslo artist Josefine Lyche, excerpts
from a Google image search today —
Material related to Lyche's experience as an adolescent with a ZX Spectrum computer —
Click "Hello World" for a larger image.
Comments Off on Space Art
Wednesday, October 17, 2018
Aesthetics
|
From "The Phenomenology of Mathematical Beauty," The Lightbulb Mistake . . . . Despite the fact that most proofs are long, and despite our need for extensive background, we think back to instances of appreciating mathematical beauty as if they had been perceived in a moment of bliss, in a sudden flash like a lightbulb suddenly being lit. The effort put into understanding the proof, the background material, the difficulties encountered in unraveling an intricate sequence of inferences fade and magically disappear the moment we become aware of the beauty of a theorem. The painful process of learning fades from memory, and only the flash of insight remains. We would like mathematical beauty to consist of this flash; mathematical beauty should be appreciated with the instantaneousness of a lightbulb being lit. However, it would be an error to pretend that the appreciation of mathematical beauty is what we vaingloriously feel it should be, namely, an instantaneous flash. Yet this very denial of the truth occurs much too frequently. The lightbulb mistake is often taken as a paradigm in teaching mathematics. Forgetful of our learning pains, we demand that our students display a flash of understanding with every argument we present. Worse yet, we mislead our students by trying to convince them that such flashes of understanding are the core of mathematical appreciation. Attempts have been made to string together beautiful mathematical results and to present them in books bearing such attractive titles as The One Hundred Most Beautiful Theorems of Mathematics . Such anthologies are seldom found on a mathematician’s bookshelf. The beauty of a theorem is best observed when the theorem is presented as the crown jewel within the context of a theory. But when mathematical theorems from disparate areas are strung together and presented as “pearls,” they are likely to be appreciated only by those who are already familiar with them. The Concept of Mathematical Beauty The lightbulb mistake is our clue to understanding the hidden sense of mathematical beauty. The stark contrast between the effort required for the appreciation of mathematical beauty and the imaginary view mathematicians cherish of a flashlike perception of beauty is the Leitfaden that leads us to discover what mathematical beauty is. Mathematicians are concerned with the truth. In mathematics, however, there is an ambiguity in the use of the word “truth.” This ambiguity can be observed whenever mathematicians claim that beauty is the raison d’être of mathematics, or that mathematical beauty is what gives mathematics a unique standing among the sciences. These claims are as old as mathematics and lead us to suspect that mathematical truth and mathematical beauty may be related. Mathematical beauty and mathematical truth share one important property. Neither of them admits degrees. Mathematicians are annoyed by the graded truth they observe in other sciences. Mathematicians ask “What is this good for?” when they are puzzled by some mathematical assertion, not because they are unable to follow the proof or the applications. Quite the contrary. Mathematicians have been able to verify its truth in the logical sense of the term, but something is still missing. The mathematician who is baffled and asks “What is this good for?” is missing the sense of the statement that has been verified to be true. Verification alone does not give us a clue as to the role of a statement within the theory; it does not explain the relevance of the statement. In short, the logical truth of a statement does not enlighten us as to the sense of the statement. Enlightenment , not truth, is what the mathematician seeks when asking, “What is this good for?” Enlightenment is a feature of mathematics about which very little has been written. The property of being enlightening is objectively attributed to certain mathematical statements and denied to others. Whether a mathematical statement is enlightening or not may be the subject of discussion among mathematicians. Every teacher of mathematics knows that students will not learn by merely grasping the formal truth of a statement. Students must be given some enlightenment as to the sense of the statement or they will quit. Enlightenment is a quality of mathematical statements that one sometimes gets and sometimes misses, like truth. A mathematical theorem may be enlightening or not, just as it may be true or false. If the statements of mathematics were formally true but in no way enlightening, mathematics would be a curious game played by weird people. Enlightenment is what keeps the mathematical enterprise alive and what gives mathematics a high standing among scientific disciplines. Mathematics seldom explicitly acknowledges the phenomenon of enlightenment for at least two reasons. First, unlike truth, enlightenment is not easily formalized. Second, enlightenment admits degrees: some statements are more enlightening than others. Mathematicians dislike concepts admitting degrees and will go to any length to deny the logical role of any such concept. Mathematical beauty is the expression mathematicians have invented in order to admit obliquely the phenomenon of enlightenment while avoiding acknowledgment of the fuzziness of this phenomenon. They say that a theorem is beautiful when they mean to say that the theorem is enlightening. We acknowledge a theorem’s beauty when we see how the theorem “fits” in its place, how it sheds light around itself, like Lichtung — a clearing in the woods. We say that a proof is beautiful when it gives away the secret of the theorem, when it leads us to perceive the inevitability of the statement being proved. The term “mathematical beauty,” together with the lightbulb mistake, is a trick mathematicians have devised to avoid facing up to the messy phenomenon of enlightenment. The comfortable one-shot idea of mathematical beauty saves us from having to deal with a concept that comes in degrees. Talk of mathematical beauty is a cop-out to avoid confronting enlightenment, a cop-out intended to keep our description of mathematics as close as possible to the description of a mechanism. This cop-out is one step in a cherished activity of mathematicians, that of building a perfect world immune to the messiness of the ordinary world, a world where what we think should be true turns out to be true, a world that is free from the disappointments, ambiguities, and failures of that other world in which we live. |
How many mathematicians does it take to screw in a lightbulb?
Comments Off on Aesthetics
Thursday, August 30, 2018
Perception* of Space
* A footnote in memory of a dancer who reportedly died
yesterday, August 29 — See posts tagged Paradigm Shift.
"Birthday, death-day — what day is not both?" — John Updike
Comments Off on Perception* of Space
Monday, August 20, 2018
The Perception of Coincidence
Ellmann on Joyce and 'the perception of coincidence' —
"Samuel Beckett has remarked that to Joyce reality was a paradigm,
an illustration of a possibly unstatable rule. Yet perhaps the rule
can be surmised. It is not a perception of order or of love; more humble
than either of these, it is the perception of coincidence. According to
this rule, reality, no matter how much we try to manipulate it, can only
assume certain forms; the roulette wheel brings up the same numbers
again and again; everyone and everything shift about in continual
movement, yet movement limited in its possibilities."
— Richard Ellmann, James Joyce , rev. ed.. Oxford, 1982, p. 551
Comments Off on The Perception of Coincidence
Saturday, June 16, 2018
For June 16
"But perhaps the desire for story
is what gets us into trouble to begin with."
— Sarah Marshall on June 5, 2018
"Beckett wrote that Joyce believed fervently in
the significance of chance events and of
random connections. ‘To Joyce reality was a paradigm,
an illustration of a possibly unstateable rule…
According to this rule, reality, no matter how much
we try to manipulate it, can only shift about
in continual movement, yet movement
limited in its possibilities…’ giving rise to
‘the notion of the world where unexpected simultaneities
are the rule.’ In other words, a coincidence … is actually
just part of a continually moving pattern, like a kaleidoscope.
Or Joyce likes to put it, a ‘collideorscape’."
— Gabrielle Carey, "Breaking Up with James Joyce,"
Sydney Review of Books , 15 June 2018
Carey's carelessness with quotations suggests a look at another
author's quoting of Ellmann on Joyce —
Comments Off on For June 16
Saturday, September 30, 2017
Where Angels Fear to Tread
From the online New York Times this morning —
"Origin is Mr. Brown’s eighth novel. It finds his familiar protagonist,
the brilliant Harvard professor of symbology and religious iconography
Robert Langdon, embroiled once more in an intellectually challenging,
life-threatening adventure involving murderous zealots, shadowy fringe
organizations, paradigm-shifting secrets with implications for the future
of humanity, symbols within puzzles and puzzles within symbols and
a female companion who is super-smart and super-hot.
As do all of Mr. Brown’s works, the new novel does not shy away from
the big questions, but rather rushes headlong into them."
— Profile of Dan Brown by Sarah Lyall
See also yesterday's Log24 post on the Feast of St. Michael and All Angels.
Comments Off on Where Angels Fear to Tread
Monday, April 17, 2017
Saturday, April 15, 2017
Quanta Dating
Comments Off on Quanta Dating
Saturday, July 26, 2014
OOPs
Or: Two Rivets Short of a Paradigm
Detail from an author photo:
|
From rivet-rivet.net:
The philosopher Graham Harman is invested in re-thinking the autonomy of objects and is part of a movement called Object-Oriented-Philosophy (OOP). Harman wants to question the authority of the human being at the center of philosophy to allow the insertion of the inanimate into the equation. With the aim of proposing a philosophy of objects themselves, Harman puts the philosophies of Bruno Latour and Martin Heidegger in dialogue. Along these lines, Harman proposes an unconventional reading of the tool-being analysis made by Heidegger. For Harman, the term tool does not refer only to human-invented tools such as hammers or screwdrivers, but to any kind of being or thing such as a stone, dog or even a human. Further, he uses the terms objects, beings, tools and things, interchangeably, placing all on the same ontological footing. In short, there is no “outside world.” Harman distinguishes two characteristics of the tool-being: invisibility and totality. Invisibility means that an object is not simply used but is: “[an object] form(s) a cosmic infrastructure of artificial and natural and perhaps supernatural forces, power by which our last action is besieged.” For instance, nails, wooden boards and plumbing tubes do their work to keep a house “running” silently (invisibly) without being viewed or noticed. Totality means that objects do not operate alone but always in relation to other objects–the smallest nail can, for example, not be disconnected from wooden boards, the plumbing tubes or from the cement. Depending on the point of view of each entity (nail, tube, etc.) a different reality will emerge within the house. For Harman, “to refer to an object as a tool-being is not to say that it is brutally exploited as a means to an end, but only that it is torn apart by the universal duel between the silent execution of an object’s reality and the glistening aura of its tangible surface.” — From "The Action of Things," an M.A. thesis at the Center for Curatorial Studies, Bard College, by Manuela Moscoso, May 2011, edited by Sarah Demeuse |
From Wikipedia, a programming paradigm:
See also posts tagged Turing's Cathedral, and Alley Oop (Feb. 11, 2003).
Comments Off on OOPs
Wednesday, July 23, 2014
Sky Captain*
This is a post in memory of artist Otto Piene, who reportedly died
at 86 on Thursday, July 17, 2014, in Berlin.
*For the title, see Alternate Reality, a post of Saturday, July 19, 2014.
See also Piene and paradigms, and Paradigm Shift from the date of death
for Piene and Hartsfield.
Comments Off on Sky Captain*
Sunday, July 20, 2014
Sunday School
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Comments Off on Sunday School
Saturday, July 19, 2014
More or Less by Chance
Comments Off on More or Less by Chance
Thursday, July 10, 2014
Emperor
“Paradigm Talent Agency are supporting with casting.
Emperor is described as a look at a debauched world
of wealth, sex, manipulation and treason.”
— The Hollywood Reporter : “Cannes: Adrien Brody
to play Charles V in Lee Tamahori‘s ‘Emperor,'”
2:54 AM PST May 19, 2014, by Scott Roxborough
Related material from Santa Cruz, California:
“On or about or between 11/22/2013 and 11/24/2013….”
Related material from this journal:
“Fiction,” a post of St. Cecilia’s Day, 11/22/2013.
See, too, yesterday’s noon post “Nowhere” and
the April 27-28, 2013, posts tagged Around the Clock.
Comments Off on Emperor
Wednesday, July 18, 2012
Incommensurables
(Continued from Midsummer Eve)
"At times, bullshit can only be countered with superior bullshit."
— Norman Mailer, March 3, 1992, PBS transcript
"Just because it is a transition between incommensurables, the transition between competing paradigms cannot be made a step at a time, forced by logic and neutral experience. Like the gestalt switch, it must occur all at once (though not necessarily in an instant) or not at all."
— Thomas Kuhn, The Structure of Scientific Revolutions , 1962, as quoted in The Enneagram of Paradigm Shifting
"In the spiritual traditions from which Jung borrowed the term, it is not the SYMMETRY of mandalas that is all-important, as Jung later led us to believe. It is their capacity to reveal the asymmetry that resides at the very heart of symmetry."
I have little respect for Enneagram enthusiasts, but they do at times illustrate Mailer's maxim.
My own interests are in the purely mathematical properties of the number nine, as well as those of the next square, sixteen.
Those who prefer bullshit may investigate non-mathematical properties of sixteen by doing a Google image search on MBTI.
For bullshit involving nine, see (for instance) Einsatz in this journal.
For non-bullshit involving nine, sixteen, and "asymmetry that resides at the very heart of symmetry," see Monday's Mapping Problem continued. (The nine occurs there as the symmetric figures in the lower right nine-sixteenths of the triangular analogs diagram.)
For non-bullshit involving psychological and philosophical terminology, see James Hillman's Re-Visioning Psychology .
In particular, see Hillman's "An Excursion on Differences Between Soul and Spirit."
Comments Off on Incommensurables
Friday, June 22, 2012
Bowling in Diagon Alley
Josefine Lyche bowling (Facebook, June 12, 2012)
A professor of philosophy in 1984 on Socrates's geometric proof in Plato's Meno dialogue—
"These recondite issues matter because theories about mathematics have had a big place in Western philosophy. All kinds of outlandish doctrines have tried to explain the nature of mathematical knowledge. Socrates set the ball rolling…."
— Ian Hacking in The New York Review of Books , Feb. 16, 1984
The same professor introducing a new edition of Kuhn's Structure of Scientific Revolutions—
"Paradigms Regained" (Los Angeles Review of Books , April 18, 2012)—
"That is the structure of scientific revolutions: normal science with a paradigm and a dedication to solving puzzles; followed by serious anomalies, which lead to a crisis; and finally resolution of the crisis by a new paradigm. Another famous word does not occur in the section titles: incommensurability. This is the idea that, in the course of a revolution and paradigm shift, the new ideas and assertions cannot be strictly compared to the old ones."
The Meno proof involves inscribing diagonals in squares. It is therefore related, albeit indirectly, to the classic Greek discovery that the diagonals of a square are incommensurable with its sides. Hence the following discussion of incommensurability seems relevant.
See also von Fritz and incommensurability in The New York Times (March 8, 2011).
For mathematical remarks related to the 10-dot triangular array of von Fritz, diagonals, and bowling, see this journal on Nov. 8, 2011— "Stoned."
Comments Off on Bowling in Diagon Alley
Wednesday, April 18, 2012
Adam in Eden
…. and John Golding, an authority on Cubism who "courted abstraction"—
"Adam in Eden was the father of Descartes." — Wallace Stevens
Fictional symbologist Robert Langdon and a cube—
From a Log24 post, "Eightfold Cube Revisited,"
on the date of Golding's death—
A related quotation—
"… quaternions provide a useful paradigm
for studying the phenomenon of 'triality.'"
— David A. Richter's webpage Zometool Triality
See also quaternions in another Log24 post
from the date of Golding's death— Easter Act.
Comments Off on Adam in Eden
Thursday, March 1, 2012
Block That Metaphor:
The Cube Model and Peano Arithmetic
The eightfold cube model of the Fano plane may or may not have influenced a new paper (with the date Feb. 10, 2011, in its URL) on an attempted consistency proof of Peano arithmetic—
The Consistency of Arithmetic, by Storrs McCall
"Is Peano arithmetic (PA) consistent? This paper contains a proof that it is. …
Axiomatic proofs we may categorize as 'syntactic', meaning that they concern only symbols and the derivation of one string of symbols from another, according to set rules. 'Semantic' proofs, on the other hand, differ from syntactic proofs in being based not only on symbols but on a non-symbolic, non-linguistic component, a domain of objects. If the sole paradigm of 'proof ' in mathematics is 'axiomatic proof ', in which to prove a formula means to deduce it from axioms using specified rules of inference, then Gödel indeed appears to have had the last word on the question of PA-consistency. But in addition to axiomatic proofs there is another kind of proof. In this paper I give a proof of PA's consistency based on a formal semantics for PA. To my knowledge, no semantic consistency proof of Peano arithmetic has yet been constructed.
The difference between 'semantic' and 'syntactic' theories is described by van Fraassen in his book The Scientific Image :
"The syntactic picture of a theory identifies it with a body of theorems, stated in one particular language chosen for the expression of that theory. This should be contrasted with the alternative of presenting a theory in the first instance by identifying a class of structures as its models. In this second, semantic, approach the language used to express the theory is neither basic nor unique; the same class of structures could well be described in radically different ways, each with its own limitations. The models occupy centre stage." (1980, p. 44)
Van Fraassen gives the example on p. 42 of a consistency proof in formal geometry that is based on a non-linguistic model. Suppose we wish to prove the consistency of the following geometric axioms:
A1. For any two lines, there is at most one point that lies on both.
A2. For any two points, there is exactly one line that lies on both.
A3. On every line there lie at least two points.
The following diagram shows the axioms to be consistent:
Figure 1
The consistency proof is not a 'syntactic' one, in which the consistency of A1-A3 is derived as a theorem of a deductive system, but is based on a non-linguistic structure. It is a semantic as opposed to a syntactic proof. The proof constructed in this paper, like van Fraassen's, is based on a non-linguistic component, not a diagram in this case but a physical domain of three-dimensional cube-shaped blocks. ….
… The semantics presented in this paper I call 'block semantics', for reasons that will become clear…. Block semantics is based on domains consisting of cube-shaped objects of the same size, e.g. children's wooden building blocks. These can be arranged either in a linear array or in a rectangular array, i.e. either in a row with no space between the blocks, or in a rectangle composed of rows and columns. A linear array can consist of a single block, and the order of individual blocks in a linear or rectangular array is irrelevant. Given three blocks A, B and C, the linear arrays ABC and BCA are indistinguishable. Two linear arrays can be joined together or concatenated into a single linear array, and a rectangle can be re-arranged or transformed into a linear array by successive concatenation of its rows. The result is called the 'linear transformation' of the rectangle. An essential characteristic of block semantics is that every domain of every block model is finite. In this respect it differs from Tarski’s semantics for first-order logic, which permits infinite domains. But although every block model is finite, there is no upper limit to the number of such models, nor to the size of their domains.
It should be emphasized that block models are physical models, the elements of which can be physically manipulated. Their manipulation differs in obvious and fundamental ways from the manipulation of symbols in formal axiomatic systems and in mathematics. For example the transformations described above, in which two linear arrays are joined together to form one array, or a rectangle of blocks is re-assembled into a linear array, are physical transformations not symbolic transformations. …"
— Storrs McCall, Department of Philosophy, McGill University
See also…
- Finite Geometry and Physical Space,
- McCall in the book search of this morning's Salvation for Gopnik, and
- the post Declarations of Jan. 29, 2012.
Comments Off on Block That Metaphor:
Wednesday, December 21, 2011
The Purloined Diamond
Stephen Rachman on "The Purloined Letter"
"Poe’s tale established the modern paradigm (which, as it happens, Dashiell Hammett and John Huston followed) of the hermetically sealed fiction of cross and double-cross in which spirited antagonists pursue a prized artifact of dubious or uncertain value."
For one such artifact, the diamond rhombus formed by two equilateral triangles, see Osserman in this journal.
Some background on the artifact is given by John T. Irwin's essay "Mysteries We Reread…" reprinted in Detecting Texts: The Metaphysical Detective Story from Poe to Postmodernism .
Related material—
Mathematics vulgarizer Robert Osserman died on St. Andrew's Day, 2011.
A Rhetorical Question
"The past decade has been an exciting one in the world of mathematics and a fabulous one (in the literal sense) for mathematicians, who saw themselves transformed from the frogs of fairy tales— regarded with a who-would-want-to-kiss-that aversion, when they were noticed at all— into fascinating royalty, portrayed on stage and screen….
Who bestowed the magic kiss on the mathematical frog?"
A Rhetorical Answer
Above: Amy Adams in "Sunshine Cleaning"
Comments Off on The Purloined Diamond
Saturday, October 1, 2011
Like an Orb
It turns out that Fabrizio Palombi, author and editor of books on the late combinatorialist-philosopher Gian-Carlo Rota, is also an expert on the French charlatan Lacan. (For recent remarks related to Rota, see yesterday's Primordiality and the link "6.7 (June 7)" in today's The Crowe Sphere.)
"We all have our little mythologies."
— "Lacan’s Mathematics," by Amadou Guissé, Alexandre Leupin, and Steven D. Wallace (a preprint from the website of Steven D. Wallace, assistant professor of mathematics at Macon State College, Macon, GA.) A more extensive quote from "Lacan's Mathematics"—
Epistemological Cuts* or Births?
An epistemological cut can be described as the production of homonyms. For example, the word orb in Ptolemaic cosmology and the same word in the Kepler’s system, albeit similar, designate two entities that have nothing in common: the first one, in the Ancients’ cosmology, is a crystal sphere to which stars are attached; orb, for Kepler, is an ellipsis whose sole material existence is the algorithm describing its path. A cut becomes major when all word of different eras change meaning. A case in point is the cut between polytheism and monotheism (Judaism): the word god or god takes an entirely different meaning, and this change affects all areas of a vision of the world. From the non created world of the Ancients, inhabited by eternal Gods, we pass on to a world created by a unique God, who is outside of his creation. This cut affects all areas of thinking. However, mythology, albeit separated from the new vision by the cut, survives as an enduring residue. Our sexual thinking, for example, is essential mythological, as proven by the endurance of the Oedipus complex or our cult of this ancient deity called Eros. Love is inherently tied to what Freud called the omnipotence of thought or magical thinking.
Of course, the quintessential major epistemological cut for us is the break effectuated by modern science in the 17th century. All the names are affected by it: however, who can claim he or she has been entirely purged of pre-scientific reasoning? Despite us living in a scientific universe, we all have our little mythologies, residues of an era before the major epistemological cut.
Any modeling of major epistemological cuts, or paradigm changes as Thomas Kuhn would have it, has therefore to account at the same time for a complete break with past names (that is, new visions of the world) as well as the survival of old names and mythologies.
* For some background on this Marxist jargon, see Epistemological Break (La Coupure Épistémologique ) at the website Concept and Form: The Cahiers pour l’Analyse and Contemporary French Thought.
Comments Off on Like an Orb
Thursday, August 4, 2011
Midnight in Oslo
For Norway's Niels Henrik Abel (1802-1829)
on his birthday, August Fifth
(6 PM Aug. 4, Eastern Time, is 12 AM Aug. 5 in Oslo.)
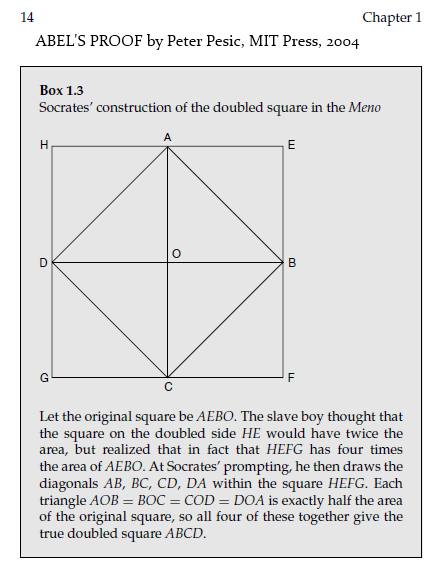
Plato's Diamond
The above version by Peter Pesic is from Chapter I of his book Abel's Proof , titled "The Scandal of the Irrational." Plato's diamond also occurs in a much later mathematical story that might be called "The Scandal of the Noncontinuous." The story—
Paradigms"These passages suggest that the Form is a character or set of characters common to a number of things, i.e. the feature in reality which corresponds to a general word. But Plato also uses language which suggests not only that the forms exist separately (χωριστά ) from all the particulars, but also that each form is a peculiarly accurate or good particular of its own kind, i.e. the standard particular of the kind in question or the model (παράδειγμα ) [i.e. paradigm ] to which other particulars approximate…. … Both in the Republic and in the Sophist there is a strong suggestion that correct thinking is following out the connexions between Forms. The model is mathematical thinking, e.g. the proof given in the Meno that the square on the diagonal is double the original square in area." – William and Martha Kneale, The Development of Logic , Oxford University Press paperback, 1985 Plato's paradigm in the Meno—
Changed paradigm in the diamond theorem (2×2 case) —
Aspects of the paradigm change— Monochrome figures to Areas to Continuous transformations to Euclidean geometry to Euclidean quantities to The 24 patterns resulting from the paradigm change—
Each pattern has some ordinary or color-interchange symmetry. This is the 2×2 case of a more general result. The patterns become more interesting in the 4×4 case. For their relationship to finite geometry and finite fields, see the diamond theorem. |
Related material: Plato's Diamond by Oslo artist Josefine Lyche.
“Plato’s Ghost evokes Yeats’s lament that any claim to worldly perfection inevitably is proven wrong by the philosopher’s ghost….”
— Princeton University Press on Plato’s Ghost: The Modernist Transformation of Mathematics (by Jeremy Gray, September 2008)
"Remember me to her."
— Closing words of the Algis Budrys novel Rogue Moon .
Background— Some posts in this journal related to Abel or to random thoughts from his birthday.
Comments Off on Midnight in Oslo
Thursday, May 5, 2011
Beyond Forgetfulness
From this journal on July 23, 2007—
|
It is not enough to cover the rock with leaves. We must be cured of it by a cure of the ground Or a cure of ourselves, that is equal to a cure
Of the ground, a cure beyond forgetfulness.
And if we ate the incipient colorings – Wallace Stevens, "The Rock" |
This quotation from Stevens (Harvard class of 1901) was posted here on when Daniel Radcliffe (i.e., Harry Potter) turned 18 in July 2007.
Other material from that post suggests it is time for a review of magic at Harvard.
On September 9, 2007, President Faust of Harvard
"encouraged the incoming class to explore Harvard’s many opportunities.
'Think of it as a treasure room of hidden objects Harry discovers at Hogwarts,' Faust said."
That class is now about to graduate.
It is not clear what "hidden objects" it will take from four years in the Harvard treasure room.
Perhaps the following from a book published in 1985 will help…
The March 8, 2011, Harvard Crimson illustrates a central topic of Metamagical Themas , the Rubik's Cube—
Hofstadter in 1985 offered a similar picture—
Hofstadter asks in his Metamagical introduction, "How can both Rubik's Cube and nuclear Armageddon be discussed at equal length in one book by one author?"
For a different approach to such a discussion, see Paradigms Lost, a post made here a few hours before the March 11, 2011, Japanese earthquake, tsunami, and nuclear disaster—
Whether Paradigms Lost is beyond forgetfulness is open to question.
Perhaps a later post, in the lighthearted spirit of Faust, will help. See April 20th's "Ready When You Are, C.B."
Comments Off on Beyond Forgetfulness
Wednesday, April 27, 2011
Atlas Shrugged
The title refers to an article in The Harvard Crimson , "Atlas to the Text," on March 8, 2011.
"Atlas to the Text," by Nicholas T. Rinehart —
"… a small set of undergraduates culminate their academic careers with a translation thesis. Ford is one such student, currently completing her edition of Euripides’ 'The Bacchae,' a Greek tragedy centered on the god Dionysus’ revenge against his mortal family."
"The guards return with Dionysus himself, disguised as his priest and the leader of the Asian maenads. Pentheus questions him, still not believing that Dionysus is a god. However, his questions reveal that he is deeply interested in the Dionysiac rites, which the stranger refuses to reveal fully to him. This greatly angers Pentheus, who has Dionysus locked up. However, being a god, he is quickly able to break free and creates more havoc, razing the palace of Pentheus to the ground in a giant earthquake and fire."
The illustration for the Crimson article formed part of a post in this journal, Paradigms Lost, on March 10—
Comments Off on Atlas Shrugged
Wednesday, April 20, 2011
Ready When You Are, C.B.
This journal at 5:48 PM EST on Thursday, March 10, 2011—
Paradigms Lost
(Continued from February 19)
The cover of the April 1, 1970 second edition of
The Structure of Scientific Revolutions , by Thomas S. Kuhn—
Note the quote on the cover—
"A landmark in intellectual history."— Science
This afternoon's online New York Times—
Google today, asked to "define:landmark," yields—
- A boundary line indicated by a stone, stake, etc.
(Deu 19:14; Deu 27:17; Pro 22:28; Pro 23:10; Job 24:2).
Landmarks could not be removed without incurring the severe displeasure of God.
sacred-texts.com/bib/ebd/ebd223.htm
Comments Off on Ready When You Are, C.B.
Wednesday, March 9, 2011
Ash Wednesday Surprise
Part 3 of 5 (See also Part 1 and Part 2) begins as follows…
"Incommensurable. It is a strange word. I wondered, why did Kuhn choose it? What was the attraction?
Here’s one clue. At the very end of 'The Road Since Structure,' a compendium of essays on Kuhn’s work, there is an interview with three Greek philosophers of science, Aristides Baltas, Kostas Gavroglu and Vassiliki Kindi. Kuhn provides a brief account of the historical origins of his idea. Here is the relevant segment of the interview.
T. KUHN: Look, 'incommensurability' is easy.
V. KINDI: You mean in mathematics?
T. KUHN: …When I was a bright high school mathematician and beginning to learn Calculus, somebody gave me—or maybe I asked for it because I’d heard about it—there was sort of a big two-volume Calculus book by, I can’t remember whom. And then I never really read it. I read the early parts of it. And early on it gives the proof of the irrationality of the square root of 2. And I thought it was beautiful. That was terribly exciting, and I learned what incommensurability was then and there. So, it was all ready for me, I mean, it was a metaphor but it got at nicely what I was after. So, that’s where I got it.
'It was all ready for me.' I thought, 'Wow.' The language was suggestive. I imagined √2 provocatively dressed, its lips rouged. But there was an unexpected surprise. The idea didn’t come from the physical sciences or philosophy or linguistics, but from mathematics ."
A footnote from Morris (no. 29)—
"Those who are familiar with the proof [of irrationality] certainly don’t want me to explain it here; likewise, those who are unfamiliar with it don’t want me to explain it here, either. There are many simple proofs in many histories of mathematics — E.T. Bell, Sir Thomas Heath, Morris Kline, etc., etc. Barry Mazur offers a proof in his book, 'Imagining Numbers (particularly the square root of minus fifteen),' New York, NY: Farrar, Straus and Giroux. 2003, 26ff. And there are two proofs in his essay, 'How Did Theaetetus Prove His Theorem?', available on Mazur’s Harvard Web site."
There may, actually, be a few who do want the proof. They may consult the sources Morris gives, or the excellent description by G.H. Hardy in A Mathematician's Apology , or, perhaps best of all for present purposes, the proof as described in a "sort of a big two-volume Calculus book" (perhaps the one Kuhn mentioned)… See page 6 and page 7 of Volume One of Richard Courant's classic Differential and Integral Calculus (second edition, 1937, reprinted many times through 1970, and again in a Wiley Classics Library Edition in 1988).
Comments Off on Ash Wednesday Surprise
Monday, March 7, 2011
Point Taken
Recommended— An essay (part 1 of 5 parts) in today's New York TImes—
|
I don’t want to die in |
"I agree with one of the earlier commenters that this is a piece of fine literary work. And in response to some of those who have wondered 'WHAT IS THE POINT?!' of this essay, I would like to say: Must literature always answer that question for us (and as quickly and efficiently as possible)?"
For an excellent survey of the essay's historical context, see The Stanford Encyclopedia of Philosophy article
"The Incommensurability of Scientific Theories,"
First published Wed., Feb. 25, 2009,
by Eric Oberheim and Paul Hoyningen-Huene.
Related material from this journal—
Paradigms, Paradigms Lost, and a search for "mere geometry." This last includes remarks contrasting Euclid's definition of a point ("that which has no parts") with a later notion useful in finite geometry.
See also (in the spirit of The Abacus Conundrum )…
(Note the Borges epigraph above.)
Comments Off on Point Taken
Thursday, July 16, 2009
Thursday July 16, 2009
The White Itself
David Ellerman has written that
"The notion of a concrete universal occurred in Plato's Theory of Forms [Malcolm 1991]."
A check shows that Malcolm indeed discussed this notion ("the Form as an Ideal Individual"), but not under the name "concrete universal."
See Plato on the Self-Predication of Forms, by John Malcolm, Oxford U. Press, 1991.
From the publisher's summary:
"Malcolm…. shows that the middle dialogues do indeed take Forms to be both universals and paradigms…. He shows that Plato's concern to explain how the truths of mathematics can indeed be true played an important role in his postulation of the Form as an Ideal Individual."
Ellerman also cites another discussion of Plato published by Oxford:
For a literary context, see W. K. Wimsatt, Jr., "The Structure of the Concrete Universal," Ch. 6 in Literary Theory: An Anthology, edited by Julie Rivkin and Michael Ryan, Wiley-Blackwell, 2004.
Other uses of the phrase "concrete universal"– Hegelian and/or theological– seem rather distant from the concerns of Plato and Wimsatt, and are best left to debates between Marxists and Catholics. (My own sympathies are with the Catholics.)
Two views of "the white itself" —
"So did God cause the big bang? Overcome by metaphysical lassitude, I finally reach over to my bookshelf for The Devil's Bible. Turning to Genesis I read: 'In the beginning there was nothing. And God said, 'Let there be light!' And there was still nothing, but now you could see it.'" -- Jim Holt, Big-Bang Theology, Slate's "High Concept" department
"The world was warm and white when I was born: Beyond the windowpane the world was white, A glaring whiteness in a leaded frame, Yet warm as in the hearth and heart of light." -- Delmore Schwartz
Comments Off on Thursday July 16, 2009
Tuesday, March 17, 2009
Tuesday March 17, 2009
Deep Structures
The Square of Oppositon
at Stanford Encylopedia of Philosophy
The Square of Opposition
in its original form
"The diagram above is from a ninth century manuscript of Apuleius' commentary on Aristotle's Perihermaneias, probably one of the oldest surviving pictures of the square."
— Edward Buckner at The Logic Museum
From the webpage "Semiotics for Beginners: Paradigmatic Analysis," by Daniel Chandler:
The Semiotic Square
"The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully (Greimas 1987,* xiv, 49). The semiotic square is intended to map the logical conjunctions and disjunctions relating key semantic features in a text. Fredric Jameson notes that 'the entire mechanism… is capable of generating at least ten conceivable positions out of a rudimentary binary opposition' (in Greimas 1987,* xiv). Whilst this suggests that the possibilities for signification in a semiotic system are richer than the either/or of binary logic, but that [sic] they are nevertheless subject to 'semiotic constraints' – 'deep structures' providing basic axes of signification."
* Greimas, Algirdas (1987): On Meaning: Selected Writings in Semiotic Theory (trans. Paul J Perron & Frank H Collins). London: Frances Pinter
Another version of the semiotic square:
Krauss says that her figure "is, of course, a Klein Group."
Here is a more explicit figure representing the Klein group:
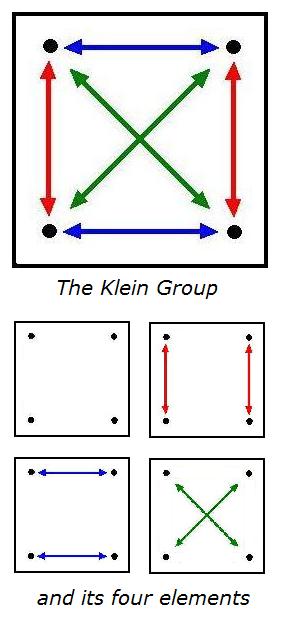
There is also the logical
diamond of opposition —
A semiotic (as opposed to logical)
diamond has been used to illustrate
remarks by Fredric Jameson,
a Marxist literary theorist:
|
"Introduction to Algirdas Greimas, Module on the Semiotic Square," by Dino Felluga at Purdue University–
The semiotic square has proven to be an influential concept not only in narrative theory but in the ideological criticism of Fredric Jameson, who uses the square as "a virtual map of conceptual closure, or better still, of the closure of ideology itself" ("Foreword"* xv). (For more on Jameson, see the [Purdue University] Jameson module on ideology.) Greimas' schema is useful since it illustrates the full complexity of any given semantic term (seme). Greimas points out that any given seme entails its opposite or "contrary." "Life" (s1) for example is understood in relation to its contrary, "death" (s2). Rather than rest at this simple binary opposition (S), however, Greimas points out that the opposition, "life" and "death," suggests what Greimas terms a contradictory pair (-S), i.e., "not-life" (-s1) and "not-death" (-s2). We would therefore be left with the following semiotic square (Fig. 1): 
As Jameson explains in the Foreword to Greimas' On Meaning, "-s1 and -s2"—which in this example are taken up by "not-death" and "not-life"—"are the simple negatives of the two dominant terms, but include far more than either: thus 'nonwhite' includes more than 'black,' 'nonmale' more than 'female'" (xiv); in our example, not-life would include more than merely death and not-death more than life.
* Jameson, Fredric. "Foreword." On Meaning: Selected Writings in Semiotic Theory. By Algirdas Greimas. Trans. Paul J. Perron and Frank H. Collins. Minneapolis: U of Minnesota P, 1976.
|
"The Game in the Ship cannot be approached as a job, a vocation, a career, or a recreation. To the contrary, it is Life and Death itself at work there. In the Inner Game, we call the Game Dhum Welur, the Mind of God."
— The Gameplayers of Zan, by M.A. Foster
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon,
Gravity's Rainbow
Crosses used by semioticians
to baffle their opponents
are illustrated above.
Some other kinds of crosses,
and another kind of opponent:
|
Monday, July 11, 2005
Logos
for St. Benedict's Day Click on either of the logos below for religious meditations– on the left, a Jewish meditation from the Conference of Catholic Bishops; on the right, an Aryan meditation from Stormfront.org.
Both logos represent different embodiments of the "story theory" of truth, as opposed to the "diamond theory" of truth. Both logos claim, in their own ways, to represent the eternal Logos of the Christian religion. I personally prefer the "diamond theory" of truth, represented by the logo below.
See also the previous entry Sunday, July 10, 2005
Mathematics
and Narrative Click on the title for a narrative about
Nikolaos K. Artemiadis,
"First of all, I'd like to
— Remark attributed to Plato
|
||||||||
Comments Off on Tuesday March 17, 2009
Wednesday, December 26, 2007
Wednesday December 26, 2007
A Wonderful Life
Part I:
Language Games
on December 19:
Language Games
on December 19:
See also the noir entry on "Nightmare Alley" for Winter Solstice 2002, as well as a solstice-related commentary on I Ching Hexagram 41, Decrease. |
Part II:
Language Game
on Christmas Day
Pennsylvania Lottery
December 25, 2007:
Part III:
A Wonderful Life
The Pennsylvania Lottery on Christmas at mid-day paired the number of the I Ching Hexagram 41, "Decrease," with the number 2911, which may be interpreted as a reference to I Chronicles 29:11—
"Thine, O LORD is the greatness, and the power, and the glory, and the victory, and the majesty: for all that is in the heaven and in the earth is thine; thine is the kingdom, O LORD, and thou art exalted as head above all."
This verse is sometimes cited as influencing the Protestant conclusion of the Lord's Prayer:
"Thine is the kingdom, and the power, and the glory, forever" (Mt 6.13b; compare 1 Chr 29.11-13)….
This traditional epilogue to the Lord's prayer protects the petition for the coming of the kingdom from being understood as an exorcism, which we derive from the Jewish prayer, the Kaddish, which belonged at the time to the synagogical liturgy.
The Pennsylvania Lottery on Christmas evening paired 173 with the beastly number 0666. The latter number suggests that perhaps being "understood as an exorcism" might not, in this case, be such a bad thing. What, therefore, might "173" have to do with exorcism? A search in the context of the phrase "language games" yields a reference to Wittgenstein's Zettel, section 173:
From Charles L. Creegan, Wittgenstein and Kierkegaard:
Language-
games give general guidelines of the application of language. Wittgenstein suggests that there are innumerably many language- games: innumerably many kinds of use of the components of language.24 The grammar of the language- game influences the possible relations of words, and things, within that game. But the players may modify the rules gradually. Some utterances within a given language- game are applications; others are 'grammatical remarks' or definitions of what is or should be possible. (Hence Wittgenstein's remark, 'Theology as grammar'25 – the grammar of religion.) The idea of the 'form of life' is a reminder about even more basic phenomena. It is clearly bound up with the idea of language. (Language and 'form of life' are explicitly connected in four of the five passages from the Investigations in which the term 'form of life' appears.) Just as grammar is subject to change through language-
uses, so 'form of life' is subject to change through changes in language. (The Copernican revolution is a paradigm case of this.) Nevertheless, 'form of life' expresses a deeper level of 'agreement.' It is the level of 'what has to be accepted, the given.'26 This is an agreement prior to agreement in opinions and decisions. Not everything can be doubted or judged at once. This suggests that 'form of life' does not denote static phenomena of fixed scope. Rather, it serves to remind us of the general need for context in our activity of meaning. But the context of our meaning is a constantly changing mosaic involving both broad strokes and fine-
grained distinctions. The more commonly understood point of the 'Private Language Argument' – concerning the root of meaning in something public – comes into play here. But it is important to show just what public phenomenon Wittgenstein has in mind. He remarks: 'Only in the stream of thought and life do words have meaning.'27
- 24
- Investigations, sec. 23.
- 25
- Investigations, sec. 373; compare Zettel, sec. 717.
- 26
- Investigations, p. 226e.
- 27
- Zettel, sec. 173. The thought is expressed many times in similar words.
And from an earlier chapter of Creegan:
The 'possibility of religion' manifested itself in considerable reading of religious works, and this in a person who chose his reading matter very carefully. Drury's recollections include conversations about Thomas à Kempis, Samuel Johnson's Prayers, Karl Barth, and, many times, the New Testament, which Wittgenstein had clearly read often and thought about.25 Wittgenstein had also thought about what it would mean to be a Christian. Some time during the 1930s, he remarked to Drury: 'There is a sense in which you and I are both Christians.'26 In this context it is certainly worth noting that he had for a time said the Lord's Prayer each day.27
Wittgenstein's last words were: 'Tell them I've had a wonderful life!'28
- 25
- Drury (1981) 'Conversations with Wittgenstein,' in Ludwig Wittgenstein: Personal Recollections, pp. 112ff.
- 26
- Drury, 'Conversations,' p. 130.
- 27
- Drury, 'Some notes,' p. 109.
- 28
- Reported by Mrs. Bevan, the wife of the doctor in whose house Wittgenstein was staying. Malcolm, Memoir, p. 81.
Part IV:
For more on the Christmas evening
number of the beast, see Dec. 3:
"Santa's Polar Opposite?" —
Comments Off on Wednesday December 26, 2007
Wednesday, September 19, 2007
Wednesday September 19, 2007
Einstein, God, and
the Consolation of Form
the Consolation of Form
“The kind of thing that would make Einstein gag”
“– …He did some equations that would make God cry for the sheer beauty of them. Take a look at this…. The sonofabitch set out equations that fit the data. Nobody believes they mean anything. Shit, when I back off, neither do I. But now and then, just once in a while…
— He joined physical and mental events. In a unified mathematical field.
— Yeah, that’s what I think he did. But the bastards in this department… bunch of goddamned positivists. Proof doesn’t mean a damned thing to them. Logical rigor, beauty, that damned perfection of something that works straight out, upside down, or sideways– they don’t give a damn.”
— “Nothing Succeeds,” in The Southern Reporter: Stories of John William Corrington, LSU Press, 1981
“The search for images of order and the loss of them constitute the meaning of The Southern Reporter.”
— Louisiana State University Press
“By equating reality with the metaphysical abstraction ‘contingency’ and explaining his paradigm by reference to simple images of order, Kermode [but see note below] defines the realist novel not as one which attempts to get to grips with society or human nature, but one which, in providing the consolation of form,* makes the occasional concession to contingency….”
“We are here in the
Church of St. Frank.“
Church of St. Frank.“
— Marjorie Garber,
Harvard University
* “The consolations of form” is a phrase Kermode quoted from Iris Murdoch. Webster does not mention Murdoch. Others have quoted Murdoch’s memorable phrase, which comes from her essay “Against Dryness: A Polemical Sketch,” Encounter, No. 88, January 1961, pp. 16-20. The essay was reprinted in a Penguin paperback collection of Murdoch’s work, Existentialists and Mystics. It was also reprinted in The Novel Today, ed. Malcolm Bradbury (Manchester, Manchester U. Press, 1977); in Revisions, ed. S. Hauerwas and A. MacIntyre (Notre Dame, U. of Notre Dame Press, 1981); and in Iris Murdoch, ed. H. Bloom (New York, Chelsea House, 1986).
Comments Off on Wednesday September 19, 2007
Monday, June 26, 2006
Monday June 26, 2006
A Little Extra Reading
In memory of
Mary Martin McLaughlin,
a scholar of Heloise and Abelard.
McLaughlin died on June 8, 2006.
"Following the parade, a speech is given by Charles Williams, based on his book The Place of the Lion. Williams explains the true meaning of the word 'realism' in both philosophy and theology. His guard of honor, bayonets gleaming, is led by William of Ockham."
A review by John D. Burlinson of Charles Williams's novel The Place of the Lion:
"… a little extra reading regarding Abelard's take on 'universals' might add a little extra spice– since Abelard is the subject of the heroine's … doctoral dissertation. I'd suggest the article 'The Medieval Problem of Universals' in the online Stanford Encyclopedia of Philosophy."
Michael L. Czapkay, a student of philosophical theology at Oxford:
"The development of logic in the schools and universities of western Europe between the eleventh and fifteenth centuries constituted a significant contribution to the history of philosophy. But no less significant was the influence of this development of logic on medieval theology. It provided the necessary conceptual apparatus for the systematization of theology. Abelard, Ockham, and Thomas Aquinas are paradigm cases of the extent to which logic played an active role in the systematic formulation of Christian theology. In fact, at certain points, for instance in modal logic, logical concepts were intimately related to theological problems, such as God's knowledge of future contingent truths."
The Medieval Problem of Universals, by Fordham's Gyula Klima, 2004:
"… for Abelard, a status is an object of the divine mind, whereby God preconceives the state of his creation from eternity."
Status Symbol
(based on Weyl's Symmetry):
"… for then we would know
Comments Off on Monday June 26, 2006
Friday, March 3, 2006
Friday March 3, 2006
Found in Translation
From “Space, Time, and Scarlett”
(Log24, Feb. 9):
“Her hair is Harlow gold….”
For Scarlett on James Merrill’s birthday
(which he shares with Jean Harlow)–
the Log24 links of Palm Sunday, 2004:
Google’s “sunlit paradigm” and
my own “Lost in Translation.”
Comments Off on Friday March 3, 2006
Sunday, April 4, 2004
Sunday April 4, 2004
Comments Off on Sunday April 4, 2004
Monday, January 26, 2004
Monday January 26, 2004
Language Game
More on "selving," a word coined by the Jesuit poet Gerard Manley Hopkins. (See Saturday's Taking Lucifer Seriously.)
"… through the calibrated truths of temporal discipline such as timetabling, serialization, and the imposition of clock-time, the subject is accorded a moment to speak in."
Framing
Intelligibility, Identity, and Selfhood:
A Reconsideration of
Spatio-Temporal Models.
The "moment to speak in" of today's previous entry, 11:29 AM, is a reference to the date 11/29 of last year's entry
That entry contains, in turn, a reference to the journal Subaltern Studies. According to a review of Reading Subaltern Studies,
"… the Subaltern Studies collective drew upon the Althusser who questioned the primacy of the subject…."
Munt also has something to say on "the primacy of the subject" —
"Poststructuralism, following particularly Michel Foucault, Jacques Derrida and Jacques Lacan, has ensured that 'the subject' is a cardinal category of contemporary thought; in any number of disciplines, it is one of the first concepts we teach to our undergraduates. But are we best served by continuing to insist on the intellectual primacy of the 'subject,' formulated as it has been within the negative paradigm of subjectivity as subjection?"
How about objectivity as objection?
I, for one, object strongly to "the Althusser who questioned the primacy of the subject."
This Althusser, a French Marxist philosopher by whom the late Michael Sprinker (Taking Lucifer Seriously) was strongly influenced, murdered his wife in 1980 and died ten years later in a lunatic asylum.
For details, see
|
|
For details of Althusser's philosophy, see the oeuvre of Michael Sprinker. For another notable French tribute to Marxism, click on the picture at left. |
Comments Off on Monday January 26, 2004
Monday, December 8, 2003
Monday December 8, 2003
Dead Poets Society
On Friday, December 5, 2003, I picked up a copy of An Introduction to Poetry, by X. J. Kennedy and Dana Gioia, 8th ed., at a used book sale for 50 cents.
The previous entry concerns a poem by Buson I found in that book, and contains a link on Kennedy’s name to a work suitable for this holiday season.
As additional thanks for the poem, here are links to a two-part interview with Gioia:
“A poem need not shout to be heard.”
— Dana Gioia
Monday, August 4, 2003
Monday August 4, 2003
A Queer Religion
August 4 headline:
This suggests the following theological meditation by a gay Christian:
|
“I can’t resist but end by pointing out the irony of the doctrine of the Trinity as seen by gay eyes. Please don’t take what I say next too seriously. I don’t believe that gender is very important or that it is any more present in God than is ‘green-ness,’ however, I simply can’t resist. The Trinity seems to be founded on the ecstatic love union of two male persons; the Father and the Son. If one takes this seriously it is incestuous pedophilia. There is no doubt that this union is generative (and so in the origin of the meaning ‘sexual’) in character, because from it bursts forth a third person: Holy Spirit; neuter in Greek, feminine in Hebrew! Whereas Islam detests the Catholic idea that the Blessed Virgin was ‘impregnated’ by God, as demeaning to the transcendence of God, the internal incestuous homosexuality that the doctrine of the Trinity amounts to should really offend more! Any orthodox account of the inner life of God is at best highly uncongenial to the paradigm of the heterosexual nuclear family. Amusingly, the contemporary Magisterium fails to notice this and even attempts to use the doctrine of the procession of the Spirit from the Father and the Son to bolster its conventional championing of ‘male-female complementarity’ and the centrality of procreation to all authentically ‘self-giving’ relationships. Absurdities will never cease!” |
Amen to the conclusion, at least.
The author of this meditation, “Pharsea,” is a “traditional Catholic” and advocate of the Latin Mass — just like Mel Gibson. One wonders how Gibson might react to Pharsea’s theology.
As for me… I always thought there was something queer about that religion.
Comments Off on Monday August 4, 2003
Friday, August 1, 2003
Friday August 1, 2003
Jack of Diamonds
KHYI plays the Jack of Diamonds again (see yesterday’s entry, Killer Radio):
“I knew a man with money in his hand.
He’d look that Jack of Diamonds in the eye….”
For another version of the Jack, see The Cube Paradigm.
Comments Off on Friday August 1, 2003