Friday, November 3, 2023
Sunday, March 6, 2022
Overarching Symmetries
By the Daniel J. Peterson whose Swarthmore honors thesis was quoted
here last night —
"What, then, is the relationship between theory-relative symmetries
(physical symmetries) and theory-independent symmetries
(overarching symmetries)? My statement of this problem is
a bit abstract, so let’s look at an example: classical Newtonian gravity
and classical electromagnetism . . . ."
— Prospects for a New Account of Time Reversal
by Daniel J. Peterson, Ph.D. dissertation, U. Mich., 2013, p. 16.
Another 2013 approach to the word "overarching" and sytmmetries —
Other terms of interest: Tenet , Nolanism , and Magic for Liars .
Comments Off on Overarching Symmetries
Saturday, January 29, 2022
On the Diamond-Theorem Group* of Order 322,560
Taormina and Wendland have often discussed this group, which they
call "overarching" within the context of their Mathieu-moonshine research.
This seems to be the first time they have attempted to explore its geometric
background as an affine group, apart from its role as "the octad group" in the
researches of R. T. Curtis and John Conway on the large Mathieu group M24.
* See a Log24 post of June 1, 2013.
Comments Off on On the Diamond-Theorem Group* of Order 322,560
Friday, October 25, 2019
Facettenreiche Gestaltung
On the word Gestaltung —
(Here “eidolon” should instead be “eidos .”)
A search for a translation of the book "Facettenreiche Mathematik " —
A paper found in the above search —
A related translation —
See also octad.design.
Comments Off on Facettenreiche Gestaltung
Tuesday, April 24, 2018
Illustrators of the Word
Tom Wolfe in The Painted Word (1975) —
“I am willing (now that so much has been revealed!)
to predict that in the year 2000, when the Metropolitan
or the Museum of Modern Art puts on the great
retrospective exhibition of American Art 1945-75,
the three artists who will be featured, the three seminal
figures of the era, will be not Pollock, de Kooning, and
Johns-but Greenberg, Rosenberg, and Steinberg.
Up on the walls will be huge copy blocks, eight and a half
by eleven feet each, presenting the protean passages of
the period … a little ‘fuliginous flatness’ here … a little
‘action painting’ there … and some of that ‘all great art
is about art’ just beyond. Beside them will be small
reproductions of the work of leading illustrators of
the Word from that period….”
The above group of 322,560 permutations appears also in a 2011 book —
— and in 2013-2015 papers by Anne Taormina and Katrin Wendland:
Comments Off on Illustrators of the Word
Monday, September 12, 2016
The Kummer Lattice
The previous post quoted Tom Wolfe on Chomsky's use of
the word "array."
An example of particular interest is the 4×4 array
(whether of dots or of unit squares) —
.
Some context for the 4×4 array —
The following definition indicates that the 4×4 array, when
suitably coordinatized, underlies the Kummer lattice .
Further background on the Kummer lattice:
Alice Garbagnati and Alessandra Sarti,
"Kummer Surfaces and K3 surfaces
with $(Z/2Z)^4$ symplectic action."
To appear in Rocky Mountain J. Math. —
The above article is written from the viewpoint of traditional
algebraic geometry. For a less traditional view of the underlying
affine 4-space from finite geometry, see the website
Finite Geometry of the Square and Cube.
Some further context …
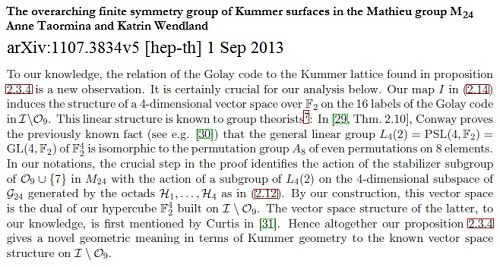
"To our knowledge, the relation of the Golay code
to the Kummer lattice … is a new observation."
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of
Kummer surfaces in the Mathieu group M24 "
As noted earlier, Taormina and Wendland seem not to be aware of
R. W. H. T. Hudson's use of the (uncoordinatized*) 4×4 array in his
1905 book Kummer's Quartic Surface. The array was coordinatized,
i.e. given a "vector space structure," by Cullinane eight years prior to
the cited remarks of Curtis.
* Update of Sept. 14: "Uncoordinatized," but parametrized by 0 and
the 15 two-subsets of a six-set. See the post of Sept. 13.
Comments Off on The Kummer Lattice
Tuesday, May 24, 2016
Rosenhain and Göpel Revisited
The authors Taormina and Wendland in the previous post
discussed some mathematics they apparently did not know was
related to a classic 1905 book by R. W. H. T. Hudson, Kummer's
Quartic Surface .
"This famous book is a prototype for the possibility
of explaining and exploring a many-faceted topic of
research, without focussing on general definitions,
formal techniques, or even fancy machinery. In this
regard, the book still stands as a highly recommendable,
unparalleled introduction to Kummer surfaces, as a
permanent source of inspiration and, last but not least,
as an everlasting symbol of mathematical culture."
— Werner Kleinert, Mathematical Reviews ,
as quoted at Amazon.com
Some 4×4 diagrams from that book are highly relevant to the
discussion by Taormina and Wendland of the 4×4 squares within
the 1974 Miracle Octad Generator of R. T. Curtis that were later,
in 1987, described by Curtis as pictures of the vector 4-space over
the two-element Galois field GF(2).
Hudson did not think of his 4×4 diagrams as illustrating a vector space,
but he did use them to picture certain subsets of the 16 cells in each
diagram that he called Rosenhain and Göpel tetrads .
Some related work of my own (click images for related posts)—
Rosenhain tetrads as 20 of the 35 projective lines in PG(3,2)
Göpel tetrads as 15 of the 35 projective lines in PG(3,2)
Related terminology describing the Göpel tetrads above
Comments Off on Rosenhain and Göpel Revisited
Monday, May 23, 2016
Springer
In memory of the late mathematician John Nash
and of the late actor Alan Young ...
A Talking Horse —
What the horse says: "First online: 28 August 2013."
See also Overarching, Psychonauts, and Spider Tale in this journal.
Comments Off on Springer
Friday, December 4, 2015
Scholium
"Encouraged by Proposition 5, one may hope…."
— Katrin Wendland in the previous post
Related material: Euclid Book I, Proposition 5.
Comments Off on Scholium
Thursday, December 3, 2015
Overarching Symmetry
From p. 34 of the preprint "Snapshots of Conformal Field Theory,"
by Katrin Wendland, arXiv, 11 April 2014 —
50. Gannon, T.: Much ado about Mathieu (arXiv:1211.5531 [math.RT])
85. Taormina, A., Wendland, K.: The overarching finite symmetry group
of Kummer surfaces in the Mathieu group M24. JHEP 08, 125 (2013)
86. Taormina, A., Wendland, K.: Symmetry-surfing the moduli space
of Kummer K3s (arXiv:1303.2931 [hep-th])
87. Taormina, A., Wendland, K.: A twist in the M24 moonshine story
(arXiv:1303.3221 [hep-th])
The Wendland paper was published on Jan. 7, 2015, in
Mathematical Aspects of Quantum Field Theories ,
edited by Damien Calaque and Thomas Strobl
(Springer Mathematical Physics Studies), pages 89-129.
Comments Off on Overarching Symmetry
Monday, May 25, 2015
A Stitch in Time
The most recent version of a passage
quoted in posts tagged "May 19 Gestalt" —
"You've got to pick up every stitch." — Donovan
Comments Off on A Stitch in Time
Sunday, May 17, 2015
Saturday, September 21, 2013
Mathematics and Narrative (continued)
Mathematics:
A review of posts from earlier this month —
Wednesday, September 4, 2013
|
Narrative:
Aooo.
Happy birthday to Stephen King.
Comments Off on Mathematics and Narrative (continued)
Wednesday, September 4, 2013
Monday, June 10, 2013
Galois Coordinates
Today's previous post on coordinate systems
suggests a look at the phrase "Galois coordinates."
A search shows that the phrase, though natural,
has apparently not been used before 2011* for solutions
to what Hermann Weyl called "the relativity problem."
A thorough historical essay on Galois coordinatization
in this sense would require more academic resources
than I have available. It would likely describe a number
of applications of Galois-field coordinates to square
(and perhaps to cubical) arrays that were studied before
1976, the date of my Diamond Theory monograph.
But such a survey might not find any such pre-1976
coordinatization of a 4×4 array by the 16 elements
of the vector 4-space over the Galois field with two
elements, GF(2).
Such coordinatizations are important because of their
close relationship to the Mathieu group M 24 .
See a preprint by Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ," with its remark
denying knowledge of any such coordinatization
prior to a 1989 paper by R. T. Curtis.
Related material:
Some images related to Galois coordinates, excerpted
from a Google search today (click to enlarge)—
* A rather abstract 2011 paper that uses the phrase
"Galois coordinates" may have some implications
for the naive form of the relativity problem
related to square and cubical arrays.
Comments Off on Galois Coordinates
Sunday, May 19, 2013
Priority Claim
From an arXiv preprint submitted July 18, 2011,
and last revised on March 11, 2013 (version 4):
"By our construction, this vector space is the dual
of our hypercube F24 built on I \ O9. The vector space
structure of the latter, to our knowledge, is first
mentioned by Curtis in [Cur89]. Hence altogether
our proposition 2.3.4 gives a novel geometric
meaning in terms of Kummer geometry to the known
vector space structure on I \ O9."
[Cur89] reference:
R. T. Curtis, "Further elementary techniques using
the miracle octad generator," Proc. Edinburgh
Math. Soc. 32 (1989), 345-353 (received on
July 20, 1987).
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ,"
arXiv.org > hep-th > arXiv:1107.3834
"First mentioned by Curtis…."
No. I claim that to the best of my knowledge, the
vector space structure was first mentioned by me,
Steven H. Cullinane, in an AMS abstract submitted
in October 1978, some nine years before the
Curtis article.
|
Update of the above paragraph on July 6, 2013—
No. The vector space structure was described by
The vector space structure as it occurs in a 4×4 array |
See Notes on Finite Geometry for some background.
See in particular The Galois Tesseract.
For the relationship of the 1978 abstract to Kummer
geometry, see Rosenhain and Göpel Tetrads in PG(3,2).
Comments Off on Priority Claim
Saturday, May 11, 2013
Core
Promotional description of a new book:
"Like Gödel, Escher, Bach before it, Surfaces and Essences will profoundly enrich our understanding of our own minds. By plunging the reader into an extraordinary variety of colorful situations involving language, thought, and memory, by revealing bit by bit the constantly churning cognitive mechanisms normally completely hidden from view, and by discovering in them one central, invariant core— the incessant, unconscious quest for strong analogical links to past experiences— this book puts forth a radical and deeply surprising new vision of the act of thinking."
"Like Gödel, Escher, Bach before it…."
Or like Metamagical Themas .
Rubik core:
Non- Rubik cores:
|
Of the odd nxnxn cube:
|
Of the even nxnxn cube:
|
Related material: The Eightfold Cube and…
"A core component in the construction
is a 3-dimensional vector space V over F2 ."
— Page 29 of "A twist in the M24 moonshine story,"
by Anne Taormina and Katrin Wendland.
(Submitted to the arXiv on 13 Mar 2013.)
Comments Off on Core
Sunday, April 28, 2013
C’mon Baby…
 |
Let's do the twist. The image at left See this journal |
A phrase from yesterday's noon post:
Sinking the Magic 8-Ball .
A scene from the above film is related to this phrase.
Another image from the film poster:
A review of the film:
"The final 'twist' seems to negate the entire story,
like a bad shaggy-dog joke."
Such a joke:
“Words and numbers are of equal value,
for, in the cloak of knowledge,
one is warp and the other woof.”
— The princesses Rhyme and Reason
in The Phantom Tollbooth
"A core component in the construction
is a 3-dimensional vector space V over F2 ."
— Page 29 of "A twist in the M24 moonshine story,"
by Anne Taormina and Katrin Wendland.
(Submitted to the arXiv on 13 Mar 2013.)
The number of points in such a space is, of course, 8.
Comments Off on C’mon Baby…
Friday, April 26, 2013
The Cruelest Month continues…
"Well in North Carolina…" — George Jones
For those averse to white lightning —
A link in yesterday 's 5:24 PM post yields moonshine.
See also Title and 24 Hour Psycho.
Comments Off on The Cruelest Month continues…
Sunday, March 17, 2013
Back to the Present
The previous post discussed some tesseract–
related mathematics from 1905.
Returning to the present, here is some arXiv activity
in the same area from March 11, 12, and 13, 2013.
Comments Off on Back to the Present