Nonsense…
See Gary Zukav, Harvard ’64, in this journal.
and damned nonsense —
“Every institution has a soul.”
— Gerald Holton in Harvard Gazette today
Commentary —
“The Ferris wheel came into view again….”
— Malcom Lowry, Under the Volcano
See also Holton in a Jan. 1977 interview:
“If people have souls, and I think a few have, it shows….”
For Poetry Month
From the home page of Alexandre Borovik:
Book in progress: Shadows of the Truth
This book (to be published soon) can be viewed
as a sequel to Mathematics under the Microscope ,
but with focus shifted on mathematics as it was
experienced by children (well, by children who
became mathematicians). The cover is designed
by Edmund Harriss.
See also Harriss's weblog post of Dec. 27, 2008, on the death
of Harold Pinter: "The Search for the Truth Can Never Stop."
This suggests a review of my own post of Dec. 3, 2012,
"The Revisiting." A figure from that post:
(A sequel to yesterday's ART WARS and this
morning's De Colores )
“Perhaps the philosophically most relevant feature
of modern science is the emergence of abstract
symbolic structures as the hard core of objectivity
behind– as Eddington puts it– the colorful tale
of the subjective storyteller mind.” — Hermann Weyl
(Philosophy of Mathematics and Natural Science ,
Princeton, 1949, p. 237)
See also Deathly Hallows.
G. H. Hardy in A Mathematician's Apology —
|
What ‘purely aesthetic’ qualities can we distinguish in such theorems as Euclid’s or Pythagoras’s? I will not risk more than a few disjointed remarks. In both theorems (and in the theorems, of course, I include the proofs) there is a very high degree of unexpectedness, combined with inevitability and economy. The arguments take so odd and surprising a form; the weapons used seem so childishly simple when compared with the far-reaching results; but there is no escape from the conclusions. There are no complications of detail—one line of attack is enough in each case; and this is true too of the proofs of many much more difficult theorems, the full appreciation of which demands quite a high degree of technical proficiency. We do not want many ‘variations’ in the proof of a mathematical theorem: ‘enumeration of cases’, indeed, is one of the duller forms of mathematical argument. A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way. |
Related material:
Kyle Smith on April 15 in the New York Post —
"The ludicrous action thriller 'Beyond the Reach'
fails to achieve the Southwestern noir potency
of 'No Country for Old Men,' but there’s no denying
it brings to mind another Southwestern classic
about malicious pursuit: the Road Runner cartoons."
Related material:
|
Welcome to ACME lab!
Yes, the name is both confusing and has
We call it ACME Creativity Machine Environment – We like recursive ideas. |
"Celebrate National Library Week 2015 (April 12-18, 2015)
with the theme "Unlimited possibilities @ your library®."
See also Library of Hell.
A page from Princeton University Press on March 18, 2012:
… "mathematics and narrative…." (top of page xvii).
I prefer the interplay of Euclidean and Galois mathematics.
The Dreaming Jewels continued
"… the icosahedron and dodecahedron have the same properties
of symmetry. For the centres of the twenty faces of an icosahedron
may be joined to form a regular dodecahedron, and conversely, the
twelve vertices of an icosahedron can be placed at the centres
of the faces of a suitable dodecahedron. Thus the icosahedral and
dodecahedral groups are identical , and either solid may be used to
examine the nature of the group elements."
— Walter Ledermann, Introduction to the Theory
of Finite Groups (Oliver and Boyd, 1949, p. 93)
Salvador Dali, The Sacrament of the Last Supper
Omar Sharif and Gregory Peck in Behold a Pale Horse
Above: soccer-ball geometry.
See also …
See as well
"In Sunlight and in Shadow."
"But what was supposed to be the source of a compound's
authority? Why, the same as that of all new religious movements:
direct access to the godhead, which in this case was Creativity."
— Tom Wolfe, From Bauhaus to Our House
"Creativity is not a matter of magical inspiration."
— Burger and Starbird, The 5 Elements of Effective Thinking (2012)
Video published on Oct 19, 2012
"In this fifth of five videos, mathematics professor
Michael Starbird talks about the fifth element
in his new book, The 5 Elements of Effective Thinking ,
co-authored with Williams College professor
Edward B. Burger."
For more on the Starbird manifesto, see Princeton University Press.
An excerpt —
See also a post for Abel's Birthday, 2011 —
Midnight in Oslo — and a four-elements image from
the Jan. 26, 2010, post Symbology —
See also Midnight Purple
and today's previous post.
"Yankee Doodle went to London" — Song lyric
“Geometry was very important to us in this movie.”
— The Missing ART (Log24, November 7th, 2014)
ART —
"Faculty Approve Theater Concentration, Affirmation
of Integrity" — Recent Harvard Crimson headline
Holy Saturday is, according to tradition, the day of
the harrowing of Hell.
Notes:
The above passage on "Die Figuren der vier Modi
im Magischen Quadrat " should be read in the context of
a Log24 post from last year's Devil's Night (the night of
October 30-31). The post, "Structure," indicates that, using
the transformations of the diamond theorem, the notorious
"magic" square of Albrecht Dürer may be transformed
into normal reading order. That order is only one of
322,560 natural reading orders for any 4×4 array of
symbols. The above four "modi" describe another.
Continued from yesterday, the date of death for German
billionaire philanthropist Klaus Tschira —
For Tschira in this journal, see Stiftung .
For some Würfel illustrations, see this morning's post
Manifest O. A related webpage —
Two mathematicians, Barry Mazur and Edward Frenkel,
have, for rhetorical effect, badly misrepresented the
history of some basic fields of mathematics. Mazur and
Frenkel like to emphasize the importance of new
research by claiming that it connects fields that previously
had no known connection— when, in fact, the fields were
known to be connected since at least the nineteenth century.
For Mazur, see The Proof and the Lie; for Frenkel, see posts
tagged Frenkel-Metaphors.
See also a story and video on Robert Langlands from the
Toronto Star on March 27, 2015:
"His conjectures are called functoriality and
reciprocity. They made it possible to link up
three branches of math: harmonic analysis,
number theory, and geometry.
To mathematicians, this is mind-blowing stuff
because these branches have nothing to do
with each other."
For a much earlier link between these three fields, see the essay
"Why Pi Matters" published in The New Yorker last month.
The title was suggested by
http://benmarcus.com/smallwork/manifesto/.
The "O" of the title stands for the octahedral group.
See the following, from http://finitegeometry.org/sc/map.html —
|
|
An invariance of symmetry The diamond theorem on a 4x4x4 cube, and a sketch of the proof. |
| 83-10-01 | Portrait of O A table of the octahedral group O using the 24 patterns from the 2×2 case of the diamond theorem. |
| 83-10-16 | Study of O A different way of looking at the octahedral group, using cubes that illustrate the 2x2x2 case of the diamond theorem. |
| 84-09-15 | Diamonds and whirls Block designs of a different sort — graphic figures on cubes. See also the University of Exeter page on the octahedral group O. |
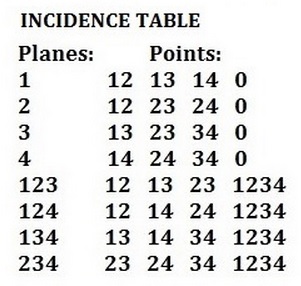
The incidences of points and planes in the
Möbius 84 configuration (8 points and 8 planes,
with 4 points on each plane and 4 planes on each point),
were described by Coxeter in a 1950 paper.*
A table from Monday's post summarizes Coxeter's
remarks, which described the incidences in
spatial terms, with the points and planes as the vertices
and face-planes of two mutually inscribed tetrahedra —
Monday's post, "Gallucci's Möbius Configuration,"
may not be completely intelligible unless one notices
that Coxeter has drawn some of the intersections in his
Fig. 24, a schematic representation of the point-plane
incidences, as dotless, and some as hollow dots. The figure,
"Gallucci's version of Möbius's 84," is shown below.
The hollow dots, representing the 8 points (as opposed
to the 8 planes ) of the configuration, are highlighted in blue.
Here a plane (represented by a dotless intersection) contains
the four points that are represented in the square array as lying
in the same row or same column as the plane.
The above Möbius incidences appear also much earlier in
Coxeter's paper, in figures 6 and 5, where they are shown
as describing the structure of a hypercube.
In figures 6 and 5, the dotless intersections representing
planes have been replaced by solid dots. The hollow dots
have again been highlighted in blue.
Figures 6 and 5 demonstrate the fact that adjacency in the set of
16 vertices of a hypercube is isomorphic to adjacency in the set
of 16 subsquares of a square 4×4 array, provided that opposite
sides of the array are identified, as in Fig. 6. The digits in
Coxeter's labels above may be viewed as naming the positions
of the 1's in (0,1) vectors (x4, x3, x2, x1) over the two-element
Galois field.† In that context, the 4×4 array may be called, instead
of a Möbius hypercube , a Galois tesseract .
* "Self-Dual Configurations and Regular Graphs,"
Bulletin of the American Mathematical Society,
Vol. 56 (1950), pp. 413-455
† The subscripts' usual 1-2-3-4 order is reversed as a reminder
that such a vector may be viewed as labeling a binary number
from 0 through 15, or alternately as labeling a polynomial in
the 16-element Galois field GF(24). See the Log24 post
Vector Addition in a Finite Field (Jan. 5, 2013).
(Continued from July 16, 2014.)
Some background from Wikipedia:
"Friedrich Ernst Peter Hirzebruch ForMemRS[2]
(17 October 1927 – 27 May 2012)
was a German mathematician, working in the fields of topology,
complex manifolds and algebraic geometry, and a leading figure
in his generation. He has been described as 'the most important
mathematician in Germany of the postwar period.'
[3][4][5][6][7][8][9][10][11]"
A search for citations of the A. E. Brouwer paper in
the previous post yields a quotation from the preface
to the third ("2013") edition of Wolfgang Ebeling's
Lattices and Codes: A Course Partially Based
on Lectures by Friedrich Hirzebruch , a book
reportedly published on September 19, 2012 —
|
"Sadly, on May 27 this year, Friedrich Hirzebruch, Hannover, July 2012 Wolfgang Ebeling "
(Prof. Dr. Wolfgang Ebeling, Institute of Algebraic Geometry, |
Also sadly …
Yesterday's post suggests a review of the following —
|
Andries Brouwer, preprint, 1982:
"The Witt designs, Golay codes and Mathieu groups" Pages 8-9: Substructures of S(5, 8, 24) An octad is a block of S(5, 8, 24). Theorem 5.1
Let B0 be a fixed octad. The 30 octads disjoint from B0
the design of the points and affine hyperplanes in AG(4, 2), Proof…. … (iv) We have AG(4, 2).
(Proof: invoke your favorite characterization of AG(4, 2) An explicit construction of the vector space is also easy….) |
Related material: Posts tagged Priority.
From H. S. M. Coxeter's 1950 paper
"Self-Dual Configurations and Regular Graphs,"
a 4×4 array and a more perspicuous rearrangement—
(Click image to enlarge.)
The above rearrangement brings Coxeter's remarks into accord
with the webpage The Galois Tesseract.
Update of Thursday, March 26, 2015 —
For an explanation of Coxeter's Fig. 24, see Thursday's later
post titled "The Möbius Hypercube."
The following figure, intended to display as
a black diamond, was produced with
HTML and Unicode characters. Depending
on the technology used to view it, the figure
may contain gaps or overlaps.
◢◣
◥◤
Some variations:
◤◥
◣◢
◤◥
◢◣
◤◣
◢◥
◤◣
◥◢
Such combined Unicode characters —
◢ black lower right triangle,
◣ black lower left triangle,
᭘ black upper left triangle,
᭙ black upper right triangle
— might be used for a text-only version of the Diamond 16 Puzzle
that is more easily programmed than the current version.
The tricky part would be coding the letter-spacing and
line-height to avoid gaps or overlaps within the figures in
a variety of browsers. The w3.org visual formatting model
may or may not be helpful here.
Update of 11:20 PM ET March 15, 2015 —
Seekers of simplicity should note that there is
a simple program in the Processing.js language, not using
such Unicode characters, that shows many random affine
permutations of a 4×4 diamond-theorem array when the
display window is clicked.
Continued from All Hallows' Eve, 2014.
Last year's Halloween post displayed the
Dürer print Knight, Death, and the Devil
(illustrated below on the cover of the book
Film and Phenomenology by Allan Casebier).
Cover illustration: Knight, Death, and the Devil,
by Albrecht Dürer
Some mathematics related to a different Dürer print —
"The Brit Awards are… the British equivalent
of the American Grammy Awards." — Wikipedia
Detail of an image from yesterday's 5:30 PM ET post:
Related material:
From a review: "Imagine 'Raiders of the Lost Ark'
set in 20th-century London, and then imagine it
written by a man steeped not in Hollywood movies
but in Dante and the things of the spirit, and you
might begin to get a picture of Charles Williams's
novel Many Dimensions ."
See also Solomon's Seal (July 26, 2012).
The words: "symplectic polarity"—
The images:
The Natural Symplectic Polarity in PG(3,2)
Symmetry Invariance in a Diamond Ring
The Diamond-Theorem Correlation
Steven Pressfield on April 25, 2012:
What exactly is High Concept?
Let’s start with its opposite, low concept.
Low concept stories are personal,
idiosyncratic, ambiguous, often European.
“Well, it’s a sensitive fable about a Swedish
sardine fisherman whose wife and daughter
find themselves conflicted over … ”
ZZZZZZZZ.
Fans of Oslo artist Josefine Lyche know she has
valiantly struggled to find a high-concept approach
to the diamond theorem. Any such approach must,
unfortunately, reckon with the following low
(i.e., not easily summarized) concept —
The Diamond Theorem Correlation:
From left to right …
http://www.log24.com/log/pix14B/140824-Diamond-Theorem-Correlation-1202w.jpg
http://www.log24.com/log/pix14B/140731-Diamond-Theorem-Correlation-747w.jpg
http://www.log24.com/log/pix14B/140824-Picturing_the_Smallest-1986.gif
http://www.log24.com/log/pix14B/140806-ProjPoints.gif
For some backstory, see ProjPoints.gif and "Symplectic Polarity" in this journal.
Review:
Monday, November 7, 2011
|
See also the phrase "a dance results" in the original
source and in yesterday's Valentine Dance.
Continued from yesterday.
The passage on Claude Chevalley quoted here
yesterday in the post Dead Reckoning was, it turns out,
also quoted by Peter Galison in his essay "Structure of Crystal,
Bucket of Dust" in Circles Disturbed: The Interplay of
Mathematics and Narrative (Princeton University Press, 2012,
ed. by Apostolos Doxiadis and Barry Mazur).
Galison gives a reference to his source:
"From 'Claude Chevalley Described by His Daughter (1988),'
in Michèle Chouchan, Nicolas Bourbaki: Faits et légendes
(Paris: Éditions du Choix, 1995), 36–40, translated and cited
in Marjorie Senechal, 'The Continuing Silence of Bourbaki:
An Interview with Pierre Cartier, June 18, 1997,'
Mathematical Intelligencer 1 (1998): 22–28."
Galison's essay compares Chevalley with the physicist
John Archibald Wheeler. His final paragraph —
"Perhaps, then, it should not surprise us too much if,
as Wheeler approaches the beginning-end of all things,
there is a bucket of Borelian dust. Out of this filth,
through the proposition machine of quantum mechanics
comes pregeometry; pregeometry makes geometry;
geometry gives rise to matter and the physical laws
and constants of the universe. At once close to and far
from the crystalline story that Bourbaki invoked,
Wheeler’s genesis puts one in mind of Genesis 3:19:
'In the sweat of thy face shalt thou eat bread, till thou
return unto the ground; for out of it wast thou taken:
for dust thou art, and unto dust shalt thou return.'"
See also posts tagged Wheeler.
… industrial designer Kenji Ekuan —
The adjective "eightfold," intrinsic to Buddhist
thought, was hijacked by Gell-Mann and later
by the Mathematical Sciences Research Institute
(MSRI, pronounced "misery"). The adjective's
application to a 2x2x2 cube consisting of eight
subcubes, "the eightfold cube," is not intended to
have either Buddhist or Semitic overtones.
It is pure mathematics.
|
From "Origins of the Logical Theory of Probability: von Kries, Wittgenstein, Waismann," by Michael Heidelberger — "Von Kries calls a range of objective possibilities of a hypothesis or event (under given laws) its Spielraum (literally: play space), which can mean ‘room to move’, ‘leeway’, ‘latitude of choice’, ‘degree of freedom’ or ‘free play’ and ‘clearance’ – or even ‘scope’. John Maynard Keynes translated it as ‘field’, but the term ‘range’ has generally been adopted in English. Von Kries now holds that if numerical probability were to make any sense at all it must be through this concept of the Spielraum . Von Kries’s theory is therefore called a ‘Spielraum theory’ or ‘range theory of probability’." — International Studies in the Philosophy of Science , Volume 15, Issue 2, 2001, pp. 177-188 |
See also the tag Points Omega.
(Scroll down to January 11-12, 2015.)
Related material:
"Now, for example, in how far are
the six sides of a symmetric die
'equally possible' upon throwing?"
— From "The Natural-Range Conception
of Probability," by Dr. Jacob Rosenthal,
page 73 in Time, Chance, and
Reduction: Philosophical Aspects of
Statistical Mechanics , ed. by
Gerhard Ernst and Andreas Hüttemann,
Cambridge U. Press, 2010, pp. 71-90
Continued from June 17, 2013
(John Baez as a savior for atheists):
As an atheists-savior, I prefer Galois…
The geometry underlying a figure that John Baez
posted four days ago, "A Hypercube of Bits," is
Galois geometry —
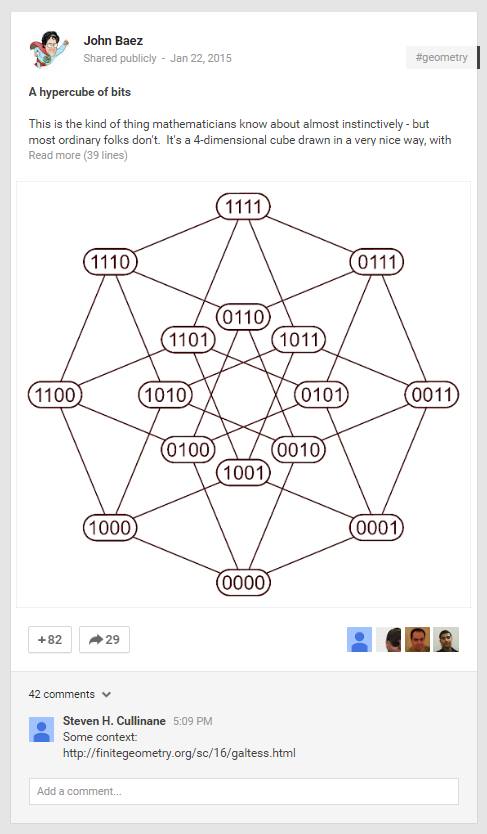
See The Galois Tesseract and an earlier
figure from Log24 on May 21, 2007:
For the genesis of the figure,
see The Geometry of Logic.
"Reality is the beginning not the end,
Naked Alpha, not the hierophant Omega,
of dense investiture, with luminous vassals."
— “An Ordinary Evening in New Haven” VI
From the series of posts tagged "Defining Form" —
The 4-point affine plane A and
the 7-point projective plane PA —
The circle-in-triangle of Yale's Figure 30b (PA ) may,
if one likes, be seen as having an occult meaning.
For the mathematical meaning of the circle in PA
see a search for "line at infinity."
A different, cubic, model of PA is perhaps more perspicuous.
Einstein and Thomas Mann, Princeton, 1938
A sequel to Princeton Requiem,
Gesamtkunstwerk , and Serial Box —
Fearful Symmetry, Princeton Style:
* See as well other instances of Kulturkampf in this journal.
Enotes.com on Herman Wouk's 1985 novel Inside, Outside :
"The 'outside' of the title is the goyish world
into which David’s profession has drawn him;
the 'inside' is the warm life of his Russian-
Jewish family on which he, as narrator, reflects
in the course of the novel."
For a different sort of 'inside' life, see this morning's post
Gesamtkunstwerk , and Nathan Shields's Feb. 8, 2011,
tribute to a serial composer "In Memoriam, Milton Babbitt."
Some other context for Shields's musical remarks —
Doctor Faustus and Dürer Square.
For a more interesting contrast of inside with outside
that has nothing to do with ethnicity, see the Feb. 10,
2014, post Mystery Box III: Inside, Outside, about
the following box:
.
The previous post displayed a set of
24 unit-square “points” within a rectangular array.
These are the points of the
Miracle Octad Generator of R. T. Curtis.
The array was labeled Ω
because that is the usual designation for
a set acted upon by a group:
* The title is an allusion to Point Omega , a novel by
Don DeLillo published on Groundhog Day 2010.
See “Point Omega” in this journal.
A professor at Harvard has written about
"the urge to seize and display something
real beyond artifice."
He reportedly died on January 3, 2015.
An image from this journal on that date:
Another Gitterkrieg image:
The 24-set Ω of R. T. Curtis
Click on the images for related material.
From the abstract of a talk, "Extremal Lattices," at TU Graz
on Friday, Jan. 11, 2013, by Prof. Dr. Gabriele Nebe
(RWTH Aachen) —
"I will give a construction of the extremal even
unimodular lattice Γ of dimension 72 I discovered
in summer 2010. The existence of such a lattice
was a longstanding open problem. The
construction that allows to obtain the
minimum by computer is similar to the one of the
Leech lattice from E8 and of the Golay code from
the Hamming code (Turyn 1967)."
On an earlier talk by Nebe at Oberwolfach in 2011 —
"Exciting new developments were presented by
Gabriele Nebe (Extremal lattices and codes ) who
sketched the construction of her recently found
extremal lattice in 72 dimensions…."
Nebe's Oberwolfach slides include one on
"The history of Turyn's construction" —
Nebe's list omits the year 1976. This was the year of
publication for "A New Combinatorial Approach to M24"
by R. T. Curtis, the paper that defined Curtis's
"Miracle Octad Generator."
Turyn's 1967 construction, uncredited by Curtis, may have
been the basis for Curtis's octad-generator construction.
See Turyn in this journal.
Wednesday, March 13, 2013
|
"I pondered deeply, then, over the
adventures of the jungle. And after
some work with a colored pencil
I succeeded in making my first drawing.
My Drawing Number One.
It looked something like this:
I showed my masterpiece to the
grown-ups, and asked them whether
the drawing frightened them.
But they answered: 'Why should
anyone be frightened by a hat?'"
* For the title, see Plato Thanks the Academy (Jan. 3).
From a NY Times obituary for an Arkansas poet,
Miller Williams, who reportedly died at 84
on New Year's Day —
The title of Lucinda Williams’s most recent album,
"Down Where the Spirit Meets the Bone,” is a
slightly altered line from one of her father’s poems,
which reads in its entirety:
Have compassion for everyone you meet,
even if they don’t want it. What seems conceit,
bad manners, or cynicism is always a sign
of things no ears have heard, no eyes have seen.
You do not know what wars are going on
down there where the spirit meets the bone.
Related material:
And from a sequel to
New Year's Greeting from Franz Kafka:
The above phrase "aimed at the heart of poetic language"
suggests an image from the poet's daughter's album —
The title refers to a set of fifteen Göpel tetrads
that form the lines of a Cremona-Richmond configuration .
"Spiel ist nicht Spielerei.
Es hat hohen Ernst
und tiefe Bedeutung."
— Friedrich W.A. Fröbel
(1782-1852)
An image that led off the year-end review yesterday in
the weblog of British combinatorialist Peter J. Cameron:
See also this weblog's post final post of 2014,
with a rectangular array illustrating the six faces
of a die, and Cameron's reference yesterday to
a die-related post…
"The things on my blog that seem to be
of continuing value are the expository
series like the one on the symmetric group
(the third post in this series was reblogged
by Gil Kalai last month, which gave it a new
lease of life)…."
A tale from an author of Prague:
| The Emperor—so they say—has sent a message, directly from his death bed, to you alone, his pathetic subject, a tiny shadow which has taken refuge at the furthest distance from the imperial sun. He ordered the herald to kneel down beside his bed and whispered the message into his ear. He thought it was so important that he had the herald repeat it back to him. He confirmed the accuracy of the verbal message by nodding his head. And in front of the entire crowd of those who’ve come to witness his death—all the obstructing walls have been broken down and all the great ones of his empire are standing in a circle on the broad and high soaring flights of stairs—in front of all of them he dispatched his herald. The messenger started off at once, a powerful, tireless man. Sticking one arm out and then another, he makes his way through the crowd. If he runs into resistance, he points to his breast where there is a sign of the sun. So he moves forward easily, unlike anyone else. But the crowd is so huge; its dwelling places are infinite. If there were an open field, how he would fly along, and soon you would hear the marvelous pounding of his fist on your door. But instead of that, how futile are all his efforts. He is still forcing his way through the private rooms of the innermost palace. He will never he win his way through. And if he did manage that, nothing would have been achieved. He would have to fight his way down the steps, and, if he managed to do that, nothing would have been achieved. He would have to stride through the courtyards, and after the courtyards the second palace encircling the first, and, then again, stairs and courtyards, and then, once again, a palace, and so on for thousands of years. And if he finally did burst through the outermost door—but that can never, never happen—the royal capital city, the centre of the world, is still there in front of him, piled high and full of sediment. No one pushes his way through here, certainly not with a message from a dead man. But you sit at your window and dream of that message when evening comes. |
See also a passage quoted in this weblog on the original
date of Cameron's Prague image, July 26, 2014 —
"The philosopher Graham Harman is invested in
re-thinking the autonomy of objects and is part
of a movement called Object-Oriented-Philosophy
(OOP)." — From “The Action of Things,” a 2011
M.A. thesis at the Center for Curatorial Studies,
Bard College, by Manuela Moscoso
— in the context of a search here for the phrase
"structure of the object." An image from that search:
Recent posts tagged Sagan Dodecahedron
mention an association between that Platonic
solid and the 5×5 grid. That grid, when extended
by the six points on a "line at infinity," yields
the 31 points of the finite projective plane of
order five.
For details of how the dodecahedron serves as
a model of this projective plane (PG(2,5)), see
Polster's A Geometrical Picture Book , p. 120:
For associations of the grid with magic rather than
with Plato, see a search for 5×5 in this journal.
The Blacklist “Pilot” Review
"There is an element of camp to this series though. Spader is
quite gleefully channeling Anthony Hopkins, complete with being
a well educated, elegant man locked away in a super-cell.
Speaking of that super-cell, it’s kind of ridiculous. They’ve got him
locked up in an abandoned post office warehouse on a little
platform with a chair inside a giant metal cube that looks like
it could have been built by Tony Stark. And as Liz approaches
to talk to him, the entire front of the cube opens and the whole
thing slides back to leave just the platform and chair. Really?
FUCKING REALLY ? "
— Kate Reilly at Geekenstein.com (Sept. 27, 2013)
The Ball-Weiner date above, 5 September 2011,
suggests a review of this journal on that date —
"Think of a DO NOT ENTER pictogram,
a circle with a diagonal slash, a type of ideogram.
It tells you what to do or not do, but not why.
The why is part of a larger context, a bigger picture."
— Customer review at Amazon.com
This passage was quoted here on August 10, 2009.
Also from that date:
The Sept. 5, 2011, Ball-Weiner paper illustrates the
"doily" view of the mathematical structure W(3,2), also
known as GQ(2,2), the Sp(4,2) generalized quadrangle.
(See Fig. 3.1 on page 33, exercise 13 on page 38, and
the answer to that exercise on page 55, illustrated by
Fig. 5.1 on page 56.)
For "another view, hidden yet true," of GQ(2,2),
see Inscape and Symplectic Polarity in this journal.
(Five by Five continued)
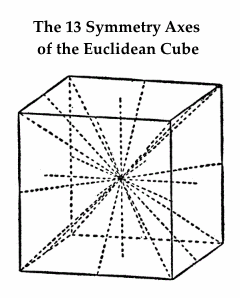
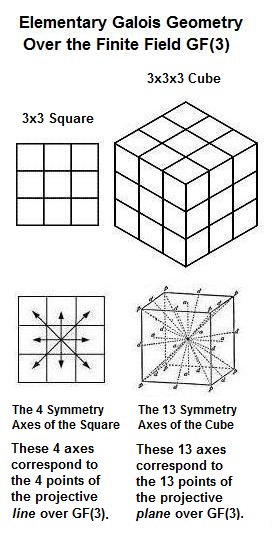
As the 3×3 grid underlies the order-3 finite projective plane,
whose 13 points may be modeled by
the 13 symmetry axes of the cube,
so the 5×5 grid underlies the order-5 finite projective plane,
whose 31 points may be modeled by
the 31 symmetry axes of the dodecahedron.
See posts tagged Galois-Plane Models.
"In digital circuit theory, combinational logic
(sometimes also referred to as time-independent logic)
is a type of digital logic which is implemented by
Boolean circuits, where the output is a pure function of
the present input only."
— Wikipedia, quoted in this morning's previous post as
commentary on Nabokov's phrase "combinational delight"
"Time past and time future
Allow but a little consciousness.
To be conscious is not to be in time
But only in time can the moment in the rose-garden,
The moment in the arbour where the rain beat,
The moment in the draughty church at smokefall
Be remembered; involved with past and future.
Only through time time is conquered."
— T. S. Eliot in Four Quartets
"I confess I do not believe in time."
— Vladimir Nabokov in Speak, Memory
From "Guardians of the Galaxy" —
"Then the Universe exploded into existence…"
For those who prefer a more traditional approach :
See also Symplectic Structure and Stevens's Rock.
C. P. Snow on G. H. Hardy in the foreword to
A Mathematician's Apology :
"… he had another favourite entertainment.
'Mark that man we met last night,'
he said, and someone had to be marked
out of 100 in each of the categories
Hardy had long since invented and defined.
STARK, BLEAK ('a stark man is not necessarily
bleak: but all bleak men without exception
want to be considered stark')…."
Related material :
Tommy Lee Jones in The New York Times on Nov. 6th, 2014,
and Pierce Brosnan in the 2014 film "The November Man" :
“Geometry was very important to us in this movie.”
— The Missing ART (Log24, November 7th, 2014)
(Continued… See "I need a photo opportunity…")
From the previous post's Yankee Puzzle link :
Saturday, January 7, 2012
|
"And I'll try to please you ev'ry day."
— Feste's song in Twelfth Night , as memorably sung by
Ben Kingsley, star of the new film "Stonehearst Asylum."
On the feast of Saint Nicholas
See also the six posts on this year's feast of Saint Andrew
and the following from the University of St. Andrews —
From Zettel (repunctuated for clarity):
249. « Nichts leichter, als sich einen 4-dimensionalen Würfel
vorstellen! Er schaut so aus… »
"Nothing easier than to imagine a 4-dimensional cube!
It looks like this…
[Here the editor supplied a picture of a 4-dimensional cube
that was omitted by Wittgenstein in the original.]
« Aber das meine ich nicht, ich meine etwas wie…
"But I don't mean that, I mean something like…
…nur mit 4 Ausdehnungen! »
but with four dimensions!
« Aber das ist nicht, was ich dir gezeigt habe,
eben etwas wie…
"But isn't what I showed you like…
…nur mit 4 Ausdehnungen? »
…only with four dimensions?"
« Nein; das meine ich nicht! »
"No, I don't mean that!"
« Was aber meine ich? Was ist mein Bild?
Nun der 4-dimensionale Würfel, wie du ihn gezeichnet hast,
ist es nicht ! Ich habe jetzt als Bild nur die Worte und
die Ablehnung alles dessen, was du mir zeigen kanst. »
"But what do I mean? What is my picture?
Well, it is not the four-dimensional cube
as you drew it. I have now for a picture only
the words and my rejection of anything
you can show me."
"Here's your damn Bild , Ludwig —"
Context: The Galois Tesseract.
Oslo artist Josefine Lyche has a new Instagram post,
this time on pyramids (the monumental kind).
My response —
Wikipedia's definition of a tetrahedron as a
"triangle-based pyramid" …
… and remarks from a Log24 post of August 14, 2013 :
|
Norway dance (as interpreted by an American)
I prefer a different, Norwegian, interpretation of "the dance of four."
Related material: |
See also some of Burkard Polster's triangle-based pyramids
and a 1983 triangle-based pyramid in a paper that Polster cites —
(Click image below to enlarge.)
Some other illustrations that are particularly relevant
for Lyche, an enthusiast of magic :
From On Art and Magic (May 5, 2011) —
|
|
(Updated at about 7 PM ET on Dec. 3.)
Continued from November 30, 2014
"Number right → Everything right." — Burkard Polster.
See also the six posts of November 30, St. Andrew's Day.
Related material —
Peter J. Cameron today discussing Julia Kristeva on poetry …
"This seems to be saying that the Kolmogorov
complexity of poetry is very low: the entire poem
can be generated from a small amount of information."
… and this journal on St. Andrew's day :
From "A Piece of the Storm,"
by the late poet Mark Strand —
A snowflake, a blizzard of one….
Flashback to St. Andrew's Day, 2013 —
Saturday, November 30, 2013
|
If the object is a cube, change arises from the fact
that the object has six faces…
and is the unit cell for the six -dimensional
hyperspace H over the two-element field —
A different representation of the unit cell of
the hyperspace H (and of the I Ching ) —
Or: Concepts of Space
1976 according to Cullinane:
1976 according to Plotnick:
“Irony and ridicule are entertaining and effective, and . . .
at the same time they are the agents of a great despair
and stasis in U.S. culture.” — David Foster Wallace,
as quoted by Adam Kirsch today at Salon
Reality's Mirror: Exploring the Mathematics of Symmetry —
"Here is a book that explains in laymen language
what symmetry is all about, from the lowliest snowflake
and flounder to the lofty group structures whose
astonishing applications to the Old One are winning
Nobel prizes. Bunch's book is a marvel of clear, witty
science writing, as delightful to read as it is informative
and up-to-date. The author is to be congratulated on
a job well done." — Martin Gardner
"But, sweet Satan, I beg of you, a less blazing eye!"
— Rimbaud, A Season in Hell
"… the lowliest snowflake and flounder…."
— Martin Gardner
From "A Piece of the Storm," by the late poet Mark Strand —
A snowflake, a blizzard of one….
From notes to Malcolm Lowry's "La Mordida" —
he had invested, in the Valley of the Shadow of Death….
See also Weyl's Symmetry in this journal.
The seven symmetry axes of the regular tetrahedron
are of two types: vertex-to-face and edge-to-edge.
Take these axes as the "points" of a Fano plane.
Each of the tetrahedron's six reflection planes contains
two vertex-to-face axes and one edge-to-edge axis.
Take these six planes as six of the "lines" of a Fano
plane. Then the seventh line is the set of three
edge-to-edge axes.
(The Fano tetrahedron is not original with me.
See Polster's 1998 A Geometrical Picture Book , pp. 16-17.)
There are three reflection planes parallel to faces
of the cube. Take the seven nonempty subsets of
the set of these three planes as the "points" of a
Fano plane. Define the Fano "lines" as those triples
of these seven subsets in which each member of
the triple is the symmetric-difference sum of the
other two members.
(This is the eightfold cube discussed at finitegeometry.org.)
For the late novelist P. D. James, author of, among other things,
The Children of Men .
Michael Caine in the film of that book —
Detail —
Raven's Progressive Matrices example —
A quote for tellers of tales —
“There have long been rumors of a mythical Ninth Element
that grants ultimate power to the Wizard who masters it.
The Order of Magick says there is no such thing. But….”
— Website of Magicka: The Ninth Element Novel
Mathematics: Galois-Plane Models.
Narrative: "The Dreaming Jewels."
Update of Nov. 30, 2014 —
It turns out that the following construction appears on
pages 16-17 of A Geometrical Picture Book , by
Burkard Polster (Springer, 1998).
"Experienced mathematicians know that often the hardest
part of researching a problem is understanding precisely
what that problem says. They often follow Polya's wise
advice: 'If you can't solve a problem, then there is an
easier problem you can't solve: find it.'"
—John H. Conway, foreword to the 2004 Princeton
Science Library edition of How to Solve It , by G. Polya
For a similar but more difficult problem involving the
31-point projective plane, see yesterday's post
"Euclidean-Galois Interplay."
The above new [see update above] Fano-plane model was
suggested by some 1998 remarks of the late Stephen Eberhart.
See this morning's followup to "Euclidean-Galois Interplay"
quoting Eberhart on the topic of how some of the smallest finite
projective planes relate to the symmetries of the five Platonic solids.
Update of Nov. 27, 2014: The seventh "line" of the tetrahedral
Fano model was redefined for greater symmetry.
Update of Nov. 30, 2014 —
For further information on the geometry in
the remarks by Eberhart below, see
pp. 16-17 of A Geometrical Picture Book ,
by Burkard Polster (Springer, 1998). Polster
cites a different article by Lemay.
A search for background to the exercise in the previous post
yields a passage from the late Stephen Eberhart:
|
The first three primes p = 2, 3, and 5 therefore yield finite projective planes with 7, 13, and 31 points and lines, respectively. But these are just the numbers of symmetry axes of the five regular solids, as described in Plato's Timaeus : The tetrahedron has 4 pairs of face planes and corner points + 3 pairs of opposite edges, totalling 7 axes; the cube has 3 pairs of faces + 6 pairs of edges + 4 pairs of corners, totalling 13 axes (the octahedron simply interchanges the roles of faces and corners); and the pentagon dodecahedron has 6 pairs of faces + 15 pairs of edges + 10 pairs of corners, totalling 31 axes (the icosahedron again interchanging roles of faces and corners). This is such a suggestive result, one would expect to find it dealt with in most texts on related subjects; instead, while "well known to those who well know such things" (as Richard Guy likes to quip), it is scarcely to be found in the formal literature [9]. The reason for the common numbers, it turns out, is that the groups of symmetry motions of the regular solids are subgroups of the groups of collineations of the respective finite planes, a face axis being different from an edge axis of a regular solid but all points of a projective plane being alike, so the latter has more symmetries than the former. [9] I am aware only of a series of in-house publications by Fernand Lemay of the Laboratoire de Didactique, Faculté des Sciences de I 'Éducation, Univ. Laval, Québec, in particular those collectively titled Genèse de la géométrie I-X.
— Stephen Eberhart, Dept. of Mathematics, |
Eberhart died of bone cancer in 2003. A memorial by his
high school class includes an Aug. 7, 2003, transcribed
letter from Eberhart to a classmate that ends…
|
… I earned MA’s in math (UW, Seattle) and history (UM, Missoula) where a math/history PhD program had been announced but canceled. So 1984 to 2002 I taught math (esp. non-Euclidean geometry) at C.S.U. Northridge. It’s been a rich life. I’m grateful. Steve |
See also another informative BRIDGES paper by Eberhart
on mathematics and the seven traditional liberal arts.
For previous remarks on this topic, as it relates to
symmetry axes of the cube, see previous posts tagged Interplay.
The above posts discuss, among other things, the Galois
projective plane of order 3, with 13 points and 13 lines.
These Galois points and lines may be modeled in Euclidean geometry
by the 13 symmetry axes and the 13 rotation planes
of the Euclidean cube. They may also be modeled in Galois geometry
by subsets of the 3x3x3 Galois cube (vector 3-space over GF(3)).
The 3×3×3 Galois Cube
Exercise: Is there any such analogy between the 31 points of the
order-5 Galois projective plane and the 31 symmetry axes of the
Euclidean dodecahedron and icosahedron? Also, how may the
31 projective points be naturally pictured as lines within the
5x5x5 Galois cube (vector 3-space over GF(5))?
Update of Nov. 30, 2014 —
For background to the above exercise, see
pp. 16-17 of A Geometrical Picture Book ,
by Burkard Polster (Springer, 1998), esp.
the citation to a 1983 article by Lemay.
Harold Rosenberg, "Art and Words,"
The New Yorker , March 29, 1969. From page 110:
"An advanced painting of this century inevitably gives rise
in the spectator to a conflict between his eye and his mind;
as Thomas Hess has pointed out, the fable of the emperor's
new clothes is echoed at the birth of every modemist art
movement. If work in a new mode is to be accepted, the
eye/mind conflict must be resolved in favor of the mind;
that is, of the language absorbed into the work. Of itself,
the eye is incapable of breaking into the intellectual system
that today distinguishes between objects that are art and
those that are not. Given its primitive function of
discriminating among things in shopping centers and on
highways, the eye will recognize a Noland as a fabric
design, a Judd as a stack of metal bins— until the eye's
outrageous philistinism has been subdued by the drone of
formulas concerning breakthroughs in color, space, and
even optical perception (this, too, unseen by the eye, of
course). It is scarcely an exaggeration to say that paintings
are today apprehended with the ears. Miss Barbara Rose,
once a promoter of striped canvases and aluminum boxes,
confesses that words are essential to the art she favored
when she writes, 'Although the logic of minimal art gained
critical respect, if not admiration, its reductiveness allowed
for a relatively limited art experience.' Recent art criticism
has reversed earlier procedures: instead of deriving principles
from what it sees, it teaches the eye to 'see' principles; the
writings of one of America's influential critics often pivot on
the drama of how he failed to respond to a painting or
sculpture the first few times he saw it but, returning to the
work, penetrated the concept that made it significant and
was then able to appreciate it. To qualify as a member of the
art public, an individual must be tuned to the appropriate
verbal reverberations of objects in art galleries, and his
receptive mechanism must be constantly adjusted to oscillate
to new vocabularies."
New vocabulary illustrated:
Graphic Design and a Symplectic Polarity —
Background: The diamond theorem
and a zero system .
“What happens when you mix the brilliant wit of Noel Coward
with the intricate plotting of Agatha Christie? Set during a
weekend in an English country manor in 1932, Death by Design
is a delightful and mysterious ‘mash-up’ of two of the greatest
English writers of all time. Edward Bennett, a playwright, and
his wife Sorel Bennett, an actress, flee London and head to
Cookham after a disastrous opening night. But various guests
arrive unexpectedly….”
— Samuel French (theatrical publisher) on a play that
opened in Houston on September 9, 2011.
Related material:
A paper from 1976 on symplectic torsors and finite geometry:
FINITE GEOMETRIES IN THE THEORY OF THETA CHARACTERISTICS
Autor(en): Rivano, Neantro Saavedra
Objekttyp: Article
Zeitschrift: L’Enseignement Mathématique
Band (Jahr): 22 (1976)
Heft 1-2: L’ENSEIGNEMENT MATHÉMATIQUE
PDF erstellt am: 14.11.2014
Persistenter Link: http://dx.doi.org/10.5169/seals-48185
(Received by the journal on February 20, 1976.)
Saavedra-Rivano was a student of Grothendieck, who reportedly died yesterday.
Yesterday's post and recent Hollywood news suggest
a meditation on a Progressive Matrix —
Click to enlarge.
"My card."
Structurally related images —
A sample Raven's Progressive Matrices test item
(such items share the 3×3 structure of the hash symbol above):
Structural background —
"We live entirely, especially if we are writers, by the imposition
of a narrative line upon disparate images…." — Joan Didion
Narrative Line:
Disparate images:
Exercise:
Can the above narrative line be imposed in any sensible way
upon the above disparate images?
“There exists a considerable literature
devoted to the Lo shu , much of it infected
with the kind of crypto-mystic twaddle
met with in Feng Shui.”
— Lee C. F. Sallows, Geometric Magic Squares ,
Dover Publications, 2013, page 121
Cf. Raiders of the Lost Theorem, Oct. 13, 2014.
See also tonight’s previous post and
“Feng Shui” in this journal.
Back together, but missing an article.
“An article (abbreviated ART) is a word (or prefix or suffix)
that is used with a noun to indicate the type of reference
being made by the noun.” — Wikipedia
Perhaps Tommy Lee Jones has it.
From a post of June 3, 2013:
New Yorker editor David Remnick at Princeton today
(from a copy of his prepared remarks):
“Finally, speaking of fabric design….”
I prefer Tom and Harold:
Tom Wolfe in The Painted Word —
“I am willing (now that so much has been revealed!)
to predict that in the year 2000, when the Metropolitan
or the Museum of Modern Art puts on the great
retrospective exhibition of American Art 1945-75,
the three artists who will be featured, the three seminal
figures of the era, will be not Pollock, de Kooning, and
Johns-but Greenberg, Rosenberg, and Steinberg.
Up on the walls will be huge copy blocks, eight and a half
by eleven feet each, presenting the protean passages of
the period … a little ‘fuliginous flatness’ here … a little
‘action painting’ there … and some of that ‘all great art
is about art’ just beyond. Beside them will be small
reproductions of the work of leading illustrators of
the Word from that period….”
Harold Rosenberg in The New Yorker (click to enlarge)—
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
— Steven H. Cullinane,
diamond theorem illustration
"Macy’s Herald Square occupies a singular place
in American retailing." — NY Times today, in print
on page BU1 of the New York edition with the headline:
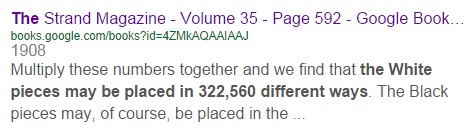
A Singular Time:
See Remember Me to Herald Square, at noon on
August 21, 2014, and related earlier Log24 posts.
Also on Aug. 21, 2014: from a blog post, 'Tiles,' by
Theo Wright, a British textile designer —
The 24 tile patterns displayed by Wright may be viewed
in their proper mathematical context at …
http://www.diamondspace.net/about.html:
Introducing a group of 322,560 affine transformations of Dürer’s ‘Magic’ Square
The four vector-space substructures of digits in 1st, 2nd, 3rd, 4th place,
together with the diamond theorem, indicate that Dürer’s square “minus one”
can be transformed by permutations of rows, columns, and quadrants to a
square with (decimal) digits in the usual numerical order, increasing from
top left to bottom right. Such permutations form a group of order 322,560.
(Continued from Vector Addition in a Finite Field, Twelfth Night, 2013.)
A print copy of next Sunday’s New York Times Book Review
arrived in today’s mail. From the front-page review:
Marcel Theroux on The Book of Strange New Things ,
a novel by Michel Faber —
“… taking a standard science fiction premise and
unfolding it with the patience and focus of a
tai chi master, until it reveals unexpected
connections, ironies and emotions.”
What is a tai chi master, and what is it that he unfolds?
Perhaps the taijitu symbol and related material will help.
The Origin of Change
“Two things of opposite natures seem to depend
On one another, as a man depends
On a woman, day on night, the imagined
On the real. This is the origin of change.
Winter and spring, cold copulars, embrace
And forth the particulars of rapture come.”
— Wallace Stevens,
“Notes Toward a Supreme Fiction,”
Canto IV of “It Must Change”
Part I:
Part II:
Click images for some context.
A post in honor of Évariste Galois (25 October 1811 – 31 May 1832)
From a book by Richard J. Trudeau titled The Non-Euclidean Revolution —
See also “non-Euclidean” in this journal.
One might argue that Galois geometry, a field ignored by Trudeau,
is also “non-Euclidean,” and (for those who like rhetoric) revolutionary.
The "Chern" of today's previous post is mathematician
Shiing-Shen Chern (b. Oct. 26, 1911, d. Dec. 3, 2004).
For an observance of the 2011 centennial of his birth,
see a website in China.
See also this journal on the centennial date —
Erlanger and Galois, a post of Oct. 26, 2011.
In the above illustration of the 3-4-5 Pythagorean triangle,
the grids on each side may be regarded as figures of
Euclidean geometry or of Galois geometry.
In Euclidean geometry, these grids illustrate a property of
the inner triangle.
In elementary Galois geometry, ignoring the connection with
the inner triangle, the grids may be regarded instead as
illustrating vector spaces over finite (i.e., Galois) fields.
Previous posts in this journal have dealt with properties of
the 3×3 and 4×4 grids. This suggests a look at properties of
the next larger grid, the 5×5 array, viewed as a picture of the
two-dimensional vector space (or affine plane) over the finite
Galois field GF(5) (also known as ℤ5).
The 5×5 array may be coordinatized in a natural way, as illustrated
in (for instance) Matters Mathematical , by I.N. Herstein and
Irving Kaplansky, 2nd ed., Chelsea Publishing, 1978, p. 171:
See Herstein and Kaplansky for the elementary Galois geometry of
the 5×5 array.
For 5×5 geometry that is not so elementary, see…
Hafner's abstract:
We describe the Hoffman-Singleton graph geometrically, showing that
it is closely related to the incidence graph of the affine plane over ℤ5.
This allows us to construct all automorphisms of the graph.
The remarks of Brouwer on graphs connect the 5×5-related geometry discussed
by Hafner with the 4×4 geometry related to the Steiner system S(5,8,24).
(See the Miracle Octad Generator of R. T. Curtis and the related coordinatization
by Cullinane of the 4×4 array as a four-dimensional vector space over GF(2).)
(Continued from Nov. 15, 2011)
Ben Bradlee, legendary Washington Post editor, dies at 93
See also a post of Jan. 20, 2011, and an earlier post on Twelfth Night, 2010.
A star figure and the Galois quaternion.
The square root of the former is the latter.
Two news items on art as a tool:
Two Log24 posts related to the 3×3 grid, the underlying structure for China’s
ancient Lo Shu “magic” square:
Finally, leftist art theorist Rosalind Krauss in this journal
on AntiChristmas, 2010:
Which is the tool here, the grid or Krauss?
(Night at the Museum continues.)
"Strategies for making or acquiring tools
While the creation of new tools marked the route to developing the social sciences,
the question remained: how best to acquire or produce those tools?"
— Jamie Cohen-Cole, “Instituting the Science of Mind: Intellectual Economies
and Disciplinary Exchange at Harvard’s Center for Cognitive Studies,”
British Journal for the History of Science vol. 40, no. 4 (2007): 567-597.
Obituary of a co-founder, in 1960, of the Center for Cognitive Studies at Harvard:
"Disciplinary Exchange" —
In exchange for the free Web tools of HTML and JavaScript,
some free tools for illustrating elementary Galois geometry —
The Kaleidoscope Puzzle, The Diamond 16 Puzzle,
The 2x2x2 Cube, and The 4x4x4 Cube
"Intellectual Economies" —
In exchange for a $10 per month subscription, an excellent
"Quilt Design Tool" —
This illustrates not geometry, but rather creative capitalism.
Related material from the date of the above Harvard death: Art Wars.
Barron's Educational Series (click to enlarge):
The Tablet of Ahkmenrah:
"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
Another educational series (this journal):
Art theorist Rosalind Krauss and The Ninefold Square
The title is from a Log24 post, "Diabolically Complex Riddle," of Sept. 27, 2014.
(See also a search for "Diabolic" in this journal, which yields an application to
"magic" squares.)
My own contribution to an event of the Mathematical Association of America:
Rick’s Tricky Six and The Judas Seat.
The Polster tetrahedral model of a finite geometry appears, notably,
in a Mathematics Magazine article from April 2009—
Parallelograms and the structure of the 3×3 array —
Click to enlarge:
A different approach to parallelograms and arrays —
Click for original post:
(Continued from Nov. 16, 2013.)
The 48 actions of GL(2,3) on a 3×3 array include the 8-element
quaternion group as a subgroup. This was illustrated in a Log24 post,
Hamilton’s Whirligig, of Jan. 5, 2006, and in a webpage whose
earliest version in the Internet Archive is from June 14, 2006.
One of these quaternion actions is pictured, without any reference
to quaternions, in a 2013 book by a Netherlands author whose
background in pure mathematics is apparently minimal:
In context (click to enlarge):
Update of later the same day —
Lee Sallows, Sept. 2011 foreword to Geometric Magic Squares —
“I first hit on the idea of a geometric magic square* in October 2001,**
and I sensed at once that I had penetrated some previously hidden portal
and was now standing on the threshold of a great adventure. It was going
to be like exploring Aladdin’s Cave. That there were treasures in the cave,
I was convinced, but how they were to be found was far from clear. The
concept of a geometric magic square is so simple that a child will grasp it
in a single glance. Ask a mathematician to create an actual specimen and
you may have a long wait before getting a response; such are the formidable
difficulties confronting the would-be constructor.”
* Defined by Sallows later in the book:
“Geometric or, less formally, geomagic is the term I use for
a magic square in which higher dimensional geometrical shapes
(or tiles or pieces ) may appear in the cells instead of numbers.”
** See some geometric matrices by Cullinane in a March 2001 webpage.
Earlier actual specimens — see Diamond Theory excerpts published in
February 1977 and a brief description of the original 1976 monograph:
“51 pp. on the symmetries & algebra of
matrices with geometric-figure entries.”
— Steven H. Cullinane, 1977 ad in
Notices of the American Mathematical Society
The recreational topic of “magic” squares is of little relevance
to my own interests— group actions on such matrices and the
matrices’ role as models of finite geometries.
(Continued from Sept. 3, 2009)
George Steiner on chess:
"At the sight of a set, even the tawdriest of plastic pocket sets,
one’s fingers arch and a coldness as in a light sleep steals over
one’s spine. Not for gain, not for knowledge or reknown, but
in some autistic enchantment, pure as one of Bach’s inverted
canons or Euler’s formula for polyhedra."
— George Steiner in “A Death of Kings,” The New Yorker,
issue dated September 7, 1968, page 133
A related remark from Dudeney:
See also a different context for 16 squares and 322,560 arrangements.
Or: Phantasmagoria Meets Pandemonium
Part I: Phantasmagoria
Rebecca Goldstein on first encountering Plato —
| “I was reading Durant’s section on Plato, struggling to understand his theory of the ideal Forms that lay in inviolable perfection out beyond the phantasmagoria. (That was the first, and I think the last, time that I encountered that word.)” |
| “We tell ourselves stories in order to live….We interpret what we see, select the most workable of multiple choices. We live entirely, especially if we are writers, by the imposition of a narrative line upon disparate images, by the ‘ideas’ with which we have learned to freeze the shifting phantasmagoria which is our actual experience.” |
Part II: Pandemonium
Terry Teachout in Commentary on Oct. 1, 2014:
“When making art or writing about it, the aesthete
tries never to moralize. Nor will he look with favor
upon artists who do so, no matter whether their
particular brand of moralizing is religious or secular.
But he can and must be fully, intensely alive to the
moral force of art whose creators aspire merely to
make the world around us more beautiful, and in
so doing to pierce the veil of the visible and give us
a glimpse of the permanently true. That is his job:
to help make sense of the pandemonium amid which
we live.”
Rivka Galchen in The New York Times Sunday Book Review
issue of October 5, 2014 (online Sept. 30):
“The story describes honestly something that is,
which is very different from proposing what ought to be.”
See also Pandemonium in this journal.
(Continued from Beautiful Mathematics, Dec. 14, 2013)
“Seemingly unrelated structures turn out to have
mysterious correspondences.” — Jim Holt, opening
paragraph of a book review in the Dec. 5, 2013, issue
of The New York Review of Books
One such correspondence:
For bibliographic information and further details, see
the March 9, 2014, update to “Beautiful Mathematics.”
See as well posts from that same March 9 now tagged “Story Creep.”
The following post suggests the Spiders and Snakes of Fritz Leiber’s
Changewar , a mythology inspired by the hallucinations of delirium tremens .
Loren Olson, Harvard ’64, a professor of mathematics
at Norway’s Tromsø University,* died June 22, 2014.
In his memory, a search in this journal for Lie Group.
That search yields a post titled Lie Groups for Holy Week (March 30, 2010).
A quotation related to that post:
* The city of Tromsø hosted some art related to group theory in 2010.
Neither that art nor my own related remarks on group theory are very
relevant to physics (yet).
This morning’s previous post quoted a sort of
invitation to damnation
from Princeton University Press:
Some related material in this journal: “Peter J. Cameron” + Magic.
"Welcome to America." — Harrison Ford in "The Devil's Own"
On readings at Mass on Sunday, Sept. 21, 2014 —
"Isaiah 55:8-9: 'For my thoughts are not your thoughts,
neither are your ways my ways, says the Lord.
For as the heavens are higher than the earth,
so are my ways higher than your ways
and my thoughts than your thoughts.'
The Gospel reading… was a perfect complement to
the passage from Isaiah…."
The America piece quoting Isaiah was titled "The Mystery of God."
The author "currently works at Xavier College Preparatory
in Palm Desert, CA, where he teaches theology…."
Related material: This journal that Sunday morning:
See also "The Mystery of God, Part II" —
Other secular stand-ins for "the thing one doesn't know"—
The mysteries of the late Joseph D. McNamara.
“Why Is Our Sci-Fi So Glum About A.I.?,”
by Jayson Greene, NY Times Sunday Magazine today —
“You come to pity these advanced beings, bumping against
the dunderheaded constraints that their less-advanced
creators have placed on them. Johansson’s Lucy grows so
powerful as her cerebral capacity multiplies that she is able
to manipulate her cellular structure. And yet, when pursued
by an entire planet’s worth of law enforcement, she settles
on a disguise straight out of Saturday-morning cartoons —
really big sunglasses and a hairdo change.”
See also this journal on Saturday morning for a definition, and
Geometry of the I Ching for examples, of …
changeable, instantiable entities, i.e., concrete universals.
The previous post discussed the anatomy of the sum 9 + 6.
A different approach: “A” and “The 6 spreads in A” below —
This post was suggested by Greg Gutfeld’s Sept. 4 remarks on Common Core math.
Problem: What is 9 + 6 ?
Here are two approaches suggested by illustrations of Desargues’s theorem.
Solution 1:
9 + 6 = 10 + 5,
as in Common Core (or, more simply, as in common sense), and
10 + 5 = 5 + 10 = 15 as in Veblen and Young:
Solution 2:
In the figure below,
9 + 6 = no. of V’s + no. of A’s + no. of C’s =
no. of nonempty squares = 16 – 1 = 15.
(Illustration from Feb. 10, 2014.)
The silly educationists’ “partner, anchor, decompose” jargon
discussed by Gutfeld was their attempt to explain “9 + 6 = 10 + 5.”
As he said of the jargon, “That’s not math, that’s the plot from ‘Silence of the Lambs.'”
Or from Richard, Frank, and Marcus in last night’s “Intruders”
(BBC America, 10 PM).
The fictional zero theorem of Terry Gilliam's current film
by that name should not be confused with the zero system
underlying the diamond theorem.
Tom Hanks as Indiana Langdon in Raiders of the Lost Articulation :
An unarticulated (but colored) cube:
A 2x2x2 articulated cube:
A 4x4x4 articulated cube built from subcubes like
the one viewed by Tom Hanks above:
An image related to the recent posts Sense and Sensibility:
A quote from yesterday's post The Eight:
A possible source for the above phrase about phenomena "carved at their joints":
See also the carving at the joints of Plato's diamond from the Meno :
Related material: Phaedrus on Kant as a diamond cutter
in Zen and the Art of Motorcycle Maintenance .
This post was suggested by the two previous posts, Sermon and Structure.
Vide below the final paragraph— in Chapter 7— of Cameron’s Parallelisms ,
as well as Baudelaire in the post Correspondences :
Comme de longs échos qui de loin se confondent
Dans une ténébreuse et profonde unité….
— Baudelaire, “Correspondances “
A related image search (click to enlarge):
Epigraphs from Parallelisms of Complete Designs
by Peter J. Cameron (Cambridge University Press, 1976)
Introduction
Through the unknown, remembered gate
When the last of earth left to discover
Is that which was the beginning
(T. S. Eliot: Little Gidding)
I The existence theorem
Here the impossible union
Of spheres of existence is actual
(T. S. Eliot: The Dry Salvages)
II The parallelogram property
A condition of complete simplicity
(Costing not less than everything)
(T. S. Eliot: Little Gidding)
III Steiner points and Veblen points
You say I am repeating
Something I have said before. I shall say it again.
Shall I say it again?
(T. S. Eliot: East Coker)
IV Edge-colourings of complete graphs
And hollyhocks that aim too high
Red into grey and tumble down
(T. S. Eliot: East Coker)
V Biplanes and metric regularity
Two and two, necessarye conjunction,
Holding eche other by the hand or the arm
Whiche betokeneth concorde.
(T. S. Eliot: East Coker)
VI Automorphism groups
At the still point of the turning world. Neither flesh nor fleshless;
Neither from nor towards; at the still point, there the dance is,
But neither arrest nor movement.
(T. S. Eliot: Burnt Norton)
VII Resolutions and partition systems
… fiddle with pentagrams
Or barbituric acids, or dissect
The recurrent image into pre-conscious terrors .. .
(T. S. Eliot: The Dry Salvages)
Structured gray matter:
Graphic symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
This post is continued from a March 12, 2013, post titled
"Smoke and Mirrors" on art in Tromsø, Norway, and from
a June 22, 2014, post on the nineteenth-century
mathematicians Rosenhain and Göpel.
The latter day was the day of death for
mathematician Loren D. Olson, Harvard '64.
For some background on that June 22 post, see the tag
Rosenhain and Göpel in this journal.
Some background on Olson, who taught at the
University of Tromsø, from the American Mathematical
Society yesterday:
Olson died not long after attending the 50th reunion of the
Harvard Class of 1964.
For another connection between that class (also my own)
and Tromsø, see posts tagged "Elegantly Packaged."
This phrase was taken from today's (print)
New York Times review of a new play titled "Smoke."
The phrase refers here to the following "package" for
some mathematical objects that were named after
Rosenhain and Göpel — a 4×4 array —
For the way these objects were packaged within the array
in 1905 by British mathematician R. W. H. T. Hudson, see
a page at finitegometry.org/sc. For the connection to the art
in Tromsø mentioned above, see the diamond theorem.
“1 + 2 + 3 + … = –1/12.”
Robin Williams and the Stages of Math
i) shock & denial
ii) anger
iii) bargaining
iv) depression
v) acceptance
And then…
vi) checking
vii) Joan Rivers:
Here and at Catholics for Classical Education.
See also Tom Wolfe on manifestos —
— and part of an interesting Sept. 2, 2014, manifesto by
Common Core supporter Keith Devlin:
“Graduate students of mathematics are introduced to further
assumptions (about handling the infinite, and various other issues),
equally reasonable and useful, and in accord both with our everyday
intuitions (insofar as they are relevant) and with the rest of
mainstream mathematics. And on the basis of those assumptions,
you can prove that
1 + 2 + 3 + … = –1/12.
That’s right, the sum of all the natural numbers equals –1/12.
This result is so much in-your-face, that people whose mathematics
education stopped at the undergraduate level (if they got that far)
typically say it is wrong. It’s not. Just as with the 0.999… example,
where we had to construct a proper meaning for an infinite decimal
expansion before we could determine what its value is, so to we
have to define what that infinite sum means. ….”
For a correction to Devlin’s remarks, see a physics professor’s weblog post —
“From a strictly mathematical point of view,
the equation 1+2+3+4+ … = -1/12 is incorrect,
and involves confusing the Dirichlet series with
the zeta function.” — Greg Gbur, May 25, 2010
The Folding
Cynthia Zarin in The New Yorker , issue dated April 12, 2004—
“Time, for L’Engle, is accordion-pleated. She elaborated,
‘When you bring a sheet off the line, you can’t handle it
until it’s folded, and in a sense, I think, the universe can’t
exist until it’s folded — or it’s a story without a book.’”
The geometry of the 4×4 square array is that of the
3-dimensional projective Galois space PG(3,2).
This space occurs, notably, in the Miracle Octad Generator (MOG)
of R. T. Curtis (submitted to Math. Proc. Camb. Phil. Soc. on
15 June 1974). Curtis did not, however, describe its geometric
properties. For these, see the Cullinane diamond theorem.
Some history:
Curtis seems to have obtained the 4×4 space by permuting,
then “folding” 1×8 binary sequences into 4×2 binary arrays.
The original 1×8 sequences came from the method of Turyn
(1967) described by van Lint in his book Coding Theory
(Springer Lecture Notes in Mathematics, No. 201 , first edition
published in 1971). Two 4×2 arrays form each 4×4 square array
within the MOG. This construction did not suggest any discussion
of the geometric properties of the square arrays.
[Rewritten for clarity on Sept. 3, 2014.]
The titles of the previous three posts refer to
Hermann Weyl’s 1918 book Raum, Zeit, Materie
(Space, Time, Matter).
This suggests a look at a poetically parallel 1950 title —
The Lion, the Witch and the Wardrobe —
and at its underlying philosophy:
I am among “those who do not know that this great myth became Fact.”
I do, however, note that some other odd things have become fact.
Those who wish more on this topic may consult:
A possible answer to the 1923 question of Walter Gropius, “Was ist Raum?“—
See also yesterday’s Source of the Finite and the image search
on the Gropius question in last night’s post.
Yesterday's 11 AM post was a requiem for a brutalist architect.
Today's LA Times has a related obituary:
"Architectural historian Alan Hess, who has written several books on
Mid-Century Modern design, said Meyer didn't have a signature style,
'which is one reason he is not as well-known as some other architects
of the period. But whatever style he was working in, he brought a real
sense of quality to his buildings.'
A notable example is another bank building, at South Beverly Drive
and Pico Boulevard, with massive concrete columns, a hallmark of
the New Brutalism style. 'This is a really good example of it,' Hess said."
— David Colker, 5:43 PM LA time, Aug. 28, 2014
A related search, suggested by this morning's post Source of the Finite:
(Click to enlarge.)
"Die Unendlichkeit ist die uranfängliche Tatsache: es wäre nur
zu erklären, woher das Endliche stamme…."
— Friedrich Nietzsche, Das Philosophenbuch/Le livre du philosophe
(Paris: Aubier-Flammarion, 1969), fragment 120, p. 118
Cited as above, and translated as "Infinity is the original fact;
what has to be explained is the source of the finite…." in
The Production of Space , by Henri Lefebvre. (Oxford: Blackwell,
1991 (1974)), p. 181.
This quotation was suggested by the Bauhaus-related phrase
"the laws of cubical space" (see yesterday's Schau der Gestalt )
and by the laws of cubical space discussed in the webpage
Cube Space, 1984-2003.
For a less rigorous approach to space at the Harvard Graduate
School of Design, see earlier references to Lefebvre in this journal.
"To every man upon this earth,
Death cometh soon or late.
And how can man die better
Than facing fearful odds,
For the ashes of his fathers,
and the temples of his gods…?"
— Macaulay, quoted in the April 2013 film "Oblivion"
"Leave a space." — Tom Stoppard, "Jumpers"
Related material: The August 16, 2014, sudden death in Scotland
of an architect of the above Cardross seminary, and a Log24 post,
Plato's Logos, from the date of the above photo: June 26, 2010.
See also…
Here “eidolon” should instead be “eidos .”
An example of eidos — Plato's diamond (from the Meno ) —
(Continued from Aug. 19, 2014)
“Christian contemplation is the opposite
of distanced consideration of an image:
as Paul says, it is the metamorphosis of
the beholder into the image he beholds
(2 Cor 3.18), the ‘realisation’ of what the
image expresses (Newman). This is
possible only by giving up one’s own
standards and being assimilated to the
dimensions of the image.”
— Hans Urs von Balthasar,
The Glory of the Lord:
A Theological Aesthetics,
Vol. I: Seeing the Form
[ Schau der Gestalt ],
Ignatius Press, 1982, p. 485
A Bauhaus approach to Schau der Gestalt :
I prefer the I Ching ‘s approach to the laws of cubical space.
Powered by WordPress
.
.jpg){kind=link}
