The fictional zero theorem of Terry Gilliam's current film
by that name should not be confused with the zero system
underlying the diamond theorem.
Saturday, September 20, 2014
Symplectic Structure
Comments Off on Symplectic Structure
Sunday, August 24, 2014
Symplectic Structure…
In the Miracle Octad Generator (MOG):
The above details from a one-page note of April 26, 1986, refer to the
Miracle Octad Generator of R. T. Curtis, as it was published in 1976:
From R. T. Curtis (1976). A new combinatorial approach to M24,
Mathematical Proceedings of the Cambridge Philosophical Society ,
79, pp 25-42. doi:10.1017/S0305004100052075.
The 1986 note assumed that the reader would be able to supply, from the
MOG itself, the missing top row of each heavy brick.
Note that the interchange of the two squares in the top row of each
heavy brick induces the diamond-theorem correlation.
Note also that the 20 pictured 3-subsets of a 6-set in the 1986 note
occur as paired complements in two pictures, each showing 10 of the
3-subsets.
This pair of pictures corresponds to the 20 Rosenhain tetrads among
the 35 lines of PG(3,2), while the picture showing the 2-subsets
corresponds to the 15 Göpel tetrads among the 35 lines.
See Rosenhain and Göpel tetrads in PG(3,2). Some further background:
Comments Off on Symplectic Structure…
Wednesday, August 13, 2014
Symplectic Structure continued
Some background for the part of the 2002 paper by Dolgachev and Keum
quoted here on January 17, 2014 —
Related material in this journal (click image for posts) —
Comments Off on Symplectic Structure continued
Wednesday, August 6, 2014
Symplectic Structure*
From Gotay and Isenberg, "The Symplectization of Science,"
Gazette des Mathématiciens 54, 59-79 (1992):
"… what is the origin of the unusual name 'symplectic'? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure 'line complex group' the 'symplectic group.'
… the adjective 'symplectic' means 'plaited together' or 'woven.'
This is wonderfully apt…."
The above symplectic structure** now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Some related passages from the literature:
* The title is a deliberate abuse of language .
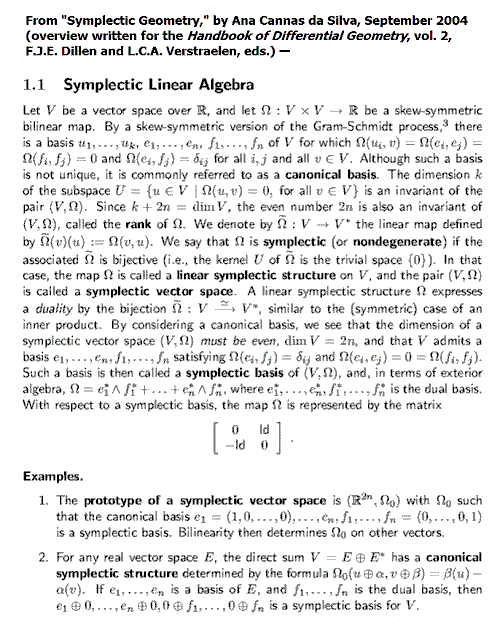
For the real definition of "symplectic structure," see (for instance)
"Symplectic Geometry," by Ana Cannas da Silva (article written for
Handbook of Differential Geometry, vol 2.) To establish that the
above figure is indeed symplectic , see the post Zero System of
July 31, 2014.
** See Steven H. Cullinane, Inscapes III, 1986
Comments Off on Symplectic Structure*
Wednesday, August 9, 2023
The Junction Function
A function (in this case, a 1-to-1 correspondence) from finite geometry:
This correspondence between points and hyperplanes underlies
the symmetries discussed in the Cullinane diamond theorem.
Academics who prefer cartoon graveyards may consult …
Cohn, N. (2014). Narrative conjunction’s junction function:
A theoretical model of “additive” inference in visual narratives.
Proceedings of the Annual Meeting of the Cognitive Science
Society, 36. See https://escholarship.org/uc/item/2050s18m .
Comments Off on The Junction Function
Wednesday, January 15, 2020
Paradigm Shift
Illustration, from a search in this journal for “Symplectic” —
.
Some background: Rift-design in this journal and …
Comments Off on Paradigm Shift
Tuesday, February 26, 2019
Citation
Some related material in this journal — See a search for k6.gif.
Some related material from Harvard —
Elkies's "15 simple transpositions" clearly correspond to the 15 edges of
the complete graph K6 and to the 15 2-subsets of a 6-set.
For the connection to PG(3,2), see Finite Geometry of the Square and Cube.
The following "manifestation" of the 2-subsets of a 6-set might serve as
the desired Wikipedia citation —
See also the above 1986 construction of PG(3,2) from a 6-set
in the work of other authors in 1994 and 2002 . . .
-
Gonzalez-Dorrego, Maria R. (Maria del Rosario),
(16,6) Configurations and Geometry of Kummer Surfaces in P3.
American Mathematical Society, Providence, RI, 1994. -
Dolgachev, Igor, and Keum, JongHae,
"Birational Automorphisms of Quartic Hessian Surfaces."
Trans. Amer. Math. Soc. 354 (2002), 3031-3057.
Comments Off on Citation
Sunday, December 10, 2017
Geometry
See also Symplectic in this journal.
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2). See also
related remarks on the notion of linear (or line ) complex
in the finite projective space PG(3,2) —
Comments Off on Geometry
Friday, April 14, 2017
Hudson and Finite Geometry
The above four-element sets of black subsquares of a 4×4 square array
are 15 of the 60 Göpel tetrads , and 20 of the 80 Rosenhain tetrads , defined
by R. W. H. T. Hudson in his 1905 classic Kummer's Quartic Surface .
Hudson did not view these 35 tetrads as planes through the origin in a finite
affine 4-space (or, equivalently, as lines in the corresponding finite projective
3-space).
In order to view them in this way, one can view the tetrads as derived,
via the 15 two-element subsets of a six-element set, from the 16 elements
of the binary Galois affine space pictured above at top left.
This space is formed by taking symmetric-difference (Galois binary)
sums of the 15 two-element subsets, and identifying any resulting four-
element (or, summing three disjoint two-element subsets, six-element)
subsets with their complements. This process was described in my note
"The 2-subsets of a 6-set are the points of a PG(3,2)" of May 26, 1986.
The space was later described in the following —
Comments Off on Hudson and Finite Geometry
Wednesday, February 15, 2017
Warp and Woof
Space —
Space structure —
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Space shuttle —
Related ethnic remarks —
… As opposed to Michael Larsen —
Funny, you don't look Danish.
Comments Off on Warp and Woof
Wednesday, November 23, 2016
Yogiism
From the American Mathematical Society (AMS) webpage today —
From the current AMS Notices —
Related material from a post of Aug. 6, 2014 —
(Here "five point sets" should be "five-point sets.")
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
The above symplectic structure* now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
* The phrase as used here is a deliberate
abuse of language . For the real definition of
“symplectic structure,” see (for instance)
“Symplectic Geometry,” by Ana Cannas da Silva
(article written for Handbook of Differential
Geometry , Vol 2.) To establish that the above
figure is indeed symplectic , see the post
Zero System of July 31, 2014.
Comments Off on Yogiism
Friday, September 16, 2016
A Counting-Pattern
Comments Off on A Counting-Pattern
Saturday, January 30, 2016
Pope’s Geometry
From page 56 of The Science Fiction of Mark Clifton ,
Southern Illinois University Press, 1980 —
See also the following image in this journal —
.
Comments Off on Pope’s Geometry
Saturday, October 31, 2015
Weaving World…
Comments Off on Weaving World…
Thursday, October 15, 2015
Contrapuntal Interweaving
The title is a phrase from R. D. Laing's book The Politics of Experience .
(Published in the psychedelic year 1967. The later "contrapuntal interweaving"
below is of a less psychedelic nature.)
An illustration of the "interweaving' part of the title —
The "deep structure" of the diamond theorem:
.
The word "symplectic" from the end of last Sunday's (Oct. 11) sermon
describes the "interwoven" nature of the above illustration.
An illustration of the "contrapuntal" part of the title (click to enlarge):
Comments Off on Contrapuntal Interweaving
Friday, July 3, 2015
Crunching Entities*
A figure I prefer to the "Golden Tablet" of Night at the Museum —
The source — The Log24 post "Zero System" of July 31, 2014.
* For the title, see The New Yorker of Sept. 22, 2014.
Comments Off on Crunching Entities*
Monday, December 15, 2014
Mythic Metaphysics
Today’s 8:01 PM post quoted Husserl on
the perception of the cube.
Another approach to perception of the cube,
from Narrative Metaphysics on St. Lucia’s Day —
 See also Symplectic Structure and Stevens’s Rock. |
From today’s 11:29 AM post —
John Burt Foster Jr. in Nabokov’s Art of Memory and
European Modernism (Princeton U. Press, 1993, p. 224) —
At the time of The Waste Land , in a comment on
Joyce’s Ulysses that influenced many later definitions
of modernism in the English-speaking world, Eliot
announced, “instead of narrative method, we may
now use the mythical method.”13
For some illuminating remarks on a mythical approach
to perception of the cube, see Gareth Knight on Schicksalstag 2012.
Comments Off on Mythic Metaphysics
Saturday, December 13, 2014
Narrative Metaphysics
From "Guardians of the Galaxy" —
"Then the Universe exploded into existence…"
For those who prefer a more traditional approach :
See also Symplectic Structure and Stevens's Rock.
Comments Off on Narrative Metaphysics
Monday, November 3, 2014
The Rhetoric of Abstract Concepts
From a post of June 3, 2013:
New Yorker editor David Remnick at Princeton today
(from a copy of his prepared remarks):
“Finally, speaking of fabric design….”
I prefer Tom and Harold:
Tom Wolfe in The Painted Word —
“I am willing (now that so much has been revealed!)
to predict that in the year 2000, when the Metropolitan
or the Museum of Modern Art puts on the great
retrospective exhibition of American Art 1945-75,
the three artists who will be featured, the three seminal
figures of the era, will be not Pollock, de Kooning, and
Johns-but Greenberg, Rosenberg, and Steinberg.
Up on the walls will be huge copy blocks, eight and a half
by eleven feet each, presenting the protean passages of
the period … a little ‘fuliginous flatness’ here … a little
‘action painting’ there … and some of that ‘all great art
is about art’ just beyond. Beside them will be small
reproductions of the work of leading illustrators of
the Word from that period….”
Harold Rosenberg in The New Yorker (click to enlarge)—
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
— Steven H. Cullinane,
diamond theorem illustration
Comments Off on The Rhetoric of Abstract Concepts
Friday, August 22, 2014
Review
Comments Off on Review
Friday, August 15, 2014
The Omega Matrix
The webpage Rosenhain and Göpel Tetrads in PG(3,2)
has been updated to include more material on symplectic structure.
Comments Off on The Omega Matrix
Wednesday, August 13, 2014
Stranger than Dreams*
Illustration from a discussion of a symplectic structure
in a 4×4 array quoted here on January 17, 2014 —
See symplectic structure in this journal.
* The final words of Point Omega , a 2010 novel by Don DeLillo.
See also Omega Matrix in this journal.
Comments Off on Stranger than Dreams*
Monday, August 11, 2014
Syntactic/Symplectic
(Continued from August 9, 2014.)
Syntactic:
Symplectic:
"Visual forms— lines, colors, proportions, etc.— are just as capable of
articulation , i.e. of complex combination, as words. But the laws that govern
this sort of articulation are altogether different from the laws of syntax that
govern language. The most radical difference is that visual forms are not
discursive . They do not present their constituents successively, but
simultaneously, so the relations determining a visual structure are grasped
in one act of vision."
– Susanne K. Langer, Philosophy in a New Key
For examples, see The Diamond-Theorem Correlation
in Rosenhain and Göpel Tetrads in PG(3,2).
This is a symplectic correlation,* constructed using the following
visual structure:
.
* Defined in (for instance) Paul B. Yale, Geometry and Symmetry ,
Holden-Day, 1968, sections 6.9 and 6.10.
Comments Off on Syntactic/Symplectic
Saturday, August 9, 2014
Syntactic/Symplectic
Syntactic Structure —
See the Lightfoot of today’s previous post:
Symplectic Structure —
See the plaited, or woven, structure of August 6:
.
See also Deep Structure (Dec. 9, 2012).
Comments Off on Syntactic/Symplectic
Thursday, August 7, 2014
Abuse of Language
From Wikipedia — Abuse of language —
“… in mathematics, a use of terminology in a way that is not formally correct
but that simplifies exposition or suggests the correct intuition.”
The phrase “symplectic structure” in the previous post
was a deliberate abuse of language. The real definition:
Comments Off on Abuse of Language
Friday, August 1, 2014
The Diamond-Theorem Correlation
Click image for a larger, clearer version.
Comments Off on The Diamond-Theorem Correlation
Thursday, July 31, 2014
Zero System
The title phrase (not to be confused with the film 'The Zero Theorem')
means, according to the Encyclopedia of Mathematics,
a null system , and
"A null system is also called null polarity,
a symplectic polarity or a symplectic correlation….
it is a polarity such that every point lies in its own
polar hyperplane."
See Reinhold Baer, "Null Systems in Projective Space,"
Bulletin of the American Mathematical Society, Vol. 51
(1945), pp. 903-906.
An example in PG(3,2), the projective 3-space over the
two-element Galois field GF(2):
See also the 10 AM ET post of Sunday, June 8, 2014, on this topic.
Comments Off on Zero System
Sunday, June 8, 2014
Vide
"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Comments Off on Vide
Friday, January 17, 2014
The 4×4 Relativity Problem
The sixteen-dot square array in yesterday’s noon post suggests
the following remarks.
“This is the relativity problem: to fix objectively a class of
equivalent coordinatizations and to ascertain the group of
transformations S mediating between them.”
— Hermann Weyl, The Classical Groups ,
Princeton University Press, 1946, p. 16
The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977—
The 1977 matrix Q is echoed in the following from 2002—
A different representation of Cullinane’s 1977 square model of the
16-point affine geometry over the two-element Galois field GF(2)
is supplied by Conway and Sloane in Sphere Packings, Lattices and Groups
(first published in 1988) :
Here a, b, c, d are basis vectors in the vector 4-space over GF(2).
(For a 1979 version of this vector space, see AMS Abstract 79T-A37.)
See also a 2011 publication of the Mathematical Association of America —
Comments Off on The 4×4 Relativity Problem