
Monday, March 24, 2025
Elegy in a Cartoon Graveyard
A Combinatorial Configuration
Related art —
From "Self-Dual Configurations and Regular Graphs" by H. S. M. Coxeter,
Bulletin of the American Mathematical Society, Vol. 56 (1950), pp. 413-455
For a related combinatorial configuration, take Oxbury's "16 lines"
to be the the 16 dots above and take the "8 points of intersection"
to be the four squares
234, 1234, 124, 24
23, 123, 12, 2
3, 13, 1, 0
34, 134, 14, 4
along with the four diamonds
234, 23, 3, 34
1234, 123, 13, 134
124, 12, 1, 14
24, 2, 0, 4.
Then each "line" is on two "points" and each "point" on
four "lines."
Note that these eight "points" — the four squares and the four diamonds
of Coxeter's figure — form the rows and columns of the following matrix:
| 234 | 1234 | 124 | 24 |
| 23 | 123 | 12 | 2 |
| 3 | 13 | 1 | 0 |
| 34 | 134 | 14 | 4 |
Related reading: Points with Parts .
Sunday, March 23, 2025
KenKen.casa
The above new URL now forwards to this journal 10 years ago.
See also KenKen.
(This post was suggested by the phrase "10 years later"
from the image in the previous post.)
“Accomplished in Steps”
For the title, see McCaffrey+Steps in this journal.
Graphic Sermon
The release date of the Paul Schrader film "First Reformed"
was May 18, 2018. This suggests the following graphic art . . .
From this journal on that date, art from The New York Times —
Ninefold-square art that is much more recent —

Thursday, March 26, 2015
The Möbius Hypercube
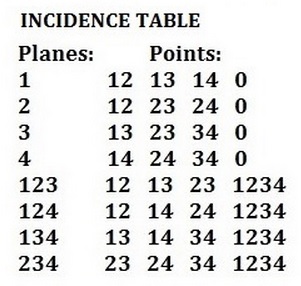
The incidences of points and planes in the
Möbius 84 configuration (8 points and 8 planes,
with 4 points on each plane and 4 planes on each point),
were described by Coxeter in a 1950 paper.*
A table from Monday's post summarizes Coxeter's
remarks, which described the incidences in
spatial terms, with the points and planes as the vertices
and face-planes of two mutually inscribed tetrahedra —
Monday's post, "Gallucci's Möbius Configuration,"
may not be completely intelligible unless one notices
that Coxeter has drawn some of the intersections in his
Fig. 24, a schematic representation of the point-plane
incidences, as dotless, and some as hollow dots. The figure,
"Gallucci's version of Möbius's 84," is shown below.
The hollow dots, representing the 8 points (as opposed
to the 8 planes ) of the configuration, are highlighted in blue.

Here a plane (represented by a dotless intersection) contains
the four points that are represented in the square array as lying
in the same row or same column as the plane.
The above Möbius incidences appear also much earlier in
Coxeter's paper, in figures 6 and 5, where they are shown
as describing the structure of a hypercube.
In figures 6 and 5, the dotless intersections representing
planes have been replaced by solid dots. The hollow dots
have again been highlighted in blue.


Figures 6 and 5 demonstrate the fact that adjacency in the set of
16 vertices of a hypercube is isomorphic to adjacency in the set
of 16 subsquares of a square 4×4 array, provided that opposite
sides of the array are identified, as in Fig. 6. The digits in
Coxeter's labels above may be viewed as naming the positions
of the 1's in (0,1) vectors (x4, x3, x2, x1) over the two-element
Galois field.† In that context, the 4×4 array may be called, instead
of a Möbius hypercube , a Galois tesseract .
* "Self-Dual Configurations and Regular Graphs,"
Bulletin of the American Mathematical Society,
Vol. 56 (1950), pp. 413-455
† The subscripts' usual 1-2-3-4 order is reversed as a reminder
that such a vector may be viewed as labeling a binary number
from 0 through 15, or alternately as labeling a polynomial in
the 16-element Galois field GF(24). See the Log24 post
Vector Addition in a Finite Field (Jan. 5, 2013).
Monday, March 23, 2015
Gallucci’s Möbius Configuration
From H. S. M. Coxeter's 1950 paper
"Self-Dual Configurations and Regular Graphs,"
a 4×4 array and a more perspicuous rearrangement—
(Click image to enlarge.)
The above rearrangement brings Coxeter's remarks into accord
with the webpage The Galois Tesseract.
Update of Thursday, March 26, 2015 —
For an explanation of Coxeter's Fig. 24, see Thursday's later
post titled "The Möbius Hypercube."




