The previous post's image illustrating the
ancient Lo Shu square as an affine transformation
suggests a similar view of Dürer's square.
That view illustrates the structural principle
underlying the diamond theorem —
Thursday, September 22, 2022
Affine Dürer
Comments Off on Affine Dürer
Wednesday, October 18, 2017
Dürer for St. Luke’s Day
Structure of the Dürer magic square
16 3 2 13
5 10 11 8 decreased by 1 is …
9 6 7 12
4 15 14 1
15 2 1 12
4 9 10 7
8 5 6 11
3 14 13 0 .
Base 4 —
33 02 01 30
10 21 22 13
20 11 12 23
03 32 31 00 .
Two-part decomposition of base-4 array
as two (non-Latin) orthogonal arrays —
3 0 0 3 3 2 1 0
1 2 2 1 0 1 2 3
2 1 1 2 0 1 2 3
0 3 3 0 3 2 1 0 .
Base 2 –
1111 0010 0001 1100
0100 1001 1010 0111
1000 0101 0110 1011
0011 1110 1101 0000 .
Four-part decomposition of base-2 array
as four affine hyperplanes over GF(2) —
1001 1001 1100 1010
0110 1001 0011 0101
1001 0110 0011 0101
0110 0110 1100 1010 .
— Steven H. Cullinane,
October 18, 2017
See also recent related analyses of
noted 3×3 and 5×5 magic squares.
Comments Off on Dürer for St. Luke’s Day
Thursday, December 6, 2012
Magic Square
This post was suggested by the December 4th death
of modernist composer Jonathan Harvey, 73,
and by Harvey's reflections on his 2007 opera
Wagner Dream .
For related reflections, see the Oct. 10 post on
the Dürer magic square in Mann's Doctor Faustus .
See also a December 2nd post on the Nov. 18 death of
chess grandmaster Elena Akhmilovskaya Donaldson.
Comments Off on Magic Square
Thursday, September 19, 2024
Embedding a Language Witch
Continuing yesterday's "Bell, Book and Candle" theme . . .
[Such lines and planes have not been, in mathematical language,
"translated."]
— Paul R. Halmos, Finite Dimensional Vector Spaces,
Princeton University Press, 1948, page 14
Candle from Sense8, Season 1, Episode 1: “Limbic Resonance”
Comments Off on Embedding a Language Witch
Thursday, July 11, 2024
Saturday, May 18, 2024
The Godfather’s Art
Cover illustration:
Spies returning from the land of
Canaan with a cluster of grapes.
Colored woodcut from
Biblia Sacra Germanica ,
Nuremberg, Anton Koberger, 1483.
Victoria and Albert Museum, London.
Related material —
The Faustus Square :
Design from 1514
Comments Off on The Godfather’s Art
Thursday, January 18, 2024
But Seriously: Mathematics for Davos
Click image to enlarge.
Comments Off on But Seriously: Mathematics for Davos
Saturday, January 21, 2023
Dead-Poet Witcraft
"Death is the mother of beauty." — Wallace Stevens
From the 2020 Feast of St. Wallace Stevens,
who reportedly died in 1955 on August 2 —
Related material —
Exercise: Can each order-4 magic square be obtained by some
transformation like the one above (i.e., preserving affine hyperplanes)?
If not, why not?
Update of 31 Jan. 2023 — Spoiler: Remarks by Tilman Piesk.
Comments Off on Dead-Poet Witcraft
Monday, January 17, 2022
Finest Trick
"The Magician’s finest trick was to
dismantle the pretensions of genius
while preserving his own lofty stature."
— Alex Ross in The New Yorker , Jan. 17, 2022
Related material —
Meanwhile . . .
Comments Off on Finest Trick
Sunday, August 2, 2020
Sunday, August 7, 2016
A Talisman for Finkelstein
The late physicist David Ritz Finkelstein on the magic square
in Dürer's "Melencolia I" —
"As a child I wondered why such a square was called magic.
The Occult Philosophy [of Agrippa] answers this question
at least. They were used as magical talismans."
The correspondence in the previous post between
Figures A and B may serve as a devotional talisman
in memory of Finkelstein, a physicist who, in the sort of
magical thinking enjoyed by traditional Catholics, might
still be lingering in Purgatory.
See also this journal on the date of Finkelstein's death —
Comments Off on A Talisman for Finkelstein
Saturday, March 7, 2015
Film and Phenomenology
Continued from All Hallows' Eve, 2014.
Last year's Halloween post displayed the
Dürer print Knight, Death, and the Devil
(illustrated below on the cover of the book
Film and Phenomenology by Allan Casebier).
Cover illustration: Knight, Death, and the Devil,
by Albrecht Dürer
Some mathematics related to a different Dürer print —
Comments Off on Film and Phenomenology
Friday, October 31, 2014
For the Late Hans Schneider
See a University of Wisconsin obituary for Schneider,
a leading expert on linear algebra who reportedly died
at 87 on Tuesday, October 28, 2014.
Some background on linear algebra and “magic” squares:
tonight’s 3 AM (ET) post and a search in this
journal for Knight, Death, and the Devil.
Click image to enlarge.
Comments Off on For the Late Hans Schneider
Structure
Introducing a group of 322,560 affine transformations of Dürer’s ‘Magic’ Square
The four vector-space substructures of digits in 1st, 2nd, 3rd, 4th place,
together with the diamond theorem, indicate that Dürer’s square “minus one”
can be transformed by permutations of rows, columns, and quadrants to a
square with (decimal) digits in the usual numerical order, increasing from
top left to bottom right. Such permutations form a group of order 322,560.
(Continued from Vector Addition in a Finite Field, Twelfth Night, 2013.)
Comments Off on Structure
Tuesday, April 9, 2013
Four Quartets
For the cruelest month
Click for a much larger version of the photo below.
These four Kountry Korn quartets are from the Fox Valleyaires
Men's Barbershop Chorus of Appleton, Wisconsin.
See also the fine arts here on Saturday, April 6, 2013—
The New York Times Magazine cover story
a decade ago, on Sunday, April 6, 2003:
"The artists demanded space
in tune with their aesthetic."
— "The Dia Generation,"
by Michael Kimmelman
Related material:
See Wikipedia for the difference between binary numbers
and binary coordinates from the finite Galois field GF(2).
For some background, see the relativity problem.
See also the chapter on vector spaces in Korn & Korn
(originally published by McGraw-Hill)—
.
Comments Off on Four Quartets
Monday, April 8, 2013
Magic for Jews
A commenter on Saturday's "Seize the Dia" has
suggested a look at the work of one Mark Collins.
Here is such a look (click to enlarge):
I find attempts to associate pure mathematics with the words
"magic" or "mystic" rather nauseating. (H. F. Baker's work
on Pascal's mystic hexagram is no exception; Baker was
stuck with Pascal's obnoxious adjective, but had no truck
with any mystic aspects of the hexagram.)
The remarks above by Clifford Pickover on Collins, Dürer, and
binary representations may interest some non-mathematicians,
who should not be encouraged to waste their time on this topic.
For the mathematics underlying the binary representation of
Dürer's square, see, for instance, my 1984 article "Binary
Coordinate Systems."
Those without the background to understand that article
may enjoy, instead of Pickover's abortive attempts above at
mathematical vulgarization, his impressively awful 2009 novel
Jews in Hyperspace .
Pickover's 2002 book on magic squares was, unfortunately,
published by the formerly reputable Princeton University Press.
Related material from today's Daily Princetonian :
See also Nash + Princeton in this journal.
Comments Off on Magic for Jews
Tuesday, January 10, 2012
Defining Form
(Continued from Epiphany and from yesterday.)
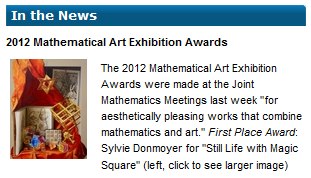
Detail from the current American Mathematical Society homepage—
Further detail, with a comparison to Dürer’s magic square—
 |
 |
The three interpenetrating planes in the foreground of Donmoyer‘s picture
provide a clue to the structure of the the magic square array behind them.
Group the 16 elements of Donmoyer’s array into four 4-sets corresponding to the
four rows of Dürer’s square, and apply the 4-color decomposition theorem.
Note the symmetry of the set of 3 line diagrams that result.
Now consider the 4-sets 1-4, 5-8, 9-12, and 13-16, and note that these
occupy the same positions in the Donmoyer square that 4-sets of
like elements occupy in the diamond-puzzle figure below—
Thus the Donmoyer array also enjoys the structural symmetry,
invariant under 322,560 transformations, of the diamond-puzzle figure.
Just as the decomposition theorem’s interpenetrating lines explain the structure
of a 4×4 square , the foreground’s interpenetrating planes explain the structure
of a 2x2x2 cube .
For an application to theology, recall that interpenetration is a technical term
in that field, and see the following post from last year—
| Saturday, June 25, 2011
— m759 @ 12:00 PM “… the formula ‘Three Hypostases in one Ousia ‘
Ousia
|
Comments Off on Defining Form