Thursday, November 16, 2017
Saturday, January 6, 2018
Yale News
The Yale of the title is not the university, but rather the
mathematician Paul B. Yale. Yale's illustration of the Fano
plane is below.
A different illustration from a mathematician named Greenberg —
This illustration of the ominous phrase "line at infinity"
may serve as a sort of Deathly Hallows for Greenberg.
According to the AMS website yesterday, he died on
December 12, 2017:
A search of this journal for Greenberg yields no mention of
the dead mathematician, but does yield some remarks
on art that are pehaps less bleak than the above illustration.
For instance —
Art adapted from the Google search screen. Discuss.
Comments Off on Yale News
Sunday, December 31, 2017
Ich, Du, etc., etc.
Recent posts involving the English pronoun IT referred to
classic tales of horror by Madeleine L'Engle and Stephen King.
Those posts suggest some further remarks by Martin Buber:
THE WORLD IS TWOFOLD for man
in accordance with his twofold attitude.
The attitude of man is twofold
in accordance with the two basic words he can speak.
The basic words are not single words but word pairs.
One basic word is the word pair I-You.
The other basic word is the word pair I-It;
but this basic word is not changed when
He or She takes the place of It.
Thus the I of man is also twofold.
For the I of the basic word I-You is different from
that in the basic word I-It.
— Buber, Martin. I and Thou, Trans. Kaufmann
(p. 53). Kindle Edition.
Four German pronouns from the above passage
by Martin Buber lead to six pronoun pairs:
ich-du, ich-es, ich-sie, du-es, du-sie, es-sie.
This is in accordance with some 1974 remarks by
Marie-Louise von Franz —
The following passage by Buber may confuse readers of
L'Engle and King with its use, in translation, of "it" instead of
the original German "sie" ("she," corresponding to "die Welt") —
Here, for comparison, are the original German and the translation.
As for "that you in which the lines of relation, though parallel,
intersect," and "intimations of eternity," see Log24 posts on
the concept "line at infinity" as well as "Lost Horizon."
Comments Off on Ich, Du, etc., etc.
Wednesday, November 1, 2017
Cameron on All Saints’ Day
"Nowdays, Halloween involves plastic figures of ghosts and bats
bought from the supermarket; this is driven by commerce and
in some people’s view is an American import. But it is clear that
this time of year was traditionally regarded as one where the barrier
between this world and the other was low, and supernatural
manifestations were to be expected."
Remarks related to another "barrier" and vértigo horizontal —
See also a search for Horizon + "Western Australia" in this journal.
From that search: A sort of horizon, a "line at infinity," that is perhaps
more meaningful to most Cameron readers than the above remarks
by Borges —
Comments Off on Cameron on All Saints’ Day
Wednesday, February 22, 2017
Horizon
A memorable phrase —
"the transcendental horizon of the ‘I’."
For some backstory, see a Google search for
Marion + transcendental + horizon.
For a perhaps more intelligible horizon, see
Line at Infinity in this journal.
Comments Off on Horizon
Sunday, January 1, 2017
Like the Horizon
(Continued from a remark by art critic Peter Schjeldahl quoted here
last year on New Year's Day in the post "Art as Religion.")
"The unhurried curve got me.
It was like the horizon of a world
that made a non-world of
all of the space outside it."
— Peter Schjeldahl, "Postscript: Ellsworth Kelly,"
The New Yorker , December 30, 2015
This suggests some further material from the paper
that was quoted here yesterday on New Year's Eve —
"In teaching a course on combinatorics I have found
students doubting the existence of a finite projective
plane geometry with thirteen points on the grounds
that they could not draw it (with 'straight' lines)
on paper although they had tried to do so. Such a
lack of appreciation of the spirit of the subject is but
a consequence of the elements of formal geometry
no longer being taught in undergraduate courses.
Yet these students were demanding the best proof of
existence, namely, production of the object described."
— Derrick Breach (See his obituary from 1996.)
A related illustration of the 13-point projective plane
from the University of Western Australia:
Projective plane of order 3
(The four points on the curve
at the right of the image are
the points on the line at infinity .)
The above image is from a post of August 7, 2012,
"The Space of Horizons." A related image —
Click on the above image for further remarks.
Comments Off on Like the Horizon
Friday, February 26, 2016
Literacy Test
Comments Off on Literacy Test
Wednesday, May 13, 2015
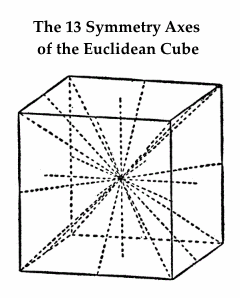
Space
Notes on space for day 13 of May, 2015 —
The 13 symmetry axes of the cube may be viewed as
the 13 points of the Galois projective space PG(2,3).
This space (a plane) may also be viewed as the nine points
of the Galois affine space AG(2,3) plus the four points on
an added "line at infinity."
Related poetic material:
The ninefold square and Apollo, as well as …
Comments Off on Space
Friday, January 16, 2015
A versus PA
"Reality is the beginning not the end,
Naked Alpha, not the hierophant Omega,
of dense investiture, with luminous vassals."
— “An Ordinary Evening in New Haven” VI
From the series of posts tagged "Defining Form" —
The 4-point affine plane A and
the 7-point projective plane PA —
The circle-in-triangle of Yale's Figure 30b (PA ) may,
if one likes, be seen as having an occult meaning.
For the mathematical meaning of the circle in PA
see a search for "line at infinity."
A different, cubic, model of PA is perhaps more perspicuous.
Comments Off on A versus PA
Monday, December 29, 2014
Dodecahedron Model of PG(2,5)
Recent posts tagged Sagan Dodecahedron
mention an association between that Platonic
solid and the 5×5 grid. That grid, when extended
by the six points on a "line at infinity," yields
the 31 points of the finite projective plane of
order five.
For details of how the dodecahedron serves as
a model of this projective plane (PG(2,5)), see
Polster's A Geometrical Picture Book , p. 120:
For associations of the grid with magic rather than
with Plato, see a search for 5×5 in this journal.
Comments Off on Dodecahedron Model of PG(2,5)
Monday, October 6, 2014
Arcs and Shards
Ben Brantley in The New York Times today on a Broadway opening:
“As Christopher navigates his way through an increasingly
unfamiliar landscape, both physical and emotional, the arcs
of his adventures are drawn into being.
So are the shards of sensory overload.”
Arc — See a search for Line at Infinity:
Shard — See Shard and Pythagorean Selfie:
Comments Off on Arcs and Shards
Tuesday, August 7, 2012
The Space of Horizons
"In the space of horizons that neither love nor hate"
— Wallace Stevens, "Things of August"
Seven years ago yesterday—
For some context, see Rosetta Stone as a Metaphor.
Related material from the University of Western Australia—
Projective plane of order 3
(The four points on the curve
at the right of the image are
the points on the line at infinity.)
Art critic Robert Hughes, who nearly died in Western
Australia in a 1999 car crash, actually met his death
yesterday at Calvary Hospital in the Bronx.
See also Hughes on "slow art" in this journal.
Comments Off on The Space of Horizons
Wednesday, August 1, 2012
Elementary Finite Geometry
I. General finite geometry (without coordinates):
A finite affine plane of order n has n^2 points.
A finite projective plane of order n has n^2 + n + 1
points because it is formed from an order-n finite affine
plane by adding a line at infinity that contains n + 1 points.
Examples—
|
|
|
II. Galois finite geometry (with coordinates over a Galois field):
A finite projective Galois plane of order n has n^2 + n + 1
points because it is formed from a finite affine Galois 3-space
of order n with n^3 points by discarding the point (0,0,0) and
identifying the points whose coordinates are multiples of the
(n-1) nonzero scalars.
Note: The resulting Galois plane of order n has
(n^3-1)/(n-1)= (n^2 + n + 1) points because
(n^2 + n + 1)(n – 1) =
(n^3 + n^2 + n – n^2 – n – 1) = (n^3 – 1) .
III. Related art:
Another version of a 1994 picture that accompanied a New Yorker
article, "Atheists with Attitude," in the issue dated May 21, 2007:
The Four Gods of Borofsky correspond to the four axes of
symmetry of a square and to the four points on a line at infinity
in an order-3 projective plane as described in Part I above.
Those who prefer literature to mathematics may, if they like,
view the Borofsky work as depicting
"Blake's Four Zoas, which represent four aspects
of the Almighty God" —Wikipedia
Comments Off on Elementary Finite Geometry
Thursday, August 28, 2008
Thursday August 28, 2008
Associations
for the writer
known as UD
for the writer
known as UD
|
"Have liberty not as the air within a grave Or down a well. Breathe freedom, oh, my native, In the space of horizons that neither love nor hate."
— Wallace Stevens, |
A related visual
association of ideas —
("The association is the idea"
— Ian Lee, The Third Word War)
|
by John Braheny
"Hook" is the term you'll hear most often in the business and craft of commercial songwriting. (Well, maybe not as much as "Sorry, we can't use your song," but it's possible that the more you hear about hooks now, the less you'll hear "we can't use it" later.) |
See also UD's recent
A Must-Read and In My Day*
as well as the five
Log24 entries ending
Sept. 20, 2002.
More seriously:
The date of The New Yorker issue quoted above is also the anniversary of the birth of Wallace Stevens and the date of death of mathematician Paul R. Halmos.
Stevens's "space of horizons" may, if one likes, be interpreted as a reference to projective geometry. Despite the bleak physicist's view of mathematics quoted above, this discipline is– thanks to Blaise Pascal— not totally lacking in literary and spiritual associations.
* Hey Hey
Comments Off on Thursday August 28, 2008