Wednesday, June 22, 2022

Click the above galaxy for a larger image.
"O God, I could be bounded in a nutshell
and count myself a king of infinite space,
were it not that I have bad dreams." — Hamlet
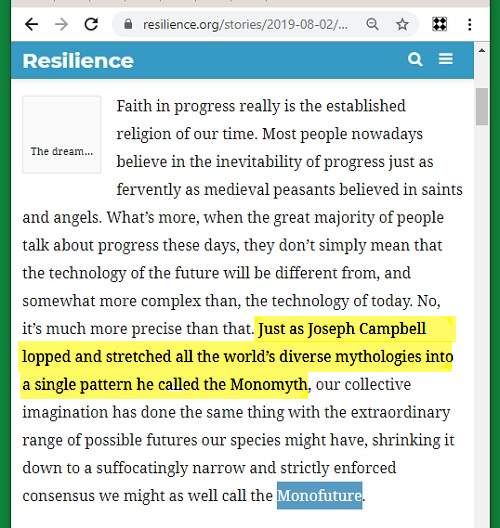
Battle of the Nutshells —

From a much larger nutshell
on the above code date—

Comments Off on Code Wars: “Use the Source, Luke.”
Tuesday, May 31, 2022
Last night's posts on triangles, and today's anniversary of the
death of Evariste Galois, suggest a review . . .

"Take triangles, perhaps" . . . as a category.
And then . . . take squares, perhaps, as another category,
and then . . . find a suitable "translation machine."
See "Square Triangles."
* Title adapted from a 2001 essay by Pierre Cartier.
Comments Off on A Mad Night’s Work*
Saturday, May 28, 2022
Last two days of the conference, May 27 and 28, 2022 —
|
27th Friday
9:00 – 10:00 Andrés Villaveces (Univ. Nacional de Colombia):
Galoisian model theory:
the role(s) of Grothendieck (à son insu! )
10:00 – 11:00 Olivia Caramello (Univ. of Insubria; by Zoom):
The “unifying notion” of topos 1
1:00 – 11:15 Coffee Break
1:15 – 12:15 Mike Shulman (Univ. of San Diego):
Lifting Grothendieck universes to Grothendieck toposes
12:15 – 1:15 José Gil-Ferez (Chapman Univ.)
The Isomorphism Theorem of Algebraic Logic:
a Categorical Perspective
1:15 – 2:30 Lunch
2:30 – 3:30 Oumar Wone (Chapman) :
Vector bundles on Riemann surfaces according to
Grothendieck and his followers
3:30 – 4:30 Claudio Bartocci (Univ. of Genova):
The inception of the theory of moduli spaces:
Grothendieck's Quot scheme
4:30 – 5:30 Christian Houzel (IUFM de Paris):
Riemann surfaces after Grothendieck
[presented by J.J. Szczeciniarz]
28th Saturday
9:00 – 10:00 Silvio Ghilardi (Univ. degli Studi, Milano):
Investigating definability in propositional logic
via Grothendieck topologies and sheaves
10:00 – 11:00 Matteo Viale (Univ. of Turin; by zoom):
The duality between Boolean valuated models and
topological presheaves
11:00 – 11:15 Coffee Break
11:15 – 12:15 Benjamin Collas (RIMS, Kyoto Univ.):
Galois-Teichmüller: arithmetic geometric principles
12:15 – 1:15 Closing: general discussion
animated by Alex Kurz (Chapman)
|
Comments Off on Grothendieck at Chapman …
Friday, May 27, 2022

The above scene from "Hanna" comes from a webpage
dated August 29, 2011. See also …
this journal on that date —

… and today's previous "Escape" post.
Comments Off on Great Escapes
"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
Scholium —
Abstracting from narrative to structure, and from structure
to pure number, the Tablet of Ahkmenrah represents the
number 9 and the Cube of Rubik represents the number 27.
Returning from pure abstract numbers to concrete representations,
9 yields the structures in Log24 posts tagged Triangle.graphics,
and 27 yields a Galois cube .
Comments Off on Plan 9 from Disney
Tuesday, May 24, 2022
From a Jamestown (NY) Post-Journal article yesterday on
"the sold-out 10,000 Maniacs 40th anniversary concert at
The Reg Lenna Center Saturday" —
" 'The theater has a special place in our hearts. It’s played
a big part in my life,' Gustafson said.
Before being known as The Reg Lenna Center for The Arts,
it was formerly known as The Palace Theater. He recalled
watching movies there as a child…."
This, and the band's name, suggest some memories perhaps
better suited to the cinematic philosophy behind "Plan 9 from
Outer Space."

"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
The above 3×3 Tablet of Ahkmenrah image comes from
a Log24 search for the finite (i.e., Galois) field GF(3) that
was, in turn, suggested by last night's post "Making Space."
See as well a mysterious document from a website in Slovenia
that mentions a 3×3 array "relating to nine halls of a mythical
palace where rites were performed in the 1st century AD" —

Comments Off on Playing the Palace
Saturday, May 7, 2022

Related material — Posts tagged Interality and Seven Seals.
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
Comments Off on Interality Meets the Seven Seals
Monday, April 25, 2022

Some related remarks —

Comments Off on Annals of Mathematical History
Monday, April 18, 2022
An illustration from posts tagged Holy Field GF(3) —
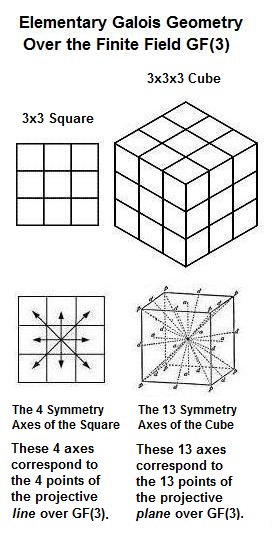
See also a Log24 search for "Four Gods."
Comments Off on Iconic Simplicity
Friday, April 8, 2022
Comments Off on Souls at Stanford
Wednesday, March 30, 2022

Click to enlarge.
Related reading — George Steiner's Fields of Force , on chess in Iceland, and . . .
The New Yorker , article by Sam Knight dated March 28, 2022 —
They went to Björk’s house. She cooked salmon.
She had seen “The Witch” and introduced Eggers
to Sjón, who had written a novel about seventeenth-
century witchcraft in Iceland. When he got home,
Eggers read Sjón’s books. “I was, like, this guy’s
a fucking magician,” Eggers said. “He sees all time,
in time, out of time.”
Comments Off on Games
Saturday, March 26, 2022
Many structures of finite geometry can be modeled by
rectangular or cubical arrays ("boxes") —
of subsquares or subcubes (also "boxes").
Here is a draft for a table of related material, arranged
as internet URL labels.
Finite Geometry Notes — Summary Chart
|
Name Tag
|
.Space
|
.Group
|
.Art
|
|
Box4
|
2×2 square representing the four-point finite affine geometry AG(2,2).
(Box4.space)
|
S4 = AGL(2,2)
(Box4.group)
|

(Box4.art)
|
|
Box6
|
3×2 (3-row, 2-column) rectangular array
representing the elements of an arbitrary 6-set.
|
S6
|
|
|
Box8
|
2x2x2 cube or 4×2 (4-row, 2-column) array.
|
S8 or A8 or AGL(3,2) of order 1344, or GL(3,2) of order 168
|
|
|
Box9
|
The 3×3 square.
|
AGL(2,3) or GL(2,3)
|
|
|
Box12
|
The 12 edges of a cube, or a 4×3 array for picturing the actions of the Mathieu group M12.
|
Symmetries of the cube or elements of the group M12
|
|
|
Box13
|
The 13 symmetry axes of the cube.
|
Symmetries of the cube.
|
|
|
Box15
|
The 15 points of PG(3,2), the projective geometry
of 3 dimensions over the 2-element Galois field.
|
Collineations of PG(3,2)
|
|
|
Box16
|
The 16 points of AG(4,2), the affine geometry
of 4 dimensions over the 2-element Galois field.
|
AGL(4,2), the affine group of
322,560 permutations of the parts
of a 4×4 array (a Galois tesseract)
|
|
|
Box20
|
The configuration representing Desargues's theorem.
|
|
|
|
Box21
|
The 21 points and 21 lines of PG(2,4).
|
|
|
|
Box24
|
The 24 points of the Steiner system S(5, 8, 24).
|
|
|
|
Box25
|
A 5×5 array representing PG(2,5).
|
|
|
|
Box27
|
The 3-dimensional Galois affine space over the
3-element Galois field GF(3).
|
|
|
|
Box28
|
The 28 bitangents of a plane quartic curve.
|
|
|
|
Box32
|
Pair of 4×4 arrays representing orthogonal
Latin squares.
|
Used to represent
elements of AGL(4,2)
|
|
|
Box35
|
A 5-row-by-7-column array representing the 35
lines in the finite projective space PG(3,2)
|
PGL(3,2), order 20,160
|
|
|
Box36
|
Eurler's 36-officer problem.
|
|
|
|
Box45
|
The 45 Pascal points of the Pascal configuration.
|
|
|
|
Box48
|
The 48 elements of the group AGL(2,3).
|
AGL(2,3).
|
|
|
Box56
|
The 56 three-sets within an 8-set or
56 triangles in a model of Klein's quartic surface or
the 56 spreads in PG(3,2).
|
|
|
|
Box60
|
The Klein configuration.
|
|
|
|
Box64
|
Solomon's cube.
|
|
|
— Steven H. Cullinane, March 26-27, 2022
Comments Off on Box Geometry: Space, Group, Art (Work in Progress)
Tuesday, March 15, 2022
See other posts now so tagged.
Hudson's Rosenhain tetrads, as 20 of the 35 projective lines in PG(3,2),
illustrate Desargues's theorem as a symmetry within 10 pairs of squares
under rotation about their main diagonals:

See also "The Square Model of Fano's 1892 Finite 3-Space."
The remaining 15 lines of PG(3,2), Hudson's Göpel tetrads, have their
own symmetries . . . as the Cremona-Richmond configuration.
Comments Off on The Rosenhain Symmetry
Sunday, February 20, 2022
The geometry of the 4×4 square may be associated with the name
Galois, as in "the Galois tesseract," or similarly with the name Kummer.
Here is a Google image search using the latter name —
(Click to enlarge.)

Comments Off on 4×4 Nomenclature
Thursday, February 17, 2022
"FILE – Retired Sandinista Gen. Hugo Torres poses for portrait
at his home, in Managua, Nicaragua, May 2, 2018."
— Photo caption from a Feb. 12 Washington Post obituary
Also on May 2, 2018 —

Related theology —
Tuesday, February 23, 2016
Filed under: General — m759 @ 7:14 PM
From a New York Times obituary today —
"The Rev. Fernando Cardenal, a son of privilege
who embraced Latin America’s poor as a revolutionary
priest and brazenly defied Pope John Paul II’s order to
quit Nicaragua’s leftist cabinet in the 1980s, died on
Saturday in Managua. He was 82."
Photo caption from the same obituary —
"Fernando Cardenal in 1990. As education minister of
Nicaragua under the Sandinistas in the 1980s, he
oversaw a sweeping campaign credited with reducing
illiteracy to 13 percent from 51 percent."
This alleged literacy improvement makes him sound like
a Protestant revolutionary.
For a Catholic view of literacy, see The Gutenberg Galaxy .
See also the post Being Interpreted (Aug. 14, 2015) —

|
Comments Off on Space Memorial
Friday, December 31, 2021

Related art — The non-Rubik 3x3x3 cube —

The above structure illustrates the affine space of three dimensions
over the three-element finite (i.e., Galois) field, GF(3). Enthusiasts
of Judith Brown's nihilistic philosophy may note the "radiance" of the
13 axes of symmetry within the "central, structuring" subcube.
I prefer the radiance (in the sense of Aquinas) of the central, structuring
eightfold cube at the center of the affine space of six dimensions over
the two-element field GF(2).
Comments Off on Aesthetics in Academia
Friday, December 10, 2021
The time of the previous post was 4:46 AM ET today.
Fourteen minutes later —

"I'm a groupie, really." — Murray Bartlett in today's online NY Times
The previous post discussed group actions on a 3×3 square array. A tune
about related group actions on a 4×4 square array (a Galois tesseract ) . . .

Comments Off on Unhinged Melody
Tuesday, December 7, 2021
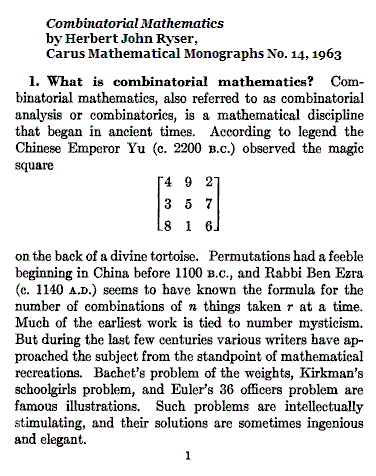
Fanciful version —

Less fanciful versions . . .
|
Unmagic Squares
Consecutive positive integers:
1 2 3
4 5 6
7 8 9
Consecutive nonnegative integers:
0 1 2
3 4 5
6 7 8
Consecutive nonnegative integers
written in base 3:
00 01 02
10 11 12
20 21 22
This last square may be viewed as
coordinates, in the 3-element Galois
field GF(3), of the ninefold square.
Note that the ninefold square so viewed
embodies the 12 lines of the two-dimensional
affine space over GF(3)…
As does, similarly, the ancient Chinese
"magic" square known as the "Lo Shu."
These squares are therefore equivalent under
affine transformations.
This method generalizes.
— Steven H. Cullinane, Nov. 20, 2021
|
.jpg)
Comments Off on Tortoise Variations
Sunday, December 5, 2021
Comments Off on The Venn Lotus
Saturday, November 20, 2021
|
Unmagic Squares
Consecutive positive integers:
1 2 3
4 5 6
7 8 9
Consecutive nonnegative integers:
0 1 2
3 4 5
6 7 8
Consecutive nonnegative integers
written in base 3:
00 01 02
10 11 12
20 21 22
This last square may be viewed as
coordinates, in the 3-element Galois
field GF(3), of the ninefold square.
Note that the ninefold square so viewed
embodies the 12 lines of the two-dimensional
affine space over GF(3)…
As does, similarly, the ancient Chinese
"magic" square known as the "Lo Shu."
These squares are therefore equivalent under
affine transformations.
This method generalizes.
— Steven H. Cullinane, Nov. 20, 2021
|
Comments Off on The Unmagicking
Monday, July 12, 2021
(Continued from St. Luke's Day, 2014)

Tablet:
.jpg)
Cube:

Comments Off on Educational Series
Saturday, May 8, 2021

The Greek capital letter Omega, Ω, is customarily
used to denote a set that is acted upon by a group.
If the group is the affine group of 322,560
transformations of the four-dimensional
affine space over the two-element Galois field,
the appropriate Ω is the 4×4 grid above.
See the Cullinane diamond theorem .
If the group is the large Mathieu group of
244,823,040 permutations of 24 things,
the appropriate Ω is the 4×6 grid below.

See the Miracle Octad Generator of R. T. Curtis.
Comments Off on A Tale of Two Omegas
Friday, February 12, 2021

A star figure and the Galois quaternion.
The square root of the former is the latter.
(This post was suggested by the definition of
the Dirac operator as a square root.)
Comments Off on Root
Thursday, December 17, 2020
Composer Harold Budd reportedly died at 84 on December 8
in Arcadia, California.
"The way I work is that
I focus entirely on a small thing
and try to milk that for all it's worth,
to find everything in it
that makes musical sense,"
Budd explained in a 1997 interview….
— Elegy for Budd at NPR
See related remarks in posts now tagged Quartet,
as well as posts now tagged Galois Window.
Comments Off on In Memoriam
Wednesday, December 16, 2020
Comments Off on Kramer’s Cross
Sunday, December 6, 2020
The title phrase is ambiguous and should be avoided.
It is used indiscriminately to denote any system of coordinates
written with 0 ‘s and 1 ‘s, whether these two symbols refer to
the Boolean-algebra truth values false and true , to the absence
or presence of elements in a subset , to the elements of the smallest
Galois field, GF(2) , or to the digits of a binary number .
Related material from the Web —

Some related remarks from “Geometry of the 4×4 Square:
Notes by Steven H. Cullinane” (webpage created March 18, 2004) —

A related anonymous change to Wikipedia today —

The deprecated “binary coordinates” phrase occurs in both
old and new versions of the “Square representation” section
on PG(3,2), but at least the misleading remark about Steiner
quadruple systems has been removed.
Comments Off on “Binary Coordinates”
Wednesday, November 11, 2020
The new domain qube.link forwards to . . .
http://finitegeometry.org/sc/64/solcube.html .
More generally, qubes.link forwards to this post,
which defines qubes .
Definition: A qube is a positive integer that is
a prime-power cube , i.e. a cube that is the order
of a Galois field. (Galois-field orders in general are
customarily denoted by the letter q .)
Examples: 8, 27, 64. See qubes.site.
Update on Nov. 18, 2020, at about 9:40 PM ET —
Problem:
For which qubes, visualized as n×n×n arrays,
is it it true that the actions of the two-dimensional
galois-geometry affine group on each n×n face, extended
throughout the whole array, generate the affine group
on the whole array? (For the cases 8 and 64, see Binary
Coordinate Systems and Affine Groups on Small
Binary Spaces.)
Comments Off on Qube
Monday, November 2, 2020
The new domain name q-bits.space does not refer to
the q in “quantum ,” but rather to the q that symbolizes
the order of a Galois field .
See the Wikipedia article “Finite field.”
The “space” suffix refers to a web page on geometry.
Comments Off on Q Bits
Tuesday, October 20, 2020

Click medal for some background. The medal may be regarded
as illustrating the 16-point Galois space.
Comments Off on The Leibniz Methods
Friday, September 11, 2020

Kauffman‘s fixation on the work of Spencer-Brown is perhaps in part
due to Kauffman’s familiarity with Boolean algebra and his ignorance of
Galois geometry. See other posts now tagged Boole vs. Galois.

See also “A Four-Color Epic” (April 16, 2020).
Comments Off on Kauffman on Algebra
Saturday, June 27, 2020



This is a continuation of the “just seventeen” posts.
Comments Off on Blank Memorial
Saturday, May 30, 2020

In other news . . .


Another red book for Stephanie —

Comments Off on MAA News
Tuesday, May 26, 2020

Or approaching.
On the Threshold:

Click the search result above for the July 1982 Omni
story that introduced into fiction the term "cyberspace."
Part of a page from the original Omni version —

For some other kinds of space, see my notes from the 1980's.
Some related remarks on space (and illustrated clams) —

— George Steiner, "A Death of Kings," The New Yorker ,
September 7, 1968, pp. 130 ff. The above is from p. 133.
See also Steiner on space, algebra, and Galois.
Comments Off on Introduction to Cyberspace
Wednesday, April 15, 2020
In memory of the author of My Time in Space * —
Tim Robinson, who reportedly died on April 3 —

See also an image from a Log24 post, Gray Space —

Related material from Robinson’s reported date of death —

* First edition, hardcover, Lilliput Press, Ireland, April 1, 2001.
Comments Off on Death Warmed Over
Saturday, April 4, 2020
Comments Off on A Schicksalstag for the Author of The Eight
Thursday, April 2, 2020
Saturday, March 7, 2020
From "Mathieu Moonshine and Symmetry Surfing" —
(Submitted on 29 Sep 2016, last revised 22 Jan 2018)
by Matthias R. Gaberdiel (1), Christoph A. Keller (2),
and Hynek Paul (1)
(1) Institute for Theoretical Physics, ETH Zurich
(2) Department of Mathematics, ETH Zurich
https://arxiv.org/abs/1609.09302v2 —
"This presentation of the symmetry groups Gi is
particularly well-adapted for the symmetry surfing
philosophy. In particular it is straightforward to
combine them into an overarching symmetry group G
by combining all the generators. The resulting group is
the so-called octad group
G = (Z2)4 ⋊ A8 .
It can be described as a maximal subgroup of M24
obtained by the setwise stabilizer of a particular
'reference octad' in the Golay code, which we take
to be O9 = {3,5,6,9,15,19,23,24} ∈ 𝒢24. The octad
subgroup is of order 322560, and its index in M24
is 759, which is precisely the number of
different reference octads one can choose."
This "octad group" is in fact the symmetry group of the affine 4-space over GF(2),
so described in 1979 in connection not with the Golay code but with the geometry
of the 4×4 square.* Its nature as an affine group acting on the Golay code was
known long before 1979, but its description as an affine group acting on
the 4×4 square may first have been published in connection with the
Cullinane diamond theorem and Abstract 79T-A37, "Symmetry invariance in a
diamond ring," by Steven H. Cullinane in Notices of the American Mathematical
Society , February 1979, pages A-193, 194.
* The Galois tesseract .
Update of March 15, 2020 —
Conway and Sloane on the "octad group" in 1993 —

Comments Off on The “Octad Group” as Symmetries of the 4×4 Square
Thursday, March 5, 2020
See the title in this journal.
Such generation occurs both in Euclidean space …

… and in some Galois spaces —
 .
.
In Galois spaces, some care must be taken in defining "reflection."
Comments Off on “Generated by Reflections”
Monday, February 24, 2020
“There is such a thing as ▦ .”
— Saying adapted from a 1962 young-adult novel.
Comments Off on Hidden Figure
Friday, February 21, 2020
Tuesday, January 28, 2020
Two of the thumbnail previews
from yesterday's 1 AM post …
"Hum a few bars"

"For 6 Prescott Street"

Further down in the "6 Prescott St." post, the link 5 Divinity Avenue
leads to …
|
A Letter from Timothy Leary, Ph.D., July 17, 1961
Harvard University
Department of Social Relations
Center for Research in Personality
Morton Prince House
5 Divinity Avenue
Cambridge 38, Massachusetts
July 17, 1961
Dr. Thomas S. Szasz
c/o Upstate Medical School
Irving Avenue
Syracuse 10, New York
Dear Dr. Szasz:
Your book arrived several days ago. I've spent eight hours on it and realize the task (and joy) of reading it has just begun.
The Myth of Mental Illness is the most important book in the history of psychiatry.
I know it is rash and premature to make this earlier judgment. I reserve the right later to revise and perhaps suggest it is the most important book published in the twentieth century.
It is great in so many ways–scholarship, clinical insight, political savvy, common sense, historical sweep, human concern– and most of all for its compassionate, shattering honesty.
. . . .
|
The small Morton Prince House in the above letter might, according to
the above-quoted remarks by Corinna S. Rohse, be called a "jewel box."
Harvard moved it in 1978 from Divinity Avenue to its current location at
6 Prescott Street.
Related "jewel box" material for those who
prefer narrative to mathematics —
"In The Electric Kool-Aid Acid Test , Tom Wolfe writes about encountering
'a young psychologist,' 'Clifton Fadiman’s nephew, it turned out,' in the
waiting room of the San Mateo County jail. Fadiman and his wife were
'happily stuffing three I-Ching coins into some interminable dense volume*
of Oriental mysticism' that they planned to give Ken Kesey, the Prankster-
in-Chief whom the FBI had just nabbed after eight months on the lam.
Wolfe had been granted an interview with Kesey, and they wanted him to
tell their friend about the hidden coins. During this difficult time, they
explained, Kesey needed oracular advice."
— Tim Doody in The Morning News web 'zine on July 26, 2012**
Oracular advice related to yesterday evening's
"jewel box" post …
A 4-dimensional hypercube H (a tesseract ) has 24 square
2-dimensional faces. In its incarnation as a Galois tesseract
(a 4×4 square array of points for which the appropriate transformations
are those of the affine 4-space over the finite (i.e., Galois) two-element
field GF(2)), the 24 faces transform into 140 4-point "facets." The Galois
version of H has a group of 322,560 automorphisms. Therefore, by the
orbit-stabilizer theorem, each of the 140 facets of the Galois version has
a stabilizer group of 2,304 affine transformations.
Similar remarks apply to the I Ching In its incarnation as
a Galois hexaract , for which the symmetry group — the group of
affine transformations of the 6-dimensional affine space over GF(2) —
has not 322,560 elements, but rather 1,290,157,424,640.
* The volume Wolfe mentions was, according to Fadiman, the I Ching.
** See also this journal on that date — July 26, 2012.
Comments Off on Very Stable Kool-Aid
Monday, January 27, 2020
The phrase "jewel box" in a New York Times obituary online this afternoon
suggests a review. See "And He Built a Crooked House" and Galois Tesseract.
Comments Off on Jewel Box
Monday, December 2, 2019

"What the piece of art is about is the gray space in the middle."
— David Bowie, as quoted in the above Crimson piece.
Bowie's "gray space" is the space between the art and the beholder.
I prefer the gray space in the following figure —

Context: The Trinity Stone (Log24, June 4, 2018).
Comments Off on Aesthetics at Harvard
Wednesday, September 18, 2019
Comments Off on Battle Song
Friday, August 16, 2019
(Continued)

A revision of the above diagram showing
the Galois-addition-table structure —

Related tables from August 10 —

See "Schoolgirl Space Revisited."
Comments Off on Nocciolo
Tuesday, August 13, 2019
The Matrix of Lévi-Strauss —
(From his “Structure and Form: Reflections on a Work by Vladimir Propp.”
Translated from a 1960 work in French. It appeared in English as
Chapter VIII of Structural Anthropology, Volume 2 (U. of Chicago Press, 1976).
Chapter VIII was originally published in Cahiers de l’Institut de Science
Économique Appliquée , No. 9 (Series M, No. 7) (Paris: ISEA, March 1960).)

The structure of the matrix of Lévi-Strauss —

Illustration from Diamond Theory , by Steven H. Cullinane (1976).
The relevant field of mathematics is not Boolean algebra, but rather
Galois geometry.
Comments Off on Putting the Structure in Structuralism
Sunday, July 7, 2019
Anonymous remarks on the schoolgirl problem at Wikipedia —
"This solution has a geometric interpretation in connection with
Galois geometry and PG(3,2). Take a tetrahedron and label its
vertices as 0001, 0010, 0100 and 1000. Label its six edge centers
as the XOR of the vertices of that edge. Label the four face centers
as the XOR of the three vertices of that face, and the body center
gets the label 1111. Then the 35 triads of the XOR solution correspond
exactly to the 35 lines of PG(3,2). Each day corresponds to a spread
and each week to a packing."
See also Polster + Tetrahedron in this journal.
There is a different "geometric interpretation in connection with
Galois geometry and PG(3,2)" that uses a square model rather
than a tetrahedral model. The square model of PG(3,2) last
appeared in the schoolgirl-problem article on Feb. 11, 2017, just
before a revision that removed it.
Comments Off on Schoolgirl Problem
Mind game on the birthday of Évariste Galois:

Comments Off on Battlefield Geometry
Tuesday, July 2, 2019


An illustration from the previous post may be interpreted
as an attempt to unbokeh an inscape —

The 15 lines above are Euclidean lines based on pairs within a six-set.
For examples of Galois lines so based, see Six-Set Geometry:

Comments Off on Depth Psychology Meets Inscape Geometry
Sunday, June 16, 2019
Comments Off on Master Plan from Outer Space
Thursday, March 21, 2019
Finite Galois geometry with the underlying field the simplest one possible —
namely, the two-element field GF(2) — is a geometry of interstices :

For some less precise remarks, see the tags Interstice and Interality.
The rationalist motto "sincerity, order, logic and clarity" was quoted
by Charles Jencks in the previous post.
This post was suggested by some remarks from Queensland that
seem to exemplify these qualities —

Comments Off on Geometry of Interstices
Monday, March 11, 2019



The 4×4 square may also be called the Galois Tesseract .
By analogy, the 4x4x4 cube may be called the Galois Hexeract .
"Think outside the tesseract."
Comments Off on Ant-Man Meets Doctor Strange
Thursday, February 28, 2019

Besides omitting the name Cullinane, the anonymous Wikipedia author
also omitted the step of representing the hypercube by a 4×4 array —
an array called in this journal a Galois tesseract.
Comments Off on Wikipedia Scholarship




The two books pictured above are From Discrete to Continuous ,
by Katherine Neal, and Geometrical Landscapes , by Amir Alexander.
Note: There is no Galois (i.e., finite) field with six elements, but
the theory of finite fields underlies applications of six-set geometry.
Comments Off on Fooling
Sunday, February 10, 2019

The previous post, on the Bauhaus 100th anniversary, suggests a review . . .
"Congratulations to the leaders of both parties:
The past 20 years you’ve taken us far.
We’re entering Weimar, baby."
— Peggy Noonan in The Wall Street Journal
on August 13, 2015

Image from yesterday's Log24 search Bauhaus Space.
Comments Off on Cold Open
Thursday, January 10, 2019

See also Spekkens in this journal.
Comments Off on Toy Story Continues.
Sunday, December 9, 2018
The previous post, on the 3×3 square in ancient China,
suggests a review of group actions on that square
that include the quaternion group.
Click to enlarge —

Three links from the above finitegeometry.org webpage on the
quaternion group —
Related material —

See as well the two Log24 posts of December 1st, 2018 —
Character and In Memoriam.
Comments Off on Quaternions in a Small Space
Sunday, December 2, 2018
A search this morning for articles mentioning the Miracle Octad Generator
of R. T. Curtis within the last year yielded an abstract for two talks given
at Hiroshima on March 8 and 9, 2018 —
http://www.math.sci.hiroshima-u.ac.jp/
branched/files/2018/abstract/Aitchison.txt
Iain AITCHISON
Title:
Construction of highly symmetric Riemann surfaces, related manifolds, and some exceptional objects, I, II
Abstract:
Since antiquity, some mathematical objects have played a special role, underpinning new mathematics as understanding deepened. Perhaps archetypal are the Platonic polyhedra, subsequently related to Platonic idealism, and the contentious notion of existence of mathematical reality independent of human consciousness.
Exceptional or unique objects are often associated with symmetry – manifest or hidden. In topology and geometry, we have natural base points for the moduli spaces of closed genus 2 and 3 surfaces (arising from the 2-fold branched cover of the sphere over the 6 vertices of the octahedron, and Klein's quartic curve, respectively), and Bring's genus 4 curve arises in Klein's description of the solution of polynomial equations of degree greater than 4, as well as in the construction of the Horrocks-Mumford bundle. Poincare's homology 3-sphere, and Kummer's surface in real dimension 4 also play special roles.
In other areas: we have the exceptional Lie algebras such as E8; the sporadic finite simple groups; the division algebras: Golay's binary and ternary codes; the Steiner triple systems S(5,6,12) and S(5,8,24); the Leech lattice; the outer automorphisms of the symmetric group S6; the triality map in dimension 8; and so on. We also note such as: the 27 lines on a cubic, the 28 bitangents of a quartic curve, the 120 tritangents of a sextic curve, and so on, related to Galois' exceptional finite groups PSL2(p) (for p= 5,7,11), and various other so-called `Arnol'd Trinities'.
Motivated originally by the `Eightfold Way' sculpture at MSRI in Berkeley, we discuss inter-relationships between a selection of these objects, illustrating connections arising via highly symmetric Riemann surface patterns. These are constructed starting with a labeled polygon and an involution on its label set.
Necessarily, in two lectures, we will neither delve deeply into, nor describe in full, contexts within which exceptional objects arise. We will, however, give sufficient definition and detail to illustrate essential inter-connectedness of those exceptional objects considered.
Our starting point will be simplistic, arising from ancient Greek ideas underlying atomism, and Plato's concepts of space. There will be some overlap with a previous talk on this material, but we will illustrate with some different examples, and from a different philosophical perspective.
Some new results arising from this work will also be given, such as an alternative graphic-illustrated MOG (Miracle Octad Generator) for the Steiner system S(5,8,24), and an alternative to Singerman – Jones' genus 70 Riemann surface previously proposed as a completion of an Arnol'd Trinity. Our alternative candidate also completes a Trinity whose two other elements are Thurston's highly symmetric 6- and 8-component links, the latter related by Thurston to Klein's quartic curve.
|
See also yesterday morning's post, "Character."
Update: For a followup, see the next Log24 post.
Comments Off on Symmetry at Hiroshima
Tuesday, November 20, 2018
(Continued)

Musical accompaniment from Sunday morning —

Update of Nov. 21 —
The reader may contrast the above Squarespace.com logo
(a rather serpentine version of the acronym SS) with a simpler logo
for a square space (the Galois window ):

Comments Off on Logos
Tuesday, October 23, 2018

Click the image for some context.
Comments Off on Plan 9 from Inner Space
Monday, October 15, 2018
The previous post, "Tesserae for a Tesseract," contains the following
passage from a 1987 review of a book about Finnegans Wake —
"Basically, Mr. Bishop sees the text from above
and as a whole — less as a sequential story than
as a box of pied type or tesserae for a mosaic,
materials for a pattern to be made."
A set of 16 of the Wechsler cubes below are tesserae that
may be used to make patterns in the Galois tesseract.

Another Bellevue story —

“History, Stephen said, is a nightmare
from which I am trying to awake.”
— James Joyce, Ulysses
Comments Off on History at Bellevue

The structure at top right is that of the
ROMA-ORAM-MARO-AMOR square
in the previous post.
* "Zingari shoolerim" is from
Finnegans Wake .
Comments Off on For Zingari Shoolerim*
Saturday, September 29, 2018
The title is from Warburg. The Zwischenraum lines and shaded "cuts"
below are to be added together in characteristic two, i.e., via the
set-theoretic symmetric difference operator.

Comments Off on “Ikonologie des Zwischenraums”
Friday, September 14, 2018


Underlying the I Ching structure is the finite affine space
of six dimensions over the Galois field with two elements.
In this field, "1 + 1 = 0," as noted here Wednesday.
See also other posts now tagged Interstice.

Comments Off on Denkraum
Monday, August 27, 2018
From …
Thinking in Four Dimensions
By Dusa McDuff
"I’ve got the rather foolhardy idea of trying to explain
to you the kind of mathematics I do, and the kind of
ideas that seem simple to me. For me, the search
for simplicity is almost synonymous with the search
for structure.
I’m a geometer and topologist, which means that
I study the structure of space …
. . . .
In each dimension there is a simplest space
called Euclidean space … "
— In Roman Kossak, ed.,
Simplicity: Ideals of Practice in Mathematics and the Arts
(Kindle Locations 705-710, 735). Kindle Edition.
For some much simpler spaces of various
dimensions, see Galois Space in this journal.


Comments Off on Geometry and Simplicity
Wednesday, June 27, 2018
A passage that may or may not have influenced Madeleine L'Engle's
writings about the tesseract :
|
From Mere Christianity , by C. S. Lewis (1952) —
"Book IV – Beyond Personality:
or First Steps in the Doctrine of the Trinity"
. . . .
I warned you that Theology is practical. The whole purpose for which we exist is to be thus taken into the life of God. Wrong ideas about what that life is, will make it harder. And now, for a few minutes, I must ask you to follow rather carefully.
You know that in space you can move in three ways—to left or right, backwards or forwards, up or down. Every direction is either one of these three or a compromise between them. They are called the three Dimensions. Now notice this. If you are using only one dimension, you could draw only a straight line. If you are using two, you could draw a figure: say, a square. And a square is made up of four straight lines. Now a step further. If you have three dimensions, you can then build what we call a solid body, say, a cube—a thing like a dice or a lump of sugar. And a cube is made up of six squares.
Do you see the point? A world of one dimension would be a straight line. In a two-dimensional world, you still get straight lines, but many lines make one figure. In a three-dimensional world, you still get figures but many figures make one solid body. In other words, as you advance to more real and more complicated levels, you do not leave behind you the things you found on the simpler levels: you still have them, but combined in new ways—in ways you could not imagine if you knew only the simpler levels.
Now the Christian account of God involves just the same principle. The human level is a simple and rather empty level. On the human level one person is one being, and any two persons are two separate beings—just as, in two dimensions (say on a flat sheet of paper) one square is one figure, and any two squares are two separate figures. On the Divine level you still find personalities; but up there you find them combined in new ways which we, who do not live on that level, cannot imagine.
In God's dimension, so to speak, you find a being who is three Persons while remaining one Being, just as a cube is six squares while remaining one cube. Of course we cannot fully conceive a Being like that: just as, if we were so made that we perceived only two dimensions in space we could never properly imagine a cube. But we can get a sort of faint notion of it. And when we do, we are then, for the first time in our lives, getting some positive idea, however faint, of something super-personal—something more than a person. It is something we could never have guessed, and yet, once we have been told, one almost feels one ought to have been able to guess it because it fits in so well with all the things we know already.
You may ask, "If we cannot imagine a three-personal Being, what is the good of talking about Him?" Well, there isn't any good talking about Him. The thing that matters is being actually drawn into that three-personal life, and that may begin any time —tonight, if you like.
. . . .
|
But beware of being drawn into the personal life of the Happy Family .
https://www.jstor.org/stable/24966339 —

"The colorful story of this undertaking begins with a bang."
And ends with …
Martin Gardner on Galois—
"Galois was a thoroughly obnoxious nerd,
suffering from what today would be called
a 'personality disorder.' His anger was
paranoid and unremitting."
Comments Off on Taken In
Monday, June 25, 2018
|
<title data-rh="true">Frank Heart, Who Linked Computers Before the Internet, Dies at 89 – The New York Times</title>
. . . .
<meta data-rh="true" name="description" itemprop="description" content="Mr. Heart’s team built the gateway device for the Arpanet, the precursor to the internet. Data networking was so new then, they made it up as they went."/>
. . . .
<meta data-rh="true" property="article:published" itemprop="datePublished" content="2018-06-25T19:16:17.000Z"/>
|
See also yesterday's "For 6/24" and …

Comments Off on The Gateway Device
Thursday, June 21, 2018
A Buddhist view —
“Just fancy a scale model of Being
made out of string and cardboard.”
— Nanavira Thera, 1 October 1957,
on a model of Kummer’s Quartic Surface
mentioned by Eddington
A Christian view —

A formal view —
From a Log24 search for High Concept:

See also Galois Tesseract.
Comments Off on Models of Being
Monday, June 11, 2018
The title was suggested by the name "ARTI" of an artificial
intelligence in the new film 2036: Origin Unknown.
The Eye of ARTI —

See also a post of May 19, "Uh-Oh" —

— and a post of June 6, "Geometry for Goyim" —

Mystery box merchandise from the 2011 J. J. Abrams film Super 8
An arty fact I prefer, suggested by the triangular computer-eye forms above —

This is from the July 29, 2012, post The Galois Tesseract.
See as well . . .

Comments Off on Arty Fact
Monday, June 4, 2018
“Unsheathe your dagger definitions.” — James Joyce, Ulysses
The “triple cross” link in the previous post referenced the eightfold cube
as a structure that might be called the trinity stone .



Comments Off on The Trinity Stone Defined
Sunday, May 20, 2018
From the date of the New York Times James Bond video
referenced in the previous post, "A Cryptic Message" —

Comments Off on Not So Cryptic
Friday, May 4, 2018

A star figure and the Galois quaternion.
The square root of the former is the latter.
See also a passage quoted here a year ago today
(May the Fourth, "Star Wars Day") —

Comments Off on Art & Design
Two excerpts from today's Art & Design section of
The New York Times —

For the deplorables of France —

For further remarks on l'ordre , see
other posts tagged Galois's Space
(… tag=galoiss-space).
* The radical of the title is Évariste Galois (1811-1832).
Comments Off on The Tuchman Radical*
Sunday, April 29, 2018
From the online New York Times this afternoon:
Disney now holds nine of the top 10
domestic openings of all time —
six of which are part of the Marvel
Cinematic Universe. “The result is
a reflection of 10 years of work:
of developing this universe, creating
stakes as big as they were, characters
that matter and stories and worlds that
people have come to love,” Dave Hollis,
Disney’s president of distribution, said
in a phone interview.
From this journal this morning:
"But she felt there must be more to this
than just the sensation of folding space
over on itself. Surely the Centaurs hadn't
spent ten years telling humanity how to
make a fancy amusement-park ride.
There had to be more—"
— Factoring Humanity , by Robert J. Sawyer,
Tom Doherty Associates, 2004 Orb edition,
page 168
"The sensation of folding space . . . ."
Or unfolding:

Click the above unfolded space for some background.
Comments Off on Amusement
Sunday, April 8, 2018
From a Log24 post of Feb. 5, 2009 —

An online logo today —

See also Harry Potter and the Lightning Bolt.
Comments Off on Design
Sunday, March 4, 2018
1955 ("Blackboard Jungle") —

1976 —

2009 —

2016 —

Comments Off on The Square Inch Space: A Brief History
Saturday, February 17, 2018
Michael Atiyah on the late Ron Shaw —

Phrases by Atiyah related to the importance in mathematics
of the two-element Galois field GF(2) —
- “The digital revolution based on the 2 symbols (0,1)”
- “The algebra of George Boole”
- “Binary codes”
- “Dirac’s spinors, with their up/down dichotomy”
These phrases are from the year-end review of Trinity College,
Cambridge, Trinity Annual Record 2017 .
I prefer other, purely geometric, reasons for the importance of GF(2) —
- The 2×2 square
- The 2x2x2 cube
- The 4×4 square
- The 4x4x4 cube
See Finite Geometry of the Square and Cube.
See also today’s earlier post God’s Dice and Atiyah on the theology of
(Boolean) algebra vs. (Galois) geometry:

Comments Off on The Binary Revolution
Friday, February 16, 2018
The Institute for Advanced Study (IAS) at Princeton in its Fall 2015 Letter
revived "Beautiful Mathematics" as a title:

This ugly phrase was earlier used by Truman State University
professor Martin Erickson as a book title. See below.
In the same IAS Fall 2015 Letter appear the following remarks
by Freeman Dyson —
". . . a special case of a much deeper connection that Ian Macdonald
discovered between two kinds of symmetry which we call modular and affine.
The two kinds of symmetry were originally found in separate parts of science,
modular in pure mathematics and affine in physics. Modular symmetry is
displayed for everyone to see in the drawings of flying angels and devils
by the artist Maurits Escher. Escher understood the mathematics and got the
details right. Affine symmetry is displayed in the peculiar groupings of particles
created by physicists with high-energy accelerators. The mathematician
Robert Langlands was the first to conjecture a connection between these and
other kinds of symmetry. . . ." (Wikipedia link added.)
The adjective "modular" might aptly be applied to . . .

The adjective "affine" might aptly be applied to . . .

The geometry of the 4×4 square combines modular symmetry
(i.e., related to theta functions) with the affine symmetry above.
Hudson's 1905 discussion of modular symmetry (that of Rosenhain
tetrads and Göpel tetrads) in the 4×4 square used a parametrization
of that square by the digit 0 and the fifteen 2-subsets of a 6-set, but
did not discuss the 4×4 square as an affine space.
For the connection of the 15 Kummer modular 2-subsets with the 16-
element affine space over the two-element Galois field GF(2), see my note
of May 26, 1986, "The 2-subsets of a 6-set are the points of a PG(3,2)" —

— and the affine structure in the 1979 AMS abstract
"Symmetry invariance in a diamond ring" —

For some historical background on the symmetry investigations by
Dyson and Macdonald, see Dyson's 1972 article "MIssed Opportunities."
For Macdonald's own use of the words "modular" and "affine," see
Macdonald, I. G., "Affine Lie algebras and modular forms,"
Séminaire N. Bourbaki , Vol. 23 (1980-1981), Talk no. 577, pp. 258-276.
Comments Off on Two Kinds of Symmetry
Thursday, January 25, 2018

"By an archetype I mean a systematic repertoire
of ideas by means of which a given thinker describes,
by analogical extension , some domain to which
those ideas do not immediately and literally apply."
— Max Black in Models and Metaphors
(Cornell, 1962, p. 241)
"Others … spoke of 'ultimate frames of reference' …."
— Ibid.
A "frame of reference" for the concept four quartets —

A less reputable analogical extension of the same
frame of reference —
Madeleine L'Engle in A Swiftly Tilting Planet :
"… deep in concentration, bent over the model
they were building of a tesseract:
the square squared, and squared again…."
See also the phrase Galois tesseract .
Comments Off on Beware of Analogical Extension
Saturday, January 20, 2018
In the following passage, Dan Brown claims that an eight-ray star
with arrowheads at the rays’ ends is “the mathematical symbol for
entropy.” Brown may have first encountered this symbol at a
questionable “Sacred Science” website. Wikipedia discusses
some even less respectable uses of the symbol.

Related news —

Related symbolism —

A star figure and the Galois quaternion.
The square root of the former is the latter.
Comments Off on The Chaos Symbol of Dan Brown
Friday, January 5, 2018
From "The Principle of Sufficient Reason," by George David Birkhoff,
in "Three Public Lectures on Scientific Subjects,"
delivered at the Rice Institute, March 6, 7, and 8, 1940 —

From the same lecture —
|
Up to the present point my aim has been to consider a variety of applications of the Principle of Sufficient Reason, without attempting any precise formulation of the Principle itself. With these applications in mind I will venture to formulate the Principle and a related Heuristic Conjecture in quasi-mathematical form as follows:
PRINCIPLE OF SUFFICIENT REASON. If there appears in any theory T a set of ambiguously determined ( i e . symmetrically entering) variables, then these variables can themselves be determined only to the extent allowed by the corresponding group G. Consequently any problem concerning these variables which has a uniquely determined solution, must itself be formulated so as to be unchanged by the operations of the group G ( i e . must involve the variables symmetrically).
HEURISTIC CONJECTURE. The final form of any scientific theory T is: (1) based on a few simple postulates; and (2) contains an extensive ambiguity, associated symmetry, and underlying group G, in such wise that, if the language and laws of the theory of groups be taken for granted, the whole theory T appears as nearly self-evident in virtue of the above Principle.
The Principle of Sufficient Reason and the Heuristic Conjecture, as just formulated, have the advantage of not involving excessively subjective ideas, while at the same time retaining the essential kernel of the matter.
In my opinion it is essentially this principle and this conjecture which are destined always to operate as the basic criteria for the scientist in extending our knowledge and understanding of the world.
It is also my belief that, in so far as there is anything definite in the realm of Metaphysics, it will consist in further applications of the same general type. This general conclusion may be given the following suggestive symbolic form:

While the skillful metaphysical use of the Principle must always be regarded as of dubious logical status, nevertheless I believe it will remain the most important weapon of the philosopher.
|
Related remarks by a founding member of the Metaphysical Club:

See also the previous post, "Seven Types of Interality."
Comments Off on Types of Ambiguity
Wednesday, December 27, 2017
See the 27-part structure of
the 3x3x3 Galois cube

as well as Autism Sunday 2015.
Comments Off on For Day 27 of December 2017
Tuesday, October 3, 2017
From Monday morning's post Advanced Study —
"Mathematical research currently relies on
a complex system of mutual trust
based on reputations."
— The late Vladimir Voevodsky,
Institute for Advanced Study, Princeton,
The Institute Letter , Summer 2014, p. 8
Related news from today's online New York Times —

A heading from the above screenshot: "SHOW US YOUR WALL."
This suggests a review of a concept from Galois geometry —

(On the wall — a Galois-geometry inscape .)
Comments Off on Show Us Your Wall
Friday, September 29, 2017
(Some Remarks for Science Addicts)
Principles —

Personalities —

* See "Tradition Twelve."
Comments Off on Principles Before Personalities*
Thursday, September 28, 2017
From the New York Times Wire last night —
"Mr. Hefner … styled himself as an emblem
of the sexual revolution."
From a Log24 post on September 23 —

A different emblem related to other remarks in the above Sept. 23 post —

(On the wall — a Galois-geometry inscape .)
Comments Off on Core
Saturday, September 23, 2017
Comments Off on The Turn of the Year
"With respect to the story's content, the frame thus acts
both as an inclusion of the exterior and as an exclusion
of the interior: it is a perturbation of the outside at the
very core of the story's inside, and as such, it is a blurring
of the very difference between inside and outside."
— Shoshana Felman on a Henry James story, p. 123 in
"Turning the Screw of Interpretation,"
Yale French Studies No. 55/56 (1977), pp. 94-207.
Published by Yale University Press.


See also the previous post and The Galois Tesseract.
Comments Off on The Turn of the Frame
Friday, September 15, 2017
Silas in "Equals" (2015) —
Ever since we were kids it's been drilled into us that …
Our purpose is to explore the universe, you know.
Outer space is where we'll find …
… the answers to why we're here and …
… and where we come from.
Related material —

See also Galois Space in this journal.
Comments Off on Space Art
Tuesday, September 5, 2017
"But unlike many who left the Communist Party, I turned left
rather than right, and returned—or rather turned for the first time—
to a critical examination of Marx's work. I found—and still find—
that his analysis of capitalism, which for me is the heart of his work,
provides the best starting point, the best critical tools, with which—
suitably developed—to understand contemporary capitalism.
I remind you that this year is also the sesquicentennial of the
Communist Manifesto , a document that still haunts the capitalist world."
— From "Autobiographical Reflections," a talk given on June 5, 1998, by
John Stachel at the Max Planck Institute for the History of Science in Berlin
on the occasion of a workshop honoring his 70th birthday,
"Space-Time, Quantum Entanglement and Critical Epistemology."
From a passage by Stachel quoted in the previous post —

From the source for Stachel's remarks on Weyl and coordinatization —

Note that Stachel distorted Weyl's text by replacing Weyl's word
"symbols" with the word "quantities." —

This replacement makes no sense if the coordinates in question
are drawn from a Galois field — a field not of quantities , but rather
of algebraic symbols .
"You've got to pick up every stitch… Must be the season of the witch."
— Donovan song at the end of Nicole Kidman's "To Die For"
Comments Off on Annals of Critical Epistemology
Or: Coordinatization for Physicists
This post was suggested by the link on the word "coordinatized"
in the previous post.


I regret that Weyl's term "coordinatization" perhaps has
too many syllables for the readers of recreational mathematics —
for example, of an article on 4×4 magic squares by Conway, Norton,
and Ryba to be published today by Princeton University Press.
Insight into the deeper properties of such squares unfortunately
requires both the ability to learn what a "Galois field" is and the
ability to comprehend seven-syllable words.
Comments Off on Florence 2001
Thursday, August 31, 2017
In a book to be published Sept. 5 by Princeton University Press,
John Conway, Simon Norton, and Alex Ryba present the following
result on order-four magic squares —

A monograph published in 1976, “Diamond Theory,” deals with
more general 4×4 squares containing entries from the Galois fields
GF(2), GF(4), or GF(16). These squares have remarkable, if not
“magic,” symmetry properties. See excerpts in a 1977 article.
See also Magic Square and Diamond Theorem in this journal.
Comments Off on A Conway-Norton-Ryba Theorem
Sunday, August 27, 2017
The “Black” of the title refers to the previous post.
For the “Well,” see Hexagram 48.

Related material —
The Galois Tesseract and, more generally, Binary Coordinate Systems.
Comments Off on Black Well
Saturday, August 26, 2017

Naive readers may suppose that this sort of thing is
related to what has been dubbed "geometric group theory."
It is not. See posts now tagged Aesthetic Distance.

Comments Off on Aesthetic Distance
Friday, August 11, 2017
A post suggested by the word tzimtzum (see Wednesday)
or tsimtsum (see this morning) —
Lifeboat from the Tsimtsum in Life of Pi —

Another sort of tsimtsum, contracting infinite space to a finite space —

Comments Off on Symmetry’s Lifeboat
Tuesday, July 11, 2017
The New York TImes reports this evening that
"Jon Underwood, Founder of Death Cafe Movement,"
died suddenly at 44 on June 27.
This journal on that date linked to a post titled "The Mystic Hexastigm."
A related remark on the complete 6-point from Sunday, April 28, 2013 —
(See, in Veblen and Young's 1910 Vol. I, exercise 11,
page 53: "A plane section of a 6-point in space can
be considered as 3 triangles perspective in pairs
from 3 collinear points with corresponding sides
meeting in 3 collinear points." This is the large
Desargues configuration. See Classical Geometry
in Light of Galois Geometry.)
This post was suggested, in part, by the philosophical ruminations
of Rosalind Krauss in her 2011 book Under Blue Cup . See
Sunday's post Perspective and Its Transections . (Any resemblance
to Freud's title Civilization and Its Discontents is purely coincidental.)
Comments Off on A Date at the Death Cafe
Sunday, July 9, 2017
The title phrase is from Rosalind Krauss (Under Blue Cup , 2011) —
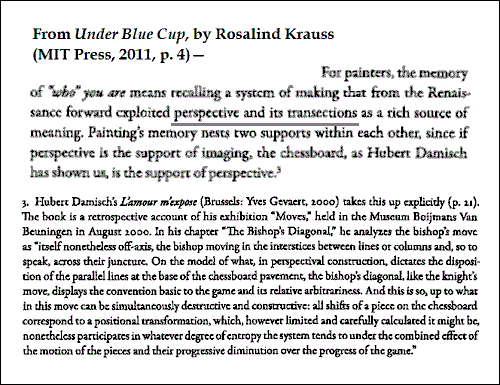
Another way of looking at the title phrase —
"A very important configuration is obtained by
taking the plane section of a complete space five-point."
(Veblen and Young, 1910, p. 39) —

For some context, see Desargues + Galois in this journal.
Comments Off on Perspective and Its Transections
Wednesday, July 5, 2017
The title refers to that of the previous post, "The Imaginarium."
In memory of a translator who reportedly died on May 22, 2017,
a passage quoted here on that date —

Related material — A paragraph added on March 15, 2017,
to the Wikipedia article on Galois geometry —
Comments Off on Imaginarium of a Different Kind
Saturday, June 3, 2017
Or: The Square

"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy

* See Expanding the Spielraum in this journal.
Comments Off on Expanding the Spielraum (Continued*)
Monday, May 29, 2017
Line from "Vide," a post of June 8, 2014 —
Vide Classical Geometry in Light of Galois Geometry.
Recall that vide means different things in Latin and in French.
See also Stevens + "Vacant Space" in this journal.
Comments Off on The American Sublime
Tuesday, May 23, 2017
Comments Off on Pursued by a Biplane
Saturday, May 20, 2017
From a review of the 2016 film "Arrival" —
"A seemingly off-hand reference to Abbott and Costello
is our gateway. In a movie as generally humorless as Arrival,
the jokes mean something. Ironically, it is Donnelly, not Banks,
who initiates the joke, naming the verbally inexpressive
Heptapod aliens after the loquacious Classical Hollywood
comedians. The squid-like aliens communicate via those beautiful,
cryptic images. Those signs, when thoroughly comprehended,
open the perceiver to a nonlinear conception of time; this is
Sapir-Whorf taken to the ludicrous extreme."
— Jordan Brower in the Los Angeles Review of Books
Further on in the review —
"Banks doesn’t fully understand the alien language, but she
knows it well enough to get by. This realization emerges
most evidently when Banks enters the alien ship and, floating
alongside Costello, converses with it in their picture-language.
She asks where Abbott is, and it responds — as presented
in subtitling — that Abbott 'is death process.'
'Death process' — dying — is not idiomatic English, and what
we see, written for us, is not a perfect translation but a
rendering of Banks’s understanding. This, it seems to me, is a
crucial moment marking the hard limit of a human mind,
working within the confines of human language to understand
an ultimately intractable xenolinguistic system."
For what may seem like an intractable xenolinguistic system to
those whose experience of mathematics is limited to portrayals
by Hollywood, see the previous post —
van Lint and Wilson Meet the Galois Tesseract.
The death process of van Lint occurred on Sept. 28, 2004.
See this journal on that date.
Comments Off on The Ludicrous Extreme
Tuesday, May 2, 2017
Comments Off on Image Albums
Saturday, April 29, 2017
A book cover from Amazon.com —

See also this journal on the above date, September 27, 2016 —
Chomsky and Levi-Strauss in China,
Or: Philosophy for Jews.
Some other remarks related to the figure on the book cover —
Field Theology and Galois Window.
* See Synchronology in this journal.
Comments Off on For the Church of Synchronology*
Friday, April 28, 2017
The title is from Don McLean's classic "American Pie."
A Finite Projective Space —

A Non-Finite Projective Space —

Comments Off on A Generation Lost in Space
Thursday, April 27, 2017

See also a figure from 2 AM ET April 26 …

" Partner, anchor, decompose. That's not math.
That's the plot to 'Silence of the Lambs.' "
— Greg Gutfeld, September 2014

Comments Off on Partner, Anchor, Decompose
Thursday, April 20, 2017

See also "Romancing the Omega" —

Related mathematics — Guitart in this journal —

See also Weyl + Palermo in this journal —

Comments Off on Stone Logic
Sunday, April 16, 2017

This post’s title is from the tags of the previous post —

The title’s “shift” is in the combined concepts of …
Space and Number
From Finite Jest (May 27, 2012):

The books pictured above are From Discrete to Continuous ,
by Katherine Neal, and Geometrical Landscapes , by Amir Alexander.
For some details of the shift, see a Log24 search for Boole vs. Galois.
From a post found in that search —
“Benedict Cumberbatch Says
a Journey From Fact to Faith
Is at the Heart of Doctor Strange“
— io9 , July 29, 2016
” ‘This man comes from a binary universe
where it’s all about logic,’ the actor told us
at San Diego Comic-Con . . . .
‘And there’s a lot of humor in the collision
between Easter [ sic ] mysticism and
Western scientific, sort of logical binary.’ “
[Typo now corrected, except in a comment.]
Comments Off on Art Space Paradigm Shift
Friday, April 14, 2017

The above four-element sets of black subsquares of a 4×4 square array
are 15 of the 60 Göpel tetrads , and 20 of the 80 Rosenhain tetrads , defined
by R. W. H. T. Hudson in his 1905 classic Kummer's Quartic Surface .
Hudson did not view these 35 tetrads as planes through the origin in a finite
affine 4-space (or, equivalently, as lines in the corresponding finite projective
3-space).
In order to view them in this way, one can view the tetrads as derived,
via the 15 two-element subsets of a six-element set, from the 16 elements
of the binary Galois affine space pictured above at top left.
This space is formed by taking symmetric-difference (Galois binary)
sums of the 15 two-element subsets, and identifying any resulting four-
element (or, summing three disjoint two-element subsets, six-element)
subsets with their complements. This process was described in my note
"The 2-subsets of a 6-set are the points of a PG(3,2)" of May 26, 1986.
The space was later described in the following —


Comments Off on Hudson and Finite Geometry
Monday, April 3, 2017
Tuesday, February 21, 2017
… Also known as quaternion —

"Diagram of an 8 leaf gathering: Quaternion (8 folio or leaf gathering).
A quaternion is composed of 4 bifolios. Conjugate folios form a bifolio
at either end of a gathering or quire. So in the diagram above folios
1 and 8 which form a bifolio are conjugate folios."
— http://employees.oneonta.edu/farberas/arth/arth214_folder/workshop.htm
The source:
SUNY Oneonta
ARTH 214
History of Northern Renaissance Art
Spring, 2013
Dr. Allen Farber, Associate Professor
Tuesday, February 26: From Workshop to Chamber:
The Paris Book Industry of the Early Fifteenth Century
"Images for class" folder
Synchronology:
An image from Publication, a Log24 post on the above date,
Feb. 26, 2013 —

Comments Off on Tetradion …
Sunday, February 19, 2017
Thursday, February 16, 2017
A Feb. 12 note in the "talk" section of the Wikipedia article
"Kirkman's schoolgirl problem" —

The illustration above was replaced by a new section in the article,
titled "Galois geometry."
The new section improves the article by giving it greater depth.
For related material, see Conwell Heptads in this journal
(or, more generally, Conwell) and a 1985 note citing Conwell's work.
Comments Off on Schoolgirls and Heptads
Sunday, February 12, 2017
Euclidean square and triangle—

Galois square and triangle—

For some backstory, see the "preface" of the
previous post and Soifer in this journal.
Comments Off on Religious Art for Sunday
Sunday, January 29, 2017
For some backstory, see Lottery in this journal,
esp. a post of June 28, 2007:
Real Numbers: An Object Lesson.
One such number, 8775, is suggested by
a Heinlein short story in a Jan. 25 post.
A search today for that number —

That Jan. 25 post, "For Your Consideration," also mentions logic.
Logic appears as well within a post from the above "8775" date,
August 16, 2016 —
|
Update of 10 am on August 16, 2016 —
See also Atiyah on the theology of
(Boolean) algebra vs. (Galois) geometry:

|
Related: Remarks by Charles Altieri on Wittgenstein in
today's previous post.
For remarks by Wittgenstein related to geometry and logic, see
(for instance) "Logical space" in "A Wittgenstein Dictionary," by
Hans-Johann Glock (Wiley-Blackwell, 1996).
Comments Off on Lottery Hermeneutics
Tuesday, January 3, 2017
The image of art historian Rosalind Krauss in the previous post
suggests a review of a page from her 1979 essay "Grids" —

The previous post illustrated a 3×3 grid. That cultist space does
provide a place for a few "vestiges of the nineteenth century" —
namely, the elements of the Galois field GF(9) — to hide.
See Coxeter's Aleph in this journal.
Comments Off on Cultist Space
Saturday, December 31, 2016

Breach's 1981 approach is not axiomatic,
but instead graphic. Another such approach —

Comments Off on Habeas
Thursday, December 1, 2016
Comments Off on What’s in a Name
Friday, November 25, 2016
Before the monograph "Diamond Theory" was distributed in 1976,
two (at least) notable figures were published that illustrate
symmetry properties of the 4×4 square:
Hudson in 1905 —

Golomb in 1967 —

It is also likely that some figures illustrating Walsh functions as
two-color square arrays were published prior to 1976.
Update of Dec. 7, 2016 —
The earlier 1950's diagrams of Veitch and Karnaugh used the
1's and 0's of Boole, not those of Galois.
Comments Off on Priority
Wednesday, October 5, 2016
From a Google image search yesterday —

Sources (left to right, top to bottom) —
Math Guy (July 16, 2014)
The Galois Tesseract (Sept. 1, 2011)
The Full Force of Roman Law (April 21, 2014)
A Great Moonshine (Sept. 25, 2015)
A Point of Identity (August 8, 2016)
Pascal via Curtis (April 6, 2013)
Correspondences (August 6, 2011)
Symmetric Generation (Sept. 21, 2011)
Comments Off on Sources
Saturday, September 24, 2016
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
Some Galois geometry —

See the previous post for more narrative.
Comments Off on The Seven Seals
« Newer Posts —
Older Posts »

























.jpg)