From a review on Schicksalstag (Nov. 9), 2019, of Jon Fosse's
The Other Name: Septology I-II —
https://www.theguardian.com/books/2019/nov/09/
the-other-name-septology-i-ii-jon-fosse-review —
Related art —
Wednesday, September 15, 2021
Septology According to Peirce
Comments Off on Septology According to Peirce
Tuesday, September 14, 2021
Bullshit Studies: Plato vs. Peirce, or Cornerstone vs. Keystone
From a post of August 13, "The Divided Square" —
From a post of April 25, 2016, "Peirce's Accounts of the Universe" —
For the Peirce arrangement, see the imaginary film
reviewed in last night's post Packend! .
Comments Off on Bullshit Studies: Plato vs. Peirce, or Cornerstone vs. Keystone
Monday, April 25, 2016
Peirce’s Accounts of the Universe
Compare and contrast Peirce's seven systems of metaphysics with
the seven projective points in a post of March 1, 2010 —
From my commentary on Carter's question —
Comments Off on Peirce’s Accounts of the Universe
Thursday, February 16, 2023
Reaching the Stillness
See http://m759.net/wordpress/?s="Mac+Lane"+Boolean.
An image from that search —
.
A related image
for T. S. Eliot:
Comments Off on Reaching the Stillness
Tuesday, December 18, 2018
Shadowhunter Tales
The recent post "Tales from Story Space," about the 18th birthday
of the protagonist in the TV series "Shadowhunters" (2016-),
suggests a review of the actual 18th birthday of actress Lily Collins.
Collins is shown below warding off evil with a magical rune as
a shadowhunter in the 2013 film "City of Bones" —
She turned 18 on March 18, 2007. A paper on symmetry and logic
referenced here on that date displays the following "runes" of
philosopher Charles Sanders Peirce —
See also Adamantine Meditation (Log24, Oct. 3, 2018)
and the webpage Geometry of the I Ching.
Comments Off on Shadowhunter Tales
Friday, January 5, 2018
Types of Ambiguity
From "The Principle of Sufficient Reason," by George David Birkhoff,
in "Three Public Lectures on Scientific Subjects,"
delivered at the Rice Institute, March 6, 7, and 8, 1940 —
From the same lecture —
|
Up to the present point my aim has been to consider a variety of applications of the Principle of Sufficient Reason, without attempting any precise formulation of the Principle itself. With these applications in mind I will venture to formulate the Principle and a related Heuristic Conjecture in quasi-mathematical form as follows: PRINCIPLE OF SUFFICIENT REASON. If there appears in any theory T a set of ambiguously determined ( i e . symmetrically entering) variables, then these variables can themselves be determined only to the extent allowed by the corresponding group G. Consequently any problem concerning these variables which has a uniquely determined solution, must itself be formulated so as to be unchanged by the operations of the group G ( i e . must involve the variables symmetrically). HEURISTIC CONJECTURE. The final form of any scientific theory T is: (1) based on a few simple postulates; and (2) contains an extensive ambiguity, associated symmetry, and underlying group G, in such wise that, if the language and laws of the theory of groups be taken for granted, the whole theory T appears as nearly self-evident in virtue of the above Principle. The Principle of Sufficient Reason and the Heuristic Conjecture, as just formulated, have the advantage of not involving excessively subjective ideas, while at the same time retaining the essential kernel of the matter. In my opinion it is essentially this principle and this conjecture which are destined always to operate as the basic criteria for the scientist in extending our knowledge and understanding of the world. It is also my belief that, in so far as there is anything definite in the realm of Metaphysics, it will consist in further applications of the same general type. This general conclusion may be given the following suggestive symbolic form:
While the skillful metaphysical use of the Principle must always be regarded as of dubious logical status, nevertheless I believe it will remain the most important weapon of the philosopher. |
Related remarks by a founding member of the Metaphysical Club:
See also the previous post, "Seven Types of Interality."
Comments Off on Types of Ambiguity
Monday, March 13, 2017
Pragmatism at the Church of the Transformers*
"I would drop the keystone into my arch . . . ."
Click the Auto Body image for some backstory.
* For the church, see Transformers in this journal.
Comments Off on Pragmatism at the Church of the Transformers*
Sunday, November 27, 2016
A Machine That Will Fit
Or: Notes for the Metaphysical Club
Northrop Frye on Wallace Stevens:
"He… stands in contrast to the the dualistic
approach of Eliot, who so often speaks of poetry
as though it were an emotional and sensational
soul looking for a 'correlative' skeleton of
thought to be provided by a philosopher, a
Cartesian ghost trying to find a machine that
will fit."
Ralph Waldo Emerson on "vacant and vain" knowledge:
"The new position of the advancing man has all
the powers of the old, yet has them all new. It
carries in its bosom all the energies of the past,
yet is itself an exhalation of the morning. I cast
away in this new moment all my once hoarded
knowledge, as vacant and vain."
Harold Bloom on Emerson:
"Emerson may not have invented the American
Sublime, yet he took eternal possession of it."
Wallace Stevens on the American Sublime:
"And the sublime comes down
To the spirit itself,
The spirit and space,
The empty spirit
In vacant space."
A founding member of the Metaphysical Club:
See also the eightfold cube.
Comments Off on A Machine That Will Fit
Sunday, September 25, 2016
Introduction to Pragmatism
Stanford Encyclopedia of Philosophy
on the origins of Pragmatism:
"Pragmatism had been born in the discussions at
a ‘metaphysical club’ in Harvard around 1870
(see Menand…*). Peirce and James participated
in these discussions along with some other philosophers
and philosophically inclined lawyers. As we have
already noted, Peirce developed these ideas in his
publications from the 1870s."
|
From "How to Make Our Ideas Clear," "The very first lesson that we have a right to demand that logic shall teach us is, how to make our ideas clear; and a most important one it is, depreciated only by minds who stand in need of it. To know what we think, to be masters of our own meaning, will make a solid foundation for great and weighty thought. It is most easily learned by those whose ideas are meagre and restricted; and far happier they than such as wallow helplessly in a rich mud of conceptions. A nation, it is true, may, in the course of generations, overcome the disadvantage of an excessive wealth of language and its natural concomitant, a vast, unfathomable deep of ideas. We may see it in history, slowly perfecting its literary forms, sloughing at length its metaphysics, and, by virtue of the untirable patience which is often a compensation, attaining great excellence in every branch of mental acquirement. The page of history is not yet unrolled which is to tell us whether such a people will or will not in the long-run prevail over one whose ideas (like the words of their language) are few, but which possesses a wonderful mastery over those which it has. For an individual, however, there can be no question that a few clear ideas are worth more than many confused ones. A young man would hardly be persuaded to sacrifice the greater part of his thoughts to save the rest; and the muddled head is the least apt to see the necessity of such a sacrifice. Him we can usually only commiserate, as a person with a congenital defect. Time will help him, but intellectual maturity with regard to clearness comes rather late, an unfortunate arrangement of Nature, inasmuch as clearness is of less use to a man settled in life, whose errors have in great measure had their effect, than it would be to one whose path lies before him. It is terrible to see how a single unclear idea, a single formula without meaning, lurking in a young man's head, will sometimes act like an obstruction of inert matter in an artery, hindering the nutrition of the brain, and condemning its victim to pine away in the fullness of his intellectual vigor and in the midst of intellectual plenty. Many a man has cherished for years as his hobby some vague shadow of an idea, too meaningless to be positively false; he has, nevertheless, passionately loved it, has made it his companion by day and by night, and has given to it his strength and his life, leaving all other occupations for its sake, and in short has lived with it and for it, until it has become, as it were, flesh of his flesh and bone of his bone; and then he has waked up some bright morning to find it gone, clean vanished away like the beautiful Melusina of the fable, and the essence of his life gone with it. I have myself known such a man; and who can tell how many histories of circle-squarers, metaphysicians, astrologers, and what not, may not be told in the old German story?" |
Peirce himself may or may not have been entirely successful
in making his ideas clear. See Where Credit Is Due (Log24,
June 11, 2016) and the Wikipedia article Categories (Peirce).
* Menand, L., 2001. The Metaphysical Club : A Story of
Ideas in America , New York: Farrar, Straus and Giroux
Comments Off on Introduction to Pragmatism
Friday, September 9, 2016
Ein Kampf
(Continued )
A 1984 master's thesis (PDF, 8+ MB) —
"Language, Linguistics, and Philosophy:
A Comparison of the Work of Roman Jakobson
and the Later Wittgenstein, with Some Attention
to the Philosophy of Charles Saunders Peirce,"
by Miles Spencer Kimball.
Two pages from that thesis —
Comments Off on Ein Kampf
Saturday, June 11, 2016
Where Credit Is Due
"White is credited with broadening the scope of
topics traditionally studied by philosophers…."
Comments Off on Where Credit Is Due
Tuesday, April 26, 2016
Interacting
"… I would drop the keystone into my arch …."
— Charles Sanders Peirce, "On Phenomenology"
" 'But which is the stone that supports the bridge?' Kublai Khan asks."
— Italo Calvino, Invisible Cities, as quoted by B. Elan Dresher.
(B. Elan Dresher. Nordlyd 41.2 (2014): 165-181,
special issue on Features edited by Martin Krämer,
Sandra Ronai and Peter Svenonius. University of Tromsø –
The Arctic University of Norway.
http://septentrio.uit.no/index.php/nordlyd)
Peter Svenonius and Martin Krämer, introduction to the
Nordlyd double issue on Features —
"Interacting with these questions about the 'geometric'
relations among features is the algebraic structure
of the features."
For another such interaction, see the previous post.
This post may be viewed as a commentary on a remark in Wikipedia —
"All of these ideas speak to the crux of Plato's Problem…."
See also The Diamond Theorem at Tromsø and Mere Geometry.
Comments Off on Interacting
Monday, April 25, 2016
Seven Seals
An old version of the Wikipedia article "Group theory"
(pictured in the previous post) —
"More poetically …"
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
The seven seals from the previous post, with some context —
These models of projective points are drawn from the underlying
structure described (in the 4×4 case) as part of the proof of the
Cullinane diamond theorem .
Comments Off on Seven Seals
Friday, March 28, 2014
Musement
(The title is from a work by Charles Sanders Peirce.)
For LYNX 760 —
For more beauty and strangeness, see Strange McEntire.
Comments Off on Musement
Monday, March 3, 2014
Monday, August 19, 2013
Midnight in the Garden
From a 2003 interview by Paul Devlin (PD) with poet John Hollander (JH),
who reportedly died Saturday—
|
PD: You wrote in the introduction to the new edition of Reflections on Espionage that whenever you have been "free of political callowness" it was partly as a result of reading W.H. Auden, George Orwell, and George Bernard Shaw. Do you think these writers might possibly be an antidote to political callowness that exists in much contemporary literary criticism? JH: If not they, then some other writers who can help one develop within one a skepticism strongly intertwined with passion, so that each can simultaneously check and reinforce the other. It provides great protection from being overcome by blind, true-believing zeal and corrupting cynicism (which may be two sides of the same false coin). Shaw was a great teacher for many in my generation. I started reading him when I was in sixth grade, and I responded strongly not only to the wit but to various modes, scene and occasions of argument and debate as they were framed by various kinds of dramatic situation. I remember being electrified when quite young by the moment in the epilogue scene of Saint Joan when the English chaplain, De Stogumber, who had been so zealous in urging for Joan’s being burned at the stake, returns to testify about how seeing her suffering the flames had made a changed man of him. The Inquisitor, Peter Cauchon, calls out (with what I imagined was a kind of moral distaste I’d never been aware of before), "Must then a Christ perish in torment in every age to save those who have no imagination?" It introduced me to a skepticism about the self-satisfaction of the born-again, of any persuasion. With Auden and Orwell, much later on and after my mental world had become more complicated, it was education in negotiating a living way between a destructively naïve idealism and the crackpot realism—equally inimical to the pragmatic. PD: Would you consider yourself a "formal" pragmatist, i.e., a student of Peirce, James, Dewey, Mead (etc.) or an "informal" pragmatist – someone taking the common-sense position on events…or someone who refuses to be pigeon-holed politically? JH: "Informal" – of the sort that often leads me to ask of theoretical formulations, "Yes, but what’s it for ?" PD: Which other authors do you think might help us negotiate between "naïve idealism" and "crackpot realism"? I think of Joyce, Wallace Stevens, perhaps Faulkner? JH: When I was in college, a strong teacher for just this question was Cervantes. One feels, in an Emersonian way, that the Don’s view of the world is correct at midnight, and Sancho’s at noon. |
Then there is mathematical realism.
A post in this journal on Saturday, the reported date of Hollander's death,
discussed a possible 21st-century application of 19th-century geometry.
For some background, see Peter J. Cameron's May 11, 2010, remarks
on Sylvester's duads and synthemes . The following figure from the
paper discussed here Saturday is related to figures in Cameron's remarks.
Comments Off on Midnight in the Garden
Wednesday, November 4, 2009
Sinner or Saint?
As noted here yesterday, Claude Levi-Strauss may have died on Devil's Night, on Halloween, or on All Saints' Day. He was apparently a myth-transformer to the end.
The Independent says today he died on Sunday, All Saints' Day. Its eulogy, by Adam Kuper, is well-written, noting that linguist Roman Jakobson was a source of Levi-Strauss's theory of oppositions in myth, and observing that
"… binary oppositions tend to accumulate to form structures…."
Yes, they do. Examples:
I. The structures in the Diamond Puzzle…
Click on image for Jungian background.
II: The structure on a recent cover of Semiotica…
The Semiotica article by mathematical linguist Solomon Marcus is a defense of the Levi-Strauss canonic formula mentioned here yesterday.
It is available online for $40.
A less expensive, and possibly more informative, look at oppositions in linguistics is available for free online in a 1984 master's thesis (pdf, 8+ mb)–
"Language, Linguistics, and Philosophy: A Comparison of the Work of Roman Jakobson and the Later Wittgenstein, with Some Attention to the Philosophy of Charles Saunders Peirce," by Miles Spencer Kimball.
Comments Off on Sinner or Saint?
Sunday, May 25, 2008
Sunday May 25, 2008
Hall of Mirrors
Epigraph to
“Deploying the Glass Bead Game, Part II,”
by Robert de Marrais:
“For a complete logical argument,”
Arthur began
with admirable solemnity,
“we need two prim Misses –”
“Of course!” she interrupted.
“I remember that word now.
And they produce — ?”
“A Delusion,” said Arthur.
— Lewis Carroll,
Sylvie and Bruno
Prim Miss 1:
Erin O’Connor’s weblog “Critical Mass” on May 24:
Erin O’Connor’s weblog “Critical Mass” on May 24:
Roger Rosenblatt’s Beet [Ecco hardcover, Jan. 29, 2008] is the latest addition to the noble sub-genre of campus fiction….
Curricular questions and the behavior of committees are at once dry as dust subjects and areas ripe for sarcastic send-up– not least because, as dull as they are, they are really both quite vital to the credibility and viability of higher education.
Here’s an excerpt from the first meeting, in which committee members propose their personal plans for a new, improved curriculum:
“… Once the students really got into playing with toy soldiers, they would understand history with hands-on excitement.”
To demonstrate his idea, he’d brought along a shoe box full of toy doughboys and grenadiers, and was about to reenact the Battle of Verdun on the committee table when Heilbrun stayed his hand. “We get it,” he said.
“That’s quite interesting, Molton,” said Booth [a chemist]. “But is it rigorous enough?”
At the mention of the word, everyone, save Peace, sat up straight.
“Rigor is so important,” said Kettlegorf.
“We must have rigor,” said Booth.
“You may be sure,” said the offended Kramer. “I never would propose anything lacking rigor.”
Smythe inhaled and looked at the ceiling. “I think I may have something of interest,” he said, as if he were at a poker game and was about to disclose a royal flush. “My proposal is called ‘Icons of Taste.’ It would consist of a galaxy of courses affixed to several departments consisting of lectures on examples of music, art, architecture, literature, and other cultural areas a student needed to indicate that he or she was sophisticated.”
“Why would a student want to do that?” asked Booth.
“Perhaps sophistication is not a problem for chemists,” said Smythe. Lipman tittered.
“What’s the subject matter?” asked Heilbrun. “Would it have rigor?”
“Of course it would have rigor. Yet it would also attract those additional students Bollovate is talking about.” Smythe inhaled again. “The material would be carefully selected,” he said. “One would need to pick out cultural icons the students were likely to bring up in conversation for the rest of their lives, so that when they spoke, others would recognize their taste as being exquisite yet eclectic and unpredictable.”
“You mean Rembrandt?” said Kramer.
Smythe smiled with weary contempt. “No, I do not mean Rembrandt. I don’t mean Beethoven or Shakespeare, either, unless something iconic has emerged about them to justify their more general appeal.”
“You mean, if they appeared on posters,” said Lipman.
“That’s it, precisely.”
Lipman blushed with pride.
“The subject matter would be fairly easy to amass,” Smythe said. “We could all make up a list off the top of our heads. Einstein–who does have a poster.” He nodded to the ecstatic Lipman. “Auden, for the same reason. Students would need to be able to quote ‘September 1939[ or at least the last lines. And it would be good to teach ‘Musee des Beaux Arts’ as well, which is off the beaten path, but not garishly. Mahler certainly. But Cole Porter too. And Sondheim, I think. Goya. Warhol, it goes without saying, Stephen Hawking, Kurosawa, Bergman, Bette Davis. They’d have to come up with some lines from Dark Victory, or better still, Jezebel. La Dolce Vita. Casablanca. King of Hearts. And Orson, naturally. Citizen Kane, I suppose, though personally I prefer F for Fake.”
“Judy!” cried Heilbrun.
“Yes, Judy too. But not ‘Over the Rainbow.’ It would be more impressive for them to do ‘The Trolley Song,’ don’t you think?” Kettlegorf hummed the intro.
“Guernica,” said Kramer. “Robert
Capa.” “Edward R. Murrow,” said Lipman.
“No! Don’t be ridiculous!” said Smythe, ending Lipman’s brief foray into the world of respectable thought.
“Marilyn Monroe!” said Kettlegorf.
“Absolutely!” said Smythe, clapping to indicate his approval.
“And the Brooklyn Bridge,” said Booth, catching on. “And the Chrysler Building.”
“Maybe,” said Smythe. “But I wonder if the Chrysler Building isn’t becoming something of a cliche.”
Peace had had enough. “And you want students to nail this stuff so they’ll do well at cocktail parties?”
Smythe sniffed criticism, always a tetchy moment for him. “You make it sound so superficial,” he said.
Prim Miss 2:
Siri Hustvedt speaks at Adelaide Writers’ Week– a story dated March 24,
“I have come to think of my books as echo chambers or halls of mirrors in which themes, ideas, associations continually reflect and reverberate inside a text. There is always point and counterpoint, to use a musical illustration. There is always repetition with difference.”
A Delusion:
Exercise — Identify in the following article the sentence that one might (by unfairly taking it out of context) argue is a delusion.
(Hint: See Reflection Groups in Finite Geometry.)
Why Borovik’s Figure 4
is included above:
Euclid, Peirce, L’Engle:
No Royal Roads.
And now, perhaps, his brother Cornell Capa, who died Friday.
† Related material: Log24 on March 24– Death and the Apple Tree— with an excerpt from George MacDonald, and an essay by David L. Neuhouser mentioning the influence of MacDonald on Lewis Carroll– Lewis Carroll: Author, Mathematician, and Christian (pdf).
No Royal Roads.
For more on Prim Miss 2
and deploying
the Glass Bead Game,
see the previous entry.
And now, perhaps, his brother Cornell Capa, who died Friday.† Related material: Log24 on March 24– Death and the Apple Tree— with an excerpt from George MacDonald, and an essay by David L. Neuhouser mentioning the influence of MacDonald on Lewis Carroll– Lewis Carroll: Author, Mathematician, and Christian (pdf).
Comments Off on Sunday May 25, 2008
Saturday, July 21, 2007
Saturday July 21, 2007
Death of a Nominalist
“All our words from loose using have lost their edge.” –Ernest Hemingway
(The Hemingway quotation is from the AP’s “Today in History” on July 21, 2007; for the context, see Death in the Afternoon.)
Today seems as good a day as any for noting the death of an author previously discussed in Log24 on January 29, 2007, and January 31, 2007.
Joseph Goguen died on July 3, 2006. (I learned of his death only after the entries of January 2007 were written. They still hold.)
Goguen’s death may be viewed in the context of the ongoing war between the realism of Plato and the nominalism of the sophists. (See, for instance, Log24 on August 10-15, 2004, and on July 3-5, 2007.)
Joseph A. Goguen, “Ontology, Society, and Ontotheology” (pdf):
“Before introducing algebraic semiotics and structural blending, it is good to be clear about their philosophical orientation. The reason for taking special care with this is that, in Western culture, mathematical formalisms are often given a status beyond what they deserve. For example, Euclid wrote, ‘The laws of nature are but the mathematical thoughts of God.’ Similarly, the ‘situations’ in the situation semantics of Barwise and Perry, which resemble conceptual spaces (but are more sophisticated– perhaps too sophisticated), are considered to be actually existing, real entities [23], even though they may include what are normally considered judgements.5 The classical semiotics of Charles Sanders Peirce [24] also tends towards a Platonist view of signs. The viewpoint of this paper is that all formalisms are constructed in the course of some task, such as scientific study or engineering design, for the heuristic purpose of facilitating consideration of certain issues in that task. Under this view, all theories are situated social entities, mathematical theories no less than others; of course, this does not mean that they are not useful.”
5 The “types” of situation theory are even further removed from concrete reality.
[23] Jon Barwise and John Perry. Situations and Attitudes. MIT (Bradford), 1983.
[24] Charles Sanders Peirce. Collected Papers. Harvard, 1965. In 6 volumes; see especially Volume 2: Elements of Logic.
From Log24 on the date of Goguen’s death:
Requiem for a clown:
“At times, bullshit can only be
countered with superior bullshit.”
This same Mailer aphorism was quoted, along with an excerpt from the Goguen passage above, in Log24 this year on the date of Norman Mailer’s birth. Also quoted on that date:
Sophia. Then these thoughts of Nature are also thoughts of God.
Alfred. Undoubtedly so, but however valuable the expression may be, I would rather that we should not make use of it till we are convinced that our investigation leads to a view of Nature, which is also the contemplation of God. We shall then feel justified by a different and more perfect knowledge to call the thoughts of Nature those of God….
Whether the above excerpt– from Hans Christian Oersted‘s The Soul in Nature (1852)– is superior to the similar remark of Goguen, the reader may decide.
Comments Off on Saturday July 21, 2007
Wednesday, May 23, 2007
Wednesday May 23, 2007
Strong Emergence Illustrated:
The Beauty Test
"There is no royal road
to geometry"
to geometry"
— Attributed to Euclid
There are, however, various non-royal roads. One of these is indicated by yesterday's Pennsylvania lottery numbers:
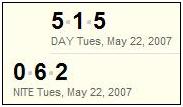
The mid-day number 515 may be taken as a reference to 5/15. (See the previous entry, "Angel in the Details," and 5/15.)
The evening number 062, in the context of Monday's entry "No Royal Roads" and yesterday's "Jewel in the Crown," may be regarded as naming a non-royal road to geometry: either U. S. 62, a major route from Mexico to Canada (home of the late geometer H.S.M. Coxeter), or a road less traveled– namely, page 62 in Coxeter's classic Introduction to Geometry (2nd ed.):
The illustration (and definition) is
of regular tessellations of the plane.
of regular tessellations of the plane.
This topic Coxeter offers as an
illustration of remarks by G. H. Hardy
that he quotes on the preceding page:
One might argue that such beauty is strongly emergent because of the "harmonious way" the parts fit together: the regularity (or fitting together) of the whole is not reducible to the regularity of the parts. (Regular triangles, squares, and hexagons fit together, but regular pentagons do not.)
The symmetries of these regular tessellations of the plane are less well suited as illustrations of emergence, since they are tied rather closely to symmetries of the component parts.
But the symmetries of regular tessellations of the sphere— i.e., of the five Platonic solids– do emerge strongly, being apparently independent of symmetries of the component parts.
Another example of strong emergence: a group of 322,560 transformations acting naturally on the 4×4 square grid— a much larger group than the group of 8 symmetries of each component (square) part.
The lottery numbers above also supply an example of strong emergence– one that nicely illustrates how it can be, in the words of Mark Bedau, "uncomfortably like magic."
(Those more comfortable with magic may note the resemblance of the central part of Coxeter's illustration to a magical counterpart– the Ojo de Dios of Mexico's Sierra Madre.)
Comments Off on Wednesday May 23, 2007
Monday, May 21, 2007
Monday May 21, 2007
No Royal Roads
A more recent royal reference:
"'Yau wants to be the king of geometry,' Michael Anderson, a geometer at Stony Brook, said. 'He believes that everything should issue from him, that he should have oversight. He doesn't like people encroaching on his territory.'" –Sylvia Nasar and David Gruber in The New Yorker, issue dated Aug. 28, 2006
Wikipedia, Cultural references to the Royal Road:
"Euclid is said to have replied to King Ptolemy's request for an easier way of learning mathematics that 'there is no royal road to geometry.' Charles S. Peirce, in his 'How to Make Our Ideas Clear' (1878), says 'There is no royal road to logic, and really valuable ideas can only be had at the price of close attention.'"
Related material:
There may be
no royal roads to
geometry or logic,
but…
Day Without Logic
(March 8, 2007)
and
The Geometry of Logic
(March 10, 2007):
There may be
no royal roads to
geometry or logic,
but…
"There is such a thing
as a tesseract."
— Madeleine L'Engle,
A Wrinkle in Time
Comments Off on Monday May 21, 2007
Sunday, March 18, 2007
Sunday March 18, 2007
Update to
The Geometry of Logic:
A detailed description of a group of 16 “logical automorphisms” of the 16 binary connectives has been given in the paper “Simetria y Logica: La notacion de Peirce para los 16 conectivos binarios,” by Mireya Garcia, Jhon Fredy Gomez, and Arnold Oostra. (Published in the Memorias del XII Encuentro de Geometria y sus Aplicaciones, Universidad Pedagogica Nacional, Bogota, June 2001; on the Web at http://www.unav.es/gep/Articulos/SimetriaYLogica.pdf.) The authors do not identify this group as a subgroup of the affine group of A (the finite affine geometry of four dimensions over the two-element field); this can serve as an exercise. Another exercise: determining whether the authors’ order-16 group includes all transformations that might reasonably be called “logical automorphisms” of the 16 binary connectives.
Comments Off on Sunday March 18, 2007
Thursday, March 8, 2007
Thursday March 8, 2007
Day Without
Logic
Logic
Symbol of the Dec. 1
“Day Without Art“
This resembles the following symbol,
due to logician Charles Sanders Peirce,
of the logic of binary opposition:
(For futher details on the role
of this symbol in logic, see
Chinese Jar Revisited.)
On this, International Women’s Day,
we might also consider the
widely quoted thoughts on logic of
Harvard professor Barbara Johnson:
Detail:
“Instead of a simple ‘either/or’ structure,
deconstruction attempts to elaborate a discourse
that says neither “either/or”, nor “both/and”
nor even “neither/nor”, while at the same time
not totally abandoning these logics either.”
It may also be of interest on
International Women’s Day
that in the “box style” I Ching
(suggested by a remark of
Jungian analyst
Marie-Louise von Franz)
the symbol
denotes
Hexagram 2,
The Receptive.
Comments Off on Thursday March 8, 2007
Friday, August 4, 2006
Friday August 4, 2006
The Double Cross
The following symbol
has been associated
with the date
December 1:
Click on the symbol
for details.
That date is connected
to today’s date since
Dec. 1 is the feast—
i.e., the deathday– of
a saint of mathematics:
G. H. Hardy, author of
the classic
A Mathematician’s Apology
(online, pdf, 52 pp. ),
while today is the birthday
of three less saintly
mathematical figures:
Sir William Rowan Hamilton,
For these birthdays, here is
a more cheerful version of
the above symbol:
For the significance of
this version, see
Chinese Jar Revisited
(Log24, June 27, 2006),
a memorial to mathematician
Irving Kaplansky
(student of Mac Lane).
This version may be regarded
as a box containing the
cross of St. Andrew.
If we add a Greek cross
(equal-armed) to the box,
we obtain the “spider,”
or “double cross,” figure
of my favorite mythology:
Fritz Leiber’s Changewar.
Comments Off on Friday August 4, 2006
Tuesday, June 27, 2006
Tuesday June 27, 2006
Chinese Jar
Revisited
Revisited
In memory of
Irving Kaplansky,
who died on
Sunday, June 25, 2006
“Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness.”
— T. S. Eliot
Kaplansky received his doctorate in mathematics at Harvard in 1941 as the first Ph.D. student of Saunders Mac Lane.
From the April 25, 2005, Harvard Crimson:
Ex-Math Prof Mac Lane, 95, Dies
Gade University Professor of Mathematics Barry Mazur, a friend of the late Mac Lane, recalled that [a Mac Lane paper of 1945] had at first been rejected from a lower-caliber mathematical journal because the editor thought that it was “more devoid of content” than any other he had read.“Saunders wrote back and said, ‘That’s the point,'” Mazur said. “And in some ways that’s the genius of it. It’s the barest, most Beckett-like vocabulary that incorporates the theory and nothing else.”
He likened it to a sparse grammar of nouns and verbs and a limited vocabulary that is presented “in such a deft way that it will help you understand any language you wish to understand and any language will fit into it.”
A sparse grammar of lines from Charles Sanders Peirce (Harvard College, class of 1859):
It is true of this set of binary connectives, as it is true of logic generally, that (as alleged above of Mac Lane’s category theory) “it will help you understand any language you wish to understand and any language will fit into it.” Of course, a great deal of questionable material has been written about these connectives. (See, for instance, Piaget and De Giacomo.) For remarks on the connectives that are not questionable, see Wittgenstein’s Tractatus Logico-Philosophicus (English version, 1922), section 5.101, and Knuth’s “Boolean Basics” (draft, 2006).
Related entry: Binary Geometry.
Comments Off on Tuesday June 27, 2006
Friday, June 23, 2006
Friday June 23, 2006
Binary Geometry
There is currently no area of mathematics named “binary geometry.” This is, therefore, a possible name for the geometry of sets with 2n elements (i.e., a sub-topic of Galois geometry and of algebraic geometry over finite fields– part of Weil’s “Rosetta stone” (pdf)).
Examples:
- Charles Sanders Peirce, “The Simplest Mathematics.”
- Donald E. Knuth’s discussion of binary hypercubes in “Boolean Basics,” a draft of section 7.1.1 in The Art of Computer Programming, Volume 4: Combinatorial Algorithms
- My own discussion of a binary hypercube in Geometry of the 4x4x4 Cube
- A more sophisticated example: the geometry of elliptic curves over a binary Galois field. For an excellent introduction, see the Certicom online elliptic curve tutorial. This has an applet illustrating elliptic curves in a space of 256 points (256=16×16, with the x and y variables of a curve each having 16 possible values).
- In summary, apart from the fact that the native language of computers has characteristic 2, “binary” mathematics, i.e. mathematics in characteristic 2, is of special interest both in the study of finite geometry (Finite Geometry of the Square and Cube) and in algebraic geometry (see, for instance, the work of Brian Conrad).
Comments Off on Friday June 23, 2006