Last year on this date:
A Riddler Wannabe —
Related material — The Krauss passage quoted as above
by Shechtman in The New Yorker in December 2021 appears
also in a Log24 post of October 18, 2017: "Three Small Grids."
Last year on this date:
A Riddler Wannabe —
Related material — The Krauss passage quoted as above
by Shechtman in The New Yorker in December 2021 appears
also in a Log24 post of October 18, 2017: "Three Small Grids."
This post was suggested by the December 4th death
of modernist composer Jonathan Harvey, 73,
and by Harvey's reflections on his 2007 opera
Wagner Dream .
For related reflections, see the Oct. 10 post on
the Dürer magic square in Mann's Doctor Faustus .
See also a December 2nd post on the Nov. 18 death of
chess grandmaster Elena Akhmilovskaya Donaldson.

"A world of made
is not a world of born— pity poor flesh
and trees, poor stars and stones, but never this
fine specimen of hypermagical
ultraomnipotence."
— e. e. cummings, 1944
For one such specimen, see The Matrix of Abraham—
a 5×5 square that is hypermagical… indeed, diabolical.
Related material on the algebra and geometry underlying some smaller structures
that have also, unfortunately, become associated with the word "magic"—
" … listen: there's a hell
of a good universe next door; let's go"
— e. e. cummings
Happy birthday, e. e.
Image from a Sunday, January 7th, 2024, post now tagged "A Seventh Seal" —
Related image from a "Mathematics for Davos" post of
Thursday, January 18, 2024 —
Two notes from this journal on December 2, 2023 —
A flashback for The November Man —
As for " my own 'diamond theorem' " —
|
Question — "What is mathematician Steven H. Cullinane known for?" From the Copilot response (shorn of footnotes) — "Steven H. Cullinane is known for his work in finite geometry, particularly his Diamond Theorem. This theorem explains the surprising symmetry properties of some simple graphic designs, such as those found in quilts. It also provides a model for the 15 points, 35 lines, and 15 hyperplanes of the finite projective space PG (3,2). Cullinane’s work underlies the structure of the 35 square patterns in R. T. Curtis’s Miracle Octad Generator. His theorem has applications to sporadic simple groups, the connection between orthogonal Latin squares and projective spreads, and the symmetry of Walsh functions." |

Updates from later the same day —
Related affine structures —
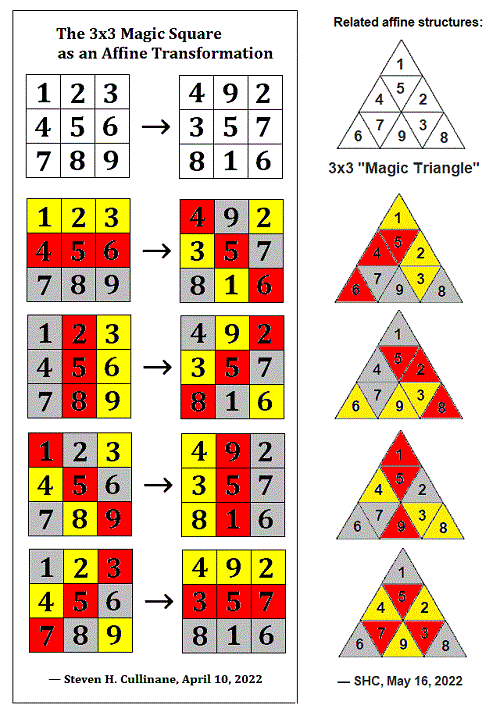
See also "Square+Triangles" in this journal.
The fishlike shapes within three of the above
ninefold colored triangles suggest some . . .
Related Entertainment —
|
Unmagic Squares Consecutive positive integers:
1 2 3 Consecutive nonnegative integers:
0 1 2
Consecutive nonnegative integers
00 01 02
This last square may be viewed as
Note that the ninefold square so viewed
As does, similarly, the ancient Chinese
These squares are therefore equivalent under This method generalizes. — Steven H. Cullinane, Nov. 20, 2021 |
For fans of “WandaVision” —
“1978 was perhaps the seminal year in the origin of chaos magic. . . .”
— Wikipedia article on Chaos Magic
Non-Chaos Non-Magic from Halloween 1978 —
Related material —
A doctoral student of a different Peter Cameron —



( Not to be confused with The Tin Man’s Hat. )
A commenter on Saturday's "Seize the Dia" has
suggested a look at the work of one Mark Collins.
Here is such a look (click to enlarge):
I find attempts to associate pure mathematics with the words
"magic" or "mystic" rather nauseating. (H. F. Baker's work
on Pascal's mystic hexagram is no exception; Baker was
stuck with Pascal's obnoxious adjective, but had no truck
with any mystic aspects of the hexagram.)
The remarks above by Clifford Pickover on Collins, Dürer, and
binary representations may interest some non-mathematicians,
who should not be encouraged to waste their time on this topic.
For the mathematics underlying the binary representation of
Dürer's square, see, for instance, my 1984 article "Binary
Coordinate Systems."
Those without the background to understand that article
may enjoy, instead of Pickover's abortive attempts above at
mathematical vulgarization, his impressively awful 2009 novel
Jews in Hyperspace .
Pickover's 2002 book on magic squares was, unfortunately,
published by the formerly reputable Princeton University Press.
Related material from today's Daily Princetonian :
See also Nash + Princeton in this journal.
From the post Belgian Puzzle Art —
Related reading . . .
— "The Devil, unlike the angels, was at home in the world of phenomena.
He knew how to combine pure concepts with empirical intuitions …
which is the basic principle of linguistic creation."
(Noah Jonathan Jacobs, Naming-Day in Eden, Macmillan, 1958 …
In Macmillan 1969 revised edition, page 21.)
The figure of 25 parts discussed in
"On Linguistic Creation"–
— "Such is the square dance of Numbers."
(Jacques Derrida, Dissemination, 1972)
— "It all adds up."
(Saul Bellow, book title, 1994)
"Death is the mother of beauty." — Wallace Stevens
From the 2020 Feast of St. Wallace Stevens,
who reportedly died in 1955 on August 2 —
Related material —

Exercise: Can each order-4 magic square be obtained by some
transformation like the one above (i.e., preserving affine hyperplanes)?
If not, why not?
Update of 31 Jan. 2023 — Spoiler: Remarks by Tilman Piesk.
Fanciful version —
Less fanciful versions . . .
|
Unmagic Squares Consecutive positive integers:
1 2 3 Consecutive nonnegative integers:
0 1 2
Consecutive nonnegative integers
00 01 02
This last square may be viewed as
Note that the ninefold square so viewed
As does, similarly, the ancient Chinese
These squares are therefore equivalent under This method generalizes. — Steven H. Cullinane, Nov. 20, 2021 |
“That really is, really, I think, the Island of the Misfit Toys at that point.
You have crossed the Rubicon, you jumped on the crazy train and
you’re headed into the cliffs that guard the flat earth at that time, brother,”
said Rep. Denver Riggleman, a Republican congressman from Virginia,
in an interview."
— Jon Ward, political correspondent, Yahoo News , Nov. 12, 2020
The instinct for heaven had its counterpart:
The instinct for earth, for New Haven, for his room,
The gay tournamonde as of a single world
In which he is and as and is are one.
— Wallace Stevens, "An Ordinary Evening in New Haven"
Related material for comedians —
See as well Sallows in this journal.
“There exists a considerable literature
devoted to the Lo shu , much of it infected
with the kind of crypto-mystic twaddle
met with in Feng Shui.”
— Lee C. F. Sallows, Geometric Magic Squares ,
Dover Publications, 2013, page 121
The elementary shapes at the top of the figure below mirror
the looking-glass property of the classical Lo Shu square.
The nine shapes at top left* and their looking-glass reflection
illustrate the looking-glass reflection relating two orthogonal
Latin squares over the three digits of modulo-three arithmetic.
Combining these two orthogonal Latin squares,** we have a
representation in base three of the numbers from 0 to 8.
Adding 1 to each of these numbers yields the Lo Shu square.

* The array at top left is from the cover of
Wonder Years:
Werkplaats Typografie 1998-2008.
** A well-known construction.
*** For other instances of what might be
called "design grammar" in combinatorics,
see a slide presentation by Robin Wilson.
No reference to the work of Chomsky is
intended.
An earlier post today, now tagged "Three Small Magic Squares,"
suggests a review of a post from October 25 three years ago
that contains the following figure —

Fans of the October Revolution may enjoy a passage
by Rosalind Krauss on grids:

Structure of the Dürer magic square
16 3 2 13
5 10 11 8 decreased by 1 is …
9 6 7 12
4 15 14 1
15 2 1 12
4 9 10 7
8 5 6 11
3 14 13 0 .
Base 4 —
33 02 01 30
10 21 22 13
20 11 12 23
03 32 31 00 .
Two-part decomposition of base-4 array
as two (non-Latin) orthogonal arrays —
3 0 0 3 3 2 1 0
1 2 2 1 0 1 2 3
2 1 1 2 0 1 2 3
0 3 3 0 3 2 1 0 .
Base 2 –
1111 0010 0001 1100
0100 1001 1010 0111
1000 0101 0110 1011
0011 1110 1101 0000 .
Four-part decomposition of base-2 array
as four affine hyperplanes over GF(2) —
1001 1001 1100 1010
0110 1001 0011 0101
1001 0110 0011 0101
0110 0110 1100 1010 .
— Steven H. Cullinane,
October 18, 2017
See also recent related analyses of
noted 3×3 and 5×5 magic squares.
See also Holy Field in this journal.
Some related mathematics —
Analysis of the Lo Shu structure —
Structure of the 3×3 magic square:
4 9 2
3 5 7 decreased by 1 is …
8 1 6
3 8 1
2 4 6
7 0 5
In base 3 —
10 22 01
02 11 20
21 00 12
As orthogonal Latin squares
(a well-known construction) —
1 2 0 0 2 1
0 1 2 2 1 0
2 0 1 1 0 2 .
— Steven H. Cullinane,
October 17, 2017
"God said to Abraham …." — Bob Dylan, "Highway 61 Revisited"
Related material —
See as well Charles Small, Harvard '64,
"Magic Squares over Fields" —
— and Conway-Norton-Ryba in this journal.
Some remarks on an order-five magic square over GF(52):
on the numbers 0 to 24:
22 5 18 1 14
3 11 24 7 15
9 17 0 13 21
10 23 6 19 2
16 4 12 20 8
Base-5:
42 10 33 01 24
03 21 44 12 30
14 32 00 23 41
20 43 11 34 02
31 04 22 40 13
Regarding the above digits as representing
elements of the vector 2-space over GF(5)
(or the vector 1-space over GF(52)) …
All vector row sums = (0, 0) (or 0, over GF(52)).
All vector column sums = same.
Above array as two
orthogonal Latin squares:
4 1 3 0 2 2 0 3 1 4
0 2 4 1 3 3 1 4 2 0
1 3 0 2 4 4 2 0 3 1
2 4 1 3 0 0 3 1 4 2
3 0 2 4 1 1 4 2 0 3
— Steven H. Cullinane,
October 16, 2017
Or: Coordinatization for Physicists
This post was suggested by the link on the word "coordinatized"
in the previous post.


I regret that Weyl's term "coordinatization" perhaps has
too many syllables for the readers of recreational mathematics —
for example, of an article on 4×4 magic squares by Conway, Norton,
and Ryba to be published today by Princeton University Press.
Insight into the deeper properties of such squares unfortunately
requires both the ability to learn what a "Galois field" is and the
ability to comprehend seven-syllable words.
In a book to be published Sept. 5 by Princeton University Press,
John Conway, Simon Norton, and Alex Ryba present the following
result on order-four magic squares —
A monograph published in 1976, “Diamond Theory,” deals with
more general 4×4 squares containing entries from the Galois fields
GF(2), GF(4), or GF(16). These squares have remarkable, if not
“magic,” symmetry properties. See excerpts in a 1977 article.
See also Magic Square and Diamond Theorem in this journal.
The late physicist David Ritz Finkelstein on the magic square
in Dürer's "Melencolia I" —
"As a child I wondered why such a square was called magic.
The Occult Philosophy [of Agrippa] answers this question
at least. They were used as magical talismans."
The correspondence in the previous post between
Figures A and B may serve as a devotional talisman
in memory of Finkelstein, a physicist who, in the sort of
magical thinking enjoyed by traditional Catholics, might
still be lingering in Purgatory.
See also this journal on the date of Finkelstein's death —
Robert Nye, author of the novel Falstaff , reportedly died
at 77 on July 2, 2016.
Harvey D. Heinz, expert on magic squares, cubes,
tesseracts, etc., reportedly died at 82 on July 6, 2013.
In memoriam —
From the date of Nye's death:
From Nye's book:
From the date of Heinz's death:
* See also a search for the title in this journal.
Continued from All Hallows' Eve, 2014.
Last year's Halloween post displayed the
Dürer print Knight, Death, and the Devil
(illustrated below on the cover of the book
Film and Phenomenology by Allan Casebier).
Cover illustration: Knight, Death, and the Devil,
by Albrecht Dürer
Some mathematics related to a different Dürer print —

“There exists a considerable literature
devoted to the Lo shu , much of it infected
with the kind of crypto-mystic twaddle
met with in Feng Shui.”
— Lee C. F. Sallows, Geometric Magic Squares ,
Dover Publications, 2013, page 121
Cf. Raiders of the Lost Theorem, Oct. 13, 2014.
See also tonight’s previous post and
“Feng Shui” in this journal.
(Continued from Nov. 16, 2013.)
The 48 actions of GL(2,3) on a 3×3 array include the 8-element
quaternion group as a subgroup. This was illustrated in a Log24 post,
Hamilton’s Whirligig, of Jan. 5, 2006, and in a webpage whose
earliest version in the Internet Archive is from June 14, 2006.
One of these quaternion actions is pictured, without any reference
to quaternions, in a 2013 book by a Netherlands author whose
background in pure mathematics is apparently minimal:

In context (click to enlarge):
Update of later the same day —
Lee Sallows, Sept. 2011 foreword to Geometric Magic Squares —
“I first hit on the idea of a geometric magic square* in October 2001,**
and I sensed at once that I had penetrated some previously hidden portal
and was now standing on the threshold of a great adventure. It was going
to be like exploring Aladdin’s Cave. That there were treasures in the cave,
I was convinced, but how they were to be found was far from clear. The
concept of a geometric magic square is so simple that a child will grasp it
in a single glance. Ask a mathematician to create an actual specimen and
you may have a long wait before getting a response; such are the formidable
difficulties confronting the would-be constructor.”
* Defined by Sallows later in the book:
“Geometric or, less formally, geomagic is the term I use for
a magic square in which higher dimensional geometrical shapes
(or tiles or pieces ) may appear in the cells instead of numbers.”
** See some geometric matrices by Cullinane in a March 2001 webpage.
Earlier actual specimens — see Diamond Theory excerpts published in
February 1977 and a brief description of the original 1976 monograph:
“51 pp. on the symmetries & algebra of
matrices with geometric-figure entries.”
— Steven H. Cullinane, 1977 ad in
Notices of the American Mathematical Society
The recreational topic of “magic” squares is of little relevance
to my own interests— group actions on such matrices and the
matrices’ role as models of finite geometries.

Yes. See …
The 48 actions of GL(2,3) on a 3×3 coordinate-array A,
when matrices of that group right-multiply the elements of A,
with A =
| (1,1) (1,0) (1,2) (0,1) (0,0) (0,2) (2,1) (2,0) (2,2) |
Actions of GL(2,p) on a pxp coordinate-array have the
same sorts of symmetries, where p is any odd prime.
Note that A, regarded in the Sallows manner as a magic square,
has the constant sum (0,0) in rows, columns, both diagonals, and
all four broken diagonals (with arithmetic modulo 3).
For a more sophisticated approach to the structure of the
ninefold square, see Coxeter + Aleph.
For the cruelest month
Click for a much larger version of the photo below.
These four Kountry Korn quartets are from the Fox Valleyaires
Men's Barbershop Chorus of Appleton, Wisconsin.
See also the fine arts here on Saturday, April 6, 2013—
The New York Times Magazine cover story
a decade ago, on Sunday, April 6, 2003:
"The artists demanded space
in tune with their aesthetic."
— "The Dia Generation,"
by Michael Kimmelman
Related material:
See Wikipedia for the difference between binary numbers
and binary coordinates from the finite Galois field GF(2).
For some background, see the relativity problem.
See also the chapter on vector spaces in Korn & Korn
(originally published by McGraw-Hill)—
 .
.
In memory of Charles Rosen:
Related material:
The Magic Square in Doctor Faustus (October 10th, 2012)
Elementary Finite Geometry (August 1st, 2012)
The Space of Horizons (August 7th, 2012)
Chromatic Plenitude (Rosen on Schoenberg)
Last Wednesday's 11 PM post mentioned the
adjacency-isomorphism relating the 4-dimensional
hypercube over the 2-element Galois field GF(2) to
the 4×4 array made up of 16 square cells, with
opposite edges of the 4×4 array identified.
A web page illustrates this property with diagrams that
enjoy the Karnaugh property— adjacent vertices, or cells,
differ in exactly one coordinate. A brief paper by two German
authors relates the Karnaugh property to the construction
of a magic square like that of Dürer (see last Wednesday).
In a similar way (search the Web for Karnaugh + cube ),
vertex adjacency in the 6-dimensional hypercube over GF(2)
is isomorphic to cell adjacency in the 4x4x4 cube, with
opposite faces of the 4x4x4 cube identified.
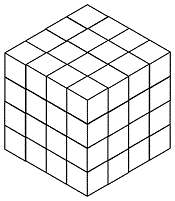
The above cube may be used to illustrate some properties
of the 64-point Galois 6-space that are more advanced
than those studied by enthusiasts of "magic" squares
and cubes.
See
Those who prefer narrative to mathematics may
consult posts in this journal containing the word "Cuber."
Occurrences of the phrase "magic square" in Lowe-Porter's translation of the Thomas Mann novel Doctor Faustus—
"On the wall above the piano was an arithmetical diagram fastened with drawing-pins, something he had found in a second-hand shop: a so-called magic square, such as appears also in Dürer's Melancolia , along with the hour-glass, the circle, the scale, the polyhedron, and other symbols. Here as there, the figure was divided into sixteen Arabic-numbered fields, in such a way that number one was in the right-hand lower corner, sixteen in the upper left; and the magic, or the oddity, simply consisted in the fact that the sum of these numerals, however you added them, straight down, crosswise, or diagonally, always came to thirty-four. What the principle was upon which this magic uniformity rested I never made out, but by virtue of the prominent place Adrian had given it over the piano, it always attracted the eye, and I believe I never visited his room without giving a quick glance, slanting up or straight down and testing once more the invariable, incredible result."
….
"Adrian kept without changing during the whole four and a half years he spent in Leipzig his two-room quarters in Peterstrasse near the Collegium Beatae Virginis, where he had again pinned the magic square above his cottage piano."
….
" 'The decisive factor is that every note, without exception, has significance and function according to its place in the basic series or its derivatives. That would guarantee what I call the indifference to harmony and melody.'
'A magic square,' I said. 'But do you hope to have people hear all that?' "
….
" 'Extraordinarily Dürerish. You love it. First "how will I shiver after the sun"; and then the houre-glasse of the Melancolia . Is the magic square coming too?' "
….
"Here I will remind the reader of a conversation I had with Adrian on a long-ago day, the day of his sister's wedding at Buchel, as we walked round the Cow Trough. He developed for me— under pressure of a headache— his idea of the 'strict style,' derived from the way in which, as in the lied 'O lieb Madel, wie schlecht bist du ' melody and harmony are determined by the permutation of a fundamental five-note motif, the symbolic letters h, e, a, e, e-flat. He showed me the 'magic square' of a style of technique which yet developed the extreme of variety out of identical material and in which there is no longer anything unthematic, anything that could not prove itself to be a variation of an ever constant element. This style, this technique, he said, admitted no note, not one, which did not fulfil its thematic function in the whole structure— there was no longer any free note."
Review of related material—
Last night's midnight post (disambiguation), the followup 1 AM post (ambiguation), today's noon post (ambiguity), and Dürer in this journal.
The tesseracts of the noon post are related to the Dürer magic square by a well-known adjacency property.
"… the once stable 'father's depression' has been transmuted into a shifting reality that shimmered in a multiplicity of facets."
— Haim Omer, Tel-Aviv University, on Milanese ambiguation therapy,
p. 321 in "Three Styles of Constructive Therapy,"
Constructive Therapies, Vol. 2 , pp. 319-333,
ed. by Michael F. Hoyt (Guilford Press paperback, 1998)
"At the still point…" — T. S. Eliot
In memory of David L. Waltz, artificial-intelligence pioneer,
who died Thursday, March 22, 2012—


The following from the First of May, 2010—

Some context–

"This pattern is a square divided into nine equal parts.
It has been called the 'Holy Field' division and
was used throughout Chinese history for many
different purposes, most of which were connected
with things religious, political, or philosophical."
– The Magic Square: Cities in Ancient China,
by Alfred Schinz, Edition Axel Menges, 1996, p. 71
(Continued from Epiphany and from yesterday.)
Detail from the current American Mathematical Society homepage—
Further detail, with a comparison to Dürer’s magic square—
 |
 |
The three interpenetrating planes in the foreground of Donmoyer‘s picture
provide a clue to the structure of the the magic square array behind them.
Group the 16 elements of Donmoyer’s array into four 4-sets corresponding to the
four rows of Dürer’s square, and apply the 4-color decomposition theorem.
Note the symmetry of the set of 3 line diagrams that result.
Now consider the 4-sets 1-4, 5-8, 9-12, and 13-16, and note that these
occupy the same positions in the Donmoyer square that 4-sets of
like elements occupy in the diamond-puzzle figure below—

Thus the Donmoyer array also enjoys the structural symmetry,
invariant under 322,560 transformations, of the diamond-puzzle figure.
Just as the decomposition theorem’s interpenetrating lines explain the structure
of a 4×4 square , the foreground’s interpenetrating planes explain the structure
of a 2x2x2 cube .
For an application to theology, recall that interpenetration is a technical term
in that field, and see the following post from last year—
| Saturday, June 25, 2011
— m759 @ 12:00 PM “… the formula ‘Three Hypostases in one Ousia ‘
Ousia
|
"Rosetta Stone" as a Metaphor
in Mathematical Narratives
For some backgound, see Mathematics and Narrative from 2005.
Yesterday's posts on mathematics and narrative discussed some properties
of the 3×3 grid (also known as the ninefold square ).
For some other properties, see (at the college-undergraduate, or MAA, level)–
Ezra Brown, 2001, "Magic Squares, Finite Planes, and Points of Inflection on Elliptic Curves."
His conclusion:
When you are done, you will be able to arrange the points into [a] 3×3 magic square,
which resembles the one in the book [5] I was reading on elliptic curves….
This result ties together threads from finite geometry, recreational mathematics,
combinatorics, calculus, algebra, and number theory. Quite a feat!
5. Viktor Prasolov and Yuri Solvyev, Elliptic Functions and Elliptic Integrals ,
American Mathematical Society, 1997.
Brown fails to give an important clue to the historical background of this topic —
the word Hessian . (See, however, this word in the book on elliptic functions that he cites.)
Investigation of this word yields a related essay at the graduate-student, or AMS, level–
Igor Dolgachev and Michela Artebani, 2009, "The Hesse Pencil of Plane Cubic Curves ."
From the Dolgachev-Artebani introduction–
In this paper we discuss some old and new results about the widely known Hesse
configuration of 9 points and 12 lines in the projective plane P2(k ): each point lies
on 4 lines and each line contains 3 points, giving an abstract configuration (123, 94).
PlanetMath.org on the Hesse configuration—

A picture of the Hesse configuration–

(See Visualizing GL(2,p), a note from 1985).
Related notes from this journal —
From last November —
|
From the December 2010 American Mathematical Society Notices—
Related material from this journal— Consolation Prize (August 19, 2010) |
From 2006 —
|
Sunday December 10, 2006
“Function defined form, expressed in a pure geometry
– J. G. Ballard on Modernism
“The greatest obstacle to discovery is not ignorance –
— Daniel J. Boorstin, |
Also from 2006 —
|
Sunday November 26, 2006
Rosalind Krauss "If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World , for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete. Or, to take a more up-to-date example…."
"He was looking at the nine engravings and at the circle,
"And it's whispered that soon if we all call the tune
The nine engravings of The Club Dumas
An example of the universal*– or, according to Krauss,
"This is the garden of Apollo, the field of Reason…."
For more on the field of reason, see
A reasonable set of "strange correspondences" Unreason is, of course, more popular. * The ninefold square is perhaps a "concrete universal" in the sense of Hegel: "Two determinations found in all philosophy are the concretion of the Idea and the presence of the spirit in the same; my content must at the same time be something concrete, present. This concrete was termed Reason, and for it the more noble of those men contended with the greatest enthusiasm and warmth. Thought was raised like a standard among the nations, liberty of conviction and of conscience in me. They said to mankind, 'In this sign thou shalt conquer,' for they had before their eyes what had been done in the name of the cross alone, what had been made a matter of faith and law and religion– they saw how the sign of the cross had been degraded."
– Hegel, Lectures on the History of Philosophy ,
"For every kind of vampire, |
And from last October —
|
Friday, October 8, 2010
Starting Out in the Evening This post was suggested by last evening's post on mathematics and narrative and by Michiko Kakutani on Vargas Llosa in this morning's New York Times .
"One must proceed cautiously, for this road— of truth and falsehood in the realm of fiction— is riddled with traps and any enticing oasis is usually a mirage."
– "Is Fiction the Art of Lying?"* by Mario Vargas Llosa,
* The Web version's title has a misprint— |
天鈞
Made famous by Ursula K. Le Guin
as the book title "Lathe of Heaven,"
this Chinese phrase, tianjun, apparently
means something more like "Scales of Heaven"–
an appropriate image for Law Day 2010.

An anonymous forum user says that
"…if you switch the two characters around,
you get: 鈞天, which is one of
the nine heavens, more specifically,
the middle heaven."
This is supported by a
non-anonymous source:
"I follow A.C. Graham’s translation of
Juntian as 'Level Heaven (the innermost
of the nine divisions of heaven)';
he renders Juntian guangyue as
'the mighty music of the innermost heaven.'"
— "Music in the World of Su Shi (1037-1101):
Terminology," by Stuart H. Sargent,
Colorado State University,
Journal of Sung-Yuan Studies 32 (2002), 39-81
The Nine Divisions of Heaven–
Some context–

"This pattern is a square divided into nine equal parts.
It has been called the 'Holy Field' division and
was used throughout Chinese history for many
different purposes, most of which were connected
with things religious, political, or philosophical."
– The Magic Square: Cities in Ancient China,
by Alfred Schinz, Edition Axel Menges, 1996, p. 71
| Lotteries on August 17, 2008 |
Pennsylvania (No revelation) |
New York (Revelation) |
| Mid-day (No belief) |
No belief, no revelation 492 Chinese 4 9 2 (See below.) |
Revelation without belief 423 4/23: |
| Evening (Belief) |
Belief without revelation 272 (See below.) |
Belief and revelation 406 4/06: |
| “What is combinatorial mathematics? Combinatorial mathematics, also referred to as combinatorial analysis or combinatorics, is a mathematical discipline that began in ancient times. According to legend the Chinese Emperor Yu (c. 2200 B.C.) observed the magic square 4 9 2
3 5 7 8 1 6 on the shell of a divine turtle….” — H.J. Ryser, Combinatorial Mathematics, Mathematical Association of America, Carus Mathematical Monographs 14 (1963) |
| From Christian Tradition Today, by Jeffrey C. K. Goh (Peeters Publishers, 2004), p. 438: “Insisting that theological statements are not simply deduced from human experience, Rahner nevertheless stresses the experience of grace as the ‘real, fundamental reality of Christianity 272 ‘Grace’ is a key category in Rahner’s theology. He has expended a great deal of energy on this topic, earning himself the title, amongst others, of a ‘theologian of the graced search for meaning.’ See G. B. Kelly (ed.), Karl Rahner, in The Making of Modern Theology series (Edinburgh: T&T Clark, 1992).” |
Part I: Random Walk

Part II: X's
3/22:

Part III: O's —
A Cartoon Graveyard
in honor of the late
Gene Persson †
Today's Garfield —

See also
Midsummer Eve's Dream:
"The meeting is closed
with the lord's‡ prayer
and refreshments are served."
† Producer of plays and musicals
including Album and
The Ruling Class
‡ Lower case in honor of
Peter O'Toole, star of
the film version of
The Ruling Class.
(This film, together with
O'Toole's My Favorite Year,
may be regarded as epitomizing
Hollywood's Jesus for Jews.)
Those who prefer
less randomness
in their religion
may consult O'Toole's
more famous film work
involving Islam,
as well as
the following structure
discussed here on
the date of Persson's death:
"The Moslems thought of the
central 1 as being symbolic
of the unity of Allah."
"Harvard seniors have
every right to demand a
Harvard-calibre speaker."
— Adam Goldenberg in
The Harvard Crimson
"Look down now, Cotton Mather"
— Wallace Stevens,
Harvard College
Class of 1901
For Thursday, June 5, 2008,
commencement day for Harvard's
Class of 2008, here are the
Pennsylvania Lottery numbers:
Mid-day 025
Evening 761
Thanks to the late
Harvard professor
Willard Van Orman Quine,
the mid-day number 025
suggests the name
"Isaac Newton."
(For the logic of this suggestion,
see On Linguistic Creation
and Raiders of the Lost Matrix.)
Thanks to Google search, the
name of Newton, combined with
Thursday's evening number 761,
suggests the following essay:
PHILOSOPHY OF SCIENCE:
|
Perhaps the Log24 entries for
the date of Koshland's death:
The Philosopher's Stone
and The Rock.
Or perhaps the following
observations:
On the figure of 25 parts
discussed in
"On Linguistic Creation"–
"The Moslems thought of the
central 1 as being symbolic
of the unity of Allah. "
— Clifford Pickover
"At the still point,
there the dance is."
— T. S. Eliot,
Harvard College
Class of 1910
|
When? Going to dark bed there was a square round Sinbad the Sailor roc's auk's egg in the night of the bed of all the auks of the rocs of Darkinbad the Brightdayler. Where?
— Ulysses, conclusion of Ch. 17 |
A Visual Meditation for
the Feast of St. Peter

For further details on this structure, see
Magic Squares, Finite Planes,
and Points of Inflection
on Elliptic Curves,
by Ezra Brown, and
Visualizing GL(2, p)
by Steven H. Cullinane.
For a more literary approach
to this structure, see
Balanchine's Birthday (Jan. 9, 2003),
Art Theory for Yom Kippur (Oct. 5, 2003),
A Form (May 22, 2004),
Ineluctable (May 27, 2004),
A Form, continued (June 5, 2004),
Parallelisms (June 6, 2004),
Deep Game (June 26, 2004), and
Gameplayers of Zen (June 27, 2004).

To appreciate fully this last entry
on Gameplayers,
one must understand
the concept of "suicide"
in the game of Go
and be reminded
by the fatuous phrase of the
Institute of Contemporary Art
quoted in Gameplayers —
"encompassed by 'nothing' " —
of John 1:5.
Jew’s on First
This entry is dedicated to those worshippers of Allah who have at one time or another cried
“Itbah al-Yahud!” … Kill the Jew!
(See June 29 entries).
|
Dead at 78 Comedian Buddy Hackett died on Tuesday, July First, 2003, according to the New York Times. According to Bloomberg.com, he died Sunday or Monday. |

Associated Press
Buddy Hackett, |
| Whatever. We may imagine he has now walked, leading a parade of many other stand-up saints, into a bar. |
|
|
MIDRASH From my May 25 entry, Matrix of the Death God: R. M. Abraham’s Diversions and Pastimes, published by Constable and Company, London, in 1933, has the following magic square:
The Matrix of Abraham A summary of the religious import of the above from Princeton University Press: “Moslems of the Middle Ages were fascinated by pandiagonal squares with 1 in the center…. The Moslems thought of the central 1 as being symbolic of the unity of Allah. Indeed, they were so awed by that symbol that they often left blank the central cell on which the 1 should be positioned.” — Clifford A. Pickover, The Zen of Magic Squares, Circles, and Stars, Princeton U. Press, 2002, pp. 71-72 Other appearances of this religious icon on the Web include:
|
In the Picasso’s Birthday version, 22 of the 25 magic square cells are correlated with pictures on the “Class of ’91” cover of Rolling Stone magazine. Number 7 is Rod† Stewart. In accordance with the theological rhyme “Seven is heaven, eight is a gate,” our site music for today is “Forever Young,” a tune made famous by Stewart.
† Roderick, actually — the name of the hero in “Madwoman of Chaillot”
— ART WARS —
Mental Health Month, Day 25:
Matrix of the Death God
Having dealt yesterday with the Death Goddess Sarah, we turn today to the Death God Abraham. (See Jacques Derrida, The Gift of Death, University of Chicago Press, 1996.) For a lengthy list of pictures of this damned homicidal lunatic about to murder his son, see The Text This Week.

See, too, The Matrix of Abraham, illustrated below. This is taken from a book by R. M. Abraham, Diversions and Pastimes, published by Constable and Company, London, in 1933.

The Matrix of Abraham
A summary of the religious import of the above from Princeton University Press:
“Moslems of the Middle Ages were fascinated by pandiagonal squares with 1 in the center…. The Moslems thought of the central 1 as being symbolic of the unity of Allah. Indeed, they were so awed by that symbol that they often left blank the central cell on which the 1 should be positioned.”
— Clifford A. Pickover, The Zen of Magic Squares, Circles, and Stars, Princeton U. Press, 2002, pp. 71-72
Other appearances of this religious icon on the Web:
A less religious approach to the icon may be found on page 393 of R. D. Carmichael’s Introduction to the Theory of Groups of Finite Order (Ginn, Boston, 1937, reprinted by Dover, 1956).
This matrix did not originate with Abraham but, unlike Neo, I have not yet found its Architect.
Exercise: Show that Dürer's 1514 "magic" square is an affine automorphism.
For a solution, see other posts now tagged Affine Squares.
Cover illustration:
Spies returning from the land of
Canaan with a cluster of grapes.
Colored woodcut from
Biblia Sacra Germanica ,
Nuremberg, Anton Koberger, 1483.
Victoria and Albert Museum, London.

Related material —
The Faustus Square :
Design from 1514

Design from 1697
Design from 1514
"One of those bells that now
and then rings" — Song lyric
Book description at Amazon.com, translated by Google —
|
Las matemáticas como herramienta
Mathematics as a tool by Raúl Ibáñez Torres Kindle edition in Spanish, 2023 Although the relationship between mathematics and art can be traced back to ancient times, mainly in geometric and technical aspects, it is with the arrival of the avant-garde and abstract art at the beginning of the 20th century that mathematics takes on greater and different relevance: as a source of inspiration and as a tool for artistic creation. Let us think, for example, of the importance of the fourth dimension for avant-garde movements or, starting with Kandisnky and later Max Bill and concrete art, the vindication of mathematical thinking in artistic creation. An idea that would have a fundamental influence on currents such as constructivism, minimalism, the fluxus movement, conceptual art, systematic art or optical art, among others. Following this approach, this book analyzes, through a variety of examples and activities, how mathematics is present in contemporary art as a creative tool. And it does so through five branches and the study of some of its mathematical topics: geometry (the Pythagorean theorem), topology (the Moebius strip), algebra (algebraic groups and matrices), combinatorics (permutations and combinations) and recreational mathematics (magic and Latin squares). |
From the book ("Cullinane Diamond Theorem" heading and picture of
book's cover added) —
Publisher: Los Libros de La Catarata (October 24, 2023)
Author: Raúl Ibáñez Torres, customarily known as Raúl Ibáñez
(Ibáñez does not mention Cullinane as the author of the above theorem
in his book (except indirectly, quoting Josefine Lyche), but he did credit
him fully in an earlier article, "The Truchet Tiles and the Diamond Puzzle"
(translation by Google).)
About Ibáñez (translated from Amazon.com by Google):
Mathematician, professor of Geometry at the University of the Basque Country
and scientific disseminator. He is part of the Chair of Scientific Culture of the
UPV/EHU and its blog Cuaderno de Cultura Cientifica. He has been a scriptwriter
and presenter of the program “Una de Mates” on the television program Órbita Laika.
He has collaborated since 2005 on the programs Graffiti and La mechanica del caracol
on Radio Euskadi. He has also been a collaborator and co-writer of the documentary
Hilos de tiempo (2020) about the artist Esther Ferrer. For 20 years he directed the
DivulgaMAT portal, Virtual Center for the Dissemination of Mathematics, and was a
member of the dissemination commission of the Royal Spanish Mathematical Society.
Author of several books, including The Secrets of Multiplication (2019) and
The Great Family of Numbers (2021), in the collection Miradas Matemáticas (Catarata).
He has received the V José María Savirón Prize for Scientific Dissemination
(national modality, 2010) and the COSCE Prize for the Dissemination of Science (2011).
Exercise: Show that Dürer's 1514 "magic" square is an affine automorphism.
For a solution, see other posts now tagged Affine Squares.
From a 1964 recreational-mathematics essay —
Note that the first two triangle-dissections above are analogous to
mutually orthogonal Latin squares . This implies a connection to
affine transformations within Galois geometry. See triangle graphics
in this journal.

Update of 4:40 AM ET —
Other mystical figures —
"Before time began, there was the Cube."
— Optimus Prime in "Transformers" (Paramount, 2007)
Alternate Title —
Types of Ambiguity:
The Circle in the Triangle,
the Singer in the Song.
From an excellent June 17 Wall Street Journal review of a new
Isaac Bashevis Singer book from Princeton University Press —
" 'Old Truths and New Clichés,' a collection of 19
prose articles, most appearing in English for the
first time, reveals that Singer was as consummate
an essayist as he was a teller of tales." — Benjamin Balint
From a search in this journal for Singer —
Related material —
From a post of June 2, "Self-Enclosing" —
|
"… the self-enclosing processes by which late 20th-century
— Colin Burrow in the June 9, 2022 issue |
From the December 14, 2021, post Notes on Lines —

The triangle, a percussion instrument that was
featured prominently in the Tom Stoppard play
"Every Good Boy Deserves Favour."
The title refers to this year's
Cannes Film Festival winner.
Related material:
From a post of June 2, "Self-Enclosing" —
|
"… the self-enclosing processes by which late 20th-century
— Colin Burrow in the June 9, 2022 issue |
From a post of June 13, "The Theater Game" —
From a post of June 12, "Triangle.graphics, 2012-2022" —
From a Jamestown (NY) Post-Journal article yesterday on
"the sold-out 10,000 Maniacs 40th anniversary concert at
The Reg Lenna Center Saturday" —
" 'The theater has a special place in our hearts. It’s played
a big part in my life,' Gustafson said.
Before being known as The Reg Lenna Center for The Arts,
it was formerly known as The Palace Theater. He recalled
watching movies there as a child…."
This, and the band's name, suggest some memories perhaps
better suited to the cinematic philosophy behind "Plan 9 from
Outer Space."

"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
The above 3×3 Tablet of Ahkmenrah image comes from
a Log24 search for the finite (i.e., Galois) field GF(3) that
was, in turn, suggested by last night's post "Making Space."
See as well a mysterious document from a website in Slovenia
that mentions a 3×3 array "relating to nine halls of a mythical
palace where rites were performed in the 1st century AD" —
An image from Slovenia missed earlier* in the search above —
"Et cetera, et cetera, et cetera." — Oscar Hammerstein
* See "Robin Wilson" in the Design Grammar post of
19 Oct. 2017. The author of the above document may
or may not be the Robin Wilson of Gresham College.
"The Magician’s finest trick was to
dismantle the pretensions of genius
while preserving his own lofty stature."
— Alex Ross in The New Yorker , Jan. 17, 2022
Related material —
Meanwhile . . .
|
Margaret Atwood on Lewis Hyde's "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159) What is "the next world"? It might be the Underworld…. The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart. |
"As a Chinese jar . . . ."
— Four Quartets
|
Rosalind Krauss "If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World , for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete. Or, to take a more up-to-date example…."
"He was looking at the nine engravings and at the circle,
"And it's whispered that soon if we all call the tune
The nine engravings of The Club Dumas
An example of the universal— or, according to Krauss,
"This is the garden of Apollo, |
The "Katz" of the August 7 post Art Angles
is a product of Princeton's
Department of Art and Archaeology.
ART —
ARCHAEOLOGY —

"This pattern is a square divided into nine equal parts.
It has been called the 'Holy Field' division and
was used throughout Chinese history for many
different purposes, most of which were connected
with things religious, political, or philosophical."
– The Magic Square: Cities in Ancient China,
by Alfred Schinz, Edition Axel Menges, 1996, p. 71
The above phrase "the intersection of storytelling and visual arts"
suggests a review . . .
Storytelling —

Visual arts —

"This pattern is a square divided into nine equal parts.
It has been called the 'Holy Field' division and
was used throughout Chinese history for many
different purposes, most of which were connected
with things religious, political, or philosophical."
– The Magic Square: Cities in Ancient China,
by Alfred Schinz, Edition Axel Menges, 1996, p. 71
A Midrash for Michener —

For a connection of the above "Holy Field"
with pure mathematics, see Coxeter's Aleph.
"Leave a space." — Tom Stoppard, "Jumpers."

Obituary of a novelist in The Washington Post yesterday —
"He gave various explanations for how he chose his nom de plume —
le Carré means 'the square' in French —
before ultimately admitting he didn’t really know."
Related material for Dan Brown — Imperial Symbology and . . .
"Together with Tolkien and Lewis, this group forms
the Oxford School of children’s fantasy literature. . . .
They all celebrate the purported wisdom of old stories,
and follow the central tenet that Tolkien set out
for fairy-stories: ‘one thing must not be made fun of,
the magic itself. That must in the story be taken seriously,
neither laughed at nor explained away.’ "
— A leftist academic's essay at aeon.co, "Empire of Fantasy,"
on St. Andrew's Day, 2020.
A more respectable writer on literature and magic —

The previous post dealt with "magic" cubes, so called because of the
analogous "magic" squares. Douglas Hofstadter has written about a
different, physical , object, promoted as "the Magic Cube," that Hofstadter
felt embodied "a deep invariant":
The three previous posts have now been tagged . . .
Tetrahedron vs. Square and Triangle vs. Cube.
Related material —
Tetrahedron vs. Square:
Labeling the Tetrahedral Model (Click to enlarge) —
Triangle vs. Cube:

… and, from the date of the above John Baez remark —
And now, General, time presses; and America is in a hurry.
Have you realized that though you may occupy towns and win battles,
you cannot conquer a nation? — The Devil's Disciple
A figure related to Dürer's "magic" square posted during Devil's Night —
Box Office Report —
"Only a peculiar can enter a time loop."
A post from Halloween season seven years ago last Saturday —
Related material — This morning's "Highway 61 Revisited."
The Cube and the Hexagram
The above illustration, by the late Harvey D. Heinz,
shows a magic cube* and a corresponding magic
hexagram, or Star of David, with the six cube faces
mapped to the six hexagram lines and the twelve
cube edges mapped to the twelve hexagram points.
The eight cube vertices correspond to eight triangles
in the hexagram (six small and two large).
Exercise: Is this noteworthy mapping** of faces to lines,
edges to points, and vertices to triangles an isolated
phenomenon, or can it be viewed in a larger context?
* See the discussion at magic-squares.net of
"perimeter-magic cubes"
** Apparently derived from the Cube + Hexagon figure
discussed here in various earlier posts. See also
"Diamonds and Whirls," a note from 1984.
This journal on Saturday, Dec. 19 —
|
“By groping toward the light we are made to realize how deep the darkness is around us.” — Arthur Koestler, The Call Girls: A Tragi-Comedy, Random House, 1973, page 118 |
In memory of Madame Claude, who
reportedly died in Nice December 19:
"There were fairies and spirits."
Amen.
On conductor Kurt Masur, who reportedly died at 88
in Greenwich, Connecticut, today, Saturday, Dec.19, 2015 —
"Rehearsal conductor at Halle State Theater,
Saxony, East Germany, conductor at Erfurt City Theater
and Leipzig Opera, and guest conductor with Leipzig
and Dresden Radio orchestras, 1951-53…."
Motifs from yesterday's 9 PM post —

Design from 1697
— and from a novel by Thomas Mann:
Design from 1514
Related text —
Holy Saturday is, according to tradition, the day of
the harrowing of Hell.
Notes:
The above passage on "Die Figuren der vier Modi
im Magischen Quadrat " should be read in the context of
a Log24 post from last year's Devil's Night (the night of
October 30-31). The post, "Structure," indicates that, using
the transformations of the diamond theorem, the notorious
"magic" square of Albrecht Dürer may be transformed
into normal reading order. That order is only one of
322,560 natural reading orders for any 4×4 array of
symbols. The above four "modi" describe another.
Oslo artist Josefine Lyche has a new Instagram post,
this time on pyramids (the monumental kind).
My response —
Wikipedia's definition of a tetrahedron as a
"triangle-based pyramid" …
… and remarks from a Log24 post of August 14, 2013 :
|
Norway dance (as interpreted by an American)
I prefer a different, Norwegian, interpretation of "the dance of four."
Related material: |
See also some of Burkard Polster's triangle-based pyramids
and a 1983 triangle-based pyramid in a paper that Polster cites —
(Click image below to enlarge.)
Some other illustrations that are particularly relevant
for Lyche, an enthusiast of magic :
From On Art and Magic (May 5, 2011) —
|
|
(Updated at about 7 PM ET on Dec. 3.)
See a University of Wisconsin obituary for Schneider,
a leading expert on linear algebra who reportedly died
at 87 on Tuesday, October 28, 2014.
Some background on linear algebra and “magic” squares:
tonight’s 3 AM (ET) post and a search in this
journal for Knight, Death, and the Devil.
Click image to enlarge.
Introducing a group of 322,560 affine transformations of Dürer’s ‘Magic’ Square
The four vector-space substructures of digits in 1st, 2nd, 3rd, 4th place,
together with the diamond theorem, indicate that Dürer’s square “minus one”
can be transformed by permutations of rows, columns, and quadrants to a
square with (decimal) digits in the usual numerical order, increasing from
top left to bottom right. Such permutations form a group of order 322,560.
(Continued from Vector Addition in a Finite Field, Twelfth Night, 2013.)
Two news items on art as a tool:
Two Log24 posts related to the 3×3 grid, the underlying structure for China’s
ancient Lo Shu “magic” square:
Finally, leftist art theorist Rosalind Krauss in this journal
on AntiChristmas, 2010:

Which is the tool here, the grid or Krauss?
The title is from a Log24 post, "Diabolically Complex Riddle," of Sept. 27, 2014.
(See also a search for "Diabolic" in this journal, which yields an application to
"magic" squares.)
For John Milton at the Cervecería XX —
Related material: Peter J. Cameron on Bertrand Russell
in A Midnight Exorcism.
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—

Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Yesterday's post Treasure Hunt, on a Brazilian weblog,
suggests a review of Brazil in this journal. The post
most relevant to yesterday's remarks is from
August 15, 2003, with a link, now broken, to the work
of Brazilian artist Nicole Sigaud* that also uses the
four half-square tiles used in 1704 by Sebastien Truchet
and somewhat later by myself in Diamond Theory
(see a 1977 version).
A more recent link that works:
|
http://vismath9.tripod.com/sigaud/e-index.html ANACOM PROJECT
APPLICATIONS
© 1997 – 2002 Nicole Sigaud |
* Sigaud shares the interests of her fellow Brazilian
whose weblog was the subject of yesterday's
Treasure Hunt.—
"For many years I have dedicated myself to the study
of medieval magic, demonology, Kabbalah, Astrology,
Alchemy, Tarot and divination in general."
— Nicole Sigaud (translated by Google) in a self-profile:
http://www.recantodasletras.com.br/autor.php?id=78359.
I do not share the interest of these authors in such matters,
except as they are reflected in the works of authors like
Charles Williams and Umberto Eco.
A magic— indeed, diabolic— square:

For the construction, see a book
by W. W. Rouse Ball, founding president
of a Cambridge University magic society.
For some related religious remarks,
see Raiders of the Lost Matrix.
This morning's New York Times obituaries—
These suggest a look at Solving Nabokov's Lolita Riddle ,
by Joanne Morgan (Sydney: Cosynch Press, 2005).
That book discusses Lolita as a character like Lewis Carroll's Alice.
(The Red Queen and Alice of course correspond to figures in
the first two thumbnails above.)
From the obituary associated with the third thumbnail above:
"Front-page headlines combined concision and dark humor."
The title of this post, Bend Sinister , is not unlike such a headline.
It is the title of a novel by Nabokov (often compared with Orwell's 1984 )
that is discussed in the Lolita Riddle book.
Related material— The bend sinister found in Log24 searches
for Hexagram 14 and for the phrase Hands-On—
 |
 |
The New York Times today—
"Reality and our perception of it are incommensurate…."
The above New York Times Wire item from 3:35 PM ET today
mentions two topics touched on in today's earlier Log24 post
Bowling in Diagon Alley— magic (implied by the title) and
incommensurability. The connection in that post
between the two topics is the diagonal of a square.
The wire item shows one detail from a Times illustration
of the linked article— a blindfolded woman.
Another detail from the same illustration—

Hands-on Wand Work
See also remarks on Magic in this journal and on Harry Potter.
I dislike both topics.
The twin topics of autism and of narrowing definitions
suggested the following remarks.
The mystical number "318" in the pilot episode
of Kiefer Sutherland's new series about autism, "Touch,"
is so small that it can easily apply (as the pilot
illustrated) to many different things: a date, a
time, a bus number, an address, etc.
The last 3/18 Log24 post— Defining Configurations—
led, after a false start and some further research,
to the writing of the webpage Configurations and Squares.
An image from that page—

Interpreting this, in an autistic manner, as the number
287501346 lets us search for more specific items
than those labeled simply 318.
The search yields, among other things, an offer of
Night Magic Cologne (unsold)—
For further mystery and magic, see, from the date
the Night Magic offer closed— May 8, 2010— "A Better Story."
See also the next day's followup, "The Ninth Gate."
The Story of N

Roberta Smith in the New York Times of July 7, 2006—
Art Review
"… The show has an endgame, end-time mood, as if we are looking at the end of the end of the end of Pop, hyperrealism and appropriation art. The techniques of replication and copying have become so meticulous that they are beside the point. This is truly magic realism: the kind you can't see, that has to be explained. It is also a time when artists cultivate hybridism and multiplicity and disdain stylistic coherence, in keeping with the fashionable interest in collectivity, lack of ego, the fluidity of individual identity. But too often these avoidance tactics eliminate the thread of a personal sensibility or focus.
I would call all these strategies fear of form, which can be parsed as fear of materials, of working with the hands in an overt way and of originality. Most of all originality. Can we just say it? This far from Andy Warhol and Duchamp, the dismissal of originality is perhaps the oldest ploy in the postmodern playbook. To call yourself an artist at all is by definition to announce a faith, however unacknowledged, in some form of originality, first for yourself, second, perhaps, for the rest of us.
Fear of form above all means fear of compression— of an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible. With a few exceptions, forms of collage and assemblage dominate this show: the putting together (or simply putting side by side) of existing images and objects prevails. The consistency of this technique in two and three dimensions should have been a red flag for the curators. Collage has driven much art since the late 1970's. Lately, and especially in this exhibition, it often seems to have become so distended and pulled apart that its components have become virtually autonomous and unrelated, which brings us back to square one. This is most obvious in the large installations of graphic works whose individual parts gain impact and meaning from juxtaposition but are in fact considered distinct artworks."
Margaret Atwood on art and the trickster—
"The pleasures of fabulation, the charming and playful lie— this line of thought leads Hyde* to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning 'to join,' 'to fit,' and 'to make.' If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart."
* Lewis Hyde, Trickster Makes This World: Mischief, Myth, and Art, Farrar Straus & Giroux, January 1998
Smith mentions "an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible."
Atwood mentions "a seamless whole."
For some related remarks, see "A Study in Art Education" and the central figure pictured above. (There "N" can stand for "number," "nine," or "narrative.")
Magic Boxes
"Somehow it seems to fill my head with ideas– only I don't exactly know what they are!…. Let's have a look at the garden first!"
— A passage from Lewis Carroll's Through the Looking-Glass. The "garden" part– but not the "ideas" part– was quoted by Jacques Derrida in Dissemination in the epigraph to Chapter 7, "The Time before First."
Commentary
on the passage:
Part I "The Magic Box," shown on Turner Classic Movies earlier tonight
Part II: "Mimsy Were the Borogoves," a classic science fiction story:
"… he lifted a square, transparent crystal block, small enough to cup in his palm– much too small to contain the maze of apparatus within it. In a moment Scott had solved that problem. The crystal was a sort of magnifying glass, vastly enlarging the things inside the block. Strange things they were, too. Miniature people, for example– They moved. Like clockwork automatons, though much more smoothly. It was rather like watching a play."
Part III: A Crystal Block —

Image of pencils is by
Diane Robertson Design.
Related material:
"A Four-Color Theorem."
Part IV:
Part I: “The Magic Box,” shown on Turner Classic Movies tonight
Part II: “Mimsy Were the Borogoves,” a classic science fiction story:
“… he lifted a square, transparent crystal block, small enough to cup in his palm– much too small to contain the maze of apparatus within it. In a moment Scott had solved that problem. The crystal was a sort of magnifying glass, vastly enlarging the things inside the block. Strange things they were, too. Miniature people, for example–
They moved. Like clockwork automatons, though much more smoothly. It was rather like watching a play.”
“Music and mathematics are among the pre-eminent wonders of the race. Levi-Strauss sees in the invention of melody ‘a key to the supreme mystery’ of man– a clue, could we but follow it, to the singular structure and genius of the species. The power of mathematics to devise actions for reasons as subtle, witty, manifold as any offered by sensory experience and to move forward in an endless unfolding of self-creating life is one of the strange, deep marks man leaves on the world. Chess, on the other hand, is a game in which thirty-two bits of ivory, horn, wood, metal, or (in stalags) sawdust stuck together with shoe polish, are pushed around on sixty-four alternately coloured squares. To the addict, such a description is blasphemy. The origins of chess are shrouded in mists of controversy, but unquestionably this very ancient, trivial pastime has seemed to many exceptionally intelligent human beings of many races and centuries to constitute a reality, a focus for the emotions, as substantial as, often more substantial than, reality itself. Cards can come to mean the same absolute. But their magnetism is impure. A mania for whist or poker hooks into the obvious, universal magic of money. The financial element in chess, where it exists at all, has always been small or accidental.
To a true chess player, the pushing about of thirty-two counters on 8×8 squares is an end in itself, a whole world next to which that of a mere biological or political or social life seems messy, stale, and contingent. Even the patzer, the wretched amateur who charges out with his knight pawn when the opponent’s bishop decamps to R4, feels this daemonic spell. There are siren moments when quite normal creatures otherwise engaged, men such as Lenin and myself, feel like giving up everything– marriage, mortgages, careers, the Russian Revolution– in order to spend their days and nights moving little carved objects up and down a quadrate board. At the sight of a set, even the tawdriest of plastic pocket sets, one’s fingers arch and a coldness as in a light sleep steals over one’s spine. Not for gain, not for knowledge or reknown, but in some autistic enchantment, pure as one of Bach’s inverted canons or Euler’s formula for polyhedra.”
— George Steiner in “A Death of Kings,” The New Yorker, issue dated September 7, 1968, page 133
“Examples are the stained-glass windows of knowledge.” —Nabokov

Click above images for some context.
Hermann Hesse's 1943 The Glass Bead Game (Picador paperback, Dec. 6, 2002, pp. 139-140)–
"For the present, the Master showed him a bulky memorandum, a proposal he had received from an organist– one of the innumerable proposals which the directorate of the Game regularly had to examine. Usually these were suggestions for the admission of new material to the Archives. One man, for example, had made a meticulous study of the history of the madrigal and discovered in the development of the style a curved that he had expressed both musically and mathematically, so that it could be included in the vocabulary of the Game. Another had examined the rhythmic structure of Julius Caesar's Latin and discovered the most striking congruences with the results of well-known studies of the intervals in Byzantine hymns. Or again some fanatic had once more unearthed some new cabala hidden in the musical notation of the fifteenth century. Then there were the tempestuous letters from abstruse experimenters who could arrive at the most astounding conclusions from, say, a comparison of the horoscopes of Goethe and Spinoza; such letters often included pretty and seemingly enlightening geometric drawings in several colors."
From Siri Hustvedt, author of Mysteries of the Rectangle: Essays on Painting (Princeton Architectural Press, 2005)– What I Loved: A Novel (Picador paperback, March 1, 2004, page 168)–
A description of the work of Bill Wechsler, a fictional artist:
"Bill worked long hours on a series of autonomous pieces about numbers. Like O's Journey, the works took place inside glass cubes, but these were twice as large– about two feet square. He drew his inspiration from sources as varied as the Cabbala, physics, baseball box scores, and stock market reports. He painted, cut, sculpted, distorted, and broke the numerical signs in each work until they became unrecognizable. He included figures, objects, books, windows, and always the written word for the number. It was rambunctious art, thick with allusion– to voids, blanks, holes, to monotheism and the individual, the the dialectic and yin-yang, to the Trinity, the three fates, and three wishes, to the golden rectangle, to seven heavens, the seven lower orders of the sephiroth, the nine Muses, the nine circles of Hell, the nine worlds of Norse mythology, but also to popular references like A Better Marriage in Five Easy Lessons and Thinner Thighs in Seven Days. Twelve-step programs were referred to in both cube one and cube two. A miniature copy of a book called The Six Mistakes Parents Make Most Often lay at the bottom of cube six. Puns appeared, usually well disguised– one, won; two, too, and Tuesday; four, for, forth; ate, eight. Bill was partial to rhymes as well, both in images and words. In cube nine, the geometric figure for a line had been painted on one glass wall. In cube three, a tiny man wearing the black-and-white prison garb of cartoons and dragging a leg iron has
— End of page 168 —
opened the door to his cell. The hidden rhyme is "free." Looking closely through the walls of the cube, one can see the parallel rhyme in another language: the German word drei is scratched into one glass wall. Lying at the bottom of the same box is a tiny black-and-white photograph cut from a book that shows the entrance to Auschwitz: ARBEIT MACHT FREI. With every number, the arbitrary dance of associations worked togethere to create a tiny mental landscape that ranged in tone from wish-fulfillment dream to nightmare. Although dense, the effect of the cubes wasn't visually disorienting. Each object, painting, drawing, bit of text, or sculpted figure found its rightful place under the glass according to the necessary, if mad, logic of numerical, pictorial, and verbal connection– and the colors of each were startling. Every number had been given a thematic hue. Bill had been interested in Goethe's color wheel and in Alfred Jensen's use of it in his thick, hallucinatory paintings of numbers. He had assigned each number a color. Like Goethe, he included black and white, although he didn't bother with the poet's meanings. Zero and one were white. Two was blue. Three was red, four was yellow, and he mixed colors: pale blue for five, purples in six, oranges in seven, greens in eight, and blacks and grays in nine. Although other colors and omnipresent newsprint always intruded on the basic scheme, the myriad shades of a single color dominated each cube.
The number pieces were the work of a man at the top of his form. An organic extension of everything Bill had done before, these knots of symbols had an explosive effect. The longer I looked at them, the more the miniature constructions seemed on the brink of bursting from internal pressure. They were tightly orchestrated semantic bombs through which Bill laid bare the arbitrary roots of meaning itself– that peculiar social contract generated by little squiggles, dashes, lines, and loops on a page."
|
From 2002:
Above: Dr. Harrison Pope, Harvard professor of psychiatry, demonstrates the use of the Wechsler Adult Intelligence Scale "block design" subtest. |
|
ZZ
Figures from the
Poem by Eugen Jost:
Mit Zeichen und Zahlen
Numbers and Names,
With numbers and names English translation A related poem:
Alphabets
From time to time
But if a savage
— Hermann Hesse (1943), |
Anthony Hopkins on time:
"For me time is God, God is time…. I'm fascinated by the fact that we can't grasp anything about time. The magical, supernatural force that is with us every second is time." —Cinema Blend
"For me time is God, God is time. It's an equation, like an Einstein equation." —Washington Square News
A Marxist on time:
"God demands scrutiny beyond his menacingly comic aspects. Primarily, the [Saramago] Gospel 's God is time, and not truth, the other attribute he asserts. Saramago, a Marxist (an eccentric one), and not a Christian, subverts St. Augustine on the theodicy of time. If time is God, then God can be forgiven nothing, and who would desire to forgive him anyway?"
—Harold Bloom on José Saramago's The Gospel According to Jesus Christ (1991). Saramago was awarded the Nobel Prize in Literature in 1998.
Augustine's Theodicy
and Joyce's Aesthetics,
From Log24 on
this date last year:
"May there be an ennui
of the first idea?
What else,
prodigious scholar,
should there be?"
— Wallace Stevens,
"Notes Toward a
Supreme Fiction"
The Associated Press,
May 25, 2007–
Thought for Today:
"I hate quotations.
Tell me what you know."
— Ralph Waldo Emerson

This "telling of what
I know" will of course
mean little to those
who, like Emerson,
have refused to learn
through quotations.
For those less obdurate
than Emerson —Harold Bloom
on Wallace Stevens
and Paul Valery's
"Dance and the Soul"–
"Stevens may be playful, yet seriously so, in describing desire, at winter's end, observing not only the emergence of the blue woman of early spring, but seeing also the myosotis, whose other name is 'forget-me-not.' Desire, hearing the calendar hymn, repudiates the negativity of the mind of winter, unable to bear what Valery's Eryximachus had called 'this cold, exact, reasonable, and moderate consideration of human life as it is.' The final form of this realization in Stevens comes in 1950, in The Course of a Particular, in the great monosyllabic line 'One feels the life of that which gives life as it is.' But even Stevens cannot bear that feeling for long. As Eryximachus goes on to say in Dance and the Soul:
A cold and perfect clarity is a poison impossible to combat. The real, in its pure state, stops the heart instantaneously….[…] To a handful of ashes is the past reduced, and the future to a tiny icicle. The soul appears to itself as an empty and measurable form. –Here, then, things as they are come together, limit one another, and are thus chained together in the most rigorous and mortal* fashion….
O Socrates, the universe cannot for one instant endure to be only what it is.
Valery's formula for reimagining the First Idea is, 'The idea introduces into what is, the leaven of what is not.' This 'murderous lucidity' can be cured only by what Valery's Socrates calls 'the intoxication due to act,' particularly Nietzschean or Dionysiac dance, for this will rescue us from the state of the Snow Man, 'the motionless and lucid observer.'" —Wallace Stevens: The Poems of Our Climate
* "la sorte… la plus mortelle":
mortal in the sense
"deadly, lethal"
Other quotations
(from March 28,
the birthday of
Reba McEntire):
Logical Songs

Logical Song I
(Supertramp)
"When I was young, it seemed that
Life was so wonderful, a miracle,
Oh it was beautiful, magical
And all the birds in the trees,
Well they'd be singing so happily,
Joyfully, playfully watching me"
Logical Song II
(Sinatra)
"You make me feel so young,
You make me feel like
Spring has sprung
And every time I see you grin
I'm such a happy in-
dividual….
You and I are
Just like a couple of tots
Running across the meadow
Picking up lots
Of forget-me-nots"
— Attributed to Euclid
There are, however, various non-royal roads. One of these is indicated by yesterday's Pennsylvania lottery numbers:
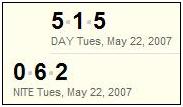
The mid-day number 515 may be taken as a reference to 5/15. (See the previous entry, "Angel in the Details," and 5/15.)
The evening number 062, in the context of Monday's entry "No Royal Roads" and yesterday's "Jewel in the Crown," may be regarded as naming a non-royal road to geometry: either U. S. 62, a major route from Mexico to Canada (home of the late geometer H.S.M. Coxeter), or a road less traveled– namely, page 62 in Coxeter's classic Introduction to Geometry (2nd ed.):

This topic Coxeter offers as an
illustration of remarks by G. H. Hardy
that he quotes on the preceding page:

Another example of strong emergence: a group of 322,560 transformations acting naturally on the 4×4 square grid— a much larger group than the group of 8 symmetries of each component (square) part.
The lottery numbers above also supply an example of strong emergence– one that nicely illustrates how it can be, in the words of Mark Bedau, "uncomfortably like magic."
(Those more comfortable with magic may note the resemblance of the central part of Coxeter's illustration to a magical counterpart– the Ojo de Dios of Mexico's Sierra Madre.)
…da ist der Tanz;
Doch weder Stillstand noch Bewegung.
Und nenne es nicht Beständigkeit,
Wo Vergangenheit und Zukunft sich sammeln.
|
|
|
| to put one's back into something |
bei etwas Einsatz zeigen |
| to up the ante |
den Einsatz erhöhen |
| to debrief | den Einsatz nachher besprechen |
| to be on duty |
im Einsatz sein |
| mil.to be in action | im Einsatz sein |
| to play for high stakes |
mit hohem Einsatz spielen |
"Nine is a very
powerful Nordic number."
— Katherine Neville,
The Magic Circle
Rosalind Krauss
in "Grids," 1979:
"If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World, for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete.
Or, to take a more up-to-date example…."
"He was looking at
the nine engravings
and at the circle,
checking strange
correspondences
between them."
— The Club Dumas,1993
"And it's whispered that soon
if we all call the tune
Then the piper will lead us
to reason."
— Robert Plant,1971
The nine engravings of
The Club Dumas
(filmed as "The Ninth Gate")
are perhaps more an example
of the concrete than of the
universal.
An example of the universal*–
or, according to Krauss, a
"staircase" to the universal–
is the ninefold square:

"This is the garden of Apollo,
the field of Reason…."
— John Outram, architect
For more on the field
of reason, see
Log24, Oct. 9, 2006.
A reasonable set of
"strange correspondences"
in the garden of Apollo
has been provided by Ezra Brown
in a mathematical essay (pdf).
Unreason is, of course,
more popular.
* The ninefold square is perhaps a "concrete universal" in the sense of Hegel:
"Two determinations found in all philosophy are the concretion of the Idea and the presence of the spirit in the same; my content must at the same time be something concrete, present. This concrete was termed Reason, and for it the more noble of those men contended with the greatest enthusiasm and warmth. Thought was raised like a standard among the nations, liberty of conviction and of conscience in me. They said to mankind, 'In this sign thou shalt conquer,' for they had before their eyes what had been done in the name of the cross alone, what had been made a matter of faith and law and religion– they saw how the sign of the cross had been degraded."
— Hegel, Lectures on the History of Philosophy, "Idea of a Concrete Universal Unity"
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon
Pandora's Box
Part I:
The Pandora Cross
— Rosalind Krauss in "Grids"

Part II:
The Opening
Remarks by the Pope on Sept. 12,
as reported by the Vatican:
Faith, Reason, and the University:
Memories and Reflections
For the result of
the Pope's remarks, see
a transcript of
yesterday's Google News
and the following
from BBC today:

Click to enlarge the screenshot.
Part III:
Hope
"In September [2005], she had a private audience with Pope Benedict XVI at Castel Gandolfo, his summer residence outside Rome. She had criticized John Paul II for making overtures to Muslims, and for not condemning terrorism heartily enough, but she has hopes for Joseph Ratzinger."
For further details, see yesterday's Log24.
Part IV:
The Sibyl's Song
— From The Magic Circle,
a spiritual narrative
by Katherine Neville
For more on "the long-mute voice
of the past," on "darkness beneath
the volcano," and on uncorking,
see Glory Season and Harrowing.
Related material from
Log24 on Dec. 2, 2005:
Benedict XVI, before he became Pope:

and a related
Christian symbol,

the Greek Cross
(adapted from
Ad Reinhardt).
Moral of the Pandora Cross:
"Nine is a very powerful Nordic number."
— Katherine Neville in The Magic Circle…
quoted in The Nine, a Log24 entry
for Hermann Weyl's birthday,
November 9, 2004.
Illustrated below:
The Restaurant

Related etymology:
OF.
from L.
— Webster's Revised
Unabridged Dictionary, 1913
Related material:
(1) A symbol of symmetry
that might have pleased
Hermann Weyl:

Source —
Timothy A. Smith on
Bach's Fugue No. 21,
the Well-Tempered
Clavier, Book II
(pdf or Shockwave)
(2) The remarks of Noam D. Elkies
on his
"Brandenburg Concerto No. 7":
"It is of course an act of chutzpah,
some would say almost heresy,
to challenge Bach so explicitly
on his own turf."
(3) The five Log24 entries
culminating on Pi Day,
March 14, 2006
(4) The following event at the
Harvard University
mathematics department
on March 14, 2006, also
featuring Noam D. Elkies:
"At 3:14 p.m., six contestants began
a pie-eating contest…. Contestants had
exactly three minutes and 14 seconds
to eat as much pie as they could.
'Five, four, pi, three, two, one,'
Elkies counted down as the
contestants shoved the last
mouthful of pie
into their mouths…."

Noam D. Elkies
"Nine is a very powerful Nordic number."
— Katherine Neville, author of The Eight,

"To live is to defend a form."
("Leben, das heisst eine Form verteidigen")
— attributed to Hölderlin
In defense of the nine-square grid:

For details on the above picture, see
Translation Plane.
The Nine
(Readings for
Weyl’s birthday)
“The grid is a staircase to the Universal….
We could think about Ad Reinhardt, who,
despite his repeated insistence that
‘Art is art,’
ended up by painting a series of…
nine-square grids in which the motif
that inescapably emerges is
a Greek cross.

Greek Cross
There is no painter in the West
who can be unaware of
the symbolic power
of the cruciform shape and the
Pandora’s box of spiritual reference
that is opened once one uses it.”
— Rosalind Krauss,
Meyer Schapiro Professor
of Modern Art and Theory
at Columbia University
(Ph.D., Harvard U., 1969),
in “Grids”

“Nine is a very powerful Nordic number.”
— Katherine Neville, author of The Eight,

in The Magic Circle,
Ballantine paperback,
1999, p. 339

“To live is to defend a form.”
(“Leben, das heisst eine Form verteidigen“)
— attributed to Hölderlin

For details on the above picture,
which deals with properties of the
nine-square grid, see
For more on the defense
of this form,

see the Log24.net entry of
June 5, 2004, A Form,
and the Art Wars entries
for St. Peter’s Day, 2004.
Story
"How much story do you want?"
— George Balanchine
While researching yesterday's entry on Balanchine, Apollo, and the nine Muses, I came across this architect's remarks, partially quoted yesterday and continued here:
"The icon that I use for this element is the nine-fold square…. This is the garden of Apollo, the field of Reason…. This is the Temple of Solomon, as inscribed, for example, by a nine-fold compartmentation to provide the ground plan of Yale, as described to me by Professor Hersey."
Checking this out yesterday, I came across the following at a Yale University Art Gallery site:
"This exhibition of nine boldly colored, asymmetrically designed quilts selected from a private collection will be displayed in the Matrix Gallery….
With the guidance of Professor Maude Southwell Wahlman, author of 'Signs and Symbols: African Images in African American Quilts,' the collector has explored and gathered examples…."
Exploring and gathering examples myself today, I received a book in the mail — W. M. Spackman's On the Decay of Humanism (Rutgers University Press, 1967) — and picked up a second-hand book at a sale — Barbara Michaels's Stitches in Time (Harper Collins Publishers, 1995).
The Spackman book includes the following poem at the end:
In sandarac etui for sepulchre
lies the cered body of a poisoned queen;
and in her mouth and hair, and at her feet,
and in the grey folds of her winding-sheet,
there sifts a dreamy powder, smooth and green,
the magic of an idle sorcerer,
an ancient spell, cast when the shroud was spun.
In death her hands clasp amourously a bowl
that still contains the fragments of her soul,
a tale of Beauty sought, and Beauty won,
his false lips kissed, and Beauty dead for her.
— Alexander B. Griswold, Princeton '28, in the
Nassau Literary Magazine of December 1925
From a synopsis of Michaels's Stitches in Time:
"Michaels follows Rachel, a graduate student studying women's crafts–weaving, spinning, quilting, embroidery–and the superstitions connected with them. Linking all important rites of passage to the garments created as markers of these occasions leads Rachel to her theory: in societies in which magic was practiced, the garment was meant to protect its wearer. She gains evidence that her theory is valid when an evil antique bridal quilt enters her life."
Although Stitches in Time is about a quilt — stitched, not spun — Griswold's line
"an ancient spell, cast when the shroud was spun"
is very closely related to the evil spell in Michaels's book.
The above events display a certain synchronicity that Wallace Stevens might appreciate, especially in light of the following remark in a review of Stitches in Time:
"…the premise is too outlandish for even the suspension of disbelief…." (Publishers Weekly, 4/24/95)
Stevens might reply,
The very man despising honest quilts
Lies quilted to his poll in his despite.— "The Comedian as the Letter C," Part V
Finally, those who prefer stories to the more formal qualities of pure dance (ballet) pure mathematics (see previous entry), pure (instrumental) music, and pure (abstract, as in quilt designs) art, can consult the oeuvre of Jodie Foster — as in my
Pearl Harbor Day entry on Buddhism.
An art historian named Griswold — perhaps that very same Griswold quoted above — might have a thing or two to say to Jodie on her recent film "Anna and the King." In the April, 1957, issue of The Journal of the Siam Society, Alexander B. Griswold takes issue with Broadway's and Hollywood's "grotesque caricature" of Siamese society, and ultimately with Anna herself:
"The real fault lies in the two books they ultimately spring from — The English Governess at the Court of Siam and The Romance of the Harem — both written by Mrs. Anna Leonowens.''
See also The Diamond 16 Puzzle for some quilt designs.
Powered by WordPress