|
A mnemonic from a course titled
“Traditionally, there are two modalities, namely,
|
For less rigorous remarks, search Log24 for Modal Diamond Box.
Saturday, July 30, 2022
Modal Diamond Box
Saturday, March 26, 2022
Box Geometry: Space, Group, Art (Work in Progress)
| Name Tag | .Space | .Group | .Art |
|---|---|---|---|
| Box4 |
2×2 square representing the four-point finite affine geometry AG(2,2). (Box4.space) |
S4 = AGL(2,2) (Box4.group) |
(Box4.art) |
| Box6 |
3×2 (3-row, 2-column) rectangular array representing the elements of an arbitrary 6-set. |
S6 | |
| Box8 | 2x2x2 cube or 4×2 (4-row, 2-column) array. | S8 or A8 or AGL(3,2) of order 1344, or GL(3,2) of order 168 | |
| Box9 | The 3×3 square. | AGL(2,3) or GL(2,3) | |
| Box12 | The 12 edges of a cube, or a 4×3 array for picturing the actions of the Mathieu group M12. | Symmetries of the cube or elements of the group M12 | |
| Box13 | The 13 symmetry axes of the cube. | Symmetries of the cube. | |
| Box15 |
The 15 points of PG(3,2), the projective geometry of 3 dimensions over the 2-element Galois field. |
Collineations of PG(3,2) | |
| Box16 |
The 16 points of AG(4,2), the affine geometry of 4 dimensions over the 2-element Galois field. |
AGL(4,2), the affine group of |
|
| Box20 | The configuration representing Desargues's theorem. | ||
| Box21 | The 21 points and 21 lines of PG(2,4). | ||
| Box24 | The 24 points of the Steiner system S(5, 8, 24). | ||
| Box25 | A 5×5 array representing PG(2,5). | ||
| Box27 |
The 3-dimensional Galois affine space over the 3-element Galois field GF(3). |
||
| Box28 | The 28 bitangents of a plane quartic curve. | ||
| Box32 |
Pair of 4×4 arrays representing orthogonal Latin squares. |
Used to represent elements of AGL(4,2) |
|
| Box35 |
A 5-row-by-7-column array representing the 35 lines in the finite projective space PG(3,2) |
PGL(3,2), order 20,160 | |
| Box36 | Eurler's 36-officer problem. | ||
| Box45 | The 45 Pascal points of the Pascal configuration. | ||
| Box48 | The 48 elements of the group AGL(2,3). | AGL(2,3). | |
| Box56 |
The 56 three-sets within an 8-set or |
||
| Box60 | The Klein configuration. | ||
| Box64 | Solomon's cube. |
— Steven H. Cullinane, March 26-27, 2022
Monday, January 27, 2020
Jewel Box
The phrase "jewel box" in a New York Times obituary online this afternoon
suggests a review. See "And He Built a Crooked House" and Galois Tesseract.
Monday, February 10, 2014
Mystery Box III: Inside, Outside
(Continued from Mystery Box, Feb. 4, and Mystery Box II, Feb. 5.)
The Box
Inside the Box
Outside the Box
For the connection of the inside notation to the outside geometry,
see Desargues via Galois.
(For a related connection to curves and surfaces in the outside
geometry, see Hudson's classic Kummer's Quartic Surface and
Rosenhain and Göpel Tetrads in PG(3,2).)
Wednesday, February 5, 2014
Mystery Box II
Continued from previous post and from Sept. 8, 2009.
Examination of the box's contents does not solve
the contents' real mystery. That requires knowledge
of the non-Euclidean geometry of Galois space.
In this case, without that knowledge, prattle (as in
today's online New York Times ) about creativity and
"thinking outside the box" is pointless.
Friday, January 29, 2016
Thursday, October 19, 2023
Math for Barbie
Continued from "Barbie at the Space Barn," Oct. 17.
"Open the Space Barn doors, Barbie." —
For those who prefer the Hollywood part of L.A.,
there is Barbierella —
Tuesday, January 28, 2020
Very Stable Kool-Aid
Two of the thumbnail previews
from yesterday's 1 AM post …
Further down in the "6 Prescott St." post, the link 5 Divinity Avenue
leads to …
|
A Letter from Timothy Leary, Ph.D., July 17, 1961
Harvard University July 17, 1961
Dr. Thomas S. Szasz Dear Dr. Szasz: Your book arrived several days ago. I've spent eight hours on it and realize the task (and joy) of reading it has just begun. The Myth of Mental Illness is the most important book in the history of psychiatry. I know it is rash and premature to make this earlier judgment. I reserve the right later to revise and perhaps suggest it is the most important book published in the twentieth century. It is great in so many ways–scholarship, clinical insight, political savvy, common sense, historical sweep, human concern– and most of all for its compassionate, shattering honesty. . . . . |
The small Morton Prince House in the above letter might, according to
the above-quoted remarks by Corinna S. Rohse, be called a "jewel box."
Harvard moved it in 1978 from Divinity Avenue to its current location at
6 Prescott Street.
Related "jewel box" material for those who
prefer narrative to mathematics —
"In The Electric Kool-Aid Acid Test , Tom Wolfe writes about encountering
'a young psychologist,' 'Clifton Fadiman’s nephew, it turned out,' in the
waiting room of the San Mateo County jail. Fadiman and his wife were
'happily stuffing three I-Ching coins into some interminable dense volume*
of Oriental mysticism' that they planned to give Ken Kesey, the Prankster-
in-Chief whom the FBI had just nabbed after eight months on the lam.
Wolfe had been granted an interview with Kesey, and they wanted him to
tell their friend about the hidden coins. During this difficult time, they
explained, Kesey needed oracular advice."
— Tim Doody in The Morning News web 'zine on July 26, 2012**
Oracular advice related to yesterday evening's
"jewel box" post …
A 4-dimensional hypercube H (a tesseract ) has 24 square
2-dimensional faces. In its incarnation as a Galois tesseract
(a 4×4 square array of points for which the appropriate transformations
are those of the affine 4-space over the finite (i.e., Galois) two-element
field GF(2)), the 24 faces transform into 140 4-point "facets." The Galois
version of H has a group of 322,560 automorphisms. Therefore, by the
orbit-stabilizer theorem, each of the 140 facets of the Galois version has
a stabilizer group of 2,304 affine transformations.
Similar remarks apply to the I Ching In its incarnation as
a Galois hexaract , for which the symmetry group — the group of
affine transformations of the 6-dimensional affine space over GF(2) —
has not 322,560 elements, but rather 1,290,157,424,640.
* The volume Wolfe mentions was, according to Fadiman, the I Ching.
** See also this journal on that date — July 26, 2012.
Monday, October 15, 2018
History at Bellevue
The previous post, "Tesserae for a Tesseract," contains the following
passage from a 1987 review of a book about Finnegans Wake —
"Basically, Mr. Bishop sees the text from above
and as a whole — less as a sequential story than
as a box of pied type or tesserae for a mosaic,
materials for a pattern to be made."
A set of 16 of the Wechsler cubes below are tesserae that
may be used to make patterns in the Galois tesseract.
Another Bellevue story —
“History, Stephen said, is a nightmare
from which I am trying to awake.”
— James Joyce, Ulysses
Monday, June 11, 2018
Arty Fact
The title was suggested by the name "ARTI" of an artificial
intelligence in the new film 2036: Origin Unknown.
The Eye of ARTI —
See also a post of May 19, "Uh-Oh" —
— and a post of June 6, "Geometry for Goyim" —
Mystery box merchandise from the 2011 J. J. Abrams film Super 8
An arty fact I prefer, suggested by the triangular computer-eye forms above —
This is from the July 29, 2012, post The Galois Tesseract.
See as well . . .
Sunday, May 20, 2018
Not So Cryptic
From the date of the New York Times James Bond video
referenced in the previous post, "A Cryptic Message" —
Monday, August 1, 2016
Cube
From this journal —
See (for instance) Sacred Order, July 18, 2006 —
From a novel published July 26, 2016, and reviewed
in yesterday's (print) New York Times Book Review —
|
The doors open slowly. I step into a hangar. From the rafters high above, lights blaze down, illuminating a twelve-foot cube the color of gunmetal. My pulse rate kicks up. I can’t believe what I’m looking at. Leighton must sense my awe, because he says, “Beautiful, isn’t it?” It is exquisitely beautiful. At first, I think the hum inside the hangar is coming from the lights, but it can’t be. It’s so deep I can feel it at the base of my spine, like the ultralow-frequency vibration of a massive engine. I drift toward the box, mesmerized.
— Crouch, Blake. Dark Matter: A Novel |
See also Log24 on the publication date of Dark Matter .
Wednesday, December 23, 2015
Splitting Apart
Sunday, September 21, 2014
Uncommon Noncore
This post was suggested by Greg Gutfeld’s Sept. 4 remarks on Common Core math.
Problem: What is 9 + 6 ?
Here are two approaches suggested by illustrations of Desargues’s theorem.
Solution 1:
9 + 6 = 10 + 5,
as in Common Core (or, more simply, as in common sense), and
10 + 5 = 5 + 10 = 15 as in Veblen and Young:
Solution 2:
In the figure below,
9 + 6 = no. of V’s + no. of A’s + no. of C’s =
no. of nonempty squares = 16 – 1 = 15.
(Illustration from Feb. 10, 2014.)
The silly educationists’ “partner, anchor, decompose” jargon
discussed by Gutfeld was their attempt to explain “9 + 6 = 10 + 5.”
As he said of the jargon, “That’s not math, that’s the plot from ‘Silence of the Lambs.'”
Or from Richard, Frank, and Marcus in last night’s “Intruders”
(BBC America, 10 PM).
Tuesday, July 9, 2013
Vril Chick
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—
Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
Sunday, July 10, 2011
Wittgenstein’s Diamond
Philosophical Investigations (1953)—
97. Thought is surrounded by a halo.
—Its essence, logic, presents an order,
in fact the a priori order of the world:
that is, the order of possibilities * ,
which must be common to both world and thought.
But this order, it seems, must be
utterly simple . It is prior to all experience,
must run through all experience;
no empirical cloudiness or uncertainty can be allowed to affect it
——It must rather be of the purest crystal.
But this crystal does not appear as an abstraction;
but as something concrete, indeed, as the most concrete,
as it were the hardest thing there is
(Tractatus Logico-Philosophicus No. 5.5563).
— Translation by G.E.M. Anscombe
All propositions of our colloquial language
are actually, just as they are, logically completely in order.
That simple thing which we ought to give here is not
a model of the truth but the complete truth itself.
(Our problems are not abstract but perhaps
the most concrete that there are.)
97. Das Denken ist mit einem Nimbus umgeben.
—Sein Wesen, die Logik, stellt eine Ordnung dar,
und zwar die Ordnung a priori der Welt,
d.i. die Ordnung der Möglichkeiten ,
die Welt und Denken gemeinsam sein muß.
Diese Ordnung aber, scheint es, muß
höchst einfach sein. Sie ist vor aller Erfahrung;
muß sich durch die ganze Erfahrung hindurchziehen;
ihr selbst darf keine erfahrungsmäßige Trübe oder Unsicherheit anhaften.
——Sie muß vielmehr vom reinsten Kristall sein.
Dieser Kristall aber erscheint nicht als eine Abstraktion;
sondern als etwas Konkretes, ja als das Konkreteste,
gleichsam Härteste . (Log. Phil. Abh. No. 5.5563.)
Related language in Łukasiewicz (1937)—
* Updates of 9:29 PM ET July 10, 2011—
A mnemonic from a course titled “Galois Connections and Modal Logics“—
“Traditionally, there are two modalities, namely, possibility and necessity.
The basic modal operators are usually written 
(square) for necessarily
and 
(diamond) for possibly. Then, for example, P can be read as
‘it is possibly the case that P .'”
| See also Intensional Semantics , lecture notes by Kai von Fintel and Irene Heim, MIT, Spring 2007 edition—
“The diamond ⋄ symbol for possibility is due to C.I. Lewis, first introduced in Lewis & Langford (1932), but he made no use of a symbol for the dual combination ¬⋄¬. The dual symbol □ was later devised by F.B. Fitch and first appeared in print in 1946 in a paper by his doctoral student Barcan (1946). See footnote 425 of Hughes & Cresswell (1968). Another notation one finds is L for necessity and M for possibility, the latter from the German möglich ‘possible.’” Barcan, Ruth C.: 1946. “A Functional Calculus of First Order Based on Strict Implication.” Journal of Symbolic Logic, 11(1): 1–16. URL http://www.jstor.org/pss/2269159. Hughes, G.E. & Cresswell, M.J.: 1968. An Introduction to Modal Logic. London: Methuen. Lewis, Clarence Irving & Langford, Cooper Harold: 1932. Symbolic Logic. New York: Century. |
Sunday, June 26, 2011
Sunday Dinner
From "Sunday Dinner" in this journal—
"'If Jesus were to visit us, it would have been
the Sunday dinner he would have insisted on
being a part of, not the worship service at the church.'"
—Judith Shulevitz at The New York Times
on Sunday, July 18, 2010
Some table topics—
Today's midday New York Lottery numbers were 027 and 7002.
The former suggests a Galois cube, the latter a course syllabus—
CSC 7002
Graduate Computer Security (Spring 2011)
University of Colorado at Denver
Department of Computer Science
An item from that syllabus:
| Six | 22 February 2011 | DES | History of DES; Encryption process; Decryption; Expander function; S-boxes and their output; Key; the function f that takes the modified key and part of the text as input; mulitple Rounds of DES; Present-day lack of Security in DES, which led to the new Encryption Standard, namely AES. Warmup for AES: the mathematics of Fields: Galois Fields, particularly the one of order 256 and its relation to the irreducible polynomial x^8 + x^4 + x^3 + x + 1 with coefficients from the field Z_2. |
Related material: A novel, PopCo , was required reading for the course.
Discuss a different novel by the same author—
Discuss the author herself, Scarlett Thomas.
Background for the discussion—
Derrida in this journal versus Charles Williams in this journal.
Related topics from the above syllabus date—
Metaphor and Gestell and Quadrat.
Some context— Midsummer Eve's Dream.
Friday, June 4, 2010
A Better Story
Continued from May 8
(Feast of Saint Robert Heinlein)
“Wells and trees were dedicated to saints. But the offerings at many wells and trees were to something other than the saint; had it not been so they would not have been, as we find they often were, forbidden. Within this double and intertwined life existed those other capacities, of which we know more now, but of which we still know little– clairvoyance, clairaudience, foresight, telepathy.”
— Charles Williams, Witchcraft, Faber and Faber, London, 1941
Why "Saint" Robert? See his accurate depiction of evil– the Eater of Souls in Glory Road.
For more on Williams's "other capacities," see Heinlein's story "Lost Legacy."
A related story– Fritz Leiber's "The Mind Spider." An excerpt:
The conference—it was much more a hyper-intimate
gabfest—proceeded.
"My static box bugged out for a few ticks this morning,"
Evelyn remarked in the course of talking over the
trivia of the past twenty-four hours.
The static boxes were an invention of Grandfather
Horn. They generated a tiny cloud of meaningless brain
waves. Without such individual thought-screens, there was
too much danger of complete loss of individual personality
—once Grandfather Horn had "become" his infant daughter
as well as himself for several hours and the unfledged
mind had come close to being permanently lost in its own
subconscious. The static boxes provided a mental wall be-
– hind which a mind could safely grow and function, similar
to the wall by which ordinary minds are apparently
always enclosed.
In spite of the boxes, the Horns shared thoughts and
emotions to an amazing degree. Their mental togetherness
was as real and as mysterious—and as incredible—as
thought itself . . . and thought is the original angel-cloud
dancing on the head of a pin. Their present conference
was as warm and intimate and tart as any actual family
gathering in one actual room around one actual table.
Five minds, joined together in the vast mental darkness
that shrouds all minds. Five minds hugged together for
comfort and safety in the infinite mental loneliness that
pervades the cosmos.
Evelyn continued, "Your boxes were all working, of
course, so I couldn't get your thoughts—just the blurs of
your boxes like little old dark grey stars. But this time
if gave me a funny uncomfortable feeling, like a spider
Crawling down my—Grayl! Don't feel so wildly! What
Is it?”
Then… just as Grayl started to think her answer…
something crept from the vast mental darkness and infinite
cosmic loneliness surrounding the five minds of the
Horns.
Grayl was the first to notice. Her panicky thought had
ttie curling too-keen edge of hysteria. "There are six of
us now! There should only be five, but there are six.
Count! Count, I tell you! Six!"
To Mort it seemed that a gigantic spider was racing
across the web of their thoughts….
See also this journal on May 30– "720 in the Book"– and on May 31– "Memorial for Galois."
("Obnoxious nerds"— a phrase Martin Gardner recently applied to Galois— will note that 720
Tuesday, September 8, 2009
Tuesday September 8, 2009
Froebel's
Magic Box
Continued from Dec. 7, 2008,
and from yesterday.
|
Non-Euclidean
Blocks
Passages from a classic story:
… he took from his pocket a gadget he had found in the box, and began to unfold it. The result resembled a tesseract, strung with beads….  Tesseract
"Your mind has been conditioned to Euclid," Holloway said. "So this– thing– bores us, and seems pointless. But a child knows nothing of Euclid. A different sort of geometry from ours wouldn't impress him as being illogical. He believes what he sees."
"Are you trying to tell me that this gadget's got a fourth dimensional extension?" Paradine demanded. "Hardening of the thought-arteries," Jane interjected. Paradine was not convinced. "Then a baby could work calculus better than Einstein? No, I don't mean that. I can see your point, more or less clearly. Only–" "Well, look. Let's suppose there are two kinds of geometry– we'll limit it, for the sake of the example. Our kind, Euclidean, and another, which we'll call x. X hasn't much relationship to Euclid. It's based on different theorems. Two and two needn't equal four in it; they could equal y, or they might not even equal. A baby's mind is not yet conditioned, except by certain questionable factors of heredity and environment. Start the infant on Euclid–" "Poor kid," Jane said. Holloway shot her a quick glance. "The basis of Euclid. Alphabet blocks. Math, geometry, algebra– they come much later. We're familiar with that development. On the other hand, start the baby with the basic principles of our x logic–" "Blocks? What kind?" Holloway looked at the abacus. "It wouldn't make much sense to us. But we've been conditioned to Euclid." — "Mimsy Were the Borogoves," Lewis Padgett, 1943 |
Padgett (pseudonym of a husband-and-wife writing team) says that alphabet blocks are the intuitive "basis of Euclid." Au contraire; they are the basis of Gutenberg.
For the intuitive basis of one type of non-Euclidean* geometry– finite geometry over the two-element Galois field– see the work of…
Friedrich Froebel
(1782-1852), who
invented kindergarten.
His "third gift" —
© 2005 The Institute for Figuringfom the Inventing Kindergarten
exhibit at The Institute for Figuring
Wednesday, August 30, 2006
Wednesday August 30, 2006
A Multicultural Farewell
to a winner of the
Nobel Prize for Literature,
the Egyptian author of
The Seventh Heaven:
Supernatural Stories —
"Jackson has identified
the seventh symbol."
— Stargate
Other versions of
the seventh symbol —
"… Max Black, the Cornell philosopher, and others have pointed out how 'perhaps every science must start with metaphor and end with algebra, and perhaps without the metaphor there would never have been any algebra' …."
— Max Black, Models and Metaphors, Cornell U. Press, 1962, page 242, as quoted in Dramas, Fields, and Metaphors, by Victor Witter Turner, Cornell U. Press, paperback, 1975, page 25
Friday, May 26, 2006
Friday May 26, 2006
A Living Church
continued from March 27
— G. K. Chesterton
 Shakespearean Fool |
Related material:
Yesterday's entries
and their link to
The Line
as well as
and the remarks
of Oxford professor
Marcus du Sautoy,
who claims that
"the right side of the brain
is responsible for mathematics."
Let us hope that Professor du Sautoy
is more reliable on zeta functions,
his real field of expertise,
than on neurology.
The picture below may help
to clear up his confusion
between left and right.
His confusion about
pseudoscience may not
be so easily remedied.
flickr.com/photos/jaycross/3975200/
(Any resemblance to the film
"Hannibal" is purely coincidental.)
Sunday, November 20, 2005
Sunday November 20, 2005
“There is a pleasantly discursive treatment of Pontius Pilate’s unanswered question ‘What is truth?'”
— H. S. M. Coxeter, 1987, introduction to Richard J. Trudeau’s remarks on the “Story Theory” of truth as opposed to the “Diamond Theory” of truth in The Non-Euclidean Revolution
“A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the ‘Story Theory’ of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called ‘true.’ The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes*….”
— Richard J. Trudeau in
The Non-Euclidean Revolution
“‘Deniers’ of truth… insist that each of us is trapped in his own point of view; we make up stories about the world and, in an exercise of power, try to impose them on others.”
— Jim Holt in The New Yorker.
(Click on the box below.)
Exercise of Power:
Show that a white horse–
a figure not unlike the
symbol of the mathematics
publisher Springer–
is traced, within a naturally
arranged rectangular array of
polynomials, by the powers of x
modulo a polynomial
irreducible over a Galois field.
This horse, or chess knight–
“Springer,” in German–
plays a role in “Diamond Theory”
(a phrase used in finite geometry
in 1976, some years before its use
by Trudeau in the above book).
Related material
On this date:
In 1490, The White Knight
(Tirant lo Blanc 
)–
a major influence on Cervantes–
was published, and in 1910
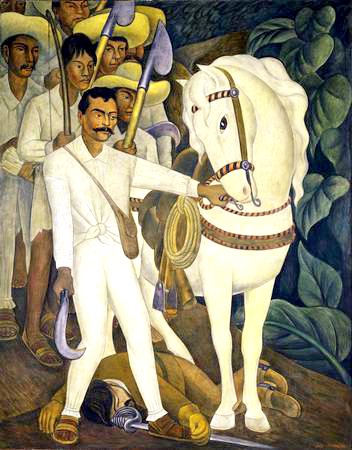
the Mexican Revolution began.
Illustration:
Zapata by Diego Rivera,
Museum of Modern Art,
New York
“First published in the Catalan language in Valencia in 1490…. Reviewing the first modern Spanish translation in 1969 (Franco had ruthlessly suppressed the Catalan language and literature), Mario Vargas Llosa hailed the epic’s author as ‘the first of that lineage of God-supplanters– Fielding, Balzac, Dickens, Flaubert, Tolstoy, Joyce, Faulkner– who try to create in their novels an all-encompassing reality.'”
Monday, January 24, 2005
Monday January 24, 2005
Old School Tie
“We are introduced to John Nash, fuddling flat-footed about the Princeton courtyard, uninterested in his classmates’ yammering about their various accolades. One chap has a rather unfortunate sense of style, but rather than tritely insult him, Nash holds a patterned glass to the sun, [director Ron] Howard shows us refracted patterns of light that take shape in a punch bowl, which Nash then displaces onto the neckwear, replying, ‘There must be a formula for how ugly your tie is.’ ”
have been extremely influential.”– Draft of
Computing with Modal Logics
(pdf), by Carlos Areces
and Maarten de Rijke
“Algebra in general is particularly suited for structuring and abstracting. Here, structure is imposed via symmetries and dualities, for instance in terms of Galois connections……. diamonds and boxes are upper and lower adjoints of Galois connections….”
and Applications: A Survey”
(pdf), by Jules Desharnais,
Bernhard Möller, and
Georg Struth, March 2004
Galois Correspondence
Evariste Galois
“Perhaps every science must
start with metaphor
and end with algebra;
and perhaps without metaphor
there would never have been
any algebra.”
— attributed, in varying forms
(1, 2, 3), to Max Black,
Models and Metaphors, 1962
For metaphor and
algebra combined, see
“Symmetry invariance
in a diamond ring,”
A.M.S. abstract 79T-A37,
Notices of the Amer. Math. Soc.,
February 1979, pages A-193, 194 —
the original version of the 4×4 case
of the diamond theorem.