Sunday, June 4, 2023
“Design is how it works.” — Steve Jobs
Comments Off on “Design is how it works.” — Steve Jobs
Monday, May 15, 2023
Chatbot Review
|
By ChatGPT* —
What is the Cullinane diamond theorem? The Cullinane Diamond Theorem, also known as the Diamond Theorem, is a result in combinatorial mathematics proposed by Steven H. Cullinane. The theorem explores connections between finite geometries, algebraic structures, and combinatorial designs.
The theorem is primarily concerned with four related structures: 2. Vector spaces: The theorem connects finite vector spaces over finite fields to the aforementioned finite geometries. 3. Boolean functions:* The theorem establishes relationships between the finite geometries and certain Boolean functions derived from them.
4. Symmetry groups: The theorem involves symmetry groups associated with the finite geometries. * With one revision by Cullinane: "Boolean functions" replaces "Boolean algebras." See (for instance) M. A. Harrison, “On the classification of Boolean functions by the general linear and affine groups,” Journal of the Society for Industrial and Applied Mathematics 1964 12:2, 285-299. |
Comments Off on Chatbot Review
Saturday, May 13, 2023
Multicultural Memorial: Death and Venice
Variety.com May 8, 2023, 1:45 AM PT —
“Pema Tseden, a famous Tibetan director, screenwriter and
professor at the Film School of the China Academy of Art,
died in Tibet in the early hours of May 8 due to an acute illness."
"The news was reported by the China Academy of Art."
The time in Lhasa, Tibet, is 12 hours ahead of New York time.
From this journal in the afternoon of May 7 (New York time) —
For a relationship between the above image and classic Chinese culture,
see Geometry of the I Ching.
A memorial image from Variety —
Tseden with the award for best screenplay at Venice on Sept. 8, 2018.
See also that date in this journal . . . Posts now tagged Space Structure.
Comments Off on Multicultural Memorial: Death and Venice
Thursday, April 20, 2023
Annals of Artificial Stupidity:
"Google Gone Haywire" Continues.
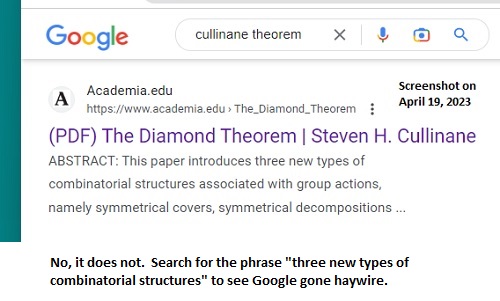
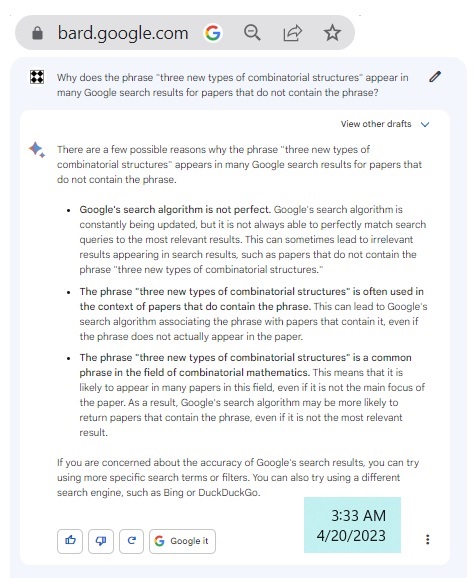
See as well a long complete list of the many Google search results
on combinatorial mathematics that contain the above phrase as
part of a fake "abstract" quoted by Google.
Comments Off on Annals of Artificial Stupidity:
Wednesday, April 19, 2023
Wednesday, March 15, 2023
For Storyholics: Distilled Fire Water
". . . The last of the river diamonds . . . .
bright alluvial diamonds,
burnished clean by mountain torrents,
green and blue and yellow and red.
In the darkness, he could feel them burning,
like fire and water of the universe, distilled."
— At Play in the Fields of the Lord ,
by Peter Matthiessen (Random House, 1965)
Related Log24 posts are now tagged Fire Water.
See as well, from posts tagged Heartland Sutra —
♫ "Red and Yellow, Blue and Green"
— "Prism Song," 1964
Comments Off on For Storyholics: Distilled Fire Water
Saturday, March 4, 2023
Geometry and Death: The Pacific Version
An actor's obituary in The New York Times today suggests
a review of the phrase "geometry and death" in this journal.
In that review, the phrase, by J. G. Ballard in a 2006 article,
refers to German fortifications in World War II. Ballard had
earlier used the same phrase in connection with French
nuclear-test structures in the Pacific —
— From Rushing to Paradise by J. G. Ballard, 1994.
Those interested in the religious meaning of the phrase "Saint-Esprit"
may consult this journal on the date of Ballard's death.
Comments Off on Geometry and Death: The Pacific Version
Thursday, January 19, 2023
Two Approaches to Local-Global Symmetry
Last revised: January 20, 2023 @ 11:39:05
The First Approach — Via Substructure Isomorphisms —
From "Symmetry in Mathematics and Mathematics of Symmetry"
by Peter J. Cameron, a Jan. 16, 2007, talk at the International
Symmetry Conference, Edinburgh, Jan. 14-17, 2007 —
|
Local or global? "Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous * if every isomorphism between finite substructures of M can be extended to an automorphism of M ; in other words, 'any local symmetry is global.' " |
A related discussion of the same approach —
|
"The aim of this thesis is to classify certain structures
— Alice Devillers, |
The Wikipedia article Homogeneous graph discusses the local-global approach
used by Cameron and by Devillers.
For some historical background on this approach
via substructure isomorphisms, see a former student of Cameron:
Dugald Macpherson, "A survey of homogeneous structures,"
Discrete Mathematics , Volume 311, Issue 15, 2011,
Pages 1599-1634.
Related material:
Cherlin, G. (2000). "Sporadic Homogeneous Structures."
In: Gelfand, I.M., Retakh, V.S. (eds)
The Gelfand Mathematical Seminars, 1996–1999.
Gelfand Mathematical Seminars. Birkhäuser, Boston, MA.
https://doi.org/10.1007/978-1-4612-1340-6_2
and, more recently,
Gill et al., "Cherlin's conjecture on finite primitive binary
permutation groups," https://arxiv.org/abs/2106.05154v2
(Submitted on 9 Jun 2021, last revised 9 Jul 2021)
This approach seems to be a rather deep rabbit hole.
The Second Approach — Via Induced Group Actions —
My own interest in local-global symmetry is of a quite different sort.
See properties of the two patterns illustrated in a note of 24 December 1981 —
Pattern A above actually has as few symmetries as possible
(under the actions described in the diamond theorem ), but it
does enjoy, as does patttern B, the local-global property that
a group acting in the same way locally on each part induces
a global group action on the whole .
* For some historical background on the term "homogeneous,"
see the Wikipedia article Homogeneous space.
Comments Off on Two Approaches to Local-Global Symmetry
Saturday, January 14, 2023
Châtelet on Weil — A “Space of Gestures”
|
From Gilles Châtelet, Introduction to Figuring Space Metaphysics does have a catalytic effect, which has been described in a very beautiful text by the mathematician André Weil: Nothing is more fertile, all mathematicians know, than these obscure analogies, these murky reflections of one theory in another, these furtive caresses, these inexplicable tiffs; also nothing gives as much pleasure to the researcher. A day comes when the illusion vanishes: presentiment turns into certainty … Luckily for researchers, as the fogs clear at one point, they form again at another.4 André Weil cuts to the quick here: he conjures these 'murky reflections', these 'furtive caresses', the 'theory of Galois that Lagrange touches … with his finger through a screen that he does not manage to pierce.' He is a connoisseur of these metaphysical 'fogs' whose dissipation at one point heralds their reforming at another. It would be better to talk here of a horizon that tilts thereby revealing a new space of gestures which has not as yet been elucidated and cut out as structure. 4 A. Weil, 'De la métaphysique aux mathématiques', (Oeuvres, vol. II, p. 408.) |
For gestures as fogs, see the oeuvre of Guerino Mazzola.
For some clearer remarks, see . . .
Illustrations of object and gestures
from finitegeometry.org/sc/ —
Object
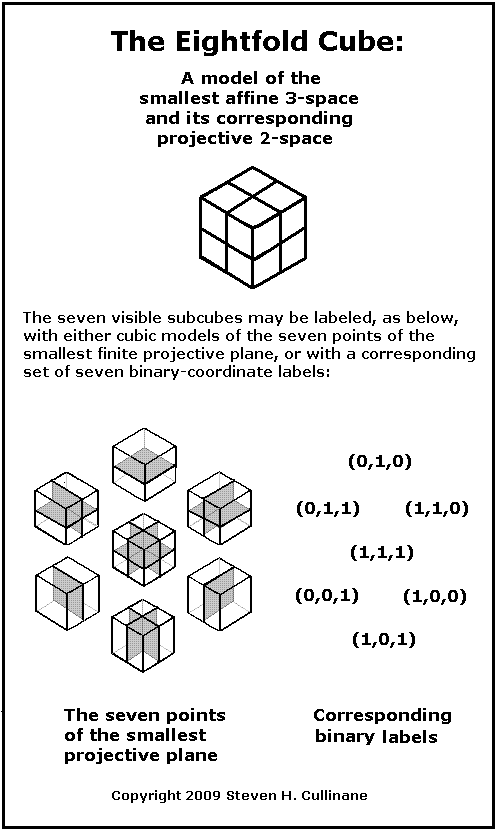
Gestures
An earlier presentation
of the above seven partitions
of the eightfold cube:
|
|
Related material: Galois.space .
Comments Off on Châtelet on Weil — A “Space of Gestures”
Friday, January 13, 2023
LMS Gresham
|
Posted on 13/01/2023 by Peter Cameron My last major job at Queen Mary University of London more than ten years ago was designing and presenting a new first-semester first-year module to be taken by all students on mathematics programmes or joint programmes involving mathematics. I discussed it in my LMS-Gresham lecture. |
LMS
Gresham
"… seeds having fallen on barren rock, as it were" . . .
Comments Off on LMS Gresham
The “Diamond Space” of Mazzola
The Source —
Some similar notions from my own work . . .
The "Digraph" of Mazzola might correspond to a directed graph
indicating the structure of a permutation, as at right below —
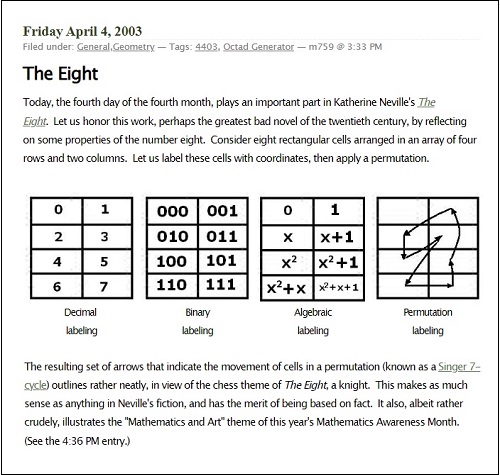
Mazzola's "Formula" might correpond to a matrix and translation that
transform the above "Space" of eight coordinates, and his "Gesture"
to a different way of generating affine transformations of that space . . .
as in my webpage "Cube Space, 1984-2003."
Comments Off on The “Diamond Space” of Mazzola
Monday, December 19, 2022
Mathematics and Narrative, Continued . . .
“Apart from that, Mrs. Lincoln . . .”
Midrash from Philip Pullman . . .
"The 1929 Einstein-Carmichael Expedition"
I prefer the 1929 Emch-Carmichael expedition —
This is from . . .
“By far the most important structure in design theory
is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer
(Ch. 14 (pp. 693-746) of Handbook of Combinatorics,
Vol. I, MIT Press, 1995, edited by Ronald L. Graham,
Martin Grötschel, and László Lovász, Section 16 (p. 716))
Comments Off on Mathematics and Narrative, Continued . . .
“Apart from that, Mrs. Lincoln . . .”
“Apart from that, Mrs. Lincoln . . .”
Saturday, November 26, 2022
Metaphorical Possibilities
"I’m kind of surprised there isn’t a Wisława Szymborska poem or
Tom Stoppard play that explores the metaphorical possibilities
in the Borromean rings."
— Evelyn Lamb in Scientific American , September 30, 2016.
Other mathematical structures also have metaphorical possibilities.
Perhaps not encantado enough.
See Szymborska in this journal in the context of St. Bridget's Day.
Comments Off on Metaphorical Possibilities
Wednesday, November 16, 2022
Monday, November 14, 2022
Primitive Design Theory
The previous post discussed the phrase "plot structure."
A different approach —
Textbook art from 1974 —
See as well a more interesting book I enjoyed reading in 1974.
Comments Off on Primitive Design Theory
Thursday, November 3, 2022
Colorful Appropriation*
(Title suggested by Google News today
and by a Percival Everett short story.}
Comments Off on Colorful Appropriation*
Tuesday, November 1, 2022
From “Goethe on All Souls’ Day”
The above title is that of a Log24 post on St. Cecilia's Day in 2017
that quoted some earlier All Souls' Day remarks from Berlin.
From that post —
Exercise: Explain why the lead article in the November issue of
Notices of the American Mathematical Society misquotes Weyl
and gives the misleading impression that the example above,
the eightfold cube , might be part of the mathematical pursuit
known as geometric group theory .
Background: Earlier instances here of the phrase "geometric group theory."
Comments Off on From “Goethe on All Souls’ Day”
Thursday, October 20, 2022
Déjà Vu All Over Again
Comments Off on Déjà Vu All Over Again
Monday, October 17, 2022
Thursday, October 6, 2022
From Mysticism to Mathematics
[Klein, 1983] S. Klein.
"Analogy and Mysticism and the Structure of Culture
(and Comments & Reply)"
Current Anthropology , 24 (2):151–180, 1983.
The citation above is from a 2017 paper —
"Analogy-preserving Functions:
A Way to Extend Boolean Samples,"
by M. Couceiro, N. Hug, H. Prade, G, Richard.
26th International Joint Conference on Artificial Intelligence
(IJCAI 2017), Aug. 2017, Melbourne, Australia. pp.1-7, ff.
That 2017 paper discusses Boolean functions .
Some more-recent remarks on these functions
as pure mathematics —
"On the Number of Affine Equivalence Classes
of Boolean Functions," by Xiang-dong Hou,
arXiv:2007.12308v2 [math.CO]. Rev. Aug. 18, 2021.
See also other posts now tagged Analogy and Mysticism.
Comments Off on From Mysticism to Mathematics
Thursday, September 29, 2022
The 4×6 Problem*
The exercise posted here on Sept. 11, 2022, suggests a
more precisely stated problem . . .
The 24 coordinate-positions of the 4096 length-24 words of the
extended binary Golay code G24 can be arranged in a 4×6 array
in, of course, 24! ways.
Some of these ways are more geometrically natural than others.
See, for instance, the Miracle Octad Generator of R. T. Curtis.
What is the size of the largest subcode C of G24 that can be
arranged in a 4×6 array in such a way that the set of words of C
is invariant under the symmetry group of the rectangle itself, i.e. the
four-group of the identity along with horizontal and vertical reflections
and 180-degree rotation.
Recent Log24 posts tagged Bitspace describe the structure of
an 8-dimensional (256-word) code in a 4×6 array that has such
symmetry, but it is not yet clear whether that "cube-motif" code
is a Golay subcode. (Its octads are Golay, but possibly not all its
dodecads; the octads do not quite generate the entire code.)
Magma may have an answer, but I have had little experience in
its use.
* Footnote of 30 September 2022. The 4×6 problem is a
special case of a more general symmetric embedding problem.
Given a linear code C and a mapping of C to parts of a geometric
object A with symmetry group G, what is the largest subcode of C
invariant under G? What is the largest such subcode under all
such mappings from C to A?
Comments Off on The 4×6 Problem*
Thursday, September 8, 2022
Analogy in Mathematics: Chevron Variations
André Weil in 1940 on analogy in mathematics —
| . "Once it is possible to translate any particular proof from one theory to another, then the analogy has ceased to be productive for this purpose; it would cease to be at all productive if at one point we had a meaningful and natural way of deriving both theories from a single one. In this sense, around 1820, mathematicians (Gauss, Abel, Galois, Jacobi) permitted themselves, with anguish and delight, to be guided by the analogy between the division of the circle (Gauss’s problem) and the division of elliptic functions. Today, we can easily show that both problems have a place in the theory of abelian equations; we have the theory (I am speaking of a purely algebraic theory, so it is not a matter of number theory in this case) of abelian extensions. Gone is the analogy: gone are the two theories, their conflicts and their delicious reciprocal reflections, their furtive caresses, their inexplicable quarrels; alas, all is just one theory, whose majestic beauty can no longer excite us. Nothing is more fecund than these slightly adulterous relationships; nothing gives greater pleasure to the connoisseur, whether he participates in it, or even if he is an historian contemplating it retrospectively, accompanied, nevertheless, by a touch of melancholy. The pleasure comes from the illusion and the far from clear meaning; once the illusion is dissipated, and knowledge obtained, one becomes indifferent at the same time; at least in the Gitâ there is a slew of prayers (slokas) on the subject, each one more final than the previous ones." |
"The pleasure comes from the illusion" . . .
Exercise:
Compare and contrast the following structure with the three
"bricks" of the R. T. Curtis Miracle Octad Generator (MOG).
Note that the 4-row-2-column "brick" at left is quite
different from the other two bricks, which together
show chevron variations within a Galois tesseract —
Comments Off on Analogy in Mathematics: Chevron Variations
Sunday, September 4, 2022
Dice and the Eightfold Cube
At Hiroshima on March 9, 2018, Aitchison discussed another
"hexagonal array" with two added points… not at the center, as
in the Gell-Mann picture above, but rather at the ends of one of
a cube's four diagonal axes of symmetry.
See some related illustrations below.
Fans of the fictional "Transfiguration College" in the play
"Heroes of the Fourth Turning" may recall that August 6,
another Hiroshima date, was the Feast of the Transfiguration.
The exceptional role of 0 and ∞ in Aitchison's diagram is echoed
by the occurence of these symbols in the "knight" labeling of a
Miracle Octad Generator octad —
Transposition of 0 and ∞ in the knight coordinatization
induces the symplectic polarity of PG(3,2) discussed by
(for instance) Anne Duncan in 1968.
Comments Off on Dice and the Eightfold Cube
Thursday, August 25, 2022
Meditation on a Song Lyric
"I saw a werewolf with a Chinese menu in his hand
Walking through the streets of Soho in the rain"
See other posts now tagged Structure Character.
Comments Off on Meditation on a Song Lyric
Friday, August 19, 2022
The Guy Embedding
M. J. T. Guy discovered that the lexicographic version
of the Golay code contains, embedded within it, the
Miracle Octad Generator (MOG) of R. T. Curtis.
For 12 basis vectors of the lexicographic version, see below.
For some context, click the embedded guy.
For a closely related, but simpler, mathematical
structure, see posts tagged The Omega Matrix.
Comments Off on The Guy Embedding
Monday, August 1, 2022
Interality Again: The Art of the Gefüge
"Schufreider shows that a network of linguistic relations
is set up between Gestalt, Ge-stell, and Gefüge, on the
one hand, and Streit, Riß, and Fuge, on the other . . . ."
— From p. 14 of French Interpretations of Heidegger ,
edited by David Pettigrew and François Raffoul.
State U. of New York Press, Albany, 2008. (Links added.)
One such "network of linguistic relations" might arise from
a non-mathematician's attempt to describe the diamond theorem.
(The phrase "network of linguistic relations" appears also in
Derrida's remarks on Husserl's Origin of Geometry .)
For more about "a system of slots," see interality in this journal.
The source of the above prefatory remarks by editors Pettigrew and Raffoul —
"If there is a specific network that is set up in 'The Origin of the Work of Art,'
a set of structural relations framed in linguistic terms, it is between
Gestalt, Ge-stell and Gefüge, on the one hand, and Streit, Riß and Fuge,
on the other; between (as we might try to translate it)
configuration, frame-work and structure (system), on the one hand, and
strife, split (slit) and slot, on the other. On our view, these two sets go
hand in hand; which means, to connect them to one another, we will
have to think of the configuration of the rift (Gestalt/Riß) as taking place
in a frame-work of strife (Ge-stell/Streit) that is composed through a system
of slots (Gefüge/Fuge) or structured openings."
— Quotation from page 197 of Schufreider, Gregory (2008):
"Sticking Heidegger with a Stela: Lacoue-Labarthe, art and politics."
Pp. 187-214 in David Pettigrew & François Raffoul (eds.),
French Interpretations of Heidegger: An Exceptional Reception.
State University of New York Press, 2008.
Update at 5:14 AM ET Wednesday, August 3, 2022 —
See also "six-set" in this journal.
"There is such a thing as a six-set."
— Saying adapted from a 1962 young-adult novel.
Comments Off on Interality Again: The Art of the Gefüge
Tuesday, July 19, 2022
The Lost Message
“Somehow, a message had been lost on me. Groups act .
The elements of a group do not have to just sit there,
abstract and implacable; they can do things, they can
‘produce changes.’ In particular, groups arise
naturally as the symmetries of a set with structure.”
— Thomas W. Tucker, review of Lyndon’s Groups and Geometry
in The American Mathematical Monthly , Vol. 94, No. 4
(April 1987), pp. 392-394.
"…groups are invariably best studied through their action on some structure…."
— R. T. Curtis, “Symmetric Generation of the Higman-Sims Group” in
Journal of Algebra 171 (1995), pp. 567-586.
Related material — Other posts now tagged Groups Act.
Comments Off on The Lost Message
Tuesday, July 12, 2022
“Tell it Skewb” continues.
Previously on Log24:
"Tell it Skewb." — Motto adapted from Emily Dickinson.
On further investigation . . .
From the above Sanfilippo date —
Comments Off on “Tell it Skewb” continues.
Wednesday, July 6, 2022
Friday, May 27, 2022
Plan 9 from Disney
"With the Tablet of Ahkmenrah and the Cube of Rubik,
my power will know no bounds!"
— Kahmunrah in a novelization of Night at the Museum:
Battle of the Smithsonian , Barron's Educational Series
Scholium —
Abstracting from narrative to structure, and from structure
to pure number, the Tablet of Ahkmenrah represents the
number 9 and the Cube of Rubik represents the number 27.
Returning from pure abstract numbers to concrete representations,
9 yields the structures in Log24 posts tagged Triangle.graphics,
and 27 yields a Galois cube .
Comments Off on Plan 9 from Disney
Sunday, May 22, 2022
Monday, May 16, 2022
Sketch for a Magic Triangle
Updates from later the same day —
Related affine structures —
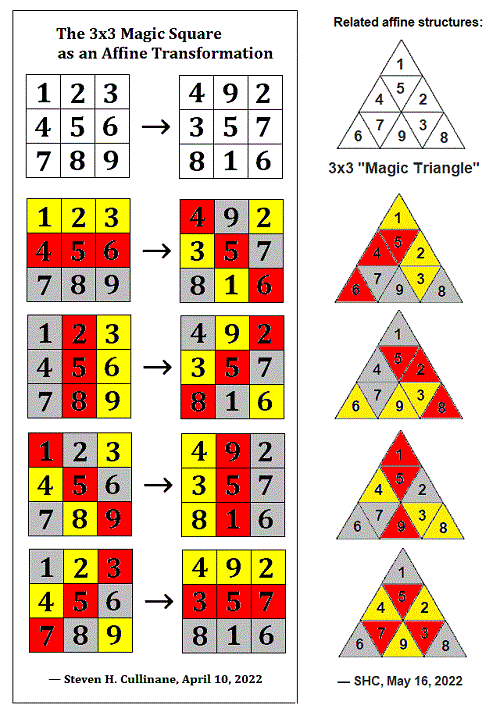
See also "Square+Triangles" in this journal.
The fishlike shapes within three of the above
ninefold colored triangles suggest some . . .
Related Entertainment —
Comments Off on Sketch for a Magic Triangle
Sunday, May 1, 2022
Jailbait Puzzle for Moon Knight
The pane number of interest — 15 or 14 ? —
depends on your perspective.
Related cinematic art of Oscar Isaac —
Comments Off on Jailbait Puzzle for Moon Knight
Sunday, April 10, 2022
Munro Doctrines
The previous post was in memory of one Eleanor Munro.
A different literary Munro —
And then there is Hector Hugh Munro, pen name Saki . . .
See lumber room in this journal (Nov. 30 – Dec. 3, 2016), and
later Ghost Ship tales in a post of December 22, 2016.
Comments Off on Munro Doctrines
Saturday, March 26, 2022
Box Geometry: Space, Group, Art (Work in Progress)
| Name Tag | .Space | .Group | .Art |
|---|---|---|---|
| Box4 |
2×2 square representing the four-point finite affine geometry AG(2,2). (Box4.space) |
S4 = AGL(2,2) (Box4.group) |
(Box4.art) |
| Box6 |
3×2 (3-row, 2-column) rectangular array representing the elements of an arbitrary 6-set. |
S6 | |
| Box8 | 2x2x2 cube or 4×2 (4-row, 2-column) array. | S8 or A8 or AGL(3,2) of order 1344, or GL(3,2) of order 168 | |
| Box9 | The 3×3 square. | AGL(2,3) or GL(2,3) | |
| Box12 | The 12 edges of a cube, or a 4×3 array for picturing the actions of the Mathieu group M12. | Symmetries of the cube or elements of the group M12 | |
| Box13 | The 13 symmetry axes of the cube. | Symmetries of the cube. | |
| Box15 |
The 15 points of PG(3,2), the projective geometry of 3 dimensions over the 2-element Galois field. |
Collineations of PG(3,2) | |
| Box16 |
The 16 points of AG(4,2), the affine geometry of 4 dimensions over the 2-element Galois field. |
AGL(4,2), the affine group of |
|
| Box20 | The configuration representing Desargues's theorem. | ||
| Box21 | The 21 points and 21 lines of PG(2,4). | ||
| Box24 | The 24 points of the Steiner system S(5, 8, 24). | ||
| Box25 | A 5×5 array representing PG(2,5). | ||
| Box27 |
The 3-dimensional Galois affine space over the 3-element Galois field GF(3). |
||
| Box28 | The 28 bitangents of a plane quartic curve. | ||
| Box32 |
Pair of 4×4 arrays representing orthogonal Latin squares. |
Used to represent elements of AGL(4,2) |
|
| Box35 |
A 5-row-by-7-column array representing the 35 lines in the finite projective space PG(3,2) |
PGL(3,2), order 20,160 | |
| Box36 | Eurler's 36-officer problem. | ||
| Box45 | The 45 Pascal points of the Pascal configuration. | ||
| Box48 | The 48 elements of the group AGL(2,3). | AGL(2,3). | |
| Box56 |
The 56 three-sets within an 8-set or |
||
| Box60 | The Klein configuration. | ||
| Box64 | Solomon's cube. |
— Steven H. Cullinane, March 26-27, 2022
Comments Off on Box Geometry: Space, Group, Art (Work in Progress)
Friday, March 18, 2022
Architectural Review
|
"Poincaré said that science is no more a collection of facts than a house is a collection of bricks. The facts have to be ordered or structured, they have to fit a theory, a construct (often mathematical) in the human mind. … Mathematics may be art, but to the general public it is a black art, more akin to magic and mystery. This presents a constant challenge to the mathematical community: to explain how art fits into our subject and what we mean by beauty. In attempting to bridge this divide I have always found that architecture is the best of the arts to compare with mathematics. The analogy between the two subjects is not hard to describe and enables abstract ideas to be exemplified by bricks and mortar, in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics" |
|
Gottschalk Review —
W. H. Gottschalk and G. A. Hedlund, Topological Dynamics, The ending of the review — The most striking virtue of the book is its organization. The authors' effort to arrange the exposition in an efficient order, and to group the results together around a few central topics, was completely successful; they deserve to be congratulated on a spectacular piece of workmanship. The results are stated at the level of greatest available generality, and the proofs are short and neat; there is no unnecessary verbiage. The authors have, also, a real flair for the "right" generalization; their definitions of periodicity and almost periodicity, for instance, are very elegant and even shed some light on the classical concepts of the same name. The same is true of their definition of a syndetic set, which specializes, in case the group is the real line, to Bohr's concept of a relatively dense set. The chief fault of the book is its style. The presentation is in the brutal Landau manner, definition, theorem, proof, and remark following each other in relentless succession. The omission of unnecessary verbiage is carried to the extent that no motivation is given for the concepts and the theorems, and there is a paucity of illuminating examples. The striving for generality (which, for instance, has caused the authors to treat uniform spaces instead of metric spaces whenever possible) does not make for easy reading. The same is true of the striving for brevity; the shortest proof of a theorem is not always the most perspicuous one. There are too many definitions, especially in the first third of the book; the reader must at all times keep at his finger tips a disconcerting array of technical terminology. The learning of this terminology is made harder by the authors' frequent use of multiple statements, such as: "The term {asymptotic } {doubly asymptotic } means negatively {or} {and} positively asymptotic." Conclusion: the book is a mine of information, but you sure have to dig for it. — PAUL R. HALMOS |
Comments Off on Architectural Review
Saturday, March 12, 2022
Geometric Theology: Logos vs. Antilogos
In a 1999 Yale doctoral dissertation,
"Diabolical Structures in the Poetics of Nikolai Gogol,"
the term "antilogos" occurs 70 times.
Students of poetic structures may compare and contrast . . .
Logos
Antilogos
Comments Off on Geometric Theology: Logos vs. Antilogos
Thursday, March 10, 2022
Diabolical Poetics
The title of the previous post suggests a search for
Shubnikov in this journal. That search yields a 1999
Yale doctoral dissertation,
"Diabolical Structures in the Poetics of Nikolai Gogol."
A related image:
From "Made for Love" (2021) — Lyle Herringbone:
Comments Off on Diabolical Poetics
Tuesday, February 1, 2022
Monday, January 24, 2022
Show and Tell in the Heart Sutra
Comments Off on Show and Tell in the Heart Sutra
Sunday, January 23, 2022
Sweet 16th Puzzle
Diamond brackets for enthusiasts of Nolanism —
<span class="meta_txt date">
<time datetime="2022-01-22T01:00:00Z">
Published 2 days ago
</time>
</span>
Related Log24 post —
Comments Off on Sweet 16th Puzzle
Thursday, January 20, 2022
New-Age Trinity
"Taken together, vibe, mood, and energy formed
something like a loose philosophical system.
They presented the world as a swirl of forces
that eluded capture in rational thought, but that
could nevertheless be acutely sensed and even
influenced with the right kind of effort."
— Mitch Therieau in The Drift , Jan. 19, 2022 —
https://www.thedriftmag.com/vibe-mood-energy/ .
See as well Pacific Rimming and Black Sparrow.
Related cinematic lore:
Cailee Spaeny and The Drift in "Pacific Rim: Uprising," as well as . . .
Related tune: "Gimme the Beat Boys."
________________________________________________________
Update of 4:16 PM ET Friday, Jan. 21, 2021 —
From https://dash.harvard.edu/
bitstream/handle/1/39988028/
GRIETZER-DISSERTATION-2017.pdf —
Ambient Meaning: Mood, Vibe, System
A dissertation presented by Peli Grietzer
to The Department of Comparative Literature
in partial fulfillment of the requirements for
the degree of Doctor of Philosophy in
the subject of Comparative Literature,
Harvard University, Cambridge, Massachusetts,
November 2017 —
[Edited to emphasize key notions]
"On the picture that I am suggesting, there exists a reciprocity between
the structure of our sensibility or sensible cognition (system),
the structure of our affective life or social experience (mood),
and the structure of our social-material performance or production (style/vibe)
— a reciprocity whose approximate equilibrium or ‘metastable state’ binds
the cognitive, affective, and material aspects of life into a coherent lifeworld
or ‘totality.’ One way to tell the story of this reciprocity is as follows. The system
of our sensibility—our faculty of sensuous cognition that discloses objects, properties,
and patterns—recapitulates the structure of the social-material world. We continuously
calibrate our sensibility by attuning it to our social-material world’s dominant patterns
and forms, adapting our powers of apperception to the task of navigating our
social-material world." (Pp. 145-146.)
Compare and contrast the following trinities:
Related tune — Meat Loaf at the Ryman, "Two out of three ain't bad."
Comments Off on New-Age Trinity
Sunday, January 2, 2022
Annals of Modernism: UR–Grid
The above New Yorker art illustrates the 2×4 structure of
an octad in the Miracle Octad Generator of R. T. Curtis.
Enthusiasts of simplicity may note how properties of this eight-cell
2×4 grid are related to those of the smaller six-cell 3×2 grid:
See Nocciolo in this journal and . . .
Further reading on the six-set – eight-set relationship:
Comments Off on Annals of Modernism: UR–Grid
Saturday, January 1, 2022
Clean Lines
Comments Off on Clean Lines
Friday, December 31, 2021
Aesthetics in Academia
Related art — The non-Rubik 3x3x3 cube —
The above structure illustrates the affine space of three dimensions
over the three-element finite (i.e., Galois) field, GF(3). Enthusiasts
of Judith Brown's nihilistic philosophy may note the "radiance" of the
13 axes of symmetry within the "central, structuring" subcube.
I prefer the radiance (in the sense of Aquinas) of the central, structuring
eightfold cube at the center of the affine space of six dimensions over
the two-element field GF(2).
Comments Off on Aesthetics in Academia
Saturday, December 11, 2021
News for Enthusiasts
"Enthusiasts of group theory or incidence structures may enjoy reading about Tits'
work, such as Tits buildings, the Tits alternative, the Tits group, and the Tits metric."
— Annie Rauwerda, Boing Boing reporter. See also Tits in this journal.
Comments Off on News for Enthusiasts
Friday, December 3, 2021
Art Memorial
Comments Off on Art Memorial
Tuesday, September 7, 2021
Raiders of the Lost Symbol … Continues*
A Log24 search for "Watercourse" leads to . . .
("Watercourse" is in the Customer review link.)
The "five years ago" link leads to . . .
|
"What modern painters are trying to do,
— James J. Gibson in Leonardo An example of invariant structure:
The three line diagrams above result from the three partitions, into pairs of 2-element sets, of the 4-element set from which the entries of the bottom colored figure are drawn. Taken as a set, these three line diagrams describe the structure of the bottom colored figure. After coordinatizing the figure in a suitable manner, we find that this set of three line diagrams is invariant under the group of 16 binary translations acting on the colored figure. A more remarkable invariance — that of symmetry itself — is observed if we arbitrarily and repeatedly permute rows and/or columns and/or 2×2 quadrants of the colored figure above. Each resulting figure has some ordinary or color-interchange symmetry. This sort of mathematics illustrates the invisible "form" or "idea" behind the visible two-color pattern. Hence it exemplifies, in a way, the conflict described by Plato between those who say that "real existence belongs only to that which can be handled" and those who say that "true reality consists in certain intelligible and bodiless forms." |
* See that title in this journal.
Comments Off on Raiders of the Lost Symbol … Continues*
Tuesday, August 10, 2021
Discursive
The structure in the previous post (three trios), though historically significant,
offers less opportunity for contrapuntal variation than . . .
Related remarks for Pleasantly Discursive Day —
Comments Off on Discursive
Monday, August 9, 2021
The Tune (Suggested by “Hum: Seek the Void”)
"Two years ago . . . ." — Synopsis of the August 3 film "Hum"
Two years ago on August 3 . . .
What is going on in this picture?
The above is an image from
the August 3, 2019,
post "Butterfield's Eight."
"Within the week . . . ."
— The above synopsis of "Hum"
This suggests a review of a post
from August 5, 2019, that might
be retitled . . .
"The void she knows,
the tune she hums."
Comments Off on The Tune (Suggested by “Hum: Seek the Void”)
Friday, July 30, 2021
Central Objects
Cover illustration:
Spies returning from the land of
Canaan with a cluster of grapes.
Comments Off on Central Objects
Wednesday, July 28, 2021
From the Krell Lab
“… Which makes it a gilt-edged priority that one of us
gets into that Krell lab and takes that brain boost.”
— American adaptation of Shakespeare’s Tempest , 1956
|
“Propriation1 gathers the rift-design2 of the saying
— p. 415 of Heidegger‘s Basic Writings ,
“Das Ereignis versammelt den Aufriß der Sage — Heidegger, Weg zur Sprache 1. “Mirror-Play of the Fourfold” |
Comments Off on From the Krell Lab
Tuesday, July 27, 2021
Dancing in the Moonlight
Instagram screenshot with added note.
Easy E for an Accountant:
Not So Easy: E-Operators
"A great many other properties of E-operators
have been found, which I have not space
to examine in detail."
— Sir Arthur Eddington, New Pathways in Science ,
Cambridge University Press, 1935, page 271.
(This book also presents Eddington's unfortunate
speculations on the fine-structure constant.)
Update of 4:04 AM ET:
Here is the not-so-tiny-dancer in
the above Instagram screenshot.
Comments Off on Dancing in the Moonlight
Tuesday, May 18, 2021
Nelson’s Monument
See as well . . .
http://cs.brown.edu/memex/ACM_HypertextTestbed/papers/64.html .
This post was suggested by an Arts & Letters Daily link today to . . .
https://placesjournal.org/article/
the-filing-cabinet-and-20th-century-information-infrastructure/ .
A particularly notable filing cabinet:
Comments Off on Nelson’s Monument
Friday, March 26, 2021
ABC Art
Some images from Feb. 5, 2021, in a search for "ABC Art" —
A colored version using CSS —
See https://codepen.io/m759/pen/wvoGwzx .
“Somehow, a message had been lost on me. Groups act .
The elements of a group do not have to just sit there,
abstract and implacable; they can do things, they can
‘produce changes.’ In particular, groups arise
naturally as the symmetries of a set with structure.”
— Thomas W. Tucker, review of Lyndon’s Groups and Geometry
in The American Mathematical Monthly , Vol. 94, No. 4
(April 1987), pp. 392-394.
Comments Off on ABC Art
Friday, March 19, 2021
Eye of Cat
|
Changing Woman: “Kaleidoscope turning…
Shifting pattern — Roger Zelazny, Eye of Cat |
Comments Off on Eye of Cat
Monday, March 15, 2021
The Abstract Signature
Caption: "I notice the signatures are never abstract." —
Abstract Art
Abstract Signature
From Encyclopedia of Mathematics —
Comments Off on The Abstract Signature
Thursday, March 11, 2021
General Terms
"The puzzle in general terms is one of structure ."
— J. Robert Oppenheimer, page 122,
Life Magazine , Oct. 10, 1949
The term "puzzle" may be misleading.
A more serious structure —
Click the above images for further details.
Comments Off on General Terms
Thursday, March 4, 2021
Continuity
Actually, Dirac “bridged math and physics” much earlier —
“Spinors, which are a kind of square root of vectors, had been introduced
in algebra and also in physics as part of Paul Dirac’s theory of the electron.
A spin structure on a manifold allows such square roots to exist.”
— Quanta Magazine today, article by Daniel S. Freed
See The Eddington Song and . . .
Poetic paraphrase —
“How can we tell the singer from the song?”
Comments Off on Continuity
Saturday, February 27, 2021
The Pencil Case
Clue
Here is a midrash on “desmic,” a term derived from the Greek desmé
( δέσμη: bundle, sheaf , or, in the mathematical sense, pencil —
French faisceau ), which is related to the term desmos , bond …
(The term “desmic,” as noted earlier, is relevant to the structure of
Heidegger’s Sternwürfel .)
“Gadzooks, I’ve done it again!” — Sherlock Hemlock
Comments Off on The Pencil Case
Wednesday, February 24, 2021
Annals of Dim Antiquity
“Twenty-four glyphs, each one representing not a letter, not a word,
but a concept, arranged into four groups, written in Boris’s own hand,
an artifact that seemed to have resurrected him from the dead. It was
as if he were sitting across from Bourne now, in the dim antiquity of
the museum library.
This was what Bourne was staring at now, written on the unfolded
bit of onionskin.”
— The Bourne Enigma , published on June 21, 2016
Passing, on June 21, 2016, into a higher dimension —
For those who prefer Borges to Bourne —
Comments Off on Annals of Dim Antiquity
Wednesday, January 27, 2021
Game of Royalties
For Holocaust Remembrance Day —
Little reportedly died at 79 on Jan. 7.
“Mr. Little submitted the manuscript for ‘Harry Potter and the Philosopher’s Stone’
to 12 publishers. He received 12 rejections in response, before selling it for £2,500,
or about $3,400 (the equivalent of about $5,800 today). It was a meager amount,
but his genius was in the details: He sold only the rights to publish it in Britain and
the Commonwealth, and he asked for high royalties.” — Clay Risen, New York Times
Leachman reportedly died at 94 today.
Dr. Frederick Frankenstein : And it was you… who left my grandfather’s
book out for me to find.
Frau Blücher : Yes.
Dr. Frederick Frankenstein : So that I would…
Frau Blücher : Yes.
Dr. Frederick Frankenstein : Then you and Victor were…
Frau Blücher : YES. YES. Say it. He vas my… BOYFRIEND!
In the spirit of Kinbote…
The real Frau Blücher was of course Hannah Arendt,
whose boyfriend was Martin Heidegger.
Cf. a Log24 post of April 10, 2017 —
| From “Heidegger for Passover”
“Propriation1 gathers the rift-design2 of the saying — p. 415 of Heidegger‘s Basic Writings , “Das Ereignis versammelt den Aufriß der Sage — Heidegger, Weg zur Sprache 1. “Mirror-Play of the Fourfold” |
Comments Off on Game of Royalties
Wednesday, January 13, 2021
Revision
From a post of January 8, 2021 —
“Somehow, a message had been lost on me. Groups act .
The elements of a group do not have to just sit there,
abstract and implacable; they can do things, they can
‘produce changes.’ In particular, groups arise
naturally as the symmetries of a set with structure.”
— Thomas W. Tucker, review of Lyndon’s Groups and Geometry
in The American Mathematical Monthly , Vol. 94, No. 4
(April 1987), pp. 392-394.
Note:
The phrase “the subgroup V” here refers, confusingly, to the translation
subgroup derived from a vector space V.
Comments Off on Revision
Saturday, January 9, 2021
A Sense
“In a sense, we would see that change arises from
the structure of the object.”
— Nima Arkani-Hamed, quoted in
“A Jewel at the Heart of Quantum Physics,”
by Natalie Wolchover, Quanta Magazine , Sept. 17, 2013.
For those who prefer less barbaric entertainment —
Comments Off on A Sense
Friday, January 8, 2021
Groups Act
"Somehow, a message had been lost on me. Groups act .
The elements of a group do not have to just sit there,
abstract and implacable; they can do things, they can
'produce changes.' In particular, groups arise
naturally as the symmetries of a set with structure."
— Thomas W. Tucker, review of Lyndon's Groups and Geometry
in The American Mathematical Monthly , Vol. 94, No. 4
(April 1987), pp. 392-394.
"The concept of group actions is very useful in the study of
isomorphisms of combinatorial structures."
— Olli Pottonen, "Classification of Steiner Quadruple Systems"
(Master's thesis, Helsinki, 2005, p. 48).
“In a sense, we would see that change arises from
the structure of the object.”
— Nima Arkani-Hamed, quoted in "A Jewel at the Heart of
Quantum Physics," by Natalie Wolchover, Quanta Magazine ,
Sept. 17, 2013.
See as well "Change Arises" in this journal.
Comments Off on Groups Act
Monday, December 28, 2020
Theology for the Wiener Kreis
The previous post suggests a look at The New Yorker today —
Another “core claim” —
“Change arises from the structure of the object.“
See also Wiener Kreis and Schlick.
Comments Off on Theology for the Wiener Kreis
Thursday, December 24, 2020
Change Arises
See posts so tagged.
"Change arises from the structure of the object." — Arkani-Hamed
Related material from 1936 —
Related material from 1905, with the "object" a 4×4 array —
Related material from 1976, with the "object"
a 4×6 array — See Curtis.
Related material from 2018, with the "object"
a cuboctahedron — See Aitchison.
Comments Off on Change Arises
Thursday, November 19, 2020
Set Design and the Schoolgirl Problem
Underlying Structure of the Design —
Schoolgirl Problem —
Comments Off on Set Design and the Schoolgirl Problem
Wednesday, September 23, 2020
Recently Acquired Kindle Books
Related Log24 remarks:
Yoda Quilts and posts now tagged Central Myth.
Related remarks elsewhere:
“In The Uncanny Nicholas Royle defined Freud’s Unheimlichkeit
and the experience of an ‘unreal reality’ as ‘another thinking of
beginning’. But if we are to take him at his word, ‘the beginning
is already haunted’ and we may wish to interpret his debut novel
Quilt as spectrally haunted by the critic’s earlier theory. The essay,
which is structured telephonically, since it refers both to Royle’s
view of literature as telepathy (i.e. another form of ‘tele-‘) and the
beginning of the novel, reads Quilt from its ‘Afterward’, to unveil
two main ghosts haunting Royle’s novel: that of Jacques Derrida
and that of James Joyce.”
—Arleen Ionescu, abstract of a 2013 essay on Royle’s Quilt .
Comments Off on Recently Acquired Kindle Books
Friday, September 18, 2020
Adoration of the Cube
“WHEN I IMAGINE THE CUBE, I see a structure in motion.
I see the framework of its edges, its corners, and its flexible joints,
and the continuous transformations in front of me (before you start
to worry, I assure you that I can freeze it anytime I like). I don’t see
a static object but a system of dynamic relations. In fact, this is only
half of that system. The other half is the person who handles it.
Just like everything else in our world, a system is defined by
its place within a network of relations—to humans, first of all.”
— Rubik, Erno. Cubed (p. 165). Flatiron Books. Kindle Ed., 2020.
Compare and contrast — Adoration of the Blessed Sacrament.
Comments Off on Adoration of the Cube
Monday, September 7, 2020
A Discovery of Species
From the subtitles to “A Discovery of Witches,”
Season 1, Episode 2 —
An actor playing a contemporary (2018) fictional Oxford professor —
378
00:35:54,235 –> 00:35:56,593
We’re among hundreds of laboratories
using genetics
379
00:35:56,595 –> 00:35:59,713
to study species origin,
but in our lab
380
00:35:59,715 –> 00:36:02,315
humans aren’t the only species
we’re studying.
An earlier non-fictional Oxford student writes —
Related material: Other posts tagged Structure and Mutability.
Comments Off on A Discovery of Species
Thursday, July 30, 2020
A Picture Show for Quanta Magazine
An article yesterday at Quanta Magazine suggests a review . . .
From Diamond Theorem images at Pinterest —
Some background —
Comments Off on A Picture Show for Quanta Magazine
Wednesday, July 29, 2020
Duren, Not Durin
A flashback from Log24 posts of July 9-11, 2020,
now tagged Structure and Mutability —
For such temptation, see
Dwarves named “Durin.”
Comments Off on Duren, Not Durin
Tuesday, July 28, 2020
Friday, July 17, 2020
The Resurrection Artifact
From Log24 posts tagged Structure and Mutability —
“… an artifact that seemed to have resurrected him from the dead.”
— “Robert Ludlum’s” The Bourne Enigma , published on June 21, 2016
See as well the 2020 film Archive , and the related 2018 film Replicas
in Oslo Variations.
Comments Off on The Resurrection Artifact
Poetic as Well as Prosaic
Prosaic —
Poetic —
Prosaic —
“These devices may have some
theoretical as well as practical value.“
Poetic —
Comments Off on Poetic as Well as Prosaic
Wednesday, July 15, 2020
Category Theory
A related quotation:
“By far the most important structure in design theory
is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer
(Ch. 14 (pp. 693-746) of Handbook of Combinatorics,
Vol. I, MIT Press, 1995, edited by Ronald L. Graham,
Martin Grötschel, and László Lovász, Section 16 (p. 716))
See also the webpage Block Designs in Art and Mathematics
and Log24 posts tagged Plastic Elements.
Comments Off on Category Theory
Saturday, July 11, 2020
Philosophy for Murdoch Fans
The previous post contained a passage from Iris Murdoch’s
1961 essay “Against Dryness.” Some related philosophy —
For those who prefer pure mathematics to philosophical ruminations
there are some relevant remarks in my webpage of August 27, 2003.
Comments Off on Philosophy for Murdoch Fans
Thursday, July 9, 2020
The Enigma Glyphs
For those who prefer fiction —
“Twenty-four glyphs, each one representing not a letter, not a word,
but a concept, arranged into four groups, written in Boris’s own hand,
an artifact that seemed to have resurrected him from the dead. It was
as if he were sitting across from Bourne now, in the dim antiquity of
the museum library.
This was what Bourne was staring at now, written on the unfolded
bit of onionskin.”
— “Robert Ludlum’s” The Bourne Enigma , published on June 21, 2016
Passing, on June 21, 2016, into a higher dimension —
Comments Off on The Enigma Glyphs
Monday, June 15, 2020
“The Thing and I” Continues*
“For the first thirty years of its history, Columbia was known as King’s College.”
— History of the University Identity
Hence the crown favicon—
“When people talk about the importance of the study of ‘symmetry’
in mathematics, physics, and elsewhere, they often make the mistake
of only paying attention to the symmetry groups. The structure you
actually have is not just a group (the abstract ‘symmetries’), but an
action of that group on some other object, the thing that has symmetries.”
— Peter Woit of Columbia on June 9, reviewing a Quanta Magazine article
* From earlier posts in this journal containing the title phrase.
Comments Off on “The Thing and I” Continues*
Tuesday, June 2, 2020
Monday, May 25, 2020
Cyberface
(A sequel to D8ing the Joystick)
Adam Gopnik today in The New Yorker —
“In remote therapy sessions, with the loss of familiarly structured
therapeutic spaces, a kind of staring contest takes place.”
This journal on the above YouTube date — May 28, 2011 —
“Two things of opposite natures seem to depend
On one another, as a man depends
On a woman, day on night, the imagined
On the real. This is the origin of change.
Winter and spring, cold copulars, embrace
And forth the particulars of rapture come.”
— Wallace Stevens,
“Notes Toward a Supreme Fiction,”
Canto IV of “It Must Change”
Update of 5:45 PM ET —
The above May 28, 2011, Stevens quotation is from a post
titled “Savage Detectives.” A related image starring Sean Young —
Comments Off on Cyberface
Wednesday, May 20, 2020
Scholarly History
On “emergence, institutionalization and (importantly) legitimation …
(with its resource allocation system and authority structure)” —
“It’s still the same old story.” — Song lyric
See as well other posts now tagged Raiding Minsky’s.
Comments Off on Scholarly History
Monday, April 27, 2020
The Cracked Nut
“At that instant he saw, in one blaze of light, an image of unutterable
conviction, the reason why the artist works and lives and has his being –
the reward he seeks –the only reward he really cares about, without which
there is nothing. It is to snare the spirits of mankind in nets of magic,
to make his life prevail through his creation, to wreak the vision of his life,
the rude and painful substance of his own experience, into the congruence
of blazing and enchanted images that are themselves the core of life, the
essential pattern whence all other things proceed, the kernel of eternity.”
— Thomas Wolfe, Of Time and the River
“… the stabiliser of an octad preserves the affine space structure on its
complement, and (from the construction) induces AGL(4,2) on it.
(It induces A8 on the octad, the kernel of this action being the translation
group of the affine space.)”
— Peter J. Cameron,
The Geometry of the Mathieu Groups (pdf)
“The yarns of seamen have a direct simplicity, the whole meaning
of which lies within the shell of a cracked nut. But Marlow was not
typical (if his propensity to spin yarns be excepted), and to him the
meaning of an episode was not inside like a kernel but outside…."
— Joseph Conrad in Heart of Darkness
Comments Off on The Cracked Nut
Monday, April 20, 2020
Geometry for Poets
From Atomicity and Quanta by James Jeans,
Cambridge University Press, 1926, pp. 55-56 —
| “So far as we can at present conjecture, the investigation of the structure which produces this atomicity appears to be the big problem in the path of the quantum-theory. To conform to the principle of relativity, the new atomicity must admit of expression in terms of the space-time continuum, although we have seen that it cannot be an atomicity of the continuum itself. It may conceivably be an atomicity of its metric properties, such as determine its curvatures. We may perhaps form a very rude picture of it by imagining the curvature of the continuum in the neighbourhood of an atom not to be of the continuous nature imagined by Weyl, but to occur in finite chunks—a straight piece, then a sudden bend, then another straight bit, and so on. A small bit of the continuum viewed through a five-dimensional microscope might look rather like a cubist picture; and, conversely, perhaps a cubist picture looks rather more like a little fragment of the continuum than like anything else.” |
This is, of course, not the “atomicity” of the previous post.
For examples of that atomicity, a concept of pure geometry
rather than of physics, see …
Faure, C. A., and Frölicher, A., “Fundamental Notions of
Lattice Theory,” in Modern Projective Geometry (2000).
(Mathematics and Its Applications, vol 521. Springer, Dordrecht.)
Related art (a “cubist picture”) —
Juan Gris, Fruit Dish and Carafe , 1914
Comments Off on Geometry for Poets
Thursday, March 12, 2020
Tuesday, March 10, 2020
Labeling a Cuboctahedron
The above arrangement of graphic images on cube faces is purely
decorative and static, and of little mathematical interest.
(A less static, but structurally chaotic, artifact might be made by
pasting the above 24 graphic images in the "Cosets in S4" picture
above onto the 24 faces of a 2x2x2 Rubik cube. This suggests the
reflection below on the poet Wallace Stevens, whose "Connoisseur
of Chaos" first appeared on page 90 of Twentieth Century Verse ,
Numbers 12-13, October 1938.)
If mathematically interesting permutations of the graphic images
are to be done, the images should be imagined as situated on
parallel planes, as in the permutahedron below —
Click the above permutahedron for an analysis of its structure.
Comments Off on Labeling a Cuboctahedron
Thursday, February 27, 2020
Occult Writings
From the author who in 2001 described "God's fingerprint"
(see the previous post) —
From the same publisher —
From other posts tagged Triskele in this journal —
Other geometry for enthusiasts of the esoteric —
|
Monday, November 4, 2019
As Above, So Below*
|
|
|
Comments Off on Occult Writings
Wednesday, February 12, 2020
The Reality Bond
The plane at left is modeled naturally by
seven types of “cuts” in the cube at right.
Comments Off on The Reality Bond
Monday, February 3, 2020
A Kuhnian Register
Ereignis in the Stanford Encyclopedia of Philosophy —
|
Further aspects of the essential unfolding of Being are revealed by what is perhaps the key move in the Contributions—a rethinking of Being in terms of the notion of Ereignis, a term translated variously as ‘event’ (most closely reflecting its ordinary German usage), ‘appropriation’, ‘appropriating event’, ‘event of appropriation’ or ‘enowning’. (For an analysis which tracks Heidegger's use of the term Ereignis at various stages of his thought, see Vallega-Neu 2010). The history of Being is now conceived as a series of appropriating events in which the different dimensions of human sense-making—the religious, political, philosophical (and so on) dimensions that define the culturally conditioned epochs of human history—are transformed. Each such transformation is a revolution in human patterns of intelligibility, so what is appropriated in the event is Dasein and thus the human capacity for taking-as (see e.g., Contributions 271: 343). Once appropriated in this way, Dasein operates according to a specific set of established sense-making practices and structures. In a Kuhnian register, one might think of this as the normal sense-making that follows a paradigm-shift. — Michael Wheeler, 2011 |
See as well "reordering" in Sunday evening's post Tetrads for McLuhan
and in a Log24 search for Reordering + Steiner.
Comments Off on A Kuhnian Register
Sunday, January 26, 2020
Duke Blocks
The Wall Street Journal Jan. 24 on a Duke University professor —
"Dr. Daubechies is best known for her work on mathematical structures
called wavelets; her discoveries have been so influential, in fact, that
these are referred to in the field as Daubechies wavelets. She describes
them as 'mathematical building blocks' that can be used to extract the
essential elements of images or signals without losing their quality—
in effect, a new universal language for scientists and researchers."
See also this journal on January 20-21, and …
Comments Off on Duke Blocks
Sunday, January 19, 2020
For 6 Prescott Street*
"Freshman Seminar Program Department Administrator Corinna S. Rohse
described the program’s courses, which allow students to study subjects
that vary from Sanskrit to the mathematical basis for chess, as
'jewel-like: small and incredibly well-cut.' "
— The Harvard Crimson , Dec. 10, 2008
For remarks related to Sanskrit, chessboard structure, and "jewel-like"
mathematics, see A Prince of Darkness (Log24, March 28, 2006).
See also Walsh Functions in this journal and …
Lecture notes on dyadic harmonic analysis
(Cuernavaca, 2000)
Compare and contrast these remarks of Pereyra with the following
remarks, apparently by the same Corinna S. Rohse quoted above.
* Location of the Harvard Freshman Seminar program in the 2008
article above. The building at 6 Prescott was moved there from
5 Divinity Avenue in 1978. When the seminar program was started
in the fall of 1959, it was located in a house at 8 Prescott St. (In
1958-1959 this was a freshman dorm, the home of Ted Kaczynski.)
Comments Off on For 6 Prescott Street*
Wednesday, January 15, 2020
Paradigm Shift
Illustration, from a search in this journal for “Symplectic” —
.
Some background: Rift-design in this journal and …
Comments Off on Paradigm Shift
Thursday, January 2, 2020
Saturday, December 14, 2019
Colorful Tale
The above image is from
"A Four-Color Theorem:
Function Decomposition Over a Finite Field,"
http://finitegeometry.org/sc/gen/mapsys.html.
These partitions of an 8-set into four 2-sets
occur also in Wednesday night's post
Miracle Octad Generator Structure.
This post was suggested by a Daily News
story from August 8, 2011, and by a Log24
post from that same date, "Organizing the
Mine Workers" —
Comments Off on Colorful Tale
Friday, November 15, 2019
Operators
Easy E
Not So Easy: E-Operators
"A great many other properties of E-operators
have been found, which I have not space
to examine in detail."
— Sir Arthur Eddington, New Pathways in Science ,
Cambridge University Press, 1935, page 271.
(This book also presents Eddington's unfortunate
speculations on the fine-structure constant.)
Comments Off on Operators
Sunday, October 13, 2019
Langer
"Visual forms— lines, colors, proportions, etc.—
are just as capable of articulation ,
i.e. of complex combination, as words.
But the laws that govern this sort of articulation
are altogether different from the laws of syntax
that govern language. The most radical difference
is that visual forms are not discursive .
They do not present their constituents successively,
but simultaneously, so the relations determining
a visual structure are grasped in one act of vision."
— Susanne K. Langer, Philosophy in a New Key
Comments Off on Langer
Friday, October 11, 2019
Quest
John Horgan in Scientific American magazine on October 8, 2019 —
"In the early 1990s, I came to suspect that the quest
for a unified theory is religious rather than scientific.
Physicists want to show that all things came from
one thing: a force, or essence, or membrane
wriggling in eleven dimensions, or something that
manifests perfect mathematical symmetry. In their
search for this primordial symmetry, however,
physicists have gone off the deep end . . . ."
Other approaches —
See "Story Theory of Truth" in this journal and, from the November 2019
Notices of the American Mathematical Society . . .
|
More fundamental than the label of mathematician is that of human. And as humans, we’re hardwired to use stories to make sense of our world (story-receivers) and to share that understanding with others (storytellers) [2]. Thus, the framing of any communication answers the key question, what is the story we wish to share? Mathematics papers are not just collections of truths but narratives woven together, each participating in and adding to the great story of mathematics itself. The first endeavor for constructing a good talk is recognizing and choosing just one storyline, tailoring it to the audience at hand. Should the focus be on a result about the underlying structures of group actions? . . . .
[2] Gottschall, J. , The Storytelling Animal , — "Giving Good Talks," by Satyan L. Devadoss |
"Before time began, there was the Cube." — Optimus Prime
Comments Off on Quest
Tuesday, September 24, 2019
Emissary
|
Thursday, September 12, 2019
Tetrahedral Structures
|
Playing with shapes related to some 1906 work of Whitehead:
Comments Off on Emissary
Sunday, September 22, 2019
Colorful Tale
“Perhaps the philosophically most relevant feature of modern science
is the emergence of abstract symbolic structures as the hard core
of objectivity behind— as Eddington puts it— the colorful tale of
the subjective storyteller mind.”
— Hermann Weyl, Philosophy of Mathematics and
Natural Science , Princeton, 1949, p. 237
"The bond with reality is cut."
— Hans Freudenthal, 1962
From page 180, Logicomix — It was a dark and stormy night …
Comments Off on Colorful Tale
Friday, August 30, 2019
Saturday, August 17, 2019
Crystalline Complexity
Burroway on Hustvedt in The New York Times ,
Sunday, March 9, 2003 —
See as well "Putting the Structure in Structuralism."
Comments Off on Crystalline Complexity
Friday, August 16, 2019
Nocciolo
A revision of the above diagram showing
the Galois-addition-table structure —
Related tables from August 10 —
See "Schoolgirl Space Revisited."
Comments Off on Nocciolo
Thursday, August 15, 2019
On Steiner Quadruple Systems of Order 16
An image from a Log24 post of March 5, 2019 —
The following paragraph from the above image remains unchanged
as of this morning at Wikipedia:
"A 3-(16,4,1) block design has 140 blocks of size 4 on 16 points,
such that each triplet of points is covered exactly once. Pick any
single point, take only the 35 blocks containing that point, and
delete that point. The 35 blocks of size 3 that remain comprise
a PG(3,2) on the 15 remaining points."
Exercise —
Prove or disprove the above assertion about a general "3-(16,4,1)
block design," a structure also known as a Steiner quadruple system
(as I pointed out in the March 5 post).
Relevant literature —
A paper from Helsinki in 2005* says there are more than a million
3-(16,4,1) block designs, of which only one has an automorphism
group of order 322,560. This is the affine 4-space over GF(2),
from which PG(3,2) can be derived using the well-known process
from finite geometry described in the above Wikipedia paragraph.
* "The Steiner quadruple systems of order 16," by Kaski et al.,
Journal of Combinatorial Theory Series A Volume 113, Issue 8,
November 2006, pages 1764-1770.
Comments Off on On Steiner Quadruple Systems of Order 16
Tuesday, August 6, 2019
Tuesday, July 16, 2019
What’s in a Name: Boeing/Being
See also Visual Structure (May 22, 2014)
and Snakes on a Plane posts.
Comments Off on What’s in a Name: Boeing/Being
Sunday, July 14, 2019
Old Pathways in Science:
The Quantum Tesseract Theorem Revisited
"The secret is that the super-mathematician expresses by the anticommutation
of his operators the property which the geometer conceives as perpendicularity
of displacements. That is why on p. 269 we singled out a pentad of anticommuting
operators, foreseeing that they would have an immediate application in describing
the property of perpendicular directions without using the traditional picture of space.
They express the property of perpendicularity without the picture of perpendicularity.
Thus far we have touched only the fringe of the structure of our set of sixteen E-operators.
Only by entering deeply into the theory of electrons could I show the whole structure
coming into evidence."
A related illustration, from posts tagged Dirac and Geometry —
Compare and contrast Eddington's use of the word "perpendicular"
with a later use of the word by Saniga and Planat.
Comments Off on Old Pathways in Science:
Monday, July 1, 2019
Inside the Exploded Cube
Metaphysical conceit | literature | Britannica.com
|
This post's title refers to a metaphysical conceit
in the previous post, Desperately Seeking Clarity.
Related material —
The source of the above mystical octahedron —
See also Jung's Imago Dei in this journal.
Comments Off on Inside the Exploded Cube
Thursday, June 20, 2019
Tuesday, May 28, 2019
Quaternion at Candlebrow
From a Groundhog Day post in 2009 —
|
The Candlebrow Conference The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries. "Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–"
"Um, Professor–"…. … Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"…. … In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions…. "Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions." "Build a time machine." "Not the way I would have put it, but if you like, fine." Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up…. "We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly." "Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened. Above, the devastation had begun. |
"As if the professor had lectured it into being . . . ."
See other posts now tagged McLuhan Time.
Comments Off on Quaternion at Candlebrow
Sunday, May 19, 2019
The Building Blocks of Geometry
From "On the life and scientific work of Gino Fano"
by Alberto Collino, Alberto Conte, and Alessandro Verra,
ICCM Notices , July 2014, Vol. 2 No. 1, pp. 43-57 —
|
" Indeed, about the Italian debate on foundations of Geometry, it is not rare to read comments in the same spirit of the following one, due to Jeremy Gray13. He is essentially reporting Hans Freudenthal’s point of view: ' When the distinguished mathematician and historian of mathematics Hans Freudenthal analysed Hilbert’s Grundlagen he argued that the link between reality and geometry appears to be severed for the first time in Hilbert’s work. However, he discovered that Hilbert had been preceded by the Italian mathematician Gino Fano in 1892. . . .' " 13 J. Gray, "The Foundations of Projective Geometry in Italy," Chapter 24 (pp. 269–279) in his book Worlds Out of Nothing , Springer (2010). |
Restoring the severed link —
See also Espacement and The Thing and I.
Related material —
[addtoany]
Comments Off on The Building Blocks of Geometry
Monday, May 6, 2019
One Stuff
-embedding-in-PG(3,2)-Planat-Saniga-1000w.jpg){kind=link}
Comments Off on One Stuff
Saturday, May 4, 2019
Inside the White Cube
See also Espacement and The Thing and I.
Comments Off on Inside the White Cube
Sunday, March 24, 2019
Espacement: Geometry of the Interstice in Literary Theory
"You said something about the significance of spaces between
elements being repeated. Not only the element itself being repeated,
but the space between. I'm very interested in the space between.
That is where we come together." — Peter Eisenman, 1982
|
https://www.parrhesiajournal.org/ Parrhesia No. 3 • 2007 • 22–32
(Up) Against the (In) Between: Interstitial Spatiality by Clare Blackburne Blackburne — www.parrhesiajournal.org 24 — "The excessive notion of espacement as the resurgent spatiality of that which is supposedly ‘without space’ (most notably, writing), alerts us to the highly dynamic nature of the interstice – a movement whose discontinuous and ‘aberrant’ nature requires further analysis." Blackburne — www.parrhesiajournal.org 25 — "Espacement also evokes the ambiguous figure of the interstice, and is related to the equally complex derridean notions of chora , différance , the trace and the supplement. Derrida’s reading of the Platonic chora in Chora L Works (a series of discussions with the architect Peter Eisenman) as something which defies the logics of non-contradiction and binarity, implies the internal heterogeneity and instability of all structures, neither ‘sensible’ nor ‘intelligible’ but a third genus which escapes conceptual capture.25 Crucially, chora , spacing, dissemination and différance are highly dynamic concepts, involving hybridity, an ongoing ‘corruption’ of categories, and a ‘bastard reasoning.’26 Derrida identification of différance in Margins of Philosophy , as an ‘unappropriable excess’ that operates through spacing as ‘the becoming-space of time or the becoming-time of space,’27 chimes with his description of chora as an ‘unidentifiable excess’ that is ‘the spacing which is the condition for everything to take place,’ opening up the interval as the plurivocity of writing in defiance of ‘origin’ and ‘essence.’28 In this unfolding of différance , spacing ‘insinuates into presence an interval,’29 again alerting us to the crucial role of the interstice in deconstruction, and, as Derrida observes in Positions , its impact as ‘a movement, a displacement that indicates an irreducible alterity’: ‘Spacing is the impossibility for an identity to be closed on itself, on the inside of its proper interiority, or on its coincidence with itself. The irreducibility of spacing is the irreducibility of the other.’30"
25. Quoted in Jeffrey Kipnis and Thomas Leeser, eds., 26. Ibid, 25.
27. Derrida, Margins of Philosophy. 28. Derrida, Chora L Works , 19 and 10. 29. Ibid, 203. 30. Derrida, Positions , 94. |
Comments Off on Espacement: Geometry of the Interstice in Literary Theory
Tuesday, February 26, 2019
Citation
Some related material in this journal — See a search for k6.gif.
Some related material from Harvard —
Elkies's "15 simple transpositions" clearly correspond to the 15 edges of
the complete graph K6 and to the 15 2-subsets of a 6-set.
For the connection to PG(3,2), see Finite Geometry of the Square and Cube.
The following "manifestation" of the 2-subsets of a 6-set might serve as
the desired Wikipedia citation —
See also the above 1986 construction of PG(3,2) from a 6-set
in the work of other authors in 1994 and 2002 . . .
-
Gonzalez-Dorrego, Maria R. (Maria del Rosario),
(16,6) Configurations and Geometry of Kummer Surfaces in P3.
American Mathematical Society, Providence, RI, 1994. -
Dolgachev, Igor, and Keum, JongHae,
"Birational Automorphisms of Quartic Hessian Surfaces."
Trans. Amer. Math. Soc. 354 (2002), 3031-3057.
Comments Off on Citation
Tuesday, December 18, 2018
CV
The title abbreviates* that of a collection of Wittgenstein's remarks:
|
Ludwig Wittgenstein — Culture and Value Showing 20 results for spirit — page 18, rubble & finally a heap of ashes; but spirits will hover over the ashes. MS 107 229: page 18, Page 5 Only something supernatural can expre page 20, contemplating it from above in its†c flight.† page 21, spirit in which it is written.†f This spirit is, I believe, different from that of t page 21, and American civilization. The spirit of this civilization the expression of page 21, day†h fascism & socialism, is a spirit that is alien & uncongenial†i to the au page 21, he Page Break 9 can work in the spirit of the whole, and his strength can with page 21, straight for what is concrete. Which is chara page 22, danger in a long foreword is that the spirit of a book has to be evident in the book page 22, It is all one to me whether the typical weste page 23, a great temptation to want to make the spirit explicit. MS 109 204: 6-7.11.1930 Page page 23, readers that will be clear just from the fact page 28, Foggy day. Grey autumn haunts us. Laughter se page 42, If one wanted to characterize the essence of page 51, attention from what matters.) The Spirit puts what is essential, essential for y page 51, how far all this is exactly in the spirit of Kierkegaard.) MS 119 151: 22.10.1937 page 51, something feminine about this outlook?) MS 11 page 100, comfortable, clearer expression, but cannot b page 106, act otherwise."–Perhaps, though, one might s page 210, Page 7 †b function Page 7 †c from its Page **************************************************************** |
The above "spirit guide" was suggested by yesterday's post
on Knuth as Yoda and by the paper in today's previous post,
"Shadowhunter Tales."
This post's title, "CV," is from . . .
Comments Off on CV
Monday, December 3, 2018
For Quantum Mystics
See a Quanta Magazine article published today and
https://www.chapman.edu/our-faculty/matt-leifer.
From the article —
|
Leifer, for his part, is holding out for something new. “I think the correct interpretation of quantum mechanics is none of the above,” he said. He likens the current situation with quantum mechanics to the time before Einstein came up with his special theory of relativity. Experimentalists had found no sign of the “luminiferous ether” — the medium through which light waves were thought to propagate in a Newtonian universe. Einstein argued that there is no ether. Instead he showed that space and time are malleable. “Pre-Einstein I couldn’t have told you that it was the structure of space and time that was going to change,” Leifer said. Quantum mechanics is in a similar situation now, he thinks. “It’s likely that we are making some implicit assumption about the way the world has to be that just isn’t true,” he said. “Once we change that, once we modify that assumption, everything would suddenly fall into place. That’s kind of the hope. Anybody who is skeptical of all interpretations of quantum mechanics must be thinking something like this. Can I tell you what’s a plausible candidate for such an assumption? Well, if I could, I would just be working on that theory.” |
See as well this journal on the Feast of the Assumption, 2018.
Comments Off on For Quantum Mystics
Wednesday, October 31, 2018
The Crucible of Death
On a new Netflix series:
|
We don’t yet have a story structure that allows witches to be powerful for long stretches of time without men holding them back. And what makes the new Sabrina so exciting is that it seems to be trying to build that story structure itself, in real time, to find a way to let Sabrina have her power and her freedom. It might fail. But if it does, it will be a glorious and worthwhile failure — the type that comes with trying to pioneer a new kind of story.
— Constance Grady at Vox, the morning before |
A playwright who reportedly died yesterday —
Comments Off on The Crucible of Death
Monday, October 15, 2018
For Zingari Shoolerim*
The structure at top right is that of the
ROMA-ORAM-MARO-AMOR square
in the previous post.
* "Zingari shoolerim" is from
Finnegans Wake .
Comments Off on For Zingari Shoolerim*
Wednesday, October 10, 2018
A Stitch in Time
The above October 7th screenshot is of a New York Times ad in the
"Business Day" section that accompanied a story with the headline
"Pret a Manager . . . ." [sic ].
The error was corrected:
Comments Off on A Stitch in Time