See as well "The Thing and I."
Wednesday, October 4, 2023
Monday, June 15, 2020
“The Thing and I” Continues*
“For the first thirty years of its history, Columbia was known as King’s College.”
— History of the University Identity
Hence the crown favicon—

“When people talk about the importance of the study of ‘symmetry’
in mathematics, physics, and elsewhere, they often make the mistake
of only paying attention to the symmetry groups. The structure you
actually have is not just a group (the abstract ‘symmetries’), but an
action of that group on some other object, the thing that has symmetries.”
— Peter Woit of Columbia on June 9, reviewing a Quanta Magazine article
* From earlier posts in this journal containing the title phrase.
Monday, May 4, 2020
Tuesday, September 10, 2019
“The Thing and I” Continues*
* From earlier posts containing the title phrase.
Saturday, January 14, 2017
Sunday, April 17, 2016
The Thing and I
The New York Times philosophy column yesterday —
The Times's philosophy column "The Stone" is named after the legendary
"philosophers' stone." The column's name, and the title of its essay yesterday
"Is that even a thing?" suggest a review of the eightfold cube as "The object
most closely resembling a 'philosophers' stone' that I know of" (Page 51 of
the current issue of a Norwegian art quarterly, KUNSTforum.as).
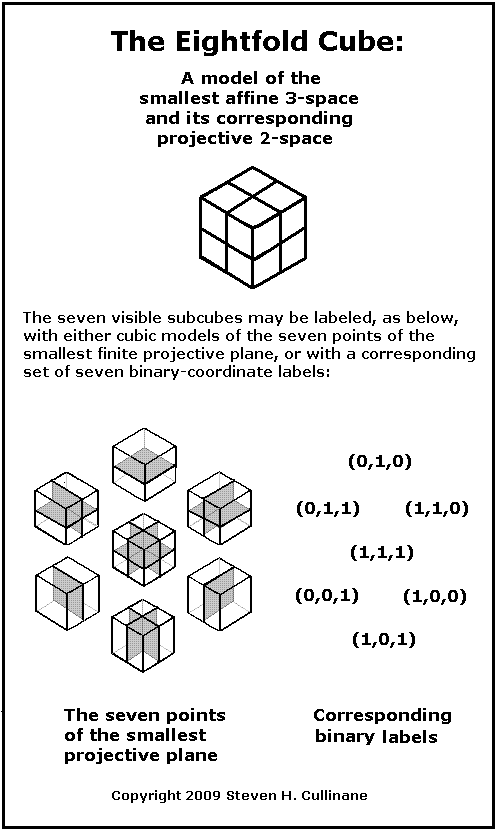
The eightfold cube —
|
Definition of Epiphany
From James Joyce’s Stephen Hero , first published posthumously in 1944. The excerpt below is from a version edited by John J. Slocum and Herbert Cahoon (New York: New Directions Press, 1959). Three Times: … By an epiphany he meant a sudden spiritual manifestation, whether in the vulgarity of speech or of gesture or in a memorable phase of the mind itself. He believed that it was for the man of letters to record these epiphanies with extreme care, seeing that they themselves are the most delicate and evanescent of moments. He told Cranly that the clock of the Ballast Office was capable of an epiphany. Cranly questioned the inscrutable dial of the Ballast Office with his no less inscrutable countenance: — Yes, said Stephen. I will pass it time after time, allude to it, refer to it, catch a glimpse of it. It is only an item in the catalogue of Dublin’s street furniture. Then all at once I see it and I know at once what it is: epiphany. — What? — Imagine my glimpses at that clock as the gropings of a spiritual eye which seeks to adjust its vision to an exact focus. The moment the focus is reached the object is epiphanised. It is just in this epiphany that I find the third, the supreme quality of beauty. — Yes? said Cranly absently. — No esthetic theory, pursued Stephen relentlessly, is of any value which investigates with the aid of the lantern of tradition. What we symbolise in black the Chinaman may symbolise in yellow: each has his own tradition. Greek beauty laughs at Coptic beauty and the American Indian derides them both. It is almost impossible to reconcile all tradition whereas it is by no means impossible to find the justification of every form of beauty which has ever been adored on the earth by an examination into the mechanism of esthetic apprehension whether it be dressed in red, white, yellow or black. We have no reason for thinking that the Chinaman has a different system of digestion from that which we have though our diets are quite dissimilar. The apprehensive faculty must be scrutinised in action. — Yes … — You know what Aquinas says: The three things requisite for beauty are, integrity, a wholeness, symmetry and radiance. Some day I will expand that sentence into a treatise. Consider the performance of your own mind when confronted with any object, hypothetically beautiful. Your mind to apprehend that object divides the entire universe into two parts, the object, and the void which is not the object. To apprehend it you must lift it away from everything else: and then you perceive that it is one integral thing, that is a thing. You recognise its integrity. Isn’t that so? — And then? — That is the first quality of beauty: it is declared in a simple sudden synthesis of the faculty which apprehends. What then? Analysis then. The mind considers the object in whole and in part, in relation to itself and to other objects, examines the balance of its parts, contemplates the form of the object, traverses every cranny of the structure. So the mind receives the impression of the symmetry of the object. The mind recognises that the object is in the strict sense of the word, a thing , a definitely constituted entity. You see? — Let us turn back, said Cranly. They had reached the corner of Grafton St and as the footpath was overcrowded they turned back northwards. Cranly had an inclination to watch the antics of a drunkard who had been ejected from a bar in Suffolk St but Stephen took his arm summarily and led him away. — Now for the third quality. For a long time I couldn’t make out what Aquinas meant. He uses a figurative word (a very unusual thing for him) but I have solved it. Claritas is quidditas . After the analysis which discovers the second quality the mind makes the only logically possible synthesis and discovers the third quality. This is the moment which I call epiphany. First we recognise that the object is one integral thing, then we recognise that it is an organised composite structure, a thing in fact: finally, when the relation of the parts is exquisite, when the parts are adjusted to the special point, we recognise that it is that thing which it is. Its soul, its whatness, leaps to us from the vestment of its appearance. The soul of the commonest object, the structure of which is so adjusted, seems to us radiant. The object achieves its epiphany. Having finished his argument Stephen walked on in silence. He felt Cranly’s hostility and he accused himself of having cheapened the eternal images of beauty. For the first time, too, he felt slightly awkward in his friend’s company and to restore a mood of flippant familiarity he glanced up at the clock of the Ballast Office and smiled: — It has not epiphanised yet, he said. |
Tuesday, May 21, 2024
For Optimus Prime
"Before time began, there was the Cube." — Optimus Prime in "Transformers"
This journal at 9 PM ET March 17, 2023 —
The use of binary coordinate systems as a conceptual tool
Natural physical transformations of square or cubical arrays
of actual physical cubes (i.e., building blocks) correspond to
natural algebraic transformations of vector spaces over GF(2).
This was apparently not previously known.
See "The Thing and I."
See as well today's post Geometry for Belgium.
Monday, January 22, 2024
Tuesday, October 24, 2023
Saturday, March 18, 2023
Zu diesem Themenkreis
From last night's update to the previous post —
|
The use of binary coordinate systems
Natural physical transformations of square or cubical arrays See "The Thing and I." |
From a post of May 1, 2016 —
|
Mathematische Appetithäppchen: Autor: Erickson, Martin —
"Weitere Informationen zu diesem Themenkreis finden sich |
Friday, March 17, 2023
Bing Chat Continues


Update at 9 PM ET March 17: A related observation by SHC —
The use of binary coordinate systems as a conceptual tool
Natural physical transformations of square or cubical arrays
of actual physical cubes (i.e., building blocks) correspond to
natural algebraic transformations of vector spaces over GF(2).
This was apparently not previously known.
See "The Thing and I."
Tuesday, January 31, 2023
The Object Subject
"The literary attack on the concept of a remembered self
comes principally from three directions:
(1) the brokenness of memory,
(2) the difficulty of affirming that all memories pertain to
the same self; and
(3) the impossibility of pretending that a subject is an object.
All three of these attacks tend to dissolve the self, to expose it
as fictitious, artificial, quaintly contrived."
"Literary and Psychological Models of the Self,” pp. 19 – 40 in
Neisser, U., & Fivush, R. (Eds.) (1994). The Remembering Self:
Construction and Accuracy in the Self-Narrative (Emory Symposia
in Cognition). Cambridge: Cambridge University Press.
See also The Thing and I.
Saturday, October 26, 2019
Director’s Cut
The title was suggested by the previous post and by
the title illustration in the weblog of the director,
Leigh Whannell, of the 2018 film “Upgrade.”
Related visual details —
For the Church of Synchronology —
Related remarks: “The Thing and I.”
Sunday, May 19, 2019
Monday, May 6, 2019
Saturday, May 4, 2019
Inside the White Cube
See also Espacement and The Thing and I.
Sunday, April 7, 2019
Block Talk
David Brooks in The New York Times Sunday Review today —
" 'In the deeps are the violence and terror of which psychology
has warned us,' Annie Dillard writes in 'Teaching a Stone to Talk.'
'But if you ride these monsters deeper down, if you drop with them
farther over the world’s rim, you find what our sciences cannot locate
or name, the substrate, the ocean or matrix or ether which buoys
the rest, which gives goodness its power for good, and evil its power
for evil, the unified field: our complex and inexplicable caring for
each other.' "
Annie Dillard on the legendary philosopher's stone —
“… if Holy the Firm is matter at its dullest, Aristotle’s materia prima ,
absolute zero, and since Holy the Firm is in touch with the Absolute
at base, then the circle is unbroken. And it is…. Holy the Firm is
in short the philosopher’s stone.”
See also "The Thing and I."
Sunday, December 2, 2018
Iacta Est.
Wednesday, November 28, 2018
Geometry and Experience
Einstein, "Geometry and Experience," lecture before the
Prussian Academy of Sciences, January 27, 1921–
|
… This view of axioms, advocated by modern axiomatics, purges mathematics of all extraneous elements, and thus dispels the mystic obscurity, which formerly surrounded the basis of mathematics. But such an expurgated exposition of mathematics makes it also evident that mathematics as such cannot predicate anything about objects of our intuition or real objects. In axiomatic geometry the words "point," "straight line," etc., stand only for empty conceptual schemata. That which gives them content is not relevant to mathematics. Yet on the other hand it is certain that mathematics generally, and particularly geometry, owes its existence to the need which was felt of learning something about the behavior of real objects. The very word geometry, which, of course, means earth-measuring, proves this. For earth-measuring has to do with the possibilities of the disposition of certain natural objects with respect to one another, namely, with parts of the earth, measuring-lines, measuring-wands, etc. It is clear that the system of concepts of axiomatic geometry alone cannot make any assertions as to the behavior of real objects of this kind, which we will call practically-rigid bodies. To be able to make such assertions, geometry must be stripped of its merely logical-formal character by the coordination of real objects of experience with the empty conceptual schemata of axiomatic geometry. To accomplish this, we need only add the proposition: solid bodies are related, with respect to their possible dispositions, as are bodies in Euclidean geometry of three dimensions. Then the propositions of Euclid contain affirmations as to the behavior of practically-rigid bodies. Geometry thus completed is evidently a natural science; we may in fact regard it as the most ancient branch of physics. Its affirmations rest essentially on induction from experience, but not on logical inferences only. We will call this completed geometry "practical geometry," and shall distinguish it in what follows from "purely axiomatic geometry." The question whether the practical geometry of the universe is Euclidean or not has a clear meaning, and its answer can only be furnished by experience. …. |
Later in the same lecture, Einstein discusses "the theory of a finite
universe." Of course he is not using "finite" in the sense of the field
of mathematics known as "finite geometry " — geometry with only finitely
many points.
Nevertheless, his remarks seem relevant to the Fano plane , an
axiomatically defined entity from finite geometry, and the eightfold cube ,
a physical object embodying the properties of the Fano plane.
|
I want to show that without any extraordinary difficulty we can illustrate the theory of a finite universe by means of a mental picture to which, with some practice, we shall soon grow accustomed. First of all, an observation of epistemological nature. A geometrical-physical theory as such is incapable of being directly pictured, being merely a system of concepts. But these concepts serve the purpose of bringing a multiplicity of real or imaginary sensory experiences into connection in the mind. To "visualize" a theory therefore means to bring to mind that abundance of sensible experiences for which the theory supplies the schematic arrangement. In the present case we have to ask ourselves how we can represent that behavior of solid bodies with respect to their mutual disposition (contact) that corresponds to the theory of a finite universe. |
Monday, July 9, 2018
Annals of Ontology
The Thing and I continues.
"… the Quantum Realm wouldn’t really become a 'thing'
in Marvel’s comic book mythology until the end of that
decade [the 1970s], and the arrival of a toy license at
the publisher."
— Graeme McMillan in The Hollywood Reporter Saturday
Thursday, February 2, 2017
An Object for New Haven
The title was suggested by a Wallace Stevens poem.
See "The Thing and I" in this journal. See also
Words and Objects according to Whorf —
— Page 240 of Language, Thought, and Reality , MIT, 1956,
in the article "Languages and Logic," reprinted from
Technol. Rev. , 43: 250-252, 266, 268, 272 (April 1941)
Saturday, April 30, 2016
A Lucifer for Walpurgisnacht
A more impressive Lucifer —
The late theoretical physicist John Archibald Wheeler,
author of the phrase "it from bit."
Related material —
"The Thing and I" (April 17, 2016) and an essay by
Julian Barbour, "Bit from It."