See also "Opening the Local/Global Seal," Log24, March 8, 2023.
Thursday, January 11, 2024
Wednesday, March 8, 2023
Opening the Local/Global Seal
Comments Off on Opening the Local/Global Seal
Wednesday, February 8, 2023
Local-Global lnduced Actions
See "Two Approaches to Local-Global Symmetry"
(this journal, Jan. 19, 2023), which discusses
local group actions on plane and solid graphic
patterns that induce global group actions.
See also local and global group actions of a different sort in
the July 11, 1986, note "Inner and Outer Group Actions."
This post was suggested by some remarks of Barry Mazur,
quoted in the previous post, on " Wittgenstein's 'language game,' "
Grothendieck, global views, local views and "locales."
Further reading on "locales" — Wikipedia, Pointless topology.
The word "locale" in mathematics was apparently* introduced by Isbell —
ISBELL, JOHN R. “ATOMLESS PARTS OF SPACES.”
Mathematica Scandinavica, vol. 31, no. 1, 1972, pp. 5–32.
JSTOR, http://www.jstor.org/stable/24490585.
* According to page 841 of . . .
Johnstone, P. (2001). "Elements of the History of Locale Theory."
Pp. 835–851 in: Aull, C.E., Lowen, R. (eds) Handbook of the
History of General Topology, Vol 3. Springer, Dordrecht.
Comments Off on Local-Global lnduced Actions
Thursday, January 19, 2023
Two Approaches to Local-Global Symmetry
Last revised: January 20, 2023 @ 11:39:05
The First Approach — Via Substructure Isomorphisms —
From "Symmetry in Mathematics and Mathematics of Symmetry"
by Peter J. Cameron, a Jan. 16, 2007, talk at the International
Symmetry Conference, Edinburgh, Jan. 14-17, 2007 —
|
Local or global? "Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous * if every isomorphism between finite substructures of M can be extended to an automorphism of M ; in other words, 'any local symmetry is global.' " |
A related discussion of the same approach —
|
"The aim of this thesis is to classify certain structures
— Alice Devillers, |
The Wikipedia article Homogeneous graph discusses the local-global approach
used by Cameron and by Devillers.
For some historical background on this approach
via substructure isomorphisms, see a former student of Cameron:
Dugald Macpherson, "A survey of homogeneous structures,"
Discrete Mathematics , Volume 311, Issue 15, 2011,
Pages 1599-1634.
Related material:
Cherlin, G. (2000). "Sporadic Homogeneous Structures."
In: Gelfand, I.M., Retakh, V.S. (eds)
The Gelfand Mathematical Seminars, 1996–1999.
Gelfand Mathematical Seminars. Birkhäuser, Boston, MA.
https://doi.org/10.1007/978-1-4612-1340-6_2
and, more recently,
Gill et al., "Cherlin's conjecture on finite primitive binary
permutation groups," https://arxiv.org/abs/2106.05154v2
(Submitted on 9 Jun 2021, last revised 9 Jul 2021)
This approach seems to be a rather deep rabbit hole.
The Second Approach — Via Induced Group Actions —
My own interest in local-global symmetry is of a quite different sort.
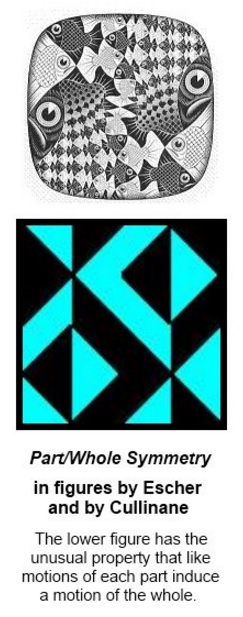
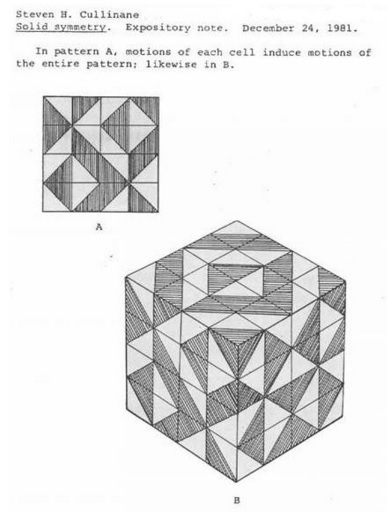
See properties of the two patterns illustrated in a note of 24 December 1981 —
Pattern A above actually has as few symmetries as possible
(under the actions described in the diamond theorem ), but it
does enjoy, as does patttern B, the local-global property that
a group acting in the same way locally on each part induces
a global group action on the whole .
* For some historical background on the term "homogeneous,"
see the Wikipedia article Homogeneous space.
Comments Off on Two Approaches to Local-Global Symmetry
Wednesday, April 27, 2016
Local and Global
Three notes on local symmetries
that induce global symmetries
From July 1, 2011 —
From November 5, 1981 —
From December 24, 1981 —
Comments Off on Local and Global
Thursday, June 21, 2007
Thursday June 21, 2007
Let No Man
Write My Epigraph
"His graceful accounts of the Bach Suites for Unaccompanied Cello illuminated the works’ structural logic as well as their inner spirituality."
—Allan Kozinn on Mstislav Rostropovich in The New York Times, quoted in Log24 on April 29, 2007
"At that instant he saw, in one blaze of light, an image of unutterable conviction…. the core of life, the essential pattern whence all other things proceed, the kernel of eternity."
— Thomas Wolfe, Of Time and the River, quoted in Log24 on June 9, 2005
"… the stabiliser of an octad preserves the affine space structure on its complement, and (from the construction) induces AGL(4,2) on it. (It induces A8 on the octad, the kernel of this action being the translation group of the affine space.)"
— Peter J. Cameron, "The Geometry of the Mathieu Groups" (pdf)
"… donc Dieu existe, réponse!"
"Only gradually did I discover
what the mandala really is:
'Formation, Transformation,
Eternal Mind's eternal recreation'"
what the mandala really is:
'Formation, Transformation,
Eternal Mind's eternal recreation'"
(Faust, Part Two, as
quoted by Jung in
Memories, Dreams, Reflections)
"Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen.
The drawing is one of
many by George Gamow
illustrating the script."
— Physics Today
"Borja dropped the mutilated book on the floor with the others. He was looking at the nine engravings and at the circle, checking strange correspondences between them.
"Pauli linked this symbolism
with the concept of automorphism."
'To meet someone' was his enigmatic answer. 'To search for the stone that the Great Architect rejected, the philosopher's stone, the basis of the philosophical work. The stone of power. The devil likes metamorphoses, Corso.'"
— The Club Dumas, basis for the Roman Polanski film "The Ninth Gate" (See 12/24/05.)
"Pauli linked this symbolism
with the concept of automorphism."
— The Innermost Kernel
(previous entry)
And from
"Symmetry in Mathematics
and Mathematics of Symmetry"
(pdf), by Peter J. Cameron,
a paper presented at the
International Symmetry Conference,
Edinburgh, Jan. 14-17, 2007,
we have
The Epigraph–
(Here "whatever" should
of course be "whenever.")
Also from the
Cameron paper:
|
Local or global?
Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous if every isomorphism between finite substructures of M can be extended to an automorphism of M; in other words, "any local symmetry is global." |
Some Log24 entries
related to the above politically
(women in mathematics)–
Global and Local:
One Small Step
and mathematically–
Structural Logic continued:
Structure and Logic (4/30/07):
This entry cites
Alice Devillers of Brussels–
"The aim of this thesis
is to classify certain structures
which are, from a certain
point of view, as homogeneous
as possible, that is which have
as many symmetries as possible."
"There is such a thing
as a tesseract."
Comments Off on Thursday June 21, 2007