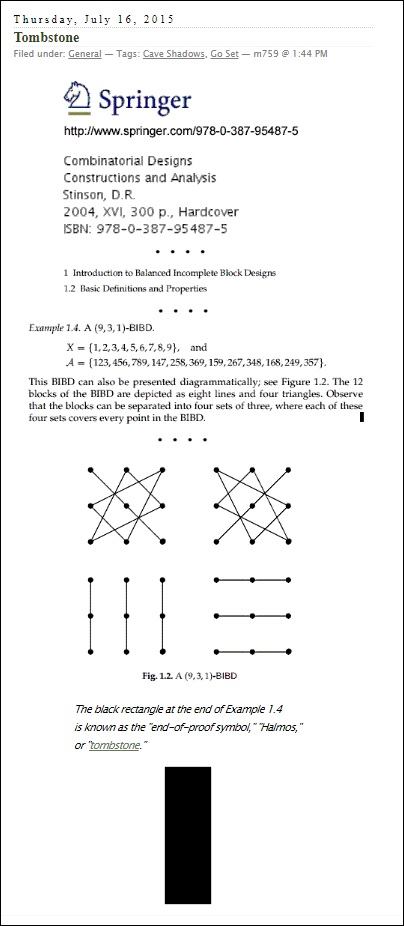
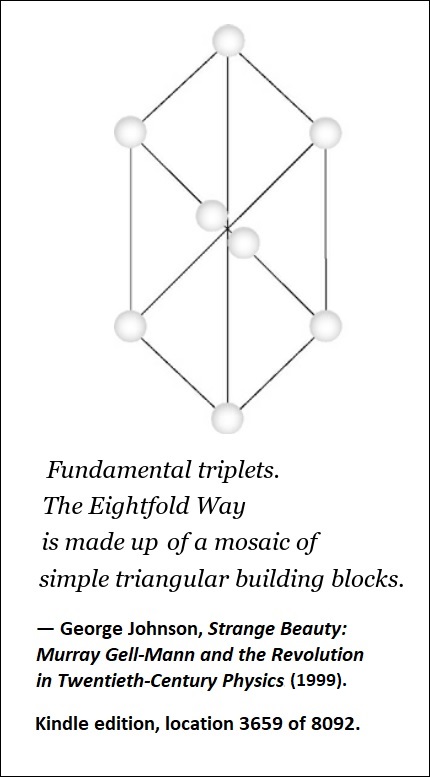
That new URL forwards to http://m759.net/wordpress/?s=Eightfold.
Tuesday, March 25, 2025
Sunday, September 4, 2022
Dice and the Eightfold Cube
At Hiroshima on March 9, 2018, Aitchison discussed another
"hexagonal array" with two added points… not at the center, as
in the Gell-Mann picture above, but rather at the ends of one of
a cube's four diagonal axes of symmetry.
See some related illustrations below.
Fans of the fictional "Transfiguration College" in the play
"Heroes of the Fourth Turning" may recall that August 6,
another Hiroshima date, was the Feast of the Transfiguration.
The exceptional role of 0 and ∞ in Aitchison's diagram is echoed
by the occurence of these symbols in the "knight" labeling of a
Miracle Octad Generator octad —
Transposition of 0 and ∞ in the knight coordinatization
induces the symplectic polarity of PG(3,2) discussed by
(for instance) Anne Duncan in 1968.
Comments Off on Dice and the Eightfold Cube
Monday, March 7, 2022
Logos: Beamery vs. Eightfold
Logos as tab icons —
Logos in context —
Related graphic design from April 1, 2018 —
Later, in 2019 —
An undated webpage says . . .
"…we’re officially announcing the launch of the new Beamery brand."
"A logo, a name, an idea: Meet the Bexa"
— https://beamery.com/resources/blogs/were-launching-beamerys-new-brand
(metadata: itemprop="datePublished" content="2019-11-20T19:00:00")
This logo design was described elsewhere —
"Beamery's new mark–The Bexa. The Bexa contains the negative space
of 3 Bs rotating clockwise within a hexagon shape to capture Beamery's
3 pillars of the Talent Operating System." — Ben Stafford for Focus Lab
"All things serve the Beamery."
— Slogan adapted from Stephen King.
Comments Off on Logos: Beamery vs. Eightfold
Saturday, May 23, 2020
Eightfold Geometry: A Surface Code “Unit Cell”
The resemblance to the eightfold cube is, of course,
completely coincidental.
Some background from the literature —
Comments Off on Eightfold Geometry: A Surface Code “Unit Cell”
Sunday, March 22, 2020
Eightfold Site
A brief summary of the eightfold cube is now at octad.us.
Comments Off on Eightfold Site
Sunday, December 22, 2019
M24 from the Eightfold Cube
Exercise: Use the Guitart 7-cycles below to relate the 56 triples
in an 8-set (such as the eightfold cube) to the 56 triangles in
a well-known Klein-quartic hyperbolic-plane tiling. Then use
the correspondence of the triples with the 56 spreads of PG(3,2)
to construct M24.
Click image below to download a Guitart PowerPoint presentation.
See as well earlier posts also tagged Triangles, Spreads, Mathieu.
Comments Off on M24 from the Eightfold Cube
Friday, May 24, 2019
Tuesday, March 5, 2019
The Eightfold Cube and PSL(2,7)
For PSL(2,7), this is ((49-1)(49-7))/((7-1)(2))=168.
The group GL(3,2), also of order 168, acts naturally
on the set of seven cube-slicings below —
Another way to picture the seven natural slicings —
Application of the above images to picturing the
isomorphism of PSL(2,7) with GL(3,2) —
.gif)
For a more detailed proof, see . . .
Comments Off on The Eightfold Cube and PSL(2,7)
Sunday, September 30, 2018
Iconology of the Eightfold Cube
Found today in an Internet image search, from the website of
an anonymous amateur mathematics enthusiast —
Forming Gray codes in the eightfold cube with the eight
I Ching trigrams (bagua ) —
This journal on Nov. 7, 2016 —
A different sort of cube, from the makers of the recent
Netflix miniseries "Maniac" —
See also Rubik in this journal.
Comments Off on Iconology of the Eightfold Cube
Monday, July 23, 2018
Eightfold Cube for Furey*
Click to enlarge:
Above are the 7 frames of an animated gif from a Wikipedia article.
* For the Furey of the title, see a July 20 Quanta Magazine piece —
See also the eightfold cube in this journal.
"Before time began . . . ." — Optimus Prime
Comments Off on Eightfold Cube for Furey*
Friday, June 29, 2018
Triangles in the Eightfold Cube
From a post of July 25, 2008, “56 Triangles,” on the Klein quartic
and the eightfold cube —
“Baez’s discussion says that the Klein quartic’s 56 triangles
can be partitioned into 7 eight-triangle Egan ‘cubes’ that
correspond to the 7 points of the Fano plane in such a way
that automorphisms of the Klein quartic correspond to
automorphisms of the Fano plane. Show that the
56 triangles within the eightfold cube can also be partitioned
into 7 eight-triangle sets that correspond to the 7 points of the
Fano plane in such a way that (affine) transformations of the
eightfold cube induce (projective) automorphisms of the Fano plane.”
Related material from 1975 —
More recently …
Comments Off on Triangles in the Eightfold Cube
Thursday, May 31, 2018
Eightfold Suffering:
A New, Improved Version of Quantum Suffering !
Background for group actions on the eightfold cube —
See also other posts now tagged Quantum Suffering
as well as — related to the image above of the Great Wall —
Comments Off on Eightfold Suffering:
Tuesday, January 10, 2017
Eightfold Epiphany
The reported death today at 105 of an admirable war correspondent,
"a perennial fixture at the Foreign Correspondents’ Club in Hong Kong,"
suggested a search in this journal for that city.
The search recalled to mind a notable quotation from
a Montreal philosopher —
“… the object sets up a kind of
frame or space or field
within which there can be epiphany.”
Charles Taylor, "Epiphanies of Modernism,"
Chapter 24 of Sources of the Self
(Cambridge U. Press, 1989, p. 477)
For some context, see St. Lucia's Day, 2012.
See also Epiphany 2017 —
Comments Off on Eightfold Epiphany
Friday, January 6, 2017
Eightfold Cube at Cornell
The assignments page for a graduate algebra course at Cornell
last fall had a link to the eightfold cube:
Comments Off on Eightfold Cube at Cornell
Wednesday, November 30, 2016
Eightfold Roman
"Frye's largely imaginary eightfold roman
may have provided him a personal substitute—
or alternative— for both ideology and myth."
— P. 63 of James C. Nohrnberg, "The Master of
the Myth of Literature: An Interpenetrative Ogdoad
for Northrop Frye," Comparative Literature Vol. 53,
No. 1 (Winter, 2001), pp. 58-82
See also today's earlier post In Nuce .
Comments Off on Eightfold Roman
Tuesday, August 30, 2016
The Eightfold Cube in Oslo
A KUNSTforum.as article online today (translation by Google) —
Update of Sept. 7, 2016: The corrections have been made,
except for the misspelling "Cullinan," which was caused by
Google translation, not by KUNSTforum.
Comments Off on The Eightfold Cube in Oslo
Thursday, March 17, 2016
On the Eightfold Cube
The following page quotes "Raiders of the Lost Crucible,"
a Log24 post from Halloween 2015.
From KUNSTforum.as, a Norwegian art quarterly, issue no. 1 of 2016.
Related posts — See Lyche Eightfold.
Comments Off on On the Eightfold Cube
Friday, October 9, 2015
Eightfold Cube in Oslo
An eightfold cube appears in this detail
of a photo by Josefine Lyche of her
installation "4D Ambassador" at the
Norwegian Sculpture Biennial 2015 —
(Detail from private Instagram photo.)
Catalog description of installation —
Google Translate version —
In a small bedroom to Foredragssalen populate
Josefine Lyche exhibition with a group sculptures
that are part of the work group 4D Ambassador
(2014-2015). Together they form an installation
where she uses light to amplify the feeling of
stepping into a new dimension, for which the title
suggests, this "ambassadors" for a dimension we
normally do not have access to. "Ambassadors"
physical forms presents nonphysical phenomena.
Lyches works have in recent years been placed
in something one might call an "esoteric direction"
in contemporary art, and defines itself this
sculpture group humorous as "glam-minimalist."
She has in many of his works returned to basic
geometric shapes, with hints to the occult,
"new space-age", mathematics and where
everything in between.
See also Lyche + "4D Ambassador" in this journal and
her website page with a 2012 version of that title.
Comments Off on Eightfold Cube in Oslo
Monday, April 9, 2012
Eightfold Cube Revisited
A search today (Élie Cartan's birthday) for material related to triality*
yielded references to something that has been called a Bhargava cube .
Two pages from a 2006 paper by Bhargava—
Bhargava's reference [4] above for "the story of the cube" is to…
Higher Composition Laws I:
A New View on Gauss Composition,
and Quadratic Generalizations
Manjul Bhargava
The Annals of Mathematics
Second Series, Vol. 159, No. 1 (Jan., 2004), pp. 217-250
Published by: Annals of Mathematics
Article Stable URL: http://www.jstor.org/stable/3597249
A brief account in the context of embedding problems (click to enlarge)—
For more ways of slicing a cube,
see The Eightfold Cube —
* Note (1) some remarks by Tony Smith
related to the above Dynkin diagram
and (2) another colorful variation on the diagram.
Comments Off on Eightfold Cube Revisited
Thursday, May 19, 2011
The Aleph, the Lottery, and the Eightfold Way
Three links with a Borges flavor—
Related material
The 236 in yesterday evening's NY lottery may be
viewed as the 236 in March 18's Defining Configurations.
For some background, see Configurations and Squares.
A new illustration for that topic—
This shows a reconcilation of the triples described by Sloane
in Defining Configurations with the square geometric
arrangement described by Coxeter in the Aleph link above.
Note that the 56 from yesterday's midday NY lottery
describes the triples that appear both in the Eightfold Way
link above and also in a possible source for
the eight triples of Sloane's 83 configuration—
The geometric square arrangement discussed in the Aleph link
above appears in a different, but still rather Borgesian, context
in yesterday morning's Minimalist Icon.
Comments Off on The Aleph, the Lottery, and the Eightfold Way
Wednesday, April 28, 2010
Eightfold Geometry
Related web pages:
Miracle Octad Generator,
Generating the Octad Generator,
Geometry of the 4×4 Square
Related folklore:
"It is commonly known that there is a bijection between the 35 unordered triples of a 7-set [i.e., the 35 partitions of an 8-set into two 4-sets] and the 35 lines of PG(3,2) such that lines intersect if and only if the corresponding triples have exactly one element in common." –"Generalized Polygons and Semipartial Geometries," by F. De Clerck, J. A. Thas, and H. Van Maldeghem, April 1996 minicourse, example 5 on page 6
The Miracle Octad Generator may be regarded as illustrating the folklore.
Update of August 20, 2010–
For facts rather than folklore about the above bijection, see The Moore Correspondence.
Comments Off on Eightfold Geometry
Tuesday, March 30, 2010
Eightfold Symmetries
Harvard Crimson headline today–
“Deconstructing Design“
Reconstructing Design
The phrase “eightfold way” in today’s
previous entry has a certain
graphic resonance…
For instance, an illustration from the
Wikipedia article “Noble Eightfold Path” —
Adapted detail–
See also, from
St. Joseph’s Day—
Harvard students who view Christian symbols
with fear and loathing may meditate
on the above as a representation of
the Gankyil rather than of the Trinity.
Comments Off on Eightfold Symmetries
Thursday, April 24, 2025
Uniting the Three Cubes
Note that the number 8, a cube, may be represented as
either a literal "eightfold cube" — a 2x2x2 array — or as,
in the manner of R. T. Curtis, a 4-row 2-column "brick."
Related art . . .
Some will prefer a more dramatic approach to uniting three cubes . . .
Sunday, April 20, 2025
From Harlan Kane’s Gotham Times Bestseller —
Liam Neeson in THE NAKED EIGHT!
. . . A Sequel to "Unknown" . . .
Friday, April 18, 2025
A Koan for Rockwell (Sam, not Norman)
Q — "Is the Eightfold Way the same as the Yellow Brick Road?"
A — "No. The former is the Klein Quartic, the latter the Klein Quadric."
Wednesday, January 8, 2025
Show Tune from the Garden of Good and Evil
From posts tagged EGBDF —
♫ “And we may see the meadow in December,
icy white* and crystalline” — Johnny Mercer
“The devil likes metamorphoses.“
Related geography . . .
* See a related (if only verbally) obituary.
Comments Off on Show Tune from the Garden of Good and Evil
Monday, December 16, 2024
Whitecaps for Beethoven
"Each of the 64 subcubes is supposed to be marked identically,
with white caps on two opposite vertices and a black band
around the subcube that separates the two white caps."
Related whitecap reading . . .
Comments Off on Whitecaps for Beethoven
Saturday, September 14, 2024
Notes on a Friday the 13th Death
A passage accessed via the new URL Starbrick.art* —
Thursday, February 25, 2021
|
A related cultural note suggested by the New York Times obituary today
of fashion designer Mary McFadden, who reportedly died yesterday
(a Friday the Thirteenth) and is described by the Times as a late-life
partner of "eightfold-way" physicist Murray Gell-Mann —
* A reference to the 2-column 4-row matrix (a "brick") that underlies
the patterns in the Miracle Octad Generator of R. T. Curtis. The only
connection of this eight-part matrix to Gell-Mann's "Eightfold Way"
that I know of is simply the number 8 itself.
Comments Off on Notes on a Friday the 13th Death
Thursday, June 13, 2024
Of London Bondage … continues.
From a bondage search . . .
“Loitering in Lara’s dressing room, she tries on
the faux-bondage harness she picked up in London….”
From Geometry for Belgium —
Comments Off on Of London Bondage … continues.
Monday, June 10, 2024
Art Logos
Comments Off on Art Logos
Tuesday, May 21, 2024
Geometry for Belgium
Other matching patterns . . .
Tuesday Weld in the 1972 film of Didion's Play It As It Lays :
Note the making of a matching pattern.
Comments Off on Geometry for Belgium
Saturday, May 4, 2024
What Lies Between
"I perceived . . . cinema is that which is between things,
not things [themselves] but between one and another."
— Jean-Luc Godard, "Introduction à une véritable histoire
du cinéma," Albatros , Paris, 1980, p. 145
|
Log24 on 10 Dec. 2008 —
|
Log24 on 12 Dec. 2008 —
|
Between the two image-dates above . . .
" 'The jury is still out on how long – and whether – people are actually
going to understand this.' It took the world 150 years to realize
the true power of the printing press . . . ." — Cade Metz
Comments Off on What Lies Between
Thursday, March 21, 2024
The Cross Section
Addendum for Christopher Nolan — Dice and the Eightfold Cube.
Comments Off on The Cross Section
Tuesday, February 20, 2024
Backlight
The epigraph of the previous post —
"To Phaedrus, this backlight from the conflict between
the Sophists and the Cosmologists adds an entirely
new dimension to the Dialogues of Plato." — Robert M. Pirsig
Related reading and art for academic nihilists — See . . .
Reading and art I prefer —
Love in the Ruins , by Walker Percy, and . . .
Van Gogh (by Ed Arno) and an image and
a passage from The Paradise of Childhood
(by Edward Wiebé):
Comments Off on Backlight
Tuesday, October 24, 2023
A Bond with Reality: The Geometry of Cuts
Illustrations of object and gestures
from finitegeometry.org/sc/ —
Object
Gestures
An earlier presentation of the above
seven partitions of the eightfold cube:
|
|
Related mathematics:
|
The use of binary coordinate systems
Natural physical transformations of square or cubical arrays See "The Thing and I." |
and . . .
Related entertainment:
Or Matt Helm by way of a Jedi cube.
Comments Off on A Bond with Reality: The Geometry of Cuts
Monday, October 23, 2023
Saturday, July 1, 2023
Mechanical Plaything (Hinged) vs. Conceptual Art (Unhinged)
"Infinity Cube" … hinged plaything, for sale —
"Eightfold Cube" … un hinged concept, not for sale—
See as well yesterday's Trickster Fuge ,
and a 1906 discussion of the eightfold cube:
Comments Off on Mechanical Plaything (Hinged) vs. Conceptual Art (Unhinged)
Wednesday, June 21, 2023
Annals of Magical Thinking: The Rushmore Embedding
Comments Off on Annals of Magical Thinking: The Rushmore Embedding
Saturday, June 10, 2023
Green, Orange, Black
The colors surrounding Watson's body in the above
"bandeau" photo suggest a review. A search in this journal
for Green+Orange+Black yields . . .
In the above image, the "hard core of objectivity" is represented
by the green-and-white eightfold cube. The orange and black are,
of course, the Princeton colors.
Comments Off on Green, Orange, Black
Sunday, June 4, 2023
The Galois Core
Comments Off on The Galois Core
Friday, June 2, 2023
Reichenbach’s Fell Swoop
See The Eightfold Cube and . . .
Art is magic delivered from
the lie of being truth.
— Theodor Adorno, Minima moralia,
London, New Left Books, 1974, p. 222
(First published in German in 1951.)
The director, Carol Reed, makes…
impeccable use of the beauty of black….
— V. B. Daniel on The Third Man
I see your ironical smile.
— Hans Reichenbach
Adorno, The Third Man, and Reichenbach
are illustrated below (l. to r.) above the names of
cities with which they are associated.
Comments Off on Reichenbach’s Fell Swoop
Tuesday, May 30, 2023
The Tour
In memory of a co-founder of Hollywood's "Magic Castle"
who reportedly died at 92 on Sunday . . .
From posts that were tagged "Blake Tour" on Sunday —
Comments Off on The Tour
Friday, January 27, 2023
The Stone
Here stands the mean, uncomely stone,
’Tis very cheap in price!
The more it is despised by fools,
The more loved by the wise.
— https://jungcurrents.com/
the-story-of-the-stone-at-bollingen
Not so cheap:
Identical copies of the above image are being offered for sale
on three websites as representing a Masonic "cubic stone."
None of the three sites say where, exactly, the image originated.
Image searches for "Masonic stone," "Masonic cube," etc.,
fail to yield any other pictures that look like the above image —
that of a 2x2x2 array of eight identical subcubes.
For purely mathematical — not Masonic — properties of such
an array, see "eightfold cube" in this journal.
The websites offering to sell the questionable image —
|
Getty —
|
|
Alamy —
https://www.alamy.com/
|
|
Photo12 —
https://www.photo12.com/en/image/
No price quoted on public page:
|
Comments Off on The Stone
Saturday, January 14, 2023
Châtelet on Weil — A “Space of Gestures”
|
From Gilles Châtelet, Introduction to Figuring Space Metaphysics does have a catalytic effect, which has been described in a very beautiful text by the mathematician André Weil: Nothing is more fertile, all mathematicians know, than these obscure analogies, these murky reflections of one theory in another, these furtive caresses, these inexplicable tiffs; also nothing gives as much pleasure to the researcher. A day comes when the illusion vanishes: presentiment turns into certainty … Luckily for researchers, as the fogs clear at one point, they form again at another.4 André Weil cuts to the quick here: he conjures these 'murky reflections', these 'furtive caresses', the 'theory of Galois that Lagrange touches … with his finger through a screen that he does not manage to pierce.' He is a connoisseur of these metaphysical 'fogs' whose dissipation at one point heralds their reforming at another. It would be better to talk here of a horizon that tilts thereby revealing a new space of gestures which has not as yet been elucidated and cut out as structure. 4 A. Weil, 'De la métaphysique aux mathématiques', (Oeuvres, vol. II, p. 408.) |
For gestures as fogs, see the oeuvre of Guerino Mazzola.
For some clearer remarks, see . . .
Illustrations of object and gestures
from finitegeometry.org/sc/ —
Object
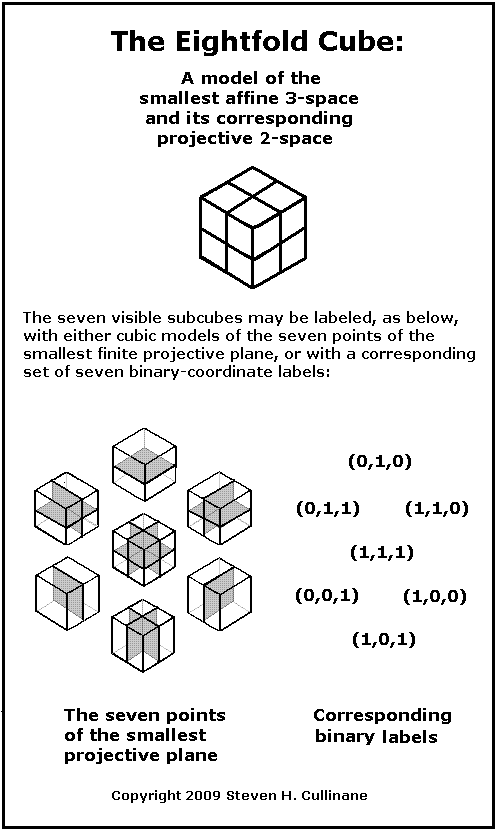
Gestures
An earlier presentation
of the above seven partitions
of the eightfold cube:
|
|
Related material: Galois.space .
Comments Off on Châtelet on Weil — A “Space of Gestures”
Monday, December 26, 2022
Super-8 Box
Comments Off on Super-8 Box
Friday, December 23, 2022
“Was ist Raum?” — Bauhaus Founder Walter Gropius
"Was ist Raum, wie können wir ihn
erfassen und gestalten?"
The Theory and
Organization of the
Bauhaus (1923)
A relevant illustration:
At math.stackexchange.com on March 1-12, 2013:
“Is there a geometric realization of the Quaternion group?” —
The above illustration, though neatly drawn, appeared under the
cloak of anonymity. No source was given for the illustrated group actions.
Possibly they stem from my Log24 posts or notes such as the Jan. 4, 2012,
note on quaternion actions at finitegeometry.org/sc (hence ultimately
from my note “GL(2,3) actions on a cube” of April 5, 1985).
These references will not appeal to those who enjoy modernism as a religion.
(For such a view, see Rosalind Krauss on grids and another writer's remarks
on the religion's 100th anniversary this year.)
Some related nihilist philosophy from Cormac McCarthy —
"Forms turning in a nameless void."
Comments Off on “Was ist Raum?” — Bauhaus Founder Walter Gropius
Tuesday, November 29, 2022
Mere Synchronology
The date — January 9, 2010 — of the Guardian book review
in the previous post was noted here by a top 40 music list
from that same date in an earlier year.
Update of 4:07 AM ET the same morning:
Fans of Cormac McCarthy's recent adventures in unreality
might enjoy interpreting the time — 3:25 AM ET — of this post
as the date 3/25, and comparing the logos, both revisited
and new, in a Log24 post from 3/25 . . .
Helen Mirren with plastic Gankyil .
. . . with the logo of a venue whose motto is
"Reality is not enough."
Comments Off on Mere Synchronology
Saturday, November 12, 2022
Inside a White Cube
For the late Brian O'Doherty, from posts now tagged "Pless Birthday 2022" —
This post was suggested by an obituary of O'Doherty and by
"The Life and Work of Vera Stepen Pless" in
Notices of the American Mathematical Society , December 2022.
Comments Off on Inside a White Cube
Tuesday, November 1, 2022
From “Goethe on All Souls’ Day”
The above title is that of a Log24 post on St. Cecilia's Day in 2017
that quoted some earlier All Souls' Day remarks from Berlin.
From that post —
Exercise: Explain why the lead article in the November issue of
Notices of the American Mathematical Society misquotes Weyl
and gives the misleading impression that the example above,
the eightfold cube , might be part of the mathematical pursuit
known as geometric group theory .
Background: Earlier instances here of the phrase "geometric group theory."
Comments Off on From “Goethe on All Souls’ Day”
Friday, October 21, 2022
Dimensional Positioning*
Comments Off on Dimensional Positioning*
Monday, October 17, 2022
Saturday, September 3, 2022
1984 Revisited
Note the three quadruplets of parallel edges in the 1984 figure above.
The above Gates article appeared earlier, in the June 2010 issue of
Physics World , with bigger illustrations. For instance —
Exercise: Describe, without seeing the rest of the article,
the rule used for connecting the balls above.
Wikipedia offers a much clearer picture of a (non-adinkra) tesseract —
And then, more simply, there is the Galois tesseract —
For parts of my own world in June 2010, see this journal for that month.
The above Galois tesseract appears there as follows:
See also the Klein correspondence in a paper from 1968
in yesterday's 2:54 PM ET post.
Comments Off on 1984 Revisited
Monday, August 1, 2022
Enowning
Related material — The Eightfold Cube.
See also . . .
"… Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to
explain how art fits into our subject and what we mean by beauty."
— Sir Michael Atiyah, “The Art of Mathematics”
in the AMS Notices , January 2010
Comments Off on Enowning
Tuesday, April 12, 2022
Dealing with Cubism
"It’s important, as art historian Reinhard Spieler has noted,
that after a brief, unproductive stay in Paris, circa 1907,
Kandinsky chose to paint in Munich. That’s where he formed
the Expressionist art group Der Blaue Reiter (The Blue Rider) —
and where he avoided having to deal with cubism."
— David Carrier,
Images from an earlier Christmas Day, in 2005 —
Comments Off on Dealing with Cubism
Friday, March 25, 2022
The Diamantova Logo
From the "Mathematics and Narrative" link in the previous post —
An image reposted here on March 12, 2022, the reported date of death
for Vera Diamantova —
Helen Mirren with plastic Gankyil .
Comments Off on The Diamantova Logo
Saturday, March 12, 2022
Geometric Theology: Logos vs. Antilogos
In a 1999 Yale doctoral dissertation,
"Diabolical Structures in the Poetics of Nikolai Gogol,"
the term "antilogos" occurs 70 times.
Students of poetic structures may compare and contrast . . .
Logos
Antilogos
Comments Off on Geometric Theology: Logos vs. Antilogos
Wednesday, March 9, 2022
Supercube Space
The new URL supercube.space forwards to http://box759.wordpress.com/.
The term supercube is from a 1982 article by Solomon W. Golomb.
The related new URL supercube.group forwards to a page that
describes how the 2x2x2 (or eightfold, or "super") cube's natural
underlying automorphism group is Klein's simple group of order 168.
For further context, see the new URL supercube.art.
For some background, see the phrase Cube Space in this journal.
Comments Off on Supercube Space
Saturday, February 12, 2022
Das Geheimnis der Einheit
Thomas Mann on "the mystery of the unity" —
"Denn um zu wiederholen, was ich anfangs sagte:
in dem Geheimnis der Einheit von Ich und Welt,
Sein und Geschehen, in der Durchschauung des
scheinbar Objectiven und Akzidentellen als
Veranstaltung der Seele glaube ich den innersten Kern
der analytischen Lehre zu erkennen." (GW IX 488)
An Einheit-Geheimnis that is perhaps* more closely related
to pure mathematics** —
"What is the nature of the original unity
that throws itself apart in this separation,
and in what sense are the separated ones
here as the essence of the abyss?
Here it cannot be a question of any kind of 'dialectic,'
but only of the essence of the ground
(that is, of truth) itself." [Tr. by Google]
" Welcher Art ist die ursprüngliche Einheit,
daß sie sich in diese Scheidung auseinanderwirft,
und in welchem Sinn sind die Geschiedenen
hier als Wesung der Ab-gründigkeit gerade einig?
Hier kann es sich nicht um irgend eine »Dialektik«
handeln, sondern nur um die Wesung des Grundes
(der Wahrheit also) selbst."
* Or perhaps not .
** For a relevant Scheidung , see Eightfold Cube.
Comments Off on Das Geheimnis der Einheit
Friday, February 11, 2022
For Space Groupies
A followup to Wednesday's post Deep Space —
Related material from this journal on July 9, 2019 —
Cube Bricks 1984 —
From "Tomorrowland" (2015) —
From other posts tagged 1984 Cubes —
Comments Off on For Space Groupies
Saturday, February 5, 2022
Mathieu Cube Labeling
Shown below is an illustration from "The Puzzle Layout Problem" —
- September 2003
-
Lecture Notes in Computer Science 2912:500-501
DOI:10.1007/978-3-540-24595-7_50 - Source: DBLP
-
Conference: Graph Drawing, 11th International Symposium,
GD 2003, Perugia, Italy, September 21-24, 2003, Revised Papers - Authors: Kozo Sugiyama, Seok-Hee Hong, Atsuhiko Maeda
Exercise: Using the above numerals 1 through 24
(with 23 as 0 and 24 as ∞) to represent the points
∞, 0, 1, 2, 3 … 22 of the projective line over GF(23),
reposition the labels 1 through 24 in the above illustration
so that they appropriately* illustrate the cube-parts discussed
by Iain Aitchison in his March 2018 Hiroshima slides on
cube-part permutations by the Mathieu group M24.
A note for Northrop Frye —
Interpenetration in the eightfold cube — the three midplanes —
A deeper example of interpenetration:
Aitchison has shown that the Mathieu group M24 has a natural
action on the 24 center points of the subsquares on the eightfold
cube's six faces (four such points on each of the six faces). Thus
the 759 octads of the Steiner system S(5, 8, 24) interpenetrate
on the surface of the cube.
* "Appropriately" — I.e. , so that the Aitchison cube octads correspond
exactly, via the projective-point labels, to the Curtis MOG octads.
Comments Off on Mathieu Cube Labeling
Friday, December 31, 2021
Aesthetics in Academia
Related art — The non-Rubik 3x3x3 cube —
The above structure illustrates the affine space of three dimensions
over the three-element finite (i.e., Galois) field, GF(3). Enthusiasts
of Judith Brown's nihilistic philosophy may note the "radiance" of the
13 axes of symmetry within the "central, structuring" subcube.
I prefer the radiance (in the sense of Aquinas) of the central, structuring
eightfold cube at the center of the affine space of six dimensions over
the two-element field GF(2).
Comments Off on Aesthetics in Academia
Thursday, December 30, 2021
Antidote to Chaos?
Some formal symmetry —
|
"… each 2×4 "brick" in the 1974 Miracle Octad Generator
Folding a 2×4 Curtis array yet again yields — Steven H. Cullinane on April 19, 2016 — The Folding. |
Related art-historical remarks:
The Shape of Time (Kubler, Yale U.P., 1962).
See yesterday's post The Thing .
Comments Off on Antidote to Chaos?
Monday, October 18, 2021
Star Logo for the Feast of St. Luke (Skywalker)
See Leiber in this journal.
Comments Off on Star Logo for the Feast of St. Luke (Skywalker)
Tuesday, October 5, 2021
Wednesday, September 15, 2021
The Razor and the Touchstone
It is often good to remember that writers of headlines (and subheadlines)
are usually not the same people as the authors of the following texts.
In particular, in the above example, neither the word "touchstone" nor
the use of "enquires" to mean "enquiries" appears in the text proper.
Still, the mixed metaphor of "razor" as "touchstone" is not without interest.
See The Eightfold Cube and Modernist Cuts.
Comments Off on The Razor and the Touchstone
Wednesday, August 18, 2021
Eight the Great
Starring J. J. Abrams as Leonhard Euler?
Related material —
The Cornell cap in the recent HBO "White Lotus" —
"I'm just playing the hand I was dealt."
Comments Off on Eight the Great
Monday, August 9, 2021
The Tune (Suggested by “Hum: Seek the Void”)
"Two years ago . . . ." — Synopsis of the August 3 film "Hum"
Two years ago on August 3 . . .
What is going on in this picture?
The above is an image from
the August 3, 2019,
post "Butterfield's Eight."
"Within the week . . . ."
— The above synopsis of "Hum"
This suggests a review of a post
from August 5, 2019, that might
be retitled . . .
"The void she knows,
the tune she hums."
Comments Off on The Tune (Suggested by “Hum: Seek the Void”)
Saturday, May 1, 2021
Monday, April 26, 2021
Desperately Seeking Symmetry
RA Wilson —”[Submitted on 20 Apr 2021 (v1),
last revised 23 Apr 2021 (this version, v2)]”
SH Cullinane — See as well
box759.wordpress.com.
Comments Off on Desperately Seeking Symmetry
Wednesday, April 7, 2021
Timeless Capsules
Drilling down . . .
My own, more abstract, academic interests are indicated by
a post from this journal on January 20, 2020 —
Dyadic Harmonic Analysis: The Fourfold Square and Eightfold Cube.
Those poetically inclined may regard that post as an instance of the
“intersection of the timeless with time.”
Comments Off on Timeless Capsules
Saturday, March 13, 2021
Eternal Spark
According to Lt. Col. Wayne M. McDonnell in June 1983 —
“… it is accurate to observe that when a person experiences
the out-of- body state he is, in fact, projecting that eternal spark
of consciousness and memory which constitutes the ultimate
source of his identity….”
— Section 27, “Consciousness in Perspective,” of
“Analysis and Assessment of Gateway Process.”
A related quotation —
“In truth, the physical AllSpark is but a shell….”
— https://tfwiki.net/wiki/AllSpark
From the post Ghost in the Shell (Feb. 26, 2019) —
See also, from posts tagged Ogdoad Space —
“Like the Valentinian Ogdoad— a self-creating theogonic system
of eight Aeons in four begetting pairs— the projected eightfold work
had an esoteric, gnostic quality; much of Frye’s formal interest lay in
the ‘schematosis’ and fearful symmetries of his own presentations.”
— From p. 61 of James C. Nohrnberg’s “The Master of the Myth
of Literature: An Interpenetrative Ogdoad for Northrop Frye,”
Comparative Literature , Vol. 53 No. 1, pp. 58-82, Duke University
Press (quarterly, January 2001)
— as well as . . .
Related illustration from posts tagged with
the quilt term Yankee Puzzle —
Comments Off on Eternal Spark
Friday, March 12, 2021
Grid
See Trinity Cube in this journal and . . .
McDonnell’s illustration is from 9 June 1983.
See as well a less official note from later that June.
Comments Off on Grid
Friday, December 25, 2020
Circle of Positivity
“A quick note on terminology. Members of the Circle
were logical empiricists, sometimes called logical positivists.
Positivism is the view that our knowledge derives from
the natural world and includes the idea that we can have
positive knowledge of it. The Circle combined this position
with the use of modern logic; the aim was to build a new
philosophy.”
— Edmonds, David. The Murder of Professor Schlick (p. vii).
Princeton University Press. Kindle Edition.
For aficionados of associative logic —
See Triple Cross in this journal and the Fano-plane circle
in the illustration below.
Comments Off on Circle of Positivity
Change Arises: Mathematical Examples
From old posts tagged Change Arises —
|
From Christmas 2005:
For the eightfold cube
For an rather more
Click on image for details. |
The phrase "change arises" is from Arkani-Hamed in 2013, describing
calculations in physics related to properties of the positive Grassmannian —
A related recent illustration from Quanta Magazine —
The above illustration of seven cells is not unrelated to
the eightfold-cube model of the seven projective points in
the Fano plane.
Comments Off on Change Arises: Mathematical Examples
Tuesday, December 15, 2020
Connection
Hurt’s dies natalis (date of death, in the saints’ sense) was,
it now seems, 25 January 2017, not 27.
A connection, for fantasy fans, between the Philosopher’s Stone
(represented by the eightfold cube) and the Deathly Hallows
(represented by the usual Fano-plane figure) —
Images from a Log24 search for “Holocron.”
Comments Off on Connection
Sunday, November 22, 2020
The Galois-Fano Plane
A figure adapted from “Magic Fano Planes,” by
Ben Miesner and David Nash, Pi Mu Epsilon Journal
Vol. 14, No. 1, 1914, CENTENNIAL ISSUE 3 2014
(Fall 2014), pp. 23-29 (7 pages) —
Related material — The Eightfold Cube.
Update at 10:51 PM ET the same day —
Essentially the same figure as above appears also in
the second arXiv version (11 Jan. 2016) of . . .
DAVID A. NASH, and JONATHAN NEEDLEMAN.
“When Are Finite Projective Planes Magic?”
Mathematics Magazine, vol. 89, no. 2, 2016, pp. 83–91.
JSTOR, www.jstor.org/stable/10.4169/math.mag.89.2.83.
Comments Off on The Galois-Fano Plane
Saturday, October 17, 2020
Modernist Cuts
"The bond with reality is cut."
— Hans Freudenthal, 1962
Related screenshot of a book review
from the November AMS Notices —
Comments Off on Modernist Cuts
Thursday, September 17, 2020
Structure and Mutability . . .
Continues in The New York Times :
"One day — 'I don’t know exactly why,' he writes — he tried to
put together eight cubes so that they could stick together but
also move around, exchanging places. He made the cubes out
of wood, then drilled a hole in the corners of the cubes to link
them together. The object quickly fell apart.
Many iterations later, Rubik figured out the unique design
that allowed him to build something paradoxical:
a solid, static object that is also fluid…." — Alexandra Alter
Another such object: the eightfold cube .
Comments Off on Structure and Mutability . . .
Tuesday, September 8, 2020
“The Eight” according to Coleridge
Metaphysical ruminations of Coleridge that might be applied to
the eightfold cube —
See also "Sprechen Sie Neutsch?".
Update of December 29, 2022 —
Comments Off on “The Eight” according to Coleridge
Saturday, September 5, 2020
Ikonologie des Zwischenraums
The title is from a Cornell page in the previous post.
Related material (click to enlarge) —
The above remarks on primitive mentality suggest
a review of Snakes on a Plane.
Comments Off on Ikonologie des Zwischenraums
Wednesday, August 26, 2020
Sunday, May 17, 2020
“The Ultimate Epistemological Fact”
"Let me say this about that." — Richard Nixon
Interpenetration in Weyl's epistemology —
Interpenetration in Mazzola's music theory —
Interpenetration in the eightfold cube — the three midplanes —
A deeper example of interpenetration:
Aitchison has shown that the Mathieu group M24 has a natural
action on the 24 center points of the subsquares on the eightfold
cube's six faces (four such points on each of the six faces). Thus
the 759 octads of the Steiner system S(5, 8, 24) interpenetrate
on the surface of the cube.
Comments Off on “The Ultimate Epistemological Fact”
Thursday, March 5, 2020
“Generated by Reflections”
See the title in this journal.
Such generation occurs both in Euclidean space …
… and in some Galois spaces —
.
In Galois spaces, some care must be taken in defining "reflection."
Comments Off on “Generated by Reflections”
Sunday, March 1, 2020
Same Staircase, Different Day
Freeman Dyson on his staircase at Trinity College
(University of Cambridge) and on Ludwig Wittgenstein:
“I held him in the highest respect and was delighted
to find him living in a room above mine on the same
staircase. I frequently met him walking up or down
the stairs, but I was too shy to start a conversation.”
Frank Close on Ron Shaw:
“Shaw arrived there in 1949 and moved into room K9,
overlooking Jesus Lane. There is nothing particularly
special about this room other than the coincidence that
its previous occupant was Freeman Dyson.”
— Close, Frank. The Infinity Puzzle (p. 78).
Basic Books. Kindle Edition.
See also other posts now tagged Trinity Staircase.
Illuminati enthusiasts may enjoy the following image:
Comments Off on Same Staircase, Different Day
Saturday, February 29, 2020
Template
|
Roberta Smith on Donald Judd’s BY ALEX GREENBERGER February 28, 2020 1:04pm If Minimalist artist Donald Judd is known as a writer at all, it’s likely for one important text— his 1965 essay “Specific Objects,” in which he observed the rise of a new kind of art that collapsed divisions between painting, sculpture, and other mediums. But Judd was a prolific critic, penning shrewd reviews for various publications throughout his career—including ARTnews . With a Judd retrospective going on view this Sunday at the Museum of Modern Art in New York, ARTnews asked New York Times co-chief art critic Roberta Smith— who, early in her career, worked for Judd as his assistant— to comment on a few of Judd’s ARTnews reviews. How would she describe his critical style? “In a word,” she said, “great.” . . . . |
And then there is Temple Eight, or Ex Fano Apollinis —
Cicero, In Verrem II. 1. 46 —
He reached Delos. There one night he secretly 46 carried off, from the much-revered sanctuary of Apollo, several ancient and beautiful statues, and had them put on board his own transport. Next day, when the inhabitants of Delos saw their sanc- tuary stripped of its treasures, they were much distressed . . . .
Delum venit. Ibi ex fano Apollinis religiosissimo noctu clam sustulit signa pulcherrima atque anti- quissima, eaque in onerariam navem suam conicienda curavit. Postridie cum fanum spoliatum viderent ii qui Delum incolebant, graviter ferebant . . . .
Comments Off on Template
Thursday, February 27, 2020
Occult Writings
From the author who in 2001 described "God's fingerprint"
(see the previous post) —
From the same publisher —
From other posts tagged Triskele in this journal —
Other geometry for enthusiasts of the esoteric —
|
Monday, November 4, 2019
As Above, So Below*
|
|
|
Comments Off on Occult Writings
Sunday, February 23, 2020
The Representation of Reality
"Although art is fundamentally everywhere and always the same,
nevertheless two main human inclinations, diametrically opposed
to each other, appear in its many and varied expressions. ….
The first aims at representing reality objectively, the second subjectively."
— Mondrian, 1936 [Links added.]
An image search today (click to enlarge) —
Comments Off on The Representation of Reality
Wednesday, February 19, 2020
Aitchison’s Octads
The 759 octads of the Steiner system S(5,8,24) are displayed
rather neatly in the Miracle Octad Generator of R. T. Curtis.
A March 9, 2018, construction by Iain Aitchison* pictures the
759 octads on the faces of a cube , with octad elements the
24 edges of a cuboctahedron :
The Curtis octads are related to symmetries of the square.
See my webpage "Geometry of the 4×4 square" from March 2004.
Aitchison's p. 42 slide includes an illustration from that page —
Aitchison's octads are instead related to symmetries of the cube.
Note that essentially the same model as Aitchison's can be pictured
by using, instead of the 24 edges of a cuboctahedron, the 24 outer
faces of subcubes in the eightfold cube .
Image from Christmas Day 2005.
* http://www.math.sci.hiroshima-u.ac.jp/branched/files/2018/
presentations/Aitchison-Hiroshima-2-2018.pdf.
See also Aitchison in this journal.
[addtoany]
Comments Off on Aitchison’s Octads
Wednesday, February 12, 2020
The Reality Bond
The plane at left is modeled naturally by
seven types of “cuts” in the cube at right.
Comments Off on The Reality Bond
Sunday, February 2, 2020
Tetrads for McLuhan, or “Blame It on Video”
"I like to put people on myself by skipping logical steps
in the conversation until they're dizzy." — Jemima Brown
in The Eiger Sanction
Related posts — See "McLuhan Tetrad" in this journal.
Related theology — See "The Meaning of Perichoresis."
Background — The New Yorker , "On Religion:
Richard Rohr Reorders the Universe," by Eliza Griswold
on February 2, 2020, and a different reordering in posts
tagged Eightfold Metaphysics.
Comments Off on Tetrads for McLuhan, or “Blame It on Video”
Sunday, January 26, 2020
Harmonic-Analysis Building Blocks
Comments Off on Harmonic-Analysis Building Blocks
Monday, January 20, 2020
Dyadic Harmonic Analysis:
The Fourfold Square and Eightfold Cube
Related material: A Google image search for “field dream” + log24.
Comments Off on Dyadic Harmonic Analysis:
Thursday, January 2, 2020
Wednesday, January 1, 2020
Saturday, December 14, 2019
Colorful Tale
The above image is from
"A Four-Color Theorem:
Function Decomposition Over a Finite Field,"
http://finitegeometry.org/sc/gen/mapsys.html.
These partitions of an 8-set into four 2-sets
occur also in Wednesday night's post
Miracle Octad Generator Structure.
This post was suggested by a Daily News
story from August 8, 2011, and by a Log24
post from that same date, "Organizing the
Mine Workers" —
Comments Off on Colorful Tale
Wednesday, December 11, 2019
Miracle Octad Generator Structure
(Adapted from Eightfold Geometry, a note of April 28, 2010.
See also the recent post Geometry of 6 and 8.)
Comments Off on Miracle Octad Generator Structure
Sunday, December 8, 2019
Geometry of 6 and 8
Just as
the finite space PG(3,2) is
the geometry of the 6-set, so is
the finite space PG(5,2)
the geometry of the 8-set.*
Selah.
* Consider, for the 6-set, the 32
(16, modulo complementation)
0-, 2-, 4-, and 6-subsets,
and, for the 8-set, the 128
(64, modulo complementation)
0-, 2-, 4-, 6-, and 8-subsets.
Update of 11:02 AM ET the same day:
See also Eightfold Geometry, a note from 2010.
Comments Off on Geometry of 6 and 8
Sunday, October 27, 2019
Friday Night Lights
Entertainment from NBC on Friday night —
The above question, and Saturday morning's post on a film director
from Melbourne, suggest an image from December's Melbourne Noir —
(March 8, 2018, was the date of death for Melbourne author Peter Temple.)
Comments Off on Friday Night Lights
Saturday, October 26, 2019
Director’s Cut
The title was suggested by the previous post and by
the title illustration in the weblog of the director,
Leigh Whannell, of the 2018 film “Upgrade.”
Related visual details —
For the Church of Synchronology —
Related remarks: “The Thing and I.”
Comments Off on Director’s Cut
Wednesday, October 9, 2019
Philosophical Infanticide
From Wallace Stevens —
"Reality is the beginning not the end,
Naked Alpha, not the hierophant Omega,
Of dense investiture, with luminous vassals."
— “An Ordinary Evening in New Haven” VI
From The Point magazine yesterday, October 8, 2019 —
Parricide: On Irad Kimhi's Thinking and Being .
Book review by Steven Methven.
The conclusion:
"Parricide is nothing that the philosopher need fear . . . .
What sustains can be no threat. Perhaps what the
unique genesis of this extraordinary work suggests is that
the true threat to philosophy is infanticide."
This remark suggests revisiting a post from Monday —
|
Monday, October 7, 2019
Berlekamp Garden vs. Kinder Garten
|
Comments Off on Philosophical Infanticide
Monday, October 7, 2019
Berlekamp Garden vs. Kinder Garten
Stevens's Omega and Alpha (see previous post) suggest a review.
Omega — The Berlekamp Garden. See Misère Play (April 8, 2019).
Alpha — The Kinder Garten. See Eighfold Cube.
Illustrations —
The sculpture above illustrates Klein's order-168 simple group.
So does the sculpture below.
Cube Bricks 1984 —
Comments Off on Berlekamp Garden vs. Kinder Garten
Tuesday, October 1, 2019
Hijacking the Vatican
Cover illustration— Arithmetic and Music,
Borgia Apartments, the Vatican.
See also Rothstein in this journal.
Related posts: The Eightfold Hijacking.
Comments Off on Hijacking the Vatican
Sunday, September 29, 2019
Spiritual Kin
"The 15 Puzzle and the Magic Cube
are spiritual kin …."
— "Metamagical Themas" column,
Douglas R. Hofstadter, Scientific American ,
Vol. 244, No. 3 (March 1981), pp. 20-39
As are the 15 Schoolgirls and the Eightfold Cube.
Comments Off on Spiritual Kin
Thursday, August 8, 2019
Saturday, August 3, 2019
Tuesday, July 16, 2019
Morf Vandewalt, Social Prism
Comments Off on Morf Vandewalt, Social Prism
Tuesday, July 9, 2019
Schoolgirl Space: 1984 Revisited
Cube Bricks 1984 —
From "Tomorrowland" (2015) —
From John Baez (2018) —
See also this morning's post Perception of Space
and yesterday's Exploring Schoolgirl Space.
Comments Off on Schoolgirl Space: 1984 Revisited
Thursday, June 20, 2019
Tuesday, June 18, 2019
Paris Review
"The loveliness of Paris seems somehow sadly gay." — Song lyric
Stewart also starred in "Equals" (2016). From a synopsis —
"Stewart plays Nia, a writer who works at a company that extols
the virtues of space exploration in a post-apocalyptic society.
She falls in love with the film's main character, Silas (Nicholas Hoult),
an illustrator . . . ."
Space art in The Paris Review —
For a different sort of space exploration, see Eightfold 1984.
Comments Off on Paris Review
Thursday, June 13, 2019
The Reality Blocks
The new Log24 tag "Eightfold Metaphysics" used in the previous post
suggests a review of posts that were tagged "The Reality Blocks" on May 24.
Then there is, of course, the May 24 death of Murray Gell-Mann, who
hijacked from Buddhism the phrase "eightfold way."
See Gell-Mann in this journal and May 24, 2003.
Comments Off on The Reality Blocks
Sunday, May 26, 2019
Burning Bright
Compare and contrast with . . .
The Brightburn Logo:
Related material from the May 12 post
"The Collective Unconscious
in a Cartoon Graveyard" —
|
"When they all finally reach their destination — " When asked about the film's similarities to the 2015 Disney movie Tomorrowland , which also posits a futuristic world that exists in an alternative dimension, Nichols sighed. 'I was a little bummed, I guess,' he said of when he first learned about the project. . . . 'Our die was cast. Sometimes this kind of collective unconscious that we're all dabbling in, sometimes you're not the first one out of the gate.' " |
Comments Off on Burning Bright
Sunday, May 19, 2019
The Building Blocks of Geometry
From "On the life and scientific work of Gino Fano"
by Alberto Collino, Alberto Conte, and Alessandro Verra,
ICCM Notices , July 2014, Vol. 2 No. 1, pp. 43-57 —
|
" Indeed, about the Italian debate on foundations of Geometry, it is not rare to read comments in the same spirit of the following one, due to Jeremy Gray13. He is essentially reporting Hans Freudenthal’s point of view: ' When the distinguished mathematician and historian of mathematics Hans Freudenthal analysed Hilbert’s Grundlagen he argued that the link between reality and geometry appears to be severed for the first time in Hilbert’s work. However, he discovered that Hilbert had been preceded by the Italian mathematician Gino Fano in 1892. . . .' " 13 J. Gray, "The Foundations of Projective Geometry in Italy," Chapter 24 (pp. 269–279) in his book Worlds Out of Nothing , Springer (2010). |
Restoring the severed link —
See also Espacement and The Thing and I.
Related material —
[addtoany]
Comments Off on The Building Blocks of Geometry
Monday, May 6, 2019
One Stuff
Comments Off on One Stuff
Saturday, May 4, 2019
Inside the White Cube
See also Espacement and The Thing and I.
Comments Off on Inside the White Cube
Thursday, April 18, 2019
Expanding the Unfolding*
From a New York Times book review of a new novel about
Timothy Leary that was in the Times online on April 10 —
"Most of the novel resides in the perspective
of Fitzhugh Loney, one of Leary’s graduate students."
"A version of this article appears in print on ,
on Page 10 of the Sunday Book Review with the headline:
Strange Days."
For material about one of Leary's non -fictional grad students,
Ralph Metzner, see posts now tagged Metzner's Pi Day.
Related material —
The reported publication date of Searching for the Philosophers' Stone
was January 1, 2019.
A related search published here on that date:
* Title suggested by two of Ralph Metzner's titles,
The Expansion of Consciousness and The Unfolding Self .
Comments Off on Expanding the Unfolding*
Monday, April 8, 2019
Misère Play
Facebook on Bloomsday 2017 —
Also on that Bloomsday —
Chalkroom Jungle Revisited —
Comments Off on Misère Play
Sunday, April 7, 2019
Wednesday, April 3, 2019
Nocturnal Object of Beauty
What is going on in this picture?
Comments Off on Nocturnal Object of Beauty
Monday, March 25, 2019
Espacement
(Continued from the previous post.)
|
In-Between "Spacing" and the "Chôra " (Ch. 2 in Henk Oosterling & Ewa Plonowska Ziarek (Eds.), Intermedialities: Philosophy, Arts, Politics , Lexington Books, October 14, 2010) "The term 'spacing' ('espacement ') is absolutely central to Derrida's entire corpus, where it is indissociable from those of différance (characterized, in the text from 1968 bearing this name, as '[at once] spacing [and] temporizing' 1), writing (of which 'spacing' is said to be 'the fundamental property' 2) and deconstruction (with one of Derrida's last major texts, Le Toucher: Jean-Luc Nancy , specifying 'spacing ' to be 'the first word of any deconstruction' 3)." 1 Jacques Derrida, “La Différance,” in Marges – de la philosophie (Paris: Minuit, 1972), p. 14. Henceforth cited as D . 2 Jacques Derrida, “Freud and the Scene of Writing,” trans. A. Bass, in Writing and Difference (Chicago: University of Chicago Press, 1978), p. 217. Henceforth cited as FSW . 3 Jacques Derrida, Le Toucher, Jean-Luc Nancy (Paris: Galilée, 2000), p. 207. . . . . "… a particularly interesting point is made in this respect by the French philosopher, Michel Haar. After remarking that the force Derrida attributes to différance consists simply of the series of its effects, and is, for this reason, 'an indefinite process of substitutions or permutations,' Haar specifies that, for this process to be something other than a simple 'actualisation' lacking any real power of effectivity, it would need “a soubassement porteur ' – let’s say a 'conducting underlay' or 'conducting medium' which would not, however, be an absolute base, nor an 'origin' or 'cause.' If then, as Haar concludes, différance and spacing show themselves to belong to 'a pure Apollonism' 'haunted by the groundless ground,' which they lack and deprive themselves of,16 we can better understand both the threat posed by the 'figures' of space and the mother in the Timaeus and, as a result, Derrida’s insistent attempts to disqualify them. So great, it would seem, is the menace to différance that Derrida must, in a 'properly' apotropaic gesture, ward off these 'figures' of an archaic, chthonic, spatial matrix in any and all ways possible…." 16 Michel Haar, “Le jeu de Nietzsche dans Derrida,” Revue philosophique de la France et de l’Etranger 2 (1990): 207-227. . . . . … "The conclusion to be drawn from Democritus' conception of rhuthmos , as well as from Plato's conception of the chôra , is not, therefore, as Derrida would have it, that a differential field understood as an originary site of inscription would 'produce' the spatiality of space but, on the contrary, that 'differentiation in general' depends upon a certain 'spatial milieu' – what Haar would name a 'groundless ground' – revealed as such to be an 'in-between' more 'originary' than the play of differences it in-forms. As such, this conclusion obviously extends beyond Derrida's conception of 'spacing,' encompassing contemporary philosophy's continual privileging of temporization in its elaboration of a pre-ontological 'opening' – or, shall we say, 'in-between.' |
For permutations and a possible "groundless ground," see
the eightfold cube and group actions both on a set of eight
building blocks arranged in a cube (a "conducting base") and
on the set of seven natural interstices (espacements ) between
the blocks. Such group actions provide an elementary picture of
the isomorphism between the groups PSL(2,7) (acting on the
eight blocks) and GL(3,2) (acting on the seven interstices).
Espacements
For the Church of Synchronology —
See also, from the reported publication date of the above book
Intermedialities , the Log24 post Synchronicity.
Comments Off on Espacement