(An error in Fig. 4 was corrected at about
10:25 AM ET on Tuesday, Oct. 6, 2020.)
Tuesday, October 6, 2020
Spreads via the Knight Cycle
Sunday, December 22, 2019
M24 from the Eightfold Cube
Exercise: Use the Guitart 7-cycles below to relate the 56 triples
in an 8-set (such as the eightfold cube) to the 56 triangles in
a well-known Klein-quartic hyperbolic-plane tiling. Then use
the correspondence of the triples with the 56 spreads of PG(3,2)
to construct M24.
Click image below to download a Guitart PowerPoint presentation.
See as well earlier posts also tagged Triangles, Spreads, Mathieu.
Friday, December 20, 2019
Triangles, Spreads, Mathieu…
Friday, November 22, 2019
Triangles, Spreads, Mathieu …
Thursday, October 31, 2019
56 Triangles
The post "Triangles, Spreads, Mathieu" of October 29 has been
updated with an illustration from the Curtis Miracle Octad Generator.
Related material — A search in this journal for "56 Triangles."
Tuesday, October 29, 2019
Triangles, Spreads, Mathieu
There are many approaches to constructing the Mathieu
group M24. The exercise below sketches an approach that
may or may not be new.
Exercise:
It is well-known that …
There are 56 triangles in an 8-set.
There are 56 spreads in PG(3,2).
The alternating group An is generated by 3-cycles.
The alternating group A8 is isomorphic to GL(4,2).
Use the above facts, along with the correspondence
described below, to construct M24.
Some background —
A Log24 post of May 19, 2013, cites …
Peter J. Cameron in a 1976 Cambridge U. Press
book — Parallelisms of Complete Designs .
See the proof of Theorem 3A.13 on pp. 59 and 60.

See also a Google search for "56 triangles" "56 spreads" Mathieu.
Update of October 31, 2019 — A related illustration —

Update of November 2, 2019 —
See also p. 284 of Geometry and Combinatorics:
Selected Works of J. J. Seidel (Academic Press, 1991).
That page is from a paper published in 1970.
Update of December 20, 2019 —
Thursday, December 6, 2018
The Mathieu Cube of Iain Aitchison
This journal ten years ago today —
Surprise Package

From a talk by a Melbourne mathematician on March 9, 2018 —

The source — Talk II below —
Search Results
|
Related material —
The 56 triangles of the eightfold cube . . .
- in Aitchison's March 9, 2018, talk (slides 32-34), and
- in this journal on July 25, 2008, and later.
Image from Christmas Day 2005.
Sunday, July 1, 2018
Deutsche Ordnung
The title is from a phrase spoken, notably, by Yul Brynner
to Christopher Plummer in the 1966 film “Triple Cross.”
Related structures —
Greg Egan’s animated image of the Klein quartic —
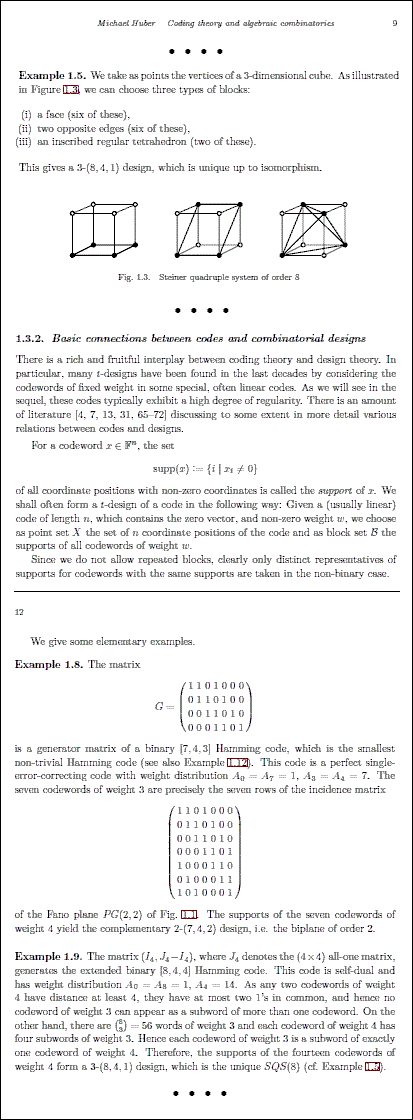
For a smaller tetrahedral arrangement, within the Steiner quadruple
system of order 8 modeled by the eightfold cube, see a book chapter
by Michael Huber of Tübingen —

For further details, see the June 29 post Triangles in the Eightfold Cube.
See also, from an April 2013 philosophical conference:
| Abstract for a talk at the City University of New York:
The Experience of Meaning Once the question of truth is settled, and often prior to it, what we value in a mathematical proof or conjecture is what we value in a work of lyric art: potency of meaning. An absence of clutter is a feature of such artifacts: they possess a resonant clarity that allows their meaning to break on our inner eye like light. But this absence of clutter is not tantamount to ‘being simple’: consider Eliot’s Four Quartets or Mozart’s late symphonies. Some truths are complex, and they are simplified at the cost of distortion, at the cost of ceasing to be truths. Nonetheless, it’s often possible to express a complex truth in a way that precipitates a powerful experience of meaning. It is that experience we seek — not simplicity per se , but the flash of insight, the sense we’ve seen into the heart of things. I’ll first try to say something about what is involved in such recognitions; and then something about why an absence of clutter matters to them. |
For the talk itself, see a YouTube video.
The conference talks also appear in a book.
The book begins with an epigraph by Hilbert —

Friday, June 29, 2018
Triangles in the Eightfold Cube
From a post of July 25, 2008, “56 Triangles,” on the Klein quartic
and the eightfold cube —
“Baez’s discussion says that the Klein quartic’s 56 triangles
can be partitioned into 7 eight-triangle Egan ‘cubes’ that
correspond to the 7 points of the Fano plane in such a way
that automorphisms of the Klein quartic correspond to
automorphisms of the Fano plane. Show that the
56 triangles within the eightfold cube can also be partitioned
into 7 eight-triangle sets that correspond to the 7 points of the
Fano plane in such a way that (affine) transformations of the
eightfold cube induce (projective) automorphisms of the Fano plane.”
Related material from 1975 —
More recently …
Friday, July 25, 2008
Friday July 25, 2008
56 Triangles

"This wonderful picture was drawn by Greg Egan with the help of ideas from Mike Stay and Gerard Westendorp. It's probably the best way for a nonmathematician to appreciate the symmetry of Klein's quartic. It's a 3-holed torus, but drawn in a way that emphasizes the tetrahedral symmetry lurking in this surface! You can see there are 56 triangles: 2 for each of the tetrahedron's 4 corners, and 8 for each of its 6 edges."
Exercise:
Click on image for further details.
Note that if eight points are arranged
in a cube (like the centers of the
eight subcubes in the figure above),
there are 56 triangles formed by
the 8 points taken 3 at a time.