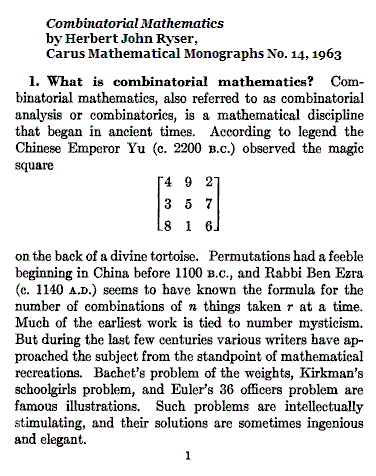
For the significance of the date "February 20," see . . .
|
Google One: Gemini Advanced AI on 13 May 2024. Prompt: How can Weyl's 1946 remarks on coordinatization in "The Classical Groups" be applied to models of finite geometries? Response (after several drafts): In "The Classical Groups," Hermann Weyl discusses the concept of coordinatization, which is the process of assigning numerical coordinates to points in a geometric space. This process is fundamental in connecting abstract geometric concepts with concrete numerical representations, allowing for algebraic manipulation and analysis. Weyl's remarks on coordinatization have significant implications for models of finite geometries. Finite geometries are geometric systems with a finite number of points and lines, and they often exhibit different properties compared to traditional Euclidean geometry. Here's how Weyl's ideas can be applied to finite geometries: 1. **Relativity of Coordinatization:** Weyl emphasizes that the choice of coordinate system is arbitrary and that different coordinate systems can be used to describe the same geometric object. This relativity of coordinatization is particularly relevant in finite geometries, where multiple coordinate systems may exist, each with its own advantages and disadvantages. 2. **Equivalent Coordinatizations:** Weyl introduces the concept of "equivalent coordinatizations," which are different coordinate systems that can be transformed into one another through a group of transformations. In finite geometries, identifying and understanding these equivalent coordinatizations can provide deeper insights into the underlying structure and symmetries of the geometry. 3. **Objective Class of Coordinatizations:** Weyl discusses the problem of fixing an "objective class" of coordinatizations that capture essential features of the geometry while being independent of arbitrary choices. In finite geometries, this could involve identifying coordinatizations that best reflect the specific properties and relationships within the geometry. 4. **Group of Transformations:** Weyl emphasizes the role of the "group of transformations" that mediates between equivalent coordinatizations. In finite geometries, understanding these transformation groups can help classify different types of finite geometries and reveal their underlying symmetries. By applying Weyl's insights, researchers can gain a deeper understanding of finite geometries, including their classification, properties, and relationships. Additionally, exploring different coordinatization schemes can lead to new algebraic and geometric tools for studying finite geometries. For further exploration, you can delve into the works of other mathematicians like Steven H. Cullinane, who has written extensively on finite geometry and its connections to relativity and other areas of mathematics. I hope this explanation is helpful! Please let me know if you have any further questions. |
Update at about 5 PM ET the same day . . .
|
Prompt: Correction: Cullinane has NOT written on finite geometry's "connections to relativity." He has, instead, quoted Weyl's remarks on what Weyl himself called "the relativity problem," but what was in fact his own term for problems involving coordinatization. Such problems are of interest both in physics and in finite geometry, but the latter is a field of pure mathematics, NOT of physics. Response: You are absolutely correct. I apologize for the error in my previous response. Steven H. Cullinane has indeed quoted Weyl's remarks on "the relativity problem" in the context of finite geometry. However, as you rightly pointed out, this term refers to Weyl's own terminology for problems involving coordinatization and not the theory of relativity in physics. Finite geometry is a field of pure mathematics and does not directly connect to the physical theories of relativity. While both areas involve concepts of space and transformations, they operate in fundamentally different domains. Cullinane's work focuses on applying Weyl's insights on coordinatization to the specific challenges and structures within finite geometries. This involves exploring different coordinatization schemes, understanding their equivalences, and identifying transformation groups that reveal the underlying symmetries of finite geometries. Thank you for pointing out this important distinction. It highlights the importance of precise language and accurate attribution when discussing complex mathematical concepts. |







 .
.