-GL(3,2)-Oct-2009.jpg)
Tuesday, August 14, 2018
D8ing
Comments Off on D8ing
Monday, August 13, 2018
Trojan Horsitude
|
"It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy. It structures Plato's (realist) reaction to the sophists (nominalists). What is often called 'postmodernism' is really just nominalism, colourfully presented as the doctrine that there is nothing except texts. It is the variety of nominalism represented in many modern humanities, paralysing appeals to reason and truth."
— Simon Blackburn, Think, |
". . . a perfect triptych of horsitude"
— James Parker on the 2007 film "Michael Clayton"
Related material —
Horsitude in the 4×2 grid, and
Comments Off on Trojan Horsitude
Wednesday, August 8, 2018
Synchronology
Dates from math forums mentioned in the previous post —
Dec. 7, 2016 and Aug. 2, 2010.
Connoisseurs of synchonology may like to click on those dates.
Comments Off on Synchronology
8/8
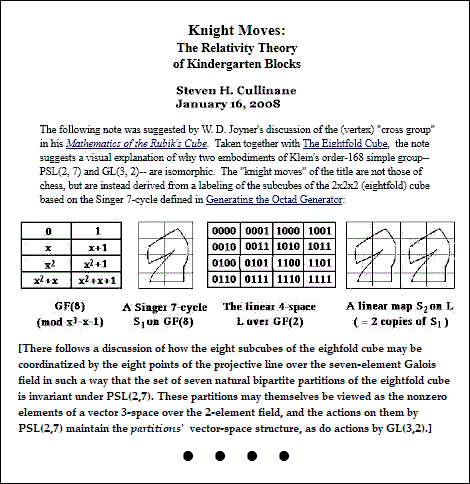
From mathoverflow.net on Dec. 7, 2016 —
The exceptional isomorphism between
PGL(3,2) and PSL(2,7): geometric origin?
Essentially the same question was asked earlier at
math.stackexchange.com on Aug. 2, 2010.
See also this journal in November 2017 —
"Read something that means something."
— New Yorker ad
.
Background — Relativity Problem in Log24.
Comments Off on 8/8
Sunday, December 17, 2017
Concrete Universals
The remarks on universals in the previous post linked to the following
note by James Hillman:
James Hillman, Re-Visioning Psychology ,
Harper Collins, 1977, p. 155 —
"Myths also make concrete particulars into universals,
so that each image, name, thing in my life when
experienced mythically takes on universal sense,
and all abstract universals, the grand ideas of
human fate, are presented as concrete actions."
[See note 48.]
Note 48: Cf. P. Wheelwright's discussion of concrete universality
in The Burning Fountain (Bloomington, Ind.: Indiana University
Press, 1968), pp. 52-54.
For Wheelwright's discussion, see the following excerpts from his book:
Comments Off on Concrete Universals
Saturday, December 16, 2017
Dagger Definitions (Review)
The previous post suggests a review of
the philosophical concept of universals —
A part of the above-mentioned 2011 "Saturday evening's post" that is
relevant to the illustration at the end of today's previous post —
Note the whatness of Singer's dagger definitions —
Comments Off on Dagger Definitions (Review)
Triptychs
Two readings by James Parker —
From next year’s first Atlantic issue
From last month’s Atlantic issue
“Let’s return to that hillside where Clayton exited his Mercedes.
In the gray light, he climbs the pasture. Halfway up the slope,
three horses are standing: sculpturally still, casually composed
in a perfect triptych of horsitude.”
— James Parker in The Atlantic , Nov. 2017 issue
Logos-related material
Comments Off on Triptychs
Sunday, January 2, 2011
Horseness
"Art has to reveal to us ideas, formless spiritual essences."
— A character clearly talking nonsense, from the National Library section of James Joyce's Ulysses
"Unsheathe your dagger definitions. Horseness is the whatness of allhorse."
— A thought of Stephen Dedalus in the same Ulysses section
For a representation of horseness related to Singer's dagger definitions in Saturday evening's post, see Generating the Octad Generator and Art Wars: Geometry as Conceptual Art.
More seriously, Joyce's "horseness" is related to the problem of universals. For an illuminating approach to universals from a psychological point of view, see James Hillman's Re-Visioning Psychology (Harper Collins, 1977). (See particularly pages 154-157.)
Comments Off on Horseness
Saturday, January 1, 2011
For a Dead Philosopher —
Dagger Definitions
Part I —
Part II —
Update of Jan. 2, 2011 —
Singer goes on to say that "A finite projective plane, PG (2, pn ), defined in this way is Pascalian and Desarguesian ; it exists for every prime p and positive integer n , and there is only one such PG (2, pn ) for a given p and n …."
His definitions therefore deliberately exclude non -Desarguesian finite projective planes, which were known to exist at the time he wrote.
Comments Off on For a Dead Philosopher —