See the above science adviser in this journal —
Monday, February 7, 2022
Science News
Comments Off on Science News
Tuesday, March 23, 2021
The Fano Hallows
From a Log24 post of Friday, February 26, 2021 —
( Not to be confused with The Tin Man’s Hat. ) |
This image may be regarded as memorializing a photographer
who died at 80 on Feb. 26 and who
“captured Warhol’s self-designed mythology in the making”
— Alex Vadukul in The New York Times today
Comments Off on The Fano Hallows
Sunday, March 21, 2021
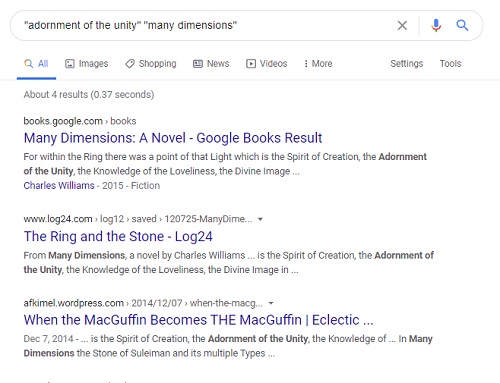
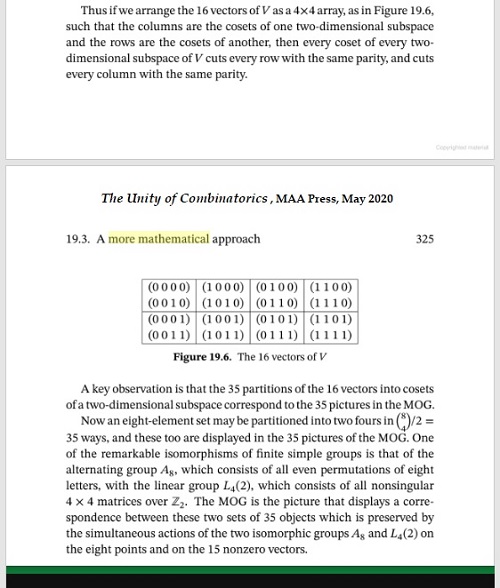
Mathematics and Narrative: The Unity
“To conquer, three boxes* have to synchronize and join together into the Unity.”
―Wonder Woman in Zack Snyder’s Justice League
See also The Unity of Combinatorics and The Miracle Octad Generator.
* Cf. Aitchison’s Octads —
Comments Off on Mathematics and Narrative: The Unity
Friday, February 26, 2021
Non-Chaos Non-Magic
For fans of “WandaVision” —
“1978 was perhaps the seminal year in the origin of chaos magic. . . .”
— Wikipedia article on Chaos Magic
Non-Chaos Non-Magic from Halloween 1978 —
Related material —
A doctoral student of a different Peter Cameron —
( Not to be confused with The Tin Man’s Hat. )
Comments Off on Non-Chaos Non-Magic
Thursday, May 28, 2020
Unity Game
“Old men ought to be explorers.” — T. S. Eliot
“Everybody’s lost but me!” — Young Indiana Jones, quoted
in a book review (“Knox Peden on Martin Hägglund”) in
Sydney Review of Books on May 26 . . .
” Here I am reminded of the words of
the young Indiana Jones alone in the desert,
decades before the Last Crusade:
‘Everybody’s lost but me.’ “
Related remarks — Now You See It, Now You Don’t.
Comments Off on Unity Game
Finite Geometry at GitHub
My website on finite geometry is now available
on GitHub at http://m759.github.io/ . The part
of greatest interest to coders is also at
https://repl.it/@m759/View-4x4x4#index.html .
Comments Off on Finite Geometry at GitHub
Wednesday, May 20, 2020
Thursday, May 7, 2020
Now You See It, Now You Don’t
“Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to explain
how art fits into our subject and what we mean by beauty.”
— Sir Michael Atiyah, quoted here on April 4, 2016
Illustrations, from the American Mathematical Society Spring
2020 book sale, of a book scheduled to be published May 28.
Comments Off on Now You See It, Now You Don’t
Kant as Diamond Cutter
“He wished Kant were alive. Kant would have appreciated it.
That master diamond cutter.”
— Robert M. Pirsig, Zen and the Art of Motorcycle Maintenance , Part III.
Kant’s “category theory” —
| “In the Transcendental Analytic, Kant deduces the table of twelve categories, or pure concepts of the understanding….
The categories must be ‘schematized’ because their non-empirical origin in pure understanding prevents their having the sort of sensible content that would connect them immediately to the objects of experience; transcendental schemata are mediating representations that are meant to establish the connection between pure concepts and appearances in a rule-governed way. Mathematical concepts are discussed in this context since they are unique in being pure but also sensible concepts: they are pure because they are strictly a priori in origin, and yet they are sensible since they are constructed in concreto . ” — Shabel, Lisa, “Kant’s Philosophy of Mathematics”, The Stanford Encyclopedia of Philosophy (Spring 2016 Edition), Edward N. Zalta (ed.), URL = <https://plato.stanford.edu/archives/spr2016/entries/kant-mathematics/>. |
See also The Diamond Theorem and Octad.us.
Comments Off on Kant as Diamond Cutter
Wednesday, May 6, 2020
“The Ship of Theseus”…
is a philosophical conundrum discussed this morning in the weblog of
David Justice.
A related statement of this “problem of identity,” from posts
in this weblog tagged “For Banff 2009” yesterday afternoon—
Remarks related to the ship of Theseus —
Comments Off on “The Ship of Theseus”…
Sunday, April 26, 2020
The Triangle of Art
This post was suggested by yesterday morning's link to The Fano Hallows.
"Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to explain
how art fits into our subject and what we mean by beauty."
— Sir Michael Atiyah, quoted here on April 4, 2016
A symbol related to The Fano Hallows —
Comments Off on The Triangle of Art
Thursday, April 26, 2018
Wednesday, April 25, 2018
Tuesday, December 27, 2011
Getting with the Program
Stanley Fish in The New York Times yesterday evening—
From the MLA program Fish discussed—
Above: An MLA session, “Defining Form,” led
by Colleen Rosenfeld of Pomona College
An example from Pomona College in 1968—
The same underlying geometries (i.e., “form”) may be modeled with
a square figure and a cubical figure rather than with the triangular
figures of 1968 shown above.
See Finite Geometry of the Square and Cube.
Those who prefer a literary approach to form may enjoy the recent post As Is.
(For some context, see Game of Shadows.)
Comments Off on Getting with the Program