Log24

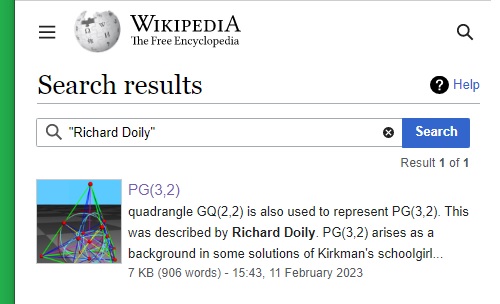
See also Doily in this  journal.

Stanley E. Payne and J. A. Thas in 1983* (previous post) —

“… a 4×4 grid together with
the affine lines on it is AG(2,4).”

Payne and Thas of course use their own definition
of affine lines on a grid.

Actually, a 4×4 grid together with the affine lines on it
is, viewed in a different way, not AG(2,4) but rather AG(4,2).

For AG(4,2) in the proper context, see
Affine Groups on Small Binary Spaces and
The Galois Tesseract.

* And 26 years later,  in 2009.

Wikipedia on what has been called “the doily” —

“The smallest non-trivial generalized quadrangle
is GQ(2,2), whose representation* has been dubbed
‘the doily’ by Stan Payne in 1973.”

A later publication relates the doily to grids.

From Finite Generalized Quadrangles , by Stanley E. Payne
and J. A. Thas, December 1983, at researchgate.net, pp. 81-82—

“Then the lines … define a 3×3 grid G  (i.e. a grid
consisting of 9 points and 6 lines).”
. . . .
“So we have shown that the grid G  can completed [sic ]
in a unique way to a grid with 8 lines and 16 points.”
. . . .
“A 4×4 grid defines a linear subspace
of  the 2−(64,4,1) design, i.e. a 4×4 grid
together with the affine lines on it is AG(2,4).”

A more graphic approach from this journal —

Click the image for further details.

* This wording implies that GQ(2,2) has a unique
visual representation. It does not. See inscape .