The Principle of Sufficient Reason
by George David Birkhoff
from "Three Public Lectures on Scientific Subjects,"
delivered at the Rice Institute, March 6, 7, and 8, 1940
EXCERPT 1—
My primary purpose will be to show how a properly formulated
Principle of Sufficient Reason plays a fundamental
role in scientific thought and, furthermore, is to be regarded
as of the greatest suggestiveness from the philosophic point
of view.2
In the preceding lecture I pointed out that three branches
of philosophy, namely Logic, Aesthetics, and Ethics, fall
more and more under the sway of mathematical methods.
Today I would make a similar claim that the other great
branch of philosophy, Metaphysics, in so far as it possesses
a substantial core, is likely to undergo a similar fate. My
basis for this claim will be that metaphysical reasoning always
relies on the Principle of Sufficient Reason, and that
the true meaning of this Principle is to be found in the
“Theory of Ambiguity” and in the associated mathematical
“Theory of Groups.”
If I were a Leibnizian mystic, believing in his “preestablished
harmony,” and the “best possible world” so
satirized by Voltaire in “Candide,” I would say that the
metaphysical importance of the Principle of Sufficient Reason
and the cognate Theory of Groups arises from the fact that
God thinks multi-dimensionally3 whereas men can only
think in linear syllogistic series, and the Theory of Groups is
2 As far as I am aware, only Scholastic Philosophy has fully recognized and ex-
ploited this principle as one of basic importance for philosophic thought
3 That is, uses multi-dimensional symbols beyond our grasp.
______________________________________________________________________
the appropriate instrument of thought to remedy our deficiency
in this respect.
The founder of the Theory of Groups was the mathematician
Evariste Galois. At the end of a long letter written in
1832 on the eve of a fatal duel, to his friend Auguste
Chevalier, the youthful Galois said in summarizing his
mathematical work,4 “You know, my dear Auguste, that
these subjects are not the only ones which I have explored.
My chief meditations for a considerable time have been
directed towards the application to transcendental Analysis
of the theory of ambiguity. . . . But I have not the time, and
my ideas are not yet well developed in this field, which is
immense.” This passage shows how in Galois’s mind the
Theory of Groups and the Theory of Ambiguity were
interrelated.5
Unfortunately later students of the Theory of Groups
have all too frequently forgotten that, philosophically
speaking, the subject remains neither more nor less than the
Theory of Ambiguity. In the limits of this lecture it is only
possible to elucidate by an elementary example the idea of a
group and of the associated ambiguity.
Consider a uniform square tile which is placed over a
marked equal square on a table. Evidently it is then impossible
to determine without further inspection which one
of four positions the tile occupies. In fact, if we designate
its vertices in order by A, B, C, D, and mark the corresponding
positions on the table, the four possibilities are for the
corners A, B, C, D of the tile to appear respectively in the
positions A, B, C, D; B, C, D, A; C, D, A, B; and D, A, B, C.
These are obtained respectively from the first position by a
4 My translation.
5 It is of interest to recall that Leibniz was interested in ambiguity to the extent
of using a special notation v (Latin, vel ) for “or.” Thus the ambiguously defined
roots 1, 5 of x2-6x+5=0 would be written x = l v 5 by him.
______________________________________________________________________
null rotation ( I ), by a rotation through 90° (R), by a rotation
through 180° (S), and by a rotation through 270° (T).
Furthermore the combination of any two of these rotations
in succession gives another such rotation. Thus a rotation R
through 90° followed by a rotation S through 180° is equivalent
to a single rotation T through 270°, Le., RS = T. Consequently,
the "group" of four operations I, R, S, T has
the "multiplication table" shown here:

This table fully characterizes the group, and shows the exact
nature of the underlying ambiguity of position.
More generally, any collection of operations such that
the resultant of any two performed in succession is one of
them, while there is always some operation which undoes
what any operation does, forms a "group."
__________________________________________________
EXCERPT 2—
Up to the present point my aim has been to consider a
variety of applications of the Principle of Sufficient Reason,
without attempting any precise formulation of the Principle
itself. With these applications in mind I will venture to
formulate the Principle and a related Heuristic Conjecture
in quasi-mathematical form as follows:
PRINCIPLE OF SUFFICIENT REASON. If there appears
in any theory T a set of ambiguously determined ( i e .
symmetrically entering) variables, then these variables can themselves
be determined only to the extent allowed by the corresponding
group G. Consequently any problem concerning these variables
which has a uniquely determined solution, must itself be
formulated so as to be unchanged by the operations of the group
G ( i e . must involve the variables symmetrically).
HEURISTIC CONJECTURE. The final form of any
scientific theory T is: (1) based on a few simple postulates; and
(2) contains an extensive ambiguity, associated symmetry, and
underlying group G, in such wise that, if the language and laws
of the theory of groups be taken for granted, the whole theory T
appears as nearly self-evident in virtue of the above Principle.
The Principle of Sufficient Reason and the Heuristic Conjecture,
as just formulated, have the advantage of not involving
excessively subjective ideas, while at the same time
retaining the essential kernel of the matter.
In my opinion it is essentially this principle and this
conjecture which are destined always to operate as the basic
criteria for the scientist in extending our knowledge and
understanding of the world.
It is also my belief that, in so far as there is anything
definite in the realm of Metaphysics, it will consist in further
applications of the same general type. This general conclu-
sion may be given the following suggestive symbolic form:

While the skillful metaphysical use of the Principle must
always be regarded as of dubious logical status, nevertheless
I believe it will remain the most important weapon of the
philosopher.
___________________________________________________________________________
A more recent lecture on the same subject —
"From Leibniz to Quantum World:
Symmetries, Principle of Sufficient Reason
and Ambiguity in the Sense of Galois"
by Jean-Pierre Ramis (Johann Bernoulli Lecture at U. of Groningen, March 2005)


.gif)

























































 .
.














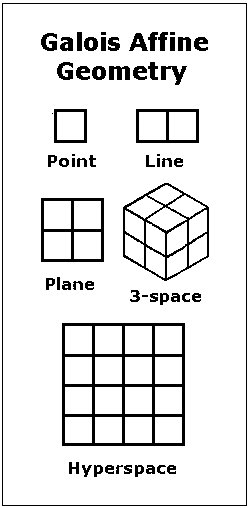



































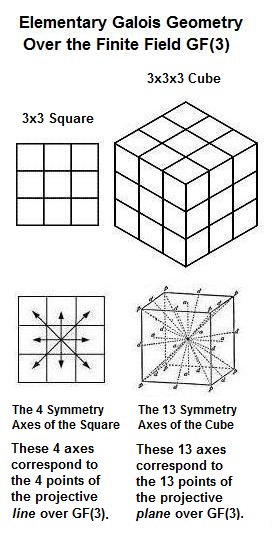


































 pure as one of Bach's inverted canons or Euler's formula for polyhedra."
pure as one of Bach's inverted canons or Euler's formula for polyhedra."


















 The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the ‘logical square’ of scholastic philosophy) as a means of analysing paired concepts more fully….”
The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the ‘logical square’ of scholastic philosophy) as a means of analysing paired concepts more fully….”





