Log24

Friday, March 2, 2012

Douat Facsimile

Filed under: General,Geometry — Tags: , — m759 @ 5:14 pm

Title of a treatise by Dominique Douat—

"Méthode pour faire une infinité de desseins différens avec des carreaux mi-partis de deux couleurs par une ligne diagonale : ou observations du Père Dominique Doüat Religieux Carmes de la Province de Toulouse sur un mémoire inséré dans l'Histoire de l'Académie Royale des Sciences de Paris l'année 1704, présenté par le Révérend Père Sébastien Truchet religieux du même ordre, Académicien honoraire  " (Paris, 1722)

"The earliest (and perhaps the rarest) treatise on the theory of design"

— E. H. Gombrich, 1979, in The Sense of Order

A facsimile version (excerpts, 108 pp., Feb. 5, 2010) of this treatise is available from

http://jacques-andre.fr/ed/ in a 23.1 MB pdf.

Sample page—

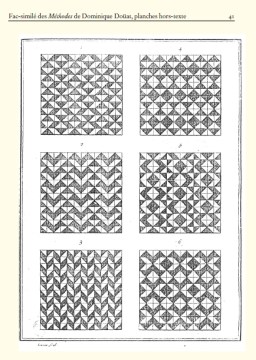
For a treatise on the finite geometry underlying such designs (based on a monograph I wrote in 1976, before I had heard of Douat or his predecessor Truchet), see Diamond Theory.

Thursday, January 29, 2004

Thursday January 29, 2004

Filed under: General,Geometry — Tags: , , — m759 @ 2:30 pm

Misunderstanding
in the Theory of Design

"Whether or not we can follow the theorist in his demonstrations, there is one misunderstanding we must avoid at all cost.  We must not confuse the analyses of geometrical symmetries with the mathematics of combination and permutation….

The earliest (and perhaps the rarest) treatise on the theory of design drives home this insight with marvellous precision."

— E. H. Gombrich, 1979, in
   The Sense of Order

This is perhaps the stupidest remark I have ever read.  The "treatise on the theory of design" that Gombrich refers to is

  • Dominique Douat, Méthode Pour Faire une Infinité de Desseins Differents…. Paris, 1722.

For some background, see

Truchet Tiling,  

Truchet & Types:
Tiling Systems and Ornaments, and

Douat Designs. 

Certain of the Truchet/Douat patterns have rather intriguing mathematical properties, sketched in my website Diamond Theory.  These properties become clear if and only if we we do what Gombrich declares that we must not do:  "confuse the analyses of geometrical symmetries with the mathematics of combination and permutation."  (The verb "confuse" should, of course, be replaced by the verb "combine.")
 

Powered by WordPress