(A review)
Friday, August 14, 2015
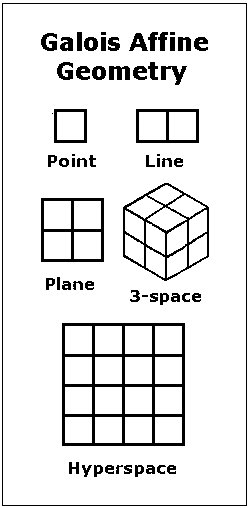
Discrete Space
Galois space:
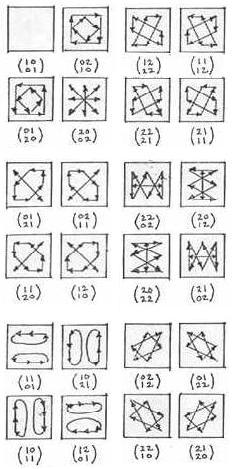
Counting symmetries of Galois space:
The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
Monday, April 10, 2023
Space
(Perspective Not as Symbolic Form)
From a post of June 8, 2014 —

See August 6, 2013 — Desargues via Galois.
Monday, September 13, 2021
Sunday, April 16, 2017
Art Space Paradigm Shift

This post’s title is from the tags of the previous post —
The title’s “shift” is in the combined concepts of …
Space and Number
From Finite Jest (May 27, 2012):
The books pictured above are From Discrete to Continuous ,
by Katherine Neal, and Geometrical Landscapes , by Amir Alexander.
For some details of the shift, see a Log24 search for Boole vs. Galois.
From a post found in that search —
“Benedict Cumberbatch Says
a Journey From Fact to Faith
Is at the Heart of Doctor Strange“
— io9 , July 29, 2016
” ‘This man comes from a binary universe
where it’s all about logic,’ the actor told us
at San Diego Comic-Con . . . .
‘And there’s a lot of humor in the collision
between Easter [ sic ] mysticism and
Western scientific, sort of logical binary.’ “
[Typo now corrected, except in a comment.]
Friday, August 14, 2015
Space Station 2015
(A sequel to Space Station 1976)
For Kathleen Gibbons* —
* Note Gibbons's work on "Discrete phase space based on finite fields."
Saturday, February 18, 2012
Symmetry
From the current Wikipedia article "Symmetry (physics)"—
"In physics, symmetry includes all features of a physical system that exhibit the property of symmetry—that is, under certain transformations, aspects of these systems are 'unchanged', according to a particular observation. A symmetry of a physical system is a physical or mathematical feature of the system (observed or intrinsic) that is 'preserved' under some change.
A family of particular transformations may be continuous (such as rotation of a circle) or discrete (e.g., reflection of a bilaterally symmetric figure, or rotation of a regular polygon). Continuous and discrete transformations give rise to corresponding types of symmetries. Continuous symmetries can be described by Lie groups while discrete symmetries are described by finite groups (see Symmetry group)."….
"A discrete symmetry is a symmetry that describes non-continuous changes in a system. For example, a square possesses discrete rotational symmetry, as only rotations by multiples of right angles will preserve the square's original appearance."
Note the confusion here between continuous (or discontinuous) transformations and "continuous" (or "discontinuous," i.e. "discrete") groups .
This confusion may impede efforts to think clearly about some pure mathematics related to current physics— in particular, about the geometry of spaces made up of individual units ("points") that are not joined together in a continuous manifold.
For an attempt to forestall such confusion, see Noncontinuous Groups.
For related material, see Erlanger and Galois as well as the opening paragraphs of Diamond Theory—
Symmetry is often described as invariance under a group of transformations. An unspoken assumption about symmetry in Euclidean 3-space is that the transformations involved are continuous.
Diamond theory rejects this assumption, and in so doing reveals that Euclidean symmetry may itself be invariant under rather interesting groups of non-continuous (and a-symmetric) transformations. (These might be called noncontinuous groups, as opposed to so-called discontinuous (or discrete ) symmetry groups. See Weyl's Symmetry .)
For example, the affine group A on the 4-space over the 2-element field has a natural noncontinuous and asymmetric but symmetry-preserving action on the elements of a 4×4 array. (Details)
(Version first archived on March 27, 2002)
Update of Sunday, February 19, 2012—
The abuse of language by the anonymous authors
of the above Wikipedia article occurs also in more
reputable sources. For instance—

Some transformations referred to by Brading and Castellani
and their editees as "discrete symmetries" are, in fact, as
linear transformations of continuous spaces, themselves
continuous transformations.
This unfortunate abuse of language is at least made explicit
in a 2003 text, Mathematical Perspectives on Theoretical
Physics (Nirmala Prakash, Imperial College Press)—
"… associated[*] with any given symmetry there always exists
a continuous or a discrete group of transformations….
A symmetry whose associated group is continuous (discrete)
is called a continuous (discrete ) symmetry ." — Pp. 235, 236
[* Associated how?]
Monday, August 29, 2005
Monday August 29, 2005
| Date: Sun, 28 Aug 2005 12:30:40 -0400 From: Alf van der Poorten AM Subject: Vale George Szekeres and Esther Klein Szekeres Members of the Number Theory List will be sad to learn that George and Esther Szekeres both died this morning. George, 94, had been quite ill for the last 2-3 days, barely conscious, and died first at 06:30. Esther, 95, died a half hour later. Both George Szekeres and Esther Klein will be recalled by number theorists as members of the group of young Hungarian mathematicians of the 1930s including Turan and Erdos. George and Esther's coming to Australia in the late 40s played an important role in the invigoration of Australian Mathematics. George was also an expert in group theory and relativity; he was my PhD supervisor. Emeritus Professor |
AVE
| "Hello! Kinch here. Put me on to Edenville. Aleph, alpha: nought, nought, one."
"A very short space of time through very short times of space…. — James Joyce, Ulysses, Proteus chapter A very short space of time through very short times of space…. "It is demonstrated that space-time should possess a discrete structure on Planck scales." — Peter Szekeres, abstract of Discrete Space-Time |
Peter Szekeres is the son of George and Esther Szekeres.
"At present, such relationships can at best be heuristically described in terms that invoke some notion of an 'intelligent user standing outside the system.'"
— Gian-Carlo Rota in Indiscrete Thoughts, p. 152
Thursday, August 25, 2005
Thursday August 25, 2005
Train of Thought
Part I: The 24-Cell
From S. H. Cullinane,
Visualizing GL(2,p),
March 26, 1985–
| From John Baez, “This Week’s Finds in Mathematical Physics (Week 198),” September 6, 2003: Noam Elkies writes to John Baez:
The enrapturing discoveries of our field systematically conceal, like footprints erased in the sand, the analogical train of thought that is the authentic life of mathematics – Gian-Carlo Rota |
Like footprints erased in the sand….
“Hello! Kinch here. Put me on to Edenville. Aleph, alpha: nought, nought, one.”
“A very short space of time through very short times of space….
Am I walking into eternity along Sandymount strand?”
— James Joyce, Ulysses, Proteus chapter
A very short space of time through very short times of space….
“It is demonstrated that space-time should possess a discrete structure on Planck scales.”
— Peter Szekeres, abstract of Discrete Space-Time
“A theory…. predicts that space and time are indeed made of discrete pieces.”
— Lee Smolin in Atoms of Space and Time (pdf), Scientific American, Jan. 2004
“… a fundamental discreteness of spacetime seems to be a prediction of the theory….”
— Thomas Thiemann, abstract of Introduction to Modern Canonical Quantum General Relativity
“Theories of discrete space-time structure are being studied from a variety of perspectives.”
— Quantum Gravity and the Foundations of Quantum Mechanics at Imperial College, London
The above speculations by physicists
are offered as curiosities.
I have no idea whether
any of them are correct.
Related material:
Stephen Wolfram offers a brief
History of Discrete Space.
For a discussion of space as discrete
by a non-physicist, see John Bigelow‘s
Space and Timaeus.
in a Discrete Space
physics, there are of course many
purely mathematical discrete spaces.
See Visible Mathematics, continued
(Aug. 4, 2005):

Sunday, January 22, 2023
The Stillwell Dichotomies
| Number | Space |
| Arithmetic | Geometry |
| Discrete | Continuous |
Related literature —

From a "Finite Fields in 1956" post —

The Nutshell:

Related Narrative:
Thursday, January 19, 2023
Two Approaches to Local-Global Symmetry
Last revised: January 20, 2023 @ 11:39:05
The First Approach — Via Substructure Isomorphisms —
From "Symmetry in Mathematics and Mathematics of Symmetry"
by Peter J. Cameron, a Jan. 16, 2007, talk at the International
Symmetry Conference, Edinburgh, Jan. 14-17, 2007 —
|
Local or global? "Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous * if every isomorphism between finite substructures of M can be extended to an automorphism of M ; in other words, 'any local symmetry is global.' " |
A related discussion of the same approach —
|
"The aim of this thesis is to classify certain structures
— Alice Devillers, |
The Wikipedia article Homogeneous graph discusses the local-global approach
used by Cameron and by Devillers.
For some historical background on this approach
via substructure isomorphisms, see a former student of Cameron:
Dugald Macpherson, "A survey of homogeneous structures,"
Discrete Mathematics , Volume 311, Issue 15, 2011,
Pages 1599-1634.
Related material:
Cherlin, G. (2000). "Sporadic Homogeneous Structures."
In: Gelfand, I.M., Retakh, V.S. (eds)
The Gelfand Mathematical Seminars, 1996–1999.
Gelfand Mathematical Seminars. Birkhäuser, Boston, MA.
https://doi.org/10.1007/978-1-4612-1340-6_2
and, more recently,
Gill et al., "Cherlin's conjecture on finite primitive binary
permutation groups," https://arxiv.org/abs/2106.05154v2
(Submitted on 9 Jun 2021, last revised 9 Jul 2021)
This approach seems to be a rather deep rabbit hole.
The Second Approach — Via Induced Group Actions —
My own interest in local-global symmetry is of a quite different sort.
See properties of the two patterns illustrated in a note of 24 December 1981 —
Pattern A above actually has as few symmetries as possible
(under the actions described in the diamond theorem ), but it
does enjoy, as does patttern B, the local-global property that
a group acting in the same way locally on each part induces
a global group action on the whole .
* For some historical background on the term "homogeneous,"
see the Wikipedia article Homogeneous space.
Monday, October 17, 2022
Thursday, February 28, 2019
Fooling

The two books pictured above are From Discrete to Continuous ,
by Katherine Neal, and Geometrical Landscapes , by Amir Alexander.
Note: There is no Galois (i.e., finite) field with six elements, but
the theory of finite fields underlies applications of six-set geometry.
Friday, November 27, 2015
Einstein and Geometry
(A Prequel to Dirac and Geometry)
"So Einstein went back to the blackboard.
And on Nov. 25, 1915, he set down
the equation that rules the universe.
As compact and mysterious as a Viking rune,
it describes space-time as a kind of sagging mattress…."
— Dennis Overbye in The New York Times online,
November 24, 2015
Some pure mathematics I prefer to the sagging Viking mattress —
Readings closely related to the above passage —
Thomas Hawkins, "From General Relativity to Group Representations:
the Background to Weyl's Papers of 1925-26," in Matériaux pour
l'histoire des mathématiques au XXe siècle: Actes du colloque
à la mémoire de Jean Dieudonné, Nice, 1996 (Soc. Math.
de France, Paris, 1998), pp. 69-100.
The 19th-century algebraic theory of invariants is discussed
as what Weitzenböck called a guide "through the thicket
of formulas of general relativity."
Wallace Givens, "Tensor Coordinates of Linear Spaces," in
Annals of Mathematics Second Series, Vol. 38, No. 2, April 1937,
pp. 355-385.
Tensors (also used by Einstein in 1915) are related to
the theory of line complexes in three-dimensional
projective space and to the matrices used by Dirac
in his 1928 work on quantum mechanics.
For those who prefer metaphors to mathematics —
Rota fails to cite the source of his metaphor.
|
Sunday, July 20, 2014
Sunday School
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Thursday, July 17, 2014
Paradigm Shift:
Continuous Euclidean space to discrete Galois space*
Euclidean space:
Counting symmetries in Euclidean space:
Galois space:
Counting symmetries of Galois space:

The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
* For related remarks, see posts of May 26-28, 2012.
Sunday, June 8, 2014
Vide

"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Tuesday, July 16, 2013
Child Buyers
The title refers to a classic 1960 novel by John Hersey.
“How do you get young people excited about space?”
— Megan Garber in The Atlantic , Aug. 16, 2012
(Italics added.) (See previous four posts.)
Allyn Jackson on “Simplicity, in Mathematics and in Art,”
in the new August 2013 issue of Notices of the American
Mathematical Society—
“As conventions evolve, so do notions of simplicity.
Franks mentioned Gauss’s 1831 paper that
established the respectability of complex numbers.”
This suggests a related image by Gauss, with a
remark on simplicity—
Here Gauss’s diagram is not, as may appear at first glance,
a 3×3 array of squares, but is rather a 4×4 array of discrete
points (part of an infinite plane array).
Related material that does feature the somewhat simpler 3×3 array
of squares, not seen as part of an infinite array—
Marketing the Holy Field
Click image for the original post.
For a purely mathematical view of the holy field, see Visualizing GL(2,p).
Monday, April 1, 2013
Desargues via Rosenhain
Background: Rosenhain and Göpel Tetrads in PG(3,2)
|
Introduction: The Large Desargues Configuration Added by Steven H. Cullinane on Friday, April 19, 2013 Desargues' theorem according to a standard textbook:
"If two triangles are perspective from a point The converse, from the same book:
"If two triangles are perspective from a line
Desargues' theorem according to Wikipedia
"Two triangles are in perspective axially [i.e., from a line]
A figure often used to illustrate the theorem,
A discussion of the "if and only if" version of the theorem
This large Desargues configuration involves a third triangle,
Point-line incidence in this larger configuration is,
The third triangle, within the larger configuration,
|

A connection discovered today (April 1, 2013)—
(Click to enlarge the image below.)
Update of April 18, 2013
Note that Baker's Desargues-theorem figure has three triangles,
ABC, A'B'C', A"B"C", instead of the two triangles that occur in
the statement of the theorem. The third triangle appears in the
course of proving, not just stating, the theorem (or, more precisely,
its converse). See, for instance, a note on a standard textbook for
further details.
(End of April 18, 2013 update.)
Update of April 14, 2013
See Baker's Proof (Edited for the Web) for a detailed explanation
of the above picture of Baker's Desargues-theorem frontispiece.
(End of April 14, 2013 update.)
Update of April 12, 2013
A different figure, from a site at National Tsing Hua University,
shows the three triangles of Baker's figure more clearly:
(End of update of April 12, 2013)
Update of April 13, 2013
Another in a series of figures illustrating
Desargues's theorem in light of Galois geometry:

See also the original Veblen-Young figure in context.
(End of update of April 13, 2013)

Rota's remarks, while perhaps not completely accurate, provide some context
for the above Desargues-Rosenhain connection. For some other context,
see the interplay in this journal between classical and finite geometry, i.e.
between Euclid and Galois.
For the recent context of the above finite-geometry version of Baker's Vol. I
frontispiece, see Sunday evening's finite-geometry version of Baker's Vol. IV
frontispiece, featuring the Göpel, rather than the Rosenhain, tetrads.
For a 1986 illustration of Göpel and Rosenhain tetrads (though not under
those names), see Picturing the Smallest Projective 3-Space.
In summary… the following classical-geometry figures
are closely related to the Galois geometry PG(3,2):
|
Volume I of Baker's Principles has a cover closely related to the Rosenhain tetrads in PG(3,2) |
Volume IV of Baker's Principles has a cover closely related to the Göpel tetrads in PG(3,2) |
|
Foundations (click to enlarge)
|
Higher Geometry (click to enlarge)
|
Saturday, May 26, 2012
Harriot’s Cubes

See also Finite Geometry and Physical Space.
Related material from MacTutor—
The paper by J. W. Shirley, Binary numeration before Leibniz, Amer. J. Physics 19 (8) (1951), 452-454, contains an interesting look at some mathematics which appears in the hand written papers of Thomas Harriot [1560-1621]. Using the photographs of the two original Harriot manuscript pages reproduced in Shirley’s paper, we explain how Harriot was doing arithmetic with binary numbers. Leibniz [1646-1716] is credited with the invention [1679-1703] of binary arithmetic, that is arithmetic using base 2. Laplace wrote:-
However, Leibniz was certainly not the first person to think of doing arithmetic using numbers to base 2. Many years earlier Harriot had experimented with the idea of different number bases…. |
For a discussion of Harriot on the discrete-vs.-continuous question,
see Katherine Neal, From Discrete to Continuous: The Broadening
of Number Concepts in Early Modern England (Springer, 2002),
pages 69-71.
Thursday, May 3, 2012
Everybody Comes to Rick’s

“The key is the cocktail that begins the proceedings.”
– Brian Harley, Mate in Two Moves
See also yesterday's Endgame , as well as Play and Interplay
from April 28… and, as a key, the following passage from
an earlier April 28 post—
| Euclidean geometry has long been applied to physics; Galois geometry has not. The cited webpage describes the interplay of both sorts of geometry— Euclidean and Galois, continuous and discrete— within physical space— if not within the space of physics . |
Saturday, April 28, 2012
Sprechen Sie Deutsch?
A Log24 post, "Bridal Birthday," one year ago today linked to
"The Discrete and the Continuous," a brief essay by David Deutsch.
From that essay—
"The idea of quantization—
the discreteness of physical quantities—
turned out to be immensely fruitful."
Deutsch's "idea of quantization" also appears in
the April 12 Log24 post Mythopoetic—
|
"Is Space Digital?" — Cover story, Scientific American "The idea that space may be digital — Physicist Sabine Hossenfelder "A quantization of space/time — Peter Woit in a comment |
It seems some clarification is in order.
Hossenfelder's "The idea that space may be digital"
and Woit's "a quantization of space/time" may not
refer to the same thing.
Scientific American on the concept of digital space—
"Space may not be smooth and continuous.
Instead it may be digital, composed of tiny bits."
Wikipedia on the concept of quantization—
Causal sets, loop quantum gravity, string theory,
and black hole thermodynamics all predict
a quantized spacetime….
For a purely mathematical approach to the
continuous-vs.-discrete issue, see
Finite Geometry and Physical Space.
The physics there is somewhat tongue-in-cheek,
but the geometry is serious.The issue there is not
continuous-vs.-discrete physics , but rather
Euclidean-vs.-Galois geometry .
Both sorts of geometry are of course valid.
Euclidean geometry has long been applied to
physics; Galois geometry has not. The cited
webpage describes the interplay of both sorts
of geometry— Euclidean and Galois, continuous
and discrete— within physical space— if not
within the space of physics.
Wednesday, March 21, 2012
Digital Theology
See also remarks on Digital Space and Digital Time in this journal.
Such remarks can, of course, easily verge on crackpot territory.
For some related pure mathematics, see Symmetry of Walsh Functions.
Wednesday, October 26, 2011
Erlanger and Galois
Peter J. Cameron yesterday on Galois—
"He was killed in a duel at the age of 20…. His work languished for another 14 years until Liouville published it in his Journal; soon it was recognised as the foundation stone of modern algebra, a position it has never lost."
Here Cameron is discussing Galois theory, a part of algebra. Galois is known also as the founder* of group theory, a more general subject.
Group theory is an essential part of modern geometry as well as of modern algebra—
"In der Galois'schen Theorie, wie hier, concentrirt sich das Interesse auf Gruppen von Änderungen. Die Objecte, auf welche sich die Änderungen beziehen, sind allerdings verschieden; man hat es dort mit einer endlichen Zahl discreter Elemente, hier mit der unendlichen Zahl von Elementen einer stetigen Mannigfaltigkeit zu thun."
— Felix Christian Klein, Erlanger Programm , 1872
("In the Galois theory, as in ours, the interest centres on groups of transformations. The objects to which the transformations are applied are indeed different; there we have to do with a finite number of discrete elements, here with the infinite number of elements in a continuous manifoldness." (Translated by M.W. Haskell, published in Bull. New York Math. Soc. 2, (1892-1893), 215-249))
Related material from Hermann Weyl, Symmetry , Princeton University Press, 1952 (paperback reprint of 1982, pp. 143-144)—
"A field is perhaps the simplest algebraic structure we can invent. Its elements are numbers…. Space is another example of an entity endowed with a structure. Here the elements are points…. What we learn from our whole discussion and what has indeed become a guiding principle in modern mathematics is this lesson: Whenever you have to do with a structure-endowed entity Σ try to determine is group of automorphisms , the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of Σ in this way."
For a simple example of a group acting on a field (of 8 elements) that is also a space (of 8 points), see Generating the Octad Generator and Knight Moves.
* Joseph J. Rotman, An Introduction to the Theory of Groups , 4th ed., Springer, 1994, page 2
Sunday, September 18, 2011
Anatomy of a Cube
R.D. Carmichael's seminal 1931 paper on tactical configurations suggests
a search for later material relating such configurations to block designs.
Such a search yields the following—
"… it seems that the relationship between
BIB [balanced incomplete block ] designs
and tactical configurations, and in particular,
the Steiner system, has been overlooked."
— D. A. Sprott, U. of Toronto, 1955
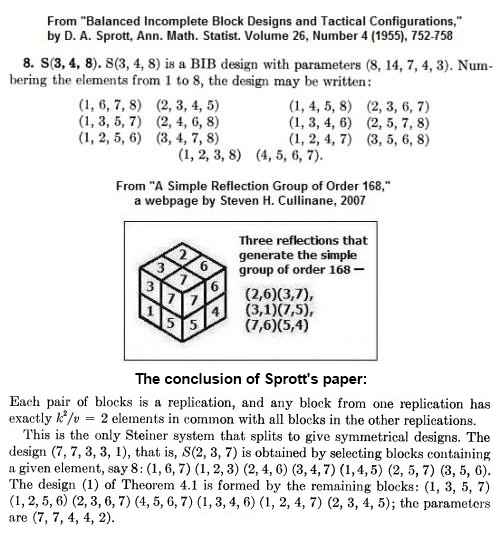
The figure by Cullinane included above shows a way to visualize Sprott's remarks.
For the group actions described by Cullinane, see "The Eightfold Cube" and
"A Simple Reflection Group of Order 168."
Update of 7:42 PM Sept. 18, 2011—
From a Summer 2011 course on discrete structures at a Berlin website—
A different illustration of the eightfold cube as the Steiner system S(3, 4, 8)—

Note that only the static structure is described by Felsner, not the
168 group actions discussed (as above) by Cullinane. For remarks on
such group actions in the literature, see "Cube Space, 1984-2003."
Tuesday, October 19, 2010
Savage Logic continued…
CHAPTER V
"This is an account of the discrete groups generated by reflections…."
— Regular Polytopes , by H.S.M. Coxeter (unabridged and corrected 1973 Dover reprint of the 1963 Macmillan second edition)
"In this article, we begin a theory linking hyperplane arrangements and invariant forms for reflection groups over arbitrary fields…. Let V be an n-dimensional vector space over a field F, and let G ≤ Gln (F) be a finite group…. An element of finite order in Gl(V ) is a reflection if its fixed point space in V is a hyperplane, called the reflecting hyperplane. There are two types of reflections: the diagonalizable reflections in Gl(V ) have a single nonidentity eigenvalue which is a root of unity; the nondiagonalizable reflections in Gl(V ) are called transvections and have determinant 1 (note that they can only occur if the characteristic of F is positive)…. A reflection group is a finite group G generated by reflections."
— Julia Hartmann and Anne V. Shepler, "Reflection Groups and Differential Forms," Mathematical Research Letters , Vol. 14, No. 6 (Nov. 2007), pp. 955-971
"… the class of reflections is larger in some sense over an arbitrary field than over a characteristic zero field. The reflections in Gl(V ) not only include diagonalizable reflections (with a single nonidentity eigenvalue), but also transvections, reflections with determinant 1 which can not be diagonalized. The transvections in Gl(V ) prevent one from developing a theory of reflection groups mirroring that for Coxeter groups or complex reflection groups."
— Julia Hartmann and Anne V. Shepler, "Jacobians of Reflection Groups," Transactions of the American Mathematical Society , Vol. 360, No. 1 (2008), pp. 123-133 (Pdf available at CiteSeer.)
See also A Simple Reflection Group of Order 168 and this morning's Savage Logic.
Tuesday, July 6, 2010
What “As” Is
or: Combinatorics (Rota) as Philosophy (Heidegger) as Geometry (Me)
“Dasein’s full existential structure is constituted by
the ‘as-structure’ or ‘well-joined structure’ of the rift-design*…”
— Gary Williams, post of January 22, 2010
Background—
Gian-Carlo Rota on Heidegger…
“… The universal as is given various names in Heidegger’s writings….
The discovery of the universal as is Heidegger’s contribution to philosophy….
The universal ‘as‘ is the surgence of sense in Man, the shepherd of Being.
The disclosure of the primordial as is the end of a search that began with Plato….
This search comes to its conclusion with Heidegger.”
— “Three Senses of ‘A is B’ in Heideggger,” Ch. 17 in Indiscrete Thoughts
… and projective points as separating rifts—
* rift-design— Definition by Deborah Levitt—
“Rift. The stroke or rending by which a world worlds, opening both the ‘old’ world and the self-concealing earth to the possibility of a new world. As well as being this stroke, the rift is the site— the furrow or crack— created by the stroke. As the ‘rift design‘ it is the particular characteristics or traits of this furrow.”
— “Heidegger and the Theater of Truth,” in Tympanum: A Journal of Comparative Literary Studies, Vol. 1, 1998
Saturday, December 26, 2009
Annals of Philosophy
Towards a Philosophy of Real Mathematics, by David Corfield, Cambridge U. Press, 2003, p. 206:
"Now, it is no easy business defining what one means by the term conceptual…. I think we can say that the conceptual is usually expressible in terms of broad principles. A nice example of this comes in the form of harmonic analysis, which is based on the idea, whose scope has been shown by George Mackey (1992) to be immense, that many kinds of entity become easier to handle by decomposing them into components belonging to spaces invariant under specified symmetries."
For a simpler example of this idea, see the entities in The Diamond Theorem, the decomposition in A Four-Color Theorem, and the space in Geometry of the 4×4 Square. The decomposition differs from that of harmonic analysis, although the subspaces involved in the diamond theorem are isomorphic to Walsh functions— well-known as discrete analogues of the trigonometric functions of traditional harmonic analysis.
Friday, October 24, 2008
Friday October 24, 2008
“The Cube Space” is a name given to the eightfold cube in a vulgarized mathematics text, Discrete Mathematics: Elementary and Beyond, by Laszlo Lovasz et al., published by Springer in 2003. The identification in a natural way of the eight points of the linear 3-space over the 2-element field GF(2) with the eight vertices of a cube is an elementary and rather obvious construction, doubtless found in a number of discussions of discrete mathematics. But the less-obvious generation of the affine group AGL(3,2) of order 1344 by permutations of parallel edges in such a cube may (or may not) have originated with me. For descriptions of this process I wrote in 1984, see Diamonds and Whirls and Binary Coordinate Systems. For a vulgarized description of this process by Lovasz, without any acknowledgement of his sources, see an excerpt from his book.
Thursday, February 28, 2008
Thursday February 28, 2008
From an entry today at the weblog of Lieven Le Bruyn (U. of Antwerp):
“MUBs (for Mutually Unbiased Bases) are quite popular at the moment. Kea is running a mini-series Mutual Unbias….”
The link to Kea (Marni Dee Sheppeard (pdf) of New Zealand) and a link in her Mutual Unbias III (Feb. 13) lead to the following illustration, from a talk, “Discrete phase space based on finite fields,” by William Wootters at the Perimeter Institute in 2005:

This illustration makes clear the
close relationship of MUB’s to the
finite geometry of the 4×4 square.
“Quantum Information Theory Related to Finite Geometry,”
and a comment at The n-Category Cafe,
On Spekkens’ toy system and finite geometry.
Sunday, September 2, 2007
Sunday September 2, 2007
Comment at the
n-Category Cafe
Re: This Week’s Finds in Mathematical Physics (Week 251)
On Spekkens’ toy system and finite geometry
Background–
- In “Week 251” (May 5, 2007), John wrote:
“Since Spekkens’ toy system resembles a qubit, he calls it a “toy bit”. He goes on to study systems of several toy bits – and the charming combinatorial geometry I just described gets even more interesting. Alas, I don’t really understand it well: I feel there must be some mathematically elegant way to describe it all, but I don’t know what it is…. All this is fascinating. It would be nice to find the mathematical structure that underlies this toy theory, much as the category of Hilbert spaces underlies honest quantum mechanics.” - In the n-Category Cafe ( May 12, 2007, 12:26 AM, ) Matt Leifer wrote:
“It’s crucial to Spekkens’ constructions, and particularly to the analog of superposition, that the state-space is discrete. Finding a good mathematical formalism for his theory (I suspect finite fields may be the way to go) and placing it within a comprehensive framework for generalized theories would be very interesting.” - In the n-category Cafe ( May 12, 2007, 6:25 AM) John Baez wrote:
“Spekkens and I spent an afternoon trying to think about his theory as quantum mechanics over some finite field, but failed — we almost came close to proving it couldnt’ work.”
On finite geometry:
- In “Week 234” (June 12, 2006), John wrote:
“For a pretty explanation of M24… try this:
… Steven H. Cullinane, Geometry of the 4 × 4 square,
http://finitegeometry.org/sc/16/geometry.html”
The actions of permutations on a 4 × 4 square in Spekkens’ paper (quant-ph/0401052), and Leifer’s suggestion of the need for a “generalized framework,” suggest that finite geometry might supply such a framework. The geometry in the webpage John cited is that of the affine 4-space over the two-element field.
Related material:
Sept. 5, 2007
See also arXiv:0707.0074v1 [quant-ph], June 30, 2007:
A fully epistemic model for a local hidden variable emulation of quantum dynamics,
by Michael Skotiniotis, Aidan Roy, and Barry C. Sanders, Institute for Quantum Information Science, University of Calgary. Abstract: "In this article we consider an augmentation of Spekkens’ toy model for the epistemic view of quantum states [1]…."
Hypercube from the Skotiniotis paper:

Reference:
Evidence for the epistemic view of quantum states: A toy theory,
Perimeter Institute for Theoretical Physics, 31 Caroline Street North, Waterloo, Ontario, Canada N2L 2Y5 (Received 11 October 2005; revised 2 November 2006; published 19 March 2007.)
Saturday, July 14, 2007
Saturday July 14, 2007
Catholic University
of America
The August 2007 issue of Notices of the American Mathematical Society contains tributes to the admirable personal qualities and mathematical work of the late Harvard professor George Mackey. For my own tributes, see Log24 on March 17, 2006, April 29, 2006, and March 10, 2007. For an entry critical of Mackey’s reductionism– a philosophical, not mathematical, error– see Log24 on May 23, 2007 (“Devil in the Details”).
Here is another attack on reductionism, from a discussion of the work of another first-rate mathematician, the late Gian-Carlo Rota of MIT:
“Another theme developed by Rota is that of ‘Fundierung.’ He shows that throughout our experience we encounter things that exist only as founded upon other things: a checkmate is founded upon moving certain pieces of chess, which in turn are founded upon certain pieces of wood or plastic. An insult is founded upon certain words being spoken, an act of generosity is founded upon something’s being handed over. In perception, for example, the evidence that occurs to us goes beyond the physical impact on our sensory organs even though it is founded upon it; what we see is far more than meets the eye. Rota gives striking examples to bring out this relationship of founding, which he takes as a logical relationship, containing all the force of logical necessity. His point is strongly antireductionist. Reductionism is the inclination to see as ‘real’ only the foundation, the substrate of things (the piece of wood in chess, the physical exchange in a social phenomenon, and especially the brain as founding the mind) and to deny the true existence of that which is founded. Rota’s arguments against reductionism, along with his colorful examples, are a marvelous philosophical therapy for the debilitating illness of reductionism that so pervades our culture and our educational systems, leading us to deny things we all know to be true, such as the reality of choice, of intelligence, of emotive insight, and spiritual understanding. He shows that ontological reductionism and the prejudice for axiomatic systems are both escapes from reality, attempts to substitute something automatic, manageable, and packaged, something coercive, in place of the human situation, which we all acknowledge by the way we live, even as we deny it in our theories.”
— Robert Sokolowski, foreword to Rota’s Indiscrete Thoughts
 Fr. Robert Sokolowski, Ph.D., is Professor of Philosophy at The Catholic University of America in Washington, D.C. Ordained a Roman Catholic priest in 1962, he is internationally recognized and honored for his work in philosophy, particularly phenomenology. In 1994, Catholic University sponsored a conference on his work and published several papers and other essays under the title, The Truthful and the Good, Essays In Honor of Robert Sokolowski. |
The tributes to Mackey are contained in the first of two feature articles in the August 2007 AMS Notices. The second feature article is a review of a new book by Douglas Hofstadter. For some remarks related to that article, see Thursday’s Log24 entry “Not Mathematics but Theology.”
Tuesday, February 20, 2007
Tuesday February 20, 2007
Today is the 21st birthday of my note “The Relativity Problem in Finite Geometry.”
Some relevant quotations:
“This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.”
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
Describing the branch of mathematics known as Galois theory, Weyl says that it
“… is nothing else but the relativity theory for the set Sigma, a set which, by its discrete and finite character, is conceptually so much simpler than the infinite set of points in space or space-time dealt with by ordinary relativity theory.”
— Weyl, Symmetry, Princeton University Press, 1952, p. 138
Weyl’s set Sigma is a finite set of complex numbers. Some other sets with “discrete and finite character” are those of 4, 8, 16, or 64 points, arranged in squares and cubes. For illustrations, see Finite Geometry of the Square and Cube. What Weyl calls “the relativity problem” for these sets involves fixing “objectively” a class of equivalent coordinatizations. For what Weyl’s “objectively” means, see the article “Symmetry and Symmetry Breaking,” by Katherine Brading and Elena Castellani, in the Stanford Encyclopedia of Philosophy:
“The old and natural idea that what is objective should not depend upon the particular perspective under which it is taken into consideration is thus reformulated in the following group-theoretical terms: what is objective is what is invariant with respect to the transformation group of reference frames, or, quoting Hermann Weyl (1952, p. 132), ‘objectivity means invariance with respect to the group of automorphisms [of space-time].‘[22]
22. The significance of the notion of invariance and its group-theoretic treatment for the issue of objectivity is explored in Born (1953), for example. For more recent discussions see Kosso (2003) and Earman (2002, Sections 6 and 7).
References:
Born, M., 1953, “Physical Reality,” Philosophical Quarterly, 3, 139-149. Reprinted in E. Castellani (ed.), Interpreting Bodies: Classical and Quantum Objects in Modern Physics, Princeton, NJ: Princeton University Press, 1998, pp. 155-167.
Earman, J., 2002, “Laws, Symmetry, and Symmetry Breaking; Invariance, Conservation Principles, and Objectivity,’ PSA 2002, Proceedings of the Biennial Meeting of the Philosophy of Science Association 2002, forthcoming [Abstract/Preprint available online]
Kosso, P., 2003, “Symmetry, objectivity, and design,” in K. Brading and E. Castellani (eds.), Symmetries in Physics: Philosophical Reflections, Cambridge: Cambridge University Press, pp. 410-421.
Weyl, H., 1952, Symmetry, Princeton, NJ: Princeton University Press.
See also
Archives Henri Poincaré (research unit UMR 7117, at Université Nancy 2, of the CNRS)–
“Minkowski, Mathematicians, and the Mathematical Theory of Relativity,” by Scott Walter, in The Expanding Worlds of General Relativity (Einstein Studies, volume 7), H. Goenner, J. Renn, J. Ritter and T. Sauer, editors, Boston/Basel: Birkhäuser, 1999, pp. 45-86–
“Developing his ideas before Göttingen mathematicians in April 1909, Klein pointed out that the new theory based on the Lorentz group (which he preferred to call ‘Invariantentheorie’) could have come from pure mathematics (1910: 19). He felt that the new theory was anticipated by the ideas on geometry and groups that he had introduced in 1872, otherwise known as the Erlangen program (see Gray 1989: 229).”
References:
Gray, Jeremy J. (1989). Ideas of Space. 2d ed. Oxford: Oxford University Press.
Klein, Felix. (1910). “Über die geometrischen Grundlagen der Lorentzgruppe.” Jahresbericht der deutschen Mathematiker-Vereinigung 19: 281-300. [Reprinted: Physikalische Zeitschrift 12 (1911): 17-27].
Related material: A pathetically garbled version of the above concepts was published in 2001 by Harvard University Press. See Invariances: The Structure of the Objective World, by Robert Nozick.
Saturday, June 4, 2005
Saturday June 4, 2005
The 4×4 Square:
French Perspectives
Earendil_Silmarils:

Les Anamorphoses:

"Pour construire un dessin en perspective,
le peintre trace sur sa toile des repères:
la ligne d'horizon (1),
le point de fuite principal (2)
où se rencontre les lignes de fuite (3)
et le point de fuite des diagonales (4)."
_______________________________
Serge Mehl,
Perspective &
Géométrie Projective:
"… la géométrie projective était souvent
synonyme de géométrie supérieure.
Elle s'opposait à la géométrie
euclidienne: élémentaire…
La géométrie projective, certes supérieure
car assez ardue, permet d'établir
de façon élégante des résultats de
la géométrie élémentaire."
Similarly…
Finite projective geometry
(in particular, Galois geometry)
is certainly superior to
the elementary geometry of
quilt-pattern symmetry
and allows us to establish
de façon élégante
some results of that
elementary geometry.
Other Related Material…
from algebra rather than
geometry, and from a German
rather than from the French:
"This is the relativity problem:
to fix objectively a class of
equivalent coordinatizations
and to ascertain
the group of transformations S
mediating between them."
— Hermann Weyl,
The Classical Groups,
Princeton U. Press, 1946

Evariste Galois
Weyl also says that the profound branch
of mathematics known as Galois theory
relativity theory for the set Sigma,
a set which, by its discrete and
finite character, is conceptually
so much simpler than the
infinite set of points in space
or space-time dealt with
by ordinary relativity theory."
— Weyl, Symmetry,
Princeton U. Press, 1952
Metaphor and Algebra…
"Perhaps every science must
start with metaphor
and end with algebra;
and perhaps without metaphor
there would never have been
any algebra."
Max Black, Models and Metaphors, 1962
For metaphor and
algebra combined, see
in a diamond ring,"
A.M.S. abstract 79T-A37,
Notices of the
American Mathematical Society,
February 1979, pages A-193, 194 —
the original version of the 4×4 case
of the diamond theorem.
More on Max Black…
"When approaching unfamiliar territory, we often, as observed earlier, try to describe or frame the novel situation using metaphors based on relations perceived in a familiar domain, and by using our powers of association, and our ability to exploit the structural similarity, we go on to conjecture new features for consideration, often not noticed at the outset. The metaphor works, according to Max Black, by transferring the associated ideas and implications of the secondary to the primary system, and by selecting, emphasising and suppressing features of the primary in such a way that new slants on it are illuminated."
— Paul Thompson, University College, Oxford,
The Nature and Role of Intuition
in Mathematical Epistemology
That intuition, metaphor (i.e., analogy), and association may lead us astray is well known. The examples of French perspective above show what might happen if someone ignorant of finite geometry were to associate the phrase "4×4 square" with the phrase "projective geometry." The results are ridiculously inappropriate, but at least the second example does, literally, illuminate "new slants"– i.e., diagonals– within the perspective drawing of the 4×4 square.
Similarly, analogy led the ancient Greeks to believe that the diagonal of a square is commensurate with the side… until someone gave them a new slant on the subject.
Friday, February 20, 2004
Friday February 20, 2004
Finite Relativity
Today is the 18th birthday of my note
“The Relativity Problem in Finite Geometry.”
That note begins with a quotation from Weyl:
“This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.”
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
Here is another quotation from Weyl, on the profound branch of mathematics known as Galois theory, which he says
“… is nothing else but the relativity theory for the set Sigma, a set which, by its discrete and finite character, is conceptually so much simpler than the infinite set of points in space or space-time dealt with by ordinary relativity theory.”
— Weyl, Symmetry, Princeton University Press, 1952, p. 138
This second quotation applies equally well to the much less profound, but more accessible, part of mathematics described in Diamond Theory and in my note of Feb. 20, 1986.
Saturday, November 1, 2003
Saturday November 1, 2003
Symmetry in Diamond Theory:
Robbing Peter to Pay Paul
"Groups arise in most areas of pure and applied mathematics, usually as a set of operators or transformations of some structure. The appearance of a group generally reflects some kind of symmetry in the object under study, and such symmetry may be considered one of the fundamental notions of mathematics."
"Counter-change is sometimes known as Robbing Peter to Pay Paul."
|
|
|
|
Paul Robeson in |
For a look at the Soviet approach
to counterchange symmetry, see
The Kishinev School of Discrete Geometry.
The larger cultural context:
See War of Ideas (Oct. 24),
The Hunt for Red October (Oct. 25),
On the Left (Oct. 25), and
ART WARS for Trotsky's Birthday (Oct. 26).
Monday, May 26, 2003
Monday May 26, 2003
Mental Health Month, Day 26:
Part III — Why 26?
At first blush, it seems unlikely that the number 26=2×13, as a product of only two small primes (and those distinct) has any purely mathematical properties of interest. (On the other hand, consider the number 6.) Parts I and II of “Many Dimensions,” notes written earlier today, deal with the struggles of string theorists to justify their contention that a space of 26 dimensions may have some significance in physics. Let them struggle. My question is whether there are any interesting purely mathematical properties of 26, and it turns out, surprisingly, that there are some such properties. All this is a longwinded way of introducing a link to the web page titled “Info on M13,” which gives details of a 1997 paper by J. H. Conway*.
|
“Conway describes the beautiful construction of a discrete mathematical structure which he calls ‘ Why do the simple groups In fact, both the Mathieu group To understand the definition of The points and the lines and the “is-contained-in” relation form an incidence structure over …the 26 objects of the incidence structure [are] 13 points and 13 lines.”
Conway’s construction involves the arrangement, in a circular Levi graph, of 26 marks representing these points and lines, and chords representing the “contains/is contained in” relation. The resulting diagram has a pleasingly symmetric appearance. For further information on the geometry of the number 26, one can look up all primitive permutation groups of degree 26. Conway’s work suggests we look at sets (not just groups) of permutations on n elements. He has shown that this is a fruitful approach for n=13. Whether it may also be fruitful for n=26, I do not know. |
There is no obvious connection to physics, although the physics writer John Baez quoted in my previous two entries shares Conway’s interest in the Mathieu groups.
* J. H. Conway, “M13,” in Surveys in Combinatorics, 1997, edited by R. A. Bailey, London Mathematical Society Lecture Note Series, 241, Cambridge University Press, Cambridge, 1997. 338 pp. ISBN 0 521 59840 0.