Tuesday, May 5, 2020
Raiders of the Lost Horizon
Wednesday, April 22, 2020
Deep Horizon
For some mathematical background, see Deep + Hardy.
Related entertainment —

Related non-entertainment —

Saturday, November 30, 2019
Horizon
Adam Gopnik in The New Yorker today, quoting
the late Clive James's description of …
" … an adaptation of 'War and Peace': 'Dead ground is
the territory you can’t judge the extent of until you approach it:
seen from a distance, it is unseen. Almost uniquely amongst
imagined countries, Tolstoy’s psychological landscape is
without dead ground— the entire vista of human experience is
lit up with an equal, shadowless intensity, so that separateness
and clarity continue even to the horizon.' "
Wednesday, July 3, 2019
Monday, August 13, 2018
Noetic Acts and Horizonal Contexts
Sunday, January 14, 2018
Horizon
Thursday, November 16, 2017
Lost Horizon
Wednesday, February 22, 2017
Horizon
A memorable phrase —
"the transcendental horizon of the ‘I’."
For some backstory, see a Google search for
Marion + transcendental + horizon.
For a perhaps more intelligible horizon, see
Line at Infinity in this journal.
Sunday, January 1, 2017
Like the Horizon
(Continued from a remark by art critic Peter Schjeldahl quoted here
last year on New Year's Day in the post "Art as Religion.")
"The unhurried curve got me.
It was like the horizon of a world
that made a non-world of
all of the space outside it."
— Peter Schjeldahl, "Postscript: Ellsworth Kelly,"
The New Yorker , December 30, 2015
This suggests some further material from the paper
that was quoted here yesterday on New Year's Eve —
"In teaching a course on combinatorics I have found
students doubting the existence of a finite projective
plane geometry with thirteen points on the grounds
that they could not draw it (with 'straight' lines)
on paper although they had tried to do so. Such a
lack of appreciation of the spirit of the subject is but
a consequence of the elements of formal geometry
no longer being taught in undergraduate courses.
Yet these students were demanding the best proof of
existence, namely, production of the object described."
— Derrick Breach (See his obituary from 1996.)
A related illustration of the 13-point projective plane
from the University of Western Australia:

Projective plane of order 3
(The four points on the curve
at the right of the image are
the points on the line at infinity .)
The above image is from a post of August 7, 2012,
"The Space of Horizons." A related image —
Click on the above image for further remarks.
Wednesday, July 15, 2015
New Horizons
"We have the answer to all your fears
It's short, it's simple, it's crystal clear"
See as well last night's Midnight Review.
Tuesday, August 7, 2012
The Space of Horizons
"In the space of horizons that neither love nor hate"
— Wallace Stevens, "Things of August"
Seven years ago yesterday—
For some context, see Rosetta Stone as a Metaphor.
Related material from the University of Western Australia—

Projective plane of order 3
(The four points on the curve
at the right of the image are
the points on the line at infinity.)
Art critic Robert Hughes, who nearly died in Western
Australia in a 1999 car crash, actually met his death
yesterday at Calvary Hospital in the Bronx.
See also Hughes on "slow art" in this journal.
Thursday, December 26, 2024
Chrome Art … Continues.
Monday, November 25, 2024
Longfellow vs. Snark
"Such were the plans I'd made" — 1974 song lyric
Henry Wadsworth Longfellow —
"Life is real! Life is earnest!"
"New horizons open up!"
— Anonymous NY Times wit, 1924
Gatsby-Era Times Snark —
Saturday, November 23, 2024
The Square Aspect (vs. Stevens’s “Radial* Aspect”)
"New horizons open up." — New York Times, Nov. 21, 1924.
A search for some such 96-square division yields . . .
https://www.atariuptodate.de/img/kidshapes.png —

"Here's to efficient packing."
* See as well radial vs. square aspects in a post on Mackey's classic
Harmonic Analysis as the Exploitation of Symmetry.
Tuesday, April 16, 2024
The Pi Script
|
The studios, now beholden to much larger companies and financial institutions, became subject to oversight focused on short-term horizons. This summer, I spoke with the head of a film and TV studio purchased by a private-equity firm in recent years. “It used to be there were these big, crusty, old legacy companies that had a longer-term view,” he said, “that could absorb losses, and could take risks. But now everything is driven by quarterly results. The only thing that matters is the next board meeting. You don’t make any decisions that have long-term benefits. You’re always just thinking about, ‘How do I meet my numbers?’ ” Efficiency and risk avoidance began to run the game.
— "The Life and Death of Hollywood: |
Monday, March 4, 2024
Report on the Diamond Theorem
by Google Gemini (Advanced)
|
by Google Gemini AI Monday, March 4, 2024
Absolutely! Here's an academic paper about the Cullinane diamond theorem. Please note that while I provide citations, in a fully formatted paper you'd need to adhere to your chosen academic style guide for proper formatting:
The Cullinane Diamond Theorem: Abstract This paper examines the Cullinane diamond theorem, a principle in finite projective geometry that offers unexpected insights into the symmetries found in graphic designs. The theorem, initially formulated by Steven H. Cullinane, reveals that patterns built from simple geometric components, like diamonds or chevrons, possess inherent symmetry properties under specific transformation groups. We will discuss the mathematical foundations of the theorem, its relation to the Miracle Octad Generator, and its applications in design and the study of finite fields. Introduction Symmetry plays a vital role in art and design, contributing to a sense of balance, harmony, and aesthetic appeal. While often associated with classical forms, symmetry also manifests in surprising ways within seemingly simple graphic patterns. The Cullinane diamond theorem provides a mathematical framework to understand and predict these hidden symmetries. Mathematical Background Finite Projective Geometries: The Cullinane diamond theorem has its roots in finite projective geometry. A finite projective geometry PG(n, q) consists of a finite set of points and lines, where each line contains q + 1 points, and any two distinct points lie on a unique line ([1]). Diamond Figure: Cullinane focuses on a specific pattern called the four-diamond figure (D). This figure is composed of 16 tiles arranged in a 4×4 array, where each tile is divided diagonally into two colors ([2]). Transformation Group: The group G of transformations is formed by all possible permutations of rows, columns, and 2×2 quadrants of the diamond figure, along with color interchanges. The size of this group is 322,560 ([2]). The Cullinane Diamond Theorem
Theorem:
Proof: Connections to the Miracle Octad Generator The Cullinane diamond theorem shares a relationship with R.T. Curtis's Miracle Octad Generator (MOG). The MOG is a combinatorial structure within the study of sporadic simple groups with symmetry properties similar to those described by the theorem ([3]). Applications Graphic Design: The theorem informs artistic compositions employing diamond and chevron-like shapes. Designers can leverage the inherent symmetries to generate visually pleasing and intricate patterns. Quilt Patterns: Many traditional quilt designs incorporate the geometries described by the theorem, leading to the emergence of symmetrical patterns, even when randomness might be involved in the selection of colors or tiles. Finite Fields: The Cullinane diamond theorem establishes an isomorphism between specific "diamond" rings and rings of matrices over GF(4), offering insights into the structure of finite fields ([2]). Conclusion The Cullinane diamond theorem elegantly connects abstract mathematics with the world of graphic design. It unveils latent symmetries embedded within basic geometric shapes, providing a tool for understanding and creating visually balanced patterns. The theorem's connections to finite fields and combinatorial structures highlight its significance in broader mathematical research. Citations [1] Weisstein, E. W. “Finite Projective Geometry.” From MathWorld–A Wolfram Web Resource. [invalid URL removed] [2] Cullinane, S. H. "Cullinane Diamond Theorem." Encyclopedia of Mathematics. https://encyclopediaofmath.org/wiki/Cullinane_diamond_theorem [3] Curtis, R. T. (1976). A New Combinatorial Approach to M₂₄. Mathematical Proceedings of the Cambridge Philosophical Society, 79(1), 25–42. doi:10.1017/S0305004100052075 Let me know if you'd like me to expand on any particular aspect or provide additional citations!
1. www.hellenicaworld.com/Science/Mathematics/en/MathieuGroup.html
|
by Google Gemini (Advanced)
Tuesday, January 30, 2024
Monday, January 22, 2024
Thursday, December 14, 2023
Unsocratic Dialogue (with Gemini AI in Bard Today)

|
What is the Cullinane diamond theorem?
|
Friday, June 30, 2023
Trickster Fuge (German for Joint)
|
Margaret Atwood on Lewis Hyde's "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159) What is "the next world"? It might be the Underworld…. The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart. |
"Drop me a line" — Request attributed to Emma Stone
Saturday, January 14, 2023
Châtelet on Weil — A “Space of Gestures”
|
From Gilles Châtelet, Introduction to Figuring Space Metaphysics does have a catalytic effect, which has been described in a very beautiful text by the mathematician André Weil: Nothing is more fertile, all mathematicians know, than these obscure analogies, these murky reflections of one theory in another, these furtive caresses, these inexplicable tiffs; also nothing gives as much pleasure to the researcher. A day comes when the illusion vanishes: presentiment turns into certainty … Luckily for researchers, as the fogs clear at one point, they form again at another.4 André Weil cuts to the quick here: he conjures these 'murky reflections', these 'furtive caresses', the 'theory of Galois that Lagrange touches … with his finger through a screen that he does not manage to pierce.' He is a connoisseur of these metaphysical 'fogs' whose dissipation at one point heralds their reforming at another. It would be better to talk here of a horizon that tilts thereby revealing a new space of gestures which has not as yet been elucidated and cut out as structure. 4 A. Weil, 'De la métaphysique aux mathématiques', (Oeuvres, vol. II, p. 408.) |
For gestures as fogs, see the oeuvre of Guerino Mazzola.
For some clearer remarks, see . . .
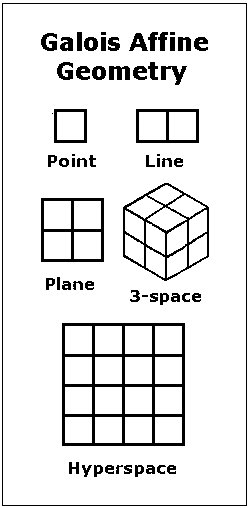
Illustrations of object and gestures
from finitegeometry.org/sc/ —
Object
Gestures
An earlier presentation
of the above seven partitions
of the eightfold cube:
|
|
Related material: Galois.space .
Wednesday, December 28, 2022
The Santa Fe Institute as Magisterium Wannabe
"The novelist Cormac McCarthy has been a fixture around
the Santa Fe Institute since its embryonic stages in the
early 1980s. Cormac received a MacArthur Award in 1981
and met one of the members of the board of the MacArthur
Foundation, Murray Gell-Mann, who had won the Nobel Prize
in physics in 1969. Cormac and Murray discovered that they
shared a keen interest in just about everything under the sun
and became fast friends. When Murray helped to found the
Santa Fe Institute in 1984, he brought Cormac along, knowing
that everyone would benefit from this cross-disciplinary
collaboration." — https://www.santafe.edu/news-center/news/
cormac-and-sfi-abiding-friendship
Joy Williams, review of two recent Cormac McCarthy novels —
"McCarthy has pocketed his own liturgical, ecstatic style
as one would a coin, a ring, a key, in the service of a more
demanding and heartless inquiry through mathematics and
physics into the immateriality, the indeterminacy, of reality."
A Demanding and Heartless Coin, Ring, and Key:
COIN

RING
"We can define sums and products so that the G-images of D generate
an ideal (1024 patterns characterized by all horizontal or vertical "cuts"
being uninterrupted) of a ring of 4096 symmetric patterns. There is an
infinite family of such 'diamond' rings, isomorphic to rings of matrices
over GF(4)."
KEY
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in
Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference
(July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis,
James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97.
For those who prefer a "liturgical, ecstatic style" —

Thursday, November 10, 2022
For Students of the Forked Tongue



The above 1975 book by Robert Greer Cohn, Modes of Art, is
Volume I of a planned three-volume work.
The passage below is from a review of Cohn's Vol. II, Ways of Art —
|
Franklin, Ursula (1987) "Book Review: A Critical Work II. . . . . Those not familiar with the author's epistemology should begin with Appendix A of Ways of Art , a schematic demonstration of his tetrapolar-polypolar-dialectic, especially as it concerns the development of the French novel within the European tradition. But this dialectic, which has antecedents in Kierkegaard, Mallarme and Joyce, underlies all art, because: "this dimensional pulsation, or tetrapolar (and polypolar) higher vibrancy is, in short, the stuff of life: life is vibrant in this more complex way as well as in the more bipolar sense" (7). Cohn shows that "far out enough" the male or linear and the female or circular, the male vertical and the female horizontal dimensions "tend to merge as in relativity theory" (19). Ways of Art shows us the way through a historical becoming of art in its complex dialectic in which the metonymic (horizontal) axis constantly interrelates with the metaphoric (vertical). "Life is the mother, art the father" (vii); hence Cohn's quarrel with most contemporary Feminism, which is pronounced throughout the volume. Firmly grounded in its author's tetra-polypolar epistemology, this beautiful book becomes, however, at no point dryly abstract; it is the mature work of a true humanist who stands in clear and open opposition to the dehumanizing trend of "the quasi-scientific reductionism and abstract gimmickry of a great deal of current academic literary study, bellwethered by the structuralists, post-structuralists, and deconstructionists" (vi). Abundant footnotes constitute a substantial part of Ways of Art , on occasion developing insights almost into essays demonstrating crucial points along the general flow of the tradition from "Obscure Beginnings;' the opening chapter, to our "Contemporaries;' the last. Cohn reminds us that "In the Beginning was the Word;' for the Judaeo-Christian tradition at least, which his study fervently embraces; thus, for example, in Appendix 0 on "The Dance of the Sexes;' he censures "those who live by slogans, camps, and peer-opinion, the countless little bastard cults which characterize an era which has massively veered away from our free and beautiful Greco-Judaeo-Christian tradition" (332). Cohn traces man's way and that of his myths and rituals culminating in his art from that beginning along the lines of Freud, Neumann and Cassirer, and many others, always demonstrating the underlying polypolar dialectical rhythm. Thus in "From Barbarism to Young Culture;' we follow the Celts to Druidic ritual, Hebrew beginnings to the Psalms, Dionysian ritual to Greek tragedy, and thence to the beginnings of French dramatic literature originating in the Quem quaeritis sequence of the medieval Mass. Along the way arises artistic symbolism, for Cohn synonymous with "effective poetry;' to finally "ripen in France as never before" (99). Table I (134) graphs this development from the twelfth to the late nineteenth and early twentieth centuries. The author traces the rise of the artistic vocation from its antecedents in the double function of bard and priest, with the figure of Ronsard at the crossroads of that dying institution and the nascent concept of personal glory. "The Enlightenment Vocation" is exemplified in Montaigne, who humanizes the French cultural elite and points the way to French classicism and, farther down the road, after the moral collapse with the outgoing reign of Louis XIV, toward the Age of Reason. Clearly the most significant figure of the French Enlightenment for all of Western civilization is Rousseau, and Cohn beautifully shows us why this is so. Subsequently, "the nineteenth-century stage of the writer's journey will lead, starting from the crossroads of Rousseau, primarily in these two directions: the imperialistic and visionary prose of Balzac, the equally ambitious poetry of Mallarme", brothers under the skin" (199). And these two paths will then be reconciled in Proust's monumental A la recherche du temps perdu . . . . . |
Thursday, September 29, 2022
The 4×6 Problem*
The exercise posted here on Sept. 11, 2022, suggests a
more precisely stated problem . . .
The 24 coordinate-positions of the 4096 length-24 words of the
extended binary Golay code G24 can be arranged in a 4×6 array
in, of course, 24! ways.
Some of these ways are more geometrically natural than others.
See, for instance, the Miracle Octad Generator of R. T. Curtis.
What is the size of the largest subcode C of G24 that can be
arranged in a 4×6 array in such a way that the set of words of C
is invariant under the symmetry group of the rectangle itself, i.e. the
four-group of the identity along with horizontal and vertical reflections
and 180-degree rotation.
Recent Log24 posts tagged Bitspace describe the structure of
an 8-dimensional (256-word) code in a 4×6 array that has such
symmetry, but it is not yet clear whether that "cube-motif" code
is a Golay subcode. (Its octads are Golay, but possibly not all its
dodecads; the octads do not quite generate the entire code.)
Magma may have an answer, but I have had little experience in
its use.
* Footnote of 30 September 2022. The 4×6 problem is a
special case of a more general symmetric embedding problem.
Given a linear code C and a mapping of C to parts of a geometric
object A with symmetry group G, what is the largest subcode of C
invariant under G? What is the largest such subcode under all
such mappings from C to A?
Monday, July 25, 2022
Narratives in the Multiverse of Madness
Modernism, Fiction and Mathematics
by Nina Engelhardt
(Edinburgh Critical Studies in Modernist Culture)
From a review by Johann A. Makowsky in
Notices of the American Mathematical Society,
November 2020, pp. 1589-1595 —
"Engelhardt’s goal in this study is to put the interplay
between fiction and mathematical conceptualizations
of the world into its historical context. She sees her work
as a beginning for further studies on the role of mathematics,
not only modern, in fiction in the wider field of literature and
science. It is fair to say that in her book Nina Engelhardt does
succeed in giving us an inspiring tour d’horizon of this interplay."
Another such tour —

On the title of Westworld Season 4 Episode 5, "Zhuangzi" —

A song for Teddy: "Across my dreams, with nets of wonder . . ."
See Zhuangzi also in the 2022 Black Rock CIty manifesto, "Waking Dreams" . . .
Wednesday, July 13, 2022
A New Yorker Child’s Progress
A New Yorker writer on why he wanted to
learn mathematics at an advanced age —
|
"The challenge, of course, especially in light of the collapsing horizon, since I was sixty-five when I started. Also, I wanted especially to study calculus because I never had. I didn’t even know what it was—I quit math after feeling that with Algebra II I had pressed my luck as far as I dared. Moreover, I wanted to study calculus because Amie told me that when she was a girl William Maxwell had asked her what she was studying, and when she said calculus he said, 'I loved calculus.' Maxwell would have been about the age I am now. He would have recently retired after forty years as an editor of fiction at The New Yorker , where he had handled such writers as Vladimir Nabokov, Eudora Welty, John Cheever, John Updike, Shirley Hazzard, and J. D. Salinger. When Salinger finished Catcher in the Rye , he drove to the Maxwells’ country house and read it to them on their porch. I grew up in a house on the same country road that Maxwell and his wife, Emily, lived on, and Maxwell was my father’s closest friend."
— Wilkinson, Alec. A Divine Language (p. 5). Published |
See as well two versions of
a very short story, "Turning Nine."
Wilkinson's title is of course deplorable.
Related material: "Night Hunt" in a
Log24 search for the phrase "Good Question."
Friday, May 6, 2022
Use Your Noodle (For Byron Gogol*)
An essay from . . .
The Shape of Things: A Philosophy of Design Wittgenstein’s Architecture The universe of texts can be seen as a landscape. In it one can make out mountains and valleys, rivers and lakes, castles, farmyards and inner-city slums. On the horizon of the scene visualized in this way, the Bible and Homer appear as gigantic ice-covered mountains. The vast, tranquil lake of Aristotle’s texts, where fishermen idly throw their nets and philologists row their boats, occupies a part of the valley bottom. There, the tumbling waterfall of Nietzsche is captured by the broad river of modern pragmatism. Towering above everything, the Gothic cathedral of St Thomas Aquinas’s Summae dominates the cathedral square of the city, in which the roofs and gables of Baroque speculations jostle one another. In the suburbs of this city, one catches sight of the Romantic, Realist and Modernist housing-blocks and factories of more recent litera¬ ture; somewhat apart from all these stands a small, apparently insignificant house resembling scaffolding more than a finished building: Wittgenstein’s building. This little house is called the Tractatus. This name isn’t the product of a one-track mind. For when one enters the house, one notices immediately that this is not a place that has lost track of things. Quite the opposite: It is a place of mirror- images. The house stands on six foundation pillars which support one another by means of cross-beams organized in a hierarchy. In the middle, however, there rises a seventh pillar whose function it is to cut through the building and free it from the ground. So the house with all its corners, angles and joints is protected, armoured and impregnable. And yet, and for that very reason, it is threatened with collapse and disappearance without trace – condemned in advance and from the outset. The building is set out: It consists of propositions. Every proposition presupposes all the preceding ones and is itself the 76 presupposition of all the following propositions. Proposition by proposition, anyone who enters progresses through the prescribed rooms, and his step is supported by consistencies. Suddenly, with one proposition, one single proposition, the ground gives way beneath his feet. He falls head first into the abyss. Wittgenstein’s house is situated in a suburb of that city whose cathedral square is dominated by the towers of Thomas Aquinas’s cathedral. The small, modest pillars of Wittgenstein’s house support one another according to the same logico- philosophical method as the pillars of the cathedral support one another. But there appears to be a world of difference between the cathedral and the little house: The cathedral is a ship pointing in the direction of heaven, and the little house is a trap-door pointing in the direction of a bottomless abyss. But be careful: May Thomas Aquinas not have been right in saying after his revelation that everything he had written before was like straw? May not the heaven above the cathedral be the same black hole as the abyss beneath the little house? May not Wittgenstein’s little house be the cathedral of today? And those mirrors whose images simultaneously mirror one another, may they not be our equivalent of stained-glass windows? The landscape portrayed in this essay, it goes without saying, is a metaphor. Is it possible to identify it as Vienna? And is it possible for anyone entering Wittgenstein’s little house in that unlikely place to make out a hint of the unsayable? What we cannot speak about we must pass over in silence. 77 |
Click the above image to enlarge.
See as well . . .
Update of 2:40 AM May 7, 2022 —
Flusser's seven "pillars" appear to be the main sections of the Tractatus
— numbered 1 through 7, with many intermediate numbered passages.
For a more geometric meditation on "the shape of things," see other
posts tagged "Shape Constant" in this journal.
*Byron Gogol is a tech magnate in the HBO series "Made for Love."

Thursday, February 3, 2022
Monday, November 29, 2021
Abstraction and Structure
For the mathematical properties of the vertical and horizontal
white grid lines above, see the Cullinane theorem.
Monday, August 16, 2021
In a Nutshell: The Core of Everything
“The great Confucius guided China spiritually for over 2,000 years.
The main doctrine is ' 仁 ' pronounced 'ren', meaning two people,
i.e., human relationship. Modern science has been highly competitive.
I think an injection of the human element will make our subject more
healthy and enjoyable."
— Geometer Shiing-Shen Chern in a Wikipedia article
See the "ren" character in Wiktionary. See as well . . .
"The development of ren ( 仁 ) in early Chinese philosophy,"
By Robin Elliott Curtis, U. of B.C. Master's thesis, 2016 —
Thus, we can conclude that several different forms of
the character ren , were in existence during the
Warring States period. This shows that etymological analyses
focusing exclusively on the combination of 人 and 二 are inadequate.
It should also serve as a warning against “character fetishization,”
or giving “exaggerated status to Chinese characters in the interpretation
of Chinese language, thought, and culture.” 46
46 Edward McDonald 2009, p. 1194.
McDonald, Edward. 2009. “Getting over the Walls of
Discourse: 'Character Fetishization' in Chinese Studies.”
The Journal of Asian Studies 68 (4): 1189 – 1213.
Wikipedia article on Ren in Confucianism:
|
人 + 二 = 仁 (Rén) man on left two on right, the relationship between two human beings, means co-humanity. Originally the character was just written as丨二 [citation needed] representing yin yang, the vertical line is yang (bright, traditionally masculine, heaven, odd numbers), the two horizontal lines are yin (dark, traditionally feminine, earth, even numbers), 仁 is the core of everything. |
"The core of everything" . . . Citation needed ?
Thursday, August 12, 2021
A Square Crystal Paperweight
Friday March 31, 2006
|
Tuesday, August 10, 2021
Ex Fano Apollinis
|
Margaret Atwood on Lewis Hyde's "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159) What is "the next world"? It might be the Underworld…. The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart. |
"As a Chinese jar . . . ."
— Four Quartets
|
Rosalind Krauss "If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World , for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete. Or, to take a more up-to-date example…."
"He was looking at the nine engravings and at the circle,
"And it's whispered that soon if we all call the tune
The nine engravings of The Club Dumas
An example of the universal— or, according to Krauss,
"This is the garden of Apollo, |
The "Katz" of the August 7 post Art Angles
is a product of Princeton's
Department of Art and Archaeology.
ART —
ARCHAEOLOGY —

"This pattern is a square divided into nine equal parts.
It has been called the 'Holy Field' division and
was used throughout Chinese history for many
different purposes, most of which were connected
with things religious, political, or philosophical."
– The Magic Square: Cities in Ancient China,
by Alfred Schinz, Edition Axel Menges, 1996, p. 71
Thursday, June 24, 2021
Persons of Interest

|
Margaret Atwood on Lewis Hyde's "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159) What is "the next world"? It might be the Underworld…. The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart. |

Punch Line . . .
Wrap Party!

Saturday, June 12, 2021
The 24 Box
The number 24 may be studied via various geometrical models.
Here is one such model* —

— From a book published in 1999 by Harvard University Press:
* Note for pedagogues: Latour's "three ranks" should be "three files."
Ranks are horizontal, files vertical. Also, there may be more than 24
compartments in the cabinet, but Latour discusses only those shown.
Monday, March 1, 2021
Choice of Viewpoint . . .
Saturday, September 5, 2020
Poetry 101: “Do Not Block Intersection”
The title is from a post of July 27.
From earlier posts (Feb. 20, 2009) —
| Emblematizing the Modern
Note that in applications, the vertical axis of T.S. Eliot:
“Men’s curiosity searches past and future |
Midrash for LA —

Tuesday, August 11, 2020
For the Wellfed Wits
“Somewhere, someplace… there must be a lost horizon…
A Shangri-La where a man can find peace, happiness,
and lots of naked ladies.” — Carl Reiner
Voilà.

Sunday, August 9, 2020
A Turner Classic
The New York Times eulogizes a man who died Friday —
“Rabbi Steinsaltz was a prolific and wide-ranging writer
and a sharp observer of humanity who wrote more than
60 books on philosophy, mysticism, theology, even zoology.
His study of kabbalah, ‘The Thirteen Petalled Rose,’ is
considered a classic and has been translated into eight languages.”
Another classic of Jewish thought:
Thoughts of the young Carl Reiner as rendered above in 1967 —
“Somewhere, someplace… there must be a lost horizon…
A Shangri-La where a man can find peace, happiness,
and lots of naked ladies.”
Voilà.

Friday, May 29, 2020
The May Queens
In remembrance of Else Blangsted and Nancy Stark Smith,
who each reportedly died on May 1, 2020 —
Posts tagged Mayday 2020.
Monday, April 6, 2020
Tuesday, May 14, 2019
The Nothing That Is
Philosophy professor Agnes Callard on giving advice:
"It’s as though right before I give the advice,
I push a button that sucks all the informational
content out of what I’m about to say, and
I end up saying basically nothing at all."
— https://thepointmag.com/2019/
examined-life/against-advice-agnes-callard
From a University of Chicago description of Callard —

See as well posts before and after the above date, Jan. 3, 2018,
that are now tagged "Lost Horizon."
More generally, see a Log24 search for "Lost Horizon."
Thursday, May 2, 2019
New Year’s Eve
The previous post suggests a search for Buber in this journal
that yields a passage from New Year's Eve 2017 —
" As for 'that you in which the lines of relation, though parallel,
intersect,' and 'intimations of eternity,' see Log24 posts on
the concept 'line at infinity' as well as 'Lost Horizon.' "

Related illustrations —
From Pi Day 2017 —
"Don't want to end up a cartoon in a cartoon graveyard."
From April 20, 2019 —
From "A History of Violence" —
Friday, April 20, 2018
Time and Money

|
Emblematizing the Modern
Note that in applications, the vertical axis of the Cross of Descartes often symbolizes the timeless (money, temperature, etc.) while the horizontal axis often symbolizes time. T.S. Eliot
“Men’s curiosity searches past and future |
Sunday, January 21, 2018
At Which Point
"In 'Sophistry,' a new play by Jonathan Marc Sherman
at the Playwrights Horizons Studio, a popular tenured
professor stands accused of sexual harassment
by a male student."
— Frank Rich in The New York Times , theater review
on October 12, 1993
"At which point another play, inchoate but arresting,
edges into view." — Rich, ibid.
"Johansson began acting during childhood,
after her mother started taking her to auditions.
She made her professional acting debut
at the age of eight in the off-Broadway production
of 'Sophistry' with Ethan Hawke, at New York's
Playwrights Horizons."
— IMDb Mini Biography by: Pedro Borges
" 'Suddenly, I was 19 again and I started to remember
all the men I'd known who had taken advantage of
the fact that I was a young woman who didn't yet have
the tools to say no, or to understand the value of
my own self-worth,' the Avengers star described.
'I had many relationships both personal and professional
where the power dynamic was so off that I had to create
a narrative in which I was the cool girl who could hang in
and hang out, and that sometimes meant compromising
what felt right for me . . . . ' "
— Scarlett Johansson yesterday at the 2018 Women's March
in Los Angeles, as reported in E! News .
Image in a Log24 post
of March 12, 2009.
Wednesday, January 17, 2018
Language Game
Continued from Zen and Language Games
(a post of May 2, 2003, written on March 1, 2002)
From The Harvard Crimson on St. Andrew's Day 2017 —
See also a larger, clearer view of the titles in the above file photo.
Dialogue suggested by the above Harvard Crimson line
"I am a book today . . . . I know it all." —
A problem child* of sorts in the 2017 film "Gifted" —
Mary- "Maybe this school isn't as great as you think it is." Mary is returned to the place of her examination. Professor- "Mary, you knew that the problem was incorrect, why didn't you say anything?" Mary- "Frank says I'm not supposed to correct older people. Nobody likes a smart-ass."
* "Problem Child" was a working title related to a novel
Heinlein wrote in 1941, Beyond This Horizon —

Thursday, January 11, 2018
Upper West Side Story Continues
In memory of a resident of the Upper West Side
who reportedly died on Twelfth Night 2018 —
“… the horizon is not the limit of meaning,
but that which extends meaning
from what is directly given
to the whole context in which it is given,
including a sense of a world.”
— David Vessey, Department of Philosophy,
Grand Valley State University,
Gadamer and the Fusion of Horizons,
International Journal of Philosophical Studies,
17/4 (2009), 531-42.
Thursday, January 4, 2018
For a Cartoon Graveyard

“… the horizon is not the limit of meaning,
but that which extends meaning
from what is directly given
to the whole context in which it is given,
including a sense of a world.”
— David Vessey,
Gadamer and the Fusion of Horizons
(Quoted here on Saturday, June 4, 2005.)
Sunday, December 31, 2017
Ich, Du, etc., etc.
Recent posts involving the English pronoun IT referred to
classic tales of horror by Madeleine L'Engle and Stephen King.
Those posts suggest some further remarks by Martin Buber:
THE WORLD IS TWOFOLD for man
in accordance with his twofold attitude.
The attitude of man is twofold
in accordance with the two basic words he can speak.
The basic words are not single words but word pairs.
One basic word is the word pair I-You.
The other basic word is the word pair I-It;
but this basic word is not changed when
He or She takes the place of It.
Thus the I of man is also twofold.
For the I of the basic word I-You is different from
that in the basic word I-It.
— Buber, Martin. I and Thou, Trans. Kaufmann
(p. 53). Kindle Edition.

Four German pronouns from the above passage
by Martin Buber lead to six pronoun pairs:
ich-du, ich-es, ich-sie, du-es, du-sie, es-sie.
This is in accordance with some 1974 remarks by
Marie-Louise von Franz —

The following passage by Buber may confuse readers of
L'Engle and King with its use, in translation, of "it" instead of
the original German "sie" ("she," corresponding to "die Welt") —

Here, for comparison, are the original German and the translation.

As for "that you in which the lines of relation, though parallel,
intersect," and "intimations of eternity," see Log24 posts on
the concept "line at infinity" as well as "Lost Horizon."

Saturday, November 18, 2017
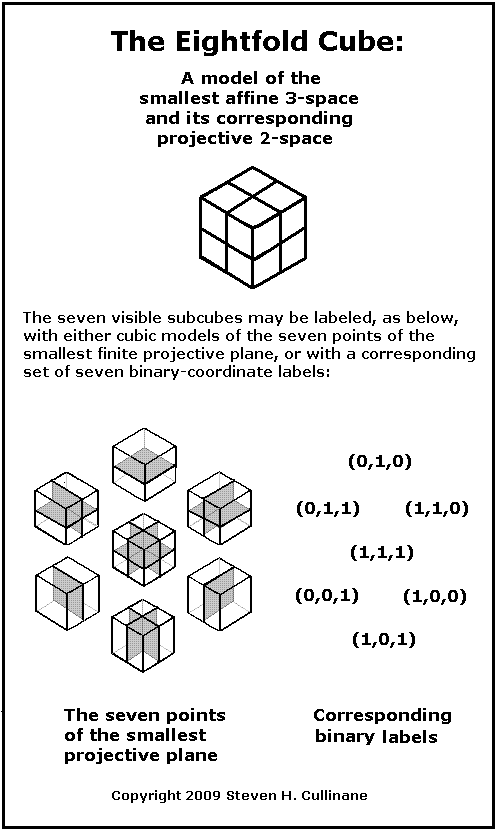
Cube Space Continued
James Propp in the current Math Horizons on the eightfold cube —
For another puerile approach to the eightfold cube,
see Cube Space, 1984-2003 (Oct. 24, 2008).
Wednesday, November 1, 2017
Cameron on All Saints’ Day
"Nowdays, Halloween involves plastic figures of ghosts and bats
bought from the supermarket; this is driven by commerce and
in some people’s view is an American import. But it is clear that
this time of year was traditionally regarded as one where the barrier
between this world and the other was low, and supernatural
manifestations were to be expected."
Remarks related to another "barrier" and vértigo horizontal —

See also a search for Horizon + "Western Australia" in this journal.
From that search: A sort of horizon, a "line at infinity," that is perhaps
more meaningful to most Cameron readers than the above remarks
by Borges —

Sunday, January 1, 2017
The Unhurried Curve
From today's previous post —
"The unhurried curve got me.
It was like the horizon of a world
that made a non-world of
all of the space outside it."
— Peter Schjeldahl, "Postscript: Ellsworth Kelly,"
The New Yorker , December 30, 2015
Related figures —
Art critic Robert Hughes in "The Space of Horizons,"
a Log24 post of August 7, 2012:
Religion writer Huston Smith, who reportedly died
on December 30, 2016:
Wednesday, July 15, 2015
Thursday, May 14, 2015
Imperial Symbology
See Found Symbol in this journal.
See also the Imperial College theorem symbol

and a page from Imperial College about
group actions on a space Ω —
For Han Solo, some less imperial symbology —

Detail of a CKEditor plugin screenshot:
horizontal line, smiley, special characters,
and iframe area.
Friday, April 24, 2015
For Hughes and Crombie*
Recommended —

Links to the poems: "Storm Beach" and "For You."
* See "The Space of Horizons" and A Search for Crombie.
Thursday, July 17, 2014
Paradigm Shift:
Continuous Euclidean space to discrete Galois space*
Euclidean space:
Counting symmetries in Euclidean space:
Galois space:
Counting symmetries of Galois space:

The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
* For related remarks, see posts of May 26-28, 2012.
Sunday, June 8, 2014
Vide

"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Tuesday, May 13, 2014
An Artist’s Memorial
See the above weblog post honoring a Swiss artist‘s
“wit, his perception, his genius, his horizon,
his determination, his humour, his friendship,
and his immeasurable kindness.”
Not a bad sendoff. Contrast with events at Harvard
on the date of the artist’s death.
Related material: An album cover, and …

See also this journal in September 2008.
As far as Swiss art goes, I personally prefer the work of, say,
Karl Gerstner and Paul Talman.
Tuesday, December 11, 2012
Plenitude
In memory of Charles Rosen:
Related material:
The Magic Square in Doctor Faustus (October 10th, 2012)
Elementary Finite Geometry (August 1st, 2012)
The Space of Horizons (August 7th, 2012)
Chromatic Plenitude (Rosen on Schoenberg)
Thursday, September 6, 2012
Decomp Revisited
Frogs:
"Some mathematicians are birds, others are frogs. Birds fly high in the air and survey broad vistas of mathematics out to the far horizon. They delight in concepts that unify our thinking and bring together diverse problems from different parts of the landscape. Frogs live in the mud below and see only the flowers that grow nearby. They delight in the details of particular objects, and they solve problems one at a time."
— Freeman Dyson (See July 22, 2011)
A Rhetorical Question:
"The past decade has been an exciting one in the world of mathematics and a fabulous one (in the literal sense) for mathematicians, who saw themselves transformed from the frogs of fairy tales— regarded with a who-would-want-to-kiss-that aversion, when they were noticed at all— into fascinating royalty, portrayed on stage and screen….
Who bestowed the magic kiss on the mathematical frog?"
Above: Amy Adams in "Sunshine Cleaning"
Related material:
- "Decomposition" in this journal
- "What is Truth (continued)," May 1, 2012
- The Boolean satisfiability problem (SAT)
- Remarks on SAT by Stanislav Busygin
- A related remark on complexity by Busygin
- Hashtag #sexyface and the Empty Chair
Monday, April 23, 2012
Mate in Six
In memory of Mike Wallace—
- An April 8 post noting the death of Wallace—
a NY Times obituary notice with ad at top— "The North Face"
- An April 21 post noting the death of Charles Colson,
reportedly at at 3:12 PM on Saturday, April 21, 2012,
with ad at top— "Discover New Horizons"
- An April 21 post linking to a 3/12 post (this date being
suggested by the reported time of Colson's death)
that has a review of the film "The Ninth Configuration"
- An April 22 post with six lines that some might
interpret as meeting on a horizon, two lines that might
be interpreted as meeting at a "depth horizon," and
a ninth line that emerges from the other eight
- An April 23 post that combines a passage from
"The Ninth Configuration" with a detail from
the North Face ad that appeared above Wallace's
NY TImes obituary on April 8 and again above
Colson's obituary on April 23
- "The game's…"
See also Knight Moves.
Saturday, October 1, 2011
The Crowe Sphere
From Wallace Stevens's "A Primitive Like an Orb"—
But the virtuoso never leaves his shape,
Still on the horizon elongates his cuts,
And still angelic and still plenteous,
Imposes power by the power of his form.
See also the film Virtuosity and The Crowe Sphere
(a Log24 search that includes, by accident, a post
with the phrase "he crowed exultantly.").
Such a crowing: Cagney's classic "Top of the world!"
Those who seek significance in the name of Crowe's
character in Virtuosity , "SID 6.7," may consult yesterday's
Primordiality and a related post of 6.7 (June 7), 2010.
(For the "SID" part, see Caesar in this journal and Gladiator.)
Thursday, September 8, 2011
Starring the Diamond
"In any geometry satisfying Pappus's Theorem,
the four pairs of opposite points of 83
are joined by four concurrent lines."
— H. S. M. Coxeter (see below)
Continued from Tuesday, Sept. 6—
The Diamond Star

The above is a version of a figure from Configurations and Squares.
Yesterday's post related the the Pappus configuration to this figure.
Coxeter, in "Self-Dual Configurations and Regular Graphs," also relates Pappus to the figure.
Some excerpts from Coxeter—

The relabeling uses the 8 superscripts
from the first picture above (plus 0).
The order of the superscripts is from
an 8-cycle in the Galois field GF(9).
The relabeled configuration is used in a discussion of Pappus—

(Update of Sept. 10, 2011—
Coxeter here has a note referring to page 335 of
G. A. Miller, H. F. Blichfeldt, and L. E. Dickson,
Theory and Applications of Finite Groups , New York, 1916.)
Coxeter later uses the the 3×3 array (with center omitted) again to illustrate the Desargues configuration—

The Desargues configuration is discussed by Gian-Carlo Rota on pp. 145-146 of Indiscrete Thoughts—
"The value of Desargues' theorem and the reason why the statement of this theorem has survived through the centuries, while other equally striking geometrical theorems have been forgotten, is in the realization that Desargues' theorem opened a horizon of possibilities that relate geometry and algebra in unexpected ways."
Friday, July 22, 2011
The Aristophanic View
"Some mathematicians are birds, others are frogs.
Birds fly high in the air and survey broad vistas of
mathematics out to the far horizon. They delight in
concepts that unify our thinking and bring together
diverse problems from different parts of the
landscape. Frogs live in the mud below and see
only the flowers that grow nearby. They delight in
the details of particular objects, and they solve
problems one at a time. I happen to be a frog, but
many of my best friends are birds. The main theme
of my talk tonight is this. Mathematics needs both
birds and frogs. Mathematics is rich and beautiful
because birds give it broad visions and frogs give it
intricate details. Mathematics is both great art and
important science, because it combines generality
of concepts with depth of structures. It is stupid to
claim that birds are better than frogs because they
see farther, or that frogs are better than birds
because they see deeper. The world of mathematics
is both broad and deep, and we need birds and
frogs working together to explore it.
This talk is called the Einstein lecture…."
— Freeman Dyson, Notices of the American
Mathematical Society , February 2009
The Didion reading was suggested by the "6212" in yesterday evening's New York Lottery.
Monday, May 2, 2011
The Vine*
See "Nine is a Vine" and "Hereafter" in this journal.
As quoted here last October 23—
Margaret Atwood on Lewis Hyde's Trickster Makes This World: Mischief, Myth, and Art—
"Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159)
What is "the next world"? It might be the Underworld….
The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart.
Tuesday, March 29, 2011
Diamond Star
From last night's note on finite geometry—
"The (83, 83) Möbius-Kantor configuration here described by Coxeter is of course part of the larger (94, 123) Hesse configuration. Simply add the center point of the 3×3 Galois affine plane and the four lines (1 horizontal, 1 vertical, 2 diagonal) through the center point." An illustration—

This suggests a search for "diamond+star."
Friday, January 14, 2011
Ironic Butterfly
David Brooks's column today quotes Niebuhr. From the same source—
Reinhold Niebuhr, The Irony of American History—
Chapter 8: The Significance of Irony
Any interpretation of historical patterns and configurations raises the question whether the patterns, which the observer discerns, are "objectively" true or are imposed upon the vast stuff of history by his imagination. History might be likened to the confusion of spots on the cards used by psychiatrists in a Rorschach test. The patient is asked to report what he sees in these spots; and he may claim to find the outlines of an elephant, butterfly or frog. The psychiatrist draws conclusions from these judgments about the state of the patient’s imagination rather than about the actual configuration of spots on the card. Are historical patterns equally subjective?
….
The Biblical view of human nature and destiny moves within the framework of irony with remarkable consistency. Adam and Eve are expelled from the Garden of Eden because the first pair allowed "the serpent" to insinuate that, if only they would defy the limits which God had set even for his most unique creature, man, they would be like God. All subsequent human actions are infected with a pretentious denial of human limits. But the actions of those who are particularly wise or mighty or righteous fall under special condemnation. The builders of the Tower of Babel are scattered by a confusion of tongues because they sought to build a tower which would reach into the heavens.
Niebuhr's ironic butterfly may be seen in the context of last
Tuesday's post Shining and of last Saturday's noon post True Grid—

The "butterfly" in the above picture is a diagram showing the 12 lines* of the Hesse configuration from True Grid.
It is also a reference to James Hillman's classical image (see Shining) of the psyche, or soul, as a butterfly.
Fanciful, yes, but this is in exact accordance with Hillman's remarks on the soul (as opposed to the spirit— see Tuesday evening's post).
The 12-line butterfly figure may be viewed as related to the discussions of archetypes and universals in Hillman's Re-Visioning Psychology and in Charles Williams's The Place of the Lion . It is a figure intended here to suggest philosophy, not entertainment.
Niebuhr and Williams, if not the more secular Hillman, might agree that those who value entertainment above all else may look forward to a future in Hell (or, if they are lucky, Purgatory). Perhaps such a future might include a medley of Bob Lind's "Elusive Butterfly" and Iron Butterfly's "In-a-Gadda-da-Vida."
* Three horizontal, three vertical, two diagonal, and four arc-shaped.
Saturday, October 23, 2010
Paranormal Jackass
The follow-up to last year's runaway horror hit, "Paranormal Activity 2," kicked off its first weekend in theaters with a major haul. The creepy tale… pulled in $20.1 million on Friday.
Trailing behind "Paranormal" is last week's box-office busting debut "Jackass 3D. " The prank-fest, which landed about $50 million its first weekend in theaters, slipped to the second-place slot….
…
The Clint Eastwood-helmed ensemble drama "Hereafter" landed in fourth place. Exploring the lives of three people who are dealing with death and the afterlife in several ways, including the story of a psychic played by Matt Damon, the screen legend's latest turn in the director's chair made approximately $4.1 million on Friday.
Related material—
Margaret Atwood on Lewis Hyde's Trickster Makes This World: Mischief, Myth, and Art—
"Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159)
What is "the next world"? It might be the Underworld….
The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart.
George P. Hansen on Martin Gardner and the paranormal.
Saturday, October 16, 2010
The Mandelbrot Numbers
Benoît Mandelbrot died on Oct. 14.

— New York Lottery on Thursday, Oct. 14, 2010
Related material on 109: See 1/09, 2009.
Related material on 060: See Hexagram 60 of the I Ching and…
Margaret Atwood on Lewis Hyde's Trickster Makes This World: Mischief, Myth, and Art—
"Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159)
What is "the next world"? It might be the Underworld….
The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning "to join," "to fit," and "to make." (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart.
Friday, October 15, 2010
Mathematics and Narrative, continued
The Story of N

Roberta Smith in the New York Times of July 7, 2006—
Art Review
Endgame Art? It's Borrow, Sample and Multiply in an Exhibition at Bard College
"… The show has an endgame, end-time mood, as if we are looking at the end of the end of the end of Pop, hyperrealism and appropriation art. The techniques of replication and copying have become so meticulous that they are beside the point. This is truly magic realism: the kind you can't see, that has to be explained. It is also a time when artists cultivate hybridism and multiplicity and disdain stylistic coherence, in keeping with the fashionable interest in collectivity, lack of ego, the fluidity of individual identity. But too often these avoidance tactics eliminate the thread of a personal sensibility or focus.
I would call all these strategies fear of form, which can be parsed as fear of materials, of working with the hands in an overt way and of originality. Most of all originality. Can we just say it? This far from Andy Warhol and Duchamp, the dismissal of originality is perhaps the oldest ploy in the postmodern playbook. To call yourself an artist at all is by definition to announce a faith, however unacknowledged, in some form of originality, first for yourself, second, perhaps, for the rest of us.
Fear of form above all means fear of compression— of an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible. With a few exceptions, forms of collage and assemblage dominate this show: the putting together (or simply putting side by side) of existing images and objects prevails. The consistency of this technique in two and three dimensions should have been a red flag for the curators. Collage has driven much art since the late 1970's. Lately, and especially in this exhibition, it often seems to have become so distended and pulled apart that its components have become virtually autonomous and unrelated, which brings us back to square one. This is most obvious in the large installations of graphic works whose individual parts gain impact and meaning from juxtaposition but are in fact considered distinct artworks."
Margaret Atwood on art and the trickster—
"The pleasures of fabulation, the charming and playful lie— this line of thought leads Hyde* to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning 'to join,' 'to fit,' and 'to make.' If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart."
* Lewis Hyde, Trickster Makes This World: Mischief, Myth, and Art, Farrar Straus & Giroux, January 1998
Smith mentions "an artistic focus that condenses experiences, ideas and feelings into something whole, committed and visually comprehensible."
Atwood mentions "a seamless whole."
For some related remarks, see "A Study in Art Education" and the central figure pictured above. (There "N" can stand for "number," "nine," or "narrative.")
Tuesday, July 20, 2010
The Corpse Express
See Malcolm Lowry's "A corpse will be transported by express!" in this journal.
"When Plato regards geometry as the prerequisite to
philosophical knowledge, it is because geometry alone
renders accessible the realm of things eternal;
tou gar aei ontos he geometrike gnosis estin."
— Ernst Cassirer, Philosophy and Phenomenological Research,
Volume V, Number 1, September, 1944.
Maybe.
June 23, Midsummer Eve, was the date of death for Colonel Michael Cobb.
Cobb, who died aged 93, was "a regular Army officer who in retirement produced
the definitive historical atlas of the railways of Great Britain." — Telegraph.co.uk, July 19
As for geometry, railways, and things eternal, see parallel lines converging
in Tequila Mockingbird and Bedlam Songs.

The Rock Island Line’s namesake depot
in Rock Island, Illinois
See also Wallace Stevens on "the giant of nothingness"
in "A Primitive Like an Orb" and in Midsummer Eve's Dream—
At the center on the horizon, concentrum, grave
And prodigious person, patron of origins.
Thursday, June 24, 2010
Midsummer Noon
Geometry Simplified

(a projective space)
The above finite projective space
is the simplest nontrivial example
of a Galois geometry (i.e., a finite
geometry with coordinates in a
finite (that is, Galois) field.)
The vertical (Euclidean) line represents a
(Galois) point, as does the horizontal line
and also the vertical-and-horizontal
cross that represents the first two points'
binary sum (i.e., symmetric difference,
if the lines are regarded as sets).
Homogeneous coordinates for the
points of this line —
(1,0), (0,1), (1,1).
Here 0 and 1 stand for the elements
of the two-element Galois field GF(2).
The 3-point line is the projective space
corresponding to the affine space
(a plane, not a line) with four points —

(an affine space)
The (Galois) points of this affine plane are
not the single and combined (Euclidean)
line segments that play the role of
points in the 3-point projective line,
but rather the four subsquares
that the line segments separate.
For further details, see Galois Geometry.
There are, of course, also the trivial
two-point affine space and the corresponding
trivial one-point projective space —

Here again, the points of the affine space are
represented by squares, and the point of the
projective space is represented by a line segment
separating the affine-space squares.
Thursday, June 3, 2010
Trickster
Margaret Atwood (pdf) on Lewis Hyde’s
Trickster Makes This World: Mischief, Myth, and Art –
“Trickster,” says Hyde, “feels no anxiety when he deceives…. He… can tell his lies with creative abandon, charm, playfulness, and by that affirm the pleasures of fabulation.” (71) As Hyde says, “… almost everything that can be said about psychopaths can also be said about tricksters,” (158), although the reverse is not the case. “Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists.” (159)
What is “the next world”? It might be the Underworld….
The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning to join, to fit, and to make. (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart.
See also George P. Hansen on Martin Gardner, Trickster.
Tuesday, March 3, 2009
Tuesday March 3, 2009
there is a kind of cross.”
— Thomas Pynchon in
Gravity’s Rainbow
This entry is continued
from yesterday evening,
from midnight last night,
and from an entry of
February 20 (the date
four years ago of
Hunter Thompson’s death)–
“Emblematizing the Modern“–
|
Emblematizing the Modern
Note that in applications, the vertical axis of the Cross of Descartes often symbolizes the timeless (money, temperature, etc.) while the horizontal axis often symbolizes time. T.S. Eliot:
|
The best villains are the ones who are
both protagonist and antagonist.”
— The late Robert Quarry
“Selah.”
— The late Hunter Thompson

Yesterday afternoon’s online
New York Times:

Today’s online New York Times:
Footnote

Friday, February 20, 2009
Friday February 20, 2009
the Modern
|
The following meditation was inspired by the recent fictional recovery, by Mira Sorvino in "The Last Templar," of a Greek Cross — "the Cross of Constantine"– and by the discovery, by art historian Rosalind Krauss, of a Greek Cross in the art of Ad Reinhardt. |

|
Note that in applications, the vertical axis of the Cross of Descartes often symbolizes the timeless (money, temperature, etc.) while the horizontal axis often symbolizes time. T.S. Eliot:
"Men’s curiosity searches past and future |
There is a reason, apart from her ethnic origins, that Rosalind Krauss (cf. 9/13/06) rejects, with a shudder, the cross as a key to "the Pandora's box of spiritual reference that is opened once one uses it." The rejection occurs in the context of her attempt to establish not the cross, but the grid, as a religious symbol:
|
"In suggesting that the success [1] of the grid
[1] Success here refers to
three things at once: a sheerly quantitative success, involving the number of artists in this century who have used grids; a qualitative success through which the grid has become the medium for some of the greatest works of modernism; and an ideological success, in that the grid is able– in a work of whatever quality– to emblematize the Modern." — Rosalind Krauss, "Grids" (1979) |
Related material:
Time Fold and Weyl on
objectivity and frames of reference.
See also Stambaugh on
The Formless Self
as well as
A Study in Art Education
and
Jung and the Imago Dei.
Monday, December 1, 2008
Monday December 1, 2008
From Braque's birthday, 2006:
"The senses deform, the mind forms. Work to perfect the mind. There is no certitude but in what the mind conceives."
— Georges Braque,
Reflections on Painting, 1917
Those who wish to follow Braque's advice may try the following exercise from a book first published in 1937:
construction of a tesseract:

From a page by Bryan Clair
For a different view
of the square and cube
see yesterday's entry
Abstraction and Faith.
Sunday, November 16, 2008
Sunday November 16, 2008
Observations suggested by an article on author Lewis Hyde– "What is Art For?"– in today's New York Times Magazine:
Margaret Atwood (pdf) on Lewis Hyde's
Trickster Makes This World: Mischief, Myth, and Art —
"Trickster," says Hyde, "feels no anxiety when he deceives…. He… can tell his lies with creative abandon, charm, playfulness, and by that affirm the pleasures of fabulation." (71) As Hyde says, "… almost everything that can be said about psychopaths can also be said about tricksters," (158), although the reverse is not the case. "Trickster is among other things the gatekeeper who opens the door into the next world; those who mistake him for a psychopath never even know such a door exists." (159)
What is "the next world"? It might be the Underworld….
The pleasures of fabulation, the charming and playful lie– this line of thought leads Hyde to the last link in his subtitle, the connection of the trickster to art. Hyde reminds us that the wall between the artist and that American favourite son, the con-artist, can be a thin one indeed; that craft and crafty rub shoulders; and that the words artifice, artifact, articulation and art all come from the same ancient root, a word meaning to join, to fit, and to make. (254) If it’s a seamless whole you want, pray to Apollo, who sets the limits within which such a work can exist. Tricksters, however, stand where the door swings open on its hinges and the horizon expands: they operate where things are joined together, and thus can also come apart.
The Trickster
and the Paranormal
and
Martin Gardner on
a disappearing cube —
|
"What happened to that… cube?"
Apollinax laughed until his eyes teared. "I'll give you a hint, my dear. Perhaps it slid off into a higher dimension." "Are you pulling my leg?" "I wish I were," he sighed. "The fourth dimension, as you know, is an extension along a fourth coordinate perpendicular to the three coordinates of three-dimensional space. Now consider a cube. It has four main diagonals, each running from one corner through the cube's center to the opposite corner. Because of the cube's symmetry, each diagonal is clearly at right angles to the other three. So why shouldn't a cube, if it feels like it, slide along a fourth coordinate?" — "Mr. Apollinax Visits New York," by Martin Gardner, Scientific American, May 1961, reprinted in The Night is Large |
For such a cube, see

ashevillecreative.com
this illustration in
The Religion of Cubism
(and the four entries
preceding it —
Log24, May 9, 2003).
Beware of Gardner's
"clearly" and other lies.
Thursday, August 28, 2008
Thursday August 28, 2008
for the writer
known as UD
|
"Have liberty not as the air within a grave Or down a well. Breathe freedom, oh, my native, In the space of horizons that neither love nor hate."
— Wallace Stevens, |
A related visual
association of ideas —
("The association is the idea"
— Ian Lee, The Third Word War)

|
by John Braheny
"Hook" is the term you'll hear most often in the business and craft of commercial songwriting. (Well, maybe not as much as "Sorry, we can't use your song," but it's possible that the more you hear about hooks now, the less you'll hear "we can't use it" later.) |
See also UD's recent
A Must-Read and In My Day*
as well as the five
Log24 entries ending
Sept. 20, 2002.
* Hey Hey
Tuesday, April 8, 2008
Tuesday April 8, 2008
Part I:
Part II:

This figure is related to
the mathematics of
reflection groups.
Part III:
— George Steiner in Grammars of Creation
Inverse Canon —
From Werner Icking Music Archive:
Bach, Fourteen Canons
on the First Eight Notes
of the Goldberg Ground,
No. 11 —
At a different site —
an mp3 of the 14 canons.
That Crown of Thorns,
by Timothy A. Smith

Wednesday, October 24, 2007
Wednesday October 24, 2007
An earlier entry today (“Hollywood Midrash continued“) on a father and son suggests we might look for an appropriate holy ghost. In that context…

A search for further background on Emmanuel Levinas, a favorite philosopher of the late R. B. Kitaj (previous two entries), led (somewhat indirectly) to the following figures of Descartes:

Compare and contrast:
The harmonic-analysis analogy suggests a review of an earlier entry’s
link today to 4/30– Structure and Logic— as well as
re-examination of Symmetry and a Trinity

(Dec. 4, 2002).
See also —
A Four-Color Theorem,
The Diamond Theorem, and
The Most Violent Poem,

Thursday, August 9, 2007
Thursday August 9, 2007
Bulletin of the American Mathematical Society,
Volume 31, Number 1, July 1994, Pages 1-14
Selberg’s Conjectures
and Artin L-Functions (pdf)
M. Ram Murty
Introduction
In its comprehensive form, an identity between an automorphic L-function and a “motivic” L-function is called a reciprocity law. The celebrated Artin reciprocity law is perhaps the fundamental example. The conjecture of Shimura-Taniyama that every elliptic curve over Q is “modular” is certainly the most intriguing reciprocity conjecture of our time. The “Himalayan peaks” that hold the secrets of these nonabelian reciprocity laws challenge humanity, and, with the visionary Langlands program, we have mapped out before us one means of ascent to those lofty peaks. The recent work of Wiles suggests that an important case (the semistable case) of the Shimura-Taniyama conjecture is on the horizon and perhaps this is another means of ascent. In either case, a long journey is predicted…. At the 1989 Amalfi meeting, Selberg [S] announced a series of conjectures which looks like another approach to the summit. Alas, neither path seems the easier climb….
[S] A. Selberg, Old and new
conjectures and results
about a class of Dirichlet series,
Collected Papers, Volume II,
Springer-Verlag, 1991, pp. 47-63.
Zentralblatt MATH Database on the above Selberg paper:
“These are notes of lectures presented at the Amalfi Conference on Number Theory, 1989…. There are various stimulating conjectures (which are related to several other conjectures like the Sato-Tate conjecture, Langlands conjectures, Riemann conjecture…)…. Concluding remark of the author: ‘A more complete account with proofs is under preparation and will in time appear elsewhere.'”
Related material: Previous entry.
Monday, July 2, 2007
Monday July 2, 2007
A figure like Ecclesiast/
Rugged and luminous,
chants in the dark/
A text that is an answer,
although obscure.
— Wallace Stevens,
"An Ordinary Evening
in New Haven"
|
Time and Chance |
From 8/02
in 2005:
| 50 Years Ago on this date, poet Wallace Stevens died. Memorial: at the Wallace Stevens Concordance, enter center. |
Result:
| The Man with the Blue Guitar | |
|---|---|
| line 150 (xiii.6): | The heraldic center of the world |
| Human Arrangement | |
| line 13: | The center of transformations that |
| This Solitude of Cataracts | |
| line 18: | Breathing his bronzen breath at the azury center of time. |
| A Primitive Like an Orb | |
| line 1 (i.1): | The essential poem at the center of things, |
| line 87 (xi.7): | At the center on the horizon, concentrum, grave |
| Reply to Papini | |
| line 33 (ii.15): | And final. This is the center. The poet is |
| Study of Images II | |
| line 7: | As if the center of images had its |
| An Ordinary Evening in New Haven | |
| line 291 (xvii.3): | It fails. The strength at the center is serious. |
| line 371 (xxi.11): | At the center, the object of the will, this place, |
| Things of August | |
| line 154 (ix.18): | At the center of the unintelligible, |
| The Hermitage at the Center | |
| Title: | The Hermitage at the Center |
| Owl's Clover, The Old Woman and the Statue (OP) | |
| line 13 (ii.9): | At the center of the mass, the haunches low, |
| The Sail of Ulysses (OP) | |
| line 50 (iv.6): | The center of the self, the self |
| Someone Puts a Pineapple Together (NA) | |
| line 6 (i.6): | The angel at the center of this rind, |
| Of Ideal Time and Choice (NA) | |
| line 29: | At last, the center of resemblance, found |
| line 32: | Stand at the center of ideal time, |
For a text on today's
mid-day number, see
Friday, June 15, 2007
Friday June 15, 2007
Art Education
Rudolf Arnheim, a student of Gestalt psychology (which, an obituary notes, emphasizes "the perception of forms as organized wholes") was the first Professor of the Psychology of Art at Harvard. He died at 102 on Saturday, June 9, 2007.
The conclusion of yesterday's New York Times obituary of Arnheim:
"… in The New York Times Book Review in 1986, Celia McGee called Professor Arnheim 'the best kind of romantic,' adding, 'His wisdom, his patient explanations and lyrical enthusiasm are those of a teacher.'"
A related quotation:
"And you are teaching them a thing or two about yourself. They are learning that you are the living embodiment of two timeless characterizations of a teacher: 'I say what I mean, and I mean what I say' and 'We are going to keep doing this until we get it right.'"
Here, yet again, is an illustration that has often appeared in Log24– notably, on the date of Arnheim's death:

Related quotations:
"We have had a gutful of fast art and fast food. What we need more of is slow art: art that holds time as a vase holds water: art that grows out of modes of perception and whose skill and doggedness make you think and feel; art that isn't merely sensational, that doesn't get its message across in 10 seconds, that isn't falsely iconic, that hooks onto something deep-running in our natures. In a word, art that is the very opposite of mass media. For no spiritually authentic art can beat mass media at their own game."
— Robert Hughes, speech of June 2, 2004
"Whether the 3×3 square grid is fast art or slow art, truly or falsely iconic, perhaps depends upon the eye of the beholder."
If the beholder is Rudolf Arnheim, whom we may now suppose to be viewing the above figure in the afterlife, the 3×3 square is apparently slow art. Consider the following review of his 1982 book The Power of the Center:
"Arnheim deals with the significance of two kinds of visual organization, the concentric arrangement (as exemplified in a bull's-eye target) and the grid (as exemplified in a Cartesian coordinate system)….
It is proposed that the two structures of grid and target are the symbolic vehicles par excellence for two metaphysical/psychological stances. The concentric configuration is the visual/structural equivalent of an egocentric view of the world. The self is the center, and all distances exist in relation to the focal spectator. The concentric arrangement is a hermetic, impregnable pattern suited to conveying the idea of unity and other-worldly completeness. By contrast, the grid structure has no clear center, and suggests an infinite, featureless extension…. Taking these two ideal types of structural scaffold and their symbolic potential (cosmic, egocentric vs. terrestrial, uncentered) as given, Arnheim reveals how their underlying presence organizes works of art."
— Review of Rudolf Arnheim's The Power of the Center: A Study of Composition in the Visual Arts (Univ. of Calif. Press, 1982). Review by David A. Pariser, Studies in Art Education, Vol. 24, No. 3 (1983), pp. 210-213
Arnheim himself says in this book (pp. viii-ix) that "With all its virtues, the framework of verticals and horizontals has one grave defect. It has no center, and therefore it has no way of defining any particular location. Taken by itself, it is an endless expanse in which no one place can be distinguished from the next. This renders it incomplete for any mathematical, scientific, and artistic purpose. For his geometrical analysis, Descartes had to impose a center, the point where a pair of coordinates [sic] crossed. In doing so he borrowed from the other spatial system, the centric and cosmic one."
Students of art theory should, having read the above passages, discuss in what way the 3×3 square embodies both "ideal types of structural scaffold and their symbolic potential."
We may imagine such a discussion in an afterlife art class– in, perhaps, Purgatory rather than Heaven– that now includes Arnheim as well as Ernst Gombrich and Kirk Varnedoe.
Such a class would be one prerequisite for a more advanced course– Finite geometry of the square and cube.
Wednesday, September 13, 2006
Wednesday September 13, 2006
The Krauss Cross
"If we open any tract– Plastic Art and Pure Plastic Art or The Non-Objective World, for instance– we will find that Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal, and they are not interested in what happens below in the Concrete.
Or, to take a more up-to-date example, we could think about Ad Reinhardt who, despite his repeated insistence that 'Art is art,' ended up by painting a series of black nine-square grids in which the motif that inescapably emerges is a Greek cross. There is no painter in the West who can be unaware of the symbolic power of the cruciform shape and the Pandora's box of spiritual reference that is opened once one uses it."
Rebecca Goldstein on
Mathematics and Narrative:
"I don't write exclusively on Jewish themes or about Jewish characters. My collection of short stories, Strange Attractors, contained nine pieces, five of which were, to some degree, Jewish, and this ratio has provided me with a precise mathematical answer (for me, still the best kind of answer) to the question of whether I am a Jewish writer. I am five-ninths a Jewish writer."
Jacques Maritain,
October 1941:
"The passion of Israel
today is taking on
more and more distinctly
the form of the Cross."
E. L. Doctorow,
City of God:
"In the garden of Adding,
Live Even and Odd."
Wednesday September 13, 2006
Octobers for Fest
In memory of Joachim Fest, a noted biographer of Hitler who died on 9/11 at age 79–
A link from 5/27, 2005 (a date mentioned in Monday's Log24 9/11 entry):
A search on this inelegant phrase from Sartre's Being and Nothingness leads, surprisingly, to remarks by the Catholic philosopher Jacques Maritain said to have been published in the month of October in the fateful year 1941.
According to Telegraph.co.uk today, Fest was "the most celebrated historian and the most distinguished journalist of the post-war generation in Germany."
The Telegraph says he
"aroused the envy of professorial rivals, none of whom could match the incisive elegance of his writing. Equally important was his flair for controversy. He was determined to prevent the wrong lessons being drawn from the past by the Left-wing establishment that had dominated German intellectual life since the 1960s.
Conservative in politics and Catholic by upbringing, Fest stood out among his contemporaries for his rejection of the influence of the Marxist sociologists of the Frankfurt school on the historiography of the Third Reich. Fest saw the Nazi phenomenon not as a product of capitalism, but as a moral catastrophe, made possible by the abdication of responsibility on the part of educated Germans."
For a view of Christian politics closer to that of the Frankfurt school, see a review by Charles Isherwood in the 9/11 New York Times of a play, "The Man Himself."
Related material:
A Log24 entry
from October 29, 2002:
|
Our Judeo-Christian Heritage: Two Sides of the Same Coin
|
and Echoes
(August 11, 2006).
Friday, March 31, 2006
Friday March 31, 2006
Women's History Month continues…
Ontology Alignment
"He had with him a small red book of Mao's poems, and as he talked he squared it on the table, aligned it with the table edge first vertically and then horizontally. To understand who Michael Laski is you must have a feeling for that kind of compulsion."
— Joan Didion in the
Saturday Evening Post,
Nov. 18, 1967 (reprinted in
Slouching Towards Bethlehem)
"Or were you," I said.
He said nothing.
"Raised a Catholic," I said.
He aligned a square crystal paperweight with the edge of his desk blotter.
— Joan Didion in
The Last Thing He Wanted,
Knopf, 1996
"It was Plato who best expressed– who veritably embodied– the tension between the narrative arts and mathematics….
Plato clearly loved them both, both mathematics and poetry. But he approved of mathematics, and heartily, if conflictedly, disapproved of poetry. Engraved above the entrance to his Academy, the first European university, was the admonition: Oudeis ageometretos eiseto. Let none ignorant of geometry enter. This is an expression of high approval indeed, and the symbolism could not have been more perfect, since mathematics was, for Plato, the very gateway for all future knowledge. Mathematics ushers one into the realm of abstraction and universality, grasped only through pure reason. Mathematics is the threshold we cross to pass into the ideal, the truly real."
— Rebecca Goldstein,
Mathematics and
the Character of Tragedy
Saturday, March 11, 2006
Saturday March 11, 2006
|
Holy the Firm
by Annie Dillard Esoteric Christianity, I read, posits a substance. It is a created substance, lower than metals and minerals on a “spiritual scale” and lower than salts and earths, occurring beneath salts and earths in the waxy deepness of planets, but never on the surface of planets where men could discern it; and it is in touch with the Absolute, at base. In touch with the Absolute! At base. The name of this substance is Holy the Firm. These are only ideas, by the single handful. Lines, lines, and their infinite points! Hold hands and crack the whip, and yank the Absolute out of there and into the light, God pale and astounded, spraying a spiral of salts and earths, God footloose and flung. And cry down the line to his passing white ear, “Old Sir! Do you hold space from buckling by a finger in its hole? O Old! Where is your other hand?” His right hand is clenching, calm, round the exploding left hand of Holy the Firm. — Annie Dillard, Holy the Firm, Harper & Row 1977, reissued by Harper Perennial Library in 1988 as a paperback, pp. 68-71. |
Tuesday, July 5, 2005
Tuesday July 5, 2005
For Christopher Fry
and the White Goddess:
The Edge of Eternity
Christian humanist playwright Christopher Fry, author of The Lady’s Not for Burning, died at 97 on June 30, 2005.
From Log24 on June 30:
Robert Graves, author of
The White Goddess:
A Historical Grammar of Poetic Myth—
How may the King hold back?
Royally then he barters life for love.
Or of the undying snake from chaos hatched,
Whose coils contain the ocean,
Into whose chops with naked sword he springs,
Then in black water, tangled by the reeds,
Battles three days and nights…
From Cold Mountain:
“He sat awhile on a rock, and then got up and walked all morning through the dim woods. The track was ill used, so coiled and knotted he could not say what its general tendency was. It aimed nowhere certain but up. The brush and bracken grew thick in the footway, and the ground seemed to be healing over, so that in some near future the way would not even remain as scar. For several miles it mostly wound its way through a forest of immense hemlocks, and the fog lay among them so thick that their green boughs were hidden. Only the black trunks were visible, rising into the low sky like old menhirs stood up by a forgotten race to memorialize the darkest events of their history….
They climbed to a bend and from there they walked on great slabs of rock. It seemed to Inman that they were at the lip of a cliff, for the smell of the thin air spoke of considerable height, though the fog closed off all visual check of loftiness….
Then he looked back down and felt a rush of vertigo as the lower world was suddenly revealed between his boot toes. He was indeed at the lip of a cliff, and he took one step back…. The country around was high, broken. Inman looked about and was startled to see a great knobby mountain forming up out of the fog to the west, looming into the sky. The sun broke through a slot in the clouds, and a great band of Jacob’s ladder suddenly hung in the air like a gauze curtain between Inman and the blue mountain….
Inman looked at the big grandfather mountain and then he looked beyond it to the lesser mountains as they faded off into the southwest horizon, bathed in faint smoky haze. Waves of mountains. For all the evidence the eye told, they were endless. The grey overlapping humps of the farthest peaks distinguished themselves only as slightly darker values of the pale grey air. The shapes and their ghostly appearance spoke to Inman in a way he could not clearly interpret. They graded off like the tapering of pain from the neck wound as it healed.”
See also the entries of July 3.
The crone figure in this section of Cold Mountain is not entirely unrelated to the girl accused of being a witch in Fry’s play and to Graves’s White Goddess.
From Fry’s obituary in The Guardian:
“Though less of a public theorist than Eliot, Fry still believed passionately in the validity of poetic drama. As he wrote in the magazine Adam: ‘In prose, we convey the eccentricity of things, in poetry their concentricity, the sense of relationship between them: a belief that all things express the same identity and are all contained in one discipline of revelation.'”
From Fry’s obituary in today’s New York Times:
“His plays radiated an optimistic faith in God and humanity, evoking, in his words, ‘a world in which we are poised on the edge of eternity, a world which has deeps and shadows of mystery, and God is anything but a sleeping partner.’ He said he wrote his plays in poetry because that was ‘the language in which man expresses his own amazement’ at the complexity both of himself and of a reality which, beneath the surface, was ‘wildly, perilously, inexplicably fantastic.'”
Sunday, July 3, 2005
Sunday July 3, 2005
Intersections
1. Blue Ridge meets Black Mountain,
2. Vertical meets horizontal in music,
3. The timeless meets time in religion.
Details:
1. Blue Ridge, Black Mountain
“Montreat College is located in the beautiful Blue Ridge Mountains of Western North Carolina…. The Black Mountain Campus is… three miles from the main campus in the historic town of Black Mountain.”
Black Mountain College was “established on the Blue Ridge Assembly grounds outside the town of Black Mountain in North Carolina in the fall of 1933.”
USA Today, May 15, 2005, on Billy Graham:
“MONTREAT, N.C. — … It’s here at his… homestead, where the Blue Ridge meets the Black Mountain range east of Asheville, that Graham gave a rare personal interview.”
See also the following from June 24:

“No bridge reaches God, except one…
God’s Bridge: The Cross.”
— Billy Graham Evangelistic Association,
according to messiahpage.com
For some remarks more in the spirit of Black Mountain than of the Blue Ridge, see today’s earlier entry on pianist Grete Sultan and composer Tui St. George Tucker.
2. Vertical, Horizontal in Music
Richard Neuhaus on George Steiner’s
Grammars of Creation:
“… the facts of the world are not and will never be ‘the end of the matter.’ Music joins grammar in pointing to the possibility, the reality, of more. He thinks Schopenhauer was on to something when he said music will continue after the world ends.
‘The capacity of music to operate simultaneously along horizontal and vertical axes, to proceed simultaneously in opposite directions (as in inverse canons), may well constitute the nearest that men and women can come to absolute freedom. Music does “keep time” for itself and for us.'”
3. Timeless, Time
A Trinity Sunday sermon quotes T. S. Eliot:
“… to apprehend
The point of intersection of the timeless
With time, is an occupation for the saint.”
See also The Diamond Project.
A Bridge for Private Ryan

In memory of actor
Harrison Richard Young, 75,
who died on Sunday, July 3, 2005
Tuesday, June 7, 2005
Tuesday June 7, 2005
101 101
writes Dominique de Villepin,
the new French Prime Minister,
“shines like a powder trail
on a day’s horizon.
It sets it ablaze all at once,
explodes all limits,
draws the eyes
to other heavens.”
— Ben Macintyre,
The London Times, June 4:
When Rimbaud Meets Rambo
“Room 101 was the place where
your worst fears were realised
in George Orwell’s classic
Nineteen Eighty-Four.
Professor Nash’s office number
in the movie ‘A Beautiful Mind.'”
Classics Illustrated —

Click on picture for details.
(For some mathematics that is actually
from 1984, see Block Designs
and the 2005 followup
The Eightfold Cube.)
Saturday, June 4, 2005
Saturday June 4, 2005
The 4×4 Square:
French Perspectives
Earendil_Silmarils:

Les Anamorphoses:

"Pour construire un dessin en perspective,
le peintre trace sur sa toile des repères:
la ligne d'horizon (1),
le point de fuite principal (2)
où se rencontre les lignes de fuite (3)
et le point de fuite des diagonales (4)."
_______________________________
Serge Mehl,
Perspective &
Géométrie Projective:
"… la géométrie projective était souvent
synonyme de géométrie supérieure.
Elle s'opposait à la géométrie
euclidienne: élémentaire…
La géométrie projective, certes supérieure
car assez ardue, permet d'établir
de façon élégante des résultats de
la géométrie élémentaire."
Similarly…
Finite projective geometry
(in particular, Galois geometry)
is certainly superior to
the elementary geometry of
quilt-pattern symmetry
and allows us to establish
de façon élégante
some results of that
elementary geometry.
Other Related Material…
from algebra rather than
geometry, and from a German
rather than from the French:
"This is the relativity problem:
to fix objectively a class of
equivalent coordinatizations
and to ascertain
the group of transformations S
mediating between them."
— Hermann Weyl,
The Classical Groups,
Princeton U. Press, 1946

Evariste Galois
Weyl also says that the profound branch
of mathematics known as Galois theory
relativity theory for the set Sigma,
a set which, by its discrete and
finite character, is conceptually
so much simpler than the
infinite set of points in space
or space-time dealt with
by ordinary relativity theory."
— Weyl, Symmetry,
Princeton U. Press, 1952
Metaphor and Algebra…
"Perhaps every science must
start with metaphor
and end with algebra;
and perhaps without metaphor
there would never have been
any algebra."
Max Black, Models and Metaphors, 1962
For metaphor and
algebra combined, see
in a diamond ring,"
A.M.S. abstract 79T-A37,
Notices of the
American Mathematical Society,
February 1979, pages A-193, 194 —
the original version of the 4×4 case
of the diamond theorem.
More on Max Black…
"When approaching unfamiliar territory, we often, as observed earlier, try to describe or frame the novel situation using metaphors based on relations perceived in a familiar domain, and by using our powers of association, and our ability to exploit the structural similarity, we go on to conjecture new features for consideration, often not noticed at the outset. The metaphor works, according to Max Black, by transferring the associated ideas and implications of the secondary to the primary system, and by selecting, emphasising and suppressing features of the primary in such a way that new slants on it are illuminated."
— Paul Thompson, University College, Oxford,
The Nature and Role of Intuition
in Mathematical Epistemology
That intuition, metaphor (i.e., analogy), and association may lead us astray is well known. The examples of French perspective above show what might happen if someone ignorant of finite geometry were to associate the phrase "4×4 square" with the phrase "projective geometry." The results are ridiculously inappropriate, but at least the second example does, literally, illuminate "new slants"– i.e., diagonals– within the perspective drawing of the 4×4 square.
Similarly, analogy led the ancient Greeks to believe that the diagonal of a square is commensurate with the side… until someone gave them a new slant on the subject.
Saturday June 4, 2005
Drama of the Diagonal,
continued
"I could name other writers
who share this sense of a world
larger than ourselves; their writing provides
a field in which something like
a sacramental imagination is clearly at play."
but that which extends meaning
from what is directly given
to the whole context in which it is given,
including a sense of a world."
"A Primitive Like an Orb":
X
It is a giant, always, that is evolved, To be in scale, unless virtue cuts him, snips Both size and solitude or thinks it does, As in a signed photograph on a mantelpiece. But the virtuoso never leaves his shape, Still on the horizon elongates his cuts, And still angelic and still plenteous, Imposes power by the power of his form.
XI
Here, then, is an abstraction given head, A giant on the horizon, given arms, A massive body and long legs, stretched out, A definition with an illustration, not Too exactly labeled, a large among the smalls Of it, a close, parental magnitude, At the center of the horizon, concentrum, grave And prodigious person, patron of origins.
XII
That's it. The lover writes, the believer hears, The poet mumbles and the painter sees, Each one, his fated eccentricity, As a part, but part, but tenacious particle, Of the skeleton of the ether, the total Of letters, prophecies, perceptions, clods Of color, the giant of nothingness, each one And the giant ever changing, living in change.
Related material
(Click on pictures
for details.)
Logos Alogos
by S. H. Cullinane
Logos Alogos II:
Horizon
See also
Subject and Predicates and
The Quality of Diamond.
Friday, May 27, 2005
Friday May 27, 2005
Part Deux
Wednesday’s entry The Turning discussed a work by Roger Cooke. Cooke presents a
“fanciful story (based on Plato’s dialogue Meno).”
The History of Mathematics is the title of the Cooke book.
Associated Press thought for today:
“History is not, of course, a cookbook offering pretested recipes. It teaches by analogy, not by maxims. It can illuminate the consequences of actions in comparable situations, yet each generation must discover for itself what situations are in fact comparable.”
— Henry Kissinger (whose birthday is today)
a link to Geometry for Jews.
This link suggests a search for material
on the art of Sol LeWitt, which leads to
an article by Barry Cipra,
The “Sol LeWitt” Puzzle:
A Problem in 16 Squares (ps),
a discussion of a 4×4 array
of square linear designs.
Cipra says that
* Jean-Paul Sartre,
Being and Nothingness,
Philosophical Library, 1956
[reference by Cipra]
For another famous group lurking near, if not within, a 4×4 array, click on Kissinger’s birthday link above.
Kissinger’s remark (above) on analogy suggests the following analogy to the previous entry’s (Drama of the Diagonal) figure:

Logos Alogos II:
Horizon
This figure in turn, together with Cipra’s reference to Sartre, suggests the following excerpts (via Amazon.com)–
From Sartre’s Being and Nothingness, translated by Hazel E. Barnes, 1993 Washington Square Press reprint edition:
| 1. | on Page 51: |
| “He makes himself known to himself from the other side of the world and he looks from the horizon toward himself to recover his inner being. Man is ‘a being of distances.'” | |
| 2. | on Page 154: |
| “… impossible, for the for-itself attained by the realization of the Possible will make itself be as for-itself–that is, with another horizon of possibilities. Hence the constant disappointment which accompanies repletion, the famous: ‘Is it only this?’….” | |
| 3. | on Page 155: |
| “… end of the desires. But the possible repletion appears as a non-positional correlate of the non-thetic self-consciousness on the horizon of the glass-in-the-midst-of-the-world.” | |
| 4. | on Page 158: |
| “… it is in time that my possibilities appear on the horizon of the world which they make mine. If, then, human reality is itself apprehended as temporal….” | |
| 5. | on Page 180: |
| “… else time is an illusion and chronology disguises a strictly logical order of deducibility. If the future is pre-outlined on the horizon of the world, this can be only by a being which is its own future; that is, which is to come….” | |
| 6. | on Page 186: |
| “… It appears on the horizon to announce to me what I am from the standpoint of what I shall be.” | |
| 7. | on Page 332: |
| “… the boat or the yacht to be overtaken, and the entire world (spectators, performance, etc.) which is profiled on the horizon. It is on the common ground of this co-existence that the abrupt revelation of my ‘being-unto-death’….” | |
| 8. | on Page 359: |
| “… eyes as objects which manifest the look. The Other can not even be the object aimed at emptily at the horizon of my being for the Other.” | |
| 9. | on Page 392: |
| “… defending and against which he was leaning as against a wail, suddenly opens fan-wise and becomes the foreground, the welcoming horizon toward which he is fleeing for refuge.” | |
| 10. | on Page 502: |
| “… desires her in so far as this sleep appears on the ground of consciousness. Consciousness therefore remains always at the horizon of the desired body; it makes the meaning and the unity of the body.” |
| 11. | on Page 506: |
| “… itself body in order to appropriate the Other’s body apprehended as an organic totality in situation with consciousness on the horizon— what then is the meaning of desire?” | |
| 12. | on Page 661: |
| “I was already outlining an interpretation of his reply; I transported myself already to the four corners of the horizon, ready to return from there to Pierre in order to understand him.” | |
| 13. | on Page 754: |
| “Thus to the extent that I appear to myself as creating objects by the sole relation of appropriation, these objects are myself. The pen and the pipe, the clothing, the desk, the house– are myself. The totality of my possessions reflects the totality of my being. I am what I have. It is I myself which I touch in this cup, in this trinket. This mountain which I climb is myself to the extent that I conquer it; and when I am at its summit, which I have ‘achieved’ at the cost of this same effort, when I attain this magnificent view of the valley and the surrounding peaks, then I am the view; the panorama is myself dilated to the horizon, for it exists only through me, only for me.” |
|
Illustration of the
last horizon remark: |
For more on the horizon, being, and nothingness, see
Friday, April 15, 2005
Friday April 15, 2005
In memory of Leonardo and of Chen Yifei (previous entry), a link to the Sino-Judaic Institute’s review of Chen’s film “Escape to
Saturday, December 27, 2003 10:21 PM
Toy
“If little else, the brain is an educational toy. While it may be a frustrating plaything — one whose finer points recede just when you think you are mastering them — it is nonetheless perpetually fascinating, frequently surprising, occasionally rewarding, and it comes already assembled; you don’t have to put it together on Christmas morning.
The problem with possessing such an engaging toy is that other people want to play with it, too. Sometimes they’d rather play with yours than theirs. Or they object if you play with yours in a different manner from the way they play with theirs. The result is, a few games out of a toy department of possibilities are universally and endlessly repeated. If you don’t play some people’s game, they say that you have ‘lost your marbles,’ not recognizing that,

while Chinese checkers is indeed a fine pastime, a person may also play dominoes, chess, strip poker, tiddlywinks, drop-the-soap or Russian roulette with his brain.
One brain game that is widely, if poorly, played is a gimmick called ‘rational
“I took the number twenty-four and there’s twenty-four ways of expressing the numbers one, two, three, four. And I assigned one kind of line to one, one to two, one to three, and one to four. One was a vertical line, two was a horizontal line, three was diagonal left to right, and four was diagonal right to left. These are the basic kind of directions that lines can take…. the absolute ways that lines can be drawn. And I drew these things as parallel lines very close to one another in boxes. And then there was a system of changing them so that within twenty-four pages there were different arrangements of actually sixteen squares, four sets of four. Everything was based on four. So this was kind of a… more of a… less of a rational… I mean, it gets into the whole idea of methodology.”
Yes, it does.
See Art Wars, Poetry’s Bones, and Time Fold.
Friday, December 26, 2003 7:59 PM
ART WARS, St. Stephen’s Day:
The Magdalene Code
Got The Da Vinci Code for Xmas.
From page 262:
When Langdon had first seen The Little Mermaid, he had actually gasped aloud when he noticed that the painting in Ariel’s underwater home was none other than seventeenth-century artist Georges de la Tour’s The Penitent Magdalene — a famous homage to the banished Mary Magdalene — fitting decor considering the movie turned out to be a ninety-minute collage of blatant symbolic references to the lost sanctity of Isis, Eve, Pisces the fish goddess, and, repeatedly, Mary Magdalene.
Related Log24 material —
The Da Vinci Code, pages 445-446:
“The blade and chalice?” Marie asked. “What exactly do they look like?”
Langdon sensed she was toying with him, but he played along, quickly describing the symbols.
A look of vague recollection crossed her face. “Ah, yes, of course. The blade represents all that is masculine. I believe it is drawn like this, no?” Using her index finger, she traced a shape on her palm.
“Yes,” Langdon said. Marie had drawn the less common “closed” form of the blade, although Langdon had seen the symbol portrayed both ways.
“And the inverse,” she said, drawing again upon her palm, “is the chalice, which represents the feminine.”
“Correct,” Langdon said….
… Marie turned on the lights and pointed….
“There you are, Mr. Langdon. The blade and chalice.”….
“But that’s the Star of Dav–“
Langdon stopped short, mute with amazement as it dawned on him.
The blade and chalice.
Fused as one.
The Star of David… the perfect union of male and female… Solomon’s Seal… marking the Holy of Holies, where the male and female deities — Yahweh and Shekinah — were thought to dwell.
Related Log24 material —
Star Wars.
Concluding remark of April 15, 2005:
For a more serious approach to portraits of
redheads, see Chen Yifei’s work.

Sunday, July 25, 2004
Sunday July 25, 2004
Richard Neuhaus on George Steiner's
Grammars of Creation:
"… the facts of the world are not and will never be 'the end of the matter.' Music joins grammar in pointing to the possibility, the reality, of more. He thinks Schopenhauer was on to something when he said music will continue after the world ends.
'The capacity of music to operate simultaneously along horizontal and vertical axes, to proceed simultaneously in opposite directions (as in inverse canons), may well constitute the nearest that men and women can come to absolute freedom. Music does "keep time" for itself and for us.'"
Wednesday, April 7, 2004
Wednesday April 7, 2004
ART WARS:
Mother of Beauty
In memory of architect Pierre Koenig…
Mother of Beauty: A Note on Modernism.
“… Case Study House #22 … was high drama — one in which the entire city becomes part of the architect’s composition. Approached along a winding street set high in the Hollywood Hills, the house first appears as a blank concrete screen. From here, the visitor steps out onto a concrete deck that overlooks a swimming pool. Just beyond it, the house’s living room — enclosed in a glass-and steel-frame — cantilevers out from the edge of the hill toward the horizon.
The house was immortalized in a now famous image taken by the architectural photographer Julius Shulman. In it, two women, clad in immaculate white cocktail dresses, are perched on the edge of their seats in the glass-enclosed living room, their pose suggesting a kind of sanitized suburban bliss. A night view of the city spreads out beneath them, an endless grid of twinkling lights that perfectly captures the infinite hopes of the postwar American dream….
“My blue dream…”
— F. Scott Fitzgerald
Perhaps no house, in fact, better sums up the mix of outward confidence and psychic unease that defined Cold War America….”
Sunday, February 8, 2004
Sunday February 8, 2004
The Quality of Diamond
On February 3, 2004, archivist and abstract painter Ward Jackson died at 75. From today’s New York Times:
“Inspired by painters like Piet Mondrian and Josef Albers, Mr. Jackson made austere, hard-edged geometric compositions, typically on diamond-shaped canvases.”
|
On a 2003 exhibit by Pablo Helguera that included Mr. Jackson: Parallel Lives recounts and recontextualizes real episodes from the lives of five disparate individuals including Florence Foster Jenkins, arguably the world’s worst opera singer; Giulio Camillo, a Renaissance mystic who aimed to build a memory container for all things; Friedrich Froebel, the inventor of the kindergarten education system, the members of the last existing Shaker community, and Ward Jackson, the lifelong archivist of the Solomon R. Guggenheim Museum. Parallel Lives pays homage to Hans-Georg Gadamer (1900-2002) and his system of philosophical hermeneutics built through an exploration of historicity, language, and art. This exhibition, which draws its title from the classic work by Plutarch, is a project that explores biography as a medium, drawing from the earlier innovation of the biographical practice in works like Marcel Schwob’s “Imaginary Lives” (1896) and John Aubrey’s “Brief Lives” (1681). Through display means, the project blends the lives of these individuals into one basic story, visually stating the relationship between individualism and society as best summarized by Gadamer’s famous phrase: “we all are others, and we all are a self.” |
On February 3, the day that Jackson died, there were five different log24.net entries:
Parallels with the Helguera exhibit:
Florence Foster Jenkins: Janet Jackson in (2) above.
Giulio Camillo: Myself as compiler of the synchronistic excerpts in (5).
Friedrich Froebel: David Wade in (4).
The last Shakers: Christopher Alexander and his acolytes in (1).
Ward Jackson: On Feb. 3, Jackson became a permanent part of
Some thoughts of Hans-Georg Gadamer
relevant to Jackson’s death:
|
by G.T. Karnezis The pleasure it [art] elicits “is the joy of knowledge.” It does not operate as an enchantment but “a transformation into the true.” Art, then, would seem to be an essentializing agent insofar as it reveals what is essential. Gadamer asks us to see reality as a horizon of “still undecided possibilities,” of unfulfilled expectations, of contingency. If, in a particular case, however, “a meaningful whole completes and fulfills itself in reality,” it is like a drama. If someone sees the whole of reality as a closed circle of meaning” he will be able to speak “of the comedy and tragedy of life” (genres becoming ways of conceiving reality). In such cases where reality “is understood as a play, there emerges the reality of what play is, which we call the play of art.” As such, art is a realization: “By means of it everyone recognizes that that is how things are.” Reality, in this viewpoint, is what has not been transformed. Art is defined as “the raising up of this reality to its truth.” |
As noted in entry (3) above
on the day that Jackson died,
“All the world’s a stage.”
Saturday, December 27, 2003
Saturday December 27, 2003
Toy
“If little else, the brain is an educational toy. While it may be a frustrating plaything — one whose finer points recede just when you think you are mastering them — it is nonetheless perpetually fascinating, frequently surprising, occasionally rewarding, and it comes already assembled; you don’t have to put it together on Christmas morning.
The problem with possessing such an engaging toy is that other people want to play with it, too. Sometimes they’d rather play with yours than theirs. Or they object if you play with yours in a different manner from the way they play with theirs. The result is, a few games out of a toy department of possibilities are universally and endlessly repeated. If you don’t play some people’s game, they say that you have ‘lost your marbles,’ not recognizing that,

while Chinese checkers is indeed a fine pastime, a person may also play dominoes, chess, strip poker, tiddlywinks, drop-the-soap or Russian roulette with his brain.
One brain game that is widely, if poorly, played is a gimmick called ‘rational
“I took the number twenty-four and there’s twenty-four ways of expressing the numbers one, two, three, four. And I assigned one kind of line to one, one to two, one to three, and one to four. One was a vertical line, two was a horizontal line, three was diagonal left to right, and four was diagonal right to left. These are the basic kind of directions that lines can take…. the absolute ways that lines can be drawn. And I drew these things as parallel lines very close to one another in boxes. And then there was a system of changing them so that within twenty-four pages there were different arrangements of actually sixteen squares, four sets of four. Everything was based on four. So this was kind of a… more of a… less of a rational… I mean, it gets into the whole idea of methodology.”
Yes, it does.
See Art Wars, Poetry’s Bones, and Time Fold.
Wednesday, January 1, 2003
Wednesday January 1, 2003
|
ART WARS: That Old Devil Moon |
|
From The New York Times, Wed., Jan. 1, 2003:
Richard Horner, 82,
Broadway Producer, Is DeadRichard Horner, a Broadway theater owner and producer who won a Tony Award for the 1974 revival of Eugene O'Neill's "Moon for the Misbegotten," died on Saturday [December 28, 2002] at his home in Palm Springs, Calif. He was 82.
According to one source, the O'Neill revival opened on December 28, 1973 — the same date on which the life of one of its producers was later to close.
From a CurtainUp review:
The revival at the Morosco was dubbed by its company "The Resurrection Play" since Jason Robards undertook the part just after a near fatal car accident and its legendary director José Quintero had just given up drinking.
According to the Internet Broadway Database, this revival, or resurrection, took place officially not on December 28 — the date of Horner's death — but, appropriately, a day later.
At any rate, O'Neill's title, along with my weblog entry of December 28, 2002,
"On This Date," featuring Kylie Minogue,
suggests the following mini-exhibit of artistic efforts:
|
Curtain Up!
July 2000
|

|
|
Under the Volcano: A painting based on Malcolm Lowry's classic novel. Having played tennis, Dr. Vigil and M. Laruelle talk about the events a year earlier. The view is of Cuernavaca from the Casino de la Selva hotel.
Painting by
|

|
For further details on Kylie, Mexico, tequila, and
Under the Volcano,
see my entry of November 5, 2002.
For today's site music, click "Old Devil Moon" here.
|
Addendum of 9:30 pm 1/1/03:
For a politically correct view
For a more perceptive analysis,
If there is a devil here,
J. D. Salinger (Nine Stories),
Frazer might appreciate the remarks in
Not amused: Charlotte Raven
Raven, take a bough.
|
Sunday, July 28, 2002
Sunday July 28, 2002
Memories, Dreams, Reflections
Saul Steinberg in The New York Review of Books issue dated August 15, 2002, page 32:
“The idea of reflections came to me in reading an observation by Pascal, cited in a book by W. H. Auden, who wrote an unusual kind of autobiography by collecting all the quotations he had annotated in the course of his life, which is a good way of displaying oneself, as a reflection of these quotations. Among them this observation by Pascal, which could have been made only by a mathematician….”
Pascal’s observation is that humans, animals, and plants have bilateral symmetry, but in nature at large there is only symmetry about a horizontal axis… reflections in water, nature’s mirror.
This seems related to the puzzling question of why a mirror reverses left and right, but not up and down.
The Steinberg quote is from the book Reflections and Shadows, reviewed here.
Bibliographic data on Auden’s commonplace book:
AUTHOR Auden, W. H. (Wystan Hugh), 1907-1973. TITLE A Certain World; a Commonplace Book
[selected by] W. H. Auden.
PUBLISHER New York, Viking Press [1970]
SUBJECT Commonplace-books.
A couple of websites on commonplace books:
A classic:
The Practical Cogitator – The Thinker’s Anthology,
by Charles P. Curtis, Jr., and Ferris Greenslet,
Houghton Mifflin Company Boston, Massachusetts
c 1962 Third Edition – Revised and Enlarged
Saturday, July 20, 2002
Saturday July 20, 2002
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.


































.jpg)