Wednesday, May 1, 2024
Microsoft Classic Paint: Tools and Shapes
Sunday, February 18, 2024
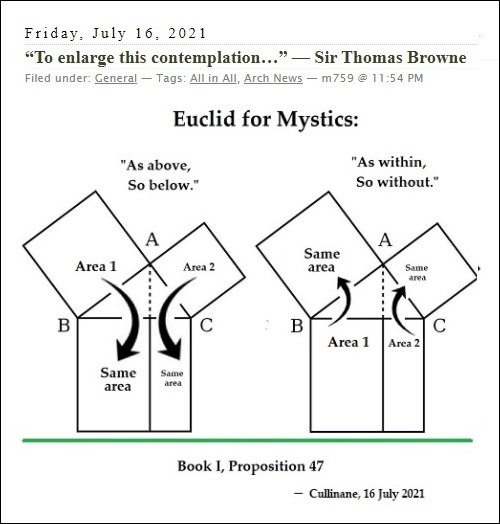
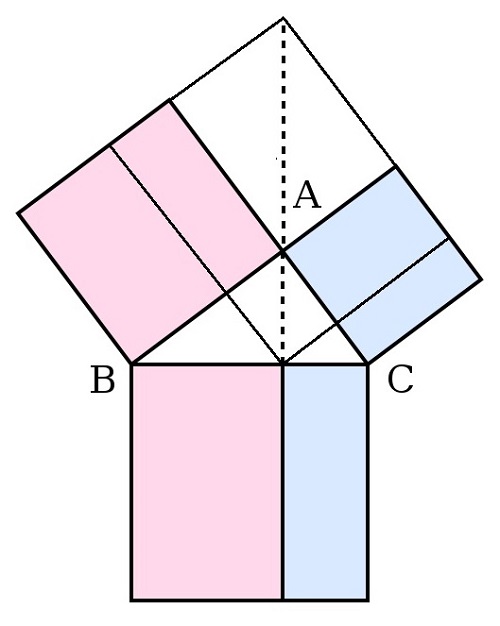
Bride’s Chair vs. One-Liner
The "bride's chair" is the figure illustrating Euclid's proof
of the Pythagorean theorem (click image to enlarge) —
A somewhat simpler approach —
"Drop me a line" — Request attributed to Emma Stone
Saturday, January 21, 2023
Saturday, May 7, 2022
“Use Your Noodle” Update
Update to yesterday's "Use Your Noodle" post . . .
Click the above image to enlarge.
Update of 2:40 AM May 7, 2022 —
Flusser's seven "pillars" appear to be the main sections of the Tractatus
— numbered 1 through 7, with many intermediate numbered passages.
For a more geometric meditation on "the shape of things," see other
posts tagged "Shape Constant" in this journal.
Friday, May 6, 2022
Use Your Noodle (For Byron Gogol*)
An essay from . . .
The Shape of Things: A Philosophy of Design Wittgenstein’s Architecture The universe of texts can be seen as a landscape. In it one can make out mountains and valleys, rivers and lakes, castles, farmyards and inner-city slums. On the horizon of the scene visualized in this way, the Bible and Homer appear as gigantic ice-covered mountains. The vast, tranquil lake of Aristotle’s texts, where fishermen idly throw their nets and philologists row their boats, occupies a part of the valley bottom. There, the tumbling waterfall of Nietzsche is captured by the broad river of modern pragmatism. Towering above everything, the Gothic cathedral of St Thomas Aquinas’s Summae dominates the cathedral square of the city, in which the roofs and gables of Baroque speculations jostle one another. In the suburbs of this city, one catches sight of the Romantic, Realist and Modernist housing-blocks and factories of more recent litera¬ ture; somewhat apart from all these stands a small, apparently insignificant house resembling scaffolding more than a finished building: Wittgenstein’s building. This little house is called the Tractatus. This name isn’t the product of a one-track mind. For when one enters the house, one notices immediately that this is not a place that has lost track of things. Quite the opposite: It is a place of mirror- images. The house stands on six foundation pillars which support one another by means of cross-beams organized in a hierarchy. In the middle, however, there rises a seventh pillar whose function it is to cut through the building and free it from the ground. So the house with all its corners, angles and joints is protected, armoured and impregnable. And yet, and for that very reason, it is threatened with collapse and disappearance without trace – condemned in advance and from the outset. The building is set out: It consists of propositions. Every proposition presupposes all the preceding ones and is itself the 76 presupposition of all the following propositions. Proposition by proposition, anyone who enters progresses through the prescribed rooms, and his step is supported by consistencies. Suddenly, with one proposition, one single proposition, the ground gives way beneath his feet. He falls head first into the abyss. Wittgenstein’s house is situated in a suburb of that city whose cathedral square is dominated by the towers of Thomas Aquinas’s cathedral. The small, modest pillars of Wittgenstein’s house support one another according to the same logico- philosophical method as the pillars of the cathedral support one another. But there appears to be a world of difference between the cathedral and the little house: The cathedral is a ship pointing in the direction of heaven, and the little house is a trap-door pointing in the direction of a bottomless abyss. But be careful: May Thomas Aquinas not have been right in saying after his revelation that everything he had written before was like straw? May not the heaven above the cathedral be the same black hole as the abyss beneath the little house? May not Wittgenstein’s little house be the cathedral of today? And those mirrors whose images simultaneously mirror one another, may they not be our equivalent of stained-glass windows? The landscape portrayed in this essay, it goes without saying, is a metaphor. Is it possible to identify it as Vienna? And is it possible for anyone entering Wittgenstein’s little house in that unlikely place to make out a hint of the unsayable? What we cannot speak about we must pass over in silence. 77 |
Click the above image to enlarge.
See as well . . .
Update of 2:40 AM May 7, 2022 —
Flusser's seven "pillars" appear to be the main sections of the Tractatus
— numbered 1 through 7, with many intermediate numbered passages.
For a more geometric meditation on "the shape of things," see other
posts tagged "Shape Constant" in this journal.
*Byron Gogol is a tech magnate in the HBO series "Made for Love."

Monday, April 4, 2022
Pythagoras via Tao, Polya, and Euclid
From a post of April 1 —
A related post by Terry Tao on September 14, 2007 —

The comments on Tao's post contain a reference to Polya's classic
Induction and Analogy in Mathematics . (See pp. 15-17.) Polya notes
on page 15 —
"Generalization, Specialization, and Analogy often concur
in solving mathematical problems. Let us take as an example
the proof of the best known theorem of elementary geometry,
the theorem of Pythagoras. The proof that we shall discuss is
not new; it is due to Euclid himself (Euclid VI, 31)."


Friday, April 1, 2022
Beauty Bare … ?
Update of 11:30 AM ET April 1, 2022 — A simpler version:
The above picture may be used to to introduce the concept of a "shape constant"
in similar figures — like the shape constant pi in a circle or the square root of 2
in a square. In each of the three similar figures at right above, the ratio of the
triangular area to the area of the attached square is a shape constant …
the same, because of their similarity, for each of the three shapes. Since the
areas of the top two triangles at right sum to that of the enclosed triangle at left,
their attached square areas sum to the area of the bottom square, Q.E.D.
The source of the proof —
Monday, March 14, 2022
Tuesday, December 28, 2021
Shape as Form (Not the Michael Fried Version)
Monday, October 11, 2021
The Dropped Line
"Drop me a line" — Imagined request by Emma Stone.

Here Ec refers not to the line it interrupts, but rather to
the area (equal to areas Ea plus Eb ) of the large triangle.
The notation is in service of an elaborate joke by Schroeder
that need not be repeated here.
I prefer the E-C humor of Robert A. Heinlein —
Saturday, October 9, 2021
This Side of Paradise
Note the book subtitle below: "minutes from an infinite paradise."

For further details, see other posts tagged Revelado.
Wednesday, August 25, 2021
Einstein Revelado
Thursday, August 19, 2021
A Scalpel for Einstein
(A sequel to this morning's post A Subtle Knife for Sean.)
Exhibit A —
Einstein in The Saturday Review, 1949 —
"In any case it was quite sufficient for me
if I could peg proofs upon propositions
the validity of which did not seem to me to be dubious.
For example, I remember that an uncle told me
the Pythagorean theorem before the holy geometry booklet
had come into my hands. After much effort I succeeded
in 'proving' this theorem on the basis of the similarity
of triangles; in doing so it seemed to me 'evident' that
the relations of the sides of the right-angled triangles
would have to be completely determined by one of the
acute angles. Only something which did not in similar fashion
seem to be 'evident' appeared to me to be in need of any proof
at all. Also, the objects with which geometry deals seemed to
be of no different type than the objects of sensory perception,
'which can be seen and touched.' This primitive idea, which
probably also lies at the bottom of the well-known Kantian
problematic concerning the possibility of 'synthetic judgments
a priori' rests obviously upon the fact that the relation of
geometrical concepts to objects of direct experience
(rigid rod, finite interval, etc.) was unconsciously present."
Exhibit B —
Strogatz in The New Yorker, 2015 —
"Einstein, unfortunately, left no … record of his childhood proof.
In his Saturday Review essay, he described it in general terms,
mentioning only that it relied on 'the similarity of triangles.'
The consensus among Einstein’s biographers is that he probably
discovered, on his own, a standard textbook proof in which similar
triangles (meaning triangles that are like photographic reductions
or enlargements of one another) do indeed play a starring role.
Walter Isaacson, Jeremy Bernstein, and Banesh Hoffman all come
to this deflating conclusion, and each of them describes the steps
that Einstein would have followed as he unwittingly reinvented
a well-known proof."
Exhibit C —

Schroeder presents an elegant and memorable proof. He attributes
the proof to Einstein, citing purely hearsay evidence in a footnote.
The only other evidence for Einstein's connection with the proof
is his 1949 Saturday Review remarks. If Einstein did come up with
the proof at age 11 and discuss it with others later, as Schroeder
claims, it seems he might have felt a certain pride and been more
specific in 1949, instead of merely mentioning the theorem in passing
before he discussed Kantian philosophy relating concepts to objects.
Strogatz says that . . .
"What we’re seeing here is a quintessential use of
a symmetry argument… scaling….
Throughout his career, Einstein would continue to
deploy symmetry arguments like a scalpel, getting to
the hidden heart of things."
Connoisseurs of bullshit may prefer a faux-Chinese approach to
"the hidden heart of things." See Log24 on August 16, 2021 —
http://m759.net/wordpress/?p=96023 —
In a Nutshell: The Core of Everything .