Monday, June 25, 2018
"A blank underlies the trials of device."
— Wallace Stevens
"Designing with just a blank piece of paper is very quiet."
— Kate Cullinane
Related material —
An image posted at 12 AM ET December 25, 2014:

The image stands for the
phrase "five by five,"
meaning "loud and clear."

Other posts featuring the above 5×5 square with some added structure:


Comments Off on The Trials of Device
Saturday, June 23, 2018

Backstory for fiction fans, from Log24 on June 11 —

Related non -fiction —

See as well the structure discussed in today's previous post.
Comments Off on Meanwhile …
Thursday, June 14, 2018
"There is a structure theory for bull-free graphs
modulo the structure of triangle-free graphs
and their complements, which again is not easy.
(The bull has a triangular face, with horns or
pendant edges at two of its three vertices.)"
— Peter J. Cameron today
For example —
The bull graph in a book by Clarence R. Wylie, Jr.
(author of the poem "Paradox" (1948)). See no. 6 below —

See also Wikipedia.
Related material —
J. Paul Getty and Minotaur, according to Hollywood —

Comments Off on Wylie’s Bull
Monday, June 4, 2018
“Unsheathe your dagger definitions.” — James Joyce, Ulysses
The “triple cross” link in the previous post referenced the eightfold cube
as a structure that might be called the trinity stone .



Comments Off on The Trinity Stone Defined
Thursday, May 3, 2018
. . . Con Figuras de Espantar
"He Who Searches is multifaceted in structure …"
— Publisher's description of a Helen Lane translation
of "Como en la Guerra ," by Luisa Valenzuela.
Also by Valenzuela —

Related material — An obituary from The Boston Globe today
on the April 5 death of Borinsky's translator, and . . .

"He Who Searches" may consult also posts tagged Date.
Comments Off on Multifaceted . . .
Friday, April 13, 2018


See also Log24 posts now tagged "Is and As."
Comments Off on Philosophy 101
Tuesday, March 27, 2018

Related material on automorphism groups —

The "Eightfold Cube" structure shown above with Weyl
competes rather directly with the "Eightfold Way" sculpture
shown above with Bryant. The structure and the sculpture
each illustrate Klein's order-168 simple group.
Perhaps in part because of this competition, fans of the Mathematical
Sciences Research Institute (MSRI, pronounced "Misery') are less likely
to enjoy, and discuss, the eight-cube mathematical structure above
than they are an eight-cube mechanical puzzle like the one below.

Note also the earlier (2006) "Design Cube 2x2x2" webpage
illustrating graphic designs on the eightfold cube. This is visually,
if not mathematically, related to the (2010) "Expert's Cube."
Comments Off on Compare and Contrast
Friday, March 9, 2018
The New York Times today on a philosopher of
history who reportedly died on Monday, March 5 —
“Perhaps White’s most controversial idea,
and one for which he was so often shunned
by his fellow historians, is that ‘all stories
are fictions,’ ” Robert Doran, a professor at
the University of Rochester … said by email.
"White held that while historical facts are
scientifically verifiable, stories are not.
Stories are made, not found in the historical data;
historical meaning is imposed on historical facts
by means of the choice of plot-type, and this choice
is inevitably ethical and political at bottom.
"This is what White called 'emplotment,' a term
he coined," Dr. Doran continued. "Even the most basic
beginning-middle-end structure of a story represents
an imposition: The historian chooses where to begin,
where to end, and what points are important in the middle.
There is no scientific test for 'historical significance.' "
From this journal on Monday, White's reported date of death —

Plan 9 continues.
Comments Off on The Cemetery Plot
Friday, February 16, 2018
The Institute for Advanced Study (IAS) at Princeton in its Fall 2015 Letter
revived "Beautiful Mathematics" as a title:

This ugly phrase was earlier used by Truman State University
professor Martin Erickson as a book title. See below.
In the same IAS Fall 2015 Letter appear the following remarks
by Freeman Dyson —
". . . a special case of a much deeper connection that Ian Macdonald
discovered between two kinds of symmetry which we call modular and affine.
The two kinds of symmetry were originally found in separate parts of science,
modular in pure mathematics and affine in physics. Modular symmetry is
displayed for everyone to see in the drawings of flying angels and devils
by the artist Maurits Escher. Escher understood the mathematics and got the
details right. Affine symmetry is displayed in the peculiar groupings of particles
created by physicists with high-energy accelerators. The mathematician
Robert Langlands was the first to conjecture a connection between these and
other kinds of symmetry. . . ." (Wikipedia link added.)
The adjective "modular" might aptly be applied to . . .

The adjective "affine" might aptly be applied to . . .

The geometry of the 4×4 square combines modular symmetry
(i.e., related to theta functions) with the affine symmetry above.
Hudson's 1905 discussion of modular symmetry (that of Rosenhain
tetrads and Göpel tetrads) in the 4×4 square used a parametrization
of that square by the digit 0 and the fifteen 2-subsets of a 6-set, but
did not discuss the 4×4 square as an affine space.
For the connection of the 15 Kummer modular 2-subsets with the 16-
element affine space over the two-element Galois field GF(2), see my note
of May 26, 1986, "The 2-subsets of a 6-set are the points of a PG(3,2)" —

— and the affine structure in the 1979 AMS abstract
"Symmetry invariance in a diamond ring" —

For some historical background on the symmetry investigations by
Dyson and Macdonald, see Dyson's 1972 article "MIssed Opportunities."
For Macdonald's own use of the words "modular" and "affine," see
Macdonald, I. G., "Affine Lie algebras and modular forms,"
Séminaire N. Bourbaki , Vol. 23 (1980-1981), Talk no. 577, pp. 258-276.
Comments Off on Two Kinds of Symmetry
Wednesday, December 27, 2017
See the 27-part structure of
the 3x3x3 Galois cube

as well as Autism Sunday 2015.
Comments Off on For Day 27 of December 2017
Tuesday, December 26, 2017
(Continued)
Two Students of Structure
A comment on Sean Kelly's Christmas Morning column on "aliveness"
in the New York Times philosophy series The Stone —

Diana Senechal's 1999 doctoral thesis at Yale was titled
"Diabolical Structures in the Poetics of Nikolai Gogol."
Her mother, Marjorie Senechal, has written extensively on symmetry
and served as editor-in-chief of The Mathematical Intelligencer .
From a 2013 memoir by Marjorie Senechal —
|
"While I was in Holland my enterprising student assistant at Smith had found, in Soviet Physics – Crystallography, an article by N. N. Sheftal' on tetrahedral penetration twins. She gave it to me on my return. It was just what I was looking for. The twins Sheftal' described had evidently begun as (111) contact twins, with the two crystallites rotated 60o with respect to one another. As they grew, he suggested, each crystal overgrew the edges of the other and proceeded to spread across the adjacent facet. When all was said and done, they looked like they'd grown through each other, but the reality was over-and-around. Brilliant! I thought. Could I apply this to cubes? No, evidently not. Cube facets are all (100) planes. But . . . these crystals might not have been cubes in their earliest stages, when twinning occurred! I wrote a paper on "The mechanism of certain growth twins of the penetration type" and sent it to Martin Buerger, editor of Neues Jarbuch für Mineralogie. This was before the Wrinch symposium; I had never met him. Buerger rejected it by return mail, mostly on the grounds that I hadn't quoted any of Buerger's many papers on twinning. And so I learned about turf wars in twin domains. In fact I hadn't read his papers but I quickly did. I added a reference to one of them, the paper was published, and we became friends.[5]
After reading Professor Sheftal's paper I wrote to him in Moscow; a warm and encouraging correspondence ensued, and we wrote a paper together long distance.[6] Then I heard about the scientific exchanges between the Academies of Science of the USSR and USA. I applied to spend a year at the Shubnikov Institute for Crystallography, where Sheftal' worked. I would, I proposed, study crystal growth with him, and color symmetry with Koptsik. To my delight, I was accepted for an 11-month stay. Of course the children, now 11 and 14, would come too and attend Russian schools and learn Russian; they'd managed in Holland, hadn't they? Diana, my older daughter, was as delighted as I was. We had gone to Holland on a Russian boat, and she had fallen in love with the language. (Today she holds a Ph.D. in Slavic Languages and Literature from Yale.) . . . .
. . . we spent the academic year 1978-79 in Moscow.
|
Philosophy professors and those whose only interest in mathematics
is as a path to the occult may consult the Log24 posts tagged Tsimtsum.
Comments Off on Raiders of the Lost Stone
Wednesday, December 20, 2017

Update of 9:29 PM ET Dec. 20, 2017 —
See in particular, in the above Notices , the article
"Algebraic Structures on Polytopes," by Federico Ardila,
within the 2018 Joint Mathematics Meeting Lecture Sampler.
Related reading:
arXiv:1711.09102v1 [hep-th] 24 Nov 2017,
"Scattering Forms and the Positive Geometry of
Kinematics, Color and the Worldsheet," by
Nima Arkani-Hamed, Yuntao Bai, Song He, Gongwang Yan
(Submitted to the arXiv on 24 Nov. 2017).
Comments Off on January 2018 AMS Notices
Monday, December 18, 2017
From the American Mathematical Society homepage today —

From concinnitasproject.org —
"Concinnitas is the title of a portfolio of fine art prints. . . .
The portfolio draws its name from a word famously used
by the Renaissance scholar, artist, architect, and philosopher
Leon Battista Alberti (1404-1472) to connote the balance of
number, outline, and position (in essence, number, geometry,
and topology) that he believed characterize a beautiful work of art."
The favicon of the Concinnitas Project —

The structure of the Concinnitas favicon —

This structure is from page 15 of
"Diamond Theory," a 1976 preprint —
 .
.
Comments Off on Mathematics and Art
Sunday, December 10, 2017

See also Symplectic in this journal.
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”

The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2). See also
related remarks on the notion of linear (or line ) complex
in the finite projective space PG(3,2) —


Comments Off on Geometry
Thursday, November 23, 2017
David Brooks in The New York Times today —
"We once had a unifying national story, celebrated each Thanksgiving.
It was an Exodus story. Americans are the people who escaped oppression,
crossed a wilderness and are building a promised land. The Puritans brought
this story with them. Each wave of immigrants saw themselves in this story.
The civil rights movement embraced this story.
But we have to admit that many today do not resonate with this story. . . .
Today, we have no common national narrative, no shared way
of interpreting the flow of events. Without a common story,
we don’t know what our national purpose is. We have no
common set of goals or ideals.
We need a new national narrative."
From a post of August 15, 2010 —

For some background, see Java Jive and Today's Theology.
Related readings —
From 1928:

From the previous post:
"Thus, instead of Propp's chronological scheme,
in which the order of succession of events
is a feature of the structure . . .
another scheme should be adopted, which would present
a structural model defined as the group of transformations
of a small number of elements. This scheme would appear
as a matrix . . . ."
— Claude Lévi-Strauss, 1960

Comments Off on The Matrix
| Claude Lévi-Strauss
From his “Structure and Form:
Reflections on a Work by Vladimir Propp” *
To maintain. as I have done. that the permutability of contents is not arbitrary amounts to saying that, if the analysis is carried to a sufficiently deep level, behind diversity we will discover constancy. And, of course. the avowed constancy of form must not hide from us that functions are also permutable.
The structure of the folktale as it is illustrated by Propp presents a chronological succession of qualitatively distinct functions. each constituting an independent genre. One can wonder whether—as with dramatis personae and their attributes— Propp does not stop too soon, seeking the form too close to the level of empirical observation. Among the thirty-one functions that he distinguishes, several are reducible to the same function reappearing at different moments of the narrative but after undergoing one or a number of transformations . I have already suggested that this could be true of the false hero (a transformation of the villain), of assigning a difficult task (a transformation of the test), etc. (see p. 181 above), and that in this case the two parties constituting the fundamental tale would themselves be transformations of each other.
Nothing prevents pushing this reduction even further and analyzing each separate partie into a small number of recurrent functions, so that several of Propp’s functions would constitute groups of transformations of one and the same function. We could treat the “violation” as the reverse of the “prohibition” and the latter as a negative transformation of the “injunction.” The “departure” of the hero and his “return” would appear as the negative and positive expressions of the same disjunctive function. The “quest” of the hero (hero pursues someone or something) would become the opposite of “pursuit” (hero is pursued by something or someone), etc.

In Vol. I of Structural Anthropology , p. 209, I have shown that this analysis alone can account for the double aspect of time representation in all mythical systems: the narrative is both “in time” (it consists of a succession of events) and “beyond” (its value is permanent). With regard to Propp’s theories my analysis offers another advantage: I can reconcile much better than Propp himself his principle of a permanent order of wondertale elements with the fact that certain functions or groups of functions are shifted from one tale to the next (pp. 97-98. p. 108) If my view is accepted, the chronological succession will come to be absorbed into an atemporal matrix structure whose form is indeed constant. The shifting of functions is then no more than a mode of permutation (by vertical columns or fractions of columns).
These critical remarks are certainly valid for the method used by Propp and for his conclusions. However. it cannot be stressed enough that Propp envisioned them and in several places formulated with perfect clarity the solutions I have just suggested. Let us take up again from this viewpoint the two essential themes of our discussion: constancy of the content (in spite of its permutability) and permutability of functions (in spite of their constancy).
* Translated from a 1960 work in French. It appeared in English as Chapter VIII
of Structural Anthropology, Volume 2 (U. of Chicago Press, 1976). Chapter VIII
was originally published in Cahiers de l’Institut de Science Économique Appliquée ,
No. 9 (Series M, No. 7) (Paris: ISEA, March 1960).
|
See also “Lévi-Strauss” + Formula in this journal.
Some background related to the previous post —

Comments Off on Lévi-Strauss vs. Propp
Monday, November 13, 2017


See also Norbert Wiener in this journal and …

Related material for the Church of Synchronology —
The Log24 post on the above New York Times death date.
Comments Off on In Nomine Patris
Monday, November 6, 2017
"Colorless green ideas sleep furiously is a sentence
composed by Noam Chomsky in his 1957 book
Syntactic Structures as an example of a sentence
that is grammatically correct, but semantically nonsensical."
— Wikipedia article on the sentence
Buddhist midrash from The New York Times today —
"For example, psychology has lately started to let go of its
once-sharp distinction between 'cognitive' and 'affective'
parts of the mind; it has started to see that feelings are so
finely intertwined with thoughts as to be part of their very
coloration."
See also other recent Log24 posts now tagged Coloration.
Comments Off on The Chomsky Koan
Sunday, October 29, 2017

Logo from the above webpage —

See also the similar structure of the eightfold cube, and …

Related dialogue from the new film "Unlocked" —
1057
01:31:59,926 –> 01:32:01,301
Nice to have you back, Alice.
1058
01:32:04,009 –> 01:32:05,467
Don't be a stranger.
Comments Off on File System… Unlocked
Wednesday, October 18, 2017
Structure of the Dürer magic square
16 3 2 13
5 10 11 8 decreased by 1 is …
9 6 7 12
4 15 14 1
15 2 1 12
4 9 10 7
8 5 6 11
3 14 13 0 .
Base 4 —
33 02 01 30
10 21 22 13
20 11 12 23
03 32 31 00 .
Two-part decomposition of base-4 array
as two (non-Latin) orthogonal arrays —
3 0 0 3 3 2 1 0
1 2 2 1 0 1 2 3
2 1 1 2 0 1 2 3
0 3 3 0 3 2 1 0 .
Base 2 –
1111 0010 0001 1100
0100 1001 1010 0111
1000 0101 0110 1011
0011 1110 1101 0000 .
Four-part decomposition of base-2 array
as four affine hyperplanes over GF(2) —
1001 1001 1100 1010
0110 1001 0011 0101
1001 0110 0011 0101
0110 0110 1100 1010 .
— Steven H. Cullinane,
October 18, 2017
See also recent related analyses of
noted 3×3 and 5×5 magic squares.
Comments Off on Dürer for St. Luke’s Day
Tuesday, October 17, 2017

See also Holy Field in this journal.
Some related mathematics —
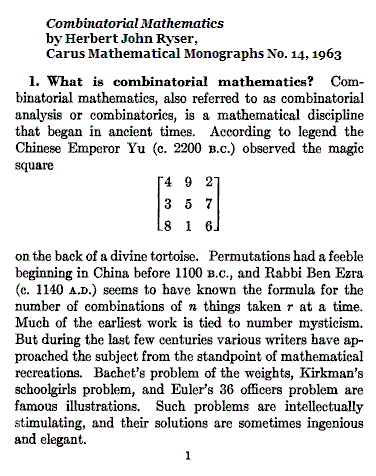
Analysis of the Lo Shu structure —
Structure of the 3×3 magic square:
4 9 2
3 5 7 decreased by 1 is …
8 1 6
3 8 1
2 4 6
7 0 5
In base 3 —
10 22 01
02 11 20
21 00 12
As orthogonal Latin squares
(a well-known construction) —
1 2 0 0 2 1
0 1 2 2 1 0
2 0 1 1 0 2 .
— Steven H. Cullinane,
October 17, 2017
Comments Off on Plan 9 Continues
Wednesday, October 4, 2017
Text —
"A field is perhaps the simplest algebraic structure we can invent."
— Hermann Weyl, 1952
Context —

See also yesterday's Personalized Book Search.
|
Full text of Symmetry – Internet Archive —
https://archive.org/details/Symmetry_482
A field is perhaps the simplest algebraic 143 structure
we can invent. Its elements are numbers. Characteristic
for its structure are the operations of addition and …
|
From a Log24 search for Mathematics+Nutshell —

Comments Off on Text and Context
Tuesday, September 12, 2017
The New York Times online this evening —
"Mr. Jobs, who died in 2011, loomed over Tuesday’s
nostalgic presentation. The Apple C.E.O., Tim Cook,
paid tribute, his voice cracking with emotion, Mr. Jobs’s
steeple-fingered image looming as big onstage as
Big Brother’s face in the classic Macintosh '1984' commercial."
— James Poniewozik
Review —
|
Thursday, September 1, 2011
Filed under: Uncategorized — Tags: Geometry, Priority — m759 @ 11:00 AM
"Design is how it works." — Steven Jobs (See Symmetry and Design.)
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
— "Block Designs," by Andries E. Brouwer
. . . .
|
See also 1984 Bricks in this journal.
Comments Off on Think Different
Saturday, September 9, 2017
Del Toro and the History of Mathematics ,
Or: Applied Bullshit Continues
For del Toro —


For the history of mathematics —
|
Thursday, September 1, 2011
Filed under: Uncategorized — Tags: Geometry, Priority — m759 @ 11:00 AM
"Design is how it works." — Steven Jobs (See Symmetry and Design.)
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
— "Block Designs," by Andries E. Brouwer
. . . .
|
Comments Off on How It Works
Monday, August 14, 2017
See also posts now tagged "Go Set."
Comments Off on Visual Impact
Thursday, July 27, 2017
Michiko Kakutani in The New York Times —
"The detective story genre concerns the finding of clues
and the search for hidden designs, and its very form
underscores Mr. Pynchon’s obsession with conspiracies
and the existence of systems too complicated to understand."
— Review of Pynchon's Bleeding Edge , Sept. 10, 2013
Background: "Moss on the Wall," this journal on that date.
A less complicated system —
"Plan 9 deals with the resurrection of the dead."
— Bill Murray in "Ed Wood"

For The Church of Plan 9
(The plan , as well as the elevation ,
of the above structure is a 3×3 grid.)
Comments Off on Keeping It Simple
Sunday, July 2, 2017

From an Anthony Lane movie review in the April 8, 2013,
issue of The New Yorker —
"When the Lord God forbade his worshippers to bow down
before any graven image, [Rosario] Dawson’s face was
exactly the kind of thing He had in mind. No other star can
boast such sculptured features—except Vincent Cassel,
who is pretty damn graven himself. When the two of them
make love, in 'Trance,' one strong bone structure pressed
against another, it’s like a clash of major religions. What if
they had a family? The kids would be practically Cubist."
As for the other film Lane reviewed in that issue, "Blancanieves" —
See Snow White + Cube in this journal.
See as well a related cartoon graveyard, also from April 8, 2013.
Comments Off on Practically Cubist
Friday, June 30, 2017
The previous post quoted one theologian on a book
by another theologian, saying its tone "is patronizing
and its arguments are hurriedly put together."
For a more leisurely sort of argument, see a 1995* remark
by a mathematician, Ronald Shaw, quoted here on the morning
of Tuesday, June 27, in an update at the end of the previous day's
post "Upgrading to Six" —
". . . recall the notions of Eddington (1936) . . . ."
* In "Finite Geometry, Dirac Groups and the
Table of Real Clifford Algebras," pages 59-99 of
R. Ablamowicz and P. Lounesto (eds.),
Clifford Algebras and Spinor Structures ,
Kluwer Academic Publishers, 1995.
Comments Off on Hurriedly Put Together
Friday, June 23, 2017
The title is from an obituary in tonight's online New York Times.
Information —



See also another art publication cover from 1976 —

Comments Off on “Information from the Middle of the Night”
Thursday, May 18, 2017
Exit stage right, enter stage center, exit stage left —

A search for "Darkness Doubled" in this journal yields a link
to a post on "endgame art" which leads in turn to a post with
the following quotation —
"It is proposed that the two structures of grid and target
are the symbolic vehicles par excellence . . . ."
— Review of Rudolf Arnheim's The Power of the Center:
A Study of Composition in the Visual Arts (U. of Calif. Press, 1982).
Review by David A. Pariser, Studies in Art Education , Vol. 24, No. 3
(1983), pp. 210-213.
"Darkness Doubled" is a phrase from a song titled "Marquee Moon."

Comments Off on Marquee Moon continues
Sunday, April 23, 2017
Comments Off on A Day in June
Friday, April 14, 2017

The above four-element sets of black subsquares of a 4×4 square array
are 15 of the 60 Göpel tetrads , and 20 of the 80 Rosenhain tetrads , defined
by R. W. H. T. Hudson in his 1905 classic Kummer's Quartic Surface .
Hudson did not view these 35 tetrads as planes through the origin in a finite
affine 4-space (or, equivalently, as lines in the corresponding finite projective
3-space).
In order to view them in this way, one can view the tetrads as derived,
via the 15 two-element subsets of a six-element set, from the 16 elements
of the binary Galois affine space pictured above at top left.
This space is formed by taking symmetric-difference (Galois binary)
sums of the 15 two-element subsets, and identifying any resulting four-
element (or, summing three disjoint two-element subsets, six-element)
subsets with their complements. This process was described in my note
"The 2-subsets of a 6-set are the points of a PG(3,2)" of May 26, 1986.
The space was later described in the following —


Comments Off on Hudson and Finite Geometry
Monday, April 10, 2017

From this journal on August 7, 2010 (footnotes added today) —
|
The title of this post, "Rift Designs," … is taken from Heidegger.
From a recent New Yorker review of Absence of Mind by Marilynne Robinson—
"Robinson is eloquent in her defense of the mind’s prerogatives, but her call for a renewed metaphysics might be better served by rereading Heidegger than by dusting off the Psalms."
Following this advice, we find—
"Propriation1 gathers the rift-design2 of the saying and unfolds it3 in such a way that it becomes the well-joined structure4 of a manifold showing."
— p. 415 of Heidegger's Basic Writings , edited by David Farrell Krell, HarperCollins paperback, 1993
"Das Ereignis versammelt den Aufriß der Sage und entfaltet ihn zum Gefüge des vielfältigen Zeigens."
— Heidegger, Weg zur Sprache
1. "Mirror-Play of the Fourfold"
2. "Christ descending into the abyss"
3. Barrancas of Cuernavaca
4. Combinatorics, Philosophy, Geometry
|
Comments Off on Heidegger for Passover
Tuesday, April 4, 2017
"Plan 9 deals with the resurrection of the dead."
— Bill Murray in "Ed Wood"

For The Church of Plan 9
(The plan , as well as the elevation ,
of the above structure is a 3×3 grid.)
Comments Off on Plan 9 Continues
Tuesday, March 28, 2017
Click here to enlarge. Click the image for the source page.

The "this page" reference is to …
Finite Geometry of the Square and Cube.
Also from March 14, 2017 —

Related material —

Comments Off on Backstory
Wednesday, March 15, 2017
The Key to All Mythologies in a Cartoon Graveyard
This is a sequel to yesterday's post Review, which
suggested a look at Lévi-Strauss's The Raw and The Cooked
in Derrida's “Structure, Sign, and Play," and then a look at the …
Financial Times of February 26, 2010 —

"The metaphor for metamorphosis no keys unlock."
— Steven H. Cullinane, November 7, 1986
Comments Off on Middle March:
Friday, March 10, 2017
"The transformed urban interior is the spatial organisation of
an achiever, one who has crossed the class divide and who uses
space to express his membership of, not aspirations towards,
an ascendant class in our society: the class of those people who
earn their living by transformation— as opposed to the mere
reproduction— of symbols, such as writers, designers, and
academics"
— The Social Logic of Space ,
by Bill Hillier and Julienne Hanson,
Cambridge University Press, 1984
For another perspective on the achievers, see The Deceivers .
Related material —
Exhibit A:


Exhibit B:

Exhibit C:

Comments Off on The Transformers
Thursday, March 9, 2017

See also Log24 posts related to "Go Set a Structure"
as well as "New Haven" + Grid.
Comments Off on Yale Architectural Figure
Thursday, February 23, 2017
Comments Off on Midnight Special
Friday, February 17, 2017
"Bertram Kostant, professor emeritus of mathematics at MIT,
died at the Hebrew Senior Rehabilitation Center in Roslindale,
Massachusetts, on Thursday, Feb. 2, at the age of 88."
— MIT News, story dated Feb. 16, 2017
See also a search for Kostant in this journal.
Regarding the discussions of symmetries and "facets" found in
that search —
Kostant:
“A word about E(8). In my opinion, and shared by others,
E(8) is the most magnificent ‘object’ in all of mathematics.
It is like a diamond with thousands of facets. Each facet
offering a different view of its unbelievable intricate internal
structure.”
Cullinane:
In the Steiner system S(5, 8, 24) each octad might be
regarded as a "facet," with the order of the system's
automorphism group, the Mathieu group M24 , obtained
by multiplying the number of such facets, 759, by the
order of the octad stabilizer group, 322,560.
Analogously …

Comments Off on Kostant Is Dead
In the recent science fiction film "Arrival," Amy Adams portrays
a linguist, Louise Banks, who must learn to translate the language of
aliens ("Heptapods") who have just arrived in their spaceships.
The point of this tale seems to have something to do with Banks
learning, along with the aliens' language, their skill of seeing into
the future.
Louise Banks wannabes might enjoy the works of one
Metod Saniga, who thinks that finite geometry might have
something to do with perceptions of time.
See Metod Saniga, “Algebraic Geometry: A Tool for Resolving
the Enigma of Time?”, in R. Buccheri, V. Di Gesù and M. Saniga (eds.),
Studies on the Structure of Time: From Physics to Psycho(patho)logy,
Kluwer Academic / Plenum Publishers, New York, 2000, pp. 137–166.
Available online at www.ta3.sk/~msaniga/pub/ftp/mathpsych.pdf .
Although I share an interest in finite geometry with Saniga —
see, for instance, his remarks on Conwell heptads in the previous post
and my own remarks in yesterday's post "Schoolgirls and Heptads" —
I do not endorse his temporal speculations.
Comments Off on Heptads and Heptapods
Wednesday, February 15, 2017

Space —

Space structure —
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”

The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Space shuttle —

Related ethnic remarks —

… As opposed to Michael Larsen —

Funny, you don't look Danish.
Comments Off on Warp and Woof
Sunday, February 12, 2017
“Perhaps the philosophically most relevant feature of modern science
is the emergence of abstract symbolic structures as the hard core
of objectivity behind— as Eddington puts it— the colorful tale of
the subjective storyteller mind.”
— Hermann Weyl, Philosophy of Mathematics and
Natural Science , Princeton, 1949, p. 237
Harvard University Press on the late Angus Fletcher, author of
The Topological Imagination and Colors of the Mind —
From the Harvard webpage for Colors of the Mind —
Angus Fletcher is one of our finest theorists of the arts,
the heir to I. A. Richards, Erich Auerbach, Northrop Frye.
This… book… aims to open another field of study:
how thought— the act, the experience of thinking—
is represented in literature.
. . . .
Fletcher’s resources are large, and his step is sure.
The reader samples his piercing vision of Milton’s
Satan, the original Thinker,
leaving the pain of thinking
as his legacy for mankind.
A 1992 review by Vinay Dharwadker of Colors of the Mind —

See also the above word "dianoia" in The Echo in Plato's Cave.
Some context …

This post was suggested by a memorial piece today in
the Los Angeles Review of Books —
A Florilegium for Angus Fletcher
By Kenneth Gross, Lindsay Waters, V. N. Alexander,
Paul Auster, Harold Bloom, Stanley Fish, K. J. Knoespel,
Mitchell Meltzer, Victoria Nelson, Joan Richardson,
Dorian Sagan, Susan Stewart, Eric Wilson, Michael Wood
Fletcher reportedly died on November 28, 2016.
"I learned from Fletcher how to apprehend
the daemonic element in poetic imagination."
— Harold Bloom in today's Los Angeles florilegium
For more on Bloom and the daemonic, see a Log24 post,
"Interpenetration," from the date of Fletcher's death.
Some backstory: Dharwadker in this journal.
Comments Off on Colorful Tales
Sunday, January 8, 2017
The title refers to the Chinese book the I Ching ,
the Classic of Changes .
The 64 hexagrams of the I Ching may be arranged
naturally in a 4x4x4 cube. The natural form of transformations
("changes") of this cube is given by the diamond theorem.
A related post —

Comments Off on A Theory of Everything
Saturday, December 24, 2016
In memory of an American artist whose work resembles that of
the Soviet constructivist Karl Ioganson (c. 1890-1929).
The American artist reportedly died on Thursday, Dec. 22, 2016.
|
"In fact, the (re-)discovery of this novel structural principle was made in 1948-49 by a young American artist whom Koleichuk also mentions, Kenneth Snelson. In the summer of 1948, Snelson had gone to study with Joseph Albers who was then teaching at Black Mountain College. . . . One of the first works he made upon his return home was Early X Piece which he dates to December 1948 . . . . "
— "In the Laboratory of Constructivism:
Karl Ioganson's Cold Structures,"
by Maria Gough, OCTOBER Magazine, MIT,
Issue 84, Spring 1998, pp. 91-117
|

The word "constructivism" also refers to a philosophy of mathematics.
See a Log24 post, "Constructivist Witness," of 1 AM ET on the above
date of death.
Comments Off on Early X Piece
Friday, December 23, 2016
From a Dec. 21 obituary posted by the
University of Tennessee at Knoxville —
"Wade was ordained as a pastor and served
at Oakwood Baptist Church in Knoxville."
Other information —

In a Log24 post, "Seeing the Finite Structure,"
of August 16, 2008, Wade appeared as a co-author
of the Walsh series book mentioned above —

Walsh Series: An Introduction
to Dyadic Harmonic Analysis,
by F. Schipp et al.,
Taylor & Francis, 1990
From the 2008 post —
The patterns on the faces of the cube on the cover
of Walsh Series above illustrate both the
Walsh functions of order 3 and the same structure
in a different guise, subspaces of the affine 3-space
over the binary field. For a note on the relationship
of Walsh functions to finite geometry, see
Symmetry of Walsh Functions.
Comments Off on Requiem for a Mathematician
Friday, December 9, 2016
See Ballet Blanc in this journal.
For a darker perspective, click on the image below.

See also Cartier in The Hexagon of Opposition.
Happy birthday to Kirk Douglas.

Comments Off on Snow Dance
Wednesday, December 7, 2016

Commentary —
|
"The close relationships between group theory and structural combinatorics go back well over a century. Given a combinatorial object, it is natural to consider its automorphism group. Conversely, given a group, there may be a nice object upon which it acts. If the group is given as a group of permutations of some set, it is natural to try to regard the elements of that set as the points of some structure which can be at least partially visualized. For example, in 1861 Mathieu… discovered five multiply transitive permutation groups. These were constructed as groups of permutations of 11, 12, 22, 23 or 24 points, by means of detailed calculations. In a little-known 1931 paper of Carmichael [5], they were first observed to be automorphism groups of exquisite finite geometries. This fact was rediscovered soon afterwards by Witt [11], who provided direct constructions for the groups and then the geometries. It is now more customary to construct first the designs, and then the groups…."
5. R. D. Carmichael, Tactical configurations of rank two,
Amer. J. Math. 53 (1931), 217-240.
11. E. Witt, Die 5-fach transitiven Gruppen von Mathieu,
Abh. Hamburg 12 (1938), 256-264.
— William M. Kantor, book review (pdf),
Bulletin of the American Mathematical Society, September 1981
|
Comments Off on Emch as a Forerunner of S(5, 8, 24)
Wednesday, November 30, 2016
|
Excerpts from James C. Nohrnberg, "The Master of the Myth of Literature: An Interpenetrative Ogdoad for Northrop Frye," Comparative Literature Vol. 53, No. 1 (Winter, 2001), pp. 58-82
From page 58 —
"… the posthumously revealed Notebooks. A major project of the latter was his 'Ogdoad': two groups of four books each. '[T]he second group of four […] were considered to be Blakean "emanations" or counterparts of the first four,' like 'the "double mirror" structure of The Great Code and Words with Power : two inter-reflecting parts of four chapters apiece,' Michael Dolzani reports.* "
* P. 22 of Rereading Frye: The Published and Unpublished Works , ed. David Boyd and Imre Salusinszky, Frye Studies [series] (Toronto: University of Toronto Press, 1998). [Abbreviated as RF .]
From page 62 —
"Visionaries like Blake and dramatists like Wagner seem to be working from some larger, mythic blueprint present in nuce from very early on."
From page 63 —
"Frye's hypothetical books and will-to-totality were obviously fruitful; if the beckoning star was illusory, it nonetheless settled on a real birthplace. The sought-for constructs substituted their scaffolding for a backbone-like confidence in pre-given beliefs; possession of the latter is why Tories like Dr. Johnson and T.S. Eliot could do quite nicely without the constructs. Frye's largely imaginary eightfold roman may have provided him a personal substitute— or alternative— for both ideology and myth."
From page 69 —
"For Frye the chief element of imaginative or expressive form is the myth, which functions structurally in literature like geometric shapes in painting."
From page 71 —
"The metaphysical skyhook lifting the artist free from unreflective social commitment is often a latent or manifest archetype that his work renews or reworks."
From page 77 —
"Frye's treatises— so little annotated themselves— are the notes writ large; the notes in the Notebooks are treatises writ small. They interpenetrate. Denham quotes 'the masters of the T'ien-tai school of Mahayana Buddhism' as saying '[t]he whole world is contained in a mustard seed' (RF 158, 160), and Frye quotes Keats: 'Every point of thought is the center of an intellectual world' (Study 159; cf. Great Code 167-68 and AC 61). …. [Frye’s] complex books were all generated out of the monadic obiter dicta . His kingdom 'is like a grain of mustard seed, which a man took, and cast into his garden, and it grew' (Luke 13:18-19)."
|
Comments Off on In Nuce
Wednesday, November 23, 2016
From the American Mathematical Society (AMS) webpage today —

From the current AMS Notices —

Related material from a post of Aug. 6, 2014 —

(Here "five point sets" should be "five-point sets.")
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”

The above symplectic structure* now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
* The phrase as used here is a deliberate
abuse of language . For the real definition of
“symplectic structure,” see (for instance)
“Symplectic Geometry,” by Ana Cannas da Silva
(article written for Handbook of Differential
Geometry , Vol 2.) To establish that the above
figure is indeed symplectic , see the post
Zero System of July 31, 2014.
Comments Off on Yogiism
Friday, October 28, 2016
|
Abstract:
"Protection of digital content from being tapped by intruders is a crucial task in the present generation of Internet world. In this paper, we proposed an implementation of new visual secret sharing scheme for gray level images using diamond theorem correlation. A secret image has broken into 4 × 4 non overlapped blocks and patterns of diamond theorem are applied sequentially to ensure the secure image transmission. Separate diamond patterns are utilized to share the blocks of both odd and even sectors. Finally, the numerical results show that a novel secret shares are generated by using diamond theorem correlations. Histogram representations demonstrate the novelty of the proposed visual secret sharing scheme."
— "New visual secret sharing scheme for gray-level images using diamond theorem correlation pattern structure," by V. Harish, N. Rajesh Kumar, and N. R. Raajan.
Published in: 2016 International Conference on Circuit, Power and Computing Technologies (ICCPCT).
Date of Conference: 18-19 March 2016. Publisher: IEEE.
Date Added to IEEE Xplore: 04 August 2016
|
Excerpts —

Related material — Posts tagged Diamond Theorem Correlation.
Comments Off on Diamond-Theorem Application
Friday, September 30, 2016
The author of the review in the previous post, Dara Horn, supplies
below a midrash on "desmic," a term derived from the Greek desmé
( δέσμη: bundle, sheaf , or, in the mathematical sense, pencil —
French faisceau ), which is related to the term desmos , bond …

(The term "desmic," as noted earlier, is relevant to the structure of
Heidegger's Sternwürfel .)
The Horn midrash —

(The "medieval philosopher" here is not the remembered pre-Christian
Ben Sirah (Ecclesiasticus ) but the philosopher being read — Maimonides:
Guide for the Perplexed , 3:51.)
Here of course "that bond" may be interpreted as corresponding to the
Greek desmos above, thus also to the desmic structure of the
stellated octahedron, a sort of three-dimensional Star of David.
See "desmic" in this journal.
Comments Off on Desmic Midrash
Wednesday, September 28, 2016

See also in this journal "desmic," a term related
to the structure of Heidegger's Sternwürfel .
Comments Off on Star Wars
Tuesday, September 27, 2016
Or: Philosophy for Jews
From a New Yorker weblog post dated Dec. 6, 2012 —
"Happy Birthday, Noam Chomsky" by Gary Marcus—
"… two titans facing off, with Chomsky, as ever,
defining the contest"
"Chomsky sees himself, correctly, as continuing
a conversation that goes back to Plato, especially
the Meno dialogue, in which a slave boy is
revealed by Socrates to know truths about
geometry that he hadn’t realized he knew."
Socrates and the slave boy discussed a rather elementary "truth
about geometry" — A diamond inscribed in a square has area 2
(and side the square root of 2) if the square itself has area 4
(and side 2).

Consider that not-particularly-deep structure from the Meno dialogue
in the light of the following…


The following analysis of the Meno diagram from yesterday's
post "The Embedding" contradicts the Lévi-Strauss dictum on
the impossibility of going beyond a simple binary opposition.
(The Chinese word taiji denotes the fundamental concept in
Chinese philosophy that such a going-beyond is both useful
and possible.)
The matrix at left below represents the feminine yin principle
and the diamond at right represents the masculine yang .
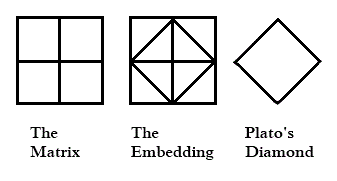
From a post of Sept. 22,
"Binary Opposition Illustrated" —

A symbol of the unity of yin and yang —

Related material:
A much more sophisticated approach to the "deep structure" of the
Meno diagram —

The larger cases —

Comments Off on Chomsky and Lévi-Strauss in China
Thursday, September 22, 2016

Click the above image for remarks on
"deep structure" and binary opposition.
See also the eightfold cube.
Comments Off on Binary Opposition Illustrated
Tuesday, September 20, 2016
Comments Off on Savage Logic
Saturday, September 17, 2016
The late Edward Albee, as quoted today in The Telegraph :
“I tell my students, if you want to know something
about the structure of a play, listen to some Bach
preludes and fugues. I discovered classical music
when I was eight, nine, 10 years old, and I think
I learnt something about the nature of dramatic
structure from the nature of the music I was
listening to. I probably think of myself half the
time as a composer.”
See also Box as Bach's.
Comments Off on Composition
Friday, September 16, 2016
Wittgenstein, 1939

Dolgachev and Keum, 2002

For some related material, see posts tagged Priority.
Comments Off on A Counting-Pattern
Monday, September 12, 2016
The previous post quoted Tom Wolfe on Chomsky's use of
the word "array."
An example of particular interest is the 4×4 array
(whether of dots or of unit squares) —

 .
.
Some context for the 4×4 array —
The following definition indicates that the 4×4 array, when
suitably coordinatized, underlies the Kummer lattice .

Further background on the Kummer lattice:
Alice Garbagnati and Alessandra Sarti,
"Kummer Surfaces and K3 surfaces
with $(Z/2Z)^4$ symplectic action."
To appear in Rocky Mountain J. Math. —

The above article is written from the viewpoint of traditional
algebraic geometry. For a less traditional view of the underlying
affine 4-space from finite geometry, see the website
Finite Geometry of the Square and Cube.
Some further context …
"To our knowledge, the relation of the Golay code
to the Kummer lattice … is a new observation."
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of
Kummer surfaces in the Mathieu group M24 "

As noted earlier, Taormina and Wendland seem not to be aware of
R. W. H. T. Hudson's use of the (uncoordinatized*) 4×4 array in his
1905 book Kummer's Quartic Surface. The array was coordinatized,
i.e. given a "vector space structure," by Cullinane eight years prior to
the cited remarks of Curtis.
* Update of Sept. 14: "Uncoordinatized," but parametrized by 0 and
the 15 two-subsets of a six-set. See the post of Sept. 13.
Comments Off on The Kummer Lattice
Wednesday, September 7, 2016
"May, / The months [sic ] of understanding" — Wallace Stevens
|
"If pure mathematics does spring from sub-conscious intuitions— already deep-structured as are grammatical patterns in the transformational-generative theory of language?— if the algebraic operation arises from wholly internalized pattern-weaving, how then can it, at so many points, mesh with, correspond to, the material forms of the world?"
— Steiner, George. Grammars of Creation
(Gifford Lectures, 1990). (Kindle Locations 2494-2496).
Open Road Media. Kindle Edition.
|
Good question.
See Bedtime Story (Sept. 1, 2016).
Comments Off on Grammar and Patterns
Sunday, September 4, 2016
Quoted here at 10 PM Pacific Time on Friday night —
"If I should die before I wake,
All my bone and sinew take
Put me in the compost pile
To decompose me for a while . . . ."
— Poem by Lee Hays



Comments Off on Piled High and Deep
Saturday, September 3, 2016
For the Church of Synchronology, a correction of
a recent New York Times obituary by Daniel Lewis —
Actor Gene Wilder died early Monday, Aug. 29, not, as
earlier reported, late Sunday, Aug. 28.


See also the last Log24 post of Sunday night, Aug. 28 (Angles of Vision)
and the first post of Monday morning, Aug. 29, 2016 (Roll Credits).
* For some reading related to the title, see an Evil Genius page
by the late David Lavery mentioning Colin Wilson's novel
The Mind Parasites . Great entertainment for the tinfoil-hat crowd —
"More and more I feel like the narrator of Colin Wilson's
The Mind Parasites , a phenomenologist who, along with
a dedicated group of compatriots, struggles clandestinely
to overthrow alien invaders that have secretly
taken captive the 'deep structure' of the human mind."
Comments Off on Phenomenology*
Friday, August 12, 2016
Peter Galison, a Harvard professor, is a defender of
the Vienna Circle and the religion of Scientism.
From Galison's “Structure of Crystal, Bucket of Dust,” in
Circles Disturbed: The Interplay of Mathematics and Narrative ,
edited by Apostolos Doxiadis and Barry Mazur, pp. 52-78
(Princeton: Princeton U. Press, 2012) …
Galison's final paragraph —
"Perhaps, then, it should not surprise us too much if,
as Wheeler approaches the beginning-end of all things,
there is a bucket of Borelian dust. Out of this filth,
through the proposition machine of quantum mechanics
comes pregeometry; pregeometry makes geometry;
geometry gives rise to matter and the physical laws
and constants of the universe. At once close to and far
from the crystalline story that Bourbaki invoked,
Wheeler’s genesis puts one in mind of Genesis 3:19:
'In the sweat of thy face shalt thou eat bread, till thou
return unto the ground; for out of it wast thou taken:
for dust thou art, and unto dust shalt thou return.' "
For fans of Scientism who prefer more colorful narratives —

Comments Off on Dustbucket Physics
Thursday, July 14, 2016
(Continued)
|
"Poincaré said that science is no more a collection of facts than a house is a collection of bricks. The facts have to be ordered or structured, they have to fit a theory, a construct (often mathematical) in the human mind.
… Mathematics may be art, but to the general public it is a black art, more akin to magic and mystery. This presents a constant challenge to the mathematical community: to explain how art fits into our subject and what we mean by beauty.
In attempting to bridge this divide I have always found that architecture is the best of the arts to compare with mathematics. The analogy between the two subjects is not hard to describe and enables abstract ideas to be exemplified by bricks and mortar, in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics"
in the AMS Notices , January 2010
|
A post from this journal later in 2010 —

The above post's date — May 20, 2010 — was
the date of death for mathematician Walter Rudin.
The above post from that date has a link to the
Heinlein story "And He Built a Crooked House."
A not-so-crooked house —

Comments Off on Midnight Special
Tuesday, July 5, 2016
(See previous posts now tagged Apple Tree Children.)

See as well the comic book in "Midnight Special" —

(Image previously posted in "Common Core vs. Central Structure")
Comments Off on For the Children in the Apple Tree (continued)
Sunday, July 3, 2016
Notes for a monkey grammarian —
"Visual forms— lines, colors, proportions, etc.—
are just as capable of articulation ,
i.e. of complex combination, as words.
But the laws that govern this sort of articulation
are altogether different from the laws of syntax
that govern language. The most radical difference
is that visual forms are not discursive .
They do not present their constituents successively,
but simultaneously, so the relations determining
a visual structure are grasped in one act of vision."
— Susanne K. Langer, Philosophy in a New Key
See also Langer's New Key in this journal.
Related material —

Comments Off on Articulation
Thursday, June 23, 2016
From a web page —
Breaking the Code of the Archetypal Self:
An Introductory Overview of the Research Discoveries
Leading to Neo-Jungian Structural Psychoanalysis
Dr. Moore will introduce his research and discoveries
with regard to the deep structures of the Self.
Tracing the foundations in the tradition of Jung’s
affirmation of the collective unconscious, Moore
will present his “decoding of the Diamond Body,”
a mapping of the deep structures of the Great Code
of the psyche. . . .
From the same web site —

Googling "Jung" + "Diamond Body" shows that
Moore's terminology differs from Jung's.
The octahedron that Moore apparently associates
with his "diamond body" was discussed by Jung
in a different context. See selections from Ch. 14
of Jung's Aion : "The Structure and Dynamics of the Self."
Dr. Moore appears as well in the murder-suicide story
of last night's 11:18 PM ET post.
For the relevance of Aion to "deep structures,"
see Jung + Diamond + Structure in this journal
and, more specifically, "Deep Structure."
Comments Off on Raiders of the Lost Code
Monday, June 20, 2016
See …
At the Still Point … (February 12, 2008)
For Balanchine's Birthday (January 9, 2007)
Go Set a Structure (Various dates)
and …

Comments Off on Plan 9 Continues
Tuesday, June 14, 2016
The title refers to the previous post, which quotes a
remark by a poetry critic in the current New Yorker .
Scholia —
From the post Structure and Sense of June 6, 2016 —
Structure

Sense

From the post Design Cube of July 23, 2015 —
Broken Symmetries in Diamond Space

Comments Off on Model Kit
Wednesday, May 25, 2016
From "Projective Geometry and PT-Symmetric Dirac Hamiltonian,"
Y. Jack Ng and H. van Dam,
Physics Letters B , Volume 673, Issue 3,
23 March 2009, Pages 237–239
(http://arxiv.org/abs/0901.2579v2, last revised Feb. 20, 2009)
" Studies of spin-½ theories in the framework of projective geometry
have been undertaken before. See, e.g., Ref. [4]. 1 "
" 1 These papers are rather mathematical and technical.
The authors of the first two papers discuss the Dirac equation
in terms of the Plucker-Klein correspondence between lines of
a three-dimensional projective space and points of a quadric
in a five-dimensional projective space. The last paper shows
that the Dirac equation bears a certain relation to Kummer’s
surface, viz., the structure of the Dirac ring of matrices is
related to that of Kummer’s 166 configuration . . . ."
[4]
O. Veblen
Proc. Natl. Acad. Sci. USA , 19 (1933), p. 503
Full Text via CrossRef
E.M. Bruins
Proc. Nederl. Akad. Wetensch. , 52 (1949), p. 1135
F.C. Taylor Jr., Master thesis, University of North Carolina
at Chapel Hill (1968), unpublished
A remark of my own on the structure of Kummer’s 166 configuration . . . .
See that structure in this journal, for instance —

See as well yesterday morning's post.
Comments Off on Kummer and Dirac
Thursday, May 19, 2016
From a check tonight of The New York Review of Books —

These NYRB stories from May 15 and May 13 suggest a
review of images on Ratner's Star and on the Eye of God.

Above image reposted from Jan. 10, 2014
|
I. The structures in the Diamond Puzzle…

Click on image for Jungian background.
II: The structure on a recent cover of Semiotica…

|
Above images reposted from May 5, 2016
Related material: The previous post, Dueling Formulas.
Comments Off on Kulturkampf
Thursday, May 5, 2016
Excerpt from a post of November 4, 2009 —
|
I. The structures in the Diamond Puzzle…

Click on image for Jungian background.
II: The structure on a recent cover of Semiotica…

|
For some related material, see a search
for Solomon Marcus in this journal.
Comments Off on Solomon’s Seal
Tuesday, April 26, 2016
"… I would drop the keystone into my arch …."
— Charles Sanders Peirce, "On Phenomenology"

" 'But which is the stone that supports the bridge?' Kublai Khan asks."
— Italo Calvino, Invisible Cities, as quoted by B. Elan Dresher.
(B. Elan Dresher. Nordlyd 41.2 (2014): 165-181,
special issue on Features edited by Martin Krämer,
Sandra Ronai and Peter Svenonius. University of Tromsø –
The Arctic University of Norway.
http://septentrio.uit.no/index.php/nordlyd)
Peter Svenonius and Martin Krämer, introduction to the
Nordlyd double issue on Features —
"Interacting with these questions about the 'geometric'
relations among features is the algebraic structure
of the features."
For another such interaction, see the previous post.
This post may be viewed as a commentary on a remark in Wikipedia —
"All of these ideas speak to the crux of Plato's Problem…."
See also The Diamond Theorem at Tromsø and Mere Geometry.
Comments Off on Interacting
Monday, April 25, 2016
An old version of the Wikipedia article "Group theory"
(pictured in the previous post) —

"More poetically …"
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
The seven seals from the previous post, with some context —

These models of projective points are drawn from the underlying
structure described (in the 4×4 case) as part of the proof of the
Cullinane diamond theorem .
Comments Off on Seven Seals
Sunday, April 17, 2016
The New York Times philosophy column yesterday —

The Times's philosophy column "The Stone" is named after the legendary
"philosophers' stone." The column's name, and the title of its essay yesterday
"Is that even a thing?" suggest a review of the eightfold cube as "The object
most closely resembling a 'philosophers' stone' that I know of" (Page 51 of
the current issue of a Norwegian art quarterly, KUNSTforum.as).
The eightfold cube —

|
Definition of Epiphany
From James Joyce’s Stephen Hero , first published posthumously in 1944. The excerpt below is from a version edited by John J. Slocum and Herbert Cahoon (New York: New Directions Press, 1959).
Three Times:
… By an epiphany he meant a sudden spiritual manifestation, whether in the vulgarity of speech or of gesture or in a memorable phase of the mind itself. He believed that it was for the man of letters to record these epiphanies with extreme care, seeing that they themselves are the most delicate and evanescent of moments. He told Cranly that the clock of the Ballast Office was capable of an epiphany. Cranly questioned the inscrutable dial of the Ballast Office with his no less inscrutable countenance:
— Yes, said Stephen. I will pass it time after time, allude to it, refer to it, catch a glimpse of it. It is only an item in the catalogue of Dublin’s street furniture. Then all at once I see it and I know at once what it is: epiphany.
— What?
— Imagine my glimpses at that clock as the gropings of a spiritual eye which seeks to adjust its vision to an exact focus. The moment the focus is reached the object is epiphanised. It is just in this epiphany that I find the third, the supreme quality of beauty.
— Yes? said Cranly absently.
— No esthetic theory, pursued Stephen relentlessly, is of any value which investigates with the aid of the lantern of tradition. What we symbolise in black the Chinaman may symbolise in yellow: each has his own tradition. Greek beauty laughs at Coptic beauty and the American Indian derides them both. It is almost impossible to reconcile all tradition whereas it is by no means impossible to find the justification of every form of beauty which has ever been adored on the earth by an examination into the mechanism of esthetic apprehension whether it be dressed in red, white, yellow or black. We have no reason for thinking that the Chinaman has a different system of digestion from that which we have though our diets are quite dissimilar. The apprehensive faculty must be scrutinised in action.
— Yes …
— You know what Aquinas says: The three things requisite for beauty are, integrity, a wholeness, symmetry and radiance. Some day I will expand that sentence into a treatise. Consider the performance of your own mind when confronted with any object, hypothetically beautiful. Your mind to apprehend that object divides the entire universe into two parts, the object, and the void which is not the object. To apprehend it you must lift it away from everything else: and then you perceive that it is one integral thing, that is a thing. You recognise its integrity. Isn’t that so?
— And then?
— That is the first quality of beauty: it is declared in a simple sudden synthesis of the faculty which apprehends. What then? Analysis then. The mind considers the object in whole and in part, in relation to itself and to other objects, examines the balance of its parts, contemplates the form of the object, traverses every cranny of the structure. So the mind receives the impression of the symmetry of the object. The mind recognises that the object is in the strict sense of the word, a thing , a definitely constituted entity. You see?
— Let us turn back, said Cranly.
They had reached the corner of Grafton St and as the footpath was overcrowded they turned back northwards. Cranly had an inclination to watch the antics of a drunkard who had been ejected from a bar in Suffolk St but Stephen took his arm summarily and led him away.
— Now for the third quality. For a long time I couldn’t make out what Aquinas meant. He uses a figurative word (a very unusual thing for him) but I have solved it. Claritas is quidditas . After the analysis which discovers the second quality the mind makes the only logically possible synthesis and discovers the third quality. This is the moment which I call epiphany. First we recognise that the object is one integral thing, then we recognise that it is an organised composite structure, a thing in fact: finally, when the relation of the parts is exquisite, when the parts are adjusted to the special point, we recognise that it is that thing which it is. Its soul, its whatness, leaps to us from the vestment of its appearance. The soul of the commonest object, the structure of which is so adjusted, seems to us radiant. The object achieves its epiphany.
Having finished his argument Stephen walked on in silence. He felt Cranly’s hostility and he accused himself of having cheapened the eternal images of beauty. For the first time, too, he felt slightly awkward in his friend’s company and to restore a mood of flippant familiarity he glanced up at the clock of the Ballast Office and smiled:
— It has not epiphanised yet, he said.
|
Comments Off on The Thing and I
Monday, April 4, 2016
Foreword by Sir Michael Atiyah —
"Poincaré said that science is no more a collection of facts
than a house is a collection of bricks. The facts have to be
ordered or structured, they have to fit a theory, a construct
(often mathematical) in the human mind. . . .
… Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to
explain how art fits into our subject and what we mean by beauty.
In attempting to bridge this divide I have always found that
architecture is the best of the arts to compare with mathematics.
The analogy between the two subjects is not hard to describe
and enables abstract ideas to be exemplified by bricks and mortar,
in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics"
in the AMS Notices , January 2010
Judy Bass, Los Angeles Times , March 12, 1989 —
"Like Rubik's Cube, The Eight demands to be pondered."
As does a figure from 1984, Cullinane's Cube —

For natural group actions on the Cullinane cube,
see "The Eightfold Cube" and
"A Simple Reflection Group of Order 168."
See also the recent post Cube Bricks 1984 —

Related remark from the literature —

Note that only the static structure is described by Felsner, not the
168 group actions discussed by Cullinane. For remarks on such
group actions in the literature, see "Cube Space, 1984-2003."
(From Anatomy of a Cube, Sept. 18, 2011.)
Comments Off on Cube for Berlin
Friday, April 1, 2016
Comments Off on Wonders of the Invisible World
Friday, March 4, 2016

Related aesthetics —
"Poincaré said that science is no more a collection of facts
than a house is a collection of bricks. The facts have to be
ordered or structured, they have to fit a theory, a construct
(often mathematical) in the human mind. . . .
… Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to
explain how art fits into our subject and what we mean by beauty.
In attempting to bridge this divide I have always found that
architecture is the best of the arts to compare with mathematics.
The analogy between the two subjects is not hard to describe
and enables abstract ideas to be exemplified by bricks and mortar,
in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics"
in the AMS Notices , January 2010
Comments Off on Cube Bricks 1984
Sunday, February 21, 2016
Reposted from an excellent weblog —
|
Thursday, April 2, 2009
Nabokov on Index Cards
I came across a recent post on Nabokov's Index Cards by Michael Leddy, which I found interesting.
Nabokov wrote with Index Cards not so much because they allowed associative progression (or because they were somehow like precursors of hypertext for him), but rather because he had such a clear vision of what he meant to create that he could start anywhere in describing it: "The pattern of the thing precedes the thing. I fill in the gaps of the crossword at any spot I happen to choose. These bits I write on index cards until the novel is done. My schedule is flexible but I am rather particular about my instruments: lined Bristol cards and well-sharpened, not too hard, pencils capped with erasers."
"… Since this entire structure, dimly illumined in one's mind, can be compared to a painting, and since you do not have to work gradually from left to right for its proper perception, I may direct my flashlight at any part or particle of the picture when setting it down in writing. I do not begin my novel at the beginning I do not reach chapter three before I reach chapter four… This is why I like writing my stories and novels on index cards, numbering them later when the whole set is complete. Every card is rewritten many times …"
"find a quiet spot (pace the booming surf and rattling wind) where to write. This I do on scrambled index cards (my text existing already there in invisible lead) which I gradually fill in and sort out, using up in the process more pencil sharpeners than pencils; but I have spoken of this in several earlier questionnaires"
Posted by MK at 8:52 PM
Labels: Index cards, Writing
|
From the date of the above Taking Note post, a post from this weblog
seems a suitable sermon for the Church of Synchronology.
Comments Off on Cards
Friday, February 19, 2016
The title refers to the Watchman Rorschach in "Go Set a Structure"
and to Christopher Nolan, director of the 2014 film "Interstellar."
"Watchmen"-like art in next Sunday's NY Times Book Review —

Comments Off on A Watchman for Nolan
Saturday, January 30, 2016
From page 56 of The Science Fiction of Mark Clifton ,
Southern Illinois University Press, 1980 —

See also the following image in this journal —
 .
.
Comments Off on Pope’s Geometry
Saturday, January 2, 2016
The New Yorker on March 2, 1992 —


Related material: Go Set a Structure.
Comments Off on A Very Strange Enchanted Town
Sunday, November 15, 2015
Anyone who clicked on the Dirac search at the end of
the previous post, "Dirac's Diamond," may wonder why the
"Solomon's Cube" post of 11 AM Sunday, March 1, 2009,
appeared in the Dirac search results, since there is no
apparent mention of Dirac in that Sunday post.
Use the source —
<!– See also "a linear transformation of V6… which preserves
the Klein quadric; in this way we arrive at the isomorphism of
Sym(8) withthe full orthogonal group O+(6; 2)." in "The
Classification of Flats in PG(9,2) which are External to the
Grassmannian G1,4,2 Authors: Shaw, Ron;
 Maks, Johannes; Gordon, Neil; Source: Designs,
Codes and Cryptography, Volume 34, Numbers 2-3, February
2005 , pp. 203-227; Publisher: Springer.  For more details,
see "Finite Geometry, Dirac Groups and the Table of Real
Clifford Algebras," by R. Shaw (U. of Hull), pp. 59-99 in
Clifford Algebras and Spinor Structures, by By Albert
Crumeyrolle, Rafał Abłamowicz, Pertti Lounesto,
published by Springer, 1995. –>
Comments Off on The Diamond and the Cube
Saturday, November 7, 2015
The previous post quoted a passage from Turing's Cathedral ,
a 2012 book by George Dyson —

It should be noted that Dyson's remarks on "two species of
bits," space, time, "structure and sequence" and logic gates
are from his own idiosyncratic attempt to create a philosophy
based on the workings of computers. These concepts are not,
so far as I can tell, part of anyone else's approach to the subject.
For a more standard introduction to how computers work, see
(for instance) a book by an author Dyson admires:
The Pattern on the Stone , by W. Daniel Hillis (Basic Books, 1998).
PREFACE: MAGIC IN THE STONE
I etch a pattern of geometric shapes onto a stone.
To the uninitiated, the shapes look mysterious and
complex, but I know that when arranged correctly
they will give the stone a special power, enabling it
to respond to incantations in a language no human
being has ever spoken. I will ask the stone questions
in this language, and it will answer by showing me a
vision: a world created by my spell, a world imagined
within the pattern on the stone.
A few hundred years ago in my native New England,
an accurate description of my occupation would have
gotten me burned at the stake. Yet my work involves
no witchcraft; I design and program computers. The
stone is a wafer of silicon, and the incantations are
software. The patterns etched on the chip and the
programs that instruct the computer may look
complicated and mysterious, but they are generated
according to a few basic principles that are easily
explained. . . . .
Hillis's title suggests some remarks unrelated to computers —
See Philosopher + Stone in this journal.
Comments Off on Clarifying Dyson
Saturday, October 31, 2015
Continues.

Addendum —
Comments Off on Weaving World…
Thursday, October 22, 2015
Software writer Richard P. Gabriel describes some work of design
philosopher Christopher Alexander in the 1960's at Harvard:

A more interesting account of these 35 structures:
"It is commonly known that there is a bijection between
the 35 unordered triples of a 7-set [i.e., the 35 partitions
of an 8-set into two 4-sets] and the 35 lines of PG(3,2)
such that lines intersect if and only if the corresponding
triples have exactly one element in common."
— "Generalized Polygons and Semipartial Geometries,"
by F. De Clerck, J. A. Thas, and H. Van Maldeghem,
April 1996 minicourse, example 5 on page 6.
For some context, see Eightfold Geometry by Steven H. Cullinane.
Comments Off on Objective Quality
Thursday, October 15, 2015
The title is a phrase from R. D. Laing's book The Politics of Experience .
(Published in the psychedelic year 1967. The later "contrapuntal interweaving"
below is of a less psychedelic nature.)
An illustration of the "interweaving' part of the title —
The "deep structure" of the diamond theorem:
 .
.
The word "symplectic" from the end of last Sunday's (Oct. 11) sermon
describes the "interwoven" nature of the above illustration.
An illustration of the "contrapuntal" part of the title (click to enlarge):

Comments Off on Contrapuntal Interweaving
Monday, October 5, 2015
Comments Off on Forms that Rhyme:
Monday, September 28, 2015
From a post of July 24, 2011 —

A review —
“The story, involving the Knights Templar, the Vatican, sunken treasure,
the fate of Christianity and a decoding device that looks as if it came out of
a really big box of medieval Cracker Jack, is the latest attempt to combine
Indiana Jones derring-do with ‘Da Vinci Code’ mysticism.”
— The New York Times
A feeble attempt at a purely mathematical "decoding device"
from this journal earlier this month —

For some background, see a question by John Baez at Math Overflow
on Aug. 20, 2015.
The nonexistence of a 24-cycle in the large Mathieu group
might discourage anyone hoping for deep new insights from
the above figure.
See Marston Conder's "Symmetric Genus of the Mathieu Groups" —

Comments Off on Cracker Jack Prize
Saturday, September 19, 2015
The latest Visual Insight post at the American Mathematical
Society website discusses group actions on the McGee graph,
pictured as 24 points arranged in a circle that are connected
by 36 symmetrically arranged edges.
Wikipedia remarks that …
"The automorphism group of the McGee graph
is of order 32 and doesn't act transitively upon
its vertices: there are two vertex orbits of lengths
8 and 16."
The partition into 8 and 16 points suggests, for those familiar
with the Miracle Octad Generator and the Mathieu group M24,
the following exercise:
Arrange the 24 points of the projective line
over GF(23) in a circle in the natural cyclic order
( ∞, 1, 2, 3, … , 22, 0 ). Can the McGee graph be
modeled by constructing edges in any natural way?

In other words, if the above set of edges has no
"natural" connection with the 24 points of the
projective line over GF(23), does some other
set of edges in an isomorphic McGee graph
have such a connection?
Update of 9:20 PM ET Sept. 20, 2015:
Backstory: A related question by John Baez
at Math Overflow on August 20.
Comments Off on Geometry of the 24-Point Circle
Tuesday, September 8, 2015
Fareed Zakaria in an online Aug. 21
New York Times book review —
" Most intellectuals think ideas matter.
In one of his most famous and oft-quoted lines,
John Maynard Keynes declared, 'Practical men
who believe themselves to be quite exempt from
any intellectual influence are usually the slaves
of some defunct economist. Madmen in authority,
who hear voices in the air, are distilling their frenzy
from some academic scribbler of a few years back.'
Scott L. Montgomery and Daniel Chirot concur,
arguing that ideas 'do not merely matter; they matter
immensely, as they have been the source for decisions
and actions that have structured the modern world.'
In The Shape of the New: Four Big Ideas and How
They Made the Modern World , Montgomery and
Chirot make the case for the importance of four
powerful ideas, rooted in the European Enlightenment,
that have created the world as we know it.
'Invading armies can be resisted,' they quote
Victor Hugo. 'Invading ideas cannot be.' "
* Related material: Point Omega , a book
by Don DeLillo, in this journal.
Comments Off on Point Omega*
Tuesday, August 18, 2015
The phrase “the permutation group Sn” refers to a
particular group of permutations that act on an
n -element set N— namely, all of them. For a given n ,
there are, in general, many permutation groups that
act on N. All but one are smaller than Sn .
In other words, the phrase “the permutation group Sn”
does not imply that “Sn ” is a symbol for a structure
associated with n called “the permutation group.”
It is instead a symbol for “the symmetric group,” the largest
of (in general) many permutation groups that act on N.
This point seems to have escaped John Baez.
For two misuses by Baez of the phrase “permutation group” at the
n-Category Café, see “A Wrinkle in the Mathematical Universe”
and “Re: A Wrinkle…” —


“There is such a thing as a permutation group.”
— Adapted from A Wrinkle in Time , by Madeleine L’Engle
Comments Off on A Wrinkle in Terms
Wednesday, August 5, 2015
Sunday, July 19, 2015

See also last night's post.
The above passage was found in a search for thoughts of Heinz Pagels
on "perfect symmetry" (the title of one of his books). The "If all" part is,
however, apparently not by Pagels. That part seems to have been
online only in an NYU file that can no longer be accessed.
For perfect symmetry with structure, see (for instance)
Go Set a Structure (July 14, 2015) and Tombstone (July 16, 2015).
Comments Off on Sunday School
Thursday, July 16, 2015
The black rectangle at the end of Example 1.4
is known as the "end-of-proof symbol," "Halmos,"
or "tombstone."

Comments Off on Tombstone
Thursday, July 9, 2015
(Continued)
A post of July 7, Haiku for DeLillo, had a link to posts tagged "Holy Field GF(3)."
As the smallest Galois field based on an odd prime, this structure
clearly is of fundamental importance.

It is, however, perhaps too small to be visually impressive.
A larger, closely related, field, GF(9), may be pictured as a 3×3 array…
… hence as the traditional Chinese Holy Field.
Marketing the Holy Field

The above illustration of China's Holy Field occurred in the context of
Log24 posts on Child Buyers. For more on child buyers, see an excellent
condemnation today by Diane Ravitch of the U. S. Secretary of Education.
Comments Off on Man and His Symbols
Friday, July 3, 2015

A figure I prefer to the "Golden Tablet" of Night at the Museum —

The source — The Log24 post "Zero System" of July 31, 2014.
* For the title, see The New Yorker of Sept. 22, 2014.
Comments Off on Crunching Entities*
Monday, April 27, 2015
A comment on the Scholarly Kitchen piece from
this morning's previous post —

This suggests …
The Beast from Hell's Kitchen

For some thoughts on mapping trees into
linear arrays, see The Forking (March 20, 2015).
See also Pitchfork in this journal.
Comments Off on The Beast from Hell’s Kitchen
Thursday, April 23, 2015
(A sequel to yesterday's ART WARS and this
morning's De Colores )
“Perhaps the philosophically most relevant feature
of modern science is the emergence of abstract
symbolic structures as the hard core of objectivity
behind– as Eddington puts it– the colorful tale
of the subjective storyteller mind.” — Hermann Weyl
(Philosophy of Mathematics and Natural Science ,
Princeton, 1949, p. 237)
See also Deathly Hallows.
Comments Off on Colorful Tale
Saturday, April 4, 2015
Holy Saturday is, according to tradition, the day of
the harrowing of Hell.
Notes:


The above passage on "Die Figuren der vier Modi
im Magischen Quadrat " should be read in the context of
a Log24 post from last year's Devil's Night (the night of
October 30-31). The post, "Structure," indicates that, using
the transformations of the diamond theorem, the notorious
"magic" square of Albrecht Dürer may be transformed
into normal reading order. That order is only one of
322,560 natural reading orders for any 4×4 array of
symbols. The above four "modi" describe another.
Comments Off on Harrowing of Hell (continued)
Thursday, March 26, 2015
The incidences of points and planes in the
Möbius 84 configuration (8 points and 8 planes,
with 4 points on each plane and 4 planes on each point),
were described by Coxeter in a 1950 paper.*
A table from Monday's post summarizes Coxeter's
remarks, which described the incidences in
spatial terms, with the points and planes as the vertices
and face-planes of two mutually inscribed tetrahedra —
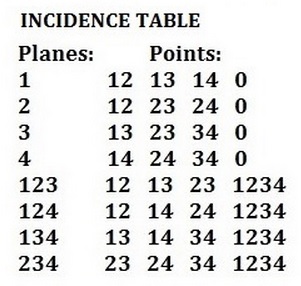
Monday's post, "Gallucci's Möbius Configuration,"
may not be completely intelligible unless one notices
that Coxeter has drawn some of the intersections in his
Fig. 24, a schematic representation of the point-plane
incidences, as dotless, and some as hollow dots. The figure,
"Gallucci's version of Möbius's 84," is shown below.
The hollow dots, representing the 8 points (as opposed
to the 8 planes ) of the configuration, are highlighted in blue.

Here a plane (represented by a dotless intersection) contains
the four points that are represented in the square array as lying
in the same row or same column as the plane.
The above Möbius incidences appear also much earlier in
Coxeter's paper, in figures 6 and 5, where they are shown
as describing the structure of a hypercube.
In figures 6 and 5, the dotless intersections representing
planes have been replaced by solid dots. The hollow dots
have again been highlighted in blue.


Figures 6 and 5 demonstrate the fact that adjacency in the set of
16 vertices of a hypercube is isomorphic to adjacency in the set
of 16 subsquares of a square 4×4 array, provided that opposite
sides of the array are identified, as in Fig. 6. The digits in
Coxeter's labels above may be viewed as naming the positions
of the 1's in (0,1) vectors (x4, x3, x2, x1) over the two-element
Galois field.† In that context, the 4×4 array may be called, instead
of a Möbius hypercube , a Galois tesseract .
* "Self-Dual Configurations and Regular Graphs,"
Bulletin of the American Mathematical Society,
Vol. 56 (1950), pp. 413-455
† The subscripts' usual 1-2-3-4 order is reversed as a reminder
that such a vector may be viewed as labeling a binary number
from 0 through 15, or alternately as labeling a polynomial in
the 16-element Galois field GF(24). See the Log24 post
Vector Addition in a Finite Field (Jan. 5, 2013).
Comments Off on The Möbius Hypercube
Tuesday, March 24, 2015
Yesterday's post suggests a review of the following —
|
Andries Brouwer, preprint, 1982:
"The Witt designs, Golay codes and Mathieu groups"
(unpublished as of 2013)
Pages 8-9:
Substructures of S(5, 8, 24)
An octad is a block of S(5, 8, 24).
Theorem 5.1
Let B0 be a fixed octad. The 30 octads disjoint from B0
form a self-complementary 3-(16,8,3) design, namely
the design of the points and affine hyperplanes in AG(4, 2),
the 4-dimensional affine space over F2.
Proof….
… (iv) We have AG(4, 2).
(Proof: invoke your favorite characterization of AG(4, 2)
or PG(3, 2), say Dembowski-Wagner or Veblen & Young.
An explicit construction of the vector space is also easy….)
|
Related material: Posts tagged Priority.
Comments Off on Brouwer on the Galois Tesseract
Friday, March 20, 2015
(Continued)
An article in the new April issue of Notices of the American
Mathmatical Society suggests a search for connections
between the Calkin-Wilf tree and the modular group.

The search yields, for instance (in chronological order) …
"Cutting sequences for geodesic flow on the modular surface
and continued fractions," David J. Grahinet, Jeffrey C. Lagaria,
arXiv, 2 April 2001
"Orderings of the rationals and dynamical systems,"
Claudio Bonanno, Stefano Isola, arXiv, 14 May 2008.
"Periods of negative-regular continued fractions. Rational numbers."
Sergey Khrushchev and Michael Tyaglov, slides PDF, 11 Sept. 2012
"The Minkowski ?(x) function, a class of singular measures,
theta-constants, and mean-modular forms," Giedrius Alkauskas,
arXiv, 20 Sept. 2012
"Forests of complex numbers,"
Melvyn B. Nathanson, arXiv, 1 Dec. 2014
Update of March 21, 2015:
For many more related papers, search by combining the
phrase "modular group" with phrases denoting forking structures
other than Calkin-Wilf, such as "cubic tree," "Stern-Brocot tree,"
and "Farey tree" (or "Farey sequence" or "Farey series" or
"Farey graph" ).
Comments Off on The Forking
Thursday, February 12, 2015
Continued from yesterday.
The passage on Claude Chevalley quoted here
yesterday in the post Dead Reckoning was, it turns out,
also quoted by Peter Galison in his essay "Structure of Crystal,
Bucket of Dust" in Circles Disturbed: The Interplay of
Mathematics and Narrative (Princeton University Press, 2012,
ed. by Apostolos Doxiadis and Barry Mazur).
Galison gives a reference to his source:
"From 'Claude Chevalley Described by His Daughter (1988),'
in Michèle Chouchan, Nicolas Bourbaki: Faits et légendes
(Paris: Éditions du Choix, 1995), 36–40, translated and cited
in Marjorie Senechal, 'The Continuing Silence of Bourbaki:
An Interview with Pierre Cartier, June 18, 1997,'
Mathematical Intelligencer 1 (1998): 22–28."
Galison's essay compares Chevalley with the physicist
John Archibald Wheeler. His final paragraph —
"Perhaps, then, it should not surprise us too much if,
as Wheeler approaches the beginning-end of all things,
there is a bucket of Borelian dust. Out of this filth,
through the proposition machine of quantum mechanics
comes pregeometry; pregeometry makes geometry;
geometry gives rise to matter and the physical laws
and constants of the universe. At once close to and far
from the crystalline story that Bourbaki invoked,
Wheeler’s genesis puts one in mind of Genesis 3:19:
'In the sweat of thy face shalt thou eat bread, till thou
return unto the ground; for out of it wast thou taken:
for dust thou art, and unto dust shalt thou return.'"
See also posts tagged Wheeler.
Comments Off on Dead Reckoning
Wednesday, February 11, 2015
Continued from yesterday evening

Today's mathematical birthday —
Claude Chevalley, 11 Feb. 1909 – 28 June 1984.
From MacTutor —
|
Chevalley's daughter, Catherine Chevalley, wrote about
her father in "Claude Chevalley described by his daughter"
(1988):—
For him it was important to see questions as a whole, to see the necessity of a proof, its global implications. As to rigour, all the members of Bourbaki cared about it: the Bourbaki movement was started essentially because rigour was lacking among French mathematicians, by comparison with the Germans, that is the Hilbertians. Rigour consisted in getting rid of an accretion of superfluous details. Conversely, lack of rigour gave my father an impression of a proof where one was walking in mud, where one had to pick up some sort of filth in order to get ahead. Once that filth was taken away, one could get at the mathematical object, a sort of crystallized body whose essence is its structure. When that structure had been constructed, he would say it was an object which interested him, something to look at, to admire, perhaps to turn around, but certainly not to transform. For him, rigour in mathematics consisted in making a new object which could thereafter remain unchanged.
The way my father worked, it seems that this was what counted most, this production of an object which then became inert— dead, really. It was no longer to be altered or transformed. Not that there was any negative connotation to this. But I must add that my father was probably the only member of Bourbaki who thought of mathematics as a way to put objects to death for aesthetic reasons.
|
Recent scholarly news suggests a search for Chapel Hill
in this journal. That search leads to Transformative Hermeneutics.
Those who, like Professor Eucalyptus of Wallace Stevens's
New Haven, seek God "in the object itself" may contemplate
yesterday's afternoon post on Eightfold Design in light of the
Transformative post and of yesterday's New Haven remarks and
Chapel Hill events.
Comments Off on Dead Reckoning
Thursday, January 1, 2015
An image that led off the year-end review yesterday in
the weblog of British combinatorialist Peter J. Cameron:

See also this weblog's post final post of 2014,
with a rectangular array illustrating the six faces
of a die, and Cameron's reference yesterday to
a die-related post…
"The things on my blog that seem to be
of continuing value are the expository
series like the one on the symmetric group
(the third post in this series was reblogged
by Gil Kalai last month, which gave it a new
lease of life)…."
A tale from an author of Prague:
|
The Emperor—so they say—has sent a message, directly from his death bed, to you alone, his pathetic subject, a tiny shadow which has taken refuge at the furthest distance from the imperial sun. He ordered the herald to kneel down beside his bed and whispered the message into his ear. He thought it was so important that he had the herald repeat it back to him. He confirmed the accuracy of the verbal message by nodding his head. And in front of the entire crowd of those who’ve come to witness his death—all the obstructing walls have been broken down and all the great ones of his empire are standing in a circle on the broad and high soaring flights of stairs—in front of all of them he dispatched his herald. The messenger started off at once, a powerful, tireless man. Sticking one arm out and then another, he makes his way through the crowd. If he runs into resistance, he points to his breast where there is a sign of the sun. So he moves forward easily, unlike anyone else. But the crowd is so huge; its dwelling places are infinite. If there were an open field, how he would fly along, and soon you would hear the marvelous pounding of his fist on your door. But instead of that, how futile are all his efforts. He is still forcing his way through the private rooms of the innermost palace. He will never he win his way through. And if he did manage that, nothing would have been achieved. He would have to fight his way down the steps, and, if he managed to do that, nothing would have been achieved. He would have to stride through the courtyards, and after the courtyards the second palace encircling the first, and, then again, stairs and courtyards, and then, once again, a palace, and so on for thousands of years. And if he finally did burst through the outermost door—but that can never, never happen—the royal capital city, the centre of the world, is still there in front of him, piled high and full of sediment. No one pushes his way through here, certainly not with a message from a dead man. But you sit at your window and dream of that message when evening comes.
|
See also a passage quoted in this weblog on the original
date of Cameron's Prague image, July 26, 2014 —
"The philosopher Graham Harman is invested in
re-thinking the autonomy of objects and is part
of a movement called Object-Oriented-Philosophy
(OOP)." — From “The Action of Things,” a 2011
M.A. thesis at the Center for Curatorial Studies,
Bard College, by Manuela Moscoso
— in the context of a search here for the phrase
"structure of the object." An image from that search:

Comments Off on New Year’s Greeting from Franz Kafka
Saturday, December 27, 2014

The Ball-Weiner date above, 5 September 2011,
suggests a review of this journal on that date —

"Think of a DO NOT ENTER pictogram,
a circle with a diagonal slash, a type of ideogram.
It tells you what to do or not do, but not why.
The why is part of a larger context, a bigger picture."
— Customer review at Amazon.com
This passage was quoted here on August 10, 2009.
Also from that date:

The Sept. 5, 2011, Ball-Weiner paper illustrates the
"doily" view of the mathematical structure W(3,2), also
known as GQ(2,2), the Sp(4,2) generalized quadrangle.
(See Fig. 3.1 on page 33, exercise 13 on page 38, and
the answer to that exercise on page 55, illustrated by
Fig. 5.1 on page 56.)
For "another view, hidden yet true," of GQ(2,2),
see Inscape and Symplectic Polarity in this journal.
Comments Off on More To Be Done
Monday, December 15, 2014
Today’s 8:01 PM post quoted Husserl on
the perception of the cube.
Another approach to perception of the cube,
from Narrative Metaphysics on St. Lucia’s Day —
From today’s 11:29 AM post —
John Burt Foster Jr. in Nabokov’s Art of Memory and
European Modernism (Princeton U. Press, 1993, p. 224) —
At the time of The Waste Land , in a comment on
Joyce’s Ulysses that influenced many later definitions
of modernism in the English-speaking world, Eliot
announced, “instead of narrative method, we may
now use the mythical method.”13
For some illuminating remarks on a mythical approach
to perception of the cube, see Gareth Knight on Schicksalstag 2012.
Comments Off on Mythic Metaphysics
Saturday, December 13, 2014
From "Guardians of the Galaxy" —

"Then the Universe exploded into existence…"
For those who prefer a more traditional approach :

See also Symplectic Structure and Stevens's Rock.
Comments Off on Narrative Metaphysics
Tuesday, December 2, 2014
Continued.
"Perhaps the philosophically most relevant feature
of modern science is the emergence of abstract
symbolic structures as the hard core of objectivity
behind— as Eddington puts it— the colorful tale
of the subjective storyteller mind."
— Hermann Weyl in Philosophy of Mathematics
and Natural Science , Princeton, 1949, p. 237
Tom Wolfe on art theorists in The Painted Word (1975) :
"It is important to repeat that Greenberg and Rosenberg
did not create their theories in a vacuum or simply turn up
with them one day like tablets brought down from atop
Green Mountain or Red Mountain (as B. H. Friedman once
called the two men). As tout le monde understood, they
were not only theories but … hot news,
straight from the studios, from the scene."
The Weyl quote is a continuing theme in this journal.
The Wolfe quote appeared here on Nov. 18, 2014,
the reported date of death of Yale graduate student
Natasha Chichilnisky-Heal.
Directions to her burial (see yesterday evening) include
a mention of "Paul Robson Street" (actually Paul
Robeson Place) near "the historic Princeton Cemetery."
This, together with the remarks by Tom Wolfe posted
here on the reported day of her death, suggests a search
for "red green black" —


The late Chichilnisky-Heal was a student of political economy.
The search colors may be interpreted, if one likes, as referring
to politics (red), economics (green), and Robeson (black).
See also Robeson in this journal.
Comments Off on Colorful Tale
Monday, December 1, 2014
Flashback to St. Andrew's Day, 2013 —
If the object is a cube, change arises from the fact
that the object has six faces…

and is the unit cell for the six -dimensional
hyperspace H over the two-element field —

A different representation of the unit cell of
the hyperspace H (and of the I Ching ) —

Comments Off on Change Arises
Sunday, November 30, 2014
Reality's Mirror: Exploring the Mathematics of Symmetry —
"Here is a book that explains in laymen language
what symmetry is all about, from the lowliest snowflake
and flounder to the lofty group structures whose
astonishing applications to the Old One are winning
Nobel prizes. Bunch's book is a marvel of clear, witty
science writing, as delightful to read as it is informative
and up-to-date. The author is to be congratulated on
a job well done." — Martin Gardner
"But, sweet Satan, I beg of you, a less blazing eye!"
— Rimbaud, A Season in Hell
"… the lowliest snowflake and flounder…."
— Martin Gardner


Comments Off on View from the Bottom
Thursday, November 13, 2014
Yesterday's post and recent Hollywood news suggest
a meditation on a Progressive Matrix —
Oct. 12-14, 2005:


Click to enlarge.
"My card."
Structurally related images —
A sample Raven's Progressive Matrices test item
(such items share the 3×3 structure of the hash symbol above):

Structural background —

Comments Off on Progressive Matrix
Monday, November 3, 2014
From a post of June 3, 2013:
New Yorker editor David Remnick at Princeton today
(from a copy of his prepared remarks):
“Finally, speaking of fabric design….”
I prefer Tom and Harold:
Tom Wolfe in The Painted Word —
“I am willing (now that so much has been revealed!)
to predict that in the year 2000, when the Metropolitan
or the Museum of Modern Art puts on the great
retrospective exhibition of American Art 1945-75,
the three artists who will be featured, the three seminal
figures of the era, will be not Pollock, de Kooning, and
Johns-but Greenberg, Rosenberg, and Steinberg.
Up on the walls will be huge copy blocks, eight and a half
by eleven feet each, presenting the protean passages of
the period … a little ‘fuliginous flatness’ here … a little
‘action painting’ there … and some of that ‘all great art
is about art’ just beyond. Beside them will be small
reproductions of the work of leading illustrators of
the Word from that period….”
Harold Rosenberg in The New Yorker (click to enlarge)—

From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
Symplectic :

— Steven H. Cullinane,
diamond theorem illustration
Comments Off on The Rhetoric of Abstract Concepts
Friday, October 31, 2014
See a University of Wisconsin obituary for Schneider,
a leading expert on linear algebra who reportedly died
at 87 on Tuesday, October 28, 2014.
Some background on linear algebra and “magic” squares:
tonight’s 3 AM (ET) post and a search in this
journal for Knight, Death, and the Devil.

Click image to enlarge.
Comments Off on For the Late Hans Schneider
Tuesday, October 28, 2014
Part I:
Part II:

Click images for some context.
Comments Off on Figural Processing
Tuesday, October 21, 2014
Two news items on art as a tool:


Two Log24 posts related to the 3×3 grid, the underlying structure for China’s
ancient Lo Shu “magic” square:


Finally, leftist art theorist Rosalind Krauss in this journal
on AntiChristmas, 2010:

Which is the tool here, the grid or Krauss?
Comments Off on Art as a Tool
Monday, October 13, 2014
Parallelograms and the structure of the 3×3 array —
Click to enlarge:

A different approach to parallelograms and arrays —
Click for original post:

Comments Off on Sallows on “The Lost Theorem”
Monday, October 6, 2014
(Continued from Beautiful Mathematics, Dec. 14, 2013)
“Seemingly unrelated structures turn out to have
mysterious correspondences.” — Jim Holt, opening
paragraph of a book review in the Dec. 5, 2013, issue
of The New York Review of Books
One such correspondence:

For bibliographic information and further details, see
the March 9, 2014, update to “Beautiful Mathematics.”
See as well posts from that same March 9 now tagged “Story Creep.”
Comments Off on Mysterious Correspondences
Sunday, September 21, 2014
“Why Is Our Sci-Fi So Glum About A.I.?,”
by Jayson Greene, NY Times Sunday Magazine today —
“You come to pity these advanced beings, bumping against
the dunderheaded constraints that their less-advanced
creators have placed on them. Johansson’s Lucy grows so
powerful as her cerebral capacity multiplies that she is able
to manipulate her cellular structure. And yet, when pursued
by an entire planet’s worth of law enforcement, she settles
on a disguise straight out of Saturday-morning cartoons —
really big sunglasses and a hairdo change.”
See also this journal on Saturday morning for a definition, and
Geometry of the I Ching for examples, of …
changeable, instantiable entities, i.e., concrete universals.

Comments Off on Saturday-Morning Concept
« Newer Posts —
Older Posts »











































































































 .
.

































































































