See also other Log24 posts tagged Dirac and Geometry.
Saturday, November 12, 2022
Thursday, June 21, 2018
Dirac and Geometry (continued)
"Just fancy a scale model of Being
made out of string and cardboard."
— Nanavira Thera, 1 October 1957,
on a model of Kummer's Quartic Surface
mentioned by Eddington
"… a treatise on Kummer's quartic surface."
The "super-mathematician" Eddington did not see fit to mention
the title or the author of the treatise he discussed.
See Hudson + Kummer in this journal.
See also posts tagged Dirac and Geometry.
Comments Off on Dirac and Geometry (continued)
Wednesday, May 25, 2016
Kummer and Dirac
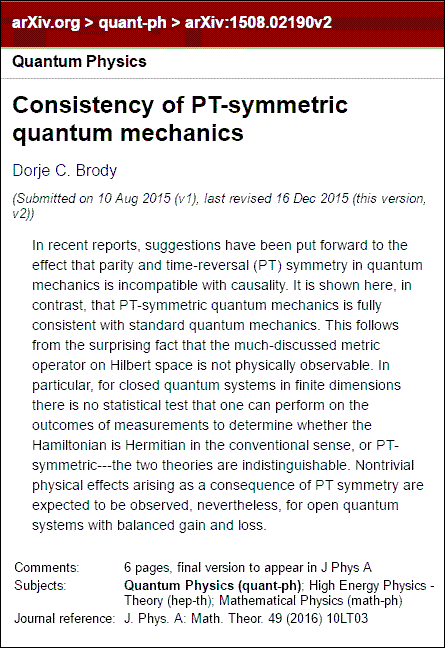
From "Projective Geometry and PT-Symmetric Dirac Hamiltonian,"
Y. Jack Ng and H. van Dam,
Physics Letters B , Volume 673, Issue 3,
23 March 2009, Pages 237–239
(http://arxiv.org/abs/0901.2579v2, last revised Feb. 20, 2009)
" Studies of spin-½ theories in the framework of projective geometry
have been undertaken before. See, e.g., Ref. [4]. 1 "
" 1 These papers are rather mathematical and technical.
The authors of the first two papers discuss the Dirac equation
in terms of the Plucker-Klein correspondence between lines of
a three-dimensional projective space and points of a quadric
in a five-dimensional projective space. The last paper shows
that the Dirac equation bears a certain relation to Kummer’s
surface, viz., the structure of the Dirac ring of matrices is
related to that of Kummer’s 166 configuration . . . ."
[4]
O. Veblen
Proc. Natl. Acad. Sci. USA , 19 (1933), p. 503
Full Text via CrossRef
E.M. Bruins
Proc. Nederl. Akad. Wetensch. , 52 (1949), p. 1135
F.C. Taylor Jr., Master thesis, University of North Carolina
at Chapel Hill (1968), unpublished
A remark of my own on the structure of Kummer’s 166 configuration . . . .
See as well yesterday morning's post.
Comments Off on Kummer and Dirac
Monday, December 14, 2015
Dirac and Geometry
See a post by Peter Woit from Sept. 24, 2005 — Dirac's Hidden Geometry.
The connection, if any, with recent Log24 posts on Dirac and Geometry
is not immediately apparent. Some related remarks from a novel —
|
From Broken Symmetries by Paul Preuss "He pondered the source of her fascination with the occult, which sooner or later seemed to entangle a lot of thoughtful people who were not already mired in establishmentarian science or religion. It was the religious impulse, at base. Even reason itself could function as a religion, he supposed— but only for those of severely limited imagination. He’d toyed with 'psi' himself, written a couple of papers now much quoted by crackpots, to his chagrin. The reason he and so many other theoretical physicists were suckers for the stuff was easy to understand— for two-thirds of a century an enigma had rested at the heart of theoretical physics, a contradiction, a hard kernel of paradox. Quantum theory was inextricable from the uncertainty relations. The classical fox knows many things, but the quantum-mechanical hedgehog knows only one big thing— at a time. 'Complementarity,' Bohr had called it, a rubbery notion the great professor had stretched to include numerous pairs of opposites. Peter Slater was willing to call it absurdity, and unlike some of his older colleagues who, following in Einstein’s footsteps, demanded causal explanations for everything (at least in principle), Peter had never thirsted after 'hidden variables' to explain what could not be pictured. Mathematical relationships were enough to satisfy him, mere formal relationships which existed at all times, everywhere, at once. It was a thin nectar, but he was convinced it was the nectar of the gods. The psychic investigators, on the other hand, demanded to know how the mind and the psychical world were related. Through ectoplasm, perhaps? Some fifth force of nature? Extra dimensions of spacetime? All these naive explanations were on a par with the assumption that psi is propagated by a species of nonlocal hidden variables, the favored explanation of sophisticates; ignotum per ignotius . 'In this connection one should particularly remember that the human language permits the construction of sentences which do not involve any consequences and which therefore have no content at all…' The words were Heisenberg’s, lecturing in 1929 on the irreducible ambiguity of the uncertainty relations. They reminded Peter of Evan Harris Walker’s ingenious theory of the psi force, a theory that assigned psi both positive and negative values in such a way that the mere presence of a skeptic in the near vicinity of a sensitive psychic investigation could force null results. Neat, Dr. Walker, thought Peter Slater— neat, and totally without content. One had to be willing to tolerate ambiguity; one had to be willing to be crazy. Heisenberg himself was only human— he’d persuasively woven ambiguity into the fabric of the universe itself, but in that same set of 1929 lectures he’d rejected Dirac’s then-new wave equations with the remark, 'Here spontaneous transitions may occur to the states of negative energy; as these have never been observed, the theory is certainly wrong.' It was a reasonable conclusion, and that was its fault, for Dirac’s equations suggested the existence of antimatter: the first antiparticles, whose existence might never have been suspected without Dirac’s crazy results, were found less than three years later. Those so-called crazy psychics were too sane, that was their problem— they were too stubborn to admit that the universe was already more bizarre than anything they could imagine in their wildest dreams of wizardry." |
Particularly relevant …
"Mathematical relationships were enough to satisfy him,
mere formal relationships which existed at all times,
everywhere, at once."
Some related pure mathematics —
Comments Off on Dirac and Geometry
Monday, November 23, 2015
Dirac and Line Geometry
Some background for my post of Nov. 20,
"Anticommuting Dirac Matrices as Skew Lines" —
His earlier paper that Bruins refers to, "Line Geometry
and Quantum Mechanics," is available in a free PDF.
For a biography of Bruins translated by Google, click here.
For some additional historical background going back to
Eddington, see Gary W. Gibbons, "The Kummer
Configuration and the Geometry of Majorana Spinors,"
pages 39-52 in Oziewicz et al., eds., Spinors, Twistors,
Clifford Algebras, and Quantum Deformations:
Proceedings of the Second Max Born Symposium held
near Wrocław, Poland, September 1992 . (Springer, 2012,
originally published by Kluwer in 1993.)
For more-recent remarks on quantum geometry, see a
paper by Saniga cited in today's update to my Nov. 20 post.
Comments Off on Dirac and Line Geometry
Friday, November 20, 2015
Anticommuting Dirac Matrices as Skew Lines
(Continued from November 13)
The work of Ron Shaw in this area, ca. 1994-1995, does not
display explicitly the correspondence between anticommutativity
in the set of Dirac matrices and skewness in a line complex of
PG(3,2), the projective 3-space over the 2-element Galois field.
Here is an explicit picture —
References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Shaw, Ron, "Finite Geometry, Dirac Groups, and the Table of
Real Clifford Algebras," undated article at ResearchGate.net
Update of November 23:
See my post of Nov. 23 on publications by E. M. Bruins
in 1949 and 1959 on Dirac matrices and line geometry,
and on another author who gives some historical background
going back to Eddington.
Some more-recent related material from the Slovak school of
finite geometry and quantum theory —
The matrices underlying the Saniga paper are those of Pauli, not
those of Dirac, but these two sorts of matrices are closely related.
Comments Off on Anticommuting Dirac Matrices as Skew Lines
Thursday, November 19, 2015
Highlights of the Dirac-Mathieu Connection
For the connection of the title, see the post of Friday, November 13th, 2015.
For the essentials of this connection, see the following two documents —
Comments Off on Highlights of the Dirac-Mathieu Connection
Sunday, November 15, 2015
Dirac’s Diamond
The title refers to a memorial stone in Westminster Abbey —
Detail from the above image —
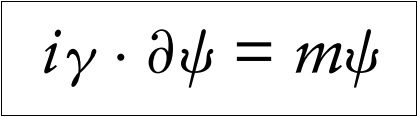
An alternate version from astrophysicist Peter Coles —
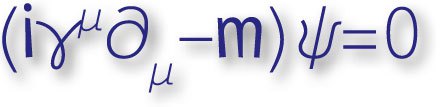
See also Dirac in this journal.
Comments Off on Dirac’s Diamond
Friday, November 13, 2015
A Connection between the 16 Dirac Matrices and the Large Mathieu Group
Note that the six anticommuting sets of Dirac matrices listed by Arfken
correspond exactly to the six spreads in the above complex of 15 projective
lines of PG(3,2) fixed under a symplectic polarity (the diamond theorem
correlation ). As I noted in 1986, this correlation underlies the Miracle
Octad Generator of R. T. Curtis, hence also the large Mathieu group.
References:
Arfken, George B., Mathematical Methods for Physicists , Third Edition,
Academic Press, 1985, pages 213-214
Cullinane, Steven H., Notes on Groups and Geometry, 1978-1986
Related material:
The 6-set in my 1986 note above also appears in a 1996 paper on
the sixteen Dirac matrices by David M. Goodmanson —
Background reading:
Ron Shaw on finite geometry, Clifford algebras, and Dirac groups
(undated compilation of publications from roughly 1994-1995)—
Comments Off on A Connection between the 16 Dirac Matrices and the Large Mathieu Group
Thursday, August 29, 2024
Fearful Symmetry
Peter Woit this afternoon on "The Terrifying Power of Mathematics" —
"… the quantum field theory of fields satisfying the Dirac equation.
Here there’s a standard apparatus of how to calculate given in
every quantum field theory textbook. These standard calculations
involving Dirac gamma-matrices fit well with Feynman’s 'physicists
finding they have the correct equations without understanding them
have been so terrified they give up trying to understand them'."
For a definition of these matrices, see . . .
Weisstein, Eric W. "Dirac Matrices."
From MathWorld — A Wolfram Web Resource.
https://mathworld.wolfram.com/DiracMatrices.html —
"The Dirac matrices are a class of 4×4 matrices which arise
in quantum electrodynamics. There are a variety of different
symbols used, and Dirac matrices are also known as
gamma matrices or Dirac gamma matrices."
For related religious remarks, see "Physics for Poets"
( Log24, April 20, 2022 ).
Comments Off on Fearful Symmetry
Thursday, October 5, 2023
For World Space Week
See also https://m759.net/wordpress/?s=Dirac+skew+anticommuting.
For fans of a different sort of space . . .
See also the Wikipedia article on Bloom.
Comments Off on For World Space Week
Monday, May 1, 2023
But Seriously . . . From the E. Street School
Comments Off on But Seriously . . . From the E. Street School
Sunday, February 19, 2023
Hoarding Space
Comments Off on Hoarding Space
Wednesday, April 20, 2022
Physics for Poets
Excerpt from a long poem by Eliza Griswold —
The square array above does not contain Arfken's variant
labels for ρ1, ρ2, and ρ3, although those variant labels were
included in Arfken's 1985 square array and in Arfken's 1985
list of six anticommuting sets, copied at MathWorld as above.
The omission of variant labels prevents a revised list of the
six anticommuting sets from containing more distinct symbols
than there are matrices.
Revised list of anticommuting sets:
α1 α2 α3 ρ2 ρ3
γ1 γ2 γ3 ρ1 ρ3
δ1 δ2 δ3 ρ1 ρ2
α1 γ1 δ1 σ2 σ3
α2 γ2 δ2 σ1 σ3
α3 γ3 δ3 σ1 σ2 .
Context for the poem: Quark Rock.
Context for the physics: Dirac Matrices.
Comments Off on Physics for Poets
Sunday, August 15, 2021
Simple Similarity
The following image (click to enlarge) is now the target of
a link on the phrase "similarly divided" in Friday's post
"The Divided Square."
Related material —
A version of the above Schroeder pages, dumbed down for
readers of The New Yorker —
Note that the proof under discussion has nothing to do with
the New Yorker 's rubric "Annals of Technology."
Note also the statement by Strogatz that
"Einstein’s proof reveals why the Pythagorean theorem
applies only to right triangles: they’re the only kind
made up of smaller copies of themselves."
Exercise: Discuss the truth or falsity of the Strogatz statement
after reviewing the webpage Triangles Are Square.
For approaches to geometry that are more advanced, see
this journal on the above New Yorker date — Nov. 19, 2015 —
Comments Off on Simple Similarity
Thursday, March 4, 2021
Continuity
Actually, Dirac “bridged math and physics” much earlier —
“Spinors, which are a kind of square root of vectors, had been introduced
in algebra and also in physics as part of Paul Dirac’s theory of the electron.
A spin structure on a manifold allows such square roots to exist.”
— Quanta Magazine today, article by Daniel S. Freed
See The Eddington Song and . . .
Poetic paraphrase —
“How can we tell the singer from the song?”
Comments Off on Continuity
Friday, February 12, 2021
Root
A star figure and the Galois quaternion.
The square root of the former is the latter.
(This post was suggested by the definition of
the Dirac operator as a square root.)
Comments Off on Root
Tuesday, March 24, 2020
The Amsterdam Connection
Comments Off on The Amsterdam Connection
Monday, October 21, 2019
Algebra and Space… Illustrated.
Related entertainment —
Detail:
George Steiner —
"Perhaps an insane conceit."
Perhaps.
See Quantum Tesseract Theorem .
Perhaps Not.
See Dirac and Geometry .
Comments Off on Algebra and Space… Illustrated.
Wednesday, October 9, 2019
The Joy of Six
Note that in the pictures below of the 15 two-subsets of a six-set,
the symbols 1 through 6 in Hudson's square array of 1905 occupy the
same positions as the anticommuting Dirac matrices in Arfken's 1985
square array. Similarly occupying these positions are the skew lines
within a generalized quadrangle (a line complex) inside PG(3,2).
Related narrative — The "Quantum Tesseract Theorem."
Comments Off on The Joy of Six
Friday, September 27, 2019
The Black List
"… Max Black, the Cornell philosopher, and others have pointed out
how 'perhaps every science must start with metaphor and end with
algebra, and perhaps without the metaphor there would never have
been any algebra' …."
— Max Black, Models and Metaphors, Cornell U. Press, 1962,
page 242, as quoted in Dramas, Fields, and Metaphors, by
Victor Witter Turner, Cornell U. Press, paperback, 1975, page 25
Metaphor —
Algebra —
|
The 16 Dirac matrices form six anticommuting sets of five matrices each (Arfken 1985, p. 214):
1.
2.
3.
4.
5.
6. SEE ALSO: Pauli Matrices REFERENCES: Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 211-217, 1985. Berestetskii, V. B.; Lifshitz, E. M.; and Pitaevskii, L. P. "Algebra of Dirac Matrices." §22 in Quantum Electrodynamics, 2nd ed. Oxford, England: Pergamon Press, pp. 80-84, 1982. Bethe, H. A. and Salpeter, E. Quantum Mechanics of One- and Two-Electron Atoms. New York: Plenum, pp. 47-48, 1977. Bjorken, J. D. and Drell, S. D. Relativistic Quantum Mechanics. New York: McGraw-Hill, 1964. Dirac, P. A. M. Principles of Quantum Mechanics, 4th ed. Oxford, England: Oxford University Press, 1982. Goldstein, H. Classical Mechanics, 2nd ed. Reading, MA: Addison-Wesley, p. 580, 1980. Good, R. H. Jr. "Properties of Dirac Matrices." Rev. Mod. Phys. 27, 187-211, 1955. Referenced on Wolfram|Alpha: Dirac Matrices CITE THIS AS: Weisstein, Eric W. "Dirac Matrices."
From MathWorld— A Wolfram Web Resource. |
, 
, 
, 
, 
,
, 
, 
, 
, 
,
, 
, 
, 
, 
,
, 
, 
, 
, 
,
, 
, 
, 
, 
,
, 
, 
, 
, 
.
Desiring the exhilarations of changes:
The motive for metaphor, shrinking from
The weight of primary noon,
The A B C of being,
The ruddy temper, the hammer
Of red and blue, the hard sound—
Steel against intimation—the sharp flash,
The vital, arrogant, fatal, dominant X.
Comments Off on The Black List
Saturday, August 10, 2019
Schoolgirl Space* Revisited:
The Square "Inscape" Model of
the Generalized Quadrangle W(2)
Click image to enlarge.
-embedding-in-PG(3,2)-Planat-Saniga-1000w.jpg)
* The title refers to the role of PG (3,2) in Kirkman's schoolgirl problem.
For some backstory, see my post Anticommuting Dirac Matrices as Skew Lines
and, more generally, posts tagged Dirac and Geometry.
Comments Off on Schoolgirl Space* Revisited:
Tuesday, July 16, 2019
Schoolgirl Space for Quantum Mystics
Comments Off on Schoolgirl Space for Quantum Mystics
Sunday, July 14, 2019
Old Pathways in Science:
The Quantum Tesseract Theorem Revisited
"The secret is that the super-mathematician expresses by the anticommutation
of his operators the property which the geometer conceives as perpendicularity
of displacements. That is why on p. 269 we singled out a pentad of anticommuting
operators, foreseeing that they would have an immediate application in describing
the property of perpendicular directions without using the traditional picture of space.
They express the property of perpendicularity without the picture of perpendicularity.
Thus far we have touched only the fringe of the structure of our set of sixteen E-operators.
Only by entering deeply into the theory of electrons could I show the whole structure
coming into evidence."
A related illustration, from posts tagged Dirac and Geometry —
Compare and contrast Eddington's use of the word "perpendicular"
with a later use of the word by Saniga and Planat.
Comments Off on Old Pathways in Science:
Monday, March 11, 2019
Overarching Metanarratives
See also "Overarching + Tesseract" in this journal. From the results
of that search, some context for the "inscape" of the previous post —
Comments Off on Overarching Metanarratives
Monday, February 18, 2019
Quantum Choreography
In the beginning of the 1954 film "Seven Brides for Seven Brothers,"
Adam, oldest of the brothers, is paired with Milly. The problem then is
to find brides for Adam's six brothers.
Above is a video illustration, published on Aug. 12, 2016, of the six couples.
Matching them involves some choreography.
See also this journal on Aug. 12, 2016 — "Dustbucket Physics" —
"… through the proposition machine of quantum mechanics
comes pregeometry; pregeometry makes geometry;
geometry gives rise to matter and the physical laws
and constants of the universe."
— Harvard professor Peter Galison,
defender of the faith of Scientism
For a different sort of quantum "proposition machine," see posts tagged
Dirac and Geometry.
Comments Off on Quantum Choreography
Wednesday, November 14, 2018
Tuesday, November 13, 2018
Blackboard Jungle Continues.
From the 1955 film "Blackboard Jungle" —
From a trailer for the recent film version of A Wrinkle in Time —
Detail of the phrase "quantum tesseract theorem":
From the 1962 book —
"There's something phoney
in the whole setup, Meg thought.
There is definitely something rotten
in the state of Camazotz."
Related mathematics from Koen Thas that some might call a
"quantum tesseract theorem" —
Some background —
See also posts tagged Dirac and Geometry. For more
background on finite geometry, see a web page
at Thas's institution, Ghent University.
Comments Off on Blackboard Jungle Continues.
Friday, July 6, 2018
Something
"… Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness."
— T. S. Eliot, "Burnt Norton," 1936
"Read something that means something."
— Advertising slogan for The New Yorker
The previous post quoted some mystic meditations of Octavio Paz
from 1974. I prefer some less mystic remarks of Eddington from
1938 (the Tanner Lectures) published by Cambridge U. Press in 1939 —
"… we have sixteen elements with which to form a group-structure" —
See as well posts tagged Dirac and Geometry.
Comments Off on Something
Tuesday, July 3, 2018
Lost in Quantum Space
Combining concepts from earlier posts today, we have the above title.
A more concise alternative title …
Lost in the Matrix
For some related non -fiction, see posts tagged Dirac and Geometry.
Comments Off on Lost in Quantum Space
Monday, March 12, 2018
“Quantum Tesseract Theorem?”
Remarks related to a recent film and a not-so-recent film.
For some historical background, see Dirac and Geometry in this journal.
Also (as Thas mentions) after Saniga and Planat —
The Saniga-Planat paper was submitted on December 21, 2006.
Excerpts from this journal on that date —
"Open the pod bay doors, HAL."
Comments Off on “Quantum Tesseract Theorem?”
Saturday, February 17, 2018
The Binary Revolution
Michael Atiyah on the late Ron Shaw —
Phrases by Atiyah related to the importance in mathematics
of the two-element Galois field GF(2) —
- “The digital revolution based on the 2 symbols (0,1)”
- “The algebra of George Boole”
- “Binary codes”
- “Dirac’s spinors, with their up/down dichotomy”
These phrases are from the year-end review of Trinity College,
Cambridge, Trinity Annual Record 2017 .
I prefer other, purely geometric, reasons for the importance of GF(2) —
- The 2×2 square
- The 2x2x2 cube
- The 4×4 square
- The 4x4x4 cube
See Finite Geometry of the Square and Cube.
See also today’s earlier post God’s Dice and Atiyah on the theology of
(Boolean) algebra vs. (Galois) geometry:
Comments Off on The Binary Revolution
Saturday, December 23, 2017
The Right Stuff
A figure related to the general connecting theorem of Koen Thas —
See also posts tagged Dirac and Geometry in this journal.
Those who prefer narrative to mathematics may, if they so fancy, call
the above Thas connecting theorem a "quantum tesseract theorem ."
Comments Off on The Right Stuff
Friday, December 22, 2017
Sunday, December 10, 2017
Geometry
See also Symplectic in this journal.
From Gotay and Isenberg, “The Symplectization of Science,”
Gazette des Mathématiciens 54, 59-79 (1992):
“… what is the origin of the unusual name ‘symplectic’? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure ‘line complex group’ the ‘symplectic group.’
… the adjective ‘symplectic’ means ‘plaited together’ or ‘woven.’
This is wonderfully apt….”
The above symplectic figure appears in remarks on
the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2). See also
related remarks on the notion of linear (or line ) complex
in the finite projective space PG(3,2) —
Comments Off on Geometry
Thursday, November 30, 2017
The Matrix for Quantum Mystics
Scholia on the title — See Quantum + Mystic in this journal.
"In Vol. I of Structural Anthropology , p. 209, I have shown that
this analysis alone can account for the double aspect of time
representation in all mythical systems: the narrative is both
'in time' (it consists of a succession of events) and 'beyond'
(its value is permanent)." — Claude Lévi-Strauss, 1976
I prefer the earlier, better-known, remarks on time by T. S. Eliot
in Four Quartets , and the following four quartets (from
The Matrix Meets the Grid) —
.
From a Log24 post of June 26-27, 2017:
A work of Eddington cited in 1974 by von Franz —
See also Dirac and Geometry and Kummer in this journal.
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
Comments Off on The Matrix for Quantum Mystics
Wednesday, November 29, 2017
Definitions
See also Inscape in this journal and, for a related Chapel Hill thesis,
the post Kummer and Dirac.
Comments Off on Definitions
Tuesday, October 10, 2017
Another 35-Year Wait
The title refers to today's earlier post "The 35-Year Wait."
A check of my activities 35 years ago this fall, in the autumn
of 1982, yields a formula I prefer to the nonsensical, but famous,
"canonical formula" of Claude Lévi-Strauss.
My "inscape" formula, from a note of Sept. 22, 1982 —
S = f ( f ( X ) ) .
Some mathematics from last year related to the 1982 formula —
See also Inscape in this journal and posts tagged Dirac and Geometry.
Comments Off on Another 35-Year Wait
Friday, June 30, 2017
Hurriedly Put Together
The previous post quoted one theologian on a book
by another theologian, saying its tone "is patronizing
and its arguments are hurriedly put together."
For a more leisurely sort of argument, see a 1995* remark
by a mathematician, Ronald Shaw, quoted here on the morning
of Tuesday, June 27, in an update at the end of the previous day's
post "Upgrading to Six" —
". . . recall the notions of Eddington (1936) . . . ."
* In "Finite Geometry, Dirac Groups and the
Table of Real Clifford Algebras," pages 59-99 of
R. Ablamowicz and P. Lounesto (eds.),
Clifford Algebras and Spinor Structures ,
Kluwer Academic Publishers, 1995.
Comments Off on Hurriedly Put Together
Monday, June 26, 2017
Upgrading to Six
This post was suggested by the previous post — Four Dots —
and by the phrase "smallest perfect" in this journal.
Related material (click to enlarge) —
Detail —
From the work of Eddington cited in 1974 by von Franz —
See also Dirac and Geometry and Kummer in this journal.
Updates from the morning of June 27 —
Ron Shaw on Eddington's triads "associated in conjugate pairs" —
For more about hyperbolic and isotropic lines in PG(3,2),
see posts tagged Diamond Theorem Correlation.
For Shaw, in memoriam — See Contrapuntal Interweaving and The Fugue.
Comments Off on Upgrading to Six
Tuesday, June 6, 2017
The Table
John Horgan and James (Jim) McClellan, according to Horgan
in Scientific American on June 1, 2017 —
|
Me: "Jim, you're a scholar! Professor! Esteemed historian of science! And yet you don’t really believe science is capable of producing truth." Jim: "Science is stories we tell about nature. And some stories are better than other stories. And you can compare stories to each other on all kinds of grounds, but you have no access to"— he pauses for dramatic effect— "The Truth. Or any mode of knowing outside of your own story-telling capabilities, which include rationality, experiment, explanatory scope and the whole thing. I would love to have some means of making knowledge about the world that would allow us to say, 'This is really it. There really are goddamn electrons.'" He whacks the table. |
See also posts tagged Dirac and Geometry and Glitch.
Comments Off on The Table
Tuesday, March 21, 2017
Special Topics
A roundup of posts now tagged "Apollo Psi" led to the name
Evan Harris Walker in the post Dirac and Geometry of
Dec. 14, 2015. That post mentions …
"… Evan Harris Walker’s ingenious theory of
the psi force, a theory that assigned psi
both positive and negative values in such a way
that the mere presence of a skeptic in the near
vicinity of a sensitive psychic investigation could
force null results. Neat, Dr. Walker, thought
Peter Slater— neat, and totally without content."
— From the 1983 novel Broken Symmetries
by Paul Preuss
It turns out that Walker died "on the evening of August 17, 2006."
From this journal on that date —
Comments Off on Special Topics
Wednesday, March 8, 2017
Inscapes
"The particulars of attention,
whether subjective or objective,
are unshackled through form,
and offered as a relational matrix …."
— Kent Johnson in a 1993 essay
|
The 16 Dirac matrices form six anticommuting sets of five matrices each (Arfken 1985, p. 214):
1.
2.
3.
4.
5.
6. SEE ALSO: Pauli Matrices REFERENCES: Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 211-217, 1985. Berestetskii, V. B.; Lifshitz, E. M.; and Pitaevskii, L. P. "Algebra of Dirac Matrices." §22 in Quantum Electrodynamics, 2nd ed. Oxford, England: Pergamon Press, pp. 80-84, 1982. Bethe, H. A. and Salpeter, E. Quantum Mechanics of One- and Two-Electron Atoms. New York: Plenum, pp. 47-48, 1977. Bjorken, J. D. and Drell, S. D. Relativistic Quantum Mechanics. New York: McGraw-Hill, 1964. Dirac, P. A. M. Principles of Quantum Mechanics, 4th ed. Oxford, England: Oxford University Press, 1982. Goldstein, H. Classical Mechanics, 2nd ed. Reading, MA: Addison-Wesley, p. 580, 1980. Good, R. H. Jr. "Properties of Dirac Matrices." Rev. Mod. Phys. 27, 187-211, 1955. Referenced on Wolfram|Alpha: Dirac Matrices CITE THIS AS: Weisstein, Eric W. "Dirac Matrices."
From MathWorld— A Wolfram Web Resource. |
Comments Off on Inscapes
Tuesday, November 22, 2016
Jargon
See "sacerdotal jargon" in this journal.
For those who prefer scientific jargon —
"… open its reading to
combinational possibilities
outside its larger narrative flow.
The particulars of attention,
whether subjective or objective,
are unshackled through form,
and offered as a relational matrix …."
— Kent Johnson in a 1993 essay
For some science that is not just jargon, see …
and, also from posts tagged Dirac and Geometry …
The above line complex also illustrates an outer automorphism
of the symmetric group S6. See last Thursday's post "Rotman and
the Outer Automorphism."
Comments Off on Jargon
Thursday, November 3, 2016
Triple Cross
(Continued … See the title in this journal, as well as Cube Bricks.)
Cube Bricks 1984 —
Related material —
Dirac and Geometry in this journal,
Kummer's Quartic Surface in this journal,
Nanavira Thera in this journal, and
The Razor's Edge and Nanavira Thera.
See as well Bill Murray's 1984 film "The Razor's Edge" …
Movie poster from 1984 —
"A thin line separates
love from hate,
success from failure,
life from death."
Three other dualities, from Nanavira Thera in 1959 —
"I find that there are, in every situation,
three independent dualities…."
(Click to enlarge.)
Comments Off on Triple Cross
Saturday, July 23, 2016
But Seriously …
Those who want a serious approach to the mathematics
of Clifford algebras — via finite geometry, the natural setting
of the four-group of the previous post — should consult
"Finite Geometry, Dirac Groups and the Table of
Real Clifford Algebras," by Ron Shaw (1995).
Comments Off on But Seriously …
Tuesday, July 12, 2016
Group Elements and Skew Lines
The following passage by Igor Dolgachev (Good Friday, 2003)
seems somewhat relevant (via its connection to Kummer's 166 )
to previous remarks here on Dirac matrices and geometry —
Note related remarks from E. M. Bruins in 1959 —
Comments Off on Group Elements and Skew Lines
Friday, June 3, 2016
Bruins and van Dam
A review of some recent posts on Dirac and geometry,
each of which mentions the late physicist Hendrik van Dam:
- Kummer and Dirac (May 25)
- Framework (May 25)
- Expanding the Spielraum (May 26)
- Dorje (May 26)
The first of these posts mentions the work of E. M. Bruins.
Some earlier posts that cite Bruins:
- Anticommuting Dirac Matrices as Skew Lines (Nov. 20, 2015)
- Dirac and Line Geometry (Nov. 23, 2015)
- Einstein and Geometry (Nov. 27, 2015)
Comments Off on Bruins and van Dam
Thursday, May 26, 2016
Dorje
Images suggested by the previous post —
Note the name "Dorje" in the first image above.
Remarks related to the name "Dorje," as well as to
"Projective Geometry and PT-Symmetric Dirac Hamiltonian,"
a 2009 paper by Y. Jack Ng and the late Hendrik van Dam —
Remarks for the Church of Synchronology from December 16, 2015,
the date of the above Dorje arXiv upload —
Comments Off on Dorje
Tuesday, May 24, 2016
Rosenhain and Göpel Revisited
The authors Taormina and Wendland in the previous post
discussed some mathematics they apparently did not know was
related to a classic 1905 book by R. W. H. T. Hudson, Kummer's
Quartic Surface .
"This famous book is a prototype for the possibility
of explaining and exploring a many-faceted topic of
research, without focussing on general definitions,
formal techniques, or even fancy machinery. In this
regard, the book still stands as a highly recommendable,
unparalleled introduction to Kummer surfaces, as a
permanent source of inspiration and, last but not least,
as an everlasting symbol of mathematical culture."
— Werner Kleinert, Mathematical Reviews ,
as quoted at Amazon.com
Some 4×4 diagrams from that book are highly relevant to the
discussion by Taormina and Wendland of the 4×4 squares within
the 1974 Miracle Octad Generator of R. T. Curtis that were later,
in 1987, described by Curtis as pictures of the vector 4-space over
the two-element Galois field GF(2).
Hudson did not think of his 4×4 diagrams as illustrating a vector space,
but he did use them to picture certain subsets of the 16 cells in each
diagram that he called Rosenhain and Göpel tetrads .
Some related work of my own (click images for related posts)—
Rosenhain tetrads as 20 of the 35 projective lines in PG(3,2)
Göpel tetrads as 15 of the 35 projective lines in PG(3,2)
Related terminology describing the Göpel tetrads above
Comments Off on Rosenhain and Göpel Revisited
Monday, February 8, 2016
A Game with Four Letters
Related material — Posts tagged Dirac and Geometry.
For an example of what Eddington calls "an open mind,"
see the 1958 letters of Nanavira Thera.
(Among the "Early Letters" in Seeking the Path ).
Comments Off on A Game with Four Letters
Wednesday, January 20, 2016
Fringe Physics and Beyond
"One day not long ago Oppenheimer stalked
up and down his office and divulged some
startling new discoveries about the 15 fundamental
particles of which the universe is made….
… physicists today are wondering if these particles
are themselves actually the final, stark, immutable
and indivisible foundation stones of the universe
that until now they have been thought to be."
—Lincoln Barnett in LIFE magazine,
Oct. 10, 1949, page 122
Fringe Physics —
"… astrophysics limits the number of fundamental particles to 15…."
— Franklin Potter at FQXi.org on Sep. 27, 2009
"I agree there can't be more than 15 fundamental particles."
— Lawrence B. Crowell at FQXi.org on Sep. 29, 2009
Beyond —
There are, at any rate, 15 "final, stark, immutable* and indivisible*
foundation stones" (namely, 15 points ) of the finite projective
space PG(3,2). See Symplectic in this journal.
For related physics, see posts tagged Dirac and Geometry.
* Update of Jan. 21, 2016 — I was carried away by Barnett's
powerful rhetoric. These adjectives are wrong.
Comments Off on Fringe Physics and Beyond
Monday, December 14, 2015
The Forking
From the previous post:
"Neat, Dr. Walker, thought Peter Slater—
neat, and totally without content."
— Paul Preuss's 1983 novel Broken Symmetries
A background check yields …
"Dr. Evan Harris Walker died on the evening of
August 17, 2006…."
A synchronicity check of that date in this journal yields a diagram
that, taken by itself, is "neat, and totally without content." —
The diagram may be viewed as a tribute
to the late Yogi Berra, to the literary
"Garden of Forking Paths," or, more
seriously, to the modular group Γ.
Comments Off on The Forking
Friday, November 27, 2015
Einstein and Geometry
(A Prequel to Dirac and Geometry)
"So Einstein went back to the blackboard.
And on Nov. 25, 1915, he set down
the equation that rules the universe.
As compact and mysterious as a Viking rune,
it describes space-time as a kind of sagging mattress…."
— Dennis Overbye in The New York Times online,
November 24, 2015
Some pure mathematics I prefer to the sagging Viking mattress —
Readings closely related to the above passage —
Thomas Hawkins, "From General Relativity to Group Representations:
the Background to Weyl's Papers of 1925-26," in Matériaux pour
l'histoire des mathématiques au XXe siècle: Actes du colloque
à la mémoire de Jean Dieudonné, Nice, 1996 (Soc. Math.
de France, Paris, 1998), pp. 69-100.
The 19th-century algebraic theory of invariants is discussed
as what Weitzenböck called a guide "through the thicket
of formulas of general relativity."
Wallace Givens, "Tensor Coordinates of Linear Spaces," in
Annals of Mathematics Second Series, Vol. 38, No. 2, April 1937,
pp. 355-385.
Tensors (also used by Einstein in 1915) are related to
the theory of line complexes in three-dimensional
projective space and to the matrices used by Dirac
in his 1928 work on quantum mechanics.
For those who prefer metaphors to mathematics —
Rota fails to cite the source of his metaphor.
|
Comments Off on Einstein and Geometry
Sunday, November 15, 2015
The Diamond and the Cube
Anyone who clicked on the Dirac search at the end of
the previous post, "Dirac's Diamond," may wonder why the
"Solomon's Cube" post of 11 AM Sunday, March 1, 2009,
appeared in the Dirac search results, since there is no
apparent mention of Dirac in that Sunday post.
<!– See also "a linear transformation of V6… which preserves
the Klein quadric; in this way we arrive at the isomorphism of
Sym(8) withthe full orthogonal group O+(6; 2)." in "The
Classification of Flats in PG(9,2) which are External to the
Grassmannian G1,4,2 Authors: Shaw, Ron;
 Maks, Johannes; Gordon, Neil; Source: Designs,
Codes and Cryptography, Volume 34, Numbers 2-3, February
2005 , pp. 203-227; Publisher: Springer.  For more details,
see "Finite Geometry, Dirac Groups and the Table of Real
Clifford Algebras," by R. Shaw (U. of Hull), pp. 59-99 in
Clifford Algebras and Spinor Structures, by By Albert
Crumeyrolle, Rafał Abłamowicz, Pertti Lounesto,
published by Springer, 1995. –>
Comments Off on The Diamond and the Cube
Saturday, November 14, 2015
Space Program
A quote that appeared here on April 14, 2013 —
"I know what 'nothing' means." — Joan Didion
Dirac on the 4×4 matrices of an underlying nothingness —
"Corresponding to the four rows and columns,
the wave function ψ must contain a variable
that takes on four values, in order that the matrices
shall be capable of being multiplied into it."
— P. A. M. Dirac, Principles of Quantum Mechanics,
Fourth Edition, Oxford University Press, 1958,
page 257
Comments Off on Space Program
Sunday, March 1, 2009
Sunday March 1, 2009
Solomon's Cube
continued
"There is a book… called A Fellow of Trinity, one of series dealing with what is supposed to be Cambridge college life…. There are two heroes, a primary hero called Flowers, who is almost wholly good, and a secondary hero, a much weaker vessel, called Brown. Flowers and Brown find many dangers in university life, but the worst is a gambling saloon in Chesterton run by the Misses Bellenden, two fascinating but extremely wicked young ladies. Flowers survives all these troubles, is Second Wrangler and Senior Classic, and succeeds automatically to a Fellowship (as I suppose he would have done then). Brown succumbs, ruins his parents, takes to drink, is saved from delirium tremens during a thunderstorm only by the prayers of the Junior Dean, has much difficulty in obtaining even an Ordinary Degree, and ultimately becomes a missionary. The friendship is not shattered by these unhappy events, and Flowers's thoughts stray to Brown, with affectionate pity, as he drinks port and eats walnuts for the first time in Senior Combination Room."
— G. H. Hardy, A Mathematician's Apology
"The Solomon Key is the working title of an unreleased novel in progress by American author Dan Brown. The Solomon Key will be the third book involving the character of the Harvard professor Robert Langdon, of which the first two were Angels & Demons (2000) and The Da Vinci Code (2003)." — Wikipedia
"One has O+(6) ≅ S8, the symmetric group of order 8! …."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint.
"The complete projective group of collineations and dualities of the [projective] 3-space is shown to be of order [in modern notation] 8! …. To every transformation of the 3-space there corresponds a transformation of the [projective] 5-space. In the 5-space, there are determined 8 sets of 7 points each, 'heptads' …."
— George M. Conwell, "The 3-space PG(3, 2) and Its Group," The Annals of Mathematics, Second Series, Vol. 11, No. 2 (Jan., 1910), pp. 60-76
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference (July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
Comments Off on Sunday March 1, 2009
Sunday, August 3, 2008
Sunday August 3, 2008
Kindergarten
Geometry
Geometry
Preview of a Tom Stoppard play presented at Town Hall in Manhattan on March 14, 2008 (Pi Day and Einstein's birthday):
The play's title, "Every Good Boy Deserves Favour," is a mnemonic for the notes of the treble clef EGBDF.
The place, Town Hall, West 43rd Street. The time, 8 p.m., Friday, March 14. One single performance only, to the tinkle– or the clang?– of a triangle. Echoing perhaps the clang-clack of Warsaw Pact tanks muscling into Prague in August 1968.
The “u” in favour is the British way, the Stoppard way, "EGBDF" being "a Play for Actors and Orchestra" by Tom Stoppard (words) and André Previn (music).
And what a play!– as luminescent as always where Stoppard is concerned. The music component of the one-nighter at Town Hall– a showcase for the Boston University College of Fine Arts– is by a 47-piece live orchestra, the significant instrument being, well, a triangle.
When, in 1974, André Previn, then principal conductor of the London Symphony, invited Stoppard "to write something which had the need of a live full-time orchestra onstage," the 36-year-old playwright jumped at the chance.
One hitch: Stoppard at the time knew "very little about 'serious' music… My qualifications for writing about an orchestra," he says in his introduction to the 1978 Grove Press edition of "EGBDF," "amounted to a spell as a triangle player in a kindergarten percussion band."
Review of the same play as presented at Chautauqua Institution on July 24, 2008:
"Stoppard's modus operandi– to teasingly introduce numerous clever tidbits designed to challenge the audience."
— Jane Vranish, Pittsburgh Post-Gazette, Saturday, August 2, 2008
"The leader of the band is tired
And his eyes are growing old
But his blood runs through
My instrument
And his song is in my soul."
— Dan Fogelberg
"He's watching us all the time."
|
Finnegans Wake, Book II, Episode 2, pp. 296-297:
I'll make you to see figuratleavely the whome of your eternal geomater. And if you flung her headdress on her from under her highlows you'd wheeze whyse Salmonson set his seel on a hexengown.1 Hissss!, Arrah, go on! Fin for fun! 1 The chape of Doña Speranza of the Nacion. |
|
Reciprocity From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity…. … E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies." — William Boyd, review of Himmelfarb, a novel by Michael Kruger, in The New York Times Book Review, October 30, 1994 Last year's entry on this date:
The picture above is of the complete graph K6 … Six points with an edge connecting every pair of points… Fifteen edges in all. Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24. If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See Reciprocity and Reversal in Lao Tzu. For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the K6 graph above, expresses the reciprocity of male and female, as my May 2003 archives illustrate. The star of David also appears as part of a graphic design for cubes that illustrate the concepts of diamond theory:
Click on the design for details. Those who prefer a Jewish approach to physics can find the star of David, in the form of K6, applied to the sixteen 4×4 Dirac matrices, in
A Graphical Representation The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See |
"Finn MacCool ate the Salmon of Knowledge."
Wikipedia:
"George Salmon spent his boyhood in Cork City, Ireland. His father was a linen merchant. He graduated from Trinity College Dublin at the age of 19 with exceptionally high honours in mathematics. In 1841 at age 21 he was appointed to a position in the mathematics department at Trinity College Dublin. In 1845 he was appointed concurrently to a position in the theology department at Trinity College Dublin, having been confirmed in that year as an Anglican priest."
Related material:
Arrangements for
56 Triangles.
For more on the
arrangement of
triangles discussed
in Finnegans Wake,
see Log24 on Pi Day,
March 14, 2008.
Happy birthday,
Martin Sheen.
Comments Off on Sunday August 3, 2008
Sunday, July 13, 2008
Sunday July 13, 2008
Christ's High Table
C. P. Snow in A Mathematician's Apology :
FOREWORD
"It was a perfectly ordinary night at Christ's high table, except that Hardy was dining as a guest. He had just returned to Cambridge as Sadleirian professor, and I had heard something of him from young Cambridge mathematicians. They were delighted to have him back: he was a real mathematician, they said, not like those Diracs and Bohrs the physicists were always talking about: he was the purest of the pure. He was also unorthodox, eccentric, radical, ready to talk about anything. This was 1931, and the phrase was not yet in English use, but in later days they would have said that in some indefinable way he had star quality."
Perhaps now also at Christ's high table– Scarlett O'Hara's Younger Sister , Evelyn Keyes, who died on July 4, 2008:
"… the memory of Evelyn Keyes looking at herself on the screen, exclaiming: 'There's star quality! Look at those tits!'"
Evelyn Keyes in 99 River Street
Comments Off on Sunday July 13, 2008
Wednesday, September 3, 2003
Wednesday September 3, 2003
Reciprocity
From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity….
… E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies."
— William Boyd, review of Himmelfarb, New York Times Book Review, October 30, 1994
Last year's entry on this date:
|
Today's birthday:
"Mathematics is the music of reason."
Sylvester, a nineteenth-century mathematician, coined the phrase "synthematic totals" to describe some structures based on 6-element sets that R. T. Curtis has called "rather unwieldy objects." See Curtis's abstract, Symmetric Generation of Finite Groups, John Baez's essay, Some Thoughts on the Number 6, and my website, Diamond Theory. |
The picture above is of the complete graph
Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24.
If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See
Reciprocity and Reversal in Lao Tzu.
For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in
Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the
Click on the design for details.
Those who prefer a Jewish approach to physics can find the star of David, in the form of
A Graphical Representation
of the Dirac Algebra.
The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See
Thursday, December 5, 2002
Thursday December 5, 2002
Sacerdotal Jargon
From the website
Abstracts and Preprints in Clifford Algebra [1996, Oct 8]:
Paper: clf-alg/good9601
From: David M. Goodmanson
Address: 2725 68th Avenue S.E., Mercer Island, Washington 98040
Title: A graphical representation of the Dirac Algebra
Abstract: The elements of the Dirac algebra are represented by sixteen 4×4 gamma matrices, each pair of which either commute or anticommute. This paper demonstrates a correspondence between the gamma matrices and the complete graph on six points, a correspondence that provides a visual picture of the structure of the Dirac algebra. The graph shows all commutation and anticommutation relations, and can be used to illustrate the structure of subalgebras and equivalence classes and the effect of similarity transformations….
Published: Am. J. Phys. 64, 870-880 (1996)
The following is a picture of K6, the complete graph on six points. It may be used to illustrate various concepts in finite geometry as well as the properties of Dirac matrices described above.
From
"The Relations between Poetry and Painting,"
by Wallace Stevens:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color. . . . The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space—which he calls the mind or heart of creation— determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
Comments Off on Thursday December 5, 2002
Wednesday, December 4, 2002
Wednesday December 4, 2002
Symmetry and a Trinity
From a web page titled Spectra:
"What we learn from our whole discussion and what has indeed become a guiding principle in modern mathematics is this lesson:
Whenever you have to do with a structure-endowed entity S try to determine its group of automorphisms, the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of S in this way. After that you may start to investigate symmetric configurations of elements, i.e., configurations which are invariant under a certain subgroup of the group of all automorphisms . . ."
— Hermann Weyl in Symmetry, Princeton University Press, 1952, page 144
"… any color at all can be made from three different colors, in our case, red, green, and blue lights. By suitably mixing the three together we can make anything at all, as we demonstrated . . .
Further, these laws are very interesting mathematically. For those who are interested in the mathematics of the thing, it turns out as follows. Suppose that we take our three colors, which were red, green, and blue, but label them A, B, and C, and call them our primary colors. Then any color could be made by certain amounts of these three: say an amount a of color A, an amount b of color B, and an amount c of color C makes X:
Now suppose another color Y is made from the same three colors:
Then it turns out that the mixture of the two lights (it is one of the consequences of the laws that we have already mentioned) is obtained by taking the sum of the components of X and Y:
It is just like the mathematics of the addition of vectors, where (a, b, c ) are the components of one vector, and (a', b', c' ) are those of another vector, and the new light Z is then the "sum" of the vectors. This subject has always appealed to physicists and mathematicians."
— According to the author of the Spectra site, this is Richard Feynman in Elementary Particles and the Laws of Physics, The 1986 Dirac Memorial Lectures, by Feynman and Steven Weinberg, Cambridge University Press, 1989.
These two concepts — symmetry as invariance under a group of transformations, and complicated things as linear combinations (the technical name for Feynman's sums) of simpler things — underlie much of modern mathematics, both pure and applied.
Comments Off on Wednesday December 4, 2002