For further background to this morning's post Plan 9 Continues,
see posts tagged Sacred Space —
.
Monday, June 20, 2016
Sacred Space
Comments Off on Sacred Space
Sunday, June 19, 2016
Saturday, June 18, 2016
Midnight in Herald Square
In memory of New Yorker artist Anatol Kovarsky,
who reportedly died at 97 on June 1.
Note the Santa, a figure associated with Macy's at Herald Square.
See also posts tagged Herald Square, as well as the following
figure from this journal on the day preceding Kovarsky's death.
A note related both to Galois space and to
the "Herald Square"-tagged posts —
"There is such a thing as a length-16 sequence."
— Saying adapted from a young-adult novel.
Comments Off on Midnight in Herald Square
Tuesday, June 14, 2016
Model Kit
The title refers to the previous post, which quotes a
remark by a poetry critic in the current New Yorker .
Scholia —
From the post Structure and Sense of June 6, 2016 —
Structure
Sense
From the post Design Cube of July 23, 2015 —
Broken Symmetries in Diamond Space
Comments Off on Model Kit
Sunday, June 12, 2016
Tuesday, June 7, 2016
Art and Space…
Continues, in memory of chess grandmaster Viktor Korchnoi,
who reportedly died at 85 yesterday in Switzerland —
The coloring of the 4×4 "base" in the above image
suggests St. Bridget's cross.
From this journal on St. Bridget's Day this year —
"Possible title:
A new graphic approach
to an old geometric approach
to a new combinatorial approach
to an old algebraic approach
to M24"
The narrative leap from image to date may be regarded as
an example of "knight's move" thinking.
Comments Off on Art and Space…
Monday, June 6, 2016
Structure and Sense
"… the war of 70-some years ago
has already become something like the Trojan War
had been for the Homeric bards:
a major event in the mythic past
that gives structure and sense to our present reality."
— Justin E. H. Smith, a professor of philosophy at
the University of Paris 7–Denis Diderot,
in the New York Times column "The Stone"
(print edition published Sunday, June 5, 2016)
In memory of a British playwright who reportedly
died at 90 this morning —
Structure
Sense
Comments Off on Structure and Sense
Sunday, June 5, 2016
Sunday School: Seven Seals
Comments Off on Sunday School: Seven Seals
Friday, June 3, 2016
Bruins and van Dam
A review of some recent posts on Dirac and geometry,
each of which mentions the late physicist Hendrik van Dam:
- Kummer and Dirac (May 25)
- Framework (May 25)
- Expanding the Spielraum (May 26)
- Dorje (May 26)
The first of these posts mentions the work of E. M. Bruins.
Some earlier posts that cite Bruins:
- Anticommuting Dirac Matrices as Skew Lines (Nov. 20, 2015)
- Dirac and Line Geometry (Nov. 23, 2015)
- Einstein and Geometry (Nov. 27, 2015)
Comments Off on Bruins and van Dam
Thursday, June 2, 2016
Bullshit Studies
"The allusion to 'the most precious square of sense' shows
Shakespeare doing an almost scholastic demonstration of
the need for a ratio and interplay among the senses as
the very constitution of rationality."
— Marshall McLuhan, The Gutenberg Galaxy ,
University of Toronto Press, 1962, page 13
"What Shakespeare refers to in Lear as the 'precious
square of sense' probably has reference to the traditional
'square of opposition' in logic and to that four-part analogy
of proportionality which is the interplay of sense and reason."
— McLuhan, ibid. , page 241
This is of course nonsense, and, in view of McLuhan's pose
as a defender of the Catholic faith, damned nonsense.
Epigraph by McLuhan —
"The Gutenberg Galaxy develops a mosaic or field
approach to its problems."
I prefer a different "mosaic or field" related to the movable
blocks of Fröbel, not the movable type of Gutenberg.
Comments Off on Bullshit Studies
Tuesday, May 31, 2016
Monday, May 30, 2016
Perfect Universe
(A sequel to the previous post, Perfect Number)
Since antiquity, six has been known as
"the smallest perfect number." The word "perfect"
here means that a number is the sum of its
proper divisors — in the case of six: 1, 2, and 3.
The properties of a six-element set (a "6-set")
divided into three 2-sets and divided into two 3-sets
are those of what Burkard Polster, using the same
adjective in a different sense, has called
"the smallest perfect universe" — PG(3,2), the projective
3-dimensional space over the 2-element Galois field.
A Google search for the phrase "smallest perfect universe"
suggests a turnaround in meaning , if not in finance,
that might please Yahoo CEO Marissa Mayer on her birthday —
The semantic turnaround here in the meaning of "perfect"
is accompanied by a model turnaround in the picture of PG(3,2) as
Polster's tetrahedral model is replaced by Cullinane's square model.
Further background from the previous post —
See also Kirkman's Schoolgirl Problem.
Comments Off on Perfect Universe
Perfect Number
"Ageometretos me eisito."—
"Let no one ignorant of geometry enter."—
Said to be a saying of Plato, part of the
seal of the American Mathematical Society—
For the birthday of Marissa Mayer, who turns 41 today —
VOGUE Magazine,
AUGUST 16, 2013 12:01 AM
by JACOB WEISBERG —
"As she works to reverse the fortunes of a failing Silicon Valley
giant, Yahoo’s Marissa Mayer has fueled a national debate
about the office life, motherhood, and what it takes to be the
CEO of the moment.
'I really like even numbers, and
I like heavily divisible numbers.
Twelve is my lucky number—
I just love how divisible it is.
I don’t like odd numbers, and
I really don’t like primes.
When I turned 37,
I put on a strong face, but
I was not looking forward to 37.
But 37 turned out to be a pretty amazing year.
Especially considering that
36 is divisible by twelve!'
A few things may strike you while listening to Marissa Mayer
deliver this riff . . . . "
Yes, they may.
A smaller number for Marissa's meditations:
Six has been known since antiquity as the first "perfect" number.
Why it was so called is of little interest to anyone but historians
of number theory (a discipline that is not, as Wikipedia notes,
to be confused with numerology .)
What part geometry , on the other hand, played in Marissa's education,
I do not know.
Here, for what it's worth, is a figure from a review of posts in this journal
on the key role played by the number six in geometry —
Comments Off on Perfect Number
Sunday, May 29, 2016
The Ideogram Principle …
According to McLuhan
Marshall McLuhan writing to Ezra Pound on Dec. 21, 1948—
"The American mind is not even close to being amenable
to the ideogram principle as yet. The reason is simply this.
America is 100% 18th Century. The 18th century had
chucked out the principle of metaphor and analogy—
the basic fact that as A is to B so is C to D. AB:CD.
It can see AB relations. But relations in four terms are still
verboten. This amounts to deep occultation of nearly all
human thought for the U.S.A.
I am trying to devise a way of stating this difficulty as it exists.
Until stated and publicly recognized for what it is, poetry and
the arts can’t exist in America."
For context, see Cameron McEwen,
"Marshall McLuhan, John Pick, and Gerard Manley Hopkins."
(Renascence , Fall 2011, Vol. 64 Issue 1, 55-76)
A relation in four terms —
A : B :: C : D as Model : Crutch :: Metaphor : Ornament —
See also Dueling Formulas and Symmetry.
Comments Off on The Ideogram Principle …
Thursday, May 26, 2016
Dorje
Images suggested by the previous post —
Note the name "Dorje" in the first image above.
Remarks related to the name "Dorje," as well as to
"Projective Geometry and PT-Symmetric Dirac Hamiltonian,"
a 2009 paper by Y. Jack Ng and the late Hendrik van Dam —
Remarks for the Church of Synchronology from December 16, 2015,
the date of the above Dorje arXiv upload —
Comments Off on Dorje
Wednesday, May 25, 2016
Framework
"Studies of spin-½ theories in the framework of projective geometry
have been undertaken before." — Y. Jack Ng and H. van Dam,
February 20, 2009
For one such framework,* see posts from that same date
four years earlier — February 20, 2005.
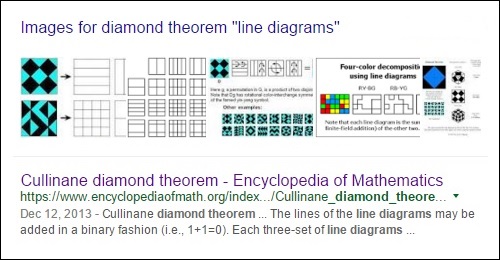
* A 4×4 array. See the 1977, 1978, and 1986 versions by
Steven H. Cullinane, the 1987 version by R. T. Curtis, and
the 1988 Conway-Sloane version illustrated below —
Cullinane, 1977
Cullinane, 1978
Cullinane, 1986
Curtis, 1987
Update of 10:42 PM ET on Sunday, June 19, 2016 —
The above images are precursors to …
Conway and Sloane, 1988
Update of 10 AM ET Sept. 16, 2016 — The excerpt from the
1977 "Diamond Theory" article was added above.
Comments Off on Framework
Kummer and Dirac
From "Projective Geometry and PT-Symmetric Dirac Hamiltonian,"
Y. Jack Ng and H. van Dam,
Physics Letters B , Volume 673, Issue 3,
23 March 2009, Pages 237–239
(http://arxiv.org/abs/0901.2579v2, last revised Feb. 20, 2009)
" Studies of spin-½ theories in the framework of projective geometry
have been undertaken before. See, e.g., Ref. [4]. 1 "
" 1 These papers are rather mathematical and technical.
The authors of the first two papers discuss the Dirac equation
in terms of the Plucker-Klein correspondence between lines of
a three-dimensional projective space and points of a quadric
in a five-dimensional projective space. The last paper shows
that the Dirac equation bears a certain relation to Kummer’s
surface, viz., the structure of the Dirac ring of matrices is
related to that of Kummer’s 166 configuration . . . ."
[4]
O. Veblen
Proc. Natl. Acad. Sci. USA , 19 (1933), p. 503
Full Text via CrossRef
E.M. Bruins
Proc. Nederl. Akad. Wetensch. , 52 (1949), p. 1135
F.C. Taylor Jr., Master thesis, University of North Carolina
at Chapel Hill (1968), unpublished
A remark of my own on the structure of Kummer’s 166 configuration . . . .
See as well yesterday morning's post.
Comments Off on Kummer and Dirac
Tuesday, May 24, 2016
Rosenhain and Göpel Revisited
The authors Taormina and Wendland in the previous post
discussed some mathematics they apparently did not know was
related to a classic 1905 book by R. W. H. T. Hudson, Kummer's
Quartic Surface .
"This famous book is a prototype for the possibility
of explaining and exploring a many-faceted topic of
research, without focussing on general definitions,
formal techniques, or even fancy machinery. In this
regard, the book still stands as a highly recommendable,
unparalleled introduction to Kummer surfaces, as a
permanent source of inspiration and, last but not least,
as an everlasting symbol of mathematical culture."
— Werner Kleinert, Mathematical Reviews ,
as quoted at Amazon.com
Some 4×4 diagrams from that book are highly relevant to the
discussion by Taormina and Wendland of the 4×4 squares within
the 1974 Miracle Octad Generator of R. T. Curtis that were later,
in 1987, described by Curtis as pictures of the vector 4-space over
the two-element Galois field GF(2).
Hudson did not think of his 4×4 diagrams as illustrating a vector space,
but he did use them to picture certain subsets of the 16 cells in each
diagram that he called Rosenhain and Göpel tetrads .
Some related work of my own (click images for related posts)—
Rosenhain tetrads as 20 of the 35 projective lines in PG(3,2)
Göpel tetrads as 15 of the 35 projective lines in PG(3,2)
Related terminology describing the Göpel tetrads above
Comments Off on Rosenhain and Göpel Revisited
Monday, May 23, 2016
Sunday, May 22, 2016
Sunday School
A less metaphysical approach to a "pre-form" —
From Wallace Stevens, "The Man with the Blue Guitar":
IX
And the color, the overcast blue
Of the air, in which the blue guitar
Is a form, described but difficult,
And I am merely a shadow hunched
Above the arrowy, still strings,
The maker of a thing yet to be made . . . .
"Arrowy, still strings" from the diamond theorem
See also "preforming" and the blue guitar
in a post of May 19, 2010.
Update of 7:11 PM ET:
More generally, see posts tagged May 19 Gestalt.
Comments Off on Sunday School
Thursday, May 19, 2016
Kulturkampf
From a check tonight of The New York Review of Books —
These NYRB stories from May 15 and May 13 suggest a
review of images on Ratner's Star and on the Eye of God.
Above image reposted from Jan. 10, 2014
|
I. The structures in the Diamond Puzzle…
Click on image for Jungian background. II: The structure on a recent cover of Semiotica…
|
Above images reposted from May 5, 2016
Related material: The previous post, Dueling Formulas.
Comments Off on Kulturkampf
Wednesday, May 18, 2016
Dueling Formulas
Note the echo of Jung's formula in the diamond theorem.
An attempt by Lévi-Strauss to defend his formula —
"… reducing the life of the mind to an abstract game . . . ." —
For a fictional version of such a game, see Das Glasperlenspiel .
Comments Off on Dueling Formulas
Tuesday, May 17, 2016
Bullshit Studies
The originator of the phrase 'Fab Four' reportedly
died at 80 on Saturday, May 14, 2016.
This suggests a review of another noted four-set.
The above image is from a study of Lévi-Strauss's "Canonical Formula" …
Midrash —
[Above photo of Lévi-Strauss and formula added June 6, 2016.]
Comments Off on Bullshit Studies
Sunday, May 15, 2016
Saturday, May 14, 2016
The Hourglass Code
A version of the I Ching’s Hexagram 19:
From Katherine Neville's The Eight , a book on the significance
of the date April 4 — the author's birthday —
|
The Eight by Katherine Neville —
“What does this have to do with why we’re here?” |
Related material: Posts now tagged Hourglass Code.
See also the hourglass in a search for Pilgrim's Progress Illustration.
Comments Off on The Hourglass Code
Friday, May 13, 2016
Geometry and Kinematics
"Just as both tragedy and comedy can be written
by using the same letters of the alphabet, the vast
variety of events in this world can be realized by
the same atoms through their different arrangements
and movements. Geometry and kinematics, which
were made possible by the void, proved to be still
more important in some way than pure being."
— Werner Heisenberg in Physics and Philosophy
For more about geometry and kinematics, see (for instance)
"An introduction to line geometry with applications,"
by Helmut Pottmann, Martin Peternell, and Bahram Ravani,
Computer-Aided Design 31 (1999), 3-16.
The concepts of line geometry (null point, null plane, null polarity,
linear complex, Klein quadric, etc.) are also of interest in finite geometry.
Some small finite spaces have as their natural models arrays of cubes .
Comments Off on Geometry and Kinematics
Monday, May 9, 2016
Search for the Lost Theorem
The three Solomons of the previous post (LeWitt,
Marcus, and Golomb) suggest the three figures
-1, 0, and 1 … symbols for the three elements
of the Galois field GF(3). This in turn suggests a
Search for The Lost Theorem. Some cross-cultural
context: The First of May, 2010.
Comments Off on Search for the Lost Theorem
Sunday, May 8, 2016
The Three Solomons
Earlier posts have dealt with Solomon Marcus and Solomon Golomb,
both of whom died this year — Marcus on Saint Patrick's Day, and
Golomb on Orthodox Easter Sunday. This suggests a review of
Solomon LeWitt, who died on Catholic Easter Sunday, 2007.
A quote from LeWitt indicates the depth of the word "conceptual"
in his approach to "conceptual art."
|
From Sol LeWitt: A Retrospective , edited by Gary Garrels, Yale University Press, 2000, p. 376:
THE SQUARE AND THE CUBE "The best that can be said for either the square or the cube is that they are relatively uninteresting in themselves. Being basic representations of two- and three-dimensional form, they lack the expressive force of other more interesting forms and shapes. They are standard and universally recognized, no initiation being required of the viewer; it is immediately evident that a square is a square and a cube a cube. Released from the necessity of being significant in themselves, they can be better used as grammatical devices from which the work may proceed." "Reprinted from Lucy R. Lippard et al ., “Homage to the Square,” Art in America 55, No. 4 (July-August 1967): 54. (LeWitt’s contribution was originally untitled.)" |
See also the Cullinane models of some small Galois spaces —
Comments Off on The Three Solomons
Friday, May 6, 2016
Thursday, May 5, 2016
Solomon’s Seal
Excerpt from a post of November 4, 2009 —
|
I. The structures in the Diamond Puzzle…
Click on image for Jungian background. II: The structure on a recent cover of Semiotica…
|
For some related material, see a search
for Solomon Marcus in this journal.
Comments Off on Solomon’s Seal
Wednesday, May 4, 2016
Golomb and Symmetry
From the webpage Diamond Theory Bibliography —
Golomb, Solomon W.
Shift register sequences (Revised edition)
Aegean Park Press, Laguna Hills, CA, 1982
The fifteen "stencils" in Golomb's Fig. VIII-8, page 219,
are the same as the fifteen affine hyperplanes that
account for patterns' symmetry in diamond theory.
This figure occurs in a discussion of Rademacher-
Walsh functions.
Comments Off on Golomb and Symmetry
Solomon Golomb, 1932-2016
Material related to the previous post, "Symmetry" —
This is the group of "8 rigid motions
generated by reflections in midplanes"
of "Solomon's Cube."
Material from this journal on May 1, the date of Golomb's death —
"Weitere Informationen zu diesem Themenkreis
finden sich unter http://www.encyclopediaofmath.org/
index.php/Cullinane_diamond_theorem und
http://finitegeometry.org/sc/gen/coord.html ."
Comments Off on Solomon Golomb, 1932-2016
Tuesday, May 3, 2016
Symmetry
A note related to the diamond theorem and to the site
Finite Geometry of the Square and Cube —
The last link in the previous post leads to a post of last October whose
final link leads, in turn, to a 2009 post titled Summa Mythologica .
Some may view the above web page as illustrating the
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate
in the spirit of the Glass Bead Game, everything actually
was all-meaningful, that every symbol and combination of
symbols led not hither and yon, not to single examples,
experiments, and proofs, but into the center, the mystery
and innermost heart of the world, into primal knowledge.
Every transition from major to minor in a sonata, every
transformation of a myth or a religious cult, every classical
or artistic formulation was, I realized in that flashing moment,
if seen with a truly meditative mind, nothing but a direct route
into the interior of the cosmic mystery, where in the alternation
between inhaling and exhaling, between heaven and earth,
between Yin and Yang, holiness is forever being created.”
A less poetic meditation on the above web page* —
"I saw that in the alternation between front and back,
between top and bottom, between left and right,
symmetry is forever being created."
Update of Sept. 5, 2016 — See also a related remark
by Lévi-Strauss in 1955: "…three different readings
become possible: left to right, top to bottom, front
to back."
* For the underlying mathematics, see a June 21, 1983, research note.
Comments Off on Symmetry
Monday, May 2, 2016
Quality
The previous post, on subjective and objective quality,
suggests a review of Pirsig —
“And finally: Phaedrus, following a path
that to his knowledge had never been taken before
in the history of Western thought,
went straight between the horns of
the subjectivity-objectivity dilemma and said
Quality is neither a part of mind, nor is it a part of matter.
It is a third entity which is independent of the two.
He was heard along the corridors
and up and down the stairs of Montana Hall
singing softly to himself, almost under his breath,
‘Holy, holy, holy…blessed Trinity.’ “
See also Guitart in this journal, noting esp. Zen and the Art.
Comments Off on Quality
Subjective Quality
The previous post deals in part with a figure from the 1988 book
Sphere Packings, Lattices and Groups , by J. H. Conway and
N. J. A. Sloane.
Siobhan Roberts recently wrote a book about the first of these
authors, Conway. I just discovered that last fall she also had an
article about the second author, Sloane, published:
"How to Build a Search Engine for Mathematics,"
Nautilus , Oct 22, 2015.
Meanwhile, in this journal …
Log24 on that same date, Oct. 22, 2015 —
Roberts's remarks on Conway and later on Sloane are perhaps
examples of subjective quality, as opposed to the objective quality
sought, if not found, by Alexander, and exemplified by the
above bijection discussed here last October.
Comments Off on Subjective Quality
Sunday, May 1, 2016
Sunday Appetizer from 1984
Judith Shulevitz in The New York Times
on Sunday, July 18, 2010
(quoted here Aug. 15, 2010) —
“What would an organic Christian Sabbath look like today?”
The 2015 German edition of Beautiful Mathematics ,
a 2011 Mathematical Association of America (MAA) book,
was retitled Mathematische Appetithäppchen —
Mathematical Appetizers . The German edition mentions
the author's source, omitted in the original American edition,
for his section 5.17, "A Group of Operations" (in German,
5.17, "Eine Gruppe von Operationen") —
|
Mathematische Appetithäppchen: Autor: Erickson, Martin —
"Weitere Informationen zu diesem Themenkreis finden sich |
That source was a document that has been on the Web
since 2002. The document was submitted to the MAA
in 1984 but was rejected. The German edition omits the
document's title, and describes it as merely a source for
"further information on this subject area."
The title of the document, "Binary Coordinate Systems,"
is highly relevant to figure 11.16c on page 312 of a book
published four years after the document was written: the
1988 first edition of Sphere Packings, Lattices and Groups ,
by J. H. Conway and N. J. A. Sloane —
A passage from the 1984 document —
Comments Off on Sunday Appetizer from 1984
Friday, April 29, 2016
Blackboard Jungle…
Comments Off on Blackboard Jungle…
Wednesday, April 27, 2016
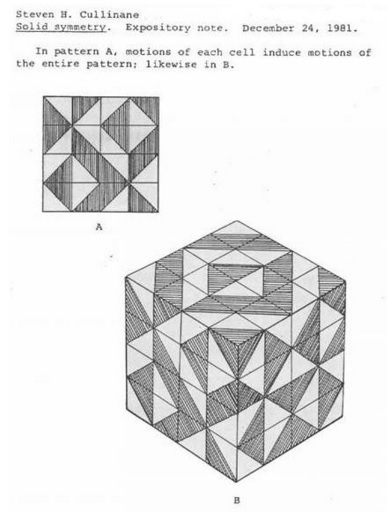
Local and Global
Three notes on local symmetries
that induce global symmetries
From July 1, 2011 —
From November 5, 1981 —
From December 24, 1981 —
Comments Off on Local and Global
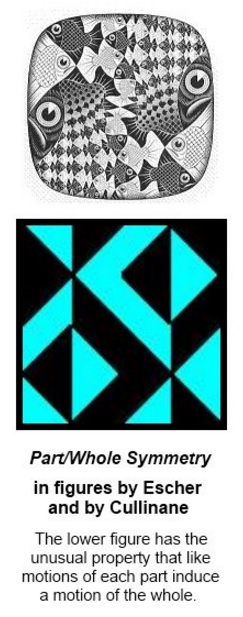
Tuesday, April 26, 2016
A Sense of Identity
Peter Schjeldahl on Wallace Stevens in the current New Yorker —
"Stevens was born in 1879 in Reading, Pennsylvania,
the second of five children. His father, from humble
beginnings, was a successful lawyer, his mother a
former schoolteacher. Each night, she read a chapter
of the Bible to the children, who attended schools
attached to both Presbyterian and Lutheran churches,
where the music left an indelible impression on Stevens.
Both sides of the family were Pennsylvania Dutch,
an identity that meant little to him when he was young
but a great deal later on, perhaps to shore up a precarious
sense of identity."
See also this journal on Christmas Day, 2010 —
It's a start. For more advanced remarks from the same date, see Mere Geometry.
Comments Off on A Sense of Identity
Interacting
"… I would drop the keystone into my arch …."
— Charles Sanders Peirce, "On Phenomenology"
" 'But which is the stone that supports the bridge?' Kublai Khan asks."
— Italo Calvino, Invisible Cities, as quoted by B. Elan Dresher.
(B. Elan Dresher. Nordlyd 41.2 (2014): 165-181,
special issue on Features edited by Martin Krämer,
Sandra Ronai and Peter Svenonius. University of Tromsø –
The Arctic University of Norway.
http://septentrio.uit.no/index.php/nordlyd)
Peter Svenonius and Martin Krämer, introduction to the
Nordlyd double issue on Features —
"Interacting with these questions about the 'geometric'
relations among features is the algebraic structure
of the features."
For another such interaction, see the previous post.
This post may be viewed as a commentary on a remark in Wikipedia —
"All of these ideas speak to the crux of Plato's Problem…."
See also The Diamond Theorem at Tromsø and Mere Geometry.
Comments Off on Interacting
Monday, April 25, 2016
Seven Seals
An old version of the Wikipedia article "Group theory"
(pictured in the previous post) —
"More poetically …"
From Hermann Weyl's 1952 classic Symmetry —
"Galois' ideas, which for several decades remained
a book with seven seals but later exerted a more
and more profound influence upon the whole
development of mathematics, are contained in
a farewell letter written to a friend on the eve of
his death, which he met in a silly duel at the age of
twenty-one. This letter, if judged by the novelty and
profundity of ideas it contains, is perhaps the most
substantial piece of writing in the whole literature
of mankind."
The seven seals from the previous post, with some context —
These models of projective points are drawn from the underlying
structure described (in the 4×4 case) as part of the proof of the
Cullinane diamond theorem .
Comments Off on Seven Seals
Peirce’s Accounts of the Universe
Compare and contrast Peirce's seven systems of metaphysics with
the seven projective points in a post of March 1, 2010 —
From my commentary on Carter's question —
Comments Off on Peirce’s Accounts of the Universe
Thursday, April 21, 2016
The Alchemist’s Chessboard
Material related to the previous post and to Alfred Bester's
1981 followup to The Stars My Destination titled The Deceivers —
|
The Lapis Philosophorum :
"The lapis was thought of as a unity and therefore often stands for the prima materia in general."
"Its discoverer was of the opinion that he had produced the equivalent of the primordial protomatter which exploded into the Universe." And from Bester's The Deceivers : Meta Physics "'… Think of a match. You've got a chemical head of potash, antimony, and stuff, full of energy waiting to be released. Friction does it. But when Meta excites and releases energy, it's like a stick of dynamite compared to a match. It's the chess legend for real.' 'I don't know it.' 'Oh, the story goes that a philosopher invented chess for the amusement of an Indian rajah. The king was so delighted that he told the inventor to name his reward and he'd get it, no matter what. The philosopher asked that one grain of rice be placed on the first square of the chessboard, two on the second, four on the third, and so on to the sixty-fourth.' 'That doesn't sound like much.'" Related material : |
Comments Off on The Alchemist’s Chessboard
Wednesday, April 20, 2016
Symmetric Generation of a Simple Group
The reference in the previous post to the work of Guitart and
The Road to Universal Logic suggests a fiction involving
the symmetric generation of the simple group of order 168.
See The Diamond Archetype and a fictional account of the road to Hell …
The cover illustration below has been adapted to
replace the flames of PyrE with the eightfold cube.
For related symmetric generation of a much larger group, see Solomon’s Cube.
Comments Off on Symmetric Generation of a Simple Group
Tuesday, April 19, 2016
The Folding
A recent post about the eightfold cube suggests a review of two
April 8, 2015, posts on what Northrop Frye called the ogdoad :
As noted on April 8, each 2×4 "brick" in the 1974 Miracle Octad Generator
of R. T. Curtis may be constructed by folding a 1×8 array from Turyn's
1967 construction of the Golay code.
Folding a 2×4 Curtis array yet again yields the 2x2x2 eightfold cube .
Those who prefer an entertainment approach to concepts of space
may enjoy a video (embedded yesterday in a story on theverge.com) —
"Ghost in the Shell: Identity in Space."
Comments Off on The Folding
Sunday, April 17, 2016
The Thing and I
The New York Times philosophy column yesterday —
The Times's philosophy column "The Stone" is named after the legendary
"philosophers' stone." The column's name, and the title of its essay yesterday
"Is that even a thing?" suggest a review of the eightfold cube as "The object
most closely resembling a 'philosophers' stone' that I know of" (Page 51 of
the current issue of a Norwegian art quarterly, KUNSTforum.as).
The eightfold cube —
|
Definition of Epiphany
From James Joyce’s Stephen Hero , first published posthumously in 1944. The excerpt below is from a version edited by John J. Slocum and Herbert Cahoon (New York: New Directions Press, 1959). Three Times: … By an epiphany he meant a sudden spiritual manifestation, whether in the vulgarity of speech or of gesture or in a memorable phase of the mind itself. He believed that it was for the man of letters to record these epiphanies with extreme care, seeing that they themselves are the most delicate and evanescent of moments. He told Cranly that the clock of the Ballast Office was capable of an epiphany. Cranly questioned the inscrutable dial of the Ballast Office with his no less inscrutable countenance: — Yes, said Stephen. I will pass it time after time, allude to it, refer to it, catch a glimpse of it. It is only an item in the catalogue of Dublin’s street furniture. Then all at once I see it and I know at once what it is: epiphany. — What? — Imagine my glimpses at that clock as the gropings of a spiritual eye which seeks to adjust its vision to an exact focus. The moment the focus is reached the object is epiphanised. It is just in this epiphany that I find the third, the supreme quality of beauty. — Yes? said Cranly absently. — No esthetic theory, pursued Stephen relentlessly, is of any value which investigates with the aid of the lantern of tradition. What we symbolise in black the Chinaman may symbolise in yellow: each has his own tradition. Greek beauty laughs at Coptic beauty and the American Indian derides them both. It is almost impossible to reconcile all tradition whereas it is by no means impossible to find the justification of every form of beauty which has ever been adored on the earth by an examination into the mechanism of esthetic apprehension whether it be dressed in red, white, yellow or black. We have no reason for thinking that the Chinaman has a different system of digestion from that which we have though our diets are quite dissimilar. The apprehensive faculty must be scrutinised in action. — Yes … — You know what Aquinas says: The three things requisite for beauty are, integrity, a wholeness, symmetry and radiance. Some day I will expand that sentence into a treatise. Consider the performance of your own mind when confronted with any object, hypothetically beautiful. Your mind to apprehend that object divides the entire universe into two parts, the object, and the void which is not the object. To apprehend it you must lift it away from everything else: and then you perceive that it is one integral thing, that is a thing. You recognise its integrity. Isn’t that so? — And then? — That is the first quality of beauty: it is declared in a simple sudden synthesis of the faculty which apprehends. What then? Analysis then. The mind considers the object in whole and in part, in relation to itself and to other objects, examines the balance of its parts, contemplates the form of the object, traverses every cranny of the structure. So the mind receives the impression of the symmetry of the object. The mind recognises that the object is in the strict sense of the word, a thing , a definitely constituted entity. You see? — Let us turn back, said Cranly. They had reached the corner of Grafton St and as the footpath was overcrowded they turned back northwards. Cranly had an inclination to watch the antics of a drunkard who had been ejected from a bar in Suffolk St but Stephen took his arm summarily and led him away. — Now for the third quality. For a long time I couldn’t make out what Aquinas meant. He uses a figurative word (a very unusual thing for him) but I have solved it. Claritas is quidditas . After the analysis which discovers the second quality the mind makes the only logically possible synthesis and discovers the third quality. This is the moment which I call epiphany. First we recognise that the object is one integral thing, then we recognise that it is an organised composite structure, a thing in fact: finally, when the relation of the parts is exquisite, when the parts are adjusted to the special point, we recognise that it is that thing which it is. Its soul, its whatness, leaps to us from the vestment of its appearance. The soul of the commonest object, the structure of which is so adjusted, seems to us radiant. The object achieves its epiphany. Having finished his argument Stephen walked on in silence. He felt Cranly’s hostility and he accused himself of having cheapened the eternal images of beauty. For the first time, too, he felt slightly awkward in his friend’s company and to restore a mood of flippant familiarity he glanced up at the clock of the Ballast Office and smiled: — It has not epiphanised yet, he said. |
Comments Off on The Thing and I
Thursday, April 14, 2016
One Funeral at a Time
On this date in 2005, mathematician Saunders Mac Lane died at 95.
Related material —
Max Planck quotations:
Mac Lane on Boolean algebra:
Mac Lane’s summary chart (note the absence of Galois geometry ):
I disagree with Mac Lane’s assertion that “the finite models of
Boolean algebra are dull.” See Boole vs. Galois in this journal.
Comments Off on One Funeral at a Time
Strange Awards
From a review of a play by the late Anne Meara* —
"Meara, known primarily as an actress/comedian
(half of the team of Stiller & Meara, and mother of
Ben Stiller), is also an accomplished writer for the
stage; her After Play was much acclaimed….
This new, more ambitious piece starts off with a sly
send-up of awards dinners as the late benefactor of
a wealthy foundation–the comically pixilated scientist
Herschel Strange (Jerry Stiller)–is seen on videotape.
This tape sets a light tone that is hilariously
heightened when John Shea, as Arthur Garden,
accepts the award given in Strange's name."
Compare and contrast —
- A figure from yesterday's 1 PM post Black List:
-
The circular device of Doctor Strange on the cover of
Entertainment Weekly , Jan. 8/15 2016:
I of course prefer the Galois I Ching .
* See the May 25, 2015, post The Secret Life of the Public Mind.
Comments Off on Strange Awards
Wednesday, April 13, 2016
Black List
A search for "Max Black" in this journal yields some images
from a post of August 30, 2006 . . .
|
"Jackson has identified the seventh symbol." |
The "Jackson" above is played by the young James Spader,
who in an older version currently stars in "The Blacklist."
|
"… the memorable models of science are 'speculative instruments,' — Max Black in Models and Metaphors , Cornell U. Press, 1962 |
Comments Off on Black List
Tuesday, April 12, 2016
Slow Art
The American Mathematical Society today got around to
publishing an obituary for Solomon Marcus, a Bucharest
mathematician who died on St. Patrick's Day, March 17.
See as well this journal on March 22.
Comments Off on Slow Art
Monday, April 11, 2016
Like Decorations in a Cartoon Graveyard
From Sunday evening's In Memoriam post —
The "from Princeton" remark in the previous post came from
Princeton, but originated with a retired professor in Rochester,
NY, one Joseph Neisendorfer.
Another remark by Neisendorfer, from his weblog —
Those familiar with the chapter on Galois in the
Eric Temple Bell classic Men of Mathematics
will know that the words quoted above by
Neisendorfer are definitely not those of Albert Einstein.
Comments Off on Like Decorations in a Cartoon Graveyard
Combinatorial Spider
“Chaos is order yet undeciphered.”
— The novel The Double , by José Saramago,
on which the film "Enemy" was based
Some background for the 2012 Douglas Glover
"Attack of the Copula Spiders" book
mentioned in Sunday's Synchronicity Check —
-
"A vision of Toronto as Hell" — Douglas Glover in the
March 25, 2011, post Combinatorial Delight - For Louise Bourgeois — a post from the date of Galois's death—
Comments Off on Combinatorial Spider
Friday, April 8, 2016
Space Cross
For George Orwell
Illustration from a book on mathematics —
This illustrates the Galois space AG(4,2).
For some related spaces, see a note from 1984.
"There is such a thing as a space cross."
— Saying adapted from a young-adult novel
Comments Off on Space Cross
Ogdoads by Curtis
As was previously noted here, the construction of the Miracle Octad Generator
of R. T. Curtis in 1974 may have involved his "folding" the 1×8 octads constructed
in 1967 by Turyn into 2×4 form.
This results in a way of picturing a well-known correspondence (Conwell, 1910)
between partitions of an 8-set and lines of the projective 3-space PG(3,2).
For some background related to the "ogdoads" of the previous post, see
A Seventh Seal (Sept. 15, 2014).
Comments Off on Ogdoads by Curtis
Tuesday, April 5, 2016
Stevens Illustrated
Comments Off on Stevens Illustrated
“Puzzle Cube of a Novel”
Comments Off on “Puzzle Cube of a Novel”
Monday, April 4, 2016
The Bauersfeld Structure*
"If you would be a poet, create works capable of
answering the challenge of apocalyptic times,
even if this meaning sounds apocalyptic."
"It's a trap!"
— Ferlinghetti's friend Erik Bauersfeld,
who reportedly died yesterday at 93
* See also, in this journal, Galois Cube and Deathtrap.
Comments Off on The Bauersfeld Structure*
Cube for Berlin
Foreword by Sir Michael Atiyah —
"Poincaré said that science is no more a collection of facts
than a house is a collection of bricks. The facts have to be
ordered or structured, they have to fit a theory, a construct
(often mathematical) in the human mind. . . .
… Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to
explain how art fits into our subject and what we mean by beauty.
In attempting to bridge this divide I have always found that
architecture is the best of the arts to compare with mathematics.
The analogy between the two subjects is not hard to describe
and enables abstract ideas to be exemplified by bricks and mortar,
in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics"
in the AMS Notices , January 2010
Judy Bass, Los Angeles Times , March 12, 1989 —
"Like Rubik's Cube, The Eight demands to be pondered."
As does a figure from 1984, Cullinane's Cube —
For natural group actions on the Cullinane cube,
see "The Eightfold Cube" and
"A Simple Reflection Group of Order 168."
See also the recent post Cube Bricks 1984 —
Related remark from the literature —
Note that only the static structure is described by Felsner, not the
168 group actions discussed by Cullinane. For remarks on such
group actions in the literature, see "Cube Space, 1984-2003."
(From Anatomy of a Cube, Sept. 18, 2011.)
Comments Off on Cube for Berlin
Sunday, March 27, 2016
In Memoriam
Slavik Jablan, a writer on symmetry.
A post from the date of his death —
See as well a post from yesterday and Fearful Princeton.
Comments Off on In Memoriam
Thursday, March 24, 2016
Truth in 1984
"The theory of elliptic curves and modular forms is
one subject where the most diverse branches
of mathematics come together: complex analysis,
algebraic geometry, representation theory, number theory."
— Neal Koblitz, first sentence of
Introduction to Elliptic Curves and Modular Forms,
First Edition, Springer-Verlag, 1984
Related material —
A quote co-authored by Koblitz appears in today's
earlier post The Wolf Gang.
See also The Proof and the Lie.
Comments Off on Truth in 1984
Tuesday, March 22, 2016
The Zero Obit
Comments Off on The Zero Obit
Saturday, March 19, 2016
Two-by-Four
For an example of "anonymous content" (the title of the
previous post), see a search for "2×4" in this journal.
Comments Off on Two-by-Four
Thursday, March 17, 2016
On the Eightfold Cube
The following page quotes "Raiders of the Lost Crucible,"
a Log24 post from Halloween 2015.
From KUNSTforum.as, a Norwegian art quarterly, issue no. 1 of 2016.
Related posts — See Lyche Eightfold.
Comments Off on On the Eightfold Cube
Tuesday, March 15, 2016
15 Projective Points Revisited
A March 10, 2016, Facebook post from KUNSTforum.as,
a Norwegian art quarterly —
Click image above for a view of pages 50-51 of a new KUNSTforum
article showing two photos relevant to my own work — those labeled
"after S. H. Cullinane."
(The phrase "den pensjonerte Oxford-professoren Stephen H. Cullinane"
on page 51 is almost completely wrong. I have never been a professor,
I was never at Oxford, and my first name is Steven, not Stephen.)
For some background on the 15 projective points at the lower left of
the above March 10 Facebook post, see "The Smallest Projective Space."
Comments Off on 15 Projective Points Revisited
Friday, March 4, 2016
Cube Bricks 1984
Related aesthetics —
"Poincaré said that science is no more a collection of facts
than a house is a collection of bricks. The facts have to be
ordered or structured, they have to fit a theory, a construct
(often mathematical) in the human mind. . . .
… Mathematics may be art, but to the general public it is
a black art, more akin to magic and mystery. This presents
a constant challenge to the mathematical community: to
explain how art fits into our subject and what we mean by beauty.
In attempting to bridge this divide I have always found that
architecture is the best of the arts to compare with mathematics.
The analogy between the two subjects is not hard to describe
and enables abstract ideas to be exemplified by bricks and mortar,
in the spirit of the Poincaré quotation I used earlier."
— Sir Michael Atiyah, "The Art of Mathematics"
in the AMS Notices , January 2010
Comments Off on Cube Bricks 1984
Wednesday, March 2, 2016
A Geometric Glitter
"In the planes that tilt hard revelations on
The eye, a geometric glitter, tiltings …."
— Wallace Stevens, "Someone Puts a Pineapple Together" (1947)
Comments Off on A Geometric Glitter
Tuesday, March 1, 2016
Art and Geometry
See "Behind the Glitter" (a recent magazine article
on Oslo artist Josefine Lyche), and the much more
informative web page Contact (from Noplace, Oslo).
From the latter —
"Semiotics is a game of ascribing meaning, or content, to mere surface."
Comments Off on Art and Geometry
Saturday, February 27, 2016
Back to the Blackboard
Comments Off on Back to the Blackboard
Friday, February 26, 2016
Overarching
"The study of social memory allows scholars to
understand how different memories form within
a collective group, thus exploring the societal
and ideological elements of disparate groups
that form the over-arching memory of Melkisedeq."
— The Melkisedeq Memoirs , by Cale Staley,
2015 master's thesis at the University of Iowa
Elements of groups that I prefer —
"Right through hell
there is a path…."
— Malcolm Lowry,
Under the Volcano
Comments Off on Overarching
Tuesday, February 23, 2016
Both Sides Now
(Continued from the link in the previous post to
a Feb. 20 NY Times essay on the brain's two sides)
From a webpage on Galois geometry —
|
Postscript From a 2002 review by Stacy G. Langton of Sherman Stein's book on mathematics, How the Other Half Thinks : "The title of Stein's book (perhaps chosen by the publisher?) seems to refer to the popular left brain/right brain dichotomy. As Stein writes (p. ix): 'I hope this book will help bridge that notorious gap that separates the two cultures: the humanities and the sciences, or should I say the right brain (intuitive, holistic) and the left brain (analytical, numerical). As the chapters will illustrate, mathematics is not restricted to the analytical and numerical; intuition plays a significant role.' Stein does well to avoid identifying mathematics with the activity of just one side of the brain. He would have done better, however, not to have endorsed the left brain/right brain ideology. While it does indeed appear to be the case that the two sides of our brain act in rather different ways, the idea that the right brain is 'intuitive, holistic,' while the left brain is 'analytical, numerical,' is a vast oversimplification, and goes far beyond the actual evidence." |
Comments Off on Both Sides Now
Sunday, February 21, 2016
Orson Card
The title is from the name of a character in a new novel.
The title is also the name of a noted author.
Related material from April 2, 2009 —
|
"It seems, as one becomes older, — T. S. Eliot, Four Quartets
"Note that at first, you can see the 'arrow of time.' — "The Ehrenfest Chains," by Kyle Siegrist, ex. 16 |
For a different Orson, click on "the direction of time."
Comments Off on Orson Card
Wednesday, February 17, 2016
“Blank Space” Accolades
A post in memory of British theatre director Peter Wood,
who reportedly died on February 11, 2016.
From the date of the director's death —
"Leave a space." — Tom Stoppard
Comments Off on “Blank Space” Accolades
Friday, February 12, 2016
The Game
"Right through hell
there is a path…."
— Malcolm Lowry,
Under the Volcano
Comments Off on The Game
Tuesday, February 9, 2016
Cubism
The hexagons above appear also in Gary W. Gibbons,
"The Kummer Configuration and the Geometry of Majorana Spinors,"
1993, in a cube model of the Kummer 166 configuration —
Related material — The Religion of Cubism (May 9, 2003).
Comments Off on Cubism
Monday, February 8, 2016
A Game with Four Letters
Related material — Posts tagged Dirac and Geometry.
For an example of what Eddington calls "an open mind,"
see the 1958 letters of Nanavira Thera.
(Among the "Early Letters" in Seeking the Path ).
Comments Off on A Game with Four Letters
Friday, February 5, 2016
Death on New Year’s Day
From the American Mathematical Society today —
Jean Pedersen (1934-2016)
Friday February 5th 2016
Jean Pedersen died January 1 at the age of 81.
She was a longtime member of the faculty at
Santa Clara University. Pedersen and Peter Hilton
co-authored A Mathematical Tapestry: Demonstrating
the Beautiful Unity of Mathematics , which used paper
folding to show connections between geometry,
number theory, and group theory. Pedersen was an
AMS member since 1979.
Related art —
"Spiel ist nicht Spielerei" — Friedrich Fröbel
Comments Off on Death on New Year’s Day
Monday, February 1, 2016
The Hiroshima Preprint
This morning at 11:44 I happened upon …
This was published as …
Toshiyuki Katsura, Shigeyuki Kondo, Ichiro Shimada,
"On the supersingular K3 surface in characteristic 5 with Artin invariant 1,"
Michigan Mathematical Journal , vol. 63, issue 4 (Dec. 2014), 803–844.
Related material from later today —
See also earlier Log24 remarks on the Hoffman-Singleton graph
and a remark on geometry for Princeton.
Comments Off on The Hiroshima Preprint
Historical Note
Possible title:
A new graphic approach
to an old geometric approach
to a new combinatorial approach
to an old algebraic approach
to M24
Comments Off on Historical Note
Sunday, January 31, 2016
Forgotten Lore
Continued from Sunday, January 24, 2016
Wikipedia on Io in Greek mythology
(a precursor to Marvel Comics) —
"Walter Burkert [18] notes that the story of Io was told
in the ancient epic tradition at least four times….
18. Burkert, Homo Necans (1974) 1983:
164 note 14, giving bibliography."
An "io" story I prefer — m24.io.
Comments Off on Forgotten Lore
Saturday, January 30, 2016
Pope’s Geometry
From page 56 of The Science Fiction of Mark Clifton ,
Southern Illinois University Press, 1980 —
See also the following image in this journal —
.
Comments Off on Pope’s Geometry
Friday, January 29, 2016
Thursday, January 28, 2016
A6!
The title refers to a line by Louis Menand quoted
at the end of the previous post.
There "a6!" refers to the chessboard square in
column a, row 6. In Geometry of the I Ching,
this square represents Hexagram 61, "Inner Truth."
See also "inner truth" in this journal.
Comments Off on A6!
Wednesday, January 27, 2016
Game Theory for Steiner
"The definition of easy to learn, hard to master"
— Alex Hern in The Guardian today on the game of Go
Not unlike music, mathematics, and chess.
Comments Off on Game Theory for Steiner
Sunday, January 24, 2016
Long Line
"The ideal of a complete mathematical theory of beauty
lies on the same long line of distinguished fantasies of
mathematical wisdom as the number mysticism of
Pythagoras and Plato, the Ars Magna of Ramon Llull
(whom Agrippa studied) and Giordano Bruno
(who studied Llull and Agrippa), the vision of Mathesis
Universalis that Descartes and Leibniz shared, and the
Ars Combinatoria of Leibniz. Dürer does not deny the
existence of absolute beauty but despairs of knowing it."
— The late David Ritz Finkelstein in 2007.
He reportedly died today.
Comments Off on Long Line
Friday, January 22, 2016
Easter Footnote
A search from Easter 2013 for "Cremona synthemes" * —
For some strictly mathematical background, see
Classical Geometry in Light of Galois Geometry.
* For more about Cremona and synthemes,
see a 1975 paper by W. L. Edge,
"A Footnote on the Mystic Hexagram."
Comments Off on Easter Footnote
Thursday, January 21, 2016
Dividing the Indivisible
My statement yesterday morning that the 15 points
of the finite projective space PG(3,2) are indivisible
was wrong. I was misled by quoting the powerful
rhetoric of Lincoln Barnett (LIFE magazine, 1949).
Points of Euclidean space are of course indivisible:
"A point is that which has no parts" (in some translations).
And the 15 points of PG(3,2) may be pictured as 15
Euclidean points in a square array (with one point removed)
or tetrahedral array (with 11 points added).
The geometry of PG(3,2) becomes more interesting,
however, when the 15 points are each divided into
several parts. For one approach to such a division,
see Mere Geometry. For another approach, click on the
image below.
Comments Off on Dividing the Indivisible
Wednesday, January 20, 2016
Fringe Physics and Beyond
"One day not long ago Oppenheimer stalked
up and down his office and divulged some
startling new discoveries about the 15 fundamental
particles of which the universe is made….
… physicists today are wondering if these particles
are themselves actually the final, stark, immutable
and indivisible foundation stones of the universe
that until now they have been thought to be."
—Lincoln Barnett in LIFE magazine,
Oct. 10, 1949, page 122
Fringe Physics —
"… astrophysics limits the number of fundamental particles to 15…."
— Franklin Potter at FQXi.org on Sep. 27, 2009
"I agree there can't be more than 15 fundamental particles."
— Lawrence B. Crowell at FQXi.org on Sep. 29, 2009
Beyond —
There are, at any rate, 15 "final, stark, immutable* and indivisible*
foundation stones" (namely, 15 points ) of the finite projective
space PG(3,2). See Symplectic in this journal.
For related physics, see posts tagged Dirac and Geometry.
* Update of Jan. 21, 2016 — I was carried away by Barnett's
powerful rhetoric. These adjectives are wrong.
Comments Off on Fringe Physics and Beyond
Monday, January 18, 2016
First and Last
"First and last, he was a skeptic …."
— Home page of Martin-Gardner.org
See also, in this journal, Alpha and Omega.
Related material from the last full day of Gardner's life —
See as well Symplectic in this journal.
Comments Off on First and Last
Saturday, January 16, 2016
The Strands of History…
… Meet the Threads of Fiction
"… where the threads of fiction and the strands of history shuttle
back and forth in the great loom of the artist's imagination"
Comments Off on The Strands of History…
Wednesday, January 13, 2016
Geometry for Jews
(Continued from previous episodes)
Boole and Galois also figure in the mathematics of space —
i.e. , geometry. See Boole + Galois in this journal.
Related material, according to Jung’s notion of synchronicity —
- This journal on the date, August 6, 2007, of the
above paper, and the following day —
posts now tagged Metamorphosis 2007 - This journal on two of the dates of the 2003 Haifa workshop
that the paper mentions in a footnote —
posts now tagged Solomon’s Mental Health Month
Comments Off on Geometry for Jews
Tuesday, January 12, 2016
Harmonic Analysis and Galois Spaces
The above sketch indicates, in a vague, hand-waving, fashion,
a connection between Galois spaces and harmonic analysis.
For more details of the connection, see (for instance) yesterday
afternoon's post Space Oddity.
Comments Off on Harmonic Analysis and Galois Spaces
Monday, January 11, 2016
Space Oddity
It is an odd fact that the close relationship between some
small Galois spaces and small Boolean spaces has gone
unremarked by mathematicians.
A Google search today for “Galois spaces” + “Boolean spaces”
yielded, apart from merely terminological sources, only some
introductory material I have put on the Web myself.
Some more sophisticated searches, however led to a few
documents from the years 1971 – 1981 …
“Harmonic Analysis of Switching Functions” ,
by Robert J. Lechner, Ch. 5 in A. Mukhopadhyay, editor,
Recent Developments in Switching Theory , Academic Press, 1971.
“Galois Switching Functions and Their Applications,”
by B. Benjauthrit and I. S. Reed,
JPL Deep Space Network Progress Report 42-27 , 1975
D.K. Pradhan, “A Theory of Galois Switching Functions,”
IEEE Trans. Computers , vol. 27, no. 3, pp. 239-249, Mar. 1978
“Switching functions constructed by Galois extension fields,”
by Iwaro Takahashi, Information and Control ,
Volume 48, Issue 2, pp. 95–108, February 1981
An illustration from the Lechner paper above —
“There is such a thing as harmonic analysis of switching functions.”
— Saying adapted from a young-adult novel
Comments Off on Space Oddity
Sunday, January 10, 2016
Sermons
This journal last Sunday —
For a more traditional sermon from last Sunday
at Nassau Presbyterian Church, Princeton NJ,
see "God for Dummies."
Comments Off on Sermons
Friday, January 8, 2016
Condescension and Hostility
For the 2016 Joint Mathematics Meetings in Seattle —
"Condescension and a certain amount of hostility
used to mark the critical reaction…."
— Emma Brockes on Stephen King in
The Guardian , 21 Sept. 2013
Related material:
Remarks from Tilings and Patterns , by Branko Grünbaum
and G. C. Shephard, quoted in the webpage Pattern Groups.
Comments Off on Condescension and Hostility
Thursday, January 7, 2016
Point Omega…
… Continues. See previous episodes.
See as well …
-
Types of Ambiguity : Galois Meets Doctor Faustus,
from December 14, 2010.
-
From Jan. 5, the date of Pierre Boulez's death,
a post on Galois geometry.
- 2016 Joint Mathematics Meetings
The above image is from April 7, 2003.
Comments Off on Point Omega…
Wednesday, January 6, 2016
Galois.io
The title is a new URL.
Midrash on the URL suffix —
" 'I/O' is a computer term of very long standing
that means 'input/output,' i.e. the means by which
a computer communicates with the outside world.
In a domain name, it's a shibboleth that implies
that the intended audience for a site is other
programmers."
— Phil Darnowsky on Dec. 18, 2014
Remarks for a wider audience —
See some Log24 posts related to Dec. 18, 2014.
Comments Off on Galois.io
Tuesday, January 5, 2016
Sunday, January 3, 2016
Focus and Clarity in the New Year
A new finite-geometry site will, I hope,
display the above attributes. It is intended
to have a more discursive approach than
my current files at finitegeometry.org/sc.
Comments Off on Focus and Clarity in the New Year
Friday, January 1, 2016
Art as Religion
A recent phrase from art critic Peter Schjeldahl —
"art in essence, immaculately conceived."
!['No results found in this book [THE LOOM OF GOD] for SYMPLECTIC'](http://www.log24.com/log/pix15A/151031-Pickover-Loom_of_God-search-for-Symplectic.jpg)
But see "symplectic" in this journal.
Comments Off on Art as Religion
Wednesday, December 30, 2015
Inverse Image
The previous post discussed some art related to the
deceptively simple concept of "four colors."
For other related material, see posts that contain a link
to "…mapsys.html."
Comments Off on Inverse Image
Monday, December 28, 2015
ART WARS Continues
Combining two headlines from this morning’s
New York Times and Washington Post , we have…
Deceptively Simple Geometries
on a Bold Scale
Voilà —
Click image for details.
More generally, see
Boole vs. Galois.
Comments Off on ART WARS Continues
Mirrors, Mirrors, on the Wall
The previous post quoted Holland Cotter's description of
the late Ellsworth Kelly as one who might have admired
"the anonymous role of the Romanesque church artist."
Work of a less anonymous sort was illustrated today by both
The New York Times and The Washington Post —
The Post 's remarks are of particular interest:
|
Philip Kennicott in The Washington Post , Dec. 28, 2015, “Sculpture for a Large Wall” consisted of 104 anodized aluminum panels, colored red, blue, yellow and black, and laid out on four long rows measuring 65 feet. Each panel seemed different from the next, subtle variations on the parallelogram, and yet together they also suggested a kind of language, or code, as if their shapes, colors and repeating patterns spelled out a basic computer language, or proto-digital message. The space in between the panels, and the shadows they cast on the wall, were also part of the effect, creating a contrast between the material substance of the art, and the cascading visual and mental ideas it conveyed. The piece was playful, and serious; present and absent; material and imaginary; visually bold and intellectually diaphanous. Often, with Kelly, you felt as if he offered up some ideal slice of the world, decontextualized almost to the point of absurdity. A single arc sliced out of a circle; a single perfect rectangle; one bold juxtaposition of color or shape. But when he allowed his work to encompass more complexity, to indulge a rhetoric of repetition, rhythmic contrasts, and multiple self-replicating ideas, it began to feel like language, or narrative. And this was always his best mode. |
Compare and contrast a 2010 work by Josefine Lyche —
Lyche's mirrors-on-the-wall installation is titled
"The 2×2 Case (Diamond Theorem)."
It is based on a smaller illustration of my own.
These variations also, as Kennicott said of Kelly's,
"suggested a kind of language, or code."
This may well be the source of their appeal for Lyche.
For me, however, such suggestiveness is irrelevant to the
significance of the variations in a larger purely geometric
context.
This context is of course quite inaccessible to most art
critics. Steve Martin, however, has a phrase that applies
to both Kelly's and Lyche's installations: "wall power."
See a post of Dec. 15, 2010.
Comments Off on Mirrors, Mirrors, on the Wall
Sunday, December 27, 2015
Rigorous
Symbol —
Monday, November 7, 2011
|
.
Comments Off on Rigorous
Friday, December 25, 2015
At Play in the Fields
See Fields of Force and recent posts.
From PR Newswire in July 2011 —
Campus Crusade for Christ Adopts New Name: Cru
60-year-old Int’l Ministry Aims to Increase
Relevance and Global Effectiveness
Related material:
Comments Off on At Play in the Fields
Dark Symbol
Related material:
The previous post (Bright Symbol) and
a post from Wednesday,
December 23, 2015, that links to posts
on Boolean algebra vs. Galois geometry.
"An analogy between mathematics and religion is apposite."
— Harvard Magazine review by Avner Ash of
Mathematics without Apologies
(Princeton University Press, January 18, 2015)
Comments Off on Dark Symbol
Thursday, December 24, 2015
Wednesday, December 23, 2015
Splitting Apart
Comments Off on Splitting Apart
Monday, December 21, 2015
Friday, December 18, 2015
Box of Nothing
Images related to the previous post —
Detail of the 1697 Leibniz medal
|
“And so that I won’t come entirely empty-handed this time, I enclose a design of that which I had the pleasure of discussing with you recently. It is in the form of a memorial coin or medallion; and though the design is mediocre and can be improved in accordance with your judgment, the thing is such, that it would be worth showing in silver now and unto future generations, if it were struck at your Highness’s command. Because one of the main points of the Christian Faith, and among those points that have penetrated least into the minds of the worldly-wise and that are difficult to make with the heathen is the creation of all things out of nothing through God’s omnipotence, it might be said that nothing is a better analogy to, or even demonstration of such creation than the origin of numbers as here represented, using only unity and zero or nothing. And it would be difficult to find a better illustration of this secret in nature or philosophy; hence I have set on the medallion design IMAGO CREATIONIS [in the image of creation]. It is no less remarkable that there appears therefrom, not only that God made everything from nothing, but also that everything that He made was good; as we can see here, with our own eyes, in this image of creation. Because instead of there appearing no particular order or pattern, as in the common representation of numbers, there appears here in contrast a wonderful order and harmony which cannot be improved upon…. Such harmonious order and beauty can be seen in the small table on the medallion up to 16 or 17; since for a larger table, say to 32, there is not enough room. One can further see that the disorder, which one imagines in the work of God, is but apparent; that if one looks at the matter with the proper perspective, there appears symmetry, which encourages one more and more to love and praise the wisdom, goodness, and beauty of the highest good, from which all goodness and beauty has flowed.” |
See also some related posts in this journal.
Comments Off on Box of Nothing
Thursday, December 17, 2015
S-Curves by Peter Dickinson
British author Peter Dickinson, who reportedly died yesterday,
Dec. 16, 2015, at 88, wrote the following (published in the UK
in 1975 and in the US in 1976) —
Inverted image of the above page —
See also, from the date of Dickinson's death, a post on
"A Fight for the Soul…" and a post on the symbol "S."
Of interest too are some remarks related to today's earlier post,
"Hint of Reality" …
Comments Off on S-Curves by Peter Dickinson
Hint of Reality
From an article* in Proceedings of Bridges 2014 —
|
As artists, we are particularly interested in the symmetries of real world physical objects. Three natural questions arise: 1. Which groups can be represented as the group of symmetries of some real-world physical object? 2. Which groups have actually been represented as the group of symmetries of some real-world physical object? 3. Are there any glaring gaps – small, beautiful groups that should have a physical representation in a symmetric object but up until now have not? |
The article was cited by Evelyn Lamb in her Scientific American
weblog on May 19, 2014.
The above three questions from the article are relevant to a more
recent (Oct. 24, 2015) remark by Lamb:
"… finite projective planes [in particular, the 7-point Fano plane,
about which Lamb is writing] seem like a triumph of purely
axiomatic thinking over any hint of reality…."
For related hints of reality, see Eightfold Cube in this journal.
* "The Quaternion Group as a Symmetry Group," by Vi Hart and Henry Segerman
Comments Off on Hint of Reality
Tuesday, December 15, 2015
Square Triangles
Click image for some background.
Exercise: Note that, modulo color-interchange, the set of 15 two-color
patterns above is invariant under the group of six symmetries of the
equilateral triangle. Are there any other such sets of 15 two-color triangular
patterns that are closed as sets , modulo color-interchange, under the six
triangle symmetries and under the 322,560 permutations of the 16
subtriangles induced by actions of the affine group AGL(4,2)
on the 16 subtriangles' centers , given a suitable coordinatization?
Comments Off on Square Triangles
Monday, December 14, 2015
The Forking
From the previous post:
"Neat, Dr. Walker, thought Peter Slater—
neat, and totally without content."
— Paul Preuss's 1983 novel Broken Symmetries
A background check yields …
"Dr. Evan Harris Walker died on the evening of
August 17, 2006…."
A synchronicity check of that date in this journal yields a diagram
that, taken by itself, is "neat, and totally without content." —
The diagram may be viewed as a tribute
to the late Yogi Berra, to the literary
"Garden of Forking Paths," or, more
seriously, to the modular group Γ.
Comments Off on The Forking
Dirac and Geometry
See a post by Peter Woit from Sept. 24, 2005 — Dirac's Hidden Geometry.
The connection, if any, with recent Log24 posts on Dirac and Geometry
is not immediately apparent. Some related remarks from a novel —
|
From Broken Symmetries by Paul Preuss "He pondered the source of her fascination with the occult, which sooner or later seemed to entangle a lot of thoughtful people who were not already mired in establishmentarian science or religion. It was the religious impulse, at base. Even reason itself could function as a religion, he supposed— but only for those of severely limited imagination. He’d toyed with 'psi' himself, written a couple of papers now much quoted by crackpots, to his chagrin. The reason he and so many other theoretical physicists were suckers for the stuff was easy to understand— for two-thirds of a century an enigma had rested at the heart of theoretical physics, a contradiction, a hard kernel of paradox. Quantum theory was inextricable from the uncertainty relations. The classical fox knows many things, but the quantum-mechanical hedgehog knows only one big thing— at a time. 'Complementarity,' Bohr had called it, a rubbery notion the great professor had stretched to include numerous pairs of opposites. Peter Slater was willing to call it absurdity, and unlike some of his older colleagues who, following in Einstein’s footsteps, demanded causal explanations for everything (at least in principle), Peter had never thirsted after 'hidden variables' to explain what could not be pictured. Mathematical relationships were enough to satisfy him, mere formal relationships which existed at all times, everywhere, at once. It was a thin nectar, but he was convinced it was the nectar of the gods. The psychic investigators, on the other hand, demanded to know how the mind and the psychical world were related. Through ectoplasm, perhaps? Some fifth force of nature? Extra dimensions of spacetime? All these naive explanations were on a par with the assumption that psi is propagated by a species of nonlocal hidden variables, the favored explanation of sophisticates; ignotum per ignotius . 'In this connection one should particularly remember that the human language permits the construction of sentences which do not involve any consequences and which therefore have no content at all…' The words were Heisenberg’s, lecturing in 1929 on the irreducible ambiguity of the uncertainty relations. They reminded Peter of Evan Harris Walker’s ingenious theory of the psi force, a theory that assigned psi both positive and negative values in such a way that the mere presence of a skeptic in the near vicinity of a sensitive psychic investigation could force null results. Neat, Dr. Walker, thought Peter Slater— neat, and totally without content. One had to be willing to tolerate ambiguity; one had to be willing to be crazy. Heisenberg himself was only human— he’d persuasively woven ambiguity into the fabric of the universe itself, but in that same set of 1929 lectures he’d rejected Dirac’s then-new wave equations with the remark, 'Here spontaneous transitions may occur to the states of negative energy; as these have never been observed, the theory is certainly wrong.' It was a reasonable conclusion, and that was its fault, for Dirac’s equations suggested the existence of antimatter: the first antiparticles, whose existence might never have been suspected without Dirac’s crazy results, were found less than three years later. Those so-called crazy psychics were too sane, that was their problem— they were too stubborn to admit that the universe was already more bizarre than anything they could imagine in their wildest dreams of wizardry." |
Particularly relevant …
"Mathematical relationships were enough to satisfy him,
mere formal relationships which existed at all times,
everywhere, at once."
Some related pure mathematics —
Comments Off on Dirac and Geometry
Sunday, December 13, 2015
The Monster as Big as the Ritz
"The colorful story of this undertaking begins with a bang."
— Martin Gardner on the death of Évariste Galois
Comments Off on The Monster as Big as the Ritz
Tuesday, December 8, 2015
Conceptual Art
A December 7th New York Times column:
A current exhibition by Joseph Kosuth in Oslo:
From the two texts by Mondrian at the right hand of Kosuth —
"The positive and negative states of being bring about action."
"Through its pure relationships, purely abstract art
can approach the expression of the universal …."
These texts may be viewed as glosses on the following image —
Click image for related posts.
Comments Off on Conceptual Art
Church for Rebecca
"Remember, Genesis IS Skynet."
Bloomberg News today:
Why 2015 Was a Breakthrough Year in Artificial Intelligence
"Computers are 'starting to open their eyes,' said a senior fellow at Google."
Comments Off on Church for Rebecca
Monday, December 7, 2015
Wittgenstein Illustrated
From "AMNESIA: VARIOUS, LUMINOUS, FIXED,"
An exhibition by Joseph Kosuth at
Sprüth Magers Gallery London,
NOVEMBER 26 2014 – FEBRUARY 14 2015 —
This journal, NOVEMBER 26 2014 –
|
Wednesday, November 26, 2014
Mathematics and Narrative
|
This journal, FEBRUARY 14 2015 —
Comments Off on Wittgenstein Illustrated
Sunday, December 6, 2015
Form and Idea
"Those early works are succinct and uncompromising
in how they give shape to the philosophical perplexities
of form and idea…."
J. J. Charlesworth, artnet news, Dec. 16, 2014
"Form" and "idea" are somewhat synonymous,
as opposed to "form" and "substance." A reading:
Comments Off on Form and Idea
Thursday, December 3, 2015
Overarching Symmetry
From p. 34 of the preprint "Snapshots of Conformal Field Theory,"
by Katrin Wendland, arXiv, 11 April 2014 —
50. Gannon, T.: Much ado about Mathieu (arXiv:1211.5531 [math.RT])
85. Taormina, A., Wendland, K.: The overarching finite symmetry group
of Kummer surfaces in the Mathieu group M24. JHEP 08, 125 (2013)
86. Taormina, A., Wendland, K.: Symmetry-surfing the moduli space
of Kummer K3s (arXiv:1303.2931 [hep-th])
87. Taormina, A., Wendland, K.: A twist in the M24 moonshine story
(arXiv:1303.3221 [hep-th])
The Wendland paper was published on Jan. 7, 2015, in
Mathematical Aspects of Quantum Field Theories ,
edited by Damien Calaque and Thomas Strobl
(Springer Mathematical Physics Studies), pages 89-129.
Comments Off on Overarching Symmetry