Figures from a search in this journal for Springer Knight
and from the All Souls' Day post The Trojan Pony —
For those who prefer pure abstraction to the quasi-figurative
1985 seven-cycle above, a different 7-cycle for M24 , from 1998 —
Compare and contrast with my own "knight" labeling
of a 4-row 2-column array (an M24 octad, in the system
of R. T. Curtis) by the 8 points of the projective line
over GF(7), from 2008 —
From a search in this journal for Springer Knight —
Related material from Academia —
See also Log24 posts from the above "magic" date,
December 4, 2014, now tagged The Pony Argument.
"The definition of easy to learn, hard to master"
— Alex Hern in The Guardian today on the game of Go
Not unlike music, mathematics, and chess.
For illustrations based on the above equations, see
Coxeter and the Relativity Problem and Singer 7-Cycles .
A Necessary Truth—
James Singer, "A Theorem in Finite Projective Geometry
and Some Applications to Number Theory," Transactions
of the American Mathematical Society 43 (1938), 377-385.
A Contingent Truth—
Singer Tony Martin reportedly died Friday evening, July 27, 2012.
In his memory, some references to a "Singer 7-Cycle."
See also this journal 7 years prior to Martin's death.
| Saturday, November 12, 2005
— m759 @ 8:00 PM (continued)
“… problems are the poetry of chess. |
From today's NY Times—
Obituaries for mystery authors
Ralph McInerny and Dick Francis
From the date (Jan. 29) of McInerny's death–
"…although a work of art 'is formed around something missing,' this 'void is its vanishing point, not its essence.'"
– Harvard University Press on Persons and Things (Walpurgisnacht, 2008), by Barbara Johnson
From the date (Feb. 14) of Francis's death–
The EIghtfold Cube
The "something missing" in the above figure is an eighth cube, hidden behind the others pictured.
This eighth cube is not, as Johnson would have it, a void and "vanishing point," but is instead the "still point" of T.S. Eliot. (See the epigraph to the chapter on automorphism groups in Parallelisms of Complete Designs, by Peter J. Cameron. See also related material in this journal.) The automorphism group here is of course the order-168 simple group of Felix Christian Klein.
For a connection to horses, see
a March 31, 2004, post
commemorating the birth of Descartes
and the death of Coxeter–
Putting Descartes Before Dehors
For a more Protestant meditation,
see The Cross of Descartes—
"I've been the front end of a horse
and the rear end. The front end is better."
— Old vaudeville joke
For further details, click on
the image below–
Notre Dame Philosophical Reviews
More Than Matter
| Wheel in Webster’s Revised Unabridged Dictionary, 1913
(f) Poetry The burden or refrain of a song. ⇒ “This meaning has a low degree of authority, but is supposed from the context in the few cases where the word is found.” Nares. You must sing a-down a-down, An you call him a-down-a. O, how the wheel becomes it! Shak. |
“In one or other of G. F. H. Shadbold’s two published notebooks, Beyond Narcissus and Reticences of Thersites, a short entry appears as to the likelihood of Ophelia’s enigmatic cry: ‘Oh, how the wheel becomes it!’ referring to the chorus or burden ‘a-down, a-down’ in the ballad quoted by her a moment before, the aptness she sees in the refrain.”
— First words of Anthony Powell’s novel “O, How the Wheel Becomes It!” (See Library Thing.)
Related material:
Photo uploaded on January 14, 2009
with caption “This nothing’s more than matter”
and the following nothings from this journal
on the same date– Jan. 14, 2009—
Singer 7-Cycles
Click on images for details.
The 1985 Cullinane version gives some algebraic background for the 1987 Curtis version.
The Singer referred to above is James Singer. See his "A Theorem in Finite Projective Geometry and Some Applications to Number Theory," Transactions of the American Mathematical Society 43 (1938), 377-385.For other singers, see Art Wars and today's obituaries.
Some background: the Log24 entry of this date seven years ago, and the entries preceding it on Las Vegas and painted ponies.
Other knight figures:
Click on the SpringerLink
knight for a free copy
(pdf, 1.2 mb) of
the following paper
dealing with the geometry
underlying the R.T. Curtis
knight figures above:
Context:
Literature and Chess and
Sporadic Group References
Details:
| Adapted (for HTML) from the opening paragraphs of the above paper, W. Jonsson's 1970 "On the Mathieu Groups M22, M23, M24…"–
"[A]… uniqueness proof is offered here based upon a detailed knowledge of the geometric aspects of the elementary abelian group of order 16 together with a knowledge of the geometries associated with certain subgroups of its automorphism group. This construction was motivated by a question posed by D.R. Hughes and by the discussion Edge [5] (see also Conwell [4]) gives of certain isomorphisms between classical groups, namely
where A8 is the alternating group on eight symbols, S6 the symmetric group on six symbols, Sp(4,2) and PSp(4,2) the symplectic and projective symplectic groups in four variables over the field GF(2) of two elements, [and] PGL, PSL and SL are the projective linear, projective special linear and special linear groups (see for example [7], Kapitel II). The symplectic group PSp(4,2) is the group of collineations of the three dimensional projective space PG(3,2) over GF(2) which commute with a fixed null polarity tau…." References 4. Conwell, George M.: The three space PG(3,2) and its group. Ann. of Math. (2) 11, 60-76 (1910). 5. Edge, W.L.: The geometry of the linear fractional group LF(4,2). Proc. London Math. Soc. (3) 4, 317-342 (1954). 7. Huppert, B.: Endliche Gruppen I. Berlin-Heidelberg-New York: Springer 1967. |
Eight is a Gate
From the most highly
rated negative review:
“I never did figure out
what ‘The Eight’ was.”
Various approaches
to this concept
(click images for details):
“There is a pleasantly discursive treatment of Pontius Pilate’s unanswered question ‘What is truth?'”
— H. S. M. Coxeter, 1987, introduction to Richard J. Trudeau’s remarks on the “Story Theory” of truth as opposed to the “Diamond Theory” of truth in The Non-Euclidean Revolution
“A new epistemology is emerging to replace the Diamond Theory of truth. I will call it the ‘Story Theory’ of truth: There are no diamonds. People make up stories about what they experience. Stories that catch on are called ‘true.’ The Story Theory of truth is itself a story that is catching on. It is being told and retold, with increasing frequency, by thinkers of many stripes*….”
— Richard J. Trudeau in
The Non-Euclidean Revolution
“‘Deniers’ of truth… insist that each of us is trapped in his own point of view; we make up stories about the world and, in an exercise of power, try to impose them on others.”
— Jim Holt in The New Yorker.
(Click on the box below.)
Exercise of Power:
Show that a white horse–
a figure not unlike the
symbol of the mathematics
publisher Springer–
is traced, within a naturally
arranged rectangular array of
polynomials, by the powers of x
modulo a polynomial
irreducible over a Galois field.
This horse, or chess knight–
“Springer,” in German–
plays a role in “Diamond Theory”
(a phrase used in finite geometry
in 1976, some years before its use
by Trudeau in the above book).
Related material
On this date:
In 1490, The White Knight
(Tirant lo Blanc 
)–
a major influence on Cervantes–
was published, and in 1910
the Mexican Revolution began.
Illustration:
Zapata by Diego Rivera,
Museum of Modern Art,
New York
“First published in the Catalan language in Valencia in 1490…. Reviewing the first modern Spanish translation in 1969 (Franco had ruthlessly suppressed the Catalan language and literature), Mario Vargas Llosa hailed the epic’s author as ‘the first of that lineage of God-supplanters– Fielding, Balzac, Dickens, Flaubert, Tolstoy, Joyce, Faulkner– who try to create in their novels an all-encompassing reality.'”
“… problems are the poetry of chess.
They demand from the composer
the same virtues that characterize
all worthwhile art:
originality, invention,
harmony, conciseness,
complexity, and
splendid insincerity.”
Into the Sunset
I just learned of Johnny’s Cash’s death. On Google News, the headline was Johnny Cash rides into sunset. The source was the Bangkok Post.
“Don’t you know that
when you play at this level
there’s no ordinary venue.”
— One Night in Bangkok (midi)
No Ordinary Venue
“They are the horses of a dream.
They are not what they seem.”
— The Hex Witch of Seldom, page 16
A Singer
7-Cycle
The Magnificent Seven:
CLICK HERE for
“the adventures of filming this epic
on location in Cuernavaca, Mexico.”
|
“He is the outlaw the people love, — The Hex Witch of Seldom, |
“Words are events.”
— Walter J. Ong, Society of Jesus
“…search for thirty-three and three…”
— The Black Queen in The Eight
Commentary
on the two previous entries
On 4:04:08:
“Je ne connais que deux sortes d’êtres immuables sur la terre: les géomètres et les animaux; ils sont conduits par deux règles invariables la démonstration et l’instinct; et encore les géomètres ont-ils eu quelques disputes, mais les animaux n’ont jamais varié.”
— Voltaire, Dictionnaire Philosophique, “Des Contradictions dans les Affaires et dans les Hommes“
On 4:04:08
and on
Particularity:
“El pan que se come no es pan.”
— Voltaire quoting Montesquieu
on the Pope’s declarations,
Spanish translation
ART WARS:
Toward Eternity
April is Poetry Month, according to the Academy of American Poets. It is also Mathematics Awareness Month, funded by the National Security Agency; this year's theme is "Mathematics and Art."
Some previous journal entries for this month seem to be summarized by Emily Dickinson's remarks:
"Because I could not stop for Death–
He kindly stopped for me–
The Carriage held but just Ourselves–
And Immortality.
|
Math Awareness Month April is Math Awareness Month.
|
|
An Offer He Couldn't Refuse Today's birthday: Francis Ford Coppola is 64.
From a note on geometry of April 28, 1985:
|
|
The Eight Today, the fourth day of the fourth month, plays an important part in Katherine Neville's The Eight. Let us honor this work, perhaps the greatest bad novel of the twentieth century, by reflecting on some properties of the number eight. Consider eight rectangular cells arranged in an array of four rows and two columns. Let us label these cells with coordinates, then apply a permutation.
The resulting set of arrows that indicate the movement of cells in a permutation (known as a Singer 7-cycle) outlines rather neatly, in view of the chess theme of The Eight, a knight. This makes as much sense as anything in Neville's fiction, and has the merit of being based on fact. It also, albeit rather crudely, illustrates the "Mathematics and Art" theme of this year's Mathematics Awareness Month. The visual appearance of the "knight" permutation is less important than the fact that it leads to a construction (due to R. T. Curtis) of the Mathieu group M24 (via the Curtis Miracle Octad Generator), which in turn leads logically to the Monster group and to related "moonshine" investigations in the theory of modular functions. See also "Pieces of Eight," by Robert L. Griess. |
Art Wars:
Mathematics and the
Emperor's New Art
From Maureen Dowd's New York Times column of June 9, 2002:
"The shape of the government is not as important as the policy of the government. If he makes the policy aggressive and pre-emptive, the president can conduct the war on terror from the National Gallery of Art."
|
NY Times, April 5, 2003: |
|
Meanwhile, at the Washington Post, another example of great determination and strength of character:
Donald Coxeter Dies: Leader in Geometry
By Martin Weil
Washington Post Staff Writer
Saturday, April 5, 2003
"Donald Coxeter, 96, a mathematician who was one of the 20th century's foremost specialists in geometry and a man of great determination and strength of character as well, died March 31 at his home in Toronto."
From another Coxeter obituary:
In the Second World War, Coxeter was asked by the American government to work in Washington as a code-breaker. He accepted, but then backed out, partly because of his pacifist views and partly for aesthetic reasons: "The work didn't really appeal to me," he explained; "it was a different sort of mathematics."
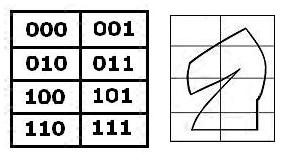
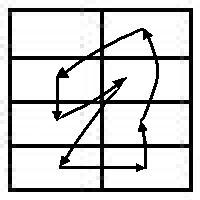
For a differing account of how geometry is related to code-breaking, see the "Singer 7-cycle" link in yesterday's entry, "The Eight," of 3:33 PM. This leads to a site titled
An Introduction to the
Applications of Geometry in Cryptography.
"Now I have precisely the right instrument, at precisely the right moment of history, in exactly the right place."
— "Patton,"
the film
Added Sunday, April 6, 2003, 3:17 PM:
The New York Times Magazine of April 6
continues this Art Wars theme.
(Cover typography revised)
The military nature of our Art Wars theme appears in the Times's choice of words for its cover headline: "The Greatest Generation." (This headline appears in the paper, but not the Internet, version.)
Some remarks in today's Times Magazine article seem especially relevant to my journal entry for Michelangelo's birthday, March 6.
"…Conceptualism — suddenly art could be nothing more than an idea….
LeWitt moved between his syntax of geometric sculptures and mental propositions for images: concepts he wrote on paper that could be realized by him or someone else or not at all. Physical things are perishable. Ideas need not be."
— Michael Kimmelman, chief art critic of the New York Times, April 6, 2003
Compare this with a mathematician's aesthetics:
"A mathematician, like a painter or a poet, is a maker of patterns. If his patterns are more permanent than theirs, it is because they are made with ideas."
— G. H. Hardy, A Mathematician's Apology (1940), reprinted 1969, Cambridge U. Press, p. 84
It seems clear from these two quotations that the real conceptual art is mathematics and that Kimmelman is peddling the emperor's new clothes.
The Eight
Today, the fourth day of the fourth month, plays an important part in Katherine Neville's The Eight. Let us honor this work, perhaps the greatest bad novel of the twentieth century, by reflecting on some properties of the number eight. Consider eight rectangular cells arranged in an array of four rows and two columns. Let us label these cells with coordinates, then apply a permutation.
|
|
|
|
|
The resulting set of arrows that indicate the movement of cells in a permutation (known as a Singer 7-cycle) outlines rather neatly, in view of the chess theme of The Eight, a knight. This makes as much sense as anything in Neville's fiction, and has the merit of being based on fact. It also, albeit rather crudely, illustrates the "Mathematics and Art" theme of this year's Mathematics Awareness Month. (See the 4:36 PM entry.)
The visual appearance of the "knight" permutation is less important than the fact that it leads to a construction (due to R. T. Curtis) of the Mathieu group M24 (via the Curtis Miracle Octad Generator), which in turn leads logically to the Monster group and to related "moonshine" investigations in the theory of modular functions. See also "Pieces of Eight," by Robert L. Griess.
Powered by WordPress
