
A star figure and the Galois quaternion.
The square root of the former is the latter.
"… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte."

A star figure and the Galois quaternion.
The square root of the former is the latter.
"… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte."
The reference to David Justice at the beginning of
yesterday afternoon's post does not imply an
endorsement of all his writings. For instance, a
Justice post from yesterday contains the following—

Correction—
The above author name and page number are wrong.

Related to the above "fundamental theme" —
Three Notes on Design
1. From the Museum of Modern Art today—
“It’s a very nice gesture of a kind of new ethos:
To make publicly accessible, unticketed space
that is attractive and has cultural programming,”
Glenn D. Lowry, MoMA’s director, said.
2. From The New York Times today—
3. From myself last December—
The title refers to that of today's previous post, which linked to
a song from the June 1, 1983, album Synchronicity .
(Cf. that term in this journal.)
For some work of my own from the following year, 1984, see…
… as well as the Orwellian dictum Triangles Are Square.
(The cubical figure at left above is from the same month,
if not the same day, as Synchronicity — June 21, 1983.)
"… the human will cannot be simultaneously
triumphant and imaginary."
— Ross Douthat, Defender of the Faith,
in this afternoon's New York Times at 3:25* PM ET
Some— even some Catholics— might say the will
cannot be triumphant unless imaginary.
Related material: The Galois Quaternion: A Story.
See also C. S. Lewis on enchantment.
* Cf., in this journal, the most recent 3/25 ,
and a bareword —
Click image for some context.
Click for a larger image.
For a different pictorial approach, see Polster's
1998 Geometrical Picture Book , pp. 77-80.
Update: Added to finitegeometry.org on Jan. 2, 2014.
(The source of the images of the 35 lines was the image
"Geometry of the Six-Element Set," with, in the final two
of the three projective-line parts, the bottom two rows
and the rightmost two columns interchanged.)
Continued from December 25—

A link from Sunday afternoon to Nov. 26, 2012,
suggests a review of one of the above structures.

The Dreaming Jewels cover at left is taken from a review
by Jo Walton at Tor.com—
"This is a book that it’s clearly been difficult
for publishers to market. The covers have been
generally pretty awful, and also very different.
I own a 1975 Corgi SF Collectors Library
paperback that I bought new for 40p in the later
seventies. It’s purple, and it has a slightly grainy
cover, and it matches my editions of The Menace
From Earth and A Canticle for Leibowitz .
(Dear old Corgi SF Collectors Editions with their
very seventies fonts! How I imprinted on them at
an early age!) I mention this, however, because
the (uncredited) illustration actually represents and
illustrates the book much better than any of the other
cover pictures I’ve seen. It shows a hexagon with an
attempt at facets, a man, a woman, hands, a snake,
and stars, all in shades of green. It isn’t attractive,
but it wouldn’t put off people who’d enjoy what’s inside
either."
The "hexagon with an attempt at facets" is actually
an icosahedron, as the above diagram shows.
(The geometric part of the diagram is from a Euclid webpage.)
For Plato's dream about these jewels, see his Timaeus.
“Design is how it works.” — Steve Jobs
“By far the most important structure in design theory
is the Steiner system S(5, 8, 24).”
— “Block Designs,” by Andries E. Brouwer (Ch. 14 (pp. 693-746),
Section 16 (p. 716) of Handbook of Combinatorics, Vol. I ,
MIT Press, 1995, edited by Ronald L. Graham, Martin Grötschel,
and László Lovász)
For some background on that Steiner system, see the footnote to
yesterday’s Christmas post.
“… her mind rotated the facts….”
Related material— hypercube rotation,* in the context
of rotational symmetries of the Platonic solids:

“I’ve heard of affairs that are strictly Platonic”
* Footnote added on Dec. 26, 2013 —
See Arnold Emch, “Triple and Multiple Systems, Their Geometric
Configurations and Groups,” Trans. Amer. Math. Soc. 31 (1929),
No. 1, 25–42.
On page 42, Emch describes the above method of rotating a
hypercube’s 8 facets (i.e., three-dimensional cubes) to count
rotational symmetries —
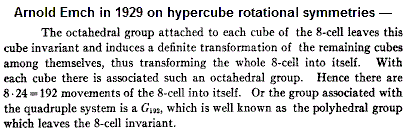
See also Diamond Theory in 1937.
Also on p. 42, Emch mentions work of Carmichael on a
Steiner system with the Mathieu group M11 as automorphism
group, and poses the problem of finding such systems and
groups that are larger. This may have inspired the 1931
discovery by Carmichael of the Steiner system S(5, 8, 24),
which has as automorphisms the Mathieu group M24 .

"She never looked up while her mind rotated the facts,
trying to see them from all sides, trying to piece them
together into theory. All she could think was that she
was flunking an IQ test."
— Steve Martin, An Object of Beauty
"So you should not feel so all alone…"
— Adapted song lyric
The title is taken from a book for ages 8-12 published
on Shakespeare's birthday, April 23, 2013.
Also from that date, a note for older readers—

… Half a dozen of the other —

For further context, see all posts for the cruelest month of this past year.
* Secrets : A sometimes dangerous word.
The Miami-Dade County Public Schools math webpage
now has a link to the Diamond 16 Puzzle.
(On His Dies Natalis )…
This is asserted in an excerpt from…
"The smallest non-rank 3 strongly regular graphs
which satisfy the 4-vertex condition"
by Mikhail Klin, Mariusz Meszka, Sven Reichard, and Alex Rosa,
BAYREUTHER MATHEMATISCHE SCHRIFTEN 73 (2005), 152-212—
(Click for clearer image)
Note that Theorem 46 of Klin et al. describes the role
of the Galois tesseract in the Miracle Octad Generator
of R. T. Curtis (original 1976 version). The tesseract
(a 4×4 array) supplies the geometric part of the above
exceptional geometric-combinatorial isomorphism.
(This morning's Text and Pretext, continued)
"… a reality that only my notes can provide."
— Kinbote in Nabokov's novel Pale Fire
Click the above remarks on screws for another perspective on reality.
Microsoft in 2009 on its new search engine name—
"We like Bing because it sounds off in our heads
when we think about that moment of discovery
and decision making— when you resolve those
important tasks."
A search on Bing today —
A colorful tale —
"How about another hand for the band?
They work real hard for it.
The Cherokee Cowboys, ladies and gentlemen."
— Ray Price, video, "Danny Boy Mid 80's Live"

Other deathly hallows suggested by today's NY Times—
Click the above image for posts from December 14.
That image mentions a death on August 5, 2005, in
"entertainment Mecca" Branson, Missouri.
Another note from August 5, 2005, reposted here
on Monday—

Happy birthday, Keith Richards.
The above image is from Geometry of the 4×4 Square.
(The link "Visible Mathematics" in today's previous post, Quartet,
led to a post linked to that page, among others.)
Note that the seventh square above, at top right of the array of 35,
is the same as the image in Quartet.

Happy Beethoven's Birthday.
Related material: Abel 2005 and, more generally, Abel.
See also Visible Mathematics.
Odin's Jewel
Jim Holt, the author of remarks in yesterday's
Saturday evening post—
"It turns out that the Kyoto school of Buddhism
makes Heidegger seem like Rush Limbaugh—
it’s so rarified, I’ve never been able to
understand it at all. I’ve been knocking my head
against it for years."
— Vanity Fair Daily , July 16, 2012

Backstory: Odin + Jewel in this journal.
See also Odin on the Kyoto school —
For another version of Odin's jewel, see Log24
on the date— July 16, 2012— that Holt's Vanity Fair
remarks were published. Scroll to the bottom of the
"Mapping Problem continued" post for an instance of
the Galois tesseract —
The title, which I dislike, is taken from a 2011 publication
of the MAA, also sold by Cambridge University Press.
Some material relevant to the title adjective:
| "For those who have learned something of higher mathematics, nothing could be more natural than to use the word 'beautiful' in connection with it. Mathematical beauty, like the beauty of, say, a late Beethoven quartet, arises from a combination of strangeness and inevitability. Simply defined abstractions disclose hidden quirks and complexities. Seemingly unrelated structures turn out to have mysterious correspondences. Uncanny patterns emerge, and they remain uncanny even after being underwritten by the rigor of logic."— Jim Holt, opening of a book review in the Dec. 5, 2013, issue of The New York Review of Books |
Some relevant links—
The above list was updated on Jan. 31, 2014, to include the
"Strangeness" and "Hidden quirks" links. See also a post of
Jan. 31, 2014.
Update of March 9, 2014 —
The link "Simply defined abstractions" is to the construction of the Steiner
system S(5, 8, 24) described by R. T. Curtis in his 1976 paper defining the
Miracle Octad Generator. It should be noted that this construction is due
to Richard J. Turyn, in a 1967 Sylvania research report. (See Emily Jennings's
talk of 1 Nov. 2012.) Compare the Curtis construction, written in 1974,
with the Turyn construction of 1967 as described in Sphere Packings, Lattices
and Groups , by J. H. Conway and N. J. A. Sloane (first published in 1988).
"… Galois was a mathematical outsider…."
— Tony Mann, "head of the department of mathematical sciences,
University of Greenwich, and president, British Society for the
History of Mathematics," in a May 6, 2010, review of Duel at Dawn
in Times Higher Education.
Related art:
(Click for a larger image.)
For a less outside version of the central image
above, see Kunstkritikk on Oct. 15, 2013.
See also last night's "Pink Champagne on Ice" post.
The "ice" in that post's title refers to the white lines
forming a tesseract in the book cover's background—
"icy white and crystalline," as Johnny Mercer put it.
(A Tune for Josefine, Nov. 25.)
See also the tag Diamond Theory tesseract in this journal.
An I Ching study quoted in Waiting for Ogdoad (St. Andrew's Day, 2013)—
(Click for clearer image.)
The author of the above I Ching study calls his lattice "Arising Heaven."
The following lattice might, therefore, be called "Heaven Descending."
Click for the source, mentioned in Anatomy of a Cube (Sept. 18, 2011).
A new website illustrates its URL.
See DiamondSpace.net.
See my Google Sites page if you would like to
download a zipped copy (31 MB) of my
Finite-Geometry Notes site
(not zipped, at finitegeometry.org/sc/map.html).
Or you can of course use a website downloader.
(Suggested by a recent NY Times piece on
a company, Citia, that splits books into pieces
for easier electronic access. The large zipped
file referred to above is sort of a reverse of this
process.)
On St. Andrew's Day.
A Connery adventure in Kuala Lumpur—
For another Kuala Lumpur adventure, see today's update
to "In Defense of Plato's Realism"—
The July 5, 2007, post linked to
"Plato, Pegasus, and the Evening Star."
For related drama from Kuala Lumpur, see
"Occam's Razor, Plato's Beard."
Continued from October 30 (Devil’s Night), 2013.
“In a sense, we would see that change
arises from the structure of the object.”
— Theoretical physicist quoted in a
Simons Foundation article of Sept. 17, 2013
This suggests a review of mathematics and the
“Classic of Change ,” the I Ching .
The physicist quoted above was discussing a rather
complicated object. His words apply to a much simpler
object, an embodiment of the eight trigrams underlying
the I Ching as the corners of a cube.
See also…
(Click for clearer image.)
The Cullinane image above illustrates the seven points of
the Fano plane as seven of the eight I Ching trigrams and as
seven natural ways of slicing the cube.
For a different approach to the mathematics of cube slices,
related to Gauss’s composition law for binary quadratic forms,
see the Bhargava cube in a post of April 9, 2012.
The title refers to a post from July 2012:
The above post, a new description of a class of figurate
numbers that has been studied at least since Pythagoras,
shows that the "triangular numbers" of tradition are not
the only triangular numbers.
"Thus the theory of description matters most.
It is the theory of the word for those
For whom the word is the making of the world…."
— Wallace Stevens, "Description Without Place"
See also Finite Relativity (St. Cecilia's Day, 2012).
"Bobbies on bicycles two by two…" — Roger Miller, 1965
A mathematics weblog in Australia today—
Clearly, the full symmetric group contains elements
with no regular cycles, but what about other groups?
Siemons and Zalesskii showed that for any group G
between PSL(n,q) and PGL(n,q) other than for
(n,q)=(2,2) or (2,3), then in any action of G, every
element of G has a regular cycle, except G=PSL(4,2)
acting on 8 points. The exceptions are due to
isomorphisms with the symmetric or alternating groups.

Yes. See …
The 48 actions of GL(2,3) on a 3×3 coordinate-array A,
when matrices of that group right-multiply the elements of A,
with A =
| (1,1) (1,0) (1,2) (0,1) (0,0) (0,2) (2,1) (2,0) (2,2) |
Actions of GL(2,p) on a pxp coordinate-array have the
same sorts of symmetries, where p is any odd prime.
Note that A, regarded in the Sallows manner as a magic square,
has the constant sum (0,0) in rows, columns, both diagonals, and
all four broken diagonals (with arithmetic modulo 3).
For a more sophisticated approach to the structure of the
ninefold square, see Coxeter + Aleph.

From the obituary of a Bletchley Park
codebreaker who reportedly died on
Armistice Day (Monday, Nov. 11)—
"The main flaw of the Enigma machine,
seen by the inventors as a security-enhancing
measure, was that it would never encipher
a letter as itself…."
Update of 9 PM ET Nov. 13—
"The rogue’s yarn that will run through much of
the material is the algebraic symmetry to which
the name of Galois is attached…."
— Robert P. Langlands,
Institute for Advanced Study, Princeton
"All the turmoil, all the emotions of the scenes
have been digested by the mind into
a grave intellectual whole. It is as though
Bach had written the 1812 Overture."
— Aldous Huxley, "The Best Picture," 1925
“DEVIL – MUSIC
20 pages of incidental music written at school
for G. K. Chesterton’s play MAGIC
by D. Coxeter.”
See also…
Related material — Chesterton + Magic in this journal.
Or: The Nutshell

What about Pascal?
For some background on Pascal's mathematics,
not his wager, see…
Richmond, H. W.,
"On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics ,
Volume 31 (1900), pp. 125-160,
dated by Richmond March 30,1899
Richmond, H. W.,
"The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen ,
Volume 53 (1900), Issue 1-2, pp 161-176,
dated by Richmond February 1, 1899
See also Nocciolo in this journal.
Recall as well that six points in space may,
if constrained to lie on a circle, be given
a religious interpretation. Richmond's
six points are secular and more general.
Yesterday afternoon's post linked to efforts by
the late Robert de Marrais to defend a mathematical
approach to structuralism and kaleidoscopic patterns.
Two examples of non-mathematical discourse on
such patterns:
1. A Royal Society paper from 2012—
Click the above image for related material in this journal.
2. A book by Junichi Toyota from 2009—
Kaleidoscopic Grammar: Investigation into the Nature of Binarism
I find such non-mathematical approaches much less interesting
than those based on the mathematics of reflection groups .
De Marrais described the approaches of Vladimir Arnold and,
earlier, of H. S. M. Coxeter, to such groups. These approaches
dealt only with groups of reflections in Euclidean spaces.
My own interest is in groups of reflections in Galois spaces.
See, for instance, A Simple Reflection Group of Order 168.
Galois spaces over fields of characteristic 2 are particularly
relevant to what Toyota calls binarism .
In "Notes on Finite Group Theory"
by Peter J. Cameron (October 2013),
http://www.maths.qmul.ac.uk/~pjc/notes/gt.pdf,
some parts are particularly related to the mathematics of
the 4×4 square (viewable in various ways as four quartets)—
Cameron is the author of Parallelisms of Complete Designs ,
a book notable in part for its chapter epigraphs from T.S. Eliot's
Four Quartets . These epigraphs, if not the text proper, seem
appropriate for All Saints' Day.
But note also Log24 posts tagged Not Theology.
The title is from an essay by James C. Nohrnberg—
"Just another shake of the kaleidoscope" —
Related material:
Kaleidoscope Puzzle,
Design Cube 2x2x2, and
Through the Looking Glass: A Sort of Eternity.
From the AP Today in History page
for October 28, 2013 —

From this journal seven years ago:

Recommended.
The Whitney Museum of American Art has stated
that artist Frank Stella in 1959
"wanted to create work that was methodical,
intellectual and passionless."
Source: Whitney Museum, transcript of audio guide.
Related material:
A figure from this journal on July 13, 2003…

… and some properties of that figure.
New! Improved!
"Euclid's edifice loomed in my consciousness
as a marvel among sciences, unique in its
clarity and unquestionable validity."
—Richard J. Trudeau in
The Non-Euclidean Revolution (First published in 1986)
Readers of this journal will be aware that Springer's new page
advertising Trudeau's book, pictured above, is a bait-and-switch
operation. In the chapter advertised, Trudeau promotes what he
calls "the Diamond Theory of Truth" as a setup for his real goal,
which he calls "the Story Theory of Truth."
For an earlier use of the phrase "Diamond Theory" in
connection with geometry, see a publication from 1977.
Yesterday's post on epistemology and geometry
suggests an Amazon customer review of Descartes's
Rules for the Direction of the Mind —
Quoted in that review —
"… we must make use of every assistance
of the intellect, the imagination, the senses,
and the memory" (Descartes, Rules, XII)
One such assistance is the calendar.
See the date of the Blasjo review, Dec. 20, 2009,
in this journal. See also Descartes.
On Monday, October 14, 2013, Jeremy Gray published
an article titled "Epistemology of Geometry" in the online
Stanford Encyclopedia of Philosophy.
Gray's article did not mention the role of finite geometry
in such epistemology.
For that role, see Finite Geometry and Physical Space
as a web page and as a Google image search.
See also my papers at Academia.edu.
Josefine Lyche’s large wall version of the twenty-four 2×2 variations
above was apparently offered for sale today in Norway —
Click image for more details and click here for a translation.
This journal on Oct. 2, the date of death for
the developer of mathematical Braille —
Clicking on the image of St. Peter's Square in that post led to…
Braille, as noted in last midnight's post, is based
on a six-dot cell. For some pure mathematics of
the six-dot cell, see
Modeling the 21-point plane
with outer automorphisms of S6
Two quotations that seem relevant —
"When Death tells a story, you really have to listen"
— Cover of The Book Thief
"This is not theology, this is mathematics."
— Steven H. Cullinane, Sept. 22, 2013
"Righty tighty, lefty loosey." — Folk saying
See also a figure from this journal
on Lee Marvin's birthday in 2011 —
The square root of the former is the latter.
From an academic's website:
For Josefine Lyche and Ignotus the Mage,
as well as Rose the Hat and other Zingari shoolerim —
Sabbatha hanti, lodsam hanti, cahanna risone hanti :
words that had been old when the True Knot moved
across Europe in wagons, selling peat turves and trinkets.
They had probably been old when Babylon was young.
The girl was powerful, but the True was all-powerful,
and Rose anticipated no real problem.
— King, Stephen (2013-09-24).
Doctor Sleep: A Novel
(pp. 278-279). Scribner. Kindle Edition.
From a post of November 10, 2008:

Twenty-four Variations on a Theme of Plato,
a version by Barry Sharples based on the earlier
kaleidoscope puzzle version of Steven H. Cullinane
"The king asked, in compensation for his toils
during this strangest of all the nights he had
ever known, that the twenty-four riddle tales
told him by the specter, together with the story
of the night itself, should be made known
over the whole earth and remain eternally
famous among men."
Frame Tale:
"The quad gospellers may own the targum
but any of the Zingari shoolerim may pick a peck
of kindlings yet from the sack of auld hensyne."
(Continued from High White Noon,
Finishing Up at Noon, and A New York Jew.)

Above: Frank Langella in "Starting Out in the Evening"
Below: Frank Langella and Johnny Depp in "The Ninth Gate"

"Not by the hair on your chinny-chin-chin."
Above: Detail from a Wikipedia photo.
For the logo, see Lostpedia.
For some backstory, see Noether.
Those seeking an escape from the eightfold nightmare
represented by the Dharma logo above may consult
the remarks of Heisenberg (the real one, not the
Breaking Bad version) to the Bavarian Academy
of Fine Arts.
Those who prefer Plato's cave to his geometry are
free to continue their Morphean adventures.
Today, beginning at about 11 AM ET, I checked out
the latest news from Oslo artist Josefine Lyche,
often mentioned in these posts.
Lyche's Facebook page has a new cover photo—
geometric diagrams from Order in Space , a 1969
book by Keith Critchlow.
A search for more information on Critchlow yielded
information on his friend the impressive Kathleen Raine,
who reportedly died at 95 on July 6, 2003.
See also references to that date in this journal.
From Raine's obituary in The Guardian :
"When asked how she wished people
to remember her, Kathleen Raine said
she would rather they didn't. Or that
Blake's words be said of her: 'That in
time of trouble, I kept the divine vision.' "
"… a list of group theoretic invariants
and their geometric incarnation…"
— David Lehavi on the Kummer 166 configuration in 2007
Related material —
"The hint half guessed, the gift half understood, is Incarnation."
— T. S. Eliot in Four Quartets
"This is not theology; this is mathematics."
— Steven H. Cullinane on four quartets
To wit:
The Kummer 166 configuration is the configuration of sixteen
6-sets within a 4×4 square array of points in which each 6-set
is determined by one of the 16 points of the array and
consists of the 3 other points in that point's row and the
3 other points in that point's column.
See Configurations and Squares.
The Wikipedia article Kummer surface uses a rather poetic
phrase* to describe the relationship of the 166 to a number
of other mathematical concepts — "geometric incarnation."
Related material from finitegeometry.org —
* Apparently from David Lehavi on March 18, 2007, at Citizendium .
Mathematics:
A review of posts from earlier this month —
Wednesday, September 4, 2013
|
Narrative:

Aooo.
Happy birthday to Stephen King.
"Take a cube, and write the numbers 1,…,6 on its faces.
Now the pairs of numbers on opposite faces
form a syntheme. (Standard dice, for example, represent
the syntheme 12|34|56.) "
— Peter J. Cameron, weblog post of May 11, 2010
"For every kind of vampire, there is a kind of cross."
— Gravity's Rainbow
"A vast space that travels down to the bedrock
upon which the towers were built, the museum
winds its way deeper and deeper underground,
taking visitors on a journey to the very bottom."
— The Associated Press in
this evening's Washington Post
This suggests a review of a different sort of
bedrock:—

"If you have built castles in the air,
your work need not be lost;
that is where they should be.
Now put the foundations under them.”
— Henry David Thoreau
|
The Philosopher's Gaze , by David Michael Levin, The post-metaphysical question—question for a post-metaphysical phenomenology—is therefore: Can the perceptual field, the ground of perception, be released from our historical compulsion to represent it in a way that accommodates our will to power and its need to totalize and reify the presencing of being? In other words: Can the ground be experienced as ground? Can its hermeneutical way of presencing, i.e., as a dynamic interplay of concealment and unconcealment, be given appropriate respect in the receptivity of a perception that lets itself be appropriated by the ground and accordingly lets the phenomenon of the ground be what and how it is? Can the coming-to-pass of the ontological difference that is constitutive of all the local figure-ground differences taking place in our perceptual field be made visible hermeneutically, and thus without violence to its withdrawal into concealment? But the question concerning the constellation of figure and ground cannot be separated from the question concerning the structure of subject and object. Hence the possibility of a movement beyond metaphysics must also think the historical possibility of breaking out of this structure into the spacing of the ontological difference: différance , the primordial, sensuous, ekstatic écart . As Heidegger states it in his Parmenides lectures, it is a question of "the way historical man belongs within the bestowal of being (Zufügung des Seins ), i.e., the way this order entitles him to acknowledge being and to be the only being among all beings to see the open" (PE* 150, PG** 223. Italics added). We might also say that it is a question of our response-ability, our capacity as beings gifted with vision, to measure up to the responsibility for perceptual responsiveness laid down for us in the "primordial de-cision" (Entscheid ) of the ontological difference (ibid.). To recognize the operation of the ontological difference taking place in the figure-ground difference of the perceptual Gestalt is to recognize the ontological difference as the primordial Riß , the primordial Ur-teil underlying all our perceptual syntheses and judgments—and recognize, moreover, that this rift, this division, decision, and scission, an ekstatic écart underlying and gathering all our so-called acts of perception, is also the only "norm" (ἀρχή ) by which our condition, our essential deciding and becoming as the ones who are gifted with sight, can ultimately be judged. * PE: Parmenides of Heidegger in English— Bloomington: Indiana University Press, 1992 ** PG: Parmenides of Heidegger in German— Gesamtausgabe , vol. 54— Frankfurt am Main: Vittorio Klostermann, 1992 |
Examples of "the primordial Riß " as ἀρχή —

For an explanation in terms of mathematics rather than philosophy,
see the diamond theorem. For more on the Riß as ἀρχή , see
Function Decomposition Over a Finite Field.
For Fans of Bad Movies*
This post was suggested by my viewing last night
the 1995 horror film Species , and by news that
Scarlett Johansson will be starring in a similar
production at the Venice Film Festival, which
opens tomorrow.
The new Johansson film, Under the Skin ,
is based on a novel by one Michel Faber.
Faber on books that have influenced him—
"Most influential has possibly been John Berger's Ways of Seeing —
not a novel at all (although Berger has written fiction) but a book of
art criticism. The influence of these wonderfully perceptive and
thought-provoking essays peeps out everywhere in my own work."
An excerpt from the Berger book—
Click image for a better view of the original.
Related material: Johansson in this journal, Sunday's NY Times
teaser for a piece on Saturday Night Live, and a more serious
approach to the geometry of perspective.
* And of Ben Kingsley, who starred both in Species and in
a previous film by the director of Under the Skin .
A useful article on finite geometry,
"21 – 6 = 15: A Connection between Two Distinguished Geometries,"
by Albrecht Beutelspacher, American Mathematical Monthly ,
Vol. 93, No. 1, January 1986, pp. 29-41, is available for purchase
at JSTOR.
This article is related to the geometry of the six-set.
For some background, see remarks from 1986 at finitegeometry.org.
In memory of author Elmore Leonard—
A graphic symbol and a search for "Nowhere"*
in this journal yield…
|
Pictorial version |
"Cotton Mather died
— Wallace Stevens, |
* See previous post.
Last midnight's post quoted poet John Hollander
on Cervantes—
"… the Don’s view of the world is correct at midnight,
and Sancho’s at noon."
The post concluded with a figure that might, if
rotated slightly, be regarded as a sort of Star of
David or Solomon's Seal. The figure's six vertices
may be viewed as an illustration of Pascal's
"mystic hexagram."

Pacal's hexagram is usually described
as a hexagon inscribed in a conic
(such as a circle). Clearly the hexagon
above may be so inscribed.
The figure suggests that last midnight's Don be
played by the nineteenth-century mathematician
James Joseph Sylvester. His 1854 remarks on
the nature of geometry describe a different approach
to the Pascal hexagram—
| "… the celebrated theorem of Pascal known under the name of the Mystic Hexagram, which is, that if you take two straight lines in a plane, and draw at random other straight lines traversing in a zigzag fashion between them, from A in the first to B in the second, from B in the second to C in the first, from C in the first to D in the second, from D in the second to E in the first, from E in the first to F in the second and finally from F in the second back again to A the starting point in the first, so as to obtain ABCDEF a twisted hexagon, or sort of cat's-cradle figure and if you arrange the six lines so drawn symmetrically in three couples: viz. the 1st and 4th in one couple, the 2nd and 5th in a second couple, the 3rd and 6th in a third couple; then (no matter how the points ACE have been selected upon one of the given lines, and BDF upon the other) the three points through which these three couples of lines respectively pass, or to which they converge (as the case may be) will lie all in one and the same straight line." |
For a Sancho view of Sylvester's "cat's cradle," see some twentieth-century
remarks on "the most important configuration of all geometry"—
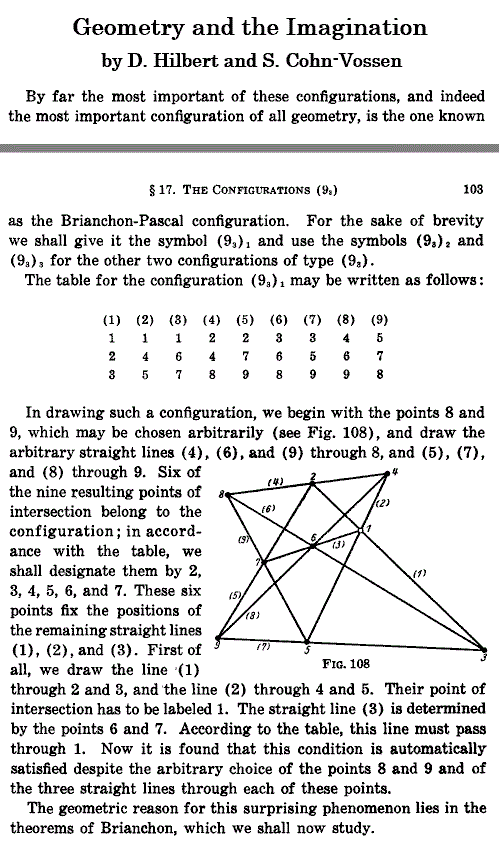
"Now look, your grace," said Sancho,
"what you see over there aren't giants,
but windmills, and what seems to be arms
are just their sails, that go around in the wind
and turn the millstone."
"Obviously," replied Don Quijote,
"you don't know much about adventures.”
From a 2003 interview by Paul Devlin (PD) with poet John Hollander (JH),
who reportedly died Saturday—
|
PD: You wrote in the introduction to the new edition of Reflections on Espionage that whenever you have been "free of political callowness" it was partly as a result of reading W.H. Auden, George Orwell, and George Bernard Shaw. Do you think these writers might possibly be an antidote to political callowness that exists in much contemporary literary criticism? JH: If not they, then some other writers who can help one develop within one a skepticism strongly intertwined with passion, so that each can simultaneously check and reinforce the other. It provides great protection from being overcome by blind, true-believing zeal and corrupting cynicism (which may be two sides of the same false coin). Shaw was a great teacher for many in my generation. I started reading him when I was in sixth grade, and I responded strongly not only to the wit but to various modes, scene and occasions of argument and debate as they were framed by various kinds of dramatic situation. I remember being electrified when quite young by the moment in the epilogue scene of Saint Joan when the English chaplain, De Stogumber, who had been so zealous in urging for Joan’s being burned at the stake, returns to testify about how seeing her suffering the flames had made a changed man of him. The Inquisitor, Peter Cauchon, calls out (with what I imagined was a kind of moral distaste I’d never been aware of before), "Must then a Christ perish in torment in every age to save those who have no imagination?" It introduced me to a skepticism about the self-satisfaction of the born-again, of any persuasion. With Auden and Orwell, much later on and after my mental world had become more complicated, it was education in negotiating a living way between a destructively naïve idealism and the crackpot realism—equally inimical to the pragmatic. PD: Would you consider yourself a "formal" pragmatist, i.e., a student of Peirce, James, Dewey, Mead (etc.) or an "informal" pragmatist – someone taking the common-sense position on events…or someone who refuses to be pigeon-holed politically? JH: "Informal" – of the sort that often leads me to ask of theoretical formulations, "Yes, but what’s it for ?" PD: Which other authors do you think might help us negotiate between "naïve idealism" and "crackpot realism"? I think of Joyce, Wallace Stevens, perhaps Faulkner? JH: When I was in college, a strong teacher for just this question was Cervantes. One feels, in an Emersonian way, that the Don’s view of the world is correct at midnight, and Sancho’s at noon. |
Then there is mathematical realism.
A post in this journal on Saturday, the reported date of Hollander's death,
discussed a possible 21st-century application of 19th-century geometry.
For some background, see Peter J. Cameron's May 11, 2010, remarks
on Sylvester's duads and synthemes . The following figure from the
paper discussed here Saturday is related to figures in Cameron's remarks.

The following excerpt from a January 20, 2013, preprint shows that
a Galois-geometry version of the large Desargues 154203 configuration,
although based on the nineteenth-century work of Galois* and of Fano,**
may at times have twenty-first-century applications.

Atkinson's paper does not use the square model of PG(3,2), which later
in 2013 provided a natural view of the large Desargues 154203 configuration.
See my own Classical Geometry in Light of Galois Geometry. Atkinson's
"subset of 20 lines" corresponds to 20 of the 80 Rosenhain tetrads
mentioned in that later article and pictured within 4×4 squares in Hudson's
1905 classic Kummer's Quartic Surface.
* E. Galois, definition of finite fields in "Sur la Théorie des Nombres,"
Bulletin des Sciences Mathématiques de M. Férussac,
Vol. 13, 1830, pp. 428-435.
** G. Fano, definition of PG(3,2) in "Sui Postulati Fondamentali…,"
Giornale di Matematiche, Vol. 30, 1892, pp. 106-132.
From April 23, 2013, in
"Classical Geometry in Light of Galois Geometry"—
Click above image for some background from 1986.
Related material on six-set geometry from the classical literature—
Baker, H. F., "Note II: On the Hexagrammum Mysticum of Pascal,"
in Principles of Geometry , Vol. II, Camb. U. Press, 1930, pp. 219-236
Richmond, H. W., "The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen (1900), Volume 53, Issue 1-2, pp 161-176
Richmond, H. W., "On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics , Vol. 31 (1900), pp. 125-160
(Continued from 24 hours ago and from May 9, 2012)
Quoted 24 hours ago in this journal—
Remark by Aldous Huxley on an artist's work:
"All the turmoil, all the emotions of the scenes
have been digested by the mind into a
grave intellectual whole."
Quoted in a video uploaded on May 9, 2012:
Norway Toilet Scene

Norway dance (as interpreted by an American)

I prefer a different, Norwegian, interpretation of "the dance of four."
Related material: The clash between square and tetrahedral versions of PG(3,2).
(Continued from this morning)

The above stylized "N," based on
an 8-cycle in the 9-element Galois field
GF(9), may also be read as an Aleph.
Graphic designers may prefer a simpler,
bolder version:

The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977—
The Galois tesseract is the basis for a representation of the smallest
projective 3-space, PG(3,2), that differs from the representation at
Wolfram Demonstrations Project. For the latter, see yesterday’s post.
The tesseract representation underlies the diamond theorem, illustrated
below in its earliest form, also from the above February 1977 article—
As noted in a more recent version, the group described by
the diamond theorem is also the group of the 35 square
patterns within the 1976 Miracle Octad Generator (MOG) of
R. T. Curtis.
The following image gives a brief description
of the geometry discussed in last spring's
Classical Geometry in Light of Galois Geometry.

Update of Aug. 7, 2013: See also an expanded PDF version.
I added links today in the following Wikipedia articles:
The links will probably soon be deleted,
but it seemed worth a try.
Today is the dies natalis of group theorist Walter Feit.
"The Steiner systems S (5,6,12) and S (5,8,24) are remarkable combinatorial
configurations unlike any others. Their automorphism groups are the Mathieu
groups M12 and M24. These are the only 5-transitive permutation groups other
than symmetric and alternating groups: (a fact long conjectured but only
proved as a consequence of the classification). The Leech lattice is a blown up
version of S (5,8,24). It is the unique even unimodular lattice in 24 dimensions
with no vectors of weight 2. This uniqueness is an essential reason why it is a
geometric object of fundamental importance. The automorphism group Co.O
of the Leech lattice involves about half of the sporadic groups and generally it
is felt that these are well understood."
— Walter Feit, book review, Bulletin of the American Mathematical Society ,
Vol. 8 (1983), 120-124, page 123
(Simplicity continued)
"Understanding a metaphor is like understanding a geometrical
truth. Features of various geometrical figures or of various contexts
are pulled into revealing alignment with one another by the
demonstration or the metaphor.
What is 'revealed' is not that the alignment is possible; rather,
that the alignment is possible reveals the presence of already-
existing shapes or correspondences that lay unnoticed. To 'see' a
proof or 'get' a metaphor is to experience the significance of the
correspondence for what the thing, concept, or figure is ."
— Jan Zwicky, Wisdom & Metaphor , page 36 (left)
Zwicky illustrates this with Plato's diamond figure
from the Meno on the facing page— her page 36 (right).
A more sophisticated geometrical figure—
Galois-geometry key to
Desargues' theorem:
| D | E | F | |
| S' | P | Q | R |
| S | P' | Q' | R' |
| O | P1 | Q1 | R1 |
For an explanation, see
Classical Geometry in Light of Galois Geometry.
This post was suggested by a search for the
Derridean phrase "necessary possibility"* that
led to web pages on a conference at Harvard
on Friday and Saturday, March 26**-27, 2010,
on Derrida and Religion .
The conference featured a talk titled
"The Poetics of the Broken Tablet."

I prefer the poetics of projective geometry.
An illustration— The restoration of the full
15-point "large" Desargues configuration in
place of the diminished 10-point Desargues
configuration that is usually discussed.
Click on the image for further details.
* See a discussion of this phrase in
the context of Brazilian religion.
** See also my own philosophical reflections
on Friday, March 26, 2010:
"You Can't Make This Stuff Up."
This is the weekend for Comic-Con International in San Diego.
The convention includes an art show. (Click above image to enlarge.)
Related material from Norway…
Suggested nominations for a Kavli Prize:
1. Josefine Lyche's highly imaginative catalog page for
the current Norwegian art exhibition I de lange nætter,
which mentions her interest in sacred geometry
2. Sacred Geometry: Drawing a Metatron Cube
… and from San Diego—
The Kavli Institutes logo:

The title refers to a classic 1960 novel by John Hersey.
“How do you get young people excited about space?”
— Megan Garber in The Atlantic , Aug. 16, 2012
(Italics added.) (See previous four posts.)
Allyn Jackson on “Simplicity, in Mathematics and in Art,”
in the new August 2013 issue of Notices of the American
Mathematical Society—
“As conventions evolve, so do notions of simplicity.
Franks mentioned Gauss’s 1831 paper that
established the respectability of complex numbers.”
This suggests a related image by Gauss, with a
remark on simplicity—
Here Gauss’s diagram is not, as may appear at first glance,
a 3×3 array of squares, but is rather a 4×4 array of discrete
points (part of an infinite plane array).
Related material that does feature the somewhat simpler 3×3 array
of squares, not seen as part of an infinite array—
Marketing the Holy Field
Click image for the original post.
For a purely mathematical view of the holy field, see Visualizing GL(2,p).
"How do you get young people excited
about space? How do you get them interested
not just in watching movies about space,
or in playing video games set in space …
but in space itself?"
— Megan Garber in The Atlantic , Aug. 16, 2012
One approach:
"There is such a thing as a tesseract" and
Diamond Theory in 1937.
See, too, Baez in this journal.
A sort of poem
by Gauss and Weyl —
Click the circle for the context in Weyl's Symmetry .
For related remarks, see the previous post.
A literary excursus—
|
Brad Leithauser in a New Yorker post of July 11, 2013: If a poet determines that a poem should begin at point A and conclude at point D, say, the mystery of how to get there—how to pass felicitously through points B and C—strikes me as an artistic task both genuine and enlivening. There are fertile mysteries of transition, no less than of termination. And I’d like to suppose that Frost himself would recognize that any ingress into a poem is better than being locked out entirely. His little two-liner, “The Secret,” suggests as much: “We dance round in a ring and suppose / But the Secret sits in the middle and knows.” Most truly good poems might be said to contain a secret: the little sacramental miracle by which you connect, intimately, with the words of a total stranger. And whether you come at the poem frontward, or backward, or inside out—whether you approach it deliberately, word by word and line by line, or you parachute into it borne on a sudden breeze from the island of Serendip—surely isn’t the important thing. What matters is whether you achieve entrance into its inner ring, and there repose companionably beside the Secret. |
One should try, of course, to avoid repose in an inner circle of Hell .
Profile picture of "Jo Lyxe" (Josefine Lyche) at Vimeo—

Compare to an image of Vril muse Maria Orsitsch.
From the catalog of a current art exhibition
(25 May – 31 August, 2013) in Norway,
I DE LANGE NÆTTER —
|
Josefine Lyche
Keywords (to help place my artwork in the (See also the original catalog page.) |
Clearly most of this (the non-highlighted parts) was taken
from my webpage Diamond Theory. I suppose I should be
flattered, but I am not thrilled to be associated with the
(apparently fictional) Vril Society.
For some background, see (for instance)
Conspiracy Theories and Secret Societies for Dummies .
From Andries Brouwer —
* Related material: Yesterday's evening post and The People's Cube.
(By the way, any 4×4 array is a tesseract .)
Short Story — (Click image for some details.)
Parts of a longer story —
"Classical Geometry in Light of Galois Geometry"
is now available at independent.academia.edu.
Related commentary: Yesterday's post Vision
and a post of February 21, 2013: Galois Space.
My diamond theorem articles at PlanetMath and at
Encyclopedia of Mathematics have been updated
to clarify the relationship between the graphic square
patterns of the diamond theorem and the schematic
square patterns of the Curtis Miracle Octad Generator.
|
"What on earth is
— Said to be an annotation |
In the spirit of the late Thomas Guinzburg…
See also "Concrete Universal" in this journal.
Related material— From a Bloomsday reply
to a Diamond Theory reader's comment, an excerpt—
The reader's comment suggests the following passages from
the book by Stirling quoted above—
Here Stirling plays a role analogous to that of Professor Irwin Corey
accepting the National Book Award for Gravity's Rainbow in 1974.
“I could a tale unfold whose lightest word
Would harrow up thy soul….“
— Hamlet’s Father’s Ghost
The results of a search in this journal for “a tale unfold” suggest
a review of the following passage from Donna Tartt’s Secret History…
A math weblog discussed this passage on January 24, 2013.
For related alliances, see this weblog on that same date.
Online biography of author Cormac McCarthy—
"… he left America on the liner Sylvania, intending to visit
the home of his Irish ancestors (a King Cormac McCarthy
built Blarney Castle)."
Two Years Ago:
Blarney in The Harvard Crimson—
Melissa C. Wong, illustration for "Atlas to the Text,"
by Nicholas T. Rinehart:

Thirty Years Ago:
Non-Blarney from a rural outpost—
Illustration for the generalized diamond theorem,
by Steven H. Cullinane:
“For in that sleep of death what dreams may come
When we have shuffled off this mortal coil,
Must give us pause.” — Hamlet
Sleep well, Mr. Matheson.
The New York Times philosophy column "The Stone"
last evening had an essay on art by a sarcastic anarchist,
one Crispin Sartwell—
"… whole generations of art lovers have been
trained in modernist dogma, and arts institutions’
access to various forms of state or foundation
support depend on it completely. One goes to
the museum to gasp at stunning works of
incomparable, super-human genius by beings
who are infinitely more exalted and important
than the mere humans staring at their paintings.
That’s why ordinary people staring at a Picasso
(allegedly) experience a kind of transcendence
or re-articulation of their lives and world."
Cubism Re-Articulated:
Click image for some backstory.
(IMAGE: Walter Gropius and Froebel's Third Gift,
from a Google image search today)
Background: Cubism in this journal and
Pilate Goes to Kindergarten.
Related material: Chesterton + Thursday in this journal.
"Da hats ein Eck" —
"you've/she's (etc.) got problems there"
St. Galluskirche:
St. Gallus's Day, 2012:
Click image for a St. Gallus's Day post.
A related problem:
Discuss the structure of the 4x4x4 "magic" cube
sent by Pierre de Fermat to Father Marin Mersenne
on April 1, 1640, in light of the above post.
This journal on May 14, 2013:
"And let us finally, then, observe the
parallel progress of the formations of thought
across the species of psychical onomatopoeia
of the primitives, and elementary symmetries
and contrasts, to the ideas of substances,
to metaphors, the faltering beginnings of logic,
formalisms, entities, metaphysical existences."
— Paul Valéry, Introduction to the Method of
Leonardo da Vinci
But first, a word from our sponsor…
For those who prefer Trudeau's
"Story Theory" of truth to his "Diamond Theory"
Related material: Click images below for the original posts.
See as well the novel "Lexicon" at Amazon.com
and the word "lexicon" in this journal.
From a weblog post on June 11, 2013, by one Pete Trbovich:
|
Here again, I don't think Steven Cullinane is really unhinged per se. At the very least, his geometric study is fun to play with, particularly when you find this toy. And I'm not really sure that anything he says is wrong per se. But you might find yourself asking "So what?" or more to the point, "Why is this supposed to be the central theory to explaining life, the universe, and everything?" |
It isn't supposed to be such a theory.
I do not know why Trbovich thinks it is .
— Steven H. Cullinane
Update of 11 PM June 16:
For one such central theory of everything, see
the I Ching . Diamond theory is, unlike that
Chinese classic, pure mathematics, but the larger
of the binary-coordinate structures it is based on
are clearly isomorphic, simply as structures , to
the I Ching 's 64 hexagrams.
Make of this what you will.
This journal on July 5, 2007 —
“It is not clear why MySpace China will be successful."
— The Chinese magazine Caijing in 2007, quoted in
Asia Sentinel on July 12, 2011
This journal on that same date, July 12, 2011 —
See also the eightfold cube and kindergarten blocks
at finitegeometry.org/sc.
Friedrich Froebel, Froebel's Chief Writings on Education ,
Part II, "The Kindergarten," Ch. III, "The Third Play":
"The little ones, who always long for novelty and change,
love this simple plaything in its unvarying form and in its
constant number, even as they love their fairy tales with
the ever-recurring dwarfs…."
This journal, Group Actions, Nov. 14, 2012:
"Those who insist on vulgarizing their mathematics
may regard linear and affine group actions on the eight
cubes as the dance of Snow White (representing (0,0,0))
and the Seven Dwarfs—
"Eight is a Gate." — Mnemonic rhyme
Today's previous post, Window, showed a version
of the Chinese character for "field"—
This suggests a related image—
The related image in turn suggests…
Unlike linear perspective, axonometry has no vanishing point,
and hence it does not assume a fixed position by the viewer.
This makes axonometry 'scrollable'. Art historians often speak of
the 'moving' or 'shifting' perspective in Chinese paintings.
Axonometry was introduced to Europe in the 17th century by
Jesuits returning from China.
As was the I Ching. A related structure:
Today's previous post on coordinate systems
suggests a look at the phrase "Galois coordinates."
A search shows that the phrase, though natural,
has apparently not been used before 2011* for solutions
to what Hermann Weyl called "the relativity problem."
A thorough historical essay on Galois coordinatization
in this sense would require more academic resources
than I have available. It would likely describe a number
of applications of Galois-field coordinates to square
(and perhaps to cubical) arrays that were studied before
1976, the date of my Diamond Theory monograph.
But such a survey might not find any such pre-1976
coordinatization of a 4×4 array by the 16 elements
of the vector 4-space over the Galois field with two
elements, GF(2).
Such coordinatizations are important because of their
close relationship to the Mathieu group M 24 .
See a preprint by Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ," with its remark
denying knowledge of any such coordinatization
prior to a 1989 paper by R. T. Curtis.
Related material:
Some images related to Galois coordinates, excerpted
from a Google search today (click to enlarge)—
* A rather abstract 2011 paper that uses the phrase
"Galois coordinates" may have some implications
for the naive form of the relativity problem
related to square and cubical arrays.
(Continued from Sept. 22, 2011)
See Taormina in this journal, and the following photo of "Anne Newton"—
Click photo for context.
Related material:
"Super Overarching" in this journal,
a group of order 322,560, and…

See also the MAA Spectrum program —
— and an excerpt from the above book:
The Mathematical Association of America has a
submit-a-review form that apparently allows readers
to comment on previously reviewed books.
This morning I submitted the following comment on
Alexander Bogomolny's March 16, 2012, review of
Martin J. Erickson's Beautiful Mathematics :
In section 5.17, pages 106-108, "A Group of Operations,"
Erickson does not acknowledge any source. This section
is based on the Cullinane diamond theorem. See that
theorem (published in an AMS abstract in 1979) at
PlanetMath.org and EncyclopediaOfMath.org, and
elsewhere on the Web. Details of the proof given by
Erickson may be found in "Binary Coordinate Systems,"
a 1984 article on the Web at
http://finitegeometry.org/sc/gen/coord.html.
If and when the comment may be published, I do not know.
Update of about 6:45 PM ET June 7:
The above comment is now online at the MAA review site.
Update of about 7 PM ET July 29:
The MAA review site's web address was changed, and the
above comment was omitted from the page at the new address.
This has now been corrected.
The Daily Princetonian today:
A different cover act, discussed here Saturday:
See also, in this journal, the Galois tesseract and the Crosswicks Curse.
"There is such a thing as a tesseract." — Crosswicks saying
See the Klein correspondence at SymOmega today and in this journal.
"The casual passerby may wonder about the name SymOmega.
This comes from the notation Sym(Ω) referring to the symmetric group
of all permutations of a set Ω, which is something all of us have
both written and read many times over."
"What we do may be small, but it has
a certain character of permanence."
— G. H. Hardy, A Mathematician's Apology
The diamond theorem group, published without acknowledgment
of its source by the Mathematical Association of America in 2011—
The hypercube model of the 4-space over the 2-element Galois field GF(2):

The phrase Galois tesseract may be used to denote a different model
of the above 4-space: the 4×4 square.
MacWilliams and Sloane discussed the Miracle Octad Generator
(MOG) of R. T. Curtis further on in their book (see below), but did not
seem to realize in 1977 that the 4×4 structures within the MOG are
based on the Galois-tesseract model of the 4-space over GF(2).

The thirty-five 4×4 structures within the MOG:

Curtis himself first described these 35 square MOG patterns
combinatorially, (as his title indicated) rather than
algebraically or geometrically:

A later book co-authored by Sloane, first published in 1988,
did recognize the 4×4 MOG patterns as based on the 4×4
Galois-tesseract model.
Between the 1977 and 1988 Sloane books came the diamond theorem.
Update of May 29, 2013:
The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977
(the year the above MacWilliams-Sloane book was first published):
Best vs. Bester
The previous post ended with a reference mentioning Rosenhain.
For a recent application of Rosenhain's work, see
Desargues via Rosenhain (April 1, 2013).
From the next day, April 2, 2013:
"The proof of Desargues' theorem of projective geometry
comes as close as a proof can to the Zen ideal.
It can be summarized in two words: 'I see!' "
– Gian-Carlo Rota in Indiscrete Thoughts (1997)
Also in that book, originally from a review in Advances in Mathematics ,
Vol. 84, Number 1, Nov. 1990, p. 136:

See, too, in the Conway-Sloane book, the Galois tesseract …
and, in this journal, Geometry for Jews and The Deceivers , by Bester.
From an arXiv preprint submitted July 18, 2011,
and last revised on March 11, 2013 (version 4):
"By our construction, this vector space is the dual
of our hypercube F24 built on I \ O9. The vector space
structure of the latter, to our knowledge, is first
mentioned by Curtis in [Cur89]. Hence altogether
our proposition 2.3.4 gives a novel geometric
meaning in terms of Kummer geometry to the known
vector space structure on I \ O9."
[Cur89] reference:
R. T. Curtis, "Further elementary techniques using
the miracle octad generator," Proc. Edinburgh
Math. Soc. 32 (1989), 345-353 (received on
July 20, 1987).
— Anne Taormina and Katrin Wendland,
"The overarching finite symmetry group of Kummer
surfaces in the Mathieu group M 24 ,"
arXiv.org > hep-th > arXiv:1107.3834
"First mentioned by Curtis…."
No. I claim that to the best of my knowledge, the
vector space structure was first mentioned by me,
Steven H. Cullinane, in an AMS abstract submitted
in October 1978, some nine years before the
Curtis article.
|
Update of the above paragraph on July 6, 2013—
No. The vector space structure was described by
The vector space structure as it occurs in a 4×4 array |
See Notes on Finite Geometry for some background.
See in particular The Galois Tesseract.
For the relationship of the 1978 abstract to Kummer
geometry, see Rosenhain and Göpel Tetrads in PG(3,2).
See Coxeter + Aleph in this journal.
Epigraph to "The Aleph," a 1945 story by Borges:
"O God! I could be bounded in a nutshell,
and count myself a King of infinite space…"
– Hamlet, II, 2

Detail from the video in the previous post:

For other permutations of points in the
order-3 affine plane—
See Quaternions in an Affine Galois Plane
and Group Actions, 1984-2009.
See, too, the Mathematics and Narrative post
from April 28, 2013, and last night's
For Indiana Spielberg.
(Continued from December 30, 2012)
"And let us finally, then, observe the
parallel progress of the formations of thought
across the species of psychical onomatopoeia
of the primitives, and elementary symmetries
and contrasts, to the ideas of substances,
to metaphors, the faltering beginnings of logic,
formalisms, entities, metaphysical existences."
— Paul Valéry, Introduction to the Method of
Leonardo da Vinci
But first, a word from our sponsor…
Brought to you by two uploads, each from Sept. 11, 2012—
Symmetry and Hierarchy and the above VINCI Genius commercial.
Western Washington University in Bellingham maintains a
website to benefit secondary-school math: MathNEXUS.
The MathNEXUS "website of the week" on April 14, 2013,
was the Diamond 16 Puzzle and its related webpages.
Click on the above image for the April 14 webpage.
Promotional description of a new book:
"Like Gödel, Escher, Bach before it, Surfaces and Essences will profoundly enrich our understanding of our own minds. By plunging the reader into an extraordinary variety of colorful situations involving language, thought, and memory, by revealing bit by bit the constantly churning cognitive mechanisms normally completely hidden from view, and by discovering in them one central, invariant core— the incessant, unconscious quest for strong analogical links to past experiences— this book puts forth a radical and deeply surprising new vision of the act of thinking."
"Like Gödel, Escher, Bach before it…."
Or like Metamagical Themas .
Rubik core:
Non- Rubik cores:
|
Of the odd nxnxn cube:
|
Of the even nxnxn cube:
|
Related material: The Eightfold Cube and…
"A core component in the construction
is a 3-dimensional vector space V over F2 ."
— Page 29 of "A twist in the M24 moonshine story,"
by Anne Taormina and Katrin Wendland.
(Submitted to the arXiv on 13 Mar 2013.)
A page with the above title has been created at
the Encyclopedia of Mathematics.
How long it will stay there remains to be seen.
Powered by WordPress