Between Two Worlds
From the world of mathematics…
“… my advisor once told me, ‘If you ever find yourself drawing one of those meaningless diagrams with arrows connecting different areas of mathematics, it’s a good sign that you’re going senile.'”
— Scott Carnahan at Secret Blogging Seminar, December 14, 2007
Carnahan’s remark in context:
“About five years ago, Cheewhye Chin gave a great year-long seminar on Langlands correspondence for GLr over function fields…. In the beginning, he drew a diagram….
If we remove all of the explanatory text, the diagram looks like this:
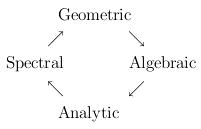
I was a bit hesitant to draw this, because my advisor once told me, ‘If you ever find yourself drawing one of those meaningless diagrams with arrows connecting different areas of mathematics, it’s a good sign that you’re going senile.’ Anyway, I’ll explain roughly how it works.
Langlands correspondence is a ‘bridge between two worlds,’ or more specifically, an assertion of a bijection….”
Compare and contrast the above…
… to the world of Rudolf Kaehr:

The above reference to “diamond theory” is from Rudolf Kaehr‘s paper titled Double Cross Playing Diamonds.
Another bridge…
Carnahan’s advisor, referring to “meaningless diagrams with arrows connecting different areas of mathematics,” probably did not have in mind diagrams like the two above, but rather diagrams like the two below–
From the world of mathematics…

“A rough sketch of
how diamond theory is
related to some other
fields of mathematics”
— Steven H. Cullinane

Related material:
For further details on
the “diamond theory” of
Cullinane, see
Finite Geometry of the
Square and Cube.
For further details on
the “diamond theory” of
Kaehr, see
Those who prefer entertainment
may enjoy an excerpt
from Log24, October 2007:
|
“Do not let me hear Anthony Hopkins Anthony Hopkins |
