In memory of David Rumelhart, who "explored the possibility of formulating a formal grammar to capture the structure of stories." (Wikipedia)
Rumelhart died on Sunday, March 13, 2011.*
I've got a little schema you oughta know….
So make it one for my baby and one more for the road.
* From that date: The Counter and Twenty-Four.
The On-Line Encyclopedia of Integer Sequences has an article titled "Number of combinatorial configurations of type (n_3)," by N.J.A. Sloane and D. Glynn.
From that article:
The following corrects the word "unique" in the example.
* This post corrects an earlier post, also numbered 14660 and dated 7 PM March 18, 2011, that was in error.
The correction was made at about 11:50 AM on March 20, 2011.
_____________________________________________________________
Update of March 21
The problem here is of course with the definition. Sloane and Glynn failed to include in their definition a condition that is common in other definitions of configurations, even abstract or purely "combinatorial" configurations. See, for instance, Configurations of Points and Lines , by Branko Grunbaum (American Mathematical Society, 2009), p. 17—
In the most general sense we shall consider combinatorial (or abstract) configurations; we shall use the term set-configurations as well. In this setting "points" are interpreted as any symbols (usually letters or integers), and "lines" are families of such symbols; "incidence" means that a "point" is an element of a "line". It follows that combinatorial configurations are special kinds of general incidence structures. Occasionally, in order to simplify and clarify the language, for "points" we shall use the term marks, and for "lines" we shall use blocks. The main property of geometric configurations that is preserved in the generalization to set-configurations (and that characterizes such configurations) is that two marks are incident with at most one block, and two blocks with at most one mark.
Whether or not omitting this "at most one" condition from the definition is aesthetically the best choice, it dramatically changes the number of configurations in the resulting theory, as the above (8_3) examples show.
Update of March 22 (itself updated on March 25)
For further background on configurations, see Dolgachev—
Note that the two examples Dolgachev mentions here, with 16 points and 9 points, are not unrelated to the geometry of 4×4 and 3×3 square arrays. For the Kummer and related 16-point configurations, see section 10.3, "The Three Biplanes of Order 4," in Burkard Polster's A Geometrical Picture Book (Springer, 1998). See also the 4×4 array described by Gordon Royle in an undated web page and in 1980 by Assmus and Sardi. For the Hesse configuration, see (for instance) the passage from Coxeter quoted in Quaternions in an Affine Galois Plane.
Update of March 27
See the above link to the (16,6) 4×4 array and the (16,6) exercises using this array in R.D. Carmichael's classic Introduction to the Theory of Groups of Finite Order (1937), pp. 42-43. For a connection of this sort of 4×4 geometry to the geometry of the diamond theorem, read "The 2-subsets of a 6-set are the points of a PG(3,2)" (a note from 1986) in light of R.W.H.T. Hudson's 1905 classic Kummer's Quartic Surface , pages 8-9, 16-17, 44-45, 76-77, 78-79, and 80.
(Continued from February 19)
The cover of the April 1, 1970 second edition of The Structure of Scientific Revolutions , by Thomas S. Kuhn—
This journal on January 19, 2011—
If Galois geometry is thought of as a paradigm shift from Euclidean geometry,
both images above— the Kuhn cover and the nine-point affine plane—
may be viewed, taken together, as illustrating the shift. The nine subcubes
of the Euclidean 3x3x3 cube on the Kuhn cover do not form an affine plane
in the coordinate system of the Galois cube in the second image, but they
at least suggest such a plane. Similarly, transformations of a
non-mathematical object, the 1974 Rubik cube, are not Galois transformations,
but they at least suggest such transformations.
See also today's online Harvard Crimson illustration of problems of translation—
not unrelated to the problems of commensurability discussed by Kuhn.
Part 3 of 5 (See also Part 1 and Part 2) begins as follows…
"Incommensurable. It is a strange word. I wondered, why did Kuhn choose it? What was the attraction?
Here’s one clue. At the very end of 'The Road Since Structure,' a compendium of essays on Kuhn’s work, there is an interview with three Greek philosophers of science, Aristides Baltas, Kostas Gavroglu and Vassiliki Kindi. Kuhn provides a brief account of the historical origins of his idea. Here is the relevant segment of the interview.
T. KUHN: Look, 'incommensurability' is easy.
V. KINDI: You mean in mathematics?
T. KUHN: …When I was a bright high school mathematician and beginning to learn Calculus, somebody gave me—or maybe I asked for it because I’d heard about it—there was sort of a big two-volume Calculus book by, I can’t remember whom. And then I never really read it. I read the early parts of it. And early on it gives the proof of the irrationality of the square root of 2. And I thought it was beautiful. That was terribly exciting, and I learned what incommensurability was then and there. So, it was all ready for me, I mean, it was a metaphor but it got at nicely what I was after. So, that’s where I got it.
'It was all ready for me.' I thought, 'Wow.' The language was suggestive. I imagined √2 provocatively dressed, its lips rouged. But there was an unexpected surprise. The idea didn’t come from the physical sciences or philosophy or linguistics, but from mathematics ."
A footnote from Morris (no. 29)—
"Those who are familiar with the proof [of irrationality] certainly don’t want me to explain it here; likewise, those who are unfamiliar with it don’t want me to explain it here, either. There are many simple proofs in many histories of mathematics — E.T. Bell, Sir Thomas Heath, Morris Kline, etc., etc. Barry Mazur offers a proof in his book, 'Imagining Numbers (particularly the square root of minus fifteen),' New York, NY: Farrar, Straus and Giroux. 2003, 26ff. And there are two proofs in his essay, 'How Did Theaetetus Prove His Theorem?', available on Mazur’s Harvard Web site."
There may, actually, be a few who do want the proof. They may consult the sources Morris gives, or the excellent description by G.H. Hardy in A Mathematician's Apology , or, perhaps best of all for present purposes, the proof as described in a "sort of a big two-volume Calculus book" (perhaps the one Kuhn mentioned)… See page 6 and page 7 of Volume One of Richard Courant's classic Differential and Integral Calculus (second edition, 1937, reprinted many times through 1970, and again in a Wiley Classics Library Edition in 1988).
Harvard Science Review (Winter 1997) on Thomas Kuhn's
The Structure of Scientific Revolutions —
"…his language often portrays paradigms as cults
and the battle between paradigms as quasi-religious wars."
Related material: This journal's "Paradigms" on February 17th
and the following notes—
The following is from the weblog of a high school mathematics teacher—
This is related to the structure of the figure on the cover of the 1976 monograph Diamond Theory—
Each small square pattern on the cover is a Latin square,
with elements that are geometric figures rather than letters or numerals.
All order-four Latin squares are represented.
For a deeper look at the structure of such squares, let the high-school
chart above be labeled with the letters A through X, and apply the
four-color decomposition theorem. The result is 24 structural diagrams—
Some of the squares are structurally congruent under the group of 8 symmetries of the square.
This can be seen in the following regrouping—
(Image corrected on Jan. 25, 2011– "seven" replaced "eight.")
* Retitled "The Order-4 (i.e., 4×4) Latin Squares" in the copy at finitegeometry.org/sc.
In memory of kaleidoscope enthusiast Cozy Baker, who died at 86, according to Saturday's Washington Post , on October 19th.
This journal on that date — Savage Logic and Savage Logic continued.
See this journal on All Saints' Day 2006 for some background to those posts—
“Savage logic works like a kaleidoscope whose chips can fall into a variety of patterns while remaining unchanged in quantity, form, or color. The number of patterns producible in this way may be large if the chips are numerous and varied enough, but it is not infinite. The patterns consist in the disposition of the chips vis-a-vis one another (that is, they are a function of the relationships among the chips rather than their individual properties considered separately). And their range of possible transformations is strictly determined by the construction of the kaleidoscope, the inner law which governs its operation. And so it is too with savage thought. Both anecdotal and geometric, it builds coherent structures out of ‘the odds and ends left over from psychological or historical process.’
These odds and ends, the chips of the kaleidoscope, are images drawn from myth, ritual, magic, and empirical lore. (How, precisely, they have come into being in the first place is one of the points on which Levi-Strauss is not too explicit, referring to them vaguely as the ‘residue of events… fossil remains of the history of an individual or a society.’) Such images are inevitably embodied in larger structures– in myths, ceremonies, folk taxonomies, and so on– for, as in a kaleidoscope, one always sees the chips distributed in some pattern, however ill-formed or irregular. But, as in a kaleidoscope, they are detachable from these structures and arrangeable into different ones of a similar sort. Quoting Franz Boas that ‘it would seem that mythological worlds have been built up, only to be shattered again, and that new worlds were built from the fragments,’ Levi-Strauss generalizes this permutational view of thinking to savage thought in general.”
– Clifford Geertz, “The Cerebral Savage: the Structural Anthropology of Claude Levi-Strauss,” in Encounter, Vol. 28 No. 4 (April 1967), pp. 25-32.
Related material —
See also "Levi-Strauss" in this journal and "At Play in the Field."
The Dreidel Is Cast
The Nietzschean phrase "ruling and Caesarian spirits" occurred in yesterday morning's post "Novel Ending."
That post was followed yesterday morning by a post marking, instead, a beginning— that of Hanukkah 2010. That Jewish holiday, whose name means "dedication," commemorates the (re)dedication of the Temple in Jerusalem in 165 BC.
The holiday is celebrated with, among other things, the Jewish version of a die— the dreidel . Note the similarity of the dreidel to an illustration of The Stone* on the cover of the 2001 Eerdmans edition of Charles Williams's 1931 novel Many Dimensions—
For mathematics related to the dreidel , see Ivars Peterson's column on this date fourteen years ago.
For mathematics related (if only poetically) to The Stone , see "Solomon's Cube" in this journal.
Here is the opening of Many Dimensions—
For a fanciful linkage of the dreidel 's concept of chance to The Stone 's concept of invariant law, note that the New York Lottery yesterday evening (the beginning of Hanukkah) was 840. See also the number 840 in the final post (July 20, 2002) of the "Solomon's Cube" search.
Some further holiday meditations on a beginning—
Today, on the first full day of Hanukkah, we may or may not choose to mark another beginning— that of George Frederick James Temple, who was born in London on this date in 1901. Temple, a mathematician, was President of the London Mathematical Society in 1951-1953. From his MacTutor biography—
"In 1981 (at the age of 80) he published a book on the history of mathematics. This book 100 years of mathematics (1981) took him ten years to write and deals with, in his own words:-
those branches of mathematics in which I had been personally involved.
He declared that it was his last mathematics book, and entered the Benedictine Order as a monk. He was ordained in 1983 and entered Quarr Abbey on the Isle of Wight. However he could not stop doing mathematics and when he died he left a manuscript on the foundations of mathematics. He claims:-
The purpose of this investigation is to carry out the primary part of Hilbert's programme, i.e. to establish the consistency of set theory, abstract arithmetic and propositional logic and the method used is to construct a new and fundamental theory from which these theories can be deduced."
For a brief review of Temple's last work, see the note by Martin Hyland in "Fundamental Mathematical Theories," by George Temple, Philosophical Transactions of the Royal Society, A, Vol. 354, No. 1714 (Aug. 15, 1996), pp. 1941-1967.
The following remarks by Hyland are of more general interest—
"… one might crudely distinguish between philosophical and mathematical motivation. In the first case one tries to convince with a telling conceptual story; in the second one relies more on the elegance of some emergent mathematical structure. If there is a tradition in logic it favours the former, but I have a sneaking affection for the latter. Of course the distinction is not so clear cut. Elegant mathematics will of itself tell a tale, and one with the merit of simplicity. This may carry philosophical weight. But that cannot be guaranteed: in the end one cannot escape the need to form a judgement of significance."
— J. M. E. Hyland. "Proof Theory in the Abstract." (pdf)
Annals of Pure and Applied Logic 114, 2002, 43-78.
Here Hyland appears to be discussing semantic ("philosophical," or conceptual) and syntactic ("mathematical," or structural) approaches to proof theory. Some other remarks along these lines, from the late Gian-Carlo Rota—
See also "Galois Connections" at alpheccar.org and "The Galois Connection Between Syntax and Semantics" at logicmatters.net.
* Williams's novel says the letters of The Stone are those of the Tetragrammaton— i.e., Yod, He, Vau, He (cf. p. 26 of the 2001 Eerdmans edition). But the letters on the 2001 edition's cover Stone include the three-pronged letter Shin , also found on the dreidel . What esoteric religious meaning is implied by this, I do not know.
An Adamantine View of "The [Philosophers'] Stone"
The New York Times column "The Stone" on Sunday, Nov. 21 had this—
"Wittgenstein was formally presenting his Tractatus Logico-Philosophicus , an already well-known work he had written in 1921, as his doctoral thesis. Russell and Moore were respectfully suggesting that they didn’t quite understand proposition 5.4541 when they were abruptly cut off by the irritable Wittgenstein. 'I don’t expect you to understand!' (I am relying on local legend here….)"
Proposition 5.4541*—
Related material, found during a further search—
A commentary on "simplex sigillum veri" leads to the phrase "adamantine crystalline structure of logic"—
For related metaphors, see The Diamond Cube, Design Cube 2x2x2, and A Simple Reflection Group of Order 168.
Here Łukasiewicz's phrase "the hardest of materials" apparently suggested the commentators' adjective "adamantine." The word "diamond" in the links above refers of course not to a material, but to a geometric form, the equiangular rhombus. For a connection of this sort of geometry with logic, see The Diamond Theorem and The Geometry of Logic.
For more about God, a Stone, logic, and cubes, see Tale (Nov. 23).
* 5.4541 in the German original—
Die Lösungen der logischen Probleme müssen einfach sein,
denn sie setzen den Standard der Einfachheit.
Die Menschen haben immer geahnt, dass es
ein Gebiet von Fragen geben müsse, deren Antworten—
a priori—symmetrisch, und zu einem abgeschlossenen,
regelmäßigen Gebilde vereint liegen.
Ein Gebiet, in dem der Satz gilt: simplex sigillum veri.
Here "einfach" means "simple," not "neat," and "Gebiet" means
"area, region, field, realm," not (except metaphorically) "sphere."
From "Deus ex Machina and the Aesthetics of Proof"
(Alan J. Cain in The Mathematical Intelligencer * of September 2010, pdf)—
Deus ex Machina
In a narrative, a deus is unsatisfying for two reasons. The
first is that any future attempt to build tension is undercut if
the author establishes that a difficulty can be resolved by a
deus. The second reason—more important for the purposes
of this essay—is that the deus does not fit with the internal
structure of the story. There is no reason internal to the
story why the deus should intervene at that moment.
Santa in the New York Thanksgiving Day Parade
Thanksgiving Day, 2010 (November 25), New York Lottery—
Midday 411, Evening 332.
For 411, see (for instance) April 11 (i.e., 4/11) in 2008 —
For 332, see "A Play for Kristen**" — March 16, 2008 —
"A search for the evening number, 332, in Log24 yields a rather famous line from Sophocles…"
Sophocles, Antigone, edited by Mark Griffith, Cambridge University Press, 1999:
“Many things are formidable (deina ) and none is more formidable (deinoteron ) than man.”
– Antigone , lines 332-333, in Valdis Leinieks, The Plays of Sophokles, John Benjamins Publishing Company, 1982, p. 62
See also the lottery numbers 411 and 332 in this journal on March 22, 2009— "The Storyteller in Chance ."
“… it’s going to be accomplished in steps,
this establishment of the Talented
in the scheme of things.”
— Anne McCaffrey, Radcliffe ’47, To Ride Pegasus
* It seems Santa has delivered an early gift — free online access to all issues of the Intelligencer .
** Teaser headline in the original version at Xanga.com
Steve Martin’s new novel An Object of Beauty will be released tomorrow.
“The most charmingly rendered female schemer since Truman Capote’s Holly Golightly.”
— Elle magazine
“Martin compresses the wild and crazy end of the millennium
and finds in this piercing novel a sardonic morality tale….
Exposes the sound and fury of the rarified Manhattan art world.”
— Publishers Weekly
“Like Steve Martin’s Shopgirl , this very different novel will captivate your attention from start to finish.”
— Joyce Carol Oates
Martin on his character Ray Porter in the novella Shopgirl (published Oct. 11, 2000)—
“He said, ‘I wrote a piece of code
that they just can’t seem to do without.’
He was a symbolic logician. That was his career….”
As the above review notes, Martin’s new book is about art at the end of the millennium.
See also Art Wars: Geometry as Conceptual Art
and some of my own notes from 2000 (March 9) in “Is Nothing Sacred?”
Some related material —
A paperback with a striking cover (artist unknown)—
Note that the background may be constructed from
any of four distinct motifs. For another approach to these
motifs in a philosophical context, see June 8, 2010.
“Visual forms— lines, colors, proportions, etc.— are just as capable of articulation , i.e. of complex combination, as words. But the laws that govern this sort of articulation are altogether different from the laws of syntax that govern language. The most radical difference is that visual forms are not discursive . They do not present their constituents successively, but simultaneously, so the relations determining a visual structure are grasped in one act of vision.”
– Susanne K. Langer, Philosophy in a New Key
In memory of S. Neil Fujita, who died last Saturday—
Fujita did the cover art for this edition.
Another book by Langer with a striking cover (artist unknown)—
Note that the background may be constructed from
any of four distinct motifs. For another approach to these
motifs in a philosophical context, see June 8, 2010.
"Visual forms— lines, colors, proportions, etc.— are just as capable of articulation , i.e. of complex combination, as words. But the laws that govern this sort of articulation are altogether different from the laws of syntax that govern language. The most radical difference is that visual forms are not discursive . They do not present their constituents successively, but simultaneously, so the relations determining a visual structure are grasped in one act of vision."
— Susanne K. Langer, Philosophy in a New Key
Mathematics and Narrative continued
A search for Ursula in this journal yields a story…
“The main character is a slave woman who discovers new patterns in the mosaics.”
Other such stories: Plato’s Meno and Changing Woman —
|
“Kaleidoscope turning…
Shifting pattern within — Roger Zelazny, Eye of Cat |
Philosophical postscript—
“That Lévi-Strauss should have been able to transmute the romantic passion of Tristes Tropiques into the hypermodern intellectualism of La Pensée Sauvage is surely a startling achievement. But there remain the questions one cannot help but ask. Is this transmutation science or alchemy? Is the ‘very simple transformation’ which produced a general theory out of a personal disappointment real or a sleight of hand? Is it a genuine demolition of the walls which seem to separate mind from mind by showing that the walls are surface structures only, or is it an elaborately disguised evasion necessitated by a failure to breach them when they were directly encountered? Is Lévi-Strauss writing, as he seems to be claiming in the confident pages of La Pensée Sauvage, a prolegomenon to all future anthropology? Or is he, like some uprooted neolithic intelligence cast away on a reservation, shuffling the debris of old traditions in a vain attempt to revivify a primitive faith whose moral beauty is still apparent but from which both relevance and credibility have long since departed?”
— Clifford Geertz, conclusion of “The Cerebral Savage: On the Work of Claude Lévi-Strauss“
"A world of made
is not a world of born— pity poor flesh
and trees, poor stars and stones, but never this
fine specimen of hypermagical
ultraomnipotence."
— e. e. cummings, 1944
For one such specimen, see The Matrix of Abraham—
a 5×5 square that is hypermagical… indeed, diabolical.
Related material on the algebra and geometry underlying some smaller structures
that have also, unfortunately, become associated with the word "magic"—
" … listen: there's a hell
of a good universe next door; let's go"
— e. e. cummings
Happy birthday, e. e.
The above is the result of a (fruitless) image search today for a current version of Giovanni Sambin's "Basic Picture: A Structure for Topology."
That search was suggested by the title of today's New York Times op-ed essay "Found in Translation" and an occurrence of that phrase in this journal on January 5, 2007.
Further information on one of the images above—
A search in this journal on the publication date of Giaquinto's Visual Thinking in Mathematics yields the following—
|
In defense of Plato’s realism (vs. sophists’ nominalism– see recent entries.) Plato cited geometry, notably in the Meno , in defense of his realism.
|
For the Meno 's diamond figure in Giaquinto, see a review—
— Review by Jeremy Avigad (preprint)
Finite geometry supplies a rather different context for Plato's "basic picture."
In that context, the Klein four-group often cited by art theorist Rosalind Krauss appears as a group of translations in the mathematical sense. (See Kernel of Eternity and Sacerdotal Jargon at Harvard.)
The Times op-ed essay today notes that linguistic translation "… is not merely a job assigned to a translator expert in a foreign language, but a long, complex and even profound series of transformations that involve the writer and reader as well."
The list of four-group transformations in the mathematical sense is neither long nor complex, but is apparently profound enough to enjoy the close attention of thinkers like Krauss.
Today is the birthday of mathematician Jean-Pierre Serre.
Some remarks related to today's day number within the month, "15"—
The Wikipedia article on finite geometry has the following link—
Carnahan, Scott (2007-10-27), "Small finite sets", Secret Blogging Seminar, http://sbseminar.wordpress.com/2007/10/27/small-finite-sets/, notes on a talk by Jean-Pierre Serre on canonical geometric properties of small finite sets.
From Carnahan's notes (October 27, 2007)—
Serre has been giving a series of lectures at Harvard for the last month, on finite groups in number theory. It started off with some ideas revolving around Chebotarev density, and recently moved into fusion (meaning conjugacy classes, not monoidal categories) and mod p representations. In between, he gave a neat self-contained talk about small finite groups, which really meant canonical structures on small finite sets.
He started by writing the numbers 2,3,4,5,6,7,8, indicating the sizes of the sets to be discussed, and then he tackled them in order.
Related material on finite geometry and the indicated small numbers may, with one apparent exception, be found at my own Notes on Finite Geometry.
The apparent exception is "5." See, however, the role played in finite geometry by this number (and by "15") as sketched by Robert Steinberg at Yale in 1967—
See also …
Or— Childhood's Rear End
This post was suggested by…
Related material:
The Zeppelin album cover, featuring rear views of nude children, was shot at the Giant's Causeway.
From a page at led-zeppelin.org—
See also Richard Rorty on Heidegger—
Safranski, the author of ''Schopenhauer and the Wild Years of Philosophy,'' never steps back and pronounces judgment on Heidegger, but something can be inferred from the German title of his book: ''Ein Meister aus Deutschland'' (''A Master From Germany''). Heidegger was, undeniably, a master, and was very German indeed. But Safranski's spine-chilling allusion is to Paul Celan's best-known poem, ''Death Fugue.'' In Michael Hamburger's translation, its last lines are:
death is a master from Germany his eyes are blue
he strikes you with leaden bullets his aim is true
a man lives in the house your golden hair Margarete
he sets his pack on us he grants us a grave in the air
he plays with the serpents and daydreams death is a master from Germany
your golden hair Margarete
your ashen hair Shulamith.
No one familiar with Heidegger's work can read Celan's poem without recalling Heidegger's famous dictum: ''Language is the house of Being. In its home man dwells.'' Nobody who makes this association can reread the poem without having the images of Hitler and Heidegger — two men who played with serpents and daydreamed — blend into each other. Heidegger's books will be read for centuries to come, but the smell of smoke from the crematories — the ''grave in the air'' — will linger on their pages.
Heidegger is the antithesis of the sort of philosopher (John Stuart Mill, William James, Isaiah Berlin) who assumes that nothing ultimately matters except human happiness. For him, human suffering is irrelevant: philosophy is far above such banalities. He saw the history of the West not in terms of increasing freedom or of decreasing misery, but as a poem. ''Being's poem,'' he once wrote, ''just begun, is man.''
For Heidegger, history is a sequence of ''words of Being'' — the words of the great philosophers who gave successive historical epochs their self-image, and thereby built successive ''houses of Being.'' The history of the West, which Heidegger also called the history of Being, is a narrative of the changes in human beings' image of themselves, their sense of what ultimately matters. The philosopher's task, he said, is to ''preserve the force of the most elementary words'' — to prevent the words of the great, houses-of-Being-building thinkers of the past from being banalized.
Related musical meditations—
Shine On (Saturday, April 21, 2007), Shine On, Part II, and Built (Sunday, April 22, 2007).
Related pictorial meditations—
The Giant's Causeway at Peter J. Cameron's weblog
and the cover illustration for Diamond Theory (1976)—
The connection between these two images is the following from Cameron's weblog today—
… as we saw, there are two different Latin squares of order 4;
one, but not the other, can be extended to a complete set
of 3 MOLS [mutually orthogonal Latin squares].
The underlying structures of the square pictures in the Diamond Theory cover are those of the two different Latin squares of order 4 mentioned by Cameron.
Connection with childhood—
The children's book A Wind in the Door, by Madeleine L'Engle. See math16.com. L'Engle's fantasies about children differ from those of Arthur C. Clarke and Led Zeppelin.
There is a remarkable correspondence between the 35 partitions of an eight-element set H into two four-element sets and the 35 partitions of the affine 4-space L over GF(2) into four parallel four-point planes. Under this correspondence, two of the H-partitions have a common refinement into 2-sets if and only if the same is true of the corresponding L-partitions (Peter J. Cameron, Parallelisms of Complete Designs, Cambridge U. Press, 1976, p. 60). The correspondence underlies the isomorphism* of the group A8 with the projective general linear group PGL(4,2) and plays an important role in the structure of the large Mathieu group M24.
A 1954 paper by W.L. Edge suggests the correspondence should be named after E.H. Moore. Hence the title of this note.
Edge says that
It is natural to ask what, if any, are the 8 objects which undergo
permutation. This question was discussed at length by Moore…**.
But, while there is no thought either of controverting Moore's claim to
have answered it or of disputing his priority, the question is primarily
a geometrical one….
Excerpts from the Edge paper—
Excerpts from the Moore paper—
Pages 432, 433, 434, and 435, as well as the section mentioned above by Edge— pp. 438 and 439
* J.W.P. Hirschfeld, Finite Projective Spaces of Three Dimensions, Oxford U. Press, 1985, p. 72
** Edge cited "E.H. Moore, Math. Annalen, 51 (1899), 417-44." A more complete citation from "The Scientific Work of Eliakim Hastings Moore," by G.A. Bliss, Bull. Amer. Math. Soc. Volume 40, Number 7 (1934), 501-514— E.H. Moore, "Concerning the General Equations of the Seventh and Eighth Degrees," Annalen, vol. 51 (1899), pp. 417-444.
From the current index to obituaries at Telegraph.co.uk—
Teufel is also featured in today's New York Times—
"Mr. Teufel became a semicelebrity, helped in no small part by his last name, which means 'devil' in German."
From Group Analysis , June 1993, vol. 26 no. 2, 203-212—
by Ronald Sandison, Ledbury, Herefordshire HR8 2EY, UK
In my contribution to the Group Analysis Special Section: "Aspects of Religion in Group Analysis" (Sandison, 1993) I hinted that any consideration of a spiritual dimension to the group involves us in a discussion on whether we are dealing with good or evil spirits. But if we say that God is in the group, why is not the Devil there also? Can good and evil coexist in the same group matrix? Is the recognition of evil "nothing but" the ability to distinguish between good and bad? If not, then what is evil? Is it no more than the absence of good?
These and other questions were worked on at a joint Institute of Group Analysis and Group-Analytic Society (London) Workshop entitled "The Problem of Good and Evil." We considered the likelihood that good and evil coexist in all of us, as well as in the whole of the natural world, not only on earth, but in the cosmos and in God himself What we actually do with good and evil is to split them apart, thereby shelving the problem but at the same time creating irreconcilable opposites. This article examines this splitting and how we can work with it psychoanalytically.
This suggests a biblical remark—
"Now there was a day… when the sons of God
came to present themselves before the Lord,
and Satan came also among them."
— Job 1:6, quoted by Chesterton in The Man Who Was Thursday
Sandison died on June 18. See the Thursday, August 5, Log24 post "The Matrix."
Teufel died on July 6. See the Log24 posts for that day.
The title of this post, "rift designs," refers to a recurring theme in the July 6 posts. It is taken from Heidegger.
From a recent New Yorker review of Absence of Mind by Marilynne Robinson—
"Robinson is eloquent in her defense of the mind’s prerogatives, but her call for a renewed metaphysics might be better served by rereading Heidegger than by dusting off the Psalms."
Following this advice, we find—
"Propriation gathers the rift-design of the saying and unfolds it in such a way that it becomes the well-joined structure of a manifold showing."
— p. 415 of Heidegger's Basic Writings , edited by David Farrell Krell, HarperCollins paperback, 1993
"Das Ereignis versammelt den Aufriß der Sage und entfaltet ihn zum Gefüge des vielfältigen Zeigens."
— Heidegger, Weg zur Sprache
"Eight is a gate."
— This journal, December 2002
Tralfamadorian Structure
in Slaughterhouse-Five
includes the following passage:
“…the nonlinear characterization of Billy Pilgrim
emphasizes that he is not simply an established
identity who undergoes a series of changes but
all the different things he is at different times.”
This suggests that the above structure be viewed
as illustrating not eight parts but rather
8! = 40,320 parts.
See also April 2, 2003.
Happy birthday to John Huston and
happy dies natalis to Richard Burton.
From a search in this journal for "Krell"—
Dialogue from an American adaptation of Shakespeare's Tempest—
“… Which makes it a gilt-edged priority that one of us
gets into that Krell lab and takes that brain boost.”
– Taken from a video, Forbidden Planet Monster Attack
From yesterday's A Manifold Showing—
"Propriation gathers the rift-design of the saying and unfolds it
in such a way that it becomes the well-joined structure of a manifold showing."
(p. 415 of Heidegger's Basic Writings, edited by David Farrell Krell,
HarperCollins paperback, 1993)
German versions found on the Web—
„Das Ereignis versammelt den Aufriß der Sage und entfaltet ihn zum Gefüge des vielfältigen Zeigens.“ 323
323 Heidegger, Weg zur Sprache, S. 259.
"Das Regende im Zeigen der Sage ist das Eignen. Es erbringt das An- und Abwesen in sein jeweilig Eigenes, aus dem dieses sich an ihm selbst zeigt und nach seiner Art verweilt. Das erbringende Eignen, das die Sage als die Zeige in ihrem Zeigen regt, heiße das Ereignen. Es er-gibt das Freie der Lichtung, in die Anwesendes anwähren, aus der Abwesendes entgehen und im Entzug sein Währen behalten kann. Was das Ereignen durch die Sage ergibt, ist nie Wirkung einer Ursache, nicht die Folge eines Grundes. Das erbringende Eignen, das Ereignen, ist gewährender als jedes Wirken, Machen und Gründen. Das Ereignende ist das Ereignis selbst – und nichts außerdem. Das Ereignis, im Zeigen der Sage erblickt, läßt sich weder als ein Vorkommnis noch als ein Geschehen vorstellen, sondern nur im Zeigen der Sage als das Gewährende erfahren. Es gibt nichts anderes, worauf das Ereignis noch zurückführt, woraus es gar erklärt werden könnte. Das Ereignen ist kein Ergebnis (Resultat) aus anderem, aber die Er-gebnis, deren reichendes Geben erst dergleichen wie ein `Es gibt' gewährt, dessen auch noch `das Sein' bedarf, um als Anwesen in sein Eigenes zu gelangen. Das Ereignis versammelt den Aufriß der Sage und entfaltet ihn zum Gefüge des Vielfältigen Zeigens. Das Ereignis ist das Unscheinbarste des Unscheinbaren, das Einfachste des Einfachen, das Nächste des Nahen und das Fernste des Fernen, darin wir Sterbliche uns zeitlebens aufhalten." 8
8 M. Heidegger: Unterwegs zur Sprache. S. 258 f.
From Google Translate:
"The event brings together the outline of the legend and unfolds it to the structure of the manifold showing."
"Heidegger suggests that we experience the saying of language as a shining forth:
'It lets what is coming to presence shine forth, lets what is withdrawing into absence vanish. The saying is by no means the supplemental linguistic expression of what shines forth; rather, all shining and fading depend on the saying that shows.' (pp. 413-414).
But what is the basis and origin of this possibility of saying? The happening of saying in the clearing, its allowing things to shine forth, can also be called an 'owning.' Owning is the event of a thing’s coming into its own, of its showing itself as itself. Heidegger also calls it 'propriating,' 'en-owning,' or Ereignis:
'Propriation gathers the rift-design of the saying and unfolds it in such a way that it becomes the well-joined structure of a manifold showing. (p. 415)'"
— "Heidegger: On the Way to Language," by Paul Livingston
Page references are apparently to Heidegger's Basic Writings, edited by David Farrell Krell, HarperCollins paperback, 1993.
See also Shining Forth.
or: Combinatorics (Rota) as Philosophy (Heidegger) as Geometry (Me)
“Dasein’s full existential structure is constituted by
the ‘as-structure’ or ‘well-joined structure’ of the rift-design*…”
— Gary Williams, post of January 22, 2010
Background—
Gian-Carlo Rota on Heidegger…
“… The universal as is given various names in Heidegger’s writings….
The discovery of the universal as is Heidegger’s contribution to philosophy….
The universal ‘as‘ is the surgence of sense in Man, the shepherd of Being.
The disclosure of the primordial as is the end of a search that began with Plato….
This search comes to its conclusion with Heidegger.”
— “Three Senses of ‘A is B’ in Heideggger,” Ch. 17 in Indiscrete Thoughts
… and projective points as separating rifts—
* rift-design— Definition by Deborah Levitt—
“Rift. The stroke or rending by which a world worlds, opening both the ‘old’ world and the self-concealing earth to the possibility of a new world. As well as being this stroke, the rift is the site— the furrow or crack— created by the stroke. As the ‘rift design‘ it is the particular characteristics or traits of this furrow.”
— “Heidegger and the Theater of Truth,” in Tympanum: A Journal of Comparative Literary Studies, Vol. 1, 1998
See David Corfield,
"The Robustness of Mathematical Entities."
This is an abstract from a paper at a conference,
"From Practice to Results in Logic and Mathematics"
(June 21st-23rd, 2010, Archives Henri Poincaré,
University of Nancy (France)).
See also Corfield's post "Inevitability in Mathematics"
at the n-Category Café today. He links to an earlier
post, "Mathematical Robustness." From that post—
…let’s see what Michiel Hazewinkel has to say
in his paper Niceness theorems:
It appears that many important mathematical objects
(including counterexamples) are unreasonably
nice, beautiful and elegant. They tend to have
(many) more (nice) properties and extra bits
of structure than one would a priori expect….
Excerpts from "The Concept of Group and the Theory of Perception,"
by Ernst Cassirer, Philosophy and Phenomenological Research,
Volume V, Number 1, September, 1944.
(Published in French in the Journal de Psychologie, 1938, pp. 368-414.)
The group-theoretical interpretation of the fundaments of geometry is,
from the standpoint of pure logic, of great importance, since it enables us to
state the problem of the "universality" of mathematical concepts in simple
and precise form and thus to disentangle it from the difficulties and ambigui-
ties with which it is beset in its usual formulation. Since the times of the
great controversies about the status of universals in the Middle Ages, logic
and psychology have always been troubled with these ambiguities….
Our foregoing reflections on the concept of group permit us to define more
precisely what is involved in, and meant by, that "rule" which renders both
geometrical and perceptual concepts universal. The rule may, in simple
and exact terms, be defined as that group of transformations with regard to
which the variation of the particular image is considered. We have seen
above that this conception operates as the constitutive principle in the con-
struction of the universe of mathematical concepts….
…Within Euclidean geometry,
a "triangle" is conceived of as a pure geometrical "essence," and this
essence is regarded as invariant with respect to that "principal group" of
spatial transformations to which Euclidean geometry refers, viz., displace-
ments, transformations by similarity. But it must always be possible to
exhibit any particular figure, chosen from this infinite class, as a concrete
and intuitively representable object. Greek mathematics could not
dispense with this requirement which is rooted in a fundamental principle
of Greek philosophy, the principle of the correlatedness of "logos" and
"eidos." It is, however, characteristic of the modern development of
mathematics, that this bond between "logos" and "eidos," which was indis-
soluble for Greek thought, has been loosened more and more, to be, in the
end, completely broken….
…This process has come to its logical
conclusion and systematic completion in the development of modern group-
theory. Geometrical figures are no longer regarded as fundamental, as
date of perception or immediate intuition. The "nature" or "essence" of a
figure is defined in terms of the operations which may be said to
generate the figure. The operations in question are, in turn, subject to
certain group conditions….
…What we
find in both cases are invariances with respect to variations undergone by
the primitive elements out of which a form is constructed. The peculiar
kind of "identity" that is attributed to apparently altogether heterogen-
eous figures in virtue of their being transformable into one another by means
of certain operations defining a group, is thus seen to exist also in the
domain of perception. This identity permits us not only to single out ele-
ments but also to grasp "structures" in perception. To the mathematical
concept of "transformability" there corresponds, in the domain of per-
ception, the concept of "transposability." The theory of the latter con-
cept has been worked out step by step and its development has gone through
various stages….
…By the acceptance of
"form" as a primitive concept, psychological theory has freed it from the
character of contingency which it possessed for its first founders. The inter-
pretation of perception as a mere mosaic of sensations, a "bundle" of simple
sense-impressions has proved untenable….
…In the domain of mathematics this state of affairs mani-
fests itself in the impossibility of searching for invariant properties of a
figure except with reference to a group. As long as there existed but one
form of geometry, i.e., as long as Euclidean geometry was considered as the
geometry kat' exochen this fact was somehow concealed. It was possible
to assume implicitly the principal group of spatial transformations that lies
at the basis of Euclidean geometry. With the advent of non-Euclidean
geometries, however, it became indispensable to have a complete and sys-
tematic survey of the different "geometries," i.e., the different theories of
invariancy that result from the choice of certain groups of transformation.
This is the task which F. Klein set to himself and which he brought to a
certain logical fulfillment in his Vergleichende Untersuchungen ueber neuere
geometrische Forschungen….
…Without discrimination between the
accidental and the substantial, the transitory and the permanent, there
would be no constitution of an objective reality.
This process, unceasingly operative in perception and, so to speak, ex-
pressing the inner dynamics of the latter, seems to have come to final per-
fection, when we go beyond perception to enter into the domain of pure
thought. For the logical advantage and peculiar privilege of the pure con –
cept seems to consist in the replacement of fluctuating perception by some-
thing precise and exactly determined. The pure concept does not lose
itself in the flux of appearances; it tends from "becoming" toward "being,"
from dynamics toward statics. In this achievement philosophers have
ever seen the genuine meaning and value of geometry. When Plato re-
gards geometry as the prerequisite to philosophical knowledge, it is because
geometry alone renders accessible the realm of things eternal; tou gar aei
ontos he geometrike gnosis estin. Can there be degrees or levels of objec-
tive knowledge in this realm of eternal being, or does not rather knowledge
attain here an absolute maximum? Ancient geometry cannot but answer
in the affirmative to this question. For ancient geometry, in the classical
form it received from Euclid, there was such a maximum, a non plus ultra.
But modern group theory thinking has brought about a remarkable change
In this matter. Group theory is far from challenging the truth of Euclidean
metrical geometry, but it does challenge its claim to definitiveness. Each
geometry is considered as a theory of invariants of a certain group; the
groups themselves may be classified in the order of increasing generality.
The "principal group" of transformations which underlies Euclidean geome-
try permits us to establish a number of properties that are invariant with
respect to the transformations in question. But when we pass from this
"principal group" to another, by including, for example, affinitive and pro-
jective transformations, all that we had established thus far and which,
from the point of view of Euclidean geometry, looked like a definitive result
and a consolidated achievement, becomes fluctuating again. With every
extension of the principal group, some of the properties that we had taken
for invariant are lost. We come to other properties that may be hierar-
chically arranged. Many differences that are considered as essential
within ordinary metrical geometry, may now prove "accidental." With
reference to the new group-principle they appear as "unessential" modifica-
tions….
… From the point of view of modern geometrical systematization,
geometrical judgments, however "true" in themselves, are nevertheless not
all of them equally "essential" and necessary. Modern geometry
endeavors to attain progressively to more and more fundamental strata of
spatial determination. The depth of these strata depends upon the com-
prehensiveness of the concept of group; it is proportional to the strictness of
the conditions that must be satisfied by the invariance that is a universal
postulate with respect to geometrical entities. Thus the objective truth
and structure of space cannot be apprehended at a single glance, but have to
be progressively discovered and established. If geometrical thought is to
achieve this discovery, the conceptual means that it employs must become
more and more universal….
A recently created Wikipedia article says that “The Miracle Octad Generator [MOG] is an array of coordinates, arranged in four rows and six columns, capable of describing any point in 24-dimensional space….” (Clearly any array with 24 parts is so capable.) The article ignores the fact that the MOG, as defined by R.T. Curtis in 1976, is not an array of coordinates, but rather a picture of a correspondence between two sets, each containing 35 structures. (As a later commentator has remarked, this correspondence is a well-known one that preserves a certain incidence property. See Eightfold Geometry.)
From the 1976 paper defining the MOG—
“There is a correspondence between the two systems of 35 groups, which is illustrated in Fig. 4 (the MOG or Miracle Octad Generator).” —R.T. Curtis, “A New Combinatorial Approach to M24,” Mathematical Proceedings of the Cambridge Philosophical Society (1976), 79: 25-42
Curtis’s 1976 Fig. 4. (The MOG.)
The Wikipedia article, like a similar article at PlanetMath, is based on a different definition, from a book first published in 1988—
I have not seen the 1973 Curtis paper, so I do not know whether it uses the 35-sets correspondence definition or the 6×4 array definition. The remarks of Conway and Sloane on page 312 of the 1998 edition of their book about “Curtis’s original way of finding octads in the MOG [Cur2]” indicate that the correspondence definition was the one Curtis used in 1973—
Here the picture of “the 35 standard sextets of the MOG”
is very like (modulo a reflection) Curtis’s 1976 picture
of the MOG as a correspondence between two 35-sets.
A later paper by Curtis does use the array definition. See “Further Elementary Techniques Using the Miracle Octad Generator,” Proceedings of the Edinburgh Mathematical Society (1989) 32, 345-353.
The array definition is better suited to Conway’s use of his hexacode to describe octads, but it obscures the close connection of the MOG with finite geometry. That connection, apparent in the phrases “vector space structure in the standard square” and “parallel 2-spaces” (Conway and Sloane, third ed., p. 312, illustrated above), was not discussed in the 1976 Curtis paper. See my own page on the MOG at finitegeometry.org.
Related web pages:
Miracle Octad Generator,
Generating the Octad Generator,
Geometry of the 4×4 Square
Related folklore:
"It is commonly known that there is a bijection between the 35 unordered triples of a 7-set [i.e., the 35 partitions of an 8-set into two 4-sets] and the 35 lines of PG(3,2) such that lines intersect if and only if the corresponding triples have exactly one element in common." –"Generalized Polygons and Semipartial Geometries," by F. De Clerck, J. A. Thas, and H. Van Maldeghem, April 1996 minicourse, example 5 on page 6
The Miracle Octad Generator may be regarded as illustrating the folklore.
Update of August 20, 2010–
For facts rather than folklore about the above bijection, see The Moore Correspondence.
From the conclusion of Weyl's Symmetry —
One example of Weyl's "structure-endowed entity" is a partition of a six-element set into three disjoint two-element sets– for instance, the partition of the six faces of a cube into three pairs of opposite faces.
The automorphism group of this faces-partition contains an order-8 subgroup that is isomorphic to the abstract group C2×C2×C2 of order eight–
The action of Klein's simple group of order 168 on the Cayley diagram of C2×C2×C2 in yesterday's post furnishes an example of Weyl's statement that
"… one may ask with respect to a given abstract group: What is the group of its automorphisms…?"
"The cube has…13 axes of symmetry:
6 C2 (axes joining midpoints of opposite edges),
4 C3 (space diagonals), and
3C4 (axes joining opposite face centroids)."
–Wolfram MathWorld article on the cube
These 13 symmetry axes can be used to illustrate the interplay between Euclidean and Galois geometry in a cubic model of the 13-point Galois plane.
The geometer's 3×3×3 cube–
27 separate subcubes unconnected
by any Rubik-like mechanism–
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
A closely related structure–
the finite projective plane
with 13 points and 13 lines–
A later version of the 13-point plane
by Ed Pegg Jr.–
A group action on the 3×3×3 cube
as illustrated by a Wolfram program
by Ed Pegg Jr. (undated, but closely
related to a March 26, 1985 note
by Steven H. Cullinane)–
The above images tell a story of sorts.
The moral of the story–
Galois projective geometries can be viewed
in the context of the larger affine geometries
from which they are derived.
The standard definition of points in a Galois projective plane is that they are lines through the (arbitrarily chosen) origin in a corresponding affine 3-space converted to a vector 3-space.
If we choose the origin as the center cube in coordinatizing the 3×3×3 cube (See Weyl's relativity problem ), then the cube's 13 axes of symmetry can, if the other 26 cubes have properly (Weyl's "objectively") chosen coordinates, illustrate nicely the 13 projective points derived from the 27 affine points in the cube model.
The 13 lines of the resulting Galois projective plane may be derived from Euclidean planes through the cube's center point that are perpendicular to the cube's 13 Euclidean symmetry axes.
The above standard definition of points in a Galois projective plane may of course also be used in a simpler structure– the eightfold cube.
(The eightfold cube also allows a less standard way to picture projective points that is related to the symmetries of "diamond" patterns formed by group actions on graphic designs.)
See also Ed Pegg Jr. on finite geometry on May 30, 2006
at the Mathematical Association of America.
Where Three Worlds Meet
From an obituary for David Brown, who died at 93 on Monday–
"David Brown was a force in the entertainment, literary and journalism worlds," Frank A. Bennack, Jr., vice chairman and chief executive officer of Hearst Corporation, said in a statement Tuesday. —Polly Anderson of the Associated Press
Mark Kramer, "Breakable Rules for Literary Journalists," Section 8–
"Readers are likely to care about how a situation came about and what happens next when they are experiencing it with the characters. Successful literary journalists never forget to be entertaining. The graver the writer's intentions, and the more earnest and crucial the message or analysis behind the story, the more readers ought to be kept engaged. Style and structure knit story and idea alluringly.
If the author does all this storytelling and digressing and industrious structure-building adroitly, readers come to feel they are heading somewhere with purpose, that the job of reading has a worthy destination. The sorts of somewheres that literary journalists reach tend to marry eternal meanings and everyday scenes. Richard Preston's 'The Mountains of Pi,' for instance, links the awkward daily lives of two shy Russian emigre mathematicians to their obscure intergalactic search for hints of underlying order in a chaotic universe."
Hints:
“Logic is all about the entertaining of possibilities.”
— Colin McGinn, Mindsight: Image, Dream, Meaning, Harvard U. Press, 2004
"According to the Buddha, scholars speak in sixteen ways of the state of the soul after death…. While I hesitate to disagree with the Compassionate One, I think there are more than sixteen possibilities described here…."
"That's entertainment!"
A NY Times review dated Jan. 20 has the headline
Trying to Paint the Deity by Numbers
Against a Backdrop of Jewish Culture
By JANET MASLIN
"…this novel’s bracing intellectual energy never flags. Though it is finally more a work of showmanship than scholarship, it affirms Ms. Goldstein’s position as a satirist…."
The title of the book under review is
36 Arguments for the
Existence of God: A Work of Fiction.
Related "by the numbers" material–
From the I Ching, commentaries on the lines of Hexagram 36–
"Here the Lord of Light is in a subordinate place and is wounded by the Lord of Darkness…."
"The dark power at first held so high a place that it could wound all who were on the side of good and of the light. But in the end it perishes of its own darkness, for evil must itself fall at the very moment when it has wholly overcome the good, and thus consumed the energy to which it owed its duration."
The Times review of 36 Arguments notes that the book's chapters of fiction number 36, as do the 36 philosophical arguments in the book's title and appendix.
The reviewer– "So much for structure. It is not Ms. Goldstein’s strong suit…."
Some structure related to the above occurrence of 36 in the I Ching—
Another example of eightfold symmetry:
The Large Hadron Collider
See also Angels & Demons in
Hollywood and in this journal.
From the September 1953 Bulletin of the American Mathematical Society—
Emil Artin, in a review of Éléments de mathématique, by N. Bourbaki, Book II, Algebra, Chaps. I-VII–
"We all believe that mathematics is an art. The author of a book, the lecturer in a classroom tries to convey the structural beauty of mathematics to his readers, to his listeners. In this attempt he must always fail. Mathematics is logical to be sure; each conclusion is drawn from previously derived statements. Yet the whole of it, the real piece of art, is not linear; worse than that its perception should be instantaneous. We all have experienced on some rare occasions the feeling of elation in realizing that we have enabled our listeners to see at a moment's glance the whole architecture and all its ramifications. How can this be achieved? Clinging stubbornly to the logical sequence inhibits the visualization of the whole, and yet this logical structure must predominate or chaos would result."
Art Versus Chaos
From an exhibit,
"Reimagining Space"
The above tesseract (4-D hypercube)
sculpted in 1967 by Peter Forakis
provides an example of what Artin
called "the visualization of the whole."
For related mathematical details see
Diamond Theory in 1937.
"'The test?' I faltered, staring at the thing.
'Yes, to determine whether you can live
in the fourth dimension or only die in it.'"
— Fritz Leiber, 1959
See also the Log24 entry for
Nov. 26, 2009, the date that
Forakis died.
"There is such a thing
as a tesseract."
— Madeleine L'Engle, 1962
“Orthodox Jews are disappearing from Jerusalem. One moment they are praying at the Western Wall, and in the blink of an eye, they seem to evaporate…. In order to build the Third Temple while being respectful of the Islamic structures on the Temple Mount, the Jews have discovered a way to access a fourth spatial dimension. They will build the Third Temple invisibly ‘above’ the Temple Mount and ‘above’ the Mosque in the direction of the fourth dimension.”
— Clifford Pickover, description of his novel Jews in Hyperspace
“If you have built castles in the air, your work need not be lost; that is where they should be. Now put the foundations under them.”
— Henry David Thoreau, conclusion of Walden
Related material: Log24 entries, morning and evening of June 11, 2009, “Text” (June 22, 2009), and Salomon Bochner‘s remarks on space in “Eight is a Gate” (Feb. 26, 2008).
As noted here yesterday, Claude Levi-Strauss may have died on Devil's Night, on Halloween, or on All Saints' Day. He was apparently a myth-transformer to the end.
The Independent says today he died on Sunday, All Saints' Day. Its eulogy, by Adam Kuper, is well-written, noting that linguist Roman Jakobson was a source of Levi-Strauss's theory of oppositions in myth, and observing that
"… binary oppositions tend to accumulate to form structures…."
Yes, they do. Examples:
I. The structures in the Diamond Puzzle…
Click on image for Jungian background.
II: The structure on a recent cover of Semiotica…
The Semiotica article by mathematical linguist Solomon Marcus is a defense of the Levi-Strauss canonic formula mentioned here yesterday.
It is available online for $40.
A less expensive, and possibly more informative, look at oppositions in linguistics is available for free online in a 1984 master's thesis (pdf, 8+ mb)–
"Language, Linguistics, and Philosophy: A Comparison of the Work of Roman Jakobson and the Later Wittgenstein, with Some Attention to the Philosophy of Charles Saunders Peirce," by Miles Spencer Kimball.
See Annals of Aesthetics,
January 13, 2009,
which features the following
example of modernism:
… and for readers of
the Sunday New York Times …
The book's author, Audrey Niffenegger, has stated that her title refers to "the doubling and twinning and opposites" that are "essential to the theme and structure of the book." For examples of doubling, twinning, and opposites that I prefer to Niffenegger's, see this journal's Saturday and Sunday entries.
Fans of the New York Times's cultural coverage may prefer Niffenegger's own art work. They may also enjoy images from the weekend's London Art Book Fair that suggested the rather different sort of book in Saturday's entry.
Abstract:
"Quantum mechanics, which has no completely accepted interpretation but many seemingly strange physical results, has been interpreted in a number of bizarre and fascinating ways over the years. The two interpretations examined in this paper, [Aage] Bohr and [Ole] Ulfbeck's 'Genuine Fortuitousness' and Stuckey, Silberstein, and Cifone's 'Relational Blockworld,' seem to be two such strange interpretations; Genuine Fortuitousness posits that causality is not fundamental to the universe, and Relational Blockworld suggests that time does not act as we perceive it to act. In this paper, I analyze these two interpretations…."
— "Genuine Fortuitousness, Relational Blockworld, Realism, and Time" (pdf), by Daniel J. Peterson, Honors Thesis, Swarthmore College, December 13, 2007
“Music and mathematics are among the pre-eminent wonders of the race. Levi-Strauss sees in the invention of melody ‘a key to the supreme mystery’ of man– a clue, could we but follow it, to the singular structure and genius of the species. The power of mathematics to devise actions for reasons as subtle, witty, manifold as any offered by sensory experience and to move forward in an endless unfolding of self-creating life is one of the strange, deep marks man leaves on the world. Chess, on the other hand, is a game in which thirty-two bits of ivory, horn, wood, metal, or (in stalags) sawdust stuck together with shoe polish, are pushed around on sixty-four alternately coloured squares. To the addict, such a description is blasphemy. The origins of chess are shrouded in mists of controversy, but unquestionably this very ancient, trivial pastime has seemed to many exceptionally intelligent human beings of many races and centuries to constitute a reality, a focus for the emotions, as substantial as, often more substantial than, reality itself. Cards can come to mean the same absolute. But their magnetism is impure. A mania for whist or poker hooks into the obvious, universal magic of money. The financial element in chess, where it exists at all, has always been small or accidental.
To a true chess player, the pushing about of thirty-two counters on 8×8 squares is an end in itself, a whole world next to which that of a mere biological or political or social life seems messy, stale, and contingent. Even the patzer, the wretched amateur who charges out with his knight pawn when the opponent’s bishop decamps to R4, feels this daemonic spell. There are siren moments when quite normal creatures otherwise engaged, men such as Lenin and myself, feel like giving up everything– marriage, mortgages, careers, the Russian Revolution– in order to spend their days and nights moving little carved objects up and down a quadrate board. At the sight of a set, even the tawdriest of plastic pocket sets, one’s fingers arch and a coldness as in a light sleep steals over one’s spine. Not for gain, not for knowledge or reknown, but in some autistic enchantment, pure as one of Bach’s inverted canons or Euler’s formula for polyhedra.”
— George Steiner in “A Death of Kings,” The New Yorker, issue dated September 7, 1968, page 133
“Examples are the stained-glass windows of knowledge.” —Nabokov
Click above images for some context.
“Inside the church, the grief was real. Sen. Edward Kennedy’s voice caught as he read his lovely eulogy, and when he was done, Caroline Kennedy Schlossberg stood up and hugged him. She bravely read from Shakespeare’s ‘The Tempest‘ (‘Our revels now are ended. We are such stuff as dreams are made on‘). Many of the 315 mourners, family and friends of the Kennedys and Bessettes, swallowed hard through a gospel choir’s rendition of ‘Amazing Grace,’ and afterward, they sang lustily as Uncle Teddy led the old Irish songs at the wake.”
— Newsweek magazine, issue dated August 2, 1999
The Ba gua (Chinese….) are eight diagrams used in Taoist cosmology to represent a range of interrelated concepts. Each consists of three lines, each either ‘broken’ or ‘unbroken,’ representing a yin line or a yang line, respectively. Due to their tripartite structure, they are often referred to as ‘trigrams’ in English. —Wikipedia
"Edward T. Hall, a cultural anthropologist
who pioneered the study of nonverbal
communication and interactions between
members of different ethnic groups,
died July 20 at his home in
Santa Fe, N.M. He was 95."
NY Times piece quoted here on
the date of Hall's death:
|
"July 20, 1969, was the moment NASA needed, more than anything else in this world, the Word. But that was something NASA's engineers had no specifications for. At this moment, that remains the only solution to recovering NASA's true destiny, which is, of course, to build that bridge to the stars." Commentary —
The Word according to St. John:
|
"Mr. Hall first became interested in
space and time as forms of cultural
expression while working on
Navajo and Hopi reservations
in the 1930s."
Log24, July 29:
|
"Kaleidoscope turning…
Shifting pattern within |
"We are the key."
— Eye of Cat
Paul Newall, "Kieślowski's Three Colours Trilogy"—
"Julie recognises the music of the busker outside playing a recorder as that of her husband's. When she asks him where he heard it, he replies that he makes up all sorts of things. This is an instance of a theory of Kieślowski's that 'different people, in different places, are thinking the same thing but for different reasons.' With regard to music in particular, he held what might be characterised as a Platonic view according to which notes pre-exist and are picked out and assembled by people. That these can accord with one another is a sign of what connects people, or so he believed."
The above photo of Juliette Binoche in Blue accompanying the quotations from Zelazny illustrates Kieślowski's concept, with graphic designs instead of musical notes. Some of the same designs are discussed in Abstraction and the Holocaust (Mark Godfrey, Yale University Press, 2007). (See the Log24 entries of June 11, 2009.)
Related material:
"Jeffrey Overstreet, in his book Through a Screen Darkly, comments extensively on Blue. He says these stones 'are like strands of suspended crystalline tears, pieces of sharp-edged grief that Julie has not been able to express.'….
Throughout the film the color blue crops up, highlighting the mood of Julie's grief. A blue light occurs frequently, when Julie is caught by some fleeting memory. Accompanied by strains of an orchestral composition, possibly her husband's, these blue screen shots hold for several seconds while Julie is clearly processing something. The meaning of this blue light is unexplained. For Overstreet, it is the spirit of reunification of broken things."
— Martin Baggs at Mosaic Movie Connect Group on Sunday, March 15, 2009. (Cf. Log24 on that date.)
For such a spirit, compare Binoche's blue mobile in Blue with Binoche's gathered shards in Bee Season.
Related material:
Dialogue from Forbidden Planet —
Dialogue from another story —
“Sizewise?”
“Brainwise, but what they did was multiply me by myself into a quadratic.”
— Psychoshop, by Bester and Zelazny, 1998 paperback, p. 7
“… which would produce a special being– by means of that ‘cloned quadratic crap.’ [P. 75] The proper term sounds something like ‘Kaleideion‘….”
“So Adam is a Kaleideion?”
She shook her head.
“Not a Kaleideion. The Kaleideion….”
— Psychoshop, 1998 paperback, p. 85
|
“Kaleidoscope turning…
Shifting pattern within
|
“When life itself seems lunatic,
who knows where madness lies?”
— For the source, see
Joyce’s Nightmare Continues.
A Tangled Tale
Proposed task for a quantum computer:
"Using Twistor Theory to determine the plotline of Bob Dylan's 'Tangled up in Blue'"
One approach to a solution:
"In this scheme the structure of spacetime is intrinsically quantum mechanical…. We shall demonstrate that the breaking of symmetry in a QST [quantum space-time] is intimately linked to the notion of quantum entanglement."
— "Theory of Quantum Space-Time," by Dorje C. Brody and Lane P. Hughston, Royal Society of London Proceedings Series A, Vol. 461, Issue 2061, August 2005, pp. 2679-2699
(See also The Klein Correspondence, Penrose Space-Time, and a Finite Model.)
For some less technical examples of broken symmetries, see yesterday's entry, "Alphabet vs. Goddess."
That entry displays a painting in 16 parts by Kimberly Brooks (daughter of Leonard Shlain– author of The Alphabet Versus the Goddess— and wife of comedian Albert Brooks (real name: Albert Einstein)). Kimberly Brooks is shown below with another of her paintings, titled "Blue."
"She was workin' in a topless place And I stopped in for a beer, I just kept lookin' at the side of her face In the spotlight so clear. And later on as the crowd thinned out I's just about to do the same, She was standing there in back of my chair Said to me, 'Don't I know your name?' I muttered somethin' underneath my breath, She studied the lines on my face. I must admit I felt a little uneasy When she bent down to tie the laces of my shoe, Tangled up in blue." -- Bob Dylan
Further entanglement with blue:
The website of the Los Angeles Police Department, designed by Kimberly Brooks's firm, Lightray Productions.
Further entanglement with shoelaces:
"Entanglement can be transmitted through chains of cause and effect– and if you speak, and another hears, that too is cause and effect. When you say 'My shoelaces are untied' over a cellphone, you're sharing your entanglement with your shoelaces with a friend."
— "What is Evidence?," by Eliezer Yudkowsky
The White Itself
David Ellerman has written that
"The notion of a concrete universal occurred in Plato's Theory of Forms [Malcolm 1991]."
A check shows that Malcolm indeed discussed this notion ("the Form as an Ideal Individual"), but not under the name "concrete universal."
See Plato on the Self-Predication of Forms, by John Malcolm, Oxford U. Press, 1991.
From the publisher's summary:
"Malcolm…. shows that the middle dialogues do indeed take Forms to be both universals and paradigms…. He shows that Plato's concern to explain how the truths of mathematics can indeed be true played an important role in his postulation of the Form as an Ideal Individual."
Ellerman also cites another discussion of Plato published by Oxford:
For a literary context, see W. K. Wimsatt, Jr., "The Structure of the Concrete Universal," Ch. 6 in Literary Theory: An Anthology, edited by Julie Rivkin and Michael Ryan, Wiley-Blackwell, 2004.
Other uses of the phrase "concrete universal"– Hegelian and/or theological– seem rather distant from the concerns of Plato and Wimsatt, and are best left to debates between Marxists and Catholics. (My own sympathies are with the Catholics.)
Two views of "the white itself" —
"So did God cause the big bang? Overcome by metaphysical lassitude, I finally reach over to my bookshelf for The Devil's Bible. Turning to Genesis I read: 'In the beginning there was nothing. And God said, 'Let there be light!' And there was still nothing, but now you could see it.'" -- Jim Holt, Big-Bang Theology, Slate's "High Concept" department
"The world was warm and white when I was born: Beyond the windowpane the world was white, A glaring whiteness in a leaded frame, Yet warm as in the hearth and heart of light." -- Delmore Schwartz
Note that the alchemical structure
at left, suited more to narrative
than to mathematics, nevertheless
is mirrored within the pure
mathematics at right.
Related material
on Galois and geometry:
| Geometries of the group PSL(2, 11) by Francis Buekenhout, Philippe Cara, and Koen Vanmeerbeek. Geom. Dedicata, 83 (1-3): 169–206, 2000–
|
"I know what
nothing means."
— Joan Didion,
Play It As It Lays
Faust
President Faust of Harvard on Joan Didion:
"She was referring to life as a kind of improvisation: that magical crossroads of rigor and ease, structure and freedom, reason and intuition. What she calls being prepared to 'go with the change.'"
"I think about swimming with him into the cave at Portuguese Bend, about the swell of clear water, the way it changed, the swiftness and power it gained as it narrowed through the rocks at the base of the point. The tide had to be just right. We had to be in the water at the very moment the tide was right. We could only have done this a half dozen times at most during the two years we lived there but it is what I remember. Each time we did it I was afraid of missing the swell, hanging back, timing it wrong. John never was. You had to feel the swell change. You had to go with the change. He told me that. No eye is on the sparrow but he did tell me that."
From the same book:
"The craziness is receding but no clarity is taking its place."
— Joan Didion, The Year of Magical Thinking
For a magical crossroads at another university, see the five Log24 entries ending on November 25, 2005:
This holy icon
appeared at
N37°25.638'
W122°09.574'
on August 22, 2003,
at the Stanford campus.
Also from that date,
an example of clarity
in another holy icon —
|
|
— in honor of better days
at Harvard and of a member
of the Radcliffe Class of 1964.
Quoted here
a year ago today:
“… she explores
the nature of identity
in a structure of
crystalline complexity.”
— Janet Burroway
(See ART WARS.)
Related material:
Amy Adams in Doubt
Amy Adams and Meryl Streep
at premiere of Doubt
Above:
Craft, 1999
“The matron had given her
leave to go out as soon as
the women’s tea was over….”
— James Joyce, “Clay”
 |
Die (Tony Smith) |
|
|
Paul Moore, Jr., retired Episcopal Bishop of New York, who died at home at 83 on the First of May, 2003 |
From “Secondary Structures,” by Tom Moody, Sculpture Magazine, June 2000:
“By the early ’90s, the perception of Minimalism as a ‘pure’ art untouched by history lay in tatters. The coup de grâce against the movement came not from an artwork, however, but from a text. Shortly after the removal of Richard Serra’s Tilted Arc from New York City’s Federal Plaza, Harvard art historian Anna Chave published ‘Minimalism and the Rhetoric of Power’ (Arts Magazine, January 1990), a rousing attack on the boys’ club that stops just short of a full-blown ad hominem rant. Analyzing artworks (Walter de Maria’s aluminum swastika, Morris’s ‘carceral images,’ Flavin’s phallic ‘hot rods’), critical vocabulary (Morris’s use of ‘intimacy’ as a negative, Judd’s incantatory use of the word ‘powerful’), even titles (Frank Stella’s National Socialist-tinged Arbeit Macht Frei and Reichstag), Chave highlights the disturbing undercurrents of hypermasculinity and social control beneath Minimalism’s bland exterior. Seeing it through the eyes of the ordinary viewer, she concludes that ‘what [most] disturbs [the public at large] about Minimalist art may be what disturbs them about their own lives and times, as the face it projects is society’s blankest, steeliest face; the impersonal face of technology, industry and commerce; the unyielding face of the father: a face that is usually far more attractively masked.'”
For a more attractively masked father figure, see the Terminator series:
For further religious background,
see “Jesus and the Terminator“
in Christianity Today.
"By far the most important structure in design theory is the Steiner system
— "Block Designs," 1995, by Andries E. Brouwer
"The Steiner system S(5, 8, 24) is a set S of 759 eight-element subsets ('octads') of a twenty-four-element set T such that any five-element subset of T is contained in exactly one of the 759 octads. Its automorphism group is the large Mathieu group M24."
— The Miracle Octad Generator (MOG) of R.T. Curtis (webpage)
"… in 1861 Mathieu… discovered five multiply transitive permutation groups…. In a little-known 1931 paper of Carmichael… they were first observed to be automorphism groups of exquisite finite geometries."
The 1931 paper of Carmichael is now available online from the publisher for $10.
"My work is motivated by a hope that there may be a way to recapture the ancient and medieval vision of both Beauty and purpose in a way which is relevant to our own century. I even dare to hope that the two ideas may be related, that Beauty is actually part of the meaning and purpose of life."
"The Reverend T. P. Kirkman knew in 1862 that there exists a group of degree 16 and order 322560 with a normal, elementary abelian, subgroup of order 16 [1, p. 108]. Frobenius identified this group in 1904 as a subgroup of the Mathieu group M24 [4, p. 570]…."
1. Biggs N.L., "T. P. Kirkman, Mathematician," Bulletin of the London Mathematical Society 13, 97–120 (1981).
4. Frobenius G., "Über die Charaktere der mehrfach transitiven Gruppen," Sitzungsber. Königl. Preuss. Akad. Wiss. zu Berlin, 558–571 (1904). Reprinted in Frobenius, Gesammelte Abhandlungen III (J.-P. Serre, editor), pp. 335–348. Springer, Berlin (1968).
Olli Pottonen, "Classification of Steiner Quadruple Systems" (Master's thesis, Helsinki, 2005)–
"The concept of group actions is very useful in the study of isomorphisms of combinatorial structures."
"Simplify, simplify."
— Thoreau
"Beauty is bound up
with symmetry."
— Weyl
Pottonen's thesis is
dated Nov. 16, 2005.
For some remarks on
images and theology,
see Log24 on that date.
Click on the above image
for some further details.
Getting All
the Meaning In
Webpage heading for the
2009 meeting of the
American Comparative
Literature Association:
The mysterious symbols on
the above map suggest the
following reflections:
From A Cure of the Mind: The Poetics of Wallace Stevens, by Theodore Sampson, published by Black Rose Books Ltd., 2000–
Page x:
"… if what he calls 'the spirit's alchemicana' (CP [Collected Poems] 471) addresses itself to the irrational element in poetry, to what extent is such an element dominant in his theory and practice of poetry, and therefore in what way is Stevens' intricate verbal music dependent on his irrational use of language– a 'pure rhetoric of a language without words?' (CP 374)?"
| From "'When Novelists Become Cubists:' The Prose Ideograms of Guy Davenport," by Andre Furlani:
Laurence Zachar argues that Davenport's writing is situated "aux frontieres intergeneriques" where manifold modes are brought into concord: "L'etonnant chez Davenport est la facon don't ce materiau qui parait l'incarnation meme du chaos– hermetique, enigmatique, obscur, avec son tropplein de references– se revele en fait etre construit, ordonne, structure. Plus l'on s'y plonge, et plus l'on distingue de cohesion dans le texte." 'What astonishes in Davenport is the way in which material that seems the very incarnation of chaos– hermetic, enigmatic, obscure, with its proliferation of allusions– in fact reveals itself to be constructed, organized, structured. The more one immerses oneself in them the more one discerns the texts' cohesion.' (62). Davenport also works along the intergeneric border between text and graphic, for he illustrates many of his texts. (1) "The prime use of words is for imagery: my writing is drawing," he states in an interview (Hoeppfner 123). Visual imagery is not subordinated to writing in Davenport, who draws on the assemblage practice of superimposing image and writing. "I trust the image; my business is to get it onto the page," he writes in the essay "Ernst Machs Max Ernst." "A page, which I think of as a picture, is essentially a texture of images. […] The text of a story is therefore a continuous graph, kin to the imagist poem, to a collage (Ernst, Willi Baumeister, El Lissitzky), a page of Pound, a Brakhage film" (Geography 374-75). Note: (1.) Davenport is an illustrator of books (such as Hugh Kenner's The Stoic Comedians and The Counterfeiters) and journals (such as The Kenyon Review, Parnassus, and Paideuma). His art is the subject of Erik Anderson Reece's monograph, A Balance of Quinces, which reveals the inseparable relationship between Davenport's literary and pictorial work. References: Davenport, Guy. The Geography of the Imagination. San Francisco: North Point Press, 1981. Rpt. New York: Pantheon, 1992. Hoepffner, Bernard. "Pleasant Hill: An Interview with Guy Davenport." Conjunctions 24 (1995): 118-24. Reece, Erik Anderson. A Balance of Quinces: The Paintings and Drawings of Guy Davenport. New York: New Directions, 1996. Zachar, Laurence. "Guy Davenport: Une Mosaique du genres." Recherches Anglaises et Nord-Americaines 21 (1994): 51-63. |
"… when novelists become Cubists; that is, when they see the possibilities of making a hieroglyph, a coherent symbol, an ideogram of the total work. A symbol comes into being when an artist sees that it is the only way to get all the meaning in."
— Guy Davenport, The Geography of the Imagination
Counters in Rows
"Music, mathematics, and chess are in vital respects dynamic acts of location. Symbolic counters are arranged in significant rows. Solutions, be they of a discord, of an algebraic equation, or of a positional impasse, are achieved by a regrouping, by a sequential reordering of individual units and unit-clusters (notes, integers, rooks or pawns)."
— George Steiner
(See March 10, "Language Game.")
Yesterday’s entry Deep Structures discussed the “semiotic square,” a device that exemplifies the saying “If you can’t dazzle ’em with brilliance, then baffle ’em with bullshit.”
A search today for what the Marxist critic Fredric Jameson might have meant by saying that the square “is capable of generating at least ten conceivable positions out of a rudimentary binary opposition” leads to two documents of interest.
1. “Theory Pictures as Trails: Diagrams and the Navigation of Theoretical Narratives” (pdf), by J.R. Osborn, Department of Communication, University of California, San Diego (Cognitive Science Online, Vol.3.2, pp.15-44, 2005)
2. “The Semiotic Square” (html), by Louis Hébert (2006), professor, Université du Québec à Rimouski, in Signo (http://www.signosemio.com).
Shown below is Osborn’s picture of the semiotic square:
On the brighter side, we have, as a sign that Gallic clarity still exists, the work of Hébert.
Here is how he approaches Jameson’s oft-quoted, but seemingly confused, remark about “ten conceivable positions”–
“The Semiotic Square,”
|
|||||||||||||||||||||||||||||
|
5. (=1+2) COMPLEX TERM
|
||||||||||||||
|
||||||||||||||
|
7. (=1+3) POSITIVE DEIXIS |
8. (=2+4) NEGATIVE DEIXIS
|
|||||||||||||
|
6. (=3+4) NEUTRAL TERM
|
||||||||||||||
LEGEND:
The + sign links the terms that are combined to make up a metaterm (a compound term); for example, 5 is the result of combining 1 and 2.
The semiotic square entails primarily the following elements (we are steering clear of the constituent relationships of the square: contrariety, contradiction, and complementarity or implication):
1. terms
2. metaterms (compound terms)
3. object(s) (classified on the square)
4. observing subject(s) (who do the classifying)
5. time (of the observation)
The semiotic square is composed of four terms:
Position 1 (term A)
Position 2 (term B)
Position 3 (term not-B)
Position 4 (term not-A)
The first two terms form the opposition (the contrary relationship) that is the basis of the square, and the other two are obtained by negating each term of the opposition.
The semiotic square includes six metaterms. The metaterms are terms created from the four simple terms. Some of the metaterms have been named. (The complex term and the neutral term, despite their names, are indeed metaterms).
Position 5 (term 1 + term 2): complex term
Position 6 (term 3 + term 4): neutral term
Position 7 (term 1 + term 3): positive deixis
Position 8 (term 2 + term 4): negative deixis
Position 9 = term 1 + term 4: unnamed
Position 10 = term 2 + term 3: unnamed
These ten “positions” are apparently meant to explain Jameson’s remark.
Hébert’s treatment has considerably greater entertainment value than Osborn’s. Besides “the living dead” and angels, Hébert’s examples and exercises include vampires, transvestites, the Passion of Christ, and the following very relevant quotation:
“Simply let your ‘Yes’ be ‘Yes,’ and your ‘No,’ ‘No’; anything beyond this comes from the evil one.” (Matthew 5:37)
The Square of Oppositon
at Stanford Encylopedia of Philosophy
The Square of Opposition
in its original form
"The diagram above is from a ninth century manuscript of Apuleius' commentary on Aristotle's Perihermaneias, probably one of the oldest surviving pictures of the square."
— Edward Buckner at The Logic Museum
From the webpage "Semiotics for Beginners: Paradigmatic Analysis," by Daniel Chandler:
The Semiotic Square
"The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully (Greimas 1987,* xiv, 49). The semiotic square is intended to map the logical conjunctions and disjunctions relating key semantic features in a text. Fredric Jameson notes that 'the entire mechanism… is capable of generating at least ten conceivable positions out of a rudimentary binary opposition' (in Greimas 1987,* xiv). Whilst this suggests that the possibilities for signification in a semiotic system are richer than the either/or of binary logic, but that [sic] they are nevertheless subject to 'semiotic constraints' – 'deep structures' providing basic axes of signification."
* Greimas, Algirdas (1987): On Meaning: Selected Writings in Semiotic Theory (trans. Paul J Perron & Frank H Collins). London: Frances Pinter
Another version of the semiotic square:
Here is a more explicit figure representing the Klein group:
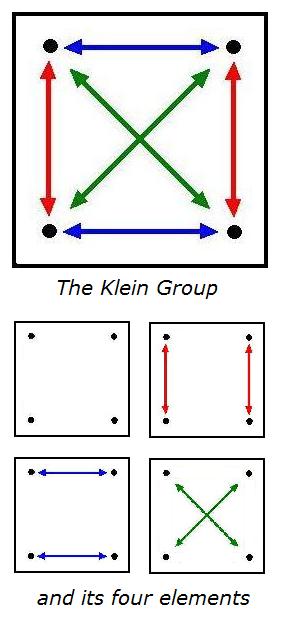
There is also the logical
diamond of opposition —
A semiotic (as opposed to logical)
diamond has been used to illustrate
remarks by Fredric Jameson,
a Marxist literary theorist:
|
"Introduction to Algirdas Greimas, Module on the Semiotic Square," by Dino Felluga at Purdue University–
The semiotic square has proven to be an influential concept not only in narrative theory but in the ideological criticism of Fredric Jameson, who uses the square as "a virtual map of conceptual closure, or better still, of the closure of ideology itself" ("Foreword"* xv). (For more on Jameson, see the [Purdue University] Jameson module on ideology.) Greimas' schema is useful since it illustrates the full complexity of any given semantic term (seme). Greimas points out that any given seme entails its opposite or "contrary." "Life" (s1) for example is understood in relation to its contrary, "death" (s2). Rather than rest at this simple binary opposition (S), however, Greimas points out that the opposition, "life" and "death," suggests what Greimas terms a contradictory pair (-S), i.e., "not-life" (-s1) and "not-death" (-s2). We would therefore be left with the following semiotic square (Fig. 1): 
As Jameson explains in the Foreword to Greimas' On Meaning, "-s1 and -s2"—which in this example are taken up by "not-death" and "not-life"—"are the simple negatives of the two dominant terms, but include far more than either: thus 'nonwhite' includes more than 'black,' 'nonmale' more than 'female'" (xiv); in our example, not-life would include more than merely death and not-death more than life.
* Jameson, Fredric. "Foreword." On Meaning: Selected Writings in Semiotic Theory. By Algirdas Greimas. Trans. Paul J. Perron and Frank H. Collins. Minneapolis: U of Minnesota P, 1976.
|
— The Gameplayers of Zan, by M.A. Foster
"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon,
Gravity's Rainbow
Crosses used by semioticians
to baffle their opponents
are illustrated above.
Some other kinds of crosses,
and another kind of opponent:
|
Monday, July 11, 2005
Logos
for St. Benedict's Day Click on either of the logos below for religious meditations– on the left, a Jewish meditation from the Conference of Catholic Bishops; on the right, an Aryan meditation from Stormfront.org.
Both logos represent different embodiments of the "story theory" of truth, as opposed to the "diamond theory" of truth. Both logos claim, in their own ways, to represent the eternal Logos of the Christian religion. I personally prefer the "diamond theory" of truth, represented by the logo below.
See also the previous entry Sunday, July 10, 2005
Mathematics
and Narrative Click on the title for a narrative about
Nikolaos K. Artemiadis,
"First of all, I'd like to
— Remark attributed to Plato
|
||||||||
Solomon's Cube
continued
"There is a book… called A Fellow of Trinity, one of series dealing with what is supposed to be Cambridge college life…. There are two heroes, a primary hero called Flowers, who is almost wholly good, and a secondary hero, a much weaker vessel, called Brown. Flowers and Brown find many dangers in university life, but the worst is a gambling saloon in Chesterton run by the Misses Bellenden, two fascinating but extremely wicked young ladies. Flowers survives all these troubles, is Second Wrangler and Senior Classic, and succeeds automatically to a Fellowship (as I suppose he would have done then). Brown succumbs, ruins his parents, takes to drink, is saved from delirium tremens during a thunderstorm only by the prayers of the Junior Dean, has much difficulty in obtaining even an Ordinary Degree, and ultimately becomes a missionary. The friendship is not shattered by these unhappy events, and Flowers's thoughts stray to Brown, with affectionate pity, as he drinks port and eats walnuts for the first time in Senior Combination Room."
— G. H. Hardy, A Mathematician's Apology
"The Solomon Key is the working title of an unreleased novel in progress by American author Dan Brown. The Solomon Key will be the third book involving the character of the Harvard professor Robert Langdon, of which the first two were Angels & Demons (2000) and The Da Vinci Code (2003)." — Wikipedia
"One has O+(6) ≅ S8, the symmetric group of order 8! …."
— "Siegel Modular Forms and Finite Symplectic Groups," by Francesco Dalla Piazza and Bert van Geemen, May 5, 2008, preprint.
"The complete projective group of collineations and dualities of the [projective] 3-space is shown to be of order [in modern notation] 8! …. To every transformation of the 3-space there corresponds a transformation of the [projective] 5-space. In the 5-space, there are determined 8 sets of 7 points each, 'heptads' …."
— George M. Conwell, "The 3-space PG(3, 2) and Its Group," The Annals of Mathematics, Second Series, Vol. 11, No. 2 (Jan., 1910), pp. 60-76
"It must be remarked that these 8 heptads are the key to an elegant proof…."
— Philippe Cara, "RWPRI Geometries for the Alternating Group A8," in Finite Geometries: Proceedings of the Fourth Isle of Thorns Conference (July 16-21, 2000), Kluwer Academic Publishers, 2001, ed. Aart Blokhuis, James W. P. Hirschfeld, Dieter Jungnickel, and Joseph A. Thas, pp. 61-97
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."
|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer… A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. |
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)
over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Cara:
Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Aesthetic satisfaction comes from an apprehension of how those themes and transformations relate to each other, or of what Brock calls their ‘relative complexity.’ Basically– and this is the nub of it– ‘if the theme is simple, then we are most satisfied when its echoes are complex… and vice versa.'”
Related material:
See also earlier tributes to
Hollywood Game Theory
and Hollywood Religion:
For some variations on the
above checkerboard theme, see
Finite Relativity and
A Wealth of Algebraic Structure.
|
The following meditation was inspired by the recent fictional recovery, by Mira Sorvino in "The Last Templar," of a Greek Cross — "the Cross of Constantine"– and by the discovery, by art historian Rosalind Krauss, of a Greek Cross in the art of Ad Reinhardt. |
|
Note that in applications, the vertical axis of the Cross of Descartes often symbolizes the timeless (money, temperature, etc.) while the horizontal axis often symbolizes time. T.S. Eliot:
"Men’s curiosity searches past and future |
|
"In suggesting that the success [1] of the grid
[1] Success here refers to
three things at once: a sheerly quantitative success, involving the number of artists in this century who have used grids; a qualitative success through which the grid has become the medium for some of the greatest works of modernism; and an ideological success, in that the grid is able– in a work of whatever quality– to emblematize the Modern." — Rosalind Krauss, "Grids" (1979) |
Related material:
Time Fold and Weyl on
objectivity and frames of reference.
See also Stambaugh on
The Formless Self
as well as
A Study in Art Education
and
Jung and the Imago Dei.
"I know what 'nothing' means…."
— Joan Didion, Play It As It Lays, Farrar, Straus and Giroux, 1990 paperback, page 214
"In 1935, near the end of a long affectionate letter to his son George in America, James Joyce wrote: 'Here I conclude. My eyes are tired. For over half a century they have gazed into nullity, where they have found a lovely nothing.'"
— Lionel Trilling, "James Joyce in His Letters," Commentary, 45, no. 2 (Feb. 1968), abstract
"The quotation is from The Letters of James Joyce, Volume III, ed. Richard Ellman (New York, 1966), p. 359. The original Italian reads 'Adesso termino. Ho gli occhi stanchi. Da più di mezzo secolo scrutano nel nulla dove hanno trovato un bellissimo niente.'"
— Lionel Trilling: Criticism and Politics, by William M. Chace, Stanford U. Press, 1980, page 198, Note 4 to Chapter 9
"Space: what you damn well have to see."
— James Joyce, Ulysses
"What happens to the concepts of space and direction if all the matter in the universe is removed save a small finite number of particles?"
— "On the Origins of Twistor Theory," by Roger Penrose
"… we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything…"
— Against the Day, by Thomas Pynchon (See previous entry.)
"A strange thing then happened."
Against the Day
is a novel by Thomas Pynchon
published on Nov. 21, 2006, in
hardcover, and in paperback on
Oct. 30, 2007 (Devil's Night).
Perhaps the day the title
refers to is one of the above
dates… or perhaps it is–
|
The Candlebrow Conference in Pynchon's Against the Day: The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries. "Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–"  Quaternion by S. H. Cullinane "Um, Professor–"…. … Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"…. … In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions…. "Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions." "Build a time machine." "Not the way I would have put it, but if you like, fine." Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up…. "We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly." "Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened. Above, the devastation had begun. |
The current (Feb. 2009) Notices of the American Mathematical Society has a written version of Freeman Dyson's 2008 Einstein Lecture, which was to have been given in October but had to be canceled. Dyson paraphrases a mathematician on Carl Jung's theory of archetypes:
"… we do not need to accept Jung’s theory as true in order to find it illuminating."
The same is true of Jung's remarks on synchronicity.
For example —
Yesterday's entry, "A Wealth of Algebraic Structure," lists two articles– each, as it happens, related to Jung's four-diamond figure from Aion as well as to my own Notes on Finite Geometry. The articles were placed online recently by Cambridge University Press on the following dates:
R. T. Curtis's 1974 article defining his Miracle Octad Generator (MOG) was published online on Oct. 24, 2008.
Curtis's 1987 article on geometry and algebraic structure in the MOG was published online on Dec. 19, 2008.
On these dates, the entries in this journal discussed…
Oct. 24:
Cube Space, 1984-2003
Material related to that entry:
Dec. 19:
Art and Religion: Inside the White Cube
That entry discusses a book by Mark C. Taylor:
The Picture in Question: Mark Tansey and the Ends of Representation (U. of Chicago Press, 1999).
"What, then, is a frame, and what is frame work?"
One possible answer —
Hermann Weyl on the relativity problem in the context of the 4×4 "frame of reference" found in the above Cambridge University Press articles.
A Wealth of
Algebraic Structure
A 1987 article by R. T. Curtis on the geometry of his Miracle Octad Generator (MOG) as it relates to the geometry of the 4×4 square is now available online ($20):
Further elementary techniques using the miracle octad generator, by R. T. Curtis. Abstract:
“In this paper we describe various techniques, some of which are already used by devotees of the art, which relate certain maximal subgroups of the Mathieu group M24, as seen in the MOG, to matrix groups over finite fields. We hope to bring out the wealth of algebraic structure* underlying the device and to enable the reader to move freely between these matrices and permutations. Perhaps the MOG was mis-named as simply an ‘octad generator’; in this paper we intend to show that it is in reality a natural diagram of the binary Golay code.”
(Received July 20 1987)
— Proceedings of the Edinburgh Mathematical Society (Series 2) (1989), 32: 345-353, doi:10.1017/S0013091500004600.
(Published online by Cambridge University Press 19 Dec 2008.)
In the above article, Curtis explains how two-thirds of his 4×6 MOG array may be viewed as the 4×4 model of the four-dimensional affine space over GF(2). (His earlier 1974 paper (below) defining the MOG discussed the 4×4 structure in a purely combinatorial, not geometric, way.)
For further details, see The Miracle Octad Generator as well as Geometry of the 4×4 Square and Curtis’s original 1974 article, which is now also available online ($20):
A new combinatorial approach to M24, by R. T. Curtis. Abstract:
“In this paper, we define M24 from scratch as the subgroup of S24 preserving a Steiner system S(5, 8, 24). The Steiner system is produced and proved to be unique and the group emerges naturally with many of its properties apparent.”
(Received June 15 1974)
— Mathematical Proceedings of the Cambridge Philosophical Society (1976), 79: 25-42, doi:10.1017/S0305004100052075.
(Published online by Cambridge University Press 24 Oct 2008.)
* For instance:
Click for details.
This pair of pictures was suggested by yesterday’s entry at Ars Mathematica containing the phrase “a dramatic extension of the notion of points.”
For other uses of the phrase “interpretive grid,” see today’s previous entry.
Part I: The White Cube
Part II: Inside
Part III: Outside
For remarks on religion
related to the above, see
Log24 on the Garden of Eden
and also Mark C. Taylor,
"What Derrida Really Meant"
(New York Times, Oct. 14, 2004).
For some background on Taylor,
see Wikipedia. Taylor, Chairman
of the Department of Religion at
Columbia University, has a
1973 doctorate in religion from
Harvard University. His opinion
of Derrida indicates that his
sympathies lie more with
the serpent than with the angel
in the Tansey picture above.
For some remarks by Taylor on
the art of Tansey relevant to the
structure of the white cube
(Part I above), see Taylor's
The Picture in Question:
Mark Tansey and the
Ends of Representation
(U. of Chicago Press, 1999):
|
From Chapter 3,
"Sutures* of Structures," p. 58: "What, then, is a frame, and what is frame work? This question is deceptive in its simplicity. A frame is, of course, 'a basic skeletal structure designed to give shape or support' (American Heritage Dictionary)…. when the frame is in question, it is difficult to determine what is inside and what is outside. Rather than being on one side or the other, the frame is neither inside nor outside. Where, then, Derrida queries, 'does the frame take place….'" * P. 61:
|
From The n-Category Cafe today:
David Corfield at 2:33 PM UTC quoting a chapter from a projected second volume of a biography:
"Grothendieck’s spontaneous reaction to whatever appeared to be causing a difficulty… was to adopt and embrace the very phenomenon that was problematic, weaving it in as an integral feature of the structure he was studying, and thus transforming it from a difficulty into a clarifying feature of the situation."
John Baez at 7:14 PM UTC on research:
"I just don’t want to reinvent a wheel, or waste my time inventing a square one."
For the adoption and embracing of such a problematic phenomenon, see The Square Wheel (this journal, Sept. 14, 2004).
For a connection of the square wheel with yesterday's entry for Julie Taymor's birthday, see a note from 2002:
Thoughts suggested by Saturday's entry–
"… with primitives the beginnings of art, science, and religion coalesce in the undifferentiated chaos of the magical mentality…."
— Carl G. Jung, "On the Relation of Analytical Psychology to Poetry," Collected Works, Vol. 15, The Spirit in Man, Art, and Literature, Princeton University Press, 1966, excerpted in Twentieth Century Theories of Art, edited by James M. Thompson.
For a video of such undifferentiated chaos, see the Four Tops' "Loco in Acapulco."
"Yes, you'll be goin' loco
down in Acapulco,
the magic down there
is so strong."
This song is from the 1988 film "Buster."
(For a related religious use of that name– "Look, Buster, do you want to live?"– see Fritz Leiber's "Damnation Morning," quoted here on Sept. 28.)
Art, science, and religion are not apparent within the undifferentiated chaos of the Four Tops' Acapulco video, which appears to incorporate time travel in its cross-cutting of scenes that seem to be from the Mexican revolution with contemporary pool-party scenes. Art, science, and religion do, however, appear within my own memories of Acapulco. While staying at a small thatched-roof hostel on a beach at Acapulco in the early 1960's, I read a paperback edition of Three Philosophical Poets, a book by George Santayana on Lucretius, Dante, and Goethe. Here we may regard art as represented by Goethe, science by Lucretius, and religion by Dante. For a more recent and personal combination of these topics, see Juneteenth through Midsummer Night, 2007, which also has references to the "primitives" and "magical mentality" discussed by Jung.
"The major structures of the psyche for Jung include the ego, which is comprised of the persona and the shadow. The persona is the 'mask' which the person presents [to] the world, while the shadow holds the parts of the self which the person feels ashamed and guilty about."
— Brent Dean Robbins, Jung page at Mythos & Logos
As for shame and guilt, see Malcolm Lowry's classic Under the Volcano, a novel dealing not with Acapulco but with a part of Mexico where in my youth I spent much more time– Cuernavaca.
Lest Lowry's reflections prove too depressing, I recommend as background music the jazz piano of the late Dave McKenna… in particular, "Me and My Shadow."
McKenna died on Saturday, the date of the entry that included "Loco in Acapulco." Saturday was also the Feast of Saint Luke.
Related material:
Dec. 16, 2003—
|
Kaleidoscope turning… |
|
"Credences of Summer," VII,
by Wallace Stevens, from
"Three times the concentred |
One possibility —
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in a recent New Yorker:
"A word about E(8). In my opinion, and shared by others, E(8) is the most magnificent 'object' in all of mathematics. It is like a diamond with thousands of facets. Each facet offering a different view of its unbelievable intricate internal structure."
Another possibility —
A more modest object —
the 4×4 square.
Update of Aug. 20-21 —
Kostant's poetic comparison might be applied also to this object.
More precisely, there are 322,560 natural rearrangements– which a poet might call facets*— of the array, each offering a different view of the array's internal structure– encoded as a unique ordered pair of symmetric graphic designs. The symmetry of the array's internal structure is reflected in the symmetry of the graphic designs. For examples, see the Diamond 16 Puzzle.
For an instance of Stevens's "three times" process, see the three parts of the 2004 web page Ideas and Art.
* For the metaphor of rearrangements as facets, note that each symmetry (rearrangement) of a Platonic solid corresponds to a rotated facet: the number of symmetries equals the number of facets times the number of rotations (edges) of each facet–
If Greek geometers had started with a finite space (as in The Eightfold Cube), the history of mathematics might have dramatically illustrated Halmos's saying (Aug. 16) that
"The problem is– the genius is– given an infinite question, to think of the right finite question to ask. Once you thought of the finite answer, then you would know the right answer to the infinite question."
The Greeks, of course, answered the infinite questions first– at least for Euclidean space. Halmos was concerned with more general modern infinite spaces (such as Hilbert space) where the intuition to be gained from finite questions is still of value.
Seeing the Finite Structure
The following supplies some context for remarks of Halmos on combinatorics.
From Paul Halmos: Celebrating 50 years of Mathematics, by John H. Ewing, Paul Richard Halmos, Frederick W. Gehring, published by Springer, 1991–
Interviews with Halmos, “Paul Halmos by Parts,” by Donald J. Albers–
“Part II: In Touch with God*“– on pp. 27-28:
The Root of All Deep Mathematics
“Albers. In the conclusion of ‘Fifty Years of Linear Algebra,’ you wrote: ‘I am inclined to believe that at the root of all deep mathematics there is a combinatorial insight… I think that in this subject (in every subject?) the really original, really deep insights are always combinatorial, and I think for the new discoveries that we need– the pendulum needs– to swing back, and will swing back in the combinatorial direction.’ I always thought of you as an analyst.
Halmos: People call me an analyst, but I think I’m a born algebraist, and I mean the same thing, analytic versus combinatorial-algebraic. I think the finite case illustrates and guides and simplifies the infinite.
Some people called me full of baloney when I asserted that the deep problems of operator theory could all be solved if we knew the answer to every finite dimensional matrix question. I still have this religion that if you knew the answer to every matrix question, somehow you could answer every operator question. But the ‘somehow’ would require genius. The problem is not, given an operator question, to ask the same question in finite dimensions– that’s silly. The problem is– the genius is– given an infinite question, to think of the right finite question to ask. Once you thought of the finite answer, then you would know the right answer to the infinite question.
Combinatorics, the finite case, is where the genuine, deep insight is. Generalizing, making it infinite, is sometimes intricate and sometimes difficult, and I might even be willing to say that it’s sometimes deep, but it is nowhere near as fundamental as seeing the finite structure.”
Whether the above sketch of the passage from operator theory to harmonic analysis to Walsh functions to finite geometry can ever help find “the right finite question to ask,” I do not know. It at least suggests that finite geometry (and my own work on models in finite geometry) may not be completely irrelevant to mathematics generally regarded as more deep.
* See the Log24 entries following Halmos’s death.
Preview of a Tom Stoppard play presented at Town Hall in Manhattan on March 14, 2008 (Pi Day and Einstein's birthday):
The play's title, "Every Good Boy Deserves Favour," is a mnemonic for the notes of the treble clef EGBDF.
The place, Town Hall, West 43rd Street. The time, 8 p.m., Friday, March 14. One single performance only, to the tinkle– or the clang?– of a triangle. Echoing perhaps the clang-clack of Warsaw Pact tanks muscling into Prague in August 1968.
The “u” in favour is the British way, the Stoppard way, "EGBDF" being "a Play for Actors and Orchestra" by Tom Stoppard (words) and André Previn (music).
And what a play!– as luminescent as always where Stoppard is concerned. The music component of the one-nighter at Town Hall– a showcase for the Boston University College of Fine Arts– is by a 47-piece live orchestra, the significant instrument being, well, a triangle.
When, in 1974, André Previn, then principal conductor of the London Symphony, invited Stoppard "to write something which had the need of a live full-time orchestra onstage," the 36-year-old playwright jumped at the chance.
One hitch: Stoppard at the time knew "very little about 'serious' music… My qualifications for writing about an orchestra," he says in his introduction to the 1978 Grove Press edition of "EGBDF," "amounted to a spell as a triangle player in a kindergarten percussion band."
Review of the same play as presented at Chautauqua Institution on July 24, 2008:
"Stoppard's modus operandi– to teasingly introduce numerous clever tidbits designed to challenge the audience."
— Jane Vranish, Pittsburgh Post-Gazette, Saturday, August 2, 2008
"The leader of the band is tired
And his eyes are growing old
But his blood runs through
My instrument
And his song is in my soul."
— Dan Fogelberg
"He's watching us all the time."
|
Finnegans Wake, Book II, Episode 2, pp. 296-297:
I'll make you to see figuratleavely the whome of your eternal geomater. And if you flung her headdress on her from under her highlows you'd wheeze whyse Salmonson set his seel on a hexengown.1 Hissss!, Arrah, go on! Fin for fun! 1 The chape of Doña Speranza of the Nacion. |
|
Reciprocity From my entry of Sept. 1, 2003:
"…the principle of taking and giving, of learning and teaching, of listening and storytelling, in a word: of reciprocity…. … E. M. Forster famously advised his readers, 'Only connect.' 'Reciprocity' would be Michael Kruger's succinct philosophy, with all that the word implies." — William Boyd, review of Himmelfarb, a novel by Michael Kruger, in The New York Times Book Review, October 30, 1994 Last year's entry on this date:
The picture above is of the complete graph K6 … Six points with an edge connecting every pair of points… Fifteen edges in all. Diamond theory describes how the 15 two-element subsets of a six-element set (represented by edges in the picture above) may be arranged as 15 of the 16 parts of a 4×4 array, and how such an array relates to group-theoretic concepts, including Sylvester's synthematic totals as they relate to constructions of the Mathieu group M24. If diamond theory illustrates any general philosophical principle, it is probably the interplay of opposites…. "Reciprocity" in the sense of Lao Tzu. See Reciprocity and Reversal in Lao Tzu. For a sense of "reciprocity" more closely related to Michael Kruger's alleged philosophy, see the Confucian concept of Shu (Analects 15:23 or 24) described in Kruger's novel is in part about a Jew: the quintessential Jewish symbol, the star of David, embedded in the K6 graph above, expresses the reciprocity of male and female, as my May 2003 archives illustrate. The star of David also appears as part of a graphic design for cubes that illustrate the concepts of diamond theory:
Click on the design for details. Those who prefer a Jewish approach to physics can find the star of David, in the form of K6, applied to the sixteen 4×4 Dirac matrices, in
A Graphical Representation The star of David also appears, if only as a heuristic arrangement, in a note that shows generating partitions of the affine group on 64 points arranged in two opposing triplets.
Having thus, as the New York Times advises, paid tribute to a Jewish symbol, we may note, in closing, a much more sophisticated and subtle concept of reciprocity due to Euler, Legendre, and Gauss. See |
"Finn MacCool ate the Salmon of Knowledge."
Wikipedia:
"George Salmon spent his boyhood in Cork City, Ireland. His father was a linen merchant. He graduated from Trinity College Dublin at the age of 19 with exceptionally high honours in mathematics. In 1841 at age 21 he was appointed to a position in the mathematics department at Trinity College Dublin. In 1845 he was appointed concurrently to a position in the theology department at Trinity College Dublin, having been confirmed in that year as an Anglican priest."
Related material:
Arrangements for
56 Triangles.
For more on the
arrangement of
triangles discussed
in Finnegans Wake,
see Log24 on Pi Day,
March 14, 2008.
Happy birthday,
Martin Sheen.
Simon tells me he has a quasi-religious faith in the Monster. One day, he says, … the Monster will expose the structure of the universe.
… although Simon says he is keen for me to write a book about him and his work on the Monster and his obsession with buses, he doesn’t like talking, has no sense of anecdotes or extended conversation, and can’t remember (or never paid any attention to) 90 per cent of the things I want him to tell me about in his past. It is not modesty. Simon is not modest or immodest: he just has no self-curiosity. To Simon, Simon is a collection of disparate facts and no interpretative glue. He is a man without adjectives. His speech is made up almost entirely of short bursts of grunts and nouns.
This is the main reason why we spent three weeks together …. I needed to find a way to make him prattle.”
Those in search of prattle and interpretive glue should consult Anthony Judge’s essay ““Potential Psychosocial Significance of Monstrous Moonshine: An Exceptional Form of Symmetry as a Rosetta Stone for Cognitive Frameworks.” This was cited here in Thursday’s entry “Symmetry in Review.” (That entry is just a list of items related in part by synchronicity, in part by mathematical content. The list, while meaningful to me and perhaps a few others, is also lacking in prattle and interpretive glue.)
Those in search of knowledge, rather than glue and prattle, should consult Symmetry and the Monster, by Mark Ronan. If they have a good undergraduate education in mathematics, Terry Gannon‘s survey paper “Monstrous Moonshine: The First Twenty-Five Years” (pdf) and book– Moonshine Beyond the Monster— may also be of interest.
“Put bluntly, who is kidding whom?”
— Anthony Judge, draft of
“Potential Psychosocial Significance
of Monstrous Moonshine:
An Exceptional Form of Symmetry
as a Rosetta Stone for
Cognitive Frameworks,”
dated September 6, 2007.
Good question.
Also from
September 6, 2007 —
the date of
Madeleine L’Engle‘s death —
 |
1. The performance of a work by
Richard Strauss,
“Death and Transfiguration,”
(Tod und Verklärung, Opus 24)
by the Chautauqua Symphony
at Chautauqua Institution on
July 24, 2008
2. Headline of a music review
in today’s New York Times:
Welcoming a Fresh Season of
Transformation and Death
3. The picture of the R. T. Curtis
Miracle Octad Generator
on the cover of the book
Twelve Sporadic Groups:
4. Freeman Dyson’s hope, quoted by
Gorenstein in 1986, Ronan in 2006,
and Judge in 2007, that the Monster
group is “built in some way into
the structure of the universe.”
5. Symmetry from Plato to
the Four-Color Conjecture
7. Yesterday’s entry,
“Theories of Everything“
Coda:
as a tesseract.“
— Madeleine L’Engle
For a profile of
L’Engle, click on
the Easter eggs.
| OF THE STANDARD MODEL WITH QUANTUM GRAVITY by Ashay Dharwadker Abstract
|
* See, for instance, “The Scientific Promise of Perfect Symmetry” in The New York Times of March 20, 2007.
Bertram Kostant, Professor Emeritus of Mathematics at MIT, on an object discussed in this week's New Yorker:
Hermann Weyl on the hard core of objectivity:
Steven H. Cullinane on the symmetries of a 4×4 array of points:
|
A Structure-Endowed Entity
"A guiding principle in modern mathematics is this lesson: Whenever you have to do with a structure-endowed entity S, try to determine its group of automorphisms, the group of those element-wise transformations which leave all structural relations undisturbed. You can expect to gain a deep insight into the constitution of S in this way." — Hermann Weyl in Symmetry Let us apply Weyl's lesson to the following "structure-endowed entity."
What is the order of the resulting group of automorphisms? |
The above group of
automorphisms plays
a role in what Weyl,
following Eddington,
called a "colorful tale"–
This puzzle shows
that the 4×4 array can
also be viewed in
thousands of ways.
"You can make 322,560
pairs of patterns. Each
pair pictures a different
symmetry of the underlying
16-point space."
— Steven H. Cullinane,
July 17, 2008
For other parts of the tale,
see Ashay Dharwadker,
the Four-Color Theorem,
and Usenet Postings.
Hard Core
David Corfield quotes Weyl in a weblog entry, "Hierarchy and Emergence," at the n-Category Cafe this morning:
"Perhaps the philosophically most relevant feature of modern science is the emergence of abstract symbolic structures as the hard core of objectivity behind– as Eddington puts it– the colorful tale of the subjective storyteller mind." (Philosophy of Mathematics and Natural Science [Princeton, 1949], p. 237)
For the same quotation in a combinatorial context, see the foreword by A. W. Tucker, "Combinatorial Problems," to a special issue of the IBM Journal of Research and Development, November 1960 (1-page pdf).
See also yesterday's Log24 entry.
|
The HSBC Logo Designer — Henry Steiner
Born in Austria and raised in New York, Steiner was educated at Yale under Paul Rand and attended the Sorbonne as a Fulbright Fellow. He is a past President of Alliance Graphique Internationale. Other professional affiliations include the American Institute of Graphic Arts, Chartered Society of Designers, Design Austria, and the New York Art Directors' Club. His Cross-Cultural Design: Communicating in the Global Marketplace was published by Thames and Hudson (1995). |
Charles Taylor,
"… the object sets up
See also Talking of Michelangelo.
|
Related material
from today —
Escape from a
cartoon graveyard:
Having survived that ominous date, I feel it is fitting to review what Wallace Stevens called "Credences of Summer"– religious principles for those who feel that faith and doubt are best reconciled by art.
|
"Credences of Summer," VII,
by Wallace Stevens, from
"Three times the concentred |
|
Definition of Epiphany
From James Joyce's Stephen Hero, first published posthumously in 1944. The excerpt below is from a version edited by John J. Slocum and Herbert Cahoon (New York: New Directions Press, 1959).
Three Times: … By an epiphany he meant a sudden spiritual manifestation, whether in the vulgarity of speech or of gesture or in a memorable phase of the mind itself. He believed that it was for the man of letters to record these epiphanies with extreme care, seeing that they themselves are the most delicate and evanescent of moments. He told Cranly that the clock of the Ballast Office was capable of an epiphany. Cranly questioned the inscrutable dial of the Ballast Office with his no less inscrutable countenance: — Yes, said Stephen. I will pass it time after time, allude to it, refer to it, catch a glimpse of it. It is only an item in the catalogue of Dublin's street furniture. Then all at once I see it and I know at once what it is: epiphany. — What? — Imagine my glimpses at that clock as the gropings of a spiritual eye which seeks to adjust its vision to an exact focus. The moment the focus is reached the object is epiphanised. It is just in this epiphany that I find the third, the supreme quality of beauty. — Yes? said Cranly absently. — No esthetic theory, pursued Stephen relentlessly, is of any value which investigates with the aid of the lantern of tradition. What we symbolise in black the Chinaman may symbolise in yellow: each has his own tradition. Greek beauty laughs at Coptic beauty and the American Indian derides them both. It is almost impossible to reconcile all tradition whereas it is by no means impossible to find the justification of every form of beauty which has ever been adored on the earth by an examination into the mechanism of esthetic apprehension whether it be dressed in red, white, yellow or black. We have no reason for thinking that the Chinaman has a different system of digestion from that which we have though our diets are quite dissimilar. The apprehensive faculty must be scrutinised in action. — Yes … — You know what Aquinas says: The three things requisite for beauty are, integrity, a wholeness, symmetry and radiance. Some day I will expand that sentence into a treatise. Consider the performance of your own mind when confronted with any object, hypothetically beautiful. Your mind to apprehend that object divides the entire universe into two parts, the object, and the void which is not the object. To apprehend it you must lift it away from everything else: and then you perceive that it is one integral thing, that is a thing. You recognise its integrity. Isn't that so? — And then? — That is the first quality of beauty: it is declared in a simple sudden synthesis of the faculty which apprehends. What then? Analysis then. The mind considers the object in whole and in part, in relation to itself and to other objects, examines the balance of its parts, contemplates the form of the object, traverses every cranny of the structure. So the mind receives the impression of the symmetry of the object. The mind recognises that the object is in the strict sense of the word, a thing, a definitely constituted entity. You see? — Let us turn back, said Cranly. They had reached the corner of Grafton St and as the footpath was overcrowded they turned back northwards. Cranly had an inclination to watch the antics of a drunkard who had been ejected from a bar in Suffolk St but Stephen took his arm summarily and led him away. — Now for the third quality. For a long time I couldn't make out what Aquinas meant. He uses a figurative word (a very unusual thing for him) but I have solved it. Claritas is quidditas. After the analysis which discovers the second quality the mind makes the only logically possible synthesis and discovers the third quality. This is the moment which I call epiphany. First we recognise that the object is one integral thing, then we recognise that it is an organised composite structure, a thing in fact: finally, when the relation of the parts is exquisite, when the parts are adjusted to the special point, we recognise that it is that thing which it is. Its soul, its whatness, leaps to us from the vestment of its appearance. The soul of the commonest object, the structure of which is so adjusted, seems to us radiant. The object achieves its epiphany. Having finished his argument Stephen walked on in silence. He felt Cranly's hostility and he accused himself of having cheapened the eternal images of beauty. For the first time, too, he felt slightly awkward in his friend's company and to restore a mood of flippant familiarity he glanced up at the clock of the Ballast Office and smiled: — It has not epiphanised yet, he said. |
|
Under the Volcano,
by Malcolm Lowry, "What have I got out of my life? Contacts with famous men… The occasion Einstein asked me the time, for instance. That summer evening…. smiles when I say I don't know. And yet asked me. Yes: the great Jew, who has upset the whole world's notions of time and space, once leaned down… to ask me… ragged freshman… at the first approach of the evening star, the time. And smiled again when I pointed out the clock neither of us had noticed."
An approach of
This figure is from a webpage,
As noted in yesterday's early- "The appearance of the evening star brings with it long-standing notions of safety within and danger without. In a letter to Harriet Monroe, written December 23, 1926, Stevens refers to the Sapphic fragment that invokes the genius of evening: 'Evening star that bringest back all that lightsome Dawn hath scattered afar, thou bringest the sheep, thou bringest the goat, thou bringest the child home to the mother.' Christmas, writes Stevens, 'is like Sappho's evening: it brings us all home to the fold' (Letters of Wallace Stevens, 248)."
— Barbara Fisher, |
John Baez, Week 266
(June 20, 2008):
"The Renaissance thinkers liked to
organize the four elements using
a chain of analogies running
from light to heavy:
fire : air :: air : water :: water : earth
They also organized them
in a diamond, like this:"
This figure of Baez
is related to a saying
attributed to Heraclitus:
For related thoughts by Jung,
see Aion, which contains the
following diagram:
"The formula reproduces exactly the essential features of the symbolic process of transformation. It shows the rotation of the mandala, the antithetical play of complementary (or compensatory) processes, then the apocatastasis, i.e., the restoration of an original state of wholeness, which the alchemists expressed through the symbol of the uroboros, and finally the formula repeats the ancient alchemical tetrameria, which is implicit in the fourfold structure of unity."
— Carl Gustav Jung
That the words Maximus of Tyre (second century A.D.) attributed to Heraclitus imply a cycle of the elements (analogous to the rotation in Jung's diagram) is not a new concept. For further details, see "The Rotation of the Elements," a 1995 webpage by one "John Opsopaus."
Related material:
Log24 entries of June 9, 2008, and
"Quintessence: A Glass Bead Game,"
by Charles Cameron.
Part I: Random Walk
Part II: X's
3/22:
Part III: O's —
A Cartoon Graveyard
in honor of the late
Gene Persson †
Today's Garfield —
See also
Midsummer Eve's Dream:
"The meeting is closed
with the lord's‡ prayer
and refreshments are served."
† Producer of plays and musicals
including Album and
The Ruling Class
‡ Lower case in honor of
Peter O'Toole, star of
the film version of
The Ruling Class.
(This film, together with
O'Toole's My Favorite Year,
may be regarded as epitomizing
Hollywood's Jesus for Jews.)
Those who prefer
less randomness
in their religion
may consult O'Toole's
more famous film work
involving Islam,
as well as
the following structure
discussed here on
the date of Persson's death:
"The Moslems thought of the
central 1 as being symbolic
of the unity of Allah."
for lifetime achievement
in arts and philosophy
this year goes to
Charles Taylor,
Montreal philosophy professor.
“The Kyoto Prize has been given in three domains since 1984:
advanced technology, basic sciences, and the arts and philosophy.
It is administered by the Inamori Foundation, whose president,
Kazuo Inamori, is founder and chairman emeritus of Kyocera and
KDDI Corporation, two Japanese telecommunications giants.”
 |
|
“The Kyocera brand symbol is composed of a corporate mark |
Related material —
Charles Taylor,
“Epiphanies of Modernism,”
Chapter 24 of Sources of the Self
(Cambridge U. Press, 1989, p. 477) —
“… the object sets up
a kind of frame or space or field
within which there can be epiphany.”
See also Talking of Michelangelo.
“The soul of the commonest object,
the structure of which is so adjusted,
seems to us radiant. The object
achieves its epiphany.”
Above: Screenshot of today’s
New York Times obituary for
mathematician Detlef Gromoll,
known for the “soul theorem.”
Gromoll died on May 31
according to his son
Hans Christian.
From his obituary:
“Detlef Gromoll was born in Berlin
in 1938, and his childhood
was disrupted by the falling
bombs of World War II.”
Related material:
The discussion here
on June 1 of a lottery number
from the date of Gromoll’s death,
childhood, mathematics,
and prewar Berlin.
Stevie Nicks
is 60 today.
On the author discussed
here yesterday,
Siri Hustvedt:
“… she explores
the nature of identity
in a structure* of
crystalline complexity.”
— Janet Burroway,
quoted in
ART WARS
“Is it safe?”
— Annals of Art Education:
Geometry and Death
* Related material:
the life and work of
Felix Christian Klein
and
Report to the Joint
Mathematics Meetings
Hermann Hesse's 1943 The Glass Bead Game (Picador paperback, Dec. 6, 2002, pp. 139-140)–
"For the present, the Master showed him a bulky memorandum, a proposal he had received from an organist– one of the innumerable proposals which the directorate of the Game regularly had to examine. Usually these were suggestions for the admission of new material to the Archives. One man, for example, had made a meticulous study of the history of the madrigal and discovered in the development of the style a curved that he had expressed both musically and mathematically, so that it could be included in the vocabulary of the Game. Another had examined the rhythmic structure of Julius Caesar's Latin and discovered the most striking congruences with the results of well-known studies of the intervals in Byzantine hymns. Or again some fanatic had once more unearthed some new cabala hidden in the musical notation of the fifteenth century. Then there were the tempestuous letters from abstruse experimenters who could arrive at the most astounding conclusions from, say, a comparison of the horoscopes of Goethe and Spinoza; such letters often included pretty and seemingly enlightening geometric drawings in several colors."
From Siri Hustvedt, author of Mysteries of the Rectangle: Essays on Painting (Princeton Architectural Press, 2005)– What I Loved: A Novel (Picador paperback, March 1, 2004, page 168)–
A description of the work of Bill Wechsler, a fictional artist:
"Bill worked long hours on a series of autonomous pieces about numbers. Like O's Journey, the works took place inside glass cubes, but these were twice as large– about two feet square. He drew his inspiration from sources as varied as the Cabbala, physics, baseball box scores, and stock market reports. He painted, cut, sculpted, distorted, and broke the numerical signs in each work until they became unrecognizable. He included figures, objects, books, windows, and always the written word for the number. It was rambunctious art, thick with allusion– to voids, blanks, holes, to monotheism and the individual, the the dialectic and yin-yang, to the Trinity, the three fates, and three wishes, to the golden rectangle, to seven heavens, the seven lower orders of the sephiroth, the nine Muses, the nine circles of Hell, the nine worlds of Norse mythology, but also to popular references like A Better Marriage in Five Easy Lessons and Thinner Thighs in Seven Days. Twelve-step programs were referred to in both cube one and cube two. A miniature copy of a book called The Six Mistakes Parents Make Most Often lay at the bottom of cube six. Puns appeared, usually well disguised– one, won; two, too, and Tuesday; four, for, forth; ate, eight. Bill was partial to rhymes as well, both in images and words. In cube nine, the geometric figure for a line had been painted on one glass wall. In cube three, a tiny man wearing the black-and-white prison garb of cartoons and dragging a leg iron has
— End of page 168 —
opened the door to his cell. The hidden rhyme is "free." Looking closely through the walls of the cube, one can see the parallel rhyme in another language: the German word drei is scratched into one glass wall. Lying at the bottom of the same box is a tiny black-and-white photograph cut from a book that shows the entrance to Auschwitz: ARBEIT MACHT FREI. With every number, the arbitrary dance of associations worked togethere to create a tiny mental landscape that ranged in tone from wish-fulfillment dream to nightmare. Although dense, the effect of the cubes wasn't visually disorienting. Each object, painting, drawing, bit of text, or sculpted figure found its rightful place under the glass according to the necessary, if mad, logic of numerical, pictorial, and verbal connection– and the colors of each were startling. Every number had been given a thematic hue. Bill had been interested in Goethe's color wheel and in Alfred Jensen's use of it in his thick, hallucinatory paintings of numbers. He had assigned each number a color. Like Goethe, he included black and white, although he didn't bother with the poet's meanings. Zero and one were white. Two was blue. Three was red, four was yellow, and he mixed colors: pale blue for five, purples in six, oranges in seven, greens in eight, and blacks and grays in nine. Although other colors and omnipresent newsprint always intruded on the basic scheme, the myriad shades of a single color dominated each cube.
The number pieces were the work of a man at the top of his form. An organic extension of everything Bill had done before, these knots of symbols had an explosive effect. The longer I looked at them, the more the miniature constructions seemed on the brink of bursting from internal pressure. They were tightly orchestrated semantic bombs through which Bill laid bare the arbitrary roots of meaning itself– that peculiar social contract generated by little squiggles, dashes, lines, and loops on a page."
|
From 2002:
Above: Dr. Harrison Pope, Harvard professor of psychiatry, demonstrates the use of the Wechsler Adult Intelligence Scale "block design" subtest. |
|
ZZ
Figures from the
Poem by Eugen Jost:
Mit Zeichen und Zahlen
Numbers and Names,
With numbers and names English translation A related poem:
Alphabets
From time to time
But if a savage
— Hermann Hesse (1943), |
From
“On the Holy Trinity,”
the entry in the 3:20 PM
French footprint:
“…while the scientist sees
everything that happens
in one point of space,
the poet feels
everything that happens
in one point of time…
all forming an
instantaneous and transparent
organism of events….”
From
“Angel in the Details,”
the entry in the 3:59 PM
French footprint:
“I dwell in Possibility –
A fairer House than Prose”
These, along with this afternoon’s
earlier entry, suggest a review
of a third Log24 item, Windmills,
with an actress from France as…
|
Changing Woman: “Kaleidoscope turning…
Shifting pattern |
“When life itself seems lunatic,
who knows where madness lies?”
— For the source, see
Joyce’s Nightmare Continues.
Look Homeward, Norman
The evening number,
411, may be interpreted
as 4/11. From Log24
on that date:
As for the mid-day number
098, a Google search
(with the aid of, in retrospect,
the above family tribute)
on "98 'Norman Mailer'"
yields
|
Amazon.com: The Time of Our Time (Modern Library Paperbacks …
With The Time of Our Time (1998) Norman Mailer has archetypalized himself and in the seven years since publication, within which films Fear and Loathing in …
|
"Surely this sense of himself
as the republic's recording angel
accounts for the structure
of Mailer's anthology…."
Related material:
From Play It As It Lays,
the paperback edition of 1990
(Farrar, Straus and Giroux) —
|
Page 170:
"… In her half sleep
By the end of a week she was thinking constantly
170
even one micro-second she would have what she had |
The number 411 from
this evening's New York Lottery
may thus be regarded as naming the
"exact point in space and time"
sought in the above passage.
For a related midrash
on the meaning of the
passage's page number,
see the previous entry.
For a more plausible
recording angel,
see Sinatra's birthday,
December 12, 2002.
Thomas Wolfe
(Harvard M.A., 1922)
versus
Rosalind Krauss
(Harvard M.A., 1964,
Ph.D., 1969)
on
"No culture has a pact with eternity."
— George Steiner, interview in
The Guardian of
"At that instant he saw,
in one blaze of light, an image
of unutterable conviction….
the core of life, the essential
pattern whence all other things
proceed, the kernel of eternity."
— Thomas Wolfe, Of Time
and the River, quoted in
Log24 on June 9, 2005
From today's online Harvard Crimson:
"… under the leadership of Faust,
Harvard students should look forward
to an ever-growing opportunity for
international experience
and artistic endeavor."
Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen
From a recent book
on Wolfgang Pauli,
The Innermost Kernel:
A belated happy birthday
to the late
Felix Christian Klein
(born on April 25) —
Another Harvard figure quoted here on Dec. 5, 2002:
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less."
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951)
From a review of Rosalind Krauss's The Optical Unconscious (MIT Press hardcover, 1993):
Krauss is concerned to present Modernism less in terms of its history than its structure, which she seeks to represent by means of a kind of diagram: "It is more interesting to think of modernism as a graph or table than a history." The "table" is a square with diagonally connected corners, of the kind most likely to be familiar to readers as the Square of Opposition, found in elementary logic texts since the mid-19th century. The square, as Krauss sees it, defines a kind of idealized space "within which to work out unbearable contradictions produced within the real field of history." This she calls, using the inevitable gallicism, "the site of Jameson's Political Unconscious" and then, in art, the optical unconscious, which consists of what Utopian Modernism had to kick downstairs, to repress, to "evacuate… from its field."
— Arthur C. Danto in ArtForum, Summer 1993
Rosalind Krauss in The Optical Unconscious (MIT Press paperback, 1994):
For a presentation of the Klein Group, see Marc Barbut, "On the Meaning of the Word 'Structure' in Mathematics," in Introduction to Structuralism, ed. Michael Lane (New York: Basic Books, 1970). Claude Lévi-Strauss uses the Klein group in his analysis of the relation between Kwakiutl and Salish masks in The Way of the Masks, trans. Sylvia Modelski (Seattle: University of Washington Press, 1982), p. 125; and in relation to the Oedipus myth in "The Structural Analysis of Myth," Structural Anthropology, trans. Claire Jackobson [sic] and Brooke Grundfest Schoepf (New York: Basic Books, 1963). In a transformation of the Klein Group, A. J. Greimas has developed the semiotic square, which he describes as giving "a slightly different formulation to the same structure," in "The Interaction of Semiotic Constraints," On Meaning (Minneapolis: University of Minnesota Press, 1987), p. 50. Jameson uses the semiotic square in The Political Unconscious (see pp. 167, 254, 256, 277) [Fredric Jameson, The Political Unconscious: Narrative as a Socially Symbolic Act (Ithaca: Cornell University Press, 1981)], as does Louis Marin in "Disneyland: A Degenerate Utopia," Glyph, no. 1 (1977), p. 64.
Wikipedia on the Klein group (denoted V, for Vierergruppe):
In this representation, V is a normal subgroup of the alternating group A4 (and also the symmetric group S4) on 4 letters. In fact, it is the kernel of a surjective map from S4 to S3. According to Galois theory, the existence of the Klein four-group (and in particular, this representation of it) explains the existence of the formula for calculating the roots of quartic equations in terms of radicals.
For material related to Klee's phrase mentioned above by Stevens, "the organic center of all movement in time and space," see the following Google search:
The black monolith of
Kubrick's 2001 is, in
its way, an example
of religious art.
One artistic shortcoming
(or strength– it is, after
all, monolithic) of
that artifact is its
resistance to being
analyzed as a whole
consisting of parts, as
in a Joycean epiphany.
The following
figure does
allow such
an epiphany.
One approach to
the epiphany:
"Transformations play
a major role in
modern mathematics."
– A biography of
Felix Christian Klein
The above 2×4 array
(2 columns, 4 rows)
furnishes an example of
a transformation acting
on the parts of
an organized whole:
For other transformations
acting on the eight parts,
hence on the 35 partitions, see
"Geometry of the 4×4 Square,"
as well as Peter J. Cameron's
"The Klein Quadric
and Triality" (pdf),
and (for added context)
"The Klein Correspondence,
Penrose Space-Time, and
a Finite Model."
For a related structure–
not rectangle but cube–
see Epiphany 2008.
On April 16, the Pope’s birthday, the evening lottery number in Pennsylvania was 441. The Log24 entries of April 17 and April 18 supplied commentaries based on 441’s incarnation as a page number in an edition of Heidegger’s writings. Here is a related commentary on a different incarnation of 441. (For a context that includes both today’s commentary and those of April 17 and 18, see Gian-Carlo Rota– a Heidegger scholar as well as a mathematician– on mathematical Lichtung.)
From R. D. Carmichael, Introduction to the Theory of Groups of Finite Order (Boston, Ginn and Co., 1937)– an exercise from the final page, 441, of the final chapter, “Tactical Configurations”–
“23. Let G be a multiply transitive group of degree n whose degree of transitivity is k; and let G have the property that a set S of m elements exists in G such that when k of the elements S are changed by a permutation of G into k of these elements, then all these m elements are permuted among themselves; moreover, let G have the property P, namely, that the identity is the only element in G which leaves fixed the n – m elements not in S. Then show that G permutes the m elements S into
m(m – 1) … (m – k + 1)
This exercise concerns an important mathematical structure said to have been discovered independently by the American Carmichael and by the German Ernst Witt.
For some perhaps more comprehensible material from the preceding page in Carmichael– 440– see Diamond Theory in 1937.
“It is said that the students of medieval Paris came to blows in the streets over the question of universals. The stakes are high, for at issue is our whole conception of our ability to describe the world truly or falsely, and the objectivity of any opinions we frame to ourselves. It is arguable that this is always the deepest, most profound problem of philosophy.”
— Simon Blackburn, Think (Oxford, 1999)
Michael Harris, mathematician at the University of Paris:
“… three ‘parts’ of tragedy identified by Aristotle that transpose to fiction of all types– plot (mythos), character (ethos), and ‘thought’ (dianoia)….”
— paper (pdf) to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.
Mythos —
A visitor from France this morning viewed the entry of Jan. 23, 2006: “In Defense of Hilbert (On His Birthday).” That entry concerns a remark of Michael Harris.
A check of Harris’s website reveals a new article:
“Do Androids Prove Theorems in Their Sleep?” (slighly longer version of article to appear in Mathematics and Narrative, A. Doxiadis and B. Mazur, eds.) (pdf).
From that article:
“The word ‘key’ functions here to structure the reading of the article, to draw the reader’s attention initially to the element of the proof the author considers most important. Compare E.M. Forster in Aspects of the Novel:
[plot is] something which is measured not be minutes or hours, but by intensity, so that when we look at our past it does not stretch back evenly but piles up into a few notable pinnacles.”
Ethos —
“Forster took pains to widen and deepen the enigmatic character of his novel, to make it a puzzle insoluble within its own terms, or without. Early drafts of A Passage to India reveal a number of false starts. Forster repeatedly revised drafts of chapters thirteen through sixteen, which comprise the crux of the novel, the visit to the Marabar Caves. When he began writing the novel, his intention was to make the cave scene central and significant, but he did not yet know how:
When I began a A Passage to India, I knew something important happened in the Malabar (sic) Caves, and that it would have a central place in the novel– but I didn’t know what it would be… The Malabar Caves represented an area in which concentration can take place. They were to engender an event like an egg.”
— E. M. Forster: A Passage to India, by Betty Jay
Dianoia —
“Despite the flagrant triviality of the proof… this result is the key point in the paper.”
— Michael Harris, op. cit., quoting a mathematical paper
Online Etymology Dictionary:
flagrant c.1500, “resplendent,” from L. flagrantem (nom. flagrans) “burning,” prp. of flagrare “to burn,” from L. root *flag-, corresponding to PIE *bhleg– (cf. Gk. phlegein “to burn, scorch,” O.E. blæc “black”). Sense of “glaringly offensive” first recorded 1706, probably from common legalese phrase in flagrante delicto “red-handed,” lit. “with the crime still blazing.”
A related use of “resplendent”– applied to a Trinity, not a triviality– appears in the Liturgy of Malabar:
— The Liturgies of SS. Mark, James, Clement, Chrysostom, and Basil, and the Church of Malabar, by the Rev. J.M. Neale and the Rev. R.F. Littledale, reprinted by Gorgias Press, 2002
Judy Davis in the Marabar Caves
In mathematics
(as opposed to narrative),
somewhere between
a flagrant triviality and
a resplendent Trinity we
have what might be called
“a resplendent triviality.”
For further details, see
“A Four-Color Theorem.”
Click image to enlarge.
The “Boy’s Life” illustration is of an Arthur C. Clarke story, “Against the Fall of Night.” This, according to the review quoted below, was Clarke’s first story, begun in 1936 and first published in 1948. The title is from a poem by
From a book review by Christopher B. Jones:
“Against the Fall of Night describes well how it often takes youth to bring forth change. The older mind becomes locked in a routine, or blocks out things because it has been told that it shouldn’t think or talk about them. But the young mind is ever the explorer, seeking out knowledge without the taboos placed on it by a rigid society. Alvin is a breath of fresh air in the don’t-look-over-the-wall society of Diaspar.
Myths play a big role, and an interesting religious overtone pervades the story with a long since departed being whose origins are unknown and who played an important part in Earth’s past. Parallels to Jesus can easily be drawn, and the forecast shown for the longevity of religions in general seems to me to be rather accurate….
Finally, when Alvin uncovers part of the truth he has been looking for, he learns of the dangers and stagnation that can befall a xenophobic society. There are still a few such societies in the world today, and this characteristic almost always comes with negative effects– even if it has been cultivated with the intention to protect.”
An example of such a xenophobic society is furnished by the Hadassah ad currently running in the New York Times obituaries section: “Who will say Kaddish in Israel?”
Another example:
Tom Stoppard, in the London Times of Sunday, March 16, 2008, on the social unrest of forty years ago in 1968–
“Altering the psyche was supposed to change the social structure but, as a Marxist, Max knows it really works the other way: changing the social structure is the only way to change the psyche. The idea that ‘make love, not war’ is a more practical slogan than ‘workers of the world unite’ is as airy-fairy as the I Ching.”
Airy-fairy, Jewey-phooey.
Clarke’s 1948 story was the basis of his 1956 novel, The City and the Stars. In memory of the star Richard Widmark, here are two illustrations from St. Mark’s Day, 2003:
Housman asks the reader
to tell him of runes to grave
or bastions to design
“against the fall of night.”
Here, as examples, are
one rune and one bastion.
|
Represents |
|
Neither part of this memorial suits the xenophobic outlook of Israel. Both parts, together, along with his classic film “The Long Ships,” seem somehow suited to the non-xenophobic outlook of Richard Widmark. As for the I Ching… perhaps Widmark has further voyages to make.
Part II
“Raiders of the Lost…”
(Feb. 17, 2006)
Part III
The Further
Adventures of
Tony Rome
(March 7, 2008)
Parts I and II above
may be summarized by
the famous phrase
“jewel in the lotus”–
which, some say, has
a sexual meaning–
and by the diagram
For discussions
of this structure
in Western thought,
see
the ovato tondo
and
Last to the Lost.
This note is prompted by the March 4 death of Richard D. Anderson, writer on geometry, President (1981-82) of the Mathematical Association of America (MAA), and member of the MAA's Icosahedron Society.
"The historical road
from the Platonic solids
to the finite simple groups
is well known."
— Steven H. Cullinane,
November 2000,
Symmetry from Plato to
the Four-Color Conjecture
"By far the most important structure in design theory is the Steiner system S(5, 8, 24)."
This Steiner system is closely connected to M24 and to the extended binary Golay code. Brouwer gives an elegant construction of that code (and therefore of M24):
"Let N be the adjacency matrix of the icosahedron (points: 12 vertices, adjacent: joined by an edge). Then the rows of the 12×24 matrix
— Op. cit., p. 719
Finite Geometry of
the Square and Cube
and
Jewel in the Crown
"There is a pleasantly discursive
treatment of Pontius Pilate's
unanswered question
'What is truth?'"
— H. S. M. Coxeter, 1987,
introduction to Trudeau's
"story theory" of truth
Those who prefer stories to truth
may consult the Log24 entries
of March 1, 2, 3, 4, and 5.
They may also consult
the poet Rubén Darío:
… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte.
Click on image
for details.
“The alternative to ‘crystalline closure’
is not, then, an endless and chaotic
‘repetition and proliferation,’ but a
*structured relationship of significance.”
— The Old New Criticism and Its Critics,
by R. V. Young, Professor of English
at North Carolina State University
On Anthony Hopkins’s new film:
“At one point during ‘Slipstream,’ Hopkins’s character stumbles upon a Dolly Parton impersonator while Parton’s wonderful song, ‘Coat of Many Colors,’ plays on the soundtrack. I told Hopkins that I thought he used the tune– which is about a multi-hued coat that little Dolly’s grandmother made for her out of random pieces of cloth when the future superstar’s family was dirt poor– as a sort of commentary on the patchwork structure of ‘Slipstream’ itself. Hopkins smiled broadly and his eyes lit up. Yes, he said, that’s exactly what he was doing. He said he even tried to get Parton to appear in the movie, but she was booked and couldn’t do it.”
— Paul Tatara, Oct. 22, 2007
“Our existence is beyond understanding. Nobody has an answer. I sense that life is such a mystery. To me, God is time.”
It’s been going on for a billion years and it will last another billion or so. Up and down the timeline, the two sides– ‘Spiders’ and ‘Snakes’– battle endlessly to change the future and the past. Our lives, our memories, are their battleground. And in the midst of the war is the Place, outside space and time, where Greta Forzane and the other Entertainers provide solace and r-&-r for tired time warriors.”
— Publisher’s description of Fritz Leiber’s Big Time.
“My God, this place must be
a million years old!”
“Dolly’s Little Diner–
Home from Home”
Meanwhile…
Country Star
Porter Wagoner, 80, Dies
Wallace Stevens,
“Country Words”–
“What is it that my feeling seeks?
I know from all the things it touched
And left beside and left behind.
It wants the diamond pivot bright.”
An earlier entry today (“Hollywood Midrash continued“) on a father and son suggests we might look for an appropriate holy ghost. In that context…
A search for further background on Emmanuel Levinas, a favorite philosopher of the late R. B. Kitaj (previous two entries), led (somewhat indirectly) to the following figures of Descartes:
Compare and contrast:
The harmonic-analysis analogy suggests a review of an earlier entry’s
link today to 4/30– Structure and Logic— as well as
re-examination of Symmetry and a Trinity
(Dec. 4, 2002).
See also —
A Four-Color Theorem,
The Diamond Theorem, and
The Most Violent Poem,
|
"Logos and logic, crystal hypothesis,
— Wallace Stevens, |
Yesterday's meditation ("Simon's Shema") on the interpenetration of opposites continues:
"The fundamental conception of Tantric Buddhist metaphysics, namely, yuganaddha, signifies the coincidence of opposites. It is symbolized by the conjugal embrace (maithuna or kama-kala) of a god and goddess or a Buddha and his consort (signifying karuna and sunyata or upaya and prajna, respectively), also commonly depicted in Tantric Buddhist iconography as the union of vajra (diamond sceptre) and padme (lotus flower). Thus, yuganaddha essentially means the interpenetration of opposites or dipolar fusion, and is a fundamental restatement of Hua-yen theoretic structures."
— p. 148 in "Part II: A Whiteheadian Process Critique of Hua-yen Buddhism," in Process Metaphysics and Hua-Yen Buddhism: A Critical Study of Cumulative Penetration vs. Interpenetration (SUNY Series in Systematic Philosophy), by Steve Odin, State University of New York Press, 1982
And on p. 163 of Odin, op. cit., in "Part III: Theology of the Deep Unconscious: A Reconstruction of Process Theology," in the section titled "Whitehead's Dipolar God as the Collective Unconscious"–
"An effort is made to transpose Whitehead's theory of the dipolar God into the terms of the collective unconscious, so that now the dipolar God is to be comprehended not as a transcendent deity, but the deepest dimension and highest potentiality of one's own psyche."
Odin obtained his Ph.D. degree from the Department of Philosophy at the State University of New York (SUNY) at Stony Brook in 1980. (See curriculum vitae (pdf).)
For an academic review of Odin's book, see David Applebaum, Philosophy East and West, Vol. 34 (1984), pp. 107-108.
It is perhaps worth noting, in light of the final footnote of Mark D. Brimblecombe's Ph.D. thesis "Dipolarity and God" quoted yesterday, that "tantra" is said to mean "loom." For some less-academic background on the Tantric iconography Odin describes, see the webpage "Love and Passion in Tantric Buddhist Art." For a fiction combining love and passion with the word "loom" in a religious context, see Clive Barker's Weaveworld. This fiction– which is, if not "supreme" in the Wallace Stevens sense, at least entertaining– may correspond to some aspects of the deep Jungian psychological reality discussed by Odin.
Click on image for details.
was the title of a symposium on quantum theory at Princeton last week dedicated to the late John von Neumann. The title was left undefined. In honor of von Neumann, here is some material that may help those searching for the title’s meaning:
Those who wisely object that popularity should not be a test of beauty may consult a little-known (at least in the West) Sino-Japanese definition of “deep beauty.” This definition– although from philosophy, not physics– may appeal to those who, like Peter Woit, are troubled by a Christian foundation’s sponsorship of last week’s scientific symposium.
“Deep beauty”
is yuugen.
On Spekkens’ toy system and finite geometry
Background–
On finite geometry:
The actions of permutations on a 4 × 4 square in Spekkens’ paper (quant-ph/0401052), and Leifer’s suggestion of the need for a “generalized framework,” suggest that finite geometry might supply such a framework. The geometry in the webpage John cited is that of the affine 4-space over the two-element field.
Related material:
See also arXiv:0707.0074v1 [quant-ph], June 30, 2007:
A fully epistemic model for a local hidden variable emulation of quantum dynamics,
by Michael Skotiniotis, Aidan Roy, and Barry C. Sanders, Institute for Quantum Information Science, University of Calgary. Abstract: "In this article we consider an augmentation of Spekkens’ toy model for the epistemic view of quantum states [1]…."
Hypercube from the Skotiniotis paper:
Reference:
Perimeter Institute for Theoretical Physics, 31 Caroline Street North, Waterloo, Ontario, Canada N2L 2Y5 (Received 11 October 2005; revised 2 November 2006; published 19 March 2007.)
In the context of quantum information theory, the following structure seems to be of interest–
"… the full two-by-two matrix ring with entries in GF(2), M2(GF(2))– the unique simple non-commutative ring of order 16 featuring six units (invertible elements) and ten zero-divisors."
— "Geometry of Two-Qubits," by Metod Saniga (pdf, 17 pp.), Jan. 25, 2007
This ring is another way of looking at the 16 elements of the affine space A4(GF(2)) over the 2-element field. (Arrange the four coordinates of each element– 1's and 0's– into a square instead of a straight line, and regard the resulting squares as matrices.) (For more on A4(GF(2)), see Finite Relativity and related notes at Finite Geometry of the Square and Cube.) Using the above ring, Saniga constructs a system of 35 objects (not unlike the 35 lines of the finite geometry PG(3,2)) that he calls a "projective line" over the ring. This system of 35 objects has a subconfiguration isomorphic to the (2,2) generalized quadrangle W2 (which occurs naturally as a subconfiguration of PG(3,2)– see Inscapes.)
Saniga concludes:
"We have demonstrated that the basic properties of a system of two interacting spin-1/2 particles are uniquely embodied in the (sub)geometry of a particular projective line, found to be equivalent to the generalized quadrangle of order two. As such systems are the simplest ones exhibiting phenomena like quantum entanglement and quantum non-locality and play, therefore, a crucial role in numerous applications like quantum cryptography, quantum coding, quantum cloning/teleportation and/or quantum computing to mention the most salient ones, our discovery thus
- not only offers a principally new geometrically-underlined insight into their intrinsic nature,
- but also gives their applications a wholly new perspective
- and opens up rather unexpected vistas for an algebraic geometrical modelling of their higher-dimensional counterparts."
Some fear that the Harry Potter books introduce children to the occult; they are not entirely mistaken.
According to Wikipedia, the “Deathly Hallows” of the final Harry Potter novel are “three fictional magical objects that appear in the book.”
The vertical line, circle, and triangle in the symbol pictured above are said to refer to these three magical objects.
One fan relates the “Deathly Hallows” symbol above, taken from the spine of a British children’s edition of the book, to a symbol for “the divine (or sacred, or secret) fire” of alchemy. She relates this fire in turn to “serpent power” and the number seven:
Kristin Devoe at a Potter fan site:
“We know that seven is a powerful number in the novels. Tom Riddle calls it ‘the most powerfully magic number.‘ The ability to balance the seven chakras within oneself allows the person to harness the secret fire. This secret fire in alchemy is the same as the kundalini or coiled snake in yogic philosophy. It is also known as ‘serpent power’ or the ‘dragon’ depending on the tradition. The kundalini is polar in nature and this energy, this internal fire, is very powerful for those who are able to harness it and it purifies the aspirant allowing them the knowledge of the universe. This secret fire is the Serpent Power which transmutes the base metals into the Perfect Gold of the Sun.
It is interesting that the symbol of the caduceus in alchemy is thought to have been taken from the symbol of the kundalini. Perched on the top of the caduceus, or the staff of Hermes, the messenger of the gods and revealer of alchemy, is the golden snitch itself! Many fans have compared this to the scene in The Order of the Phoenix where Harry tells Dumbledore about the attack on Mr. Weasley and says, ‘I was the snake, I saw it from the snake’s point of view.‘
The chapter continues with Dumbledore consulting ‘one of the fragile silver instruments whose function Harry had never known,’ tapping it with his wand:
The instrument tinkled into life at once with rhythmic clinking noises. Tiny puffs of pale green smoke issued from the minuscule silver tube at the top. Dumbledore watched the smoke closely, his brow furrowed, and after a few seconds, the tiny puffs became a steady stream of smoke that thickened and coiled into he air… A serpent’s head grew out of the end of it, opening its mouth wide. Harry wondered whether the instrument was confirming his story; He looked eagerly at Dumbledore for a sign that he was right, but Dumbledore did not look up.
“Naturally, Naturally,” muttered Dumbledore apparently to himself, still observing the stream of smoke without the slightest sign of surprise. “But in essence divided?”
Harry could make neither head not tail of this question. The smoke serpent, however split instantly into two snakes, both coiling and undulating in the dark air. With a look of grim satisfaction Dumbledore gave the instrument another gentle tap with his wand; The clinking noise slowed and died, and the smoke serpents grew faint, became a formless haze, and vanished.
Could these coiling serpents of smoke be foreshadowing events to come in Deathly Hallows where Harry learns to ‘awaken the serpent’ within himself? Could the snake’s splitting in two symbolize the dual nature of the kundalini?”
and the following
famous illustration of
the double-helix
structure of DNA:
This is taken from
a figure accompanying
an obituary, in today’s
New York Times, of the
artist who drew the figure.
The double helix
is not a structure
from magic; it may,
however, as the Rowling
quote above shows, have
certain occult uses,
better suited to
Don Henley’s
Garden of Allah
than to the
Garden of Apollo.
Similarly, the three objects
above (Log24 on April 9)
are from pure mathematics–
the realm of Apollo, not
of those in Henley’s song.
The similarity of the
top object of the three —
the “Fano plane” — to
the “Deathly Hallows”
symbol is probably
entirely coincidental.
| Odile Crick with her husband, Francis H.C. Crick, in Cambridge, England. Mrs. Crick, an artist, illustrated the work of her husband, whose team received a Nobel Prize for its DNA research. |
| Photo Credit: Courtesy Of The Salk Institute For Biological Studies |
“Her graceful drawing of the double-helix structure of DNA with intertwined helical loops has become a symbol of the achievements of science and its aspirations to understand the secrets of life. The image represents the base pairs of nucleic acids, twisted around a center line to show the axis of the helix. Terrence J. Sejnowski, a neuroscientist at the Salk Institute for Biological Studies in La Jolla, where Francis Crick later worked, said: ‘Mrs. Crick’s drawing was an abstract representation of DNA, but it was accurate with regard to its shape and size of its spacing.
‘The models you see now have all the atoms in them,’ Sejnowski said. ‘The one in Nature was the backbone and gave the bare outline. It may be the most famous [scientific] drawing of the 20th century, in that it defines modern biology.'”
Illustration from
Log24, April 7, 2003:
April is Math Awareness Month.
This year’s theme is “mathematics and art.”
Illustration from
this morning’s
New York Times:
Illustration from
the journal Nature, 1953:
The August 2007 issue of Notices of the American Mathematical Society contains a review of a new book by Douglas Hofstadter, I Am a Strange Loop. (2007, Basic Books, New York. $26.95, 412 pages.)
A better review, in the Los Angeles Times of March 18, 2007, notes an important phrase in the book, "interpenetration of souls," that the AMS Notices review ignores.
Here is an Amazon.com search on "interpenetration" in the Hofstadter book:
| 1. | on Page 217: |
| "… described does not create a profound blurring of two people's identities. Tennis and driving do not give rise to deep interpenetrations of souls. …" | |
| 2. | on Page 237: |
| "… What seems crucial here is the depth of interpenetration of souls the sense of shared goals, which leads to shared identity. Thus, for instance, Carol always had a deep, …" | |
| 3. | on Page 270: |
| "… including the most private feelings and the most confidential confessions, then the interpenetration of our worlds becomes so great that our worldviews start to fuse. Just as I could jump to California when …" | |
| 4. | on Page 274: |
| "… we choose to downplay or totally ignore the implications of the everyday manifestations of the interpenetration of souls. Consider how profoundly wrapped up you can become in a close friend's successes and failures, in their very …" | |
| 5. | on Page 276: |
| "… Interpenetration of National Souls Earlier in this chapter, I briefly offered the image of a self as analogous to a country …" | |
| 6. | from Index: |
| "… birthday party for, 350 "bachelor", elusiveness of concept, 178 bad-breath analogy, 150 bandwidth of communication as determinant of degree of interpenetration, 212 213, 220, …" | |
| 7. | from Index: |
| "… phrases denying interpenetration of souls, 270 271; physical phenomena that lack consciousness, 281 282; physical structures lacking hereness, 283; potential personal attributes, 183; …" |
The American Mathematical Society editors and reviewer seem to share Hofstadter's ignorance of Christian doctrine; they might otherwise have remembered a rather famous remark: "This is not mathematics, it is theology."
For more on the theology of interpenetration, see Log24 on "Perichoresis, or Coinherence" (Jan. 22, 2004).
For a more mathematical approach to this topic, see Spirituality Today, Spring 1991:
"… the most helpful image is perhaps the ellipse often used to surround divine figures in ancient art, a geometrical figure resulting from the overlapping, greater or lesser, of two independent circles, an interpenetration or coinherence which will, in some sense, reunify divided humanity, thus restoring to some imperfect degree the original image of God."
See also the trinitarian doctrine implicit in related Log24 entries of July 1, 2007, which include the following illustration of the geometrical figure described, in a somewhat confused manner, above:
"Values are rooted
in narrative."
— Harvey Cox,
Hollis Professor
of Divinity
at Harvard,
Atlantic Monthly,
November 1995
Related material:
Steps Toward Salvation:
An Examination of
Co-Inherence and
Substitution in
the Seven Novels
of Charles Williams,
by Dennis L. Weeks
In Defense of
Plato’s Realism
(vs. sophists’ nominalism–
see recent entries.)
Plato cited geometry,
notably in the Meno,
in defense of his realism.
Consideration of the
Meno’s diamond figure
leads to the following:
Click on image for details.
As noted in an entry,
Plato, Pegasus, and
the Evening Star,
linked to
at the end of today’s
previous entry,
the “universals”
of Platonic realism
are exemplified by
the hexagrams of
the I Ching,
which in turn are
based on the seven
trigrams above and
on the eighth trigram,
of all yin lines,
not shown above:
K’un
The Receptive
_____________________________________________
Update of Nov. 30, 2013:
From a little-known website in Kuala Lumpur:
(Click to enlarge.)
The remarks on Platonic realism are from Wikipedia.
The eightfold cube is apparently from this post.
(continued from
June 18, 2002)
The “ignorance” referred to
is Fish’s ignorance of the
philosophical background
of the words
“particular” and “universal.”
“Although it may not at first be obvious, the substitution for real religions of a religion drained of particulars is of a piece with the desire to exorcise postmodernism.”
“What must be protected, then, is the general, the possibility of making pronouncements from a perspective at once detached from and superior to the sectarian perspectives of particular national interests, ethnic concerns, and religious obligations; and the threat to the general is posed by postmodernism and strong religiosity alike, postmodernism because its critique of master narratives deprives us of a mechanism for determining which of two or more fiercely held beliefs is true (which is not to deny the category of true belief, just the possibility of identifying it uncontroversially), strong religiosity because it insists on its own norms and refuses correction from the outside. The antidote to both is the separation of the private from the public, the establishing of a public sphere to which all could have recourse and to the judgments of which all, who are not criminal or insane, would assent. The point of the public sphere is obvious: it is supposed to be the location of those standards and measures that belong to no one but apply to everyone. It is to be the location of the universal. The problem is not that there is no universal–the universal, the absolutely true, exists, and I know what it is. The problem is that you know, too, and that we know different things, which puts us right back where we were a few sentences ago, armed with universal judgments that are irreconcilable, all dressed up and nowhere to go for an authoritative adjudication.
What to do? Well, you do the only thing you can do, the only honest thing: you assert that your universal is the true one, even though your adversaries clearly do not accept it, and you do not attribute their recalcitrance to insanity or mere criminality–the desired public categories of condemnation–but to the fact, regrettable as it may be, that they are in the grip of a set of beliefs that is false. And there you have to leave it, because the next step, the step of proving the falseness of their beliefs to everyone, including those in their grip, is not a step available to us as finite situated human beings. We have to live with the knowledge of two things: that we are absolutely right and that there is no generally accepted measure by which our rightness can be independently validated. That’s just the way it is, and we should just get on with it, acting in accordance with our true beliefs (what else could we do?) without expecting that some God will descend, like the duck in the old Groucho Marx TV show, and tell us that we have uttered the true and secret word.”
From the public spheres
of the Pennsylvania Lottery:
“‘From your lips
to God’s ears,’
goes the old
Yiddish wish.
The writer, by contrast,
tries to read God’s lips
and pass along
the words….”
— Richard Powers
268 —
This is a page number
that appears, notably,
in my June 2002
journal entry on Fish,
and again in an entry,
“The Transcendent Signified,”
dated July 26, 2003,
that argues against
Fish’s school, postmodernism,
and in favor of what the pomos
call “logocentrism.”
Page 268
of Simon Blackburn’s Think
(Oxford Univ. Press, 1999):
When not arguing politics,
Fish, though from
a Jewish background, is
said to be a Milton scholar.
Let us therefore hope he
is by now, or comes to be,
aware of the Christian
approach to universals–
an approach true to the
philosophical background
sketched in 1999 by
Blackburn and made
particular in a 1931 novel
by Charles Williams,
The Place of the Lion.
Let No Man
Write My Epigraph
"His graceful accounts of the Bach Suites for Unaccompanied Cello illuminated the works’ structural logic as well as their inner spirituality."
—Allan Kozinn on Mstislav Rostropovich in The New York Times, quoted in Log24 on April 29, 2007
"At that instant he saw, in one blaze of light, an image of unutterable conviction…. the core of life, the essential pattern whence all other things proceed, the kernel of eternity."
— Thomas Wolfe, Of Time and the River, quoted in Log24 on June 9, 2005
"… the stabiliser of an octad preserves the affine space structure on its complement, and (from the construction) induces AGL(4,2) on it. (It induces A8 on the octad, the kernel of this action being the translation group of the affine space.)"
— Peter J. Cameron, "The Geometry of the Mathieu Groups" (pdf)
"… donc Dieu existe, réponse!"
(Faust, Part Two, as
quoted by Jung in
Memories, Dreams, Reflections)
"Pauli as Mephistopheles
in a 1932 parody of
Goethe's Faust at Niels Bohr's
institute in Copenhagen.
The drawing is one of
many by George Gamow
illustrating the script."
— Physics Today
'To meet someone' was his enigmatic answer. 'To search for the stone that the Great Architect rejected, the philosopher's stone, the basis of the philosophical work. The stone of power. The devil likes metamorphoses, Corso.'"
— The Club Dumas, basis for the Roman Polanski film "The Ninth Gate" (See 12/24/05.)
— The Innermost Kernel
(previous entry)
And from
"Symmetry in Mathematics
and Mathematics of Symmetry"
(pdf), by Peter J. Cameron,
a paper presented at the
International Symmetry Conference,
Edinburgh, Jan. 14-17, 2007,
we have
The Epigraph–
(Here "whatever" should
of course be "whenever.")
Also from the
Cameron paper:
|
Local or global?
Among other (mostly more vague) definitions of symmetry, the dictionary will typically list two, something like this:
• exact correspondence of parts; Mathematicians typically consider the second, global, notion, but what about the first, local, notion, and what is the relationship between them? A structure M is homogeneous if every isomorphism between finite substructures of M can be extended to an automorphism of M; in other words, "any local symmetry is global." |
Some Log24 entries
related to the above politically
(women in mathematics)–
Global and Local:
One Small Step
and mathematically–
Structural Logic continued:
Structure and Logic (4/30/07):
This entry cites
Alice Devillers of Brussels–
"The aim of this thesis
is to classify certain structures
which are, from a certain
point of view, as homogeneous
as possible, that is which have
as many symmetries as possible."
"There is such a thing
as a tesseract."
Rudolf Arnheim, a student of Gestalt psychology (which, an obituary notes, emphasizes "the perception of forms as organized wholes") was the first Professor of the Psychology of Art at Harvard. He died at 102 on Saturday, June 9, 2007.
The conclusion of yesterday's New York Times obituary of Arnheim:
"… in The New York Times Book Review in 1986, Celia McGee called Professor Arnheim 'the best kind of romantic,' adding, 'His wisdom, his patient explanations and lyrical enthusiasm are those of a teacher.'"
A related quotation:
"And you are teaching them a thing or two about yourself. They are learning that you are the living embodiment of two timeless characterizations of a teacher: 'I say what I mean, and I mean what I say' and 'We are going to keep doing this until we get it right.'"
Here, yet again, is an illustration that has often appeared in Log24– notably, on the date of Arnheim's death:
Related quotations:
"We have had a gutful of fast art and fast food. What we need more of is slow art: art that holds time as a vase holds water: art that grows out of modes of perception and whose skill and doggedness make you think and feel; art that isn't merely sensational, that doesn't get its message across in 10 seconds, that isn't falsely iconic, that hooks onto something deep-running in our natures. In a word, art that is the very opposite of mass media. For no spiritually authentic art can beat mass media at their own game."
— Robert Hughes, speech of June 2, 2004
"Whether the 3×3 square grid is fast art or slow art, truly or falsely iconic, perhaps depends upon the eye of the beholder."
If the beholder is Rudolf Arnheim, whom we may now suppose to be viewing the above figure in the afterlife, the 3×3 square is apparently slow art. Consider the following review of his 1982 book The Power of the Center:
"Arnheim deals with the significance of two kinds of visual organization, the concentric arrangement (as exemplified in a bull's-eye target) and the grid (as exemplified in a Cartesian coordinate system)….
It is proposed that the two structures of grid and target are the symbolic vehicles par excellence for two metaphysical/psychological stances. The concentric configuration is the visual/structural equivalent of an egocentric view of the world. The self is the center, and all distances exist in relation to the focal spectator. The concentric arrangement is a hermetic, impregnable pattern suited to conveying the idea of unity and other-worldly completeness. By contrast, the grid structure has no clear center, and suggests an infinite, featureless extension…. Taking these two ideal types of structural scaffold and their symbolic potential (cosmic, egocentric vs. terrestrial, uncentered) as given, Arnheim reveals how their underlying presence organizes works of art."
— Review of Rudolf Arnheim's The Power of the Center: A Study of Composition in the Visual Arts (Univ. of Calif. Press, 1982). Review by David A. Pariser, Studies in Art Education, Vol. 24, No. 3 (1983), pp. 210-213
Arnheim himself says in this book (pp. viii-ix) that "With all its virtues, the framework of verticals and horizontals has one grave defect. It has no center, and therefore it has no way of defining any particular location. Taken by itself, it is an endless expanse in which no one place can be distinguished from the next. This renders it incomplete for any mathematical, scientific, and artistic purpose. For his geometrical analysis, Descartes had to impose a center, the point where a pair of coordinates [sic] crossed. In doing so he borrowed from the other spatial system, the centric and cosmic one."
Students of art theory should, having read the above passages, discuss in what way the 3×3 square embodies both "ideal types of structural scaffold and their symbolic potential."
We may imagine such a discussion in an afterlife art class– in, perhaps, Purgatory rather than Heaven– that now includes Arnheim as well as Ernst Gombrich and Kirk Varnedoe.
Such a class would be one prerequisite for a more advanced course– Finite geometry of the square and cube.
This morning was the
Princeton commencement.
Meanwhile…
From the May 18 Harvard Crimson:
“Paul B. Davis ’07-’08, who contributed
to a collection of student essays
written in 2005 on the purpose
and structure of a Harvard education,
said that ‘the devil is in the details’….”
For the details, see
Al Gore and the
Absence of Truth
(May 30, 2007).
From the May 18 Harvard Crimson:
“Paul B. Davis ’07-’08, who contributed to a collection of student essays written in 2005 on the purpose and structure of a Harvard education, said that ‘the devil is in the details’….”
Related material:
“In philosophy, reductionism is a theory that asserts that the nature of complex things is reduced to the nature of sums of simpler or more fundamental things.” —Wikipedia
“In the 1920’s… the discovery of quantum mechanics went a very long way toward reducing chemistry to the solution of well-defined mathematical problems. Indeed, only the extreme difficulty of many of these problems prevents the present day theoretical chemist from being able to predict the outcome of every laboratory experiment by making suitable calculations. More recently the molecular biologists have made startling progress in reducing the study of life back to the study of chemistry. The living cell is a miniature but extremely active and elaborate chemical factory and many, if not most, biologists today are confident that there is no mysterious ‘vital principle,’ but that life is just very complicated chemistry. With biology reduced to chemistry and chemistry to mathematics, the measurable aspects of the world become quite pervasive.” –Harvard mathematician George Mackey, “What Do Mathematicians Do?“
Opposed to reductionism are “emergence” and “strong emergence“–
“Although strong emergence is logically possible, it is uncomfortably like magic.” —Mark A. Bedau
Or comfortably.
"… Mondrian and Malevich
are not discussing canvas
or pigment or graphite
or any other form of matter.
They are talking about about
Being or Mind or Spirit.
From their point of view,
the grid is a staircase
to the Universal…."
“Paul B. Davis ’07-’08, who contributed to a collection of student essays written in 2005 on the purpose and structure of a Harvard education, said that ‘the devil is in the details’….”
From the weblog of Peter Woit:
“The New Yorker keeps its physics theme going this week with cover art that includes a blackboard full of basic equations from quantum mechanics.”
Detail
The detail suggests
the following
religious images from
Twelfth Night 2003:
|
Devil’s Claws, or |
Yankee Puzzle, or |
“Mercilessly tasteful”
— Andrew Mueller,
review of Suzanne Vega’s
“Songs in Red and Gray“
Symbology:
“Also known as ‘processual symbolic analysis,’ this concept was developed by Victor Turner in the mid-1970s to refer to the use of symbols within cultural contexts, in particular ritual. In anthropology, symbology originated as part of Victor Turner’s concept of ‘comparative symbology.’ Turner (1920-1983) was professor of Anthropology at Cornell University, the University of Chicago, and finally he was Professor of Anthropology and Religion at the University of Virginia.” —Wikipedia
Symbology and Communitas:
|
From Beth Barrie’s
“Victor Turner“— “‘The positional meaning of a symbol derives from its relationship to other symbols in a totality, a Gestalt, whose elements acquire their significance from the system as a whole’ (Turner, 1967:51). Turner considered himself a comparative symbologist, which suggests he valued his contributions to the study of ritual symbols. It is in the closely related study of ritual processes that he had the most impact.
The most important contribution Turner made to the field of anthropology is his work on liminality and communitas. Believing the liminal stage to be of ‘crucial importance’ in the ritual process, Turner explored the idea of liminality more seriously than other anthropologists of his day. As noted earlier Turner elaborated on van Gennep’s concept of liminality in rites of passage. Liminality is a state of being in between phases. In a rite of passage the individual in the liminal phase is neither a member of the group she previously belonged to nor is she a member of the group she will belong to upon the completion of the rite. The most obvious example is the teenager who is neither an adult nor a child. ‘Liminal entities are neither here nor there; they are betwixt and between the positions assigned and arrayed by law, custom, convention, and ceremonial’ (Turner, 1969:95). Turner extended the liminal concept to modern societies in his study of liminoid phenomena in western society. He pointed out the similarities between the ‘leisure genres of art and entertainment in complex industrial societies and the rituals and myths of archaic, tribal and early agrarian cultures’ (1977:43). Closely associated to liminality is communitas which describes a society during a liminal period that is ‘unstructured or rudimentarily structured [with] a relatively undifferentiated comitatus, community, or even communion of equal individuals who submit together to the general authority of the ritual elders’ (Turner, 1969:96). The notion of communitas is enhanced by Turner’s concept of anti-structure. In the following passage Turner clarifies the ideas of liminal, communitas and anti-structure:
It is the potential of an anti-structured liminal person or liminal society (i.e., communitas) that makes Turner’s ideas so engaging. People or societies in a liminal phase are a ‘kind of institutional capsule or pocket which contains the germ of future social developments, of societal change’ (Turner, 1982:45). Turner’s ideas on liminality and communitas have provided scholars with language to describe the state in which societal change takes place.”
Turner, V. (1967). The forest of symbols: Aspects of Ndembu ritual. Ithaca, NY: Cornell University Press. Turner, V. (1969). The ritual process: structure and anti-structure. Chicago: Aldine Publishing Co. Turner, V. (1977). Variations of the theme of liminality. In Secular ritual. Ed. S. Moore & B. Myerhoff. Assen: Van Gorcum, 36-52. Turner, V. (1982). From ritual to theater: The human seriousness of play. New York: PAJ Publications. |
Related material on Turner in Log24:
Aug. 27, 2006 and Aug. 30, 2006. For further context, see archive of Aug. 19-31, 2006.
Related material on Cuernavaca:
Google search on Cuernavaca + Log24.
May '68 Revisited
"At his final Paris campaign rally… Mr. Sarkozy declared himself the candidate of the 'silent majority,' tired of a 'moral crisis in France not seen since the time of Joan of Arc.'
'I want to turn the page on May 1968,' he said of the student protests cum social revolution that rocked France almost four decades ago.
'The heirs of May '68 have imposed the idea that everything has the same worth, that there is no difference between good and evil, no difference between the true and the false, between the beautiful and the ugly and that the victim counts for less than the delinquent.'
Denouncing the eradication of 'values and hierarchy,' Mr. Sarkozy accused the Left of being the true heirs and perpetuators of the ideology of 1968."
— Emma-Kate Symons, Paris, May 1, 2007, in The Australian
Related material:
From the translator's introduction to Dissemination, by Jacques Derrida, translated by Barbara Johnson, University of Chicago Press, 1981, page xxxi —
"Both Numbers and 'Dissemination' are attempts to enact rather than simply state the theoretical upheavals produced in the course of a radical reevaluation of the nature and function of writing undertaken by Derrida, Sollers, Roland Barthes, Julia Kristeva and other contributors to the journal Tel Quel in the late 1960s. Ideological and political as well as literary and critical, the Tel Quel program attempted to push to their utmost limits the theoretical revolutions wrought by Marx, Freud, Nietzsche, Mallarme, Levi-Strauss, Saussure, and Heidegger."
This is the same Barbara Johnson who has served as the Frederic Wertham Professor of Law and Psychiatry in Society at Harvard.
Johnson has attacked "the very essence of Logic"–
"… the logic of binary opposition, the principle of non-contradiction, often thought of as the very essence of Logic as such….
Now, my understanding of what is most radical in deconstruction is precisely that it questions this basic logic of binary opposition….
Instead of a simple 'either/or' structure, deconstruction attempts to elaborate a discourse that says neither 'either/or', nor 'both/and' nor even 'neither/nor', while at the same time not totally abandoning these logics either."
— "Nothing Fails Like Success," SCE Reports 8, 1980
Such contempt for logic has resulted, for instance, in the following passage, quoted approvingly on page 342 of Johnson's translation of Dissemination, from Philippe Sollers's Nombres (1966):
"The minimum number of rows– lines or columns– that contain all the zeros in a matrix is equal to the maximum number of zeros located in any individual line or column."
For a correction of Sollers's Johnson's damned nonsense, click here.
Update of May 29, 2014:
The error, as noted above, was not Sollers's, but Johnson's.
See also the post of May 29, 2014 titled 'Lost in Translation.'
"Some postmodern theorists like to talk about the relationship between 'intertextuality' and 'hypertextuality'; intertextuality makes each text a 'mosaic of quotations' [Kristeva, Desire in Language, Columbia U. Pr., 1980, 66] and part of a larger mosaic of texts, just as each hypertext can be a web of links and part of the whole World-Wide Web." —Wikipedia
Day Without Logic,
Introduction to Logic,
The Geometry of Logic,
Structure and Logic,
Spider-Man and Fan:
"There is such a thing
as a tesseract."
— A Wrinkle in Time
The phrase “structural logic” in yesterday’s entry was applied to Bach’s cello suites. It may equally well be applied to geometry. In particular:
“The aim of this thesis is to classify certain structures which are, from a certain point of view, as homogeneous as possible, that is which have as many symmetries as possible.”
— Alice Devillers, “Classification of Some Homogeneous and Ultrahomogeneous Structures,” Ph.D. thesis, Université Libre de Bruxelles, academic year 2001-2002
Related material:
The above models for the corresponding projective spaces may be regarded as illustrating the phrase “structural logic.”
For a possible application of the 16-point space’s “many symmetries” to logic proper, see The Geometry of Logic.
Ben Brantley in this morning's New York Times:
"Television mows down a titan in 'Frost/Nixon,' the briskly entertaining new play by Peter Morgan* about the 1977 face-off between its title characters, the British talk show host (as in David) and the former American president (as in Richard M.)….
Structured as a prize fight between two starkly ambitious men in professional crisis, 'Frost/Nixon' makes it clear that the competitor who controls the camera reaps the spoils."
Another application of this
"control the camera" philosophy:
the multimedia manifesto of
the Virginia Tech author of
"Richard McBeef"
— Bernard Holland in
The New York Times
Monday, May 20, 1996
The headline for Edward Rothstein’s “Connections” column in The New York Times of Monday, March 26, 2007, was “Texts That Run Rings Around Everyday Linear Logic.”
|
The New York Lottery,
Friday, March 30, 2007: Mid-day 002 Evening 085 |
Continuing yesterday’s lottery meditation, let us examine today’s New York results in the light of Rothstein’s essay. The literary “ring” structure he describes is not immediately apparent in Friday’s numbers, although the mid-day number, 002– which in the I Ching signifies yin, the feminine, receptive principle– might be interpreted as referring to a ring of sorts.
Illustration from
an entry of
March 2, 2004
“A random selection from Hopkins’s journal shows how the sun acts as a focus….”
Today’s New York evening number,
85, reinforces this “ring” reference.
For related material, see
an entry for Reba McEntire’s
birthday four years ago.
"As noted previously, in Figure 2 viewed as a lattice the 16 digital labels 0000, 0001, etc., may be interpreted as naming the 16 subsets of a 4-set; in this case the partial ordering in the lattice is the structure preserved by the lattice's group of 24 automorphisms– the same automorphism group as that of the 16 Boolean connectives. If, however, these 16 digital labels are interpreted as naming the 16 functions from a 4-set to a 2-set (of two truth values, of two colors, of two finite-field elements, and so forth), it is not obvious that the notion of partial order is relevant. For such a set of 16 functions, the relevant group of automorphisms may be the affine group of A mentioned above. One might argue that each Venn diagram in Fig. 3 constitutes such a function– specifically, a mapping of four nonoverlapping regions within a rectangle to a set of two colors– and that the diagrams, considered simply as a set of two-color mappings, have an automorphism group of order larger than 24… in fact, of order 322,560. Whether such a group can be regarded as forming part of a 'geometry of logic' is open to debate."
The epigraph to "Finite Relativity" is:
"This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them."
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
The added paragraph seems to fit this description.
"The logic behind such utterances is the logic
of binary opposition, the principle of non-contra-
diction, often thought of as the very essence of
Logic as such….
Now, my understanding of what is most radical
in deconstruction is precisely that it questions
this basic logic of binary opposition….
Instead of a simple 'either/or' structure,
deconstruction attempts to elaborate a discourse
that says neither "either/or", nor "both/and"
nor even "neither/nor", while at the same time
not totally abandoning these logics either."
— Harvard professor Barbara Johnson
in "Nothing Fails Like Success."
(See the previous entry, Day Without Logic.)
Those who value literary theory
more than they value truth
may prefer, on this
International Women's Day,
the "mandorla" interpretation
of the above diagrams.
For this interpretation, see
Death and the Spirit III,
Burning Bright,
and
The Agony and the Ya-Ya.
Symbol of the Dec. 1
“Day Without Art“
This resembles the following symbol,
due to logician Charles Sanders Peirce,
of the logic of binary opposition:
(For futher details on the role
of this symbol in logic, see
Chinese Jar Revisited.)
On this, International Women’s Day,
we might also consider the
widely quoted thoughts on logic of
Harvard professor Barbara Johnson:
Detail:
“Instead of a simple ‘either/or’ structure,
deconstruction attempts to elaborate a discourse
that says neither “either/or”, nor “both/and”
nor even “neither/nor”, while at the same time
not totally abandoning these logics either.”
It may also be of interest on
International Women’s Day
that in the “box style” I Ching
(suggested by a remark of
Jungian analyst
Marie-Louise von Franz)
the symbol
denotes
Hexagram 2,
The Receptive.
Today is the 21st birthday of my note “The Relativity Problem in Finite Geometry.”
Some relevant quotations:
“This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them.”
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
Describing the branch of mathematics known as Galois theory, Weyl says that it
“… is nothing else but the relativity theory for the set Sigma, a set which, by its discrete and finite character, is conceptually so much simpler than the infinite set of points in space or space-time dealt with by ordinary relativity theory.”
— Weyl, Symmetry, Princeton University Press, 1952, p. 138
Weyl’s set Sigma is a finite set of complex numbers. Some other sets with “discrete and finite character” are those of 4, 8, 16, or 64 points, arranged in squares and cubes. For illustrations, see Finite Geometry of the Square and Cube. What Weyl calls “the relativity problem” for these sets involves fixing “objectively” a class of equivalent coordinatizations. For what Weyl’s “objectively” means, see the article “Symmetry and Symmetry Breaking,” by Katherine Brading and Elena Castellani, in the Stanford Encyclopedia of Philosophy:
“The old and natural idea that what is objective should not depend upon the particular perspective under which it is taken into consideration is thus reformulated in the following group-theoretical terms: what is objective is what is invariant with respect to the transformation group of reference frames, or, quoting Hermann Weyl (1952, p. 132), ‘objectivity means invariance with respect to the group of automorphisms [of space-time].‘[22]
22. The significance of the notion of invariance and its group-theoretic treatment for the issue of objectivity is explored in Born (1953), for example. For more recent discussions see Kosso (2003) and Earman (2002, Sections 6 and 7).
References:
Born, M., 1953, “Physical Reality,” Philosophical Quarterly, 3, 139-149. Reprinted in E. Castellani (ed.), Interpreting Bodies: Classical and Quantum Objects in Modern Physics, Princeton, NJ: Princeton University Press, 1998, pp. 155-167.
Earman, J., 2002, “Laws, Symmetry, and Symmetry Breaking; Invariance, Conservation Principles, and Objectivity,’ PSA 2002, Proceedings of the Biennial Meeting of the Philosophy of Science Association 2002, forthcoming [Abstract/Preprint available online]
Kosso, P., 2003, “Symmetry, objectivity, and design,” in K. Brading and E. Castellani (eds.), Symmetries in Physics: Philosophical Reflections, Cambridge: Cambridge University Press, pp. 410-421.
Weyl, H., 1952, Symmetry, Princeton, NJ: Princeton University Press.
See also
Archives Henri Poincaré (research unit UMR 7117, at Université Nancy 2, of the CNRS)–
“Minkowski, Mathematicians, and the Mathematical Theory of Relativity,” by Scott Walter, in The Expanding Worlds of General Relativity (Einstein Studies, volume 7), H. Goenner, J. Renn, J. Ritter and T. Sauer, editors, Boston/Basel: Birkhäuser, 1999, pp. 45-86–
“Developing his ideas before Göttingen mathematicians in April 1909, Klein pointed out that the new theory based on the Lorentz group (which he preferred to call ‘Invariantentheorie’) could have come from pure mathematics (1910: 19). He felt that the new theory was anticipated by the ideas on geometry and groups that he had introduced in 1872, otherwise known as the Erlangen program (see Gray 1989: 229).”
References:
Gray, Jeremy J. (1989). Ideas of Space. 2d ed. Oxford: Oxford University Press.
Klein, Felix. (1910). “Über die geometrischen Grundlagen der Lorentzgruppe.” Jahresbericht der deutschen Mathematiker-Vereinigung 19: 281-300. [Reprinted: Physikalische Zeitschrift 12 (1911): 17-27].
Related material: A pathetically garbled version of the above concepts was published in 2001 by Harvard University Press. See Invariances: The Structure of the Objective World, by Robert Nozick.
Powered by WordPress
He is an internationally recognized corporate identity consultant. Based in Hong Kong, his work for clients such as HongkongBank, IBM and Unilever is a major influence in Pacific Rim design.
