Log24

"The diagram above is from a ninth century manuscript of Apuleius' commentary on Aristotle's Perihermaneias, probably one of the oldest surviving pictures of the square."

— Edward Buckner at The Logic Museum

From the webpage "Semiotics for Beginners: Paradigmatic Analysis," by Daniel Chandler:
 

The Semiotic Square

"The structuralist semiotician Algirdas Greimas introduced the semiotic square (which he adapted from the 'logical square' of scholastic philosophy) as a means of analysing paired concepts more fully (Greimas 1987,* xiv, 49). The semiotic square is intended to map the logical conjunctions and disjunctions relating key semantic features in a text. Fredric Jameson notes that 'the entire mechanism… is capable of generating at least ten conceivable positions out of a rudimentary binary opposition' (in Greimas 1987,* xiv). Whilst this suggests that the possibilities for signification in a semiotic system are richer than the either/or of binary logic, but that [sic] they are nevertheless subject to 'semiotic constraints' – 'deep structures' providing basic axes of signification."

* Greimas, Algirdas (1987): On Meaning: Selected Writings in Semiotic Theory (trans. Paul J Perron & Frank H Collins). London: Frances Pinter

Another version of the semiotic square:

Krauss says that her figure "is, of course, a Klein Group."

Here is a more explicit figure representing the Klein group:

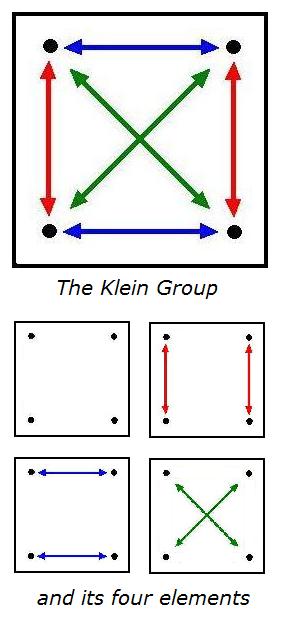
There is also the logical
    diamond of opposition —

A semiotic (as opposed to logical)
diamond has been used to illustrate
remarks by Fredric Jameson,
 a Marxist literary theorist:

"Introduction to Algirdas Greimas, Module on the Semiotic Square," by Dino Felluga at Purdue University–   The semiotic square has proven to be an influential concept not only in narrative theory but in the ideological criticism of Fredric Jameson, who uses the square as "a virtual map of conceptual closure, or better still, of the closure of ideology itself" ("Foreword"* xv). (For more on Jameson, see the [Purdue University] Jameson module on ideology.) Greimas' schema is useful since it illustrates the full complexity of any given semantic term (seme). Greimas points out that any given seme entails its opposite or "contrary." "Life" (s1) for example is understood in relation to its contrary, "death" (s2). Rather than rest at this simple binary opposition (S), however, Greimas points out that the opposition, "life" and "death," suggests what Greimas terms a contradictory pair (-S), i.e., "not-life" (-s1) and "not-death" (-s2). We would therefore be left with the following semiotic square (Fig. 1):   As Jameson explains in the Foreword to Greimas' On Meaning, "-s1 and -s2"—which in this example are taken up by "not-death" and "not-life"—"are the simple negatives of the two dominant terms, but include far more than either: thus 'nonwhite' includes more than 'black,' 'nonmale' more than 'female'" (xiv); in our example, not-life would include more than merely death and not-death more than life.   * Jameson, Fredric. "Foreword." On Meaning: Selected Writings in Semiotic Theory. By Algirdas Greimas. Trans. Paul J. Perron and Frank H. Collins. Minneapolis: U of Minnesota P, 1976.

 

"The Game in the Ship cannot be approached as a job, a vocation, a career, or a recreation. To the contrary, it is Life and Death itself at work there. In the Inner Game, we call the Game Dhum Welur, the Mind of God."

— The Gameplayers of Zan, by M.A. Foster

"For every kind of vampire,
there is a kind of cross."
— Thomas Pynchon,
 Gravity's Rainbow

Crosses used by semioticians
to baffle their opponents
are illustrated above.

Some other kinds of crosses,
and another kind of opponent:

Monday, July 11, 2005 Logos for St. Benedict's Day Click on either of the logos below for religious meditations– on the left, a Jewish meditation from the Conference of Catholic Bishops; on the right, an Aryan meditation from Stormfront.org.       Both logos represent different embodiments of the "story theory" of truth, as opposed to the "diamond theory" of truth.  Both logos claim, in their own ways, to represent the eternal Logos of the Christian religion.  I personally prefer the "diamond theory" of truth, represented by the logo below. See also the previous entry (below) and the entries   of 7/11, 2003.   Sunday, July 10, 2005 Mathematics and Narrative   Click on the title for a narrative about Nikolaos K. Artemiadis,  (co-) author of   From Artemiadis's website: 1986: Elected Regular Member of the Academy of Athens 1999: Vice President of the Academy of Athens 2000: President of the Academy of Athens   "First of all, I'd like to    thank the Academy…" — Remark attributed to Plato