"…as we saw, there are two different Latin squares of order 4…."
— Peter J. Cameron, "The Shrikhande Graph," August 26, 2010
Cameron counts Latin squares as the same if they are isotopic .
Some further context for Cameron's remark—
Cover Illustration Number 1 (1976):
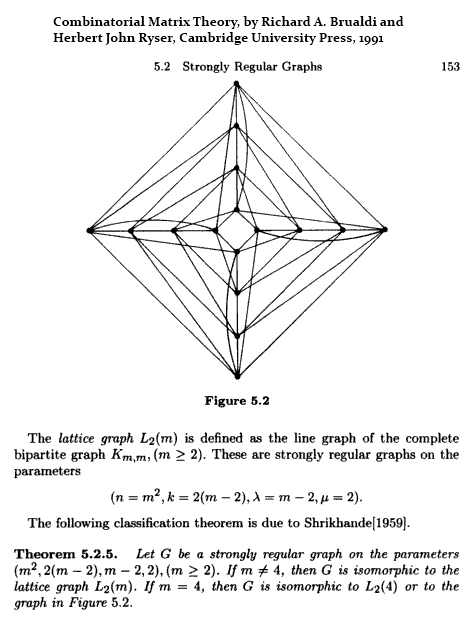
Cover Illustration Number 2 (1991):
The Shrikhande Graph
______________________________________________________________________________
This post was prompted by two remarks…
1. In a different weblog, also on August 26, 2010—
The Accidental Mathematician— "The Girl Who Played with Fermat's Theorem."
"The worst thing about the series is the mathematical interludes in The Girl Who Played With Fire….
Salander is fascinated by a theorem on perfect numbers—
one can verify it for as many numbers as one wishes, and it never fails!—
and then advances through 'Archimedes, Newton, Martin Gardner,*
and a dozen other classical mathematicians,' all the way to Fermat’s last theorem."
2. "The fact that the pattern retains its symmetry when you permute the rows and columns
is very well known to combinatorial theorists who work with matrices."
[My italics; note resemblance to the Brualdi-Ryser title above.]
–Martin Gardner in 1976 on the diamond theorem
* Compare Eric Temple Bell (as quoted at the MacTutor history of mathematics site)—
"Archimedes, Newton, and Gauss, these three, are in a class by themselves
among the great mathematicians, and it is not for ordinary mortals
to attempt to range them in order of merit."
This is from the chapter on Gauss in Men of Mathematics .