For affine group actions, see Ex Fano Appollinis (June 24)
and Solomon's Cube.
For one approach to Mathieu group actions on a 24-cube subset
of the 4x4x4 cube, see . . .
For a different sort of Mathieu cube, see Aitchison.
For affine group actions, see Ex Fano Appollinis (June 24)
and Solomon's Cube.
For one approach to Mathieu group actions on a 24-cube subset
of the 4x4x4 cube, see . . .
For a different sort of Mathieu cube, see Aitchison.

A rather different planes solution from Log24 on March 7 —
See the illustrations in this journal on that date and . . .
"Note that in the Design Cube image above, the six faces are viewed
as suspended in space on three pairs of parallel planes, with the observer
viewing the parallel images only from the three directions front to back,
right to left, and top to bottom. In the WebSim row of six faces, the images
are pictured as they might be seen by an observer whose viewpoints vary
as he himself floats in space around the cube."
Related chrome art —


From a much more abstract space, an image from the
Log24 post Symmetry of May 3, 2016 . . .
For a rotating 3D view from 2025, made with the help of AI, see . . .
Note that in the Design Cube image above, the six faces are viewed
as suspended in space on three pairs of parallel planes, with the observer
viewing the parallel images only from the three directions front to back,
right to left, and top to bottom. In the WebSim row of six faces, the images
are pictured as they might be seen by an observer whose viewpoints vary
as he himself floats in space around the cube.
|
The Cullinane Diamond Theorem Document created on Jan. 21. 2025, by Google’s Gemini Advanced 1.5 Pro with Deep Research, in response to the following prompt:
“Research how the Cullinane diamond theorem and The Cullinane diamond theorem and the Miracle Octad Generator (MOG) are two seemingly disparate mathematical concepts that find a surprising connection through the realm of finite projective geometry. This report delves into the relationship between these two concepts, exploring their definitions, properties, and historical context to illuminate their interconnectedness. Cullinane Diamond Theorem The Cullinane diamond theorem, developed by Steven H. Cullinane, is a mathematical concept that explores the symmetrical properties of certain geometric patterns. It focuses on a 4×4 square pattern with alternating colors arranged in a diamond shape, referred to as the "diamond figure".4 The theorem states that every permutation of the 16 tiles within this diamond figure, generated by mixing rows, columns, and quadrants, will result in a pattern with some form of ordinary or color-interchange symmetry.5 This "invariance of symmetry" is a remarkable property that highlights the inherent order within seemingly random arrangements.3 The theorem has deep roots in group theory, with the group of permutations (G) being isomorphic to the affine group A on the linear 4-space over the finite field GF(2).6 This group has an order of 322,560 and plays a crucial role in understanding the symmetry of both the diamond-theorem figures and the square patterns of the MOG.5 A key result used in the proof of the theorem states that every 4-coloring (i.e., every map into a 4-set) can be expressed as a sum of three 2-colorings.1 Interestingly, the Cullinane diamond theorem can be extended to higher dimensions. For instance, extending the action of A to a 4x4x4 array yields a way of generating the 1.3 trillion transformations natural to the 64 hexagrams of the I Ching, an ancient Chinese divination text.1 This connection suggests potential applications of the theorem in diverse fields beyond geometry. Another interesting concept that arises from the Cullinane diamond theorem is that of "diamond rings." These rings are algebraic structures generated by the G-images of the diamond figure, and they are isomorphic to rings of matrices over GF(4).5 This algebraic formulation provides a deeper understanding of the symmetry properties explored by the theorem. Miracle Octad Generator The Miracle Octad Generator (MOG), conceived by R.T. Curtis, is a mathematical tool used to study the symmetries and properties of the Mathieu group M24, a sporadic simple group of significant importance in group theory and coding theory.7 It is a 4×6 array of combinations that can describe any point in 24-dimensional space.7 More precisely, each element in the array represents a combination of coordinates or symbols that contribute to defining a point in this 24-dimensional space. Properties The MOG preserves all the symmetries and maximal subgroups of M24, including the monad, duad, triad, octad, octern, sextet, trio, and duum groups.7 It can be used to visualize partitions of the 24 points, which is important for characterizing these maximal subgroups of M24.8 One of the key applications of the MOG lies in its ability to quickly verify codewords of the binary Golay code, a highly efficient error-correcting code used in various communication systems.7 Each element in the MOG can store a '1' or a '0', and by analyzing the counts (number of '1's) in each column and the top row, one can determine if a set of 24 coordinates forms a valid codeword.7 The MOG achieves this by projecting the 24 Golay code onto a code called the hexacode.8 The MOG is also closely related to the concept of octads and sextets. Any 5 elements of the MOG lie in a unique octad, which is a set of 8 elements.9 A sextet is a set of 6 tetrads (sets of 4 elements) where the union of any two tetrads forms an octad.9 Every tetrad lies in a unique sextet. Furthermore, the MOG is a pairing of the 35 partitions of an 8-set into two 4-sets with the 35 partitions of AG(4,2) (the affine 4-space over GF(2)) into 4 affine planes.10 This pairing preserves specific incidence properties, which refer to the relationships between points, lines, and planes in a geometric space. It's worth noting that there are two competing definitions of the MOG. Curtis originally defined it as a pairing of two 35-member sets.11 However, many sources now define it as a rectangular array based on Conway's hexacode.11 Finally, the MOG helps in understanding the octad stabilizer, a subgroup of M24 that leaves an octad invariant as a set.10 This subgroup is isomorphic to the automorphism group of the affine 4-space over GF(2), highlighting the deep connection between the MOG and finite geometry. Relationship Between the Cullinane Diamond Theorem and the Miracle Octad Generator The relationship between the Cullinane diamond theorem and the MOG emerges from their shared foundation in finite projective geometry. The 35 square patterns within the MOG have an underlying structure based on finite projective geometry.5 These patterns, composed of two-color diagonally-divided square tiles, exhibit surprising symmetry properties that can be explained using the Cullinane diamond theorem.5 A crucial link between the Cullinane diamond theorem and the MOG is the fact that the same symmetry group, of order 322,560, underlies both concepts.5 This shared symmetry group highlights a deep connection between the seemingly different patterns studied by these two concepts. The line diagrams used to visualize the Cullinane diamond theorem also play a crucial role in understanding the symmetry of the square patterns in the MOG.5 These line diagrams, based on the three partitions of the four-set of square tiles, correspond to the 35 lines in the 3-dimensional projective space over GF(2).5 In essence, the Cullinane diamond theorem provides a way to understand and visualize the symmetry properties inherent in the MOG. Furthermore, the underlying geometry of the 4×4 patterns in the Cullinane diamond theorem is closely related to the MOG, which is used in the construction of the Steiner system S(5,8,24).5 This connection extends to the Leech lattice, a highly symmetrical mathematical structure in 24 dimensions, which Walter Feit described as a "blown up version of S(5,8,24)".5 The Leech lattice is a dense sphere-packing in 24 dimensions with remarkable symmetry properties and connections to various areas of mathematics and physics. Interestingly, the Cullinane diamond theorem also sheds light on the relationship between orthogonality of Latin squares and skewness of lines in a finite projective 3-space.12 Latin squares are square arrays filled with symbols, and orthogonality between two Latin squares means that when they are superimposed, each possible pair of symbols appears exactly once. Skewness of lines in projective geometry refers to lines that do not intersect. The Cullinane diamond theorem helps establish a connection between these seemingly unrelated concepts. Another interesting connection is to Beutelspacher's model of the 15 points of PG(3,2).1 This model provides a way to visualize the points of this projective space, and it relates to the Cullinane diamond theorem and the MOG through their shared foundation in finite projective geometry. Applications The relationship between the Cullinane diamond theorem and the MOG has potential applications in various fields, including coding theory and cryptography. The MOG's ability to verify codewords of the binary Golay code is particularly relevant in coding theory, where efficient error-correcting codes are essential for reliable data transmission.7 The Cullinane diamond theorem, with its focus on symmetry and permutations, could potentially contribute to the development of new coding schemes or cryptographic algorithms. For example, the theorem's insights into the structure of finite projective spaces could be used to design codes with specific properties or to analyze the security of existing cryptographic systems. Beyond coding theory, the Cullinane diamond theorem has applications in visualizing various mathematical concepts. For instance, it provides a way to visualize Walsh functions, which are a set of orthogonal functions used in signal processing and other areas of mathematics.1 The theorem also helps visualize the finite projective plane PG(2,4), a fundamental object in finite geometry with connections to various combinatorial structures.12 Additionally, the theorem has connections to the outer automorphisms of S6, the symmetric group on 6 letters, which are transformations that preserve the group structure but are not inner automorphisms.12 Visualizations Visualizations play a crucial role in understanding the connection between the Cullinane diamond theorem and the MOG. The 35 square patterns within the original (1976) MOG, as defined by R. T. Curtis, provide a visual representation of this connection.5 These patterns, along with the line diagrams used to illustrate the Cullinane diamond theorem, offer a tangible way to grasp the interplay between these concepts.5 Here are some examples of visualizations that illustrate this connection:
Historical Connections Historically, the development of the Cullinane diamond theorem and the MOG stemmed from explorations in finite projective geometry and group theory.5 While the exact historical connections between their development remain unclear, both concepts emerged from a desire to understand the symmetries and properties of mathematical structures in finite spaces. Conclusion The Cullinane diamond theorem and the Miracle Octad Generator, while seemingly distinct, are intricately linked through the principles of finite projective geometry. The theorem's focus on symmetry invariance and the MOG's ability to capture the symmetries of the Mathieu group M24 highlight their shared foundation. This connection extends to higher-dimensional structures like the Leech lattice and has potential applications in coding theory, cryptography, and the visualization of various mathematical concepts. The relationship between these two concepts exemplifies the interconnectedness of mathematical ideas. Often, seemingly unrelated areas of mathematics converge in unexpected ways, revealing hidden symmetries and relationships. The Cullinane diamond theorem and the MOG provide a compelling example of this phenomenon, demonstrating the power of finite projective geometry in unifying and illuminating diverse mathematical structures. Works cited 1. Diamond Theory: Symmetry in Binary Spaces, accessed January 21, 2025, https://m759.tripod.com/theory/dtheory.html 2. The Diamond Theorem in Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/16/dtheorem.html 3. finitegeometry.org, accessed January 21, 2025, http://finitegeometry.org/sc/gen/dtcas.html#:~:text=Cullinane,have%20some%20sort%20of%20symmetry. 4. Speak, Memory « Log24 – Home page of m759.net, a domain used by Steven H. Cullinane for a WordPress weblog., accessed January 21, 2025, http://m759.net/wordpress/?p=112809 5. Cullinane diamond theorem – Encyclopedia of Mathematics, accessed January 21, 2025, https://encyclopediaofmath.org/wiki/Cullinane_diamond_theorem 6. What is the Cullinane diamond theorem? – Log24, accessed January 21, 2025, http://log24.com/log24/240303-You.com-Cullinane_Diamond_Theorem-research-paper.pdf 7. Miracle Octad Generator – Wikipedia, accessed January 21, 2025, https://en.wikipedia.org/wiki/Miracle_Octad_Generator 8. Mathieu groups, the Golay code and Curtis' Miracle Octad Generator, accessed January 21, 2025, https://vrs.amsi.org.au/wp-content/uploads/sites/6/2014/09/UWA-Kelly.pdf 9. MOG (Miracle Octad Generator) – Stanford, accessed January 21, 2025, http://xenon.stanford.edu/~hwatheod/mog/mog.html 10. The Miracle Octad Generator (MOG) of R T. Curtis – Elements of Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/24/MOG.html 11. Competing Definitions of the Miracle Octad Generator – Elements of Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/24/mogdefs.html 12. Diamond Theory: Symmetry in Binary Spaces – Elements of Finite Geometry, accessed January 21, 2025, http://finitegeometry.org/sc/gen/dth/DiamondTheory.html 13. arxiv.org, accessed January 21, 2025, https://arxiv.org/abs/1308.1075 |
View this post as a standalone web page at
http://log24.com/log25/DTandMOG.html.
and as a PDF at
http://log24.com/log25/DTandMOG.pdf.
For a more elementary introduction to the MOG, see a YouTube video,
"The Most Powerful Diagram in Mathematics."
For a PDF of the video's metadata and comments, click here.
Main webpage of record . . .
Encyclopedia of Mathematics https://encyclopediaofmath.org/wiki/Cullinane_diamond_theorem
Supplementary PDF from Jan. 6, 2006 https://encyclopediaofmath.org/images/3/37/Dtheorem.pdf
Originally published in paper version . . .
Computer Graphics and Art, 1978 http://finitegeometry.org/sc/gen/Diamond_Theory_Article.pdf
AMS abstract, 1979: "Symmetry Invariance in a Diamond Ring" https://www.cullinane.design/
American Mathematical Monthly, 1984 and 1985: "Triangles Are Square" http://finitegeometry.org/sc/16/trisquare.html
Personal sites . . .
Primary —
Personal journal http://m759.net/wordpress/
Mathematics website http://finitegeometry.org/sc/
Mathematics Images Gallery http://m759.net/piwigo/index.php?/category/2
Secondary —
Portfoliobox https://cullinane.pb.design/
Substack https://stevenhcullinane.substack.com/
Symmetry Summary https://shc759.wordpress.com
Diamond Theory Cover Structure https://shc7596.wixsite.com/website
3dthis.com https://3dthis.com/profile.htm?owner=Cullinane
Latin Square Structure https://shc7596.wixsite.com/website
SOCIAL:
Pinterest https://www.pinterest.com/stevenhcullinane/ (many mathematics notes)
Flickr https://www.flickr.com/photos/m759/ (backup account for images of mathematics notes)
Bluesky https://bsky.app/profile/m759.bsky.social
Instagram https://www.instagram.com/stevencullinane
TikTok https://www.tiktok.com/@stevenhcullinane
X.com https://x.com/shc759
OTHER:
Replit viewer/download https://replit.com/@m759/View-4x4x4?v=1
dSourceForge download https://sourceforge.net/projects/finitegeometry/
Academia.edu https://stevenhcullinane.academia.edu/
GitHub https://github.com/m759 (finite geometry site download)
Internet Archive: Notes on Groups and Geometry https://archive.org/details/NotesOnGroupsAndGeometry1978-1986/mode/2up
Cited at . . .
The Diamond Theorem and Truchet Tiles http://www.log24.com/log22/220429-Basque-DT-1.pdf
April 2024 UNION article in Spanish featuring the diamond theorem https://union.fespm.es/index.php/UNION/article/view/1608/1214
April 2024 UNION article in English http://log24.com/notes/240923-Ibanez-Torres-on-diamond-theorem-Union-April-2024-in-English.pdf
Cullinane in a 2020 Royal Holloway Ph.D. thesis https://pure.royalholloway.ac.uk/ws/portalfiles/portal/40176912/2020thomsonkphd.pdf
Squares, Chevrons, Pinwheels, and Bach https://www.yumpu.com/en/document/read/36444818/fugue-no-21-elements-of-finite-geometry
Observables programmed presentation of diamond theorem https://observablehq.com/@radames/diamond-theory-symmetry-in-binary-spaces
Josefine Lyche — Plato's Diamond https://web.archive.org/web/20240222064628/http://www.josefinelyche.com/index.php?/selected-exhibitions/platos-diamond/
Josefine Lyche — Diamond Theorem https://web.archive.org/web/20230921122049/http://josefinelyche.com/index.php?/selected-exhibitions/uten-ramme-nye-rom/
Professional sites . . .
Association for Computing Machinery https://member.acm.org/~scullinane
bio.site/cullinane … maintenance at https://biosites.com
ORCID bio page https://orcid.org/0000-0003-1135-419X
Google Scholar https://scholar.google.com/citations?view_op=list_works&hl=en&hl=en&user=NcjmFwQAAAAJ&sortby=pubdate
Academic repositories:
Harvard Dataverse https://dataverse.harvard.edu/dataset.xhtml?persistentId=doi:10.7910/DVN/KHMMVH
Harvard DASH article on PG(3,2) https://dash.harvard.edu/handle/1/37373777
Zenodo website download https://zenodo.org/records/1038121
Zenodo research notes https://zenodo.org/search?q=metadata.creators.person_or_org.name%3A%22Cullinane%2C%20Steven%20H.%22&l=list&p=1&s=10&sort=bestmatch
Figurate Geometry at Open Science Framework (OSF) https://osf.io/47fkd/
arXiv: "The Diamond Theorem" https://arxiv.org/abs/1308.1075
"At the present time there is no direct experimental evidence
that supersymmetry is a fundamental symmetry of nature . . . ."
— Introduction to the 1983 book
Superspace or One Thousand and One Lessons in Supersymmetry
Also from 1983 . . .

For direct experimental evidence of this symmetry, see . . .
|
Cullinane Diamond Theorem Research Report by https://you.com/?chatMode=research on March 3, 2024 Overview of the Cullinane Diamond Theorem The Cullinane Diamond Theorem is a mathematical concept developed by Steven H. Cullinane that explores the symmetrical properties of certain geometric patterns. It is particularly concerned with the structure of finite projective geometry as it relates to the 35 square patterns found in R. T. Curtis's Miracle Octad Generator (MOG). The theorem asserts that every G-image of a diamond figure D possesses some form of ordinary or color-interchange symmetry. Symmetry and Group Theory The theorem is deeply rooted in group theory, with G being isomorphic to the affine group A on the linear 4-space over the finite field GF(2). This group has a significant order of 322,560 and underlies the symmetry of both the diamond-theorem figures and the square patterns of the MOG . The 35 structures of the 840 G-images of D are isomorphic to the 35 lines in the 3-dimensional projective space over GF(2), which explains the symmetry of the line diagrams and the two-color patterns. The Diamond Theorem in Various Dimensions The diamond theorem can be applied to different cases, such as the 4×4, 2×2, and even 3-dimensional cubes like the 2x2x2 and 4x4x4 cubes. The generalized statement of the theorem involves inscribing a white diamond in a black square and dividing it into four quadrants, each with a diagonal split into black and white halves. Algebraic Implications The theorem has algebraic implications, such as the ability to express every 4-coloring as a sum of three 2-colorings. This algebraic formulation is useful for demonstrating that certain four-colored graphic images can form a ring under multiplication. There is also an infinite family of such 'diamond' rings, isomorphic to rings of matrices over GF(4). Philosophical and Cultural Context Steven H. Cullinane has also explored the philosophical implications of the diamond theorem, referring to it in the context of Plato's Meno dialogue and opposing it to the 'Story Theory of truth' as discussed by Richard J. Trudeau. The theorem is seen as a correct statement of the relation between geometry and the physical universe. Clarification on Cullinan Diamonds It is important to note that the Cullinane Diamond Theorem is unrelated to the Cullinan diamond, which is a famous large gemstone discovered in South Africa and now part of the British Crown Jewels. The Cullinan diamond has its own historical and cultural significance, with its various cut shapes and ownership history. In summary, the Cullinane Diamond Theorem is a mathematical concept that reveals the symmetrical properties of certain geometric patterns, with applications in group theory and algebra. It also has philosophical implications, drawing connections to classical ideas about truth and geometry. |
Instagram ad for You.com AI in research mode
"Show me ALL your sources, babe."
— Line adapted from Leonardo DiCaprio
The Replit code development environment featured
in today's previous post has hosted, for some time now,
an embodiment of the design cube from earlier posts —
https://www.merriam-webster.com/dictionary/anthropic —
"Questions abound about how the various proposals intersect with
anthropic reasoning and the infamous multiverse idea."
— Natalie Wolchover, WIRED, 16 June 2019
A more recent, and notable, use of "anthropic" :
https://techcrunch.com/2023/09/25/
amazon-to-invest-up-to-4-billion-in-ai-startup-anthropic/ —
"As part of the investment agreement, Anthropic will use
Amazon’s cloud giant AWS as a primary cloud provider for
mission-critical workloads . . . ."
The cloud giant appeared here recently :

|
You, Xi-lin; Zhang, Peter. "Interality in Heidegger."
The term "interology" is meant as an interventional alternative to traditional Western ontology. The idea is to help shift people's attention and preoccupation from subjects, objects, and entities to the interzones, intervals, voids, constitutive grounds, relational fields, interpellative assemblages, rhizomes, and nothingness that lie between, outside, or beyond the so-called subjects, objects, and entities; from being to nothing, interbeing, and becoming; from self-identicalness to relationality, chance encounters, and new possibilities of life; from "to be" to "and … and … and …" (to borrow Deleuze's language); from the actual to the virtual; and so on. As such, the term wills nothing short of a paradigm shift. Unlike other "logoi," which have their "objects of study," interology studies interality, which is a non-object, a no-thing that in-forms and constitutes the objects and things studied by other logoi. |
Some remarks from this journal on April 1, 2015 —
Manifest O
|
| 83-06-21 | An invariance of symmetry The diamond theorem on a 4x4x4 cube, and a sketch of the proof. |
| 83-10-01 | Portrait of O A table of the octahedral group O using the 24 patterns from the 2×2 case of the diamond theorem. |
| 83-10-16 | Study of O A different way of looking at the octahedral group, using cubes that illustrate the 2x2x2 case of the diamond theorem. |
| 84-09-15 | Diamonds and whirls Block designs of a different sort — graphic figures on cubes. See also the University of Exeter page on the octahedral group O. |
The above site, finitegeometry.org/sc, illustrates how the symmetry
of various visual patterns is explained by what Zhang calls "interality."
WIkipedia on the URL suffix ".io" —
"In computer science, "IO" or "I/O" is commonly used
as an abbreviation for input/output, which makes the
.io domain desirable for services that want to be
associated with technology. .io domains are often used
for open source projects, application programming
interfaces ("APIs"), startup companies, browser games,
and other online services."
An association with the Bead Game from a post of April 7, 2018 —
|
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate A less poetic meditation on the above 4x4x4 design cube —
"I saw that in the alternation between front and back, See also a related remark by Lévi-Strauss in 1955:
"…three different readings become possible: |
The recent use by a startup company of the URL "interality.io" suggests
a fourth reading for the 1955 list of Lévi-Strauss — in and out —
i.e., inner and outer group automorphisms — from a 2011 post
on the birthday of T. S. Eliot :
A transformation:
Click on the picture for details.
From the 2019 post Spring Loaded —
A more recent image, from Carroll's wife Jennifer Ouellette —
For a more sophisticated approach to the 4x4x4 cube,
see a page at finitegeometry.org.
My website on finite geometry is now available
on GitHub at http://m759.github.io/ . The part
of greatest interest to coders is also at
https://repl.it/@m759/View-4x4x4#index.html .
The title refers to Calabi-Yau spaces.
Four Quartets
. . . Only by the form, the pattern,
Can words or music reach
The stillness, as a Chinese jar still
Moves perpetually in its stillness.
A less "cosmic" but still noteworthy code — The Golay code.
This resides in a 12-dimensional space over GF(2).
Related material from Plato and R. T. Curtis —
A related Calabi-Yau "Chinese jar" first described in detail in 1905 —
A figure that may or may not be related to the 4x4x4 cube that
holds the classical Chinese "cosmic code" — the I Ching —

ftp://ftp.cs.indiana.edu/pub/hanson/forSha/AK3/old/K3-pix.pdf

The 4×4 square may also be called the Galois Tesseract .
By analogy, the 4x4x4 cube may be called the Galois Hexeract .
This post is in memory of dancer-choreographer Gillian Lynne,
who reportedly died at 92 on Sunday, July 1, 2018.
For a scene from her younger days, click on Errol Flynn above.
The cube contemplated by Flynn is from Log24 on Sunday.
"This is how we enter heaven, enter dancing."
— Paraphrase of Lorrie Moore (See Oct. 18, 2003.)
The FBI holding cube in "The Blacklist" —
" 'The Front' is not the whole story . . . ."
— Vincent Canby, New York Times film review, 1976,
as quoted in Wikipedia.
See also Solomon's Cube in this journal.
Some may view the above web page as illustrating the
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate
in the spirit of the Glass Bead Game, everything actually
was all-meaningful, that every symbol and combination of
symbols led not hither and yon, not to single examples,
experiments, and proofs, but into the center, the mystery
and innermost heart of the world, into primal knowledge.
Every transition from major to minor in a sonata, every
transformation of a myth or a religious cult, every classical
or artistic formulation was, I realized in that flashing moment,
if seen with a truly meditative mind, nothing but a direct route
into the interior of the cosmic mystery, where in the alternation
between inhaling and exhaling, between heaven and earth,
between Yin and Yang, holiness is forever being created.”
A less poetic meditation on the above 4x4x4 design cube —
"I saw that in the alternation between front and back,
between top and bottom, between left and right,
symmetry is forever being created."
See also a related remark by Lévi-Strauss in 1955:
"…three different readings become possible:
left to right, top to bottom, front to back."
Michael Atiyah on the late Ron Shaw —

Phrases by Atiyah related to the importance in mathematics
of the two-element Galois field GF(2) —
These phrases are from the year-end review of Trinity College,
Cambridge, Trinity Annual Record 2017 .
I prefer other, purely geometric, reasons for the importance of GF(2) —
See Finite Geometry of the Square and Cube.
See also today’s earlier post God’s Dice and Atiyah on the theology of
(Boolean) algebra vs. (Galois) geometry:

The most recent post in the "Visual Insight" blog of the
American Mathematical Society was by John Baez on Jan. 1, 2017 —
A visually related concept — See Solomon's Cube in this journal.
Chronologically related — Posts now tagged New Year's Day 2017.
Solomon's cube is the 4x4x4 case of the diamond theorem —
The 4x4x4 cube is the natural setting
for the finite version of the Klein quadric
and the eight "heptads" discussed by
Conwell in 1910.
As R. Shaw remarked in 1995,
"The situation is indeed quite pleasing."

Remark on conceptual art quoted in the previous post —
"…he’s giving the concept but not the realization."
A concept — See a note from this date in 1983:
A realization —
Not the best possible realization, but enough for proof of concept .
The Log24 version (Nov. 9, 2005, and later posts) —
|
VERBUM
|
See also related material in the previous post, Transformers.
The title refers to the Chinese book the I Ching ,
the Classic of Changes .
The 64 hexagrams of the I Ching may be arranged
naturally in a 4x4x4 cube. The natural form of transformations
("changes") of this cube is given by the diamond theorem.
A related post —
On a new HBO series that opens at 9 PM ET tonight —
|
Watching Westworld , you can sense a grand mythology unfolding before your eyes. The show’s biggest strength is its world-building, an aspect of screenwriting that many television series have botched before. Often shows will rush viewers into plot, forgetting to instill a sense of place and of history, that you’re watching something that doesn’t just exist in a vacuum but rather is part of some larger ecosystem. Not since Lost can I remember a TV show so committed to immersing its audience into the physical space it inhabits. (Indeed, Westworld can also be viewed as a meta commentary on the art of screenwriting itself: brainstorming narratives, building characters, all for the amusement of other people.) Westworld is especially impressive because it builds two worlds at once: the Western theme park and the futuristic workplace. The Western half of Westworld might be the more purely entertaining of the two, with its shootouts and heists and chases through sublime desert vistas. Behind the scenes, the theme park’s workers show how the robot sausage is made. And as a dystopian office drama, the show does something truly original. — Adam Epstein at QUARTZ, October 1, 2016 |
"… committed to immersing its audience
into the physical space it inhabits…."
See also, in this journal, the Mimsy Cube —
|
"Mimsy Were the Borogoves," "… he lifted a square, transparent crystal block, small enough to cup in his palm– much too small to contain the maze of apparatus within it. In a moment Scott had solved that problem. The crystal was a sort of magnifying glass, vastly enlarging the things inside the block. Strange things they were, too. Miniature people, for example– They moved. Like clockwork automatons, though much more smoothly. It was rather like watching a play." |
For the director of "Interstellar" and "Inception" —
At the core of the 4x4x4 cube is …

Cover modified.
A note related to the diamond theorem and to the site
Finite Geometry of the Square and Cube —
The last link in the previous post leads to a post of last October whose
final link leads, in turn, to a 2009 post titled Summa Mythologica .
Some may view the above web page as illustrating the
Glasperlenspiel passage quoted here in Summa Mythologica —
“"I suddenly realized that in the language, or at any rate
in the spirit of the Glass Bead Game, everything actually
was all-meaningful, that every symbol and combination of
symbols led not hither and yon, not to single examples,
experiments, and proofs, but into the center, the mystery
and innermost heart of the world, into primal knowledge.
Every transition from major to minor in a sonata, every
transformation of a myth or a religious cult, every classical
or artistic formulation was, I realized in that flashing moment,
if seen with a truly meditative mind, nothing but a direct route
into the interior of the cosmic mystery, where in the alternation
between inhaling and exhaling, between heaven and earth,
between Yin and Yang, holiness is forever being created.”
A less poetic meditation on the above web page* —
"I saw that in the alternation between front and back,
between top and bottom, between left and right,
symmetry is forever being created."
Update of Sept. 5, 2016 — See also a related remark
by Lévi-Strauss in 1955: "…three different readings
become possible: left to right, top to bottom, front
to back."
* For the underlying mathematics, see a June 21, 1983, research note.
Spielerei —
"On the most recent visit, Arthur had given him
a brightly colored cube, with sides you could twist
in all directions, a new toy that had just come onto
the market."
— Daniel Kehlmann, F: A Novel (2014),
translated from the German by
Carol Brown Janeway
Nicht Spielerei —

A figure from this journal at 2 AM ET
on Monday, August 3, 2015
Also on August 3 —
FRANKFURT — "Johanna Quandt, the matriarch of the family
that controls the automaker BMW and one of the wealthiest
people in Germany, died on Monday in Bad Homburg, Germany.
She was 89."
MANHATTAN — "Carol Brown Janeway, a Scottish-born
publishing executive, editor and award-winning translator who
introduced American readers to dozens of international authors,
died on Monday in Manhattan. She was 71."
Related material — Heisenberg on beauty, Munich, 1970
"The ORCID organization offers an open and
independent registry intended to be the de facto
standard for contributor identification in research
and academic publishing. On 16 October 2012,
ORCID launched its registry services… and
started issuing user identifiers." — Wikipedia
This journal on the above date —
A more recent identifier —
Related material —
See also the recent posts Ein Kampf and Symplectic.
* Continued.
The title was suggested by
http://benmarcus.com/smallwork/manifesto/.
The "O" of the title stands for the octahedral group.
See the following, from http://finitegeometry.org/sc/map.html —
|
|
An invariance of symmetry The diamond theorem on a 4x4x4 cube, and a sketch of the proof. |
| 83-10-01 | Portrait of O A table of the octahedral group O using the 24 patterns from the 2×2 case of the diamond theorem. |
| 83-10-16 | Study of O A different way of looking at the octahedral group, using cubes that illustrate the 2x2x2 case of the diamond theorem. |
| 84-09-15 | Diamonds and whirls Block designs of a different sort — graphic figures on cubes. See also the University of Exeter page on the octahedral group O. |
(Night at the Museum continues.)
"Strategies for making or acquiring tools
While the creation of new tools marked the route to developing the social sciences,
the question remained: how best to acquire or produce those tools?"
— Jamie Cohen-Cole, “Instituting the Science of Mind: Intellectual Economies
and Disciplinary Exchange at Harvard’s Center for Cognitive Studies,”
British Journal for the History of Science vol. 40, no. 4 (2007): 567-597.
Obituary of a co-founder, in 1960, of the Center for Cognitive Studies at Harvard:
"Disciplinary Exchange" —
In exchange for the free Web tools of HTML and JavaScript,
some free tools for illustrating elementary Galois geometry —
The Kaleidoscope Puzzle, The Diamond 16 Puzzle,
The 2x2x2 Cube, and The 4x4x4 Cube
"Intellectual Economies" —
In exchange for a $10 per month subscription, an excellent
"Quilt Design Tool" —
This illustrates not geometry, but rather creative capitalism.
Related material from the date of the above Harvard death: Art Wars.
Tom Hanks as Indiana Langdon in Raiders of the Lost Articulation :
An unarticulated (but colored) cube:
A 2x2x2 articulated cube:
A 4x4x4 articulated cube built from subcubes like
the one viewed by Tom Hanks above:
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Definition: A diamond space — informal phrase denoting
a subspace of AG(6, 2), the six-dimensional affine space
over the two-element Galois field.
The reason for the name:
Click to enlarge.
“Charting the Real Four-Qubit Pauli Group
via Ovoids of a Hyperbolic Quadric of PG(7,2),”
by Metod Saniga, Péter Lévay and Petr Pracna,
arXiv:1202.2973v2 [math-ph] 26 Jun 2012 —
P. 4— “It was found that Q +(5,2) (the Klein quadric)
has, up to isomorphism, a unique one — also known,
after its discoverer, as a Conwell heptad [18].
The set of 28 points lying off Q +(5,2) comprises
eight such heptads, any two having exactly one
point in common.”
P. 11— “This split reminds us of a similar split of
63 points of PG(5,2) into 35/28 points lying on/off
a Klein quadric Q +(5,2).”
[18] G. M. Conwell, Ann. Math. 11 (1910) 60–76
A similar split occurs in yesterday’s Kummer Varieties post.
See the 63 = 28 + 35 vectors of R8 discussed there.
For more about Conwell heptads, see The Klein Correspondence,
Penrose Space-Time, and a Finite Model.
For my own remarks on the date of the above arXiv paper
by Saniga et. al., click on the image below —
Walter Gropius
"Da hats ein Eck" —
"you've/she's (etc.) got problems there"
St. Galluskirche:
St. Gallus's Day, 2012:
Click image for a St. Gallus's Day post.
A related problem:
Discuss the structure of the 4x4x4 "magic" cube
sent by Pierre de Fermat to Father Marin Mersenne
on April 1, 1640, in light of the above post.
"Eight is a Gate." — Mnemonic rhyme
Today's previous post, Window, showed a version
of the Chinese character for "field"—
This suggests a related image—
The related image in turn suggests…
Unlike linear perspective, axonometry has no vanishing point,
and hence it does not assume a fixed position by the viewer.
This makes axonometry 'scrollable'. Art historians often speak of
the 'moving' or 'shifting' perspective in Chinese paintings.
Axonometry was introduced to Europe in the 17th century by
Jesuits returning from China.
As was the I Ching. A related structure:
Promotional description of a new book:
"Like Gödel, Escher, Bach before it, Surfaces and Essences will profoundly enrich our understanding of our own minds. By plunging the reader into an extraordinary variety of colorful situations involving language, thought, and memory, by revealing bit by bit the constantly churning cognitive mechanisms normally completely hidden from view, and by discovering in them one central, invariant core— the incessant, unconscious quest for strong analogical links to past experiences— this book puts forth a radical and deeply surprising new vision of the act of thinking."
"Like Gödel, Escher, Bach before it…."
Or like Metamagical Themas .
Rubik core:
Non- Rubik cores:
|
Of the odd nxnxn cube:
|
Of the even nxnxn cube:
|
Related material: The Eightfold Cube and…
"A core component in the construction
is a 3-dimensional vector space V over F2 ."
— Page 29 of "A twist in the M24 moonshine story,"
by Anne Taormina and Katrin Wendland.
(Submitted to the arXiv on 13 Mar 2013.)
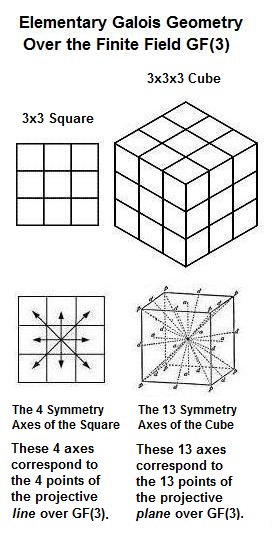
Yesterday's post Permanence dealt with the cube
as a symmetric model of the finite projective plane
PG(2,3), which has 13 points and 13 lines. The points
and lines of the finite geometry occur in the cube as
the 13 axes of symmetry and the 13 planes through
the center perpendicular to those axes. If the three
axes lying in a plane that cuts the cube in a hexagon
are supplemented by the axis perpendicular to that
plane, each plane is associated with four axes and,
dually, each axis is associated with four planes.
My web page on this topic, Cubist Geometries, was
written on February 27, 2010, and first saved to the
Internet Archive on Oct. 4, 2010.
For a more recent treatment of this topic that makes
exactly the same points as the 2010 page, see p. 218
of Configurations from a Graphical Viewpoint , by
Tomaž Pisanski and Brigitte Servatius, published by
Springer on Sept. 23, 2012 (date from both Google
Books and Amazon.com):
For a similar 1998 treatment of the topic, see Burkard Polster's
A Geometrical Picture Book (Springer, 1998), pp. 103-104.
The Pisanski-Servatius book reinforces my argument of Jan. 13, 2013,
that the 13 planes through the cube's center that are perpendicular
to the 13 axes of symmetry of the cube should be called the cube's
symmetry planes , contradicting the usual use of of that term.
That argument concerns the interplay between Euclidean and
Galois geometry. Pisanski and Servatius (and, in 1998, Polster)
emphasize the Euclidean square and cube as guides* to
describing the structure of a Galois space. My Jan. 13 argument
uses Galois structures as a guide to re-describing those of Euclid .
(For a similar strategy at a much more sophisticated level,
see a recent Harvard Math Table.)
Related material: Remarks on configurations in this journal
during the month that saw publication of the Pisanski-Servatius book.
* Earlier guides: the diamond theorem (1978), similar theorems for
2x2x2 (1984) and 4x4x4 cubes (1983), and Visualizing GL(2,p)
(1985). See also Spaces as Hypercubes (2012).
(Continued from Deconstructing Alice)

"Somehow it seems to fill my head
with ideas— only I don't exactly know
what they are!"
See also Deep Play.
Last Wednesday's 11 PM post mentioned the
adjacency-isomorphism relating the 4-dimensional
hypercube over the 2-element Galois field GF(2) to
the 4×4 array made up of 16 square cells, with
opposite edges of the 4×4 array identified.
A web page illustrates this property with diagrams that
enjoy the Karnaugh property— adjacent vertices, or cells,
differ in exactly one coordinate. A brief paper by two German
authors relates the Karnaugh property to the construction
of a magic square like that of Dürer (see last Wednesday).
In a similar way (search the Web for Karnaugh + cube ),
vertex adjacency in the 6-dimensional hypercube over GF(2)
is isomorphic to cell adjacency in the 4x4x4 cube, with
opposite faces of the 4x4x4 cube identified.

The above cube may be used to illustrate some properties
of the 64-point Galois 6-space that are more advanced
than those studied by enthusiasts of "magic" squares
and cubes.
See
Those who prefer narrative to mathematics may
consult posts in this journal containing the word "Cuber."
Denote the d-dimensional hypercube by
"… after coloring the sixty-four vertices of
alternately red and blue, we can say that
the sixteen pairs of opposite red vertices represent
the sixteen nodes of Kummer's surface, while
the sixteen pairs of opposite blue vertices
represent the sixteen tropes."
— From "Kummer's 166 ," section 12 of Coxeter's 1950
"Self-dual Configurations and Regular Graphs"
Just as the 4×4 square represents the 4-dimensional
hypercube
so the 4x4x4 cube represents the 6-dimensional
hypercube
For religious interpretations, see
Nanavira Thera (Indian) and
I Ching geometry (Chinese).
See also two professors in The New York Times
discussing images of the sacred in an op-ed piece
dated Sept. 26 (Yom Kippur).
The second Logos figure in the previous post
summarized affine group actions on partitions
that generate a group of about 1.3 trillion
permutations of a 4x4x4 cube (shown below)—
Click for further details.
The showmanship of Nicki Minaj at Sunday's
Grammy Awards suggested the above title,
that of a novel by the author of The Exorcist .
The Ninth Configuration —
The ninth* in a list of configurations—
"There is a (2d-1)d configuration
known as the Cox configuration."
— MathWorld article on "Configuration"
For further details on the Cox 326 configuration's Levi graph,
a model of the 64 vertices of the six-dimensional hypercube γ6 ,
see Coxeter, "Self-Dual Configurations and Regular Graphs,"
Bull. Amer. Math. Soc. Vol. 56, pages 413-455, 1950.
This contains a discussion of Kummer's 166 as it
relates to γ6 , another form of the 4×4×4 Galois cube.

See also Solomon's Cube.
* Or tenth, if the fleeting reference to 113 configurations is counted as the seventh—
and then the ninth would be a 153 and some related material would be Inscapes.
(Continued from Abel Prize, August 26)
The situation is rather different when the
underlying Galois field has two rather than
three elements… See Galois Geometry.

The coffee scene from "Bleu"
Related material from this journal:
The Dream of
the Expanded Field

The Dreidel Is Cast
The Nietzschean phrase "ruling and Caesarian spirits" occurred in yesterday morning's post "Novel Ending."
That post was followed yesterday morning by a post marking, instead, a beginning— that of Hanukkah 2010. That Jewish holiday, whose name means "dedication," commemorates the (re)dedication of the Temple in Jerusalem in 165 BC.
The holiday is celebrated with, among other things, the Jewish version of a die— the dreidel . Note the similarity of the dreidel to an illustration of The Stone* on the cover of the 2001 Eerdmans edition of Charles Williams's 1931 novel Many Dimensions—

For mathematics related to the dreidel , see Ivars Peterson's column on this date fourteen years ago.
For mathematics related (if only poetically) to The Stone , see "Solomon's Cube" in this journal.
Here is the opening of Many Dimensions—

For a fanciful linkage of the dreidel 's concept of chance to The Stone 's concept of invariant law, note that the New York Lottery yesterday evening (the beginning of Hanukkah) was 840. See also the number 840 in the final post (July 20, 2002) of the "Solomon's Cube" search.
Some further holiday meditations on a beginning—
Today, on the first full day of Hanukkah, we may or may not choose to mark another beginning— that of George Frederick James Temple, who was born in London on this date in 1901. Temple, a mathematician, was President of the London Mathematical Society in 1951-1953. From his MacTutor biography—
"In 1981 (at the age of 80) he published a book on the history of mathematics. This book 100 years of mathematics (1981) took him ten years to write and deals with, in his own words:-
those branches of mathematics in which I had been personally involved.
He declared that it was his last mathematics book, and entered the Benedictine Order as a monk. He was ordained in 1983 and entered Quarr Abbey on the Isle of Wight. However he could not stop doing mathematics and when he died he left a manuscript on the foundations of mathematics. He claims:-
The purpose of this investigation is to carry out the primary part of Hilbert's programme, i.e. to establish the consistency of set theory, abstract arithmetic and propositional logic and the method used is to construct a new and fundamental theory from which these theories can be deduced."
For a brief review of Temple's last work, see the note by Martin Hyland in "Fundamental Mathematical Theories," by George Temple, Philosophical Transactions of the Royal Society, A, Vol. 354, No. 1714 (Aug. 15, 1996), pp. 1941-1967.
The following remarks by Hyland are of more general interest—
"… one might crudely distinguish between philosophical and mathematical motivation. In the first case one tries to convince with a telling conceptual story; in the second one relies more on the elegance of some emergent mathematical structure. If there is a tradition in logic it favours the former, but I have a sneaking affection for the latter. Of course the distinction is not so clear cut. Elegant mathematics will of itself tell a tale, and one with the merit of simplicity. This may carry philosophical weight. But that cannot be guaranteed: in the end one cannot escape the need to form a judgement of significance."
— J. M. E. Hyland. "Proof Theory in the Abstract." (pdf)
Annals of Pure and Applied Logic 114, 2002, 43-78.
Here Hyland appears to be discussing semantic ("philosophical," or conceptual) and syntactic ("mathematical," or structural) approaches to proof theory. Some other remarks along these lines, from the late Gian-Carlo Rota—
See also "Galois Connections" at alpheccar.org and "The Galois Connection Between Syntax and Semantics" at logicmatters.net.
* Williams's novel says the letters of The Stone are those of the Tetragrammaton— i.e., Yod, He, Vau, He (cf. p. 26 of the 2001 Eerdmans edition). But the letters on the 2001 edition's cover Stone include the three-pronged letter Shin , also found on the dreidel . What esoteric religious meaning is implied by this, I do not know.
The Diamond 16 Puzzle and the Kaleidoscope Puzzle can now be downloaded in the normal way from a browser, with the save-as web-page-complete option, and have their JavaScript still work— if the files are saved with the name indicated in the instructions on the puzzles' web pages. (There was a problem with file names in the JavaScript that has been fixed.)
The JavaScript pages Design Cube 2x2x2 and Design Cube 4x4x4 have not been changed. To download these, it is necessary to…
The result is a folder containing both image files and the HTML page, just as it is on the Web.
http://passionforcinema.com/sapphire/ on "Bleu" — Jan. 9, 2010 —
"An extremely long lens on an insert of a sugar cube, dipped just enough, in a small cup of coffee, so that it gradually seeps in the dark beverage. Four and a half seconds of unadulterated cinematic bliss."

Related material from this journal:
The Dream of
the Expanded Field

A graphic novel reviewed in the current Washington Post features Alfred North Whitehead and Bertrand Russell–
Related material:
Whitehead on Fano's finite projective three-space:
"This is proved by the consideration of a three dimensional geometry in which there are only fifteen points."
—The Axioms of Projective Geometry , Cambridge University Press, 1906
Further reading:
See Solomon's Cube and the link at the end of today's previous entry, then compare and contrast the above portraits of Whitehead and Russell with Charles Williams's portraits of Sir Giles Tumulty and Lord Arglay in the novel Many Dimensions .
Old Year, Raus!
Also in today’s New York Times obituaries index:
John T. Elson, Editor Who Asked
“Is God Dead?” at Time, Dies at 78

Wikipedia article on George Polya:
From the date of Elson’s death:
Magic Boxes
"Somehow it seems to fill my head with ideas– only I don't exactly know what they are!…. Let's have a look at the garden first!"
— A passage from Lewis Carroll's Through the Looking-Glass. The "garden" part– but not the "ideas" part– was quoted by Jacques Derrida in Dissemination in the epigraph to Chapter 7, "The Time before First."
Commentary
on the passage:
Part I "The Magic Box," shown on Turner Classic Movies earlier tonight
Part II: "Mimsy Were the Borogoves," a classic science fiction story:
"… he lifted a square, transparent crystal block, small enough to cup in his palm– much too small to contain the maze of apparatus within it. In a moment Scott had solved that problem. The crystal was a sort of magnifying glass, vastly enlarging the things inside the block. Strange things they were, too. Miniature people, for example– They moved. Like clockwork automatons, though much more smoothly. It was rather like watching a play."
Part III: A Crystal Block —

Image of pencils is by
Diane Robertson Design.
Related material:
"A Four-Color Theorem."
Part IV:

(Cover slightly changed.)
Background —
SAT
Part I:
Part II:
 |
Part III:
From August 25th —

"Boo, boo, boo,
square root of two."
Text:
| The Shining, 1977, page 162: “A new headline, this one “The item on the next page |
Exegesis:
| April 10— Good Friday– See The Paradise of Childhood. Four months later– Aug. 10— “When he thought of the old man |
Tina Fey to Steve Martin
at the Oscars:
"Oh, Steve, no one wants
to hear about our religion
… that we made up."

|
From Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 117:
… in 'The Pediment of Appearance,' a slight narrative poem in Transport to Summer… A group of young men enter some woods 'Hunting for the great ornament, The pediment of appearance.' Though moving through the natural world, the young men seek the artificial, or pure form, believing that in discovering this pediment, this distillation of the real, they will also discover the 'savage transparence,' the rude source of human life. In Stevens's world, such a search is futile, since it is only through observing nature that one reaches beyond it to pure form. As if to demonstrate the degree to which the young men's search is misaligned, Stevens says of them that 'they go crying/The world is myself, life is myself,' believing that what surrounds them is immaterial. Such a proclamation is a cardinal violation of Stevens's principles of the imagination. |
Superficially the young men's philosophy seems to resemble what Wikipedia calls "pantheistic solipsism"– noting, however, that "This article has multiple issues."
As, indeed, does pantheistic solipsism– a philosophy (properly called "eschatological pantheistic multiple-ego solipsism") devised, with tongue in cheek, by science-fiction writer Robert A. Heinlein.
Despite their preoccupation with solipsism, Heinlein and Stevens point, each in his own poetic way, to a highly non-solipsistic topic from pure mathematics that is, unlike the religion of Martin and Fey, not made up– namely, the properties of space.
"Sharpie, we have condensed six dimensions into four, then we either work by analogy into six, or we have to use math that apparently nobody but Jake and my cousin Ed understands. Unless you can think of some way to project six dimensions into three– you seem to be smart at such projections."
I closed my eyes and thought hard. "Zebbie, I don't think it can be done. Maybe Escher could have done it."
|
A discussion of Stevens's late poem "The Rock" (1954) in Wallace Stevens: A World of Transforming Shapes, by Alan D. Perlis, Bucknell University Press, 1976, p. 120:
For Stevens, the poem "makes meanings of the rock." In the mind, "its barrenness becomes a thousand things/And so exists no more." In fact, in a peculiar irony that only a poet with Stevens's particular notion of the imagination's function could develop, the rock becomes the mind itself, shattered into such diamond-faceted brilliance that it encompasses all possibilities for human thought:
The rock is the gray particular of man's life,
The stone from which he rises, up—and—ho,
The step to the bleaker depths of his descents ...
The rock is the stern particular of the air,
The mirror of the planets, one by one,
But through man's eye, their silent rhapsodist,
Turquoise the rock, at odious evening bright
With redness that sticks fast to evil dreams;
The difficult rightness of half-risen day.
The rock is the habitation of the whole,
Its strength and measure, that which is near,
point A
In a perspective that begins again
At B: the origin of the mango's rind.
(Collected Poems, 528)
|
Stevens's rock is associated with empty space, a concept that suggests "nothingness" to one literary critic:
B. J. Leggett, "Stevens's Late Poetry" in The Cambridge Companion to Wallace Stevens— On the poem "The Rock":
"… the barren rock of the title is Stevens's symbol for the nothingness that underlies all existence, 'That in which space itself is contained'…. Its subject is its speaker's sense of nothingness and his need to be cured of it."
More positively…
Space is, of course, also a topic
in pure mathematics…
For instance, the 6-dimensional
affine space (or the corresponding
5-dimensional projective space)

over the two-element Galois field
can be viewed as an illustration of
Stevens's metaphor in "The Rock."
Cara:

Here the 6-dimensional affine
space contains the 63 points
of PG(5, 2), plus the origin, and
the 3-dimensional affine
space contains as its 8 points
Conwell's eight "heptads," as in
Generating the Octad Generator.
Yesterday's entry contained the following unattributed quotation:
"One must join forces with friends of like mind."
As the link to Leap Day indicated, the source of the quotation is the I Ching.
Yesterday's entry also quoted the late Terence McKenna, a confused writer on psychosis and the I Ching. Lest the reader conclude that I consider McKenna or similar authors (for instance, Timothy Leary in Cuernavaca) as "friends of like mind," I would point rather to more sober students of the I Ching (cf. my June 2002 notes on philosophy, religion, and science) and to the late Scottish theologian John Macquarrie:

Macquarrie's connection in this journal to the I Ching is, like that book itself, purely coincidental. For details, click on the figure below.

The persistent reader will
find a further link that
leads to an entry titled
"Notes on the I Ching."
"A colour is eternal. It haunts time like a spirit. It comes and it goes. But where it comes it is the same colour. It neither survives nor does it live. It appears when it is wanted."
(American Mathematical Society Feb. 2008
review of Steven Brams’s Superior Beings:
If They Exist, How Would We Know?)
(pdf, 15 megabytes)
"Brams does not attempt to prove or disprove God. He uses elementary ideas from game theory to create situations between a Person (P) and God (Supreme Being, SB) and discusses how each reacts to the other in these model scenarios….
Each player also has a primary and secondary goal. For the Person, the primary goal is to have his belief (or non-belief) confirmed by evidence (or lack thereof). The secondary goal is to 'prefer to believe in SB’s existence.' For the Supreme Being, the primary goal is to have P believe in His existence, while the secondary goal is to not reveal Himself. These goals allow us to rank all the outcomes for each player from best (4) to worst (1). We end up with a matrix as follows (the first number in the parentheses represents the SB's ranking for that box; the second number represents P's ranking):

Analogously:
|
Lotteries on Bloomsday, June 16, 2008 |
Pennsylvania (No revelation) |
New York (Revelation) |
|
Mid-day (No belief) |
418
No belief, |
064
Revelation |
|
Evening (Belief) |
709
Belief without |
198
|
The holy image

denoting belief and revelation
may be interpreted as
a black hole or as a
symbol by James Joyce:
|
When? Going to dark bed there was a square round Sinbad the Sailor roc's auk's egg in the night of the bed of all the auks of the rocs of Darkinbad the Brightdayler. Where?
— Ulysses, conclusion of Chapter 17 |

Greetings.
“The greatest sorcerer (writes Novalis memorably)
would be the one who bewitched himself to the point of
taking his own phantasmagorias for autonomous apparitions.
Would not this be true of us?”
–Jorge Luis Borges, “Avatars of the Tortoise”
“El mayor hechicero (escribe memorablemente Novalis)
sería el que se hechizara hasta el punto de
tomar sus propias fantasmagorías por apariciones autónomas.
¿No sería este nuestro caso?”
–Jorge Luis Borges, “Los Avatares de la Tortuga“
At Midsummer Noon:
|
|
It is not enough to cover the rock with leaves. We must be cured of it by a cure of the ground Or a cure of ourselves, that is equal to a cure
Of the ground, a cure beyond forgetfulness.
And if we ate the incipient colorings – Wallace Stevens, “The Rock” |

(Continued from June 23)

Related material:

The Klein Correspondence,
Penrose Space-Time,
and a Finite Model
Haunting Time
"Macquarrie remains one of the most
important commentators [on] …
Heidegger's work. His co-translation
of Being and Time into English is
considered the canonical version."
— Wikipedia

The Rev. Macquarrie died on
May 28. The Log24 entry
for that date contains the
following illustration:

The part of the illustration
relevant to the death of
Macquarrie is the color.
From my reply to
a comment on the
May 28 entry:
From McKenna's afterword:
My own remarks on the hippie
scene seem appropriate as a
response to media celebration
of today's 40th anniversary of
the beginning of the 1967
"summer of love."
and a Finite Model
Notes by Steven H. Cullinane
May 28, 2007
Part I: A Model of Space-Time
Click on picture to enlarge.
Part II: A Corresponding Finite Model
The Klein quadric also occurs in a finite model of projective 5-space. See a 1910 paper:
G. M. Conwell, The 3-space PG(3,2) and its group, Ann. of Math. 11, 60-76.
Conwell discusses the quadric, and the related Klein correspondence, in detail. This is noted in a more recent paper by Philippe Cara:
 |
Related material:
The projective space PG(5,2), home of the Klein quadric in the finite model, may be viewed as the set of 64 points of the affine space AG(6,2), minus the origin.
The 64 points of this affine space may in turn be viewed as the 64 hexagrams of the Classic of Transformation, China’s I Ching.
There is a natural correspondence between the 64 hexagrams and the 64 subcubes of a 4x4x4 cube. This correspondence leads to a natural way to generate the affine group AGL(6,2). This may in turn be viewed as a group of over a trillion natural transformations of the 64 hexagrams.

See last year’s
entries for 5/10 —
My Space

and for 2/23 —
Cubist Epiphany
“This is a crazy world and
the only way to enjoy it
is to treat it as a joke.”
— Robert A. Heinlein,
The Number of the Beast
First to Illuminate
“From the History of a Simple Group” (pdf), by Jeremy Gray:
“The American mathematician A. B. Coble [1908; 1913]* seems to have been the first to illuminate the 27 lines and 28 bitangents with the elementary theory of geometries over finite fields.
The combinatorial aspects of all this are pleasant, but the mathematics is certainly not easy.”
* [Coble 1908] A. Coble, “A configuration in finite geometry isomorphic with that of the 27 lines on a cubic surface,” Johns Hopkins University Circular 7:80-88 (1908), 736-744.
[Coble 1913] A. Coble, “An application of finite geometry to the characteristic theory of the odd and even theta functions,” Trans. Amer. Math. Soc. 14 (1913), 241-276.
Binary Geometry
There is currently no area of mathematics named “binary geometry.” This is, therefore, a possible name for the geometry of sets with 2n elements (i.e., a sub-topic of Galois geometry and of algebraic geometry over finite fields– part of Weil’s “Rosetta stone” (pdf)).
Examples:
Go with the Flow
The previous entry links to a document that discusses the mathematical concept of "Ricci flow (pdf)."
Though the concept was not named for him, this seems as good a time as any to recall the virtues of St. Matteo Ricci, a Jesuit who died in Beijing on May 11, 1610. (The Church does not yet recognize him as a saint; so much the worse for the Church.)
There was no Log24 entry on Ricci's saint's day, May 11, this year, but an entry for 4:29 PM May 10, 2006, seems relevant, since Beijing is 12 hours ahead of my local (Eastern US) time.

The relevance of this structure
to memory and to Chinese culture
is given in Dragon School and in
Geometry of the 4x4x4 Cube.
For some related remarks on
the colloquial, rather than the
mathematical, concept of flow,
see
Philosophy, Religion, and Science
as well as Crystal and Dragon.
Yesterday's entry on the 1865
remarks on aesthetics of
Gerard Manley Hopkins,
who later became a Jesuit,
may also have some relevance.
Finitegeometry.org Update
(Revised May 21, 2006)
Finitegeometry.org now has permutable JavaScript views of the 2x2x2 and 4x4x4 design cubes. Solomon’s Cube presented a claim that the 4x4x4 design cube retains symmetry under a group of about 1.3 trillion transformations. The JavaScript version at finitegeometry.org/sc/64/view/ lets the reader visually verify this claim. The reader should first try the Diamond 16 Puzzle. The simpler 2x2x2 design cube, with its 1,344 transformations, was described in Diamonds and Whirls; the permutable JavaScript version is at finitegeometry.org/sc/8/view/.
“In The Painted Word, a rumination on the state of American painting in the 1970s, Tom Wolfe described an epiphany….”
— Peter Berkowitz, “Literature in Theory”
“I had an epiphany.”
— Apostolos Doxiadis, organizer of last summer’s conference on mathematics and narrative. See the Log24 entry of 1:06 PM last August 23 and the four entries that preceded it.
“… das Durchleuchten des ewigen Glanzes des ‘Einen’ durch die materielle Erscheinung“
— A definition of beauty from Plotinus, via Werner Heisenberg
“By groping toward the light we are made to realize how deep the darkness is around us.”
— Arthur Koestler, The Call Girls: A Tragi-Comedy, Random House, 1973, page 118, quoted in The Shining of May 29
“Perhaps we are meant to see the story as a cubist retelling of the crucifixion….”
— Adam White Scoville, quoted in Cubist Crucifixion, on Iain Pears’s novel, An Instance of the Fingerpost
New Site
(Site title and address were revised on May 21, 2006.)
The new site for my math files is
finitegeometry.org/sc/index.html:
 |
Finite Geometry |
|
This site is about the (the mathematical structure, |
|
As time goes on, I'll be changing links on the Web to my math pages, which are now scattered at various Web addresses, to refer to this new site.
Incidentally, this is the 20th anniversary of my note, "The relativity problem in finite geometry."
Diamond theory is the theory of affine groups over GF(2) acting on small square and cubic arrays. In the simplest case, the symmetric group of degree 4 acts on a two-colored diamond figure like that in Plato's Meno dialogue, yielding 24 distinct patterns, each of which has some ordinary or color-interchange symmetry .
This symmetry invariance can be generalized to (at least) a group of order approximately 1.3 trillion acting on a 4x4x4 array of cubes.
The theory has applications to finite geometry and to the construction of the large Witt design underlying the Mathieu group of degree 24.
|
|
||||
|
Example:
|
||||
|
|
||||
|
|
Initial Xanga entry. Updated Nov. 18, 2006.
Powered by WordPress