Symplectic Macbeth
Monday, August 25, 2014
A Challenging Story:
Sunday, August 24, 2014
Symplectic Structure…
In the Miracle Octad Generator (MOG):
The above details from a one-page note of April 26, 1986, refer to the
Miracle Octad Generator of R. T. Curtis, as it was published in 1976:
From R. T. Curtis (1976). A new combinatorial approach to M24,
Mathematical Proceedings of the Cambridge Philosophical Society ,
79, pp 25-42. doi:10.1017/S0305004100052075.
The 1986 note assumed that the reader would be able to supply, from the
MOG itself, the missing top row of each heavy brick.
Note that the interchange of the two squares in the top row of each
heavy brick induces the diamond-theorem correlation.
Note also that the 20 pictured 3-subsets of a 6-set in the 1986 note
occur as paired complements in two pictures, each showing 10 of the
3-subsets.
This pair of pictures corresponds to the 20 Rosenhain tetrads among
the 35 lines of PG(3,2), while the picture showing the 2-subsets
corresponds to the 15 Göpel tetrads among the 35 lines.
See Rosenhain and Göpel tetrads in PG(3,2). Some further background:
Thursday, August 21, 2014
Nox
( A sequel to Lux )
“By groping toward the light we are made to realize
how deep the darkness is around us.”
— Arthur Koestler, The Call Girls: A Tragi-Comedy ,
Random House, 1973, page 118

Robin Williams and the Stages of Math
i) shock & denial
ii) anger
iii) bargaining
iv) depression
v) acceptance
A related description of the process —
“You know how sometimes someone tells you a theorem,
and it’s obviously false, and you reach for one of the many
easy counterexamples only to realize that it’s not a
counterexample after all, then you reach for another one
and another one and find that they fail too, and you begin
to concede the possibility that the theorem might not
actually be false after all, and you feel your world start to
shift on its axis, and you think to yourself: ‘Why did no one
tell me this before?’ “
— Tom Leinster yesterday at The n-Category Café
Friday, August 15, 2014
The Omega Matrix
The webpage Rosenhain and Göpel Tetrads in PG(3,2)
has been updated to include more material on symplectic structure.
Wednesday, August 13, 2014
Symplectic Structure continued
Some background for the part of the 2002 paper by Dolgachev and Keum
quoted here on January 17, 2014 —
Related material in this journal (click image for posts) —
Stranger than Dreams*
Illustration from a discussion of a symplectic structure
in a 4×4 array quoted here on January 17, 2014 —

See symplectic structure in this journal.
* The final words of Point Omega , a 2010 novel by Don DeLillo.
See also Omega Matrix in this journal.
Monday, August 11, 2014
Syntactic/Symplectic
(Continued from August 9, 2014.)
Syntactic:
Symplectic:
"Visual forms— lines, colors, proportions, etc.— are just as capable of
articulation , i.e. of complex combination, as words. But the laws that govern
this sort of articulation are altogether different from the laws of syntax that
govern language. The most radical difference is that visual forms are not
discursive . They do not present their constituents successively, but
simultaneously, so the relations determining a visual structure are grasped
in one act of vision."
– Susanne K. Langer, Philosophy in a New Key
For examples, see The Diamond-Theorem Correlation
in Rosenhain and Göpel Tetrads in PG(3,2).
This is a symplectic correlation,* constructed using the following
visual structure:
 .
.
* Defined in (for instance) Paul B. Yale, Geometry and Symmetry ,
Holden-Day, 1968, sections 6.9 and 6.10.
Sunday, August 10, 2014
Knight Moves
Some illustrations:
Chess Knight
(in German, Springer)
See also…
More technically (click image for details):
Saturday, August 9, 2014
Syntactic/Symplectic
Syntactic Structure —
See the Lightfoot of today’s previous post:

Symplectic Structure —
See the plaited, or woven, structure of August 6:
 .
.
See also Deep Structure (Dec. 9, 2012).
Thursday, August 7, 2014
Abuse of Language
From Wikipedia — Abuse of language —
“… in mathematics, a use of terminology in a way that is not formally correct
but that simplifies exposition or suggests the correct intuition.”
The phrase “symplectic structure” in the previous post
was a deliberate abuse of language. The real definition:
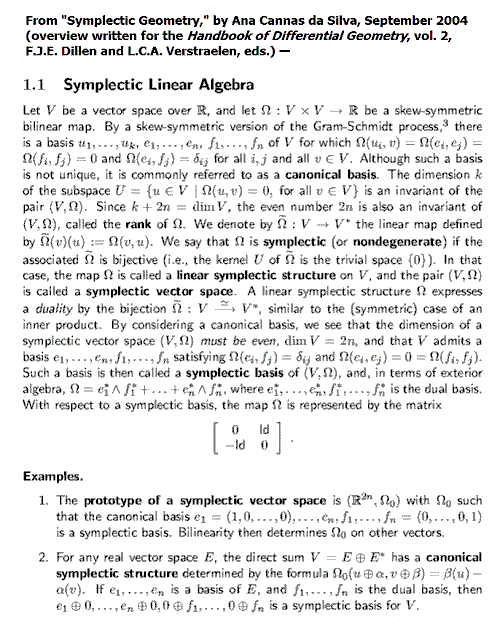
Wednesday, August 6, 2014
Symplectic Structure*
From Gotay and Isenberg, "The Symplectization of Science,"
Gazette des Mathématiciens 54, 59-79 (1992):
"… what is the origin of the unusual name 'symplectic'? ….
Its mathematical usage is due to Hermann Weyl who,
in an effort to avoid a certain semantic confusion, renamed
the then obscure 'line complex group' the 'symplectic group.'
… the adjective 'symplectic' means 'plaited together' or 'woven.'
This is wonderfully apt…."

The above symplectic structure** now appears in the figure
illustrating the diamond-theorem correlation in the webpage
Rosenhain and Göpel Tetrads in PG(3,2).
Some related passages from the literature:


* The title is a deliberate abuse of language .
For the real definition of "symplectic structure," see (for instance)
"Symplectic Geometry," by Ana Cannas da Silva (article written for
Handbook of Differential Geometry, vol 2.) To establish that the
above figure is indeed symplectic , see the post Zero System of
July 31, 2014.
** See Steven H. Cullinane, Inscapes III, 1986
Monday, August 4, 2014
A Wrinkle in Space
"There is such a thing as a tesseract." — Madeleine L'Engle
An approach via the Omega Matrix:
See, too, Rosenhain and Göpel as The Shadow Guests .
Sunday, August 3, 2014
The Omega Matrix
Shown below is the matrix Omega from notes of Richard Evan Schwartz.
See also earlier versions (1976-1979) by Steven H. Cullinane.

Backstory: The Schwartz Notes (June 1, 2011), and Schwartz on
the American Mathematical Society's current home page:
Friday, August 1, 2014
The Diamond-Theorem Correlation
Click image for a larger, clearer version.
Thursday, July 31, 2014
Zero System
The title phrase (not to be confused with the film 'The Zero Theorem')
means, according to the Encyclopedia of Mathematics,
a null system , and
"A null system is also called null polarity,
a symplectic polarity or a symplectic correlation….
it is a polarity such that every point lies in its own
polar hyperplane."
See Reinhold Baer, "Null Systems in Projective Space,"
Bulletin of the American Mathematical Society, Vol. 51
(1945), pp. 903-906.
An example in PG(3,2), the projective 3-space over the
two-element Galois field GF(2):

See also the 10 AM ET post of Sunday, June 8, 2014, on this topic.
Sunday, July 27, 2014
George Johnson’s Theory of Everything
George Johnson, a science writer, in The New York Times
on July 21, 2014:
“New particles may yet be discovered, and even new laws.
But it is almost taken for granted that everything
from physics to biology, including the mind,
ultimately comes down to four fundamental concepts:
matter and energy interacting in an arena of space and time.”
Related material:
Ite, missa est.
Sunday, July 20, 2014
Sunday School
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).
Thursday, July 17, 2014
Paradigm Shift:
Continuous Euclidean space to discrete Galois space*
Euclidean space:
Counting symmetries in Euclidean space:
Galois space:
Counting symmetries of Galois space:

The reason for these graphic symmetries in affine Galois space —
symmetries of the underlying projective Galois space:
* For related remarks, see posts of May 26-28, 2012.
Wednesday, July 16, 2014
Tuesday, July 15, 2014
Photo Opportunity
"I need a photo opportunity, I want a shot at redemption.
Don't want to end up a cartoon in a cartoon graveyard."
– Paul Simon

|
"The theory of poetry, that is to say, the total of the theories of poetry, often seems to become in time a mystical theology or, more simply, a mystique. The reason for this must by now be clear. The reason is the same reason why the pictures in a museum of modern art often seem to become in time a mystical aesthetic, a prodigious search of appearance, as if to find a way of saying and of establishing that all things, whether below or above appearance, are one and that it is only through reality, in which they are reflected or, it may be, joined together, that we can reach them. Under such stress, reality changes from substance to subtlety, a subtlety in which it was natural for Cézanne to say: 'I see planes bestriding each other and sometimes straight lines seem to me to fall' or 'Planes in color…. The colored area where shimmer the souls of the planes, in the blaze of the kindled prism, the meeting of planes in the sunlight.' The conversion of our Lumpenwelt went far beyond this. It was from the point of view of another subtlety that Klee could write: 'But he is one chosen that today comes near to the secret places where original law fosters all evolution. And what artist would not establish himself there where the organic center of all movement in time and space– which he calls the mind or heart of creation– determines every function.' Conceding that this sounds a bit like sacerdotal jargon, that is not too much to allow to those that have helped to create a new reality, a modern reality, since what has been created is nothing less.
— Wallace Stevens, Harvard College Class of 1901, "The Relations between Poetry and Painting" in The Necessary Angel (Knopf, 1951) |
For background on the planes illustrated above,
see Diamond theory in 1937.
Monday, July 14, 2014
Hexagram 14
Related material:
Lead obituary in today’s online New York Times and Los Angeles Times —

Maazel reportedly died on Sunday, July 13, 2014.
From a search in this journal for Iconic Notation,
a related image from August 14, 2010—
See also…
Epiphany
Saturday, July 12, 2014
Mars Package

“For me it is a sign that we have fundamentally different
conceptions of the work of the intelligence services.”
— Germany’s Chancellor Angela Merkel in
theguardian.com, Saturday, 12 July 2014, 14.32 EDT
Another sort of service, thanks to Dan Brown and Tom Hanks:
Friday, July 11, 2014 |
Sequel
A sequel to the 1974 film
Thunderbolt and Lightfoot :
Contingent and Fluky
Some variations on a thunderbolt theme:
These variations also exemplify the larger
Verbum theme:


A search today for Verbum in this journal yielded
a Georgetown University Chomskyite, Professor
David W. Lightfoot.
"Dr. Lightfoot writes mainly on syntactic theory,
language acquisition and historical change, which
he views as intimately related. He argues that
internal language change is contingent and fluky,
takes place in a sequence of bursts, and is best
viewed as the cumulative effect of changes in
individual grammars, where a grammar is a
'language organ' represented in a person's
mind/brain and embodying his/her language
faculty."
Some syntactic work by another contingent and fluky author
is related to the visual patterns illustrated above.
See Tecumseh Fitch in this journal.
For other material related to the large Verbum cube,
see posts for the 18th birthday of Harry Potter.
That birthday was also the upload date for the following:
See esp. the comments section.
Friday, July 11, 2014
Spiegel-Spiel des Gevierts
Wednesday, July 9, 2014
Nowhere
From this morning’s post:
“Beyond Noplace, far into wide Nowhere” — John Hollander
Vide Le Guin Geometry.

Monday, July 7, 2014
Tricky Task
Roger Cooke in the Notices of the American
Mathematical Society , April 2010 —
"Life on the Mathematical Frontier:
Legendary Figures and Their Adventures"
"In most cases involving the modern era, there
are enough documents to produce a clear picture
of mathematical developments, and conjectures
for which there is no eyewitness or documentary
evidence are not needed. Even so, legends do
arise. (Who has not heard the 'explanation' of
the absence of a Nobel Prize in mathematics?)
The situation is different regarding ancient math-
ematics, however, especially in the period before
Plato’s students began to study geometry. Much
of the prehistory involves allegations about the
mysterious Pythagoreans, and sorting out what is
reliable from what is not is a tricky task.
In this article, I will begin with some modern
anecdotes that have become either legend or
folklore, then work backward in time to take a
more detailed look at Greek mathematics, especially
the Pythagoreans, Plato, and Euclid. I hope at the
very least that the reader finds my examples
amusing, that being one of my goals. If readers
also take away some new insight or mathematical
aphorisms, expressing a sense of the worthiness of
our calling, that would be even better."
Aphorism: "Triangles are square."
(American Mathematical Monthly , June-July 1984)
Insight: The Square-Triangle Theorem.
Sunday, July 6, 2014
Sunday School
The date of a Vanity Fair post on Hugh Jackman—
Sunday, June 8, 2014— suggests a review of the following
quotation from this journal on that date —
Zen ideal —

Sticks and Stones
The title is from this morning's previous post.
From a theater review in that post—
… "all flying edges and angles, a perpetually moving and hungry soul"
… "a formidably centered presence, the still counterpoint"
A more abstract perspective:
See also Desargues via Galois (August 6, 2013).
Friday, July 4, 2014
Knockout
(Continued from yesterday’s noon post, from “Block That Metaphor,”
and from “Mystery Box III: Inside, Outside“)
“In one corner are the advocates of the Common Core,
led by the Bill and Melinda Gates Foundation, which
helped develop the standards and has defended them
against efforts by some states to roll them back. In the
challengers’ corner, a lineup of foundations and
philanthropists…. Other funders in the opponents’ corner
read like a ‘who’s who’ of well-heeled conservative
philanthropists, including Pittsburgh media magnate
Richard Mellon Scaife….”
— “Meet the Funders Fighting the Common Core,”
from Inside Philanthropy , Feb. 10, 2014
Scaife reportedly died this morning.
Thursday, June 26, 2014
Study This Example, Part II
(Continued from 10:09 AM today)
The quotation below is from a webpage on media magnate
Walter Annenberg.
Annenberg Hall at Harvard, originally constructed to honor
the Civil War dead, was renamed in 1996 for his son Roger,
Harvard Class of ’62.
www.broadcastpioneers.com/
walterannenberg.html —
“It was said that Roger was ‘moody and sullen’
spending large parts of his time reading poetry
and playing classical music piano. It had been
reported that Roger attempted suicide at the
age of eleven by slitting his wrists. He recovered
and was graduated Magna Cum Laude from
Episcopal Academy in our area. For awhile,
Roger attended Harvard, but he was removed
from the school’s rolls after Roger stopped doing
his school work and spent almost all his time
reading poetry in his room. He then was sent to
an exclusive and expensive treatment center
in Bucks County, Pennsylvania. At that facility,
Roger became more remote. It was said that he
often didn’t recognize or acknowledge his father.
On August 7, 1962, Roger Annenberg died from
an overdose of sleeping pills.”
A more appropriate Annenberg memorial, an article
in The Atlantic magazine on June 25, notes that…
“Among those who ended up losing their battles
with mental illness through suicide are
Virginia Woolf, Ernest Hemingway, Vincent van Gogh,
John Berryman, Hart Crane, Mark Rothko, Diane Arbus,
Anne Sexton, and Arshile Gorky.”
Study This Example
The authors of the following offer an introduction to symmetry
in quilt blocks. They assume, perhaps rightly, that their audience
is intellectually impaired:
“A quilt block is made of 16 smaller squares.
Each small square consists of two triangles.”
Study this example of definition.
(It applies quite precisely to the sorts of square patterns
discussed in the 1976 monograph Diamond Theory , but
has little relevance for quilt blocks in general.)
Some background for those who are not intellectually impaired:
Robinson’s book Definition , in this journal and at Amazon.
The McLuhan Dimension
"History is a deep and complicated puzzle—
especially when it involves more dimensions than time."
— Introduction to a novella in Analog Science Fiction
"Annenberg Hall" at Harvard was originally part of a memorial for
Civil War dead. Formerly "Alumni Hall," it was renamed in 1996.
Sunday, June 22, 2014
Göpel and Rosenhain
Some bizarre remarks on “purity” in the previous post
suggest a review of some pure mathematics.
Tuesday, June 17, 2014
Finite Relativity
Anyone tackling the Raumproblem described here
on Feb. 21, 2014 should know the history of coordinatizations
of the 4×6 Miracle Octad Generator (MOG) array by R. T. Curtis
and J. H. Conway. Some documentation:

The above two images seem to contradict a statement by R. T. Curtis
in a 1989 paper. Curtis seemed in that paper to be saying, falsely, that
his original 1973 and 1976 MOG coordinates were those in array M below—

This seemingly false statement involved John H. Conway's supposedly
definitive and natural canonical coordinatization of the 4×6 MOG
array by the symbols for the 24 points of the projective line over GF(23)—
{∞, 0, 1, 2, 3… , 21, 22}:

An explanation of the apparent falsity in Curtis's 1989 paper:
By "two versions of the MOG" Curtis seems to have meant merely that the
octads , and not the projective-line coordinates , in his earlier papers were
mirror images of the octads that resulted later from the Conway coordinates,
as in the images below.

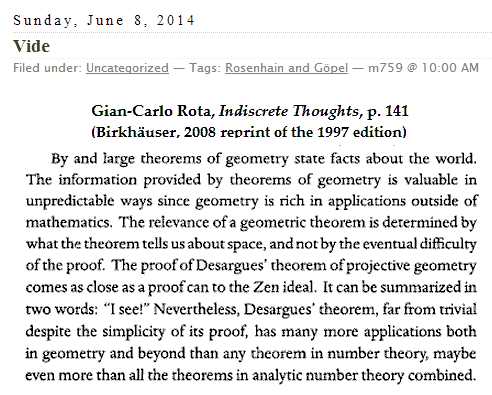
Sunday, June 8, 2014
Vide

"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
Thursday, June 5, 2014
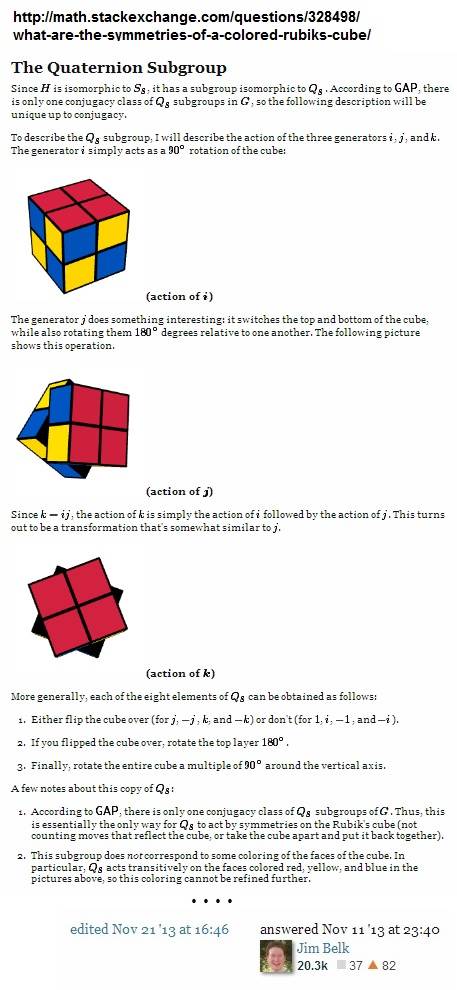
Twisty Quaternion Symmetry
The previous post told how user58512 at math.stackexchange.com
sought in 2013 a geometric representation of Q8 , the quaternion group.
He ended up displaying an illustration that very possibly was drawn,
without any acknowledgement of its source, from my own work.
On the date that user58512 published that illustration, he further
pursued his March 1, 2013, goal of a “twisty” quaternion model.
On March 12, 2013, he suggested that the quaternion group might be
the symmetry group of the following twisty-cube coloring:
Illustration by Jim Belk
Here is part of a reply by Jim Belk from Nov. 11, 2013, elaborating on
that suggestion:
Belk argues that the colored cube is preserved under the group
of actions he describes. It is, however, also preserved under a
larger group. (Consider, say, rotation of the entire cube by 180
degrees about the center of any one of its checkered faces.) The
group Belk describes seems therefore to be a symmetry group,
not the symmetry group, of the colored cube.
I do not know if any combination puzzle has a coloring with
precisely the quaternion group as its symmetry group.
(Updated at 12:15 AM June 6 to point out the larger symmetry group
and delete a comment about an arXiv paper on quaternion group models.)
Wednesday, June 4, 2014
Monkey Business
The title refers to a Scientific American weblog item
discussed here on May 31, 2014:
Some closely related material appeared here on
Dec. 30, 2011:
A version of the above quaternion actions appeared
at math.stackexchange.com on March 12, 2013:
"Is there a geometric realization of Quaternion group?" —

The above illustration, though neatly drawn, appeared under the
cloak of anonymity. No source was given for the illustrated group actions.
Possibly they stem from my Log24 posts or notes such as the Jan. 4, 2012,
note on quaternion actions at finitegeometry.org/sc (hence ultimately
from my note "GL(2,3) actions on a cube" of April 5, 1985).
Tuesday, June 3, 2014
Robert Steinberg, 1922-2014
Galois matrices, the subject of the previous post,
are of course not new. See, for instance, Steinberg in 1951:

The American Mathematical Society reports that Steinberg died
on May 25, 2014.
As the above 1951 paper indicates, Steinberg was well acquainted with
what Weyl called "the devil of abstract algebra." In this journal, however,
Steinberg himself appears rather as an angel of geometry.
Galois Matrices
The webpage Galois.us, on Galois matrices , has been created as
a starting point for remarks on the algebra (as opposed to the geometry)
underlying the rings of matrices mentioned in AMS abstract 79T-A37,
“Symmetry invariance in a diamond ring.”
See also related historical remarks by Weyl and by Atiyah.
Saturday, May 31, 2014
Quaternion Group Models:
The ninefold square, the eightfold cube, and monkeys.

For posts on the models above, see quaternion
in this journal. For the monkeys, see
"Nothing Is More Fun than a Hypercube of Monkeys,"
Evelyn Lamb's Scientific American weblog, May 19, 2014:
The Scientific American item is about the preprint
"The Quaternion Group as a Symmetry Group,"
by Vi Hart and Henry Segerman (April 26, 2014):
See also Finite Geometry and Physical Space.
Friday, May 30, 2014
Combinatorial Matrix Classes
A book by this title, Richard A. Brualdi’s Combinatorial Matrix Classes ,
was published by Cambridge University Press in 2006:
For some related remarks, see The Counter (March 13, 2011).
My own work deals with combinatorial properties of matrices
of 0’s and 1’s, but in the context of Galois (i.e., finite) fields,
not the real or complex fields. Despite the generality of
their titles, Combinatorial Matrix Theory and Combinatorial
Matrix Classes do not deal with Galois matrices.
Sunday, May 25, 2014
Sils-Maria
"In August 1884, he wrote to Resa von Schirnhofer:
'Here one can live well, in this strong, bright atmosphere,
here where nature is amazingly mild and solemn
and mysterious all at once— in fact, there is no place
that I like better than Sils-Maria.'"
For more about Resa, see another weblog's post
of April 30, 2013.
A remark on Nietzsche from the epigraph of that weblog:
"His life's work was devoted to finding one's 'style'
within the chaos of existence. The trick, obviously,
is not to lose your mind in the process."
A remark from this weblog on the above date —
Walpurgisnacht 2013 —
Friday, May 23, 2014
Free-Floating Signs
“You’ve got to pick up every stitch…”
— Donovan, song on closing credits of To Die For
“…’Supersymmetry’ was originally written
specifically for Her ….” — Pitchfork
“Eventually we see snow particles….”
— Her screenplay by Spike Jonze
This journal on January 24, 2006:
Context: See Free-Floating Signs.
Backstory: Digital Member and Uneven Break.
Thursday, May 22, 2014
Visual Structure
“Chaos is order yet undeciphered.”
— The novel The Double , by José Saramago,
on which the recent film "Enemy" was based
For Louise Bourgeois — a post from the date of Galois's death—
For Toronto — Scene from a film that premiered there on Sept. 8, 2013:
Related material: This journal on that date, Sept. 8, 2013:
"I still haven't found what I'm looking for." — Bono
"In fact Surrealism found what it had been looking for
from the first in the 1920 collages [by Max Ernst],
which introduced an entirely original scheme of
visual structure…."
— Rosalind Krauss quoting André Breton*
in "The Master's Bedroom"
* "Artistic Genesis and Perspective of Surrealism"
(1941), in Surrealism and Painting (New York,
Harper & Row, 1972, p. 64).
See also Damnation Morning in this journal.
Wednesday, May 21, 2014
The Tetrahedral Model of PG(3,2)
The page of Whitehead linked to this morning
suggests a review of Polster's tetrahedral model
of the finite projective 3-space PG(3,2) over the
two-element Galois field GF(2).


|
The above passage from Whitehead's 1906 book suggests
that the tetrahedral model may be older than Polster thinks.
Shown at right below is a correspondence between Whitehead's
version of the tetrahedral model and my own square model,
based on the 4×4 array I call the Galois tesseract (at left below).
(Click to enlarge.)
Through the Vanishing Point*
Marshall McLuhan in "Annie Hall" —
"You know nothing of my work."
Related material —
"I need a photo opportunity
I want a shot at redemption
Don't want to end up a cartoon
In a cartoon graveyard"
— Paul Simon
It was a dark and stormy night…

— Page 180, Logicomix
A photo opportunity for Whitehead
(from Romancing the Cube, April 20, 2011)—
See also Absolute Ambition (Nov. 19, 2010).
* For the title, see Vanishing Point in this journal.
Tuesday, May 20, 2014
Play
From a recreational-mathematics weblog yesterday:
"This appears to be the arts section of the post,
so I’ll leave Martin Probert’s page on
The Survival, Origin and Mathematics of String Figures
here. I’ll be back to pick it up at the end. Maybe it’d like
to play with Steven H. Cullinane’s pages on the
Finite Geometry of the Square and Cube."
I doubt they would play well together.
Perhaps the offensive linking of the purely recreational topic
of string figures to my own work was suggested by the
string figures' resemblance to figures of projective geometry.
A pairing I prefer: Desargues and Galois —
For further details, see posts on Desargues and Galois.
Monday, May 19, 2014
Cube Space
A sequel to this afternoon's Rubik Quote:
"The Cube was born in 1974 as a teaching tool
to help me and my students better understand
space and 3D. The Cube challenged us to find
order in chaos."
— Professor Ernő Rubik at Chrome Cube Lab
(Click image below to enlarge.)
Saturday, May 17, 2014
It’s Time for You to…
See the Field
“There have long been rumors of a mythical Ninth Element
that grants ultimate power to the Wizard who masters it.
The Order of Magick says there is no such thing. But….”
— Website of Magicka: The Ninth Element Novel
William Worthy in Beijing —
This journal on the date of Worthy’s death,
May 4, 2014, had a link to…
Friday, May 16, 2014
Way to Go
Or: Death Edit
Log24 on the reported date of Sturtevant’s death:
Conceptual ArtFiled under: Uncategorized — m759 @ 2:01 AM
Yesterday’s online New York Times has the following quote: “The idea becomes a machine that makes the art.” For instance, some conceptual art not by LeWitt: Diamond Theory Roulette (Feb. 2, 2014). |
Thursday, May 15, 2014
Tuesday, May 13, 2014
An Artist’s Memorial
See the above weblog post honoring a Swiss artist‘s
“wit, his perception, his genius, his horizon,
his determination, his humour, his friendship,
and his immeasurable kindness.”
Not a bad sendoff. Contrast with events at Harvard
on the date of the artist’s death.
Related material: An album cover, and …

See also this journal in September 2008.
As far as Swiss art goes, I personally prefer the work of, say,
Karl Gerstner and Paul Talman.
Saturday, May 10, 2014
Test Patterns
Raven’s Progressive Matrices intelligence test—

Wechsler Adult Intelligence Scale test—

Related art — (Click images for further details.)
Patterns suggesting those of the Raven test:
Patterns suggesting those of the Wechsler test:
The latter patterns were derived from the former.
Friday, May 9, 2014
Models of Everything
“The About page contains detailed descriptions of the project….”
— The Illustris project on constructing a model of the universe
For the mathematics of a simpler traditional Chinese model
of everything, see
- Geometry of the I Ching ,
- Solomon’s Cube, and the
- Diamond Space about page.
Wednesday, May 7, 2014
Conceptual Art
Yesterday’s online New York Times has the following quote:
“The idea becomes a machine that makes the art.”
— Sol LeWitt
For instance, some conceptual art not by LeWitt:
Diamond Theory Roulette (Feb. 2, 2014).
Tuesday, May 6, 2014
Fictions
From The Ninth Element website:

Cover of a Norwegian author’s forthcoming novel:
For some Norwegian non-fiction, see an Oslo artist’s Instagram page.
Sunday, May 4, 2014
Geometry for Scarlett
Scarlett Johansson stars in a new film, "Lucy," due to be
released on August 8, directed by Luc Besson, auteur of
The Fifth Element (1997). In other pop culture…
"There have long been rumors of a mythical Ninth Element
that grants ultimate power to the Wizard who masters it.
The Order of Magick says there is no such thing. But…."
— Website of Magicka: The Ninth Element Novel
See also, in this journal, Holy Field as well as Power of the Center.
Saturday, May 3, 2014
Gray Space
Or: Three Shades of Gray
(Continued from previous Gray Space posts.)
Click the above image for some related mathematics.
Those who prefer “magic” approaches to mathematics*
may consult the works of Robert J. Stewart and his
mentor William G. Gray.
Robert J. Stewart (left) and a pentagram photo posted yesterday evening
by Oslo artist Josefine Lyche. See also Lyche in this journal.
* See the April 2014 banners displayed at the websites
of the American Mathematical Society and of the
Mathematical Association of America, as well as
a mathematician’s remarks linked to here last evening.
Sunday, April 27, 2014
Sunday School
Galois and Abel vs. Rubik
"Abel was done to death by poverty, Galois by stupidity.
In all the history of science there is no completer example
of the triumph of crass stupidity…."
— Eric Temple Bell, Men of Mathematics
Gray Space (Continued)
… For The Church of Plan 9.
Friday, April 25, 2014
Creativity
Quoted here on April 11 —
“…direct access to the godhead, which
in this case was Creativity.”
— Tom Wolfe, From Bauhaus to Our House
From “Today in History: April 25, 2014,” by The Associated Press:
“Five years ago… University of Georgia professor
George Zinkhan, 57, shot and killed his wife
and two men outside a community theater in Athens
before taking his own life.”
Related material:
A Google Scholar search for Zinkhan’s 1993 paper,
“Creativity in Advertising,” Journal of Advertising 22,2: 1-3 —

Obiter Dicta:
“Dour wit” — Obituary of a Scots herald who died on Palm Sunday
“Remember me to Herald Square.” — Song lyric
“Welcome to Scotland.” — Kincade in Skyfall
Thursday, April 24, 2014
The Inscape of 24
“The more intellectual, less physical, the spell of contemplation
the more complex must be the object, the more close and elaborate
must be the comparison the mind has to keep making between
the whole and the parts, the parts and the whole.”
— The Journals and Papers of Gerard Manley Hopkins ,
edited by Humphry House, 2nd ed. (London: Oxford
University Press, 1959), p. 126, as quoted by Philip A.
Ballinger in The Poem as Sacrament
Related material from All Saints’ Day in 2012:
Thursday, April 17, 2014
Thursday with the Nashes
“For every kind of vampire, there is a kind of cross.” — Gravity’s Rainbow
“I don’t write exclusively on Jewish themes or about Jewish characters.
My collection of short stories, Strange Attractors , contained nine pieces,
five of which were, to some degree, Jewish, and this ratio has provided me
with a precise mathematical answer (for me, still the best kind of answer)
to the question of whether I am a Jewish writer. I am five-ninths a Jewish writer.”
— Rebecca Goldstein, “Against Logic”
Midrashim for Rebecca:
The Diamond Theory vs. the Story Theory (of truth)
Story Theory and the Number of the Beast
The Palm Sunday post “Gray Space”
For those who prefer the diamond theory of truth,
a “precise mathematical” view of a Gray code —
For those who prefer the story theory of truth,
Thursday with the Nashes —
The actors who portrayed Mr. and Mrs. John Nash in
‘A Beautiful Mind’ now portray Mr. and Mrs. Noah…

Tuesday, April 8, 2014
Miami Mosaic
He “…believed the art to be real….”
— Lawyer for Mosaic Miami Church pastor
accused of trying to sell forged Damien Hirst art
A Miami mosaic from this journal last Dec. 21—
The indicated link is to…
See also St. Ursula’s Day, 2010, and Emil Artin’s dies natalis in 2003.
Friday, April 4, 2014
Eight Gate
From a Huffington Post discussion of aesthetics by Colm Mulcahy
of Spelman College, Atlanta:
"The image below on the left… is… overly simplistic, and lacks reality:
It's all a matter of perspective: the problem here is that opposite sides
of the cube, which are parallel in real life, actually look parallel in the
left image! The image on the right is better…."
A related discussion: Eight is a Gate.
Tuesday, April 1, 2014
Kindergarten Geometry
A screenshot of the new page on the eightfold cube at Froebel Decade:
Click screenshot to enlarge.
For April 1
Monday, March 31, 2014
Art Wars for Coxeter
Geometer H. S. M. Coxeter died on this date in 2003.
This evening’s daily number from the Keystone state: 822.
Friday, March 28, 2014
Chinese Rune
"The Geometry of the I Ching introduces something called the Cullinane sequence
for the hexagrams, and uses a notation based on the four sides and two diagonals
in a square to indicate the yin and yang lines. The resulting rune-like symbols
are intriguing…."
— Andreas Schöter's I Ching home page
Actually, the geometry is a bit deeper than the rune-like symbols.
" 'Harriet Burden has been really great to me,'
Rune says in an interview, 'not only as a collector
of my work but as a true supporter. And I think of her
as a muse for the project … ' "
— In The Blazing World , the artist known as Rune
Thursday, March 27, 2014
Diamond Space
Definition: A diamond space — informal phrase denoting
a subspace of AG(6, 2), the six-dimensional affine space
over the two-element Galois field.
The reason for the name:
Click to enlarge.
Saturday, March 22, 2014
Two Types of Symmetry
Friday, March 21, 2014
Three Constructions of the Miracle Octad Generator
See also a Log24 post on this subject from Dec. 14, 2013,
especially (scroll down) the update of March 9, 2014.
Related material on the Turyn-Curtis construction
from the University of Cambridge —

— Slide by "Dr. Parker" — Apparently Richard A. Parker —
Lecture 4, "Discovering M24," in slides for lectures 1-8 from lectures
at Cambridge in 2010-2011 on "Sporadic and Related Groups."
See also the Parker lectures of 2012-2013 on the same topic.
A third construction of Curtis's 35 4×6 1976 MOG arrays would use
Cullinane's analysis of the 4×4 subarrays' affine and projective structure,
and point out the fact that Conwell's 1910 correspondence of the 35
4+4-partitions of an 8-set with the 35 lines of the projective 3-space
over the 2-element field, PG(3, 2), is essentially the same correspondence
as that constituting Curtis's 1976 MOG.
See The Diamond Theorem, Finite Relativity, Galois Space,
Generating the Octad Generator, and The Klein Correspondence.
Update of March 22-March 23 —
Adding together as (0,1)-matrices over GF(2) the black parts (black
squares as 1's, all other squares as 0's) of the 35 4×6 arrays of the 1976
Curtis MOG would then reveal* the symmetric role played in octads
by what Curtis called the heavy brick , and so reveal also the action of
S3 on the three Curtis bricks that leaves invariant the set of all 759
octads of the S(5, 8, 24) constructed from the 35 MOG arrays. For more
details of this "by-hand" construction, see Geometry of the 4×4 Square.
For the mathematical properties of the S(5, 8, 24), it is convenient to
have a separate construction (such as Turyn's), not by hand, of the
extended binary Golay code. See the Brouwer preprint quoted above.
* "Then a miracle occurs," as in the classic 1977 Sidney Harris cartoon.
Illustration of array addition from March 23 —
Thursday, March 20, 2014
Classical Galois
Saturday, March 15, 2014
Friday, March 14, 2014
Thursday, March 13, 2014
Entartete Kunst
The title refers to a New York Times story about
an art exhibition that opened today.
This evening’s NY Lottery numbers: 016 and 2858.
Pictures from these links:
016 (Blackboard Jungle , 1955) —

2858 (number of a Log24 post, 2007) —
Wednesday, March 12, 2014
Obiter Dictum
The title is both a legal phrase and a phrase
used by Tom Wolfe in his writings on art.
See, too, the pattern of nine triangular half-squares
arranged in a 3×3 square used in the logo of the
Jean Stephen art galleries in Minneapolis…

… and in a print at the Tate in London (click to enlarge)—
See as well an obit of the print’s artist, Justin Knowles, who reportedly died
on Feb. 24, 2004.
Some instances of that date in this journal are related to Knowles’s aesthetics.
Tuesday, March 11, 2014
Dark Fields of the Republic
This post was suggested by today's previous post, Depth,
by Plato's Diamond, and by Rebecca Newberger Goldstein's
recent fanciful fiction about Plato.
Plato, Republic , Book II, Paul Shorey translation at Perseus—
“Consider,” [382a] said I; “would a god wish to deceive, or lie, by presenting in either word or action what is only appearance?” “I don’t know,” said he. “Don’t you know,” said I, “that the veritable lie, if the expression is permissible, is a thing that all gods and men abhor?” “What do you mean?” he said. “This,” said I, “that falsehood in the most vital part of themselves, and about their most vital concerns, is something that no one willingly accepts, but it is there above all that everyone fears it.” “I don’t understand yet either.” “That is because you suspect me of some grand meaning,” [382b] I said; “but what I mean is, that deception in the soul about realities, to have been deceived and to be blindly ignorant and to have and hold the falsehood there, is what all men would least of all accept, and it is in that case that they loathe it most of all.” “Quite so,” he said.
Related material —
A meditation from the Feast of St. Francis, 2012 —
A post from Sept. 30, 2012, the reported date of death
for British children's author Helen Nicoll —
The New Criterion on the death of Hilton Kramer —
This uncredited translation of Plato is, Google Books tells us,
by "Francis MacDonald Cornfield." The name is an error,
but the error is illuminating —
Depth
"… this notion of ‘depth’ is an elusive one
even for a mathematician who can recognize it…."
— G. H. Hardy, A Mathematician's Apology
Part I: An Inch Deep

Part II: An Inch Wide
See a search for "square inch space" in this journal.
See also recent posts with the tag depth.
Monday, March 10, 2014
Strategic Hamlets
From an obituary for a Kennedy advisor
who reportedly died at 94 on February 23, 2014*—
“He favored withdrawing rural civilians
into what he called ‘strategic hamlets’
and spraying defoliants to cut off
the enemy’s food supply.”
Other rhetoric: Hamlet and Infinite Space in this journal,
as well as King of Infinite Space , Part I and Part II.
These “King” links, to remarks on Coxeter and Saniga ,
are about two human beings to whom Hamlet’s
phrase “king of infinite space” has been applied.
The phrase would, of course, be more accurately
applied to God.
* The date of the ‘God’s Architecture’ sermon
at Princeton discussed in this afternoon’s post.
Sunday, March 9, 2014
The Story Creeps Up
For Women’s History Month —
Conclusion of “The Storyteller,” a story
by Cynthia Zarin about author Madeleine L’Engle—

See also the exercise on the Miracle Octad Generator (MOG) at the end of
the previous post, and remarks on the MOG by Emily Jennings (non -fiction)
on All Saints’ Day, 2012 (the date the L’Engle quote was posted here).
Hesse’s Table
From “Quartic Curves and Their Bitangents,” by
Daniel Plaumann, Bernd Sturmfels, and Cynthia Vinzant,
arXiv:1008.4104v2 [math.AG] 10 Jan 2011 —

The table mentioned (from 1855) is…
Exercise: Discuss the relationship, if any, to
the Miracle Octad Generator of R. T. Curtis.
At Play in the Fields of Brazil
From Facebook, a photo from the Feast of St. Francis, 2013:
Neantro Saavedra-Rivano, author of the 1976 paper “Finite
Geometries in the Theory of Theta Characteristics,” in Brasilia—
On the same date, art from Inception and from Diamonds Studio
in Brazil —
Sermon
On Theta Characteristics

— From Zentralblatt-math.org. 8 PM ET update: See also a related search.

Some may prefer a more politically correct— and simpler— sermon.
Background for the simpler sermon: Quilt Geometry.
Saturday, March 8, 2014
Conwell Heptads in Eastern Europe
“Charting the Real Four-Qubit Pauli Group
via Ovoids of a Hyperbolic Quadric of PG(7,2),”
by Metod Saniga, Péter Lévay and Petr Pracna,
arXiv:1202.2973v2 [math-ph] 26 Jun 2012 —
P. 4— “It was found that Q +(5,2) (the Klein quadric)
has, up to isomorphism, a unique one — also known,
after its discoverer, as a Conwell heptad [18].
The set of 28 points lying off Q +(5,2) comprises
eight such heptads, any two having exactly one
point in common.”
P. 11— “This split reminds us of a similar split of
63 points of PG(5,2) into 35/28 points lying on/off
a Klein quadric Q +(5,2).”
[18] G. M. Conwell, Ann. Math. 11 (1910) 60–76
A similar split occurs in yesterday’s Kummer Varieties post.
See the 63 = 28 + 35 vectors of R8 discussed there.
For more about Conwell heptads, see The Klein Correspondence,
Penrose Space-Time, and a Finite Model.
For my own remarks on the date of the above arXiv paper
by Saniga et. al., click on the image below —
Walter Gropius
Friday, March 7, 2014
Kummer Varieties
The Dream of the Expanded Field continues…
From Klein's 1893 Lectures on Mathematics —

"The varieties introduced by Wirtinger may be called Kummer varieties…."
— E. Spanier, 1956
From this journal on March 10, 2013 —
From a recent paper on Kummer varieties,
arXiv:1208.1229v3 [math.AG] 12 Jun 2013,
"The Universal Kummer Threefold," by
Qingchun Ren, Steven V Sam, Gus Schrader, and Bernd Sturmfels —

Two such considerations —
Update of 10 PM ET March 7, 2014 —
The following slides by one of the "Kummer Threefold" authors give
some background related to the above 64-point vector space and
to the Weyl group of type E7, W (E7):
The Cayley reference is to "Algorithm for the characteristics of the
triple ϑ-functions," Journal für die Reine und Angewandte
Mathematik 87 (1879): 165-169. <http://eudml.org/doc/148412>.
To read this in the context of Cayley's other work, see pp. 441-445
of Volume 10 of his Collected Mathematical Papers .
Monday, March 3, 2014
Blackboard Jungle Revisited

Blackboard Jungle , 1955
"We are going to keep doing this
until we get it right." — June 15, 2007
"Her wall is filled with pictures,
she gets 'em one by one" — Chuck Berry
See too a more advanced geometry lesson
that also uses the diagram pictured above.
Sunday, March 2, 2014
Sermon
Raiders of the Lost (Continued)
"Socrates: They say that the soul of man is immortal…."
From August 16, 2012—
In the geometry of Plato illustrated below,
"the figure of eight [square] feet" is not , at this point
in the dialogue, the diamond in Jowett's picture.
An 1892 figure by Jowett illustrating Plato's Meno—
A more correct version, from hermes-press.com —
|
Socrates: He only guesses that because the square is double, the line is double.Meno: True.
Socrates: Observe him while he recalls the steps in regular order. (To the Boy.) Tell me, boy, do you assert that a double space comes from a double line? Remember that I am not speaking of an oblong, but of a figure equal every way, and twice the size of this-that is to say of eight feet; and I want to know whether you still say that a double square comes from double line? [Boy] Yes. Socrates: But does not this line (AB) become doubled if we add another such line here (BJ is added)? [Boy] Certainly.
Socrates: And four such lines [AJ, JK, KL, LA] will make a space containing eight feet? [Boy] Yes. Socrates: Let us draw such a figure: (adding DL, LK, and JK). Would you not say that this is the figure of eight feet? [Boy] Yes. Socrates: And are there not these four squares in the figure, each of which is equal to the figure of four feet? (Socrates draws in CM and CN) [Boy] True. Socrates: And is not that four times four? [Boy] Certainly. Socrates: And four times is not double? [Boy] No, indeed. Socrates: But how much? [Boy] Four times as much. Socrates: Therefore the double line, boy, has given a space, not twice, but four times as much. [Boy] True. Socrates: Four times four are sixteen— are they not? [Boy] Yes. |
As noted in the 2012 post, the diagram of greater interest is
Jowett's incorrect version rather than the more correct version
shown above. This is because the 1892 version inadvertently
illustrates a tesseract:
A 4×4 square version, by Coxeter in 1950, of a tesseract—
This square version we may call the Galois tesseract.
Saturday, March 1, 2014
HaShem

From New World Encyclopedia —

See also Tetragrammaton in this journal.
For further context, see Solomon's Cube and Oct. 16, 2013.
Friday, February 28, 2014
Code
|
From Northrop Frye's The Great Code: The Bible and Literature , Ch. 3: Metaphor I — "In the preceding chapter we considered words in sequence, where they form narratives and provide the basis for a literary theory of myth. Reading words in sequence, however, is the first of two critical operations. Once a verbal structure is read, and reread often enough to be possessed, it 'freezes.' It turns into a unity in which all parts exist at once, without regard to the specific movement of the narrative. We may compare it to the study of a music score, where we can turn to any part without regard to sequential performance. The term 'structure,' which we have used so often, is a metaphor from architecture, and may be misleading when we are speaking of narrative, which is not a simultaneous structure but a movement in time. The term 'structure' comes into its proper context in the second stage, which is where all discussion of 'spatial form' and kindred critical topics take their origin." |
Related material:
|
"The Great Code does not end with a triumphant conclusion or the apocalypse that readers may feel is owed them or even with a clear summary of Frye’s position, but instead trails off with a series of verbal winks and nudges. This is not so great a fault as it would be in another book, because long before this it has been obvious that the forward motion of Frye’s exposition was illusory, and that in fact the book was devoted to a constant re-examination of the same basic data from various closely related perspectives: in short, the method of the kaleidoscope. Each shake of the machine produces a new symmetry, each symmetry as beautiful as the last, and none of them in any sense exclusive of the others. And there is always room for one more shake."
— Charles Wheeler, "Professor Frye and the Bible," South Atlantic Quarterly 82 (Spring 1983), pp. 154-164, reprinted in a collection of reviews of the book. |
For code in a different sense, but related to the first passage above,
see Diamond Theory Roullete, a webpage by Radamés Ajna.
For "the method of the kaleidoscope" mentioned in the second
passage above, see both the Ajna page and a webpage of my own,
Kaleidoscope Puzzle.
Thursday, February 27, 2014
The Crosswicks Curse
"There is such a thing as a tesseract."
— Saying from Crosswicks
See also March 5, 2011.
Adapted from the above passage —
"So did L'Engle understand four-dimensional geometry?"
Saturday, February 22, 2014
Green Fields
Some narrative notes in memory of a
Bowling Green State University math professor
who reportedly died at 72 on Feb. 13—
That date in this journal and Green Fields.
See also Nine is a Vine.
Those who prefer mathematics to narrative may
also prefer to read, instead of the notes above,
some material on the dead professor's specialty,
Diophantine equations. Recommended:
Mordell on Lang and Lang on Mordell as well as
Lang's article titled
"Mordell's Review, Siegel's Letter to Mordell,
Diophantine Geometry, and 20th Century Mathematics."
Some background —
Friday, February 21, 2014
Raumproblem*
Despite the blocking of Doodles on my Google Search
screen, some messages get through.
Today, for instance —
"Your idea just might change the world.
Enter Google Science Fair 2014"
Clicking the link yields a page with the following image—

Clearly there is a problem here analogous to
the square-triangle coordinatization problem,
but with the 4×6 rectangle of the R. T. Curtis
Miracle Octad Generator playing the role of
the square.
I once studied this 24-triangle-hexagon
coordinatization problem, but was unable to
obtain any results of interest. Perhaps
someone else will have better luck.
* For a rather different use of this word,
see Hermann Weyl in the Stanford
Encyclopedia of Philosophy.
Night’s Hymn of the Rock
One way of interpreting the symbol ![]()
at the end of yesterday's post is via
the phrase "necessary possibility."
See that phrase in (for instance) a post
of July 24, 2013, The Broken Tablet .
The Tablet post may be viewed in light
of a Tom Wolfe passage quoted here on
the preceding day, July 23, 2013—

On that day (July 23) another weblog had
a post titled
Wallace Stevens: Night's Hymn of the Rock.
Some related narrative —
I prefer the following narrative —
Part I: Stevens's verse from "The Rock" (1954) —
"That in which space itself is contained"
Part II: Mystery Box III: Inside, Outside (2014)
Thursday, February 20, 2014
Relativity Blues
A review of this date in 2005 —
Modal Theology
“We symbolize logical necessity
with the box (![]() )
)
and logical possibility
with the diamond (![]() ).”
).”
— Keith Allen Korcz
And what do we
symbolize by ![]() ?
?
Tuesday, February 18, 2014
Eichler’s Reciprocity Law
Edward Frenkel on Eichler's reciprocity law
(Love and Math , Kindle edition of 2013-10-01,
page 88, location 1812)—
"It seems nearly unbelievable that there
would be a rule generating these numbers.
And yet, German mathematician Martin
Eichler discovered one in 1954.11 "
"11. I follow the presentation of this result
given in Richard Taylor, Modular arithmetic:
driven by inherent beauty and human
curiosity , The Letter of the Institute for
Advanced Study [IAS], Summer 2012,
pp. 6– 8. I thank Ken Ribet for useful
comments. According to André Weil’s book
Dirichlet Series and Automorphic Forms ,
Springer-Verlag, 1971 [pp. 143-144], the
cubic equation we are discussing in this
chapter was introduced by John Tate,
following Robert Fricke."
|
Update of Feb. 19:
Actually, the cubic equation discussed Y 2 + Y = X 3 – X 2
whereas the equation given by Weil, Y 2 – Y = X 3 – X 2 .
Whether this is a misprint in Weil's book,
At any rate, the cubic equation discussed by
For further background, see (for instance) |
Richard Taylor, op. cit. —
|
One could ask for a similar method that given any number of polynomials in any number of variables helps one to determine the number of solutions to those equations in arithmetic modulo a variable prime number p . Such results are referred to as “reciprocity laws.” In the 1920s, Emil Artin gave what was then thought to be the most general reciprocity law possible—his abelian reciprocity law. However, Artin’s reciprocity still only applied to very special equations—equations with only one variable that have “abelian Galois group.” Stunningly, in 1954, Martin Eichler (former IAS Member) found a totally new reciprocity law, not included in Artin’s theorem. (Such reciprocity laws are often referred to as non-abelian.) More specifically, he found a reciprocality [sic ] law for the two variable equation Y 2 + Y = X 3 – X 2. He showed that the number of solutions to this equation in arithmetic modulo a prime number p differs from p [in the negative direction] by the coefficient of q p in the formal (infinite) product
q (1 – q 2 )(1 – q 11) 2 (1 – q 2)2 For example, you see that the coefficient of q5 is 1, so Eichler’s theorem tells us that Y 2 + Y = X 3 − X 2 should have 5 − 1 = 4 solutions in arithmetic modulo 5. You can check this by checking the twenty-five possibilities for (X,Y) modulo 5, and indeed you will find exactly four solutions: (X,Y) ≡ (0,0), (0,4), (1,0), (1,4) mod 5. Within less than three years, Yutaka Taniyama and Goro Shimura (former IAS Member) proposed a daring generalization of Eichler’s reciprocity law to all cubic equations in two variables. A decade later, André Weil (former IAS Professor) added precision to this conjecture, and found strong heuristic evidence supporting the Shimura-Taniyama reciprocity law. This conjecture completely changed the development of number theory. |
With this account and its context, Taylor has
perhaps atoned for his ridiculous remarks
quoted at Log24 in The Proof and the Lie.
Saturday, February 15, 2014
Rosenhain and Göpel
See The Oslo Version in this journal and the New Year’s Day 2014 post.
The pictures of the 56 spreads in that post (shown below) are based on
the 20 Rosenhain and 15 Göpel tetrads that make up the 35 lines of
PG(3,2), the finite projective 3-space over the 2-element Galois field.
Click for a larger image.
Friday, February 14, 2014
Epiphany
“… the object sets up a kind of
frame or space or field
within which there can be epiphany.”

Related material —
Star Wars (January 11, 2014),
The Lyche Gate Asterisk , from 10:31 AM ET on May 22, 2010,
the date of Martin Gardner's death —

— and the March 2014 issue of the
Notices of the American Mathematical Society —
See as well Epiphany 2014 (Jan. 6) in this journal and the
March Notices on the Shaw prize —
"Established under the auspices of Run Run Shaw
in November 2002, the prize is managed and
administered by the Shaw Prize Foundation
based in Hong Kong."
Wednesday, February 12, 2014
But Seriously…
(A sequel to yesterday's Raiders of the Lost Music Box)
See, in this book, "Walsh Functions: A Digital Fourier Series,"
by Benjamin Jacoby (BYTE , September 1977). Some context:
Symmetry of Walsh Functions.
Monday, February 10, 2014
Mystery Box III: Inside, Outside
(Continued from Mystery Box, Feb. 4, and Mystery Box II, Feb. 5.)
The Box

Inside the Box
Outside the Box
For the connection of the inside notation to the outside geometry,
see Desargues via Galois.
(For a related connection to curves and surfaces in the outside
geometry, see Hudson's classic Kummer's Quartic Surface and
Rosenhain and Göpel Tetrads in PG(3,2).)
Thursday, February 6, 2014
The Representation of Minus One
For the late mathematics educator Zoltan Dienes.
“There comes a time when the learner has identified
the abstract content of a number of different games
and is practically crying out for some sort of picture
by means of which to represent that which has been
gleaned as the common core of the various activities.”
— Article by “Melanie” at Zoltan Dienes’s website
Dienes reportedly died at 97 on Jan. 11, 2014.
From this journal on that date —

A star figure and the Galois quaternion.
The square root of the former is the latter.
Update of 5:01 PM ET Feb. 6, 2014 —
An illustration by Dienes related to the diamond theorem —
See also the above 15 images in …
… and versions of the 4×4 coordinatization in The 4×4 Relativity Problem
(Jan. 17, 2014).
Wednesday, February 5, 2014
Mystery Box II
Continued from previous post and from Sept. 8, 2009.

Examination of the box's contents does not solve
the contents' real mystery. That requires knowledge
of the non-Euclidean geometry of Galois space.
In this case, without that knowledge, prattle (as in
today's online New York Times ) about creativity and
"thinking outside the box" is pointless.
Tuesday, February 4, 2014
Sunday, February 2, 2014
Diamond Theory Roulette
A ReCode Project program from Radamés Ajna of São Paulo —
At the program's webpage, click the image to
generate random permutations of rows, columns,
and quadrants. Note the resulting image's ordinary
or color-interchange symmetry.

|
Saturday, February 1, 2014
ART WARS (continued)
A sequel to Friday afternoon's Diamond Star
Diamond Star —

|
A doodle from this year's [2012’s] Feast of the Epiphany—
A doodle based on today's previous post and on
|
Context — All posts tagged "Eden."
The Delft Version
My webpage "The Order-4 Latin Squares" has a rival—
"Latin squares of order 4: Enumeration of the
24 different 4×4 Latin squares. Symmetry and
other features."
The author — Yp de Haan, a professor emeritus of
materials science at Delft University of Technology —
The main difference between de Haan's approach and my own
is my use of the four-color decomposition theorem, a result that
I discovered in 1976. This would, had de Haan known it, have
added depth to his "symmetry and other features" remarks.
Sunday, January 26, 2014
Blazing Bride’s Chair
A sequel to last night's link Shear —
Some dead poet's words —
The "bride's chair" is the figure illustrating Euclid's proof
of the Pythagorean theorem (click image to enlarge) —
See also…
Not since Madeline Kahn in Blazing Saddles …
A Midnight Exorcism
The summoning of the spirit of Bertrand Russell
yesterday by Peter J. Cameron at his weblog
suggests a review of this weblog’s posts of
Christmas Eve, December 24-25, 2013.
(Recall that Robert D. Carmichael, who, in a book
linked to at midnight last Christmas Eve discusses
some “magic” mathematical structures,
reportedly was trained as a Presbyterian minister.
See also The Presbyterian Exorcist.)
Thursday, January 23, 2014
Riddled
From this journal on Dec. 20, 2003 ("White, Geometric, and Eternal") —
Riddled:
The Absolutist Faith
of The New York Times

See also Dead Poets' Word and A Riddle for Davos, as well as…
Wednesday, January 22, 2014
A Riddle for Davos
Einstein and Thomas Mann, Princeton, 1938
See also the life of Diogenes Allen, a professor at Princeton
Theological Seminary, a life that reportedly ended on the date—
January 13, 2013— of the above Log24 post.
January 13 was also the dies natalis of St. James Joyce.
Some related reflections —

"Praeterit figura huius mundi " — I Corinthians 7:31 —

|
Conclusion of of "The Dead," by James Joyce— The air of the room chilled his shoulders. He stretched himself cautiously along under the sheets and lay down beside his wife. One by one, they were all becoming shades. Better pass boldly into that other world, in the full glory of some passion, than fade and wither dismally with age. He thought of how she who lay beside him had locked in her heart for so many years that image of her lover's eyes when he had told her that he did not wish to live. Generous tears filled Gabriel's eyes. He had never felt like that himself towards any woman, but he knew that such a feeling must be love. The tears gathered more thickly in his eyes and in the partial darkness he imagined he saw the form of a young man standing under a dripping tree. Other forms were near. His soul had approached that region where dwell the vast hosts of the dead. He was conscious of, but could not apprehend, their wayward and flickering existence. His own identity was fading out into a grey impalpable world: the solid world itself, which these dead had one time reared and lived in, was dissolving and dwindling. A few light taps upon the pane made him turn to the window. It had begun to snow again. He watched sleepily the flakes, silver and dark, falling obliquely against the lamplight. The time had come for him to set out on his journey westward. Yes, the newspapers were right: snow was general all over Ireland. It was falling on every part of the dark central plain, on the treeless hills, falling softly upon the Bog of Allen and, farther westward, softly falling into the dark mutinous Shannon waves. It was falling, too, upon every part of the lonely churchyard on the hill where Michael Furey lay buried. It lay thickly drifted on the crooked crosses and headstones, on the spears of the little gate, on the barren thorns. His soul swooned slowly as he heard the snow falling faintly through the universe and faintly falling, like the descent of their last end, upon all the living and the dead. |
Saturday, January 18, 2014
The Triangle Relativity Problem
A sequel to last night's post The 4×4 Relativity Problem —
In other words, how should the triangle corresponding to
the above square be coordinatized ?
See also a post of July 8, 2012 — "Not Quite Obvious."
Context — "Triangles Are Square," a webpage stemming
from an American Mathematical Monthly item published
in 1984.
Friday, January 17, 2014
The 4×4 Relativity Problem
The sixteen-dot square array in yesterday’s noon post suggests
the following remarks.
“This is the relativity problem: to fix objectively a class of
equivalent coordinatizations and to ascertain the group of
transformations S mediating between them.”
— Hermann Weyl, The Classical Groups ,
Princeton University Press, 1946, p. 16
The Galois tesseract appeared in an early form in the journal
Computer Graphics and Art , Vol. 2, No. 1, February 1977—
The 1977 matrix Q is echoed in the following from 2002—

A different representation of Cullinane’s 1977 square model of the
16-point affine geometry over the two-element Galois field GF(2)
is supplied by Conway and Sloane in Sphere Packings, Lattices and Groups
(first published in 1988) :
Here a, b, c, d are basis vectors in the vector 4-space over GF(2).
(For a 1979 version of this vector space, see AMS Abstract 79T-A37.)
See also a 2011 publication of the Mathematical Association of America —
Thursday, January 16, 2014
Confession of a Sucker
Today’s 11 AM (ET) post was suggested by a New York Times
article, online yesterday, about art gallery owner Lisa Cooley.
A check of Cooley’s website yields the image below,
related to Beckett’s Molloy .
For the relevant passage from Molloy , click the following:
“I took advantage of being at the seaside
to lay in a store of sucking-stones.“
For posts on Molloy in this journal, click Beckett + Molloy .
Cynthia Daignault, 2011:
The one I shall now describe, if I can…
Oil on linen, in 2 parts: 40 x 30 inches, 96 x 75 inches
Related art theory —
Wednesday, January 15, 2014
Entertainment Theory
From "Entertainment," a 1981 story by M. A. Foster—
|
"For some time, Cormen had enjoyed a peculiar suspicion, which he had learned from his wanderings around the city, and cultivated with a little notebook, in which he had made a detailed series of notes and jottings, as well as crude, but effective, charts and maps of certain districts. 'Cormen's Problem,' as it was known, was familiar to the members of the circle in which he moved; in fact, if he had not been so effective with his productions and so engaging in his personality, they might have considered him a bore. It seemed, so the suspicion went, that the city was slowly shrinking, as evidenced by abandoned districts along the city edges. Beyond the empty houses were ruins, and beyond that, traces of foundations and street lines. Moreover, it had recently dawned on him that there were no roads out of the city, although there were no restraints. One hardly noticed this—it was the norm. But like many an easy assumption, once broken it became increasingly obvious. Cormen's acquaintances were tolerant of his aberration, but generally unsympathetic. What he needed was proof, something he could demonstrate in black and white—and color if required. But the city was reluctant, so it appeared, to give up its realities so easily. The Master Entertainment Center, MEC, would not answer direct queries about this, even though it would obediently show him presentations, pictorial or symbolic as he required, of the areas in question. But it was tiresome detail work, in which he had to proceed completely on his own." |
Lily Collins in City of Bones (2013)—
American Folk Art (see August 23, 2011) —

See as well Ballet Blanc .
Tuesday, January 14, 2014
Release Date
The premiere of the Lily Collins film Abduction
(see previous post) was reportedly in Sydney, Australia,
on August 23, 2011.
From that date in this journal—

For the eight-limbed star at the top of the quaternion array above, She drew from her handbag a pale grey gleaming implement that looked by quick turns to me like a knife, a gun, a slim sceptre, and a delicate branding iron—especially when its tip sprouted an eight-limbed star of silver wire. “The test?” I faltered, staring at the thing. “Yes, to determine whether you can live in the fourth dimension or only die in it.” — Fritz Leiber, short story, 1959 |
Related material from Wikipedia, suggested by the reference quoted
in this morning's post to "a four-dimensionalist (perdurantist) ontology"—
"… perdurantism also applies if one believes there are temporal
but non-spatial abstract entities (like immaterial souls…)."
Monday, January 13, 2014
A Prime for Marissa
Sunday, January 12, 2014
The Feast
"And in the Master's chambers…" — Eagles
Related fiction — Amy + Dinner and today's previous post.
Related non-fiction — a page of pure mathematics —










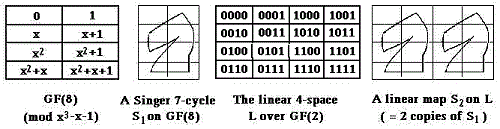








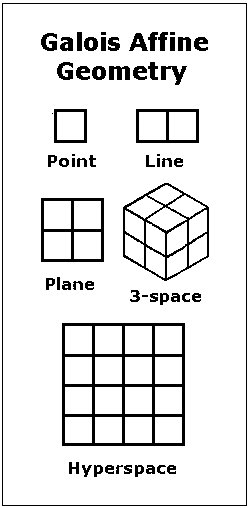















































































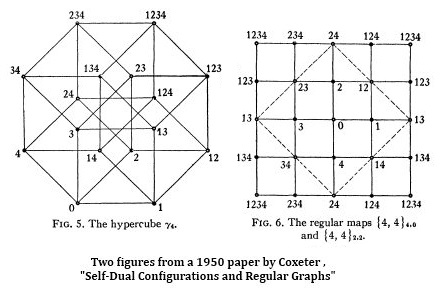
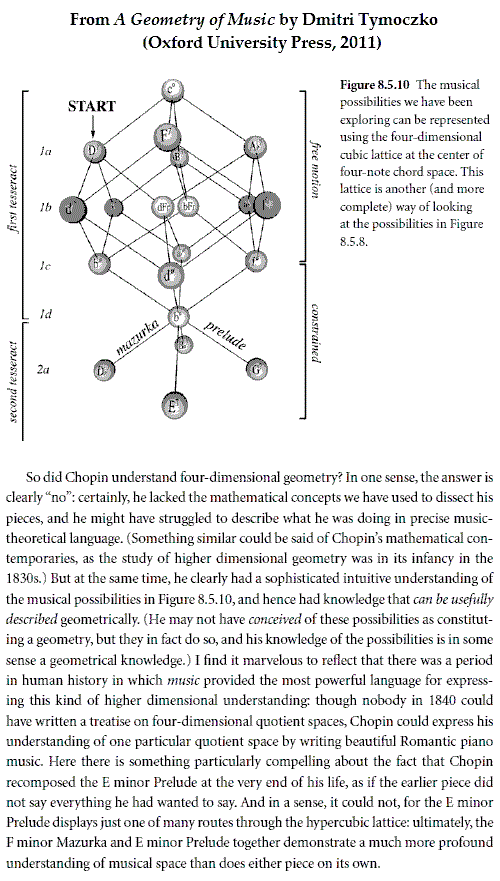













 .
.



















