Down the Up Ladder
Sunday, September 1, 2019
Symmetry and the Quaternion Group:
Tuesday, May 28, 2019
Quaternion at Candlebrow
From a Groundhog Day post in 2009 —
|
The Candlebrow Conference The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries. "Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–" "Um, Professor–"…. … Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"…. … In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions…. "Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions." "Build a time machine." "Not the way I would have put it, but if you like, fine." Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up…. "We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly." "Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened. Above, the devastation had begun. |
"As if the professor had lectured it into being . . . ."
See other posts now tagged McLuhan Time.
Sunday, December 9, 2018
Quaternions in a Small Space
The previous post, on the 3×3 square in ancient China,
suggests a review of group actions on that square
that include the quaternion group.
Click to enlarge —
Three links from the above finitegeometry.org webpage on the
quaternion group —
-
Visualizing GL(2,p) — A 1985 note illustrating group actions
on the 3×3 (ninefold) square. -
Another 1985 note showing group actions on the 3×3 square
transferred to the 2x2x2 (eightfold) cube. - Quaternions in an Affine Galois Plane — A webpage from 2010.
Related material —
See as well the two Log24 posts of December 1st, 2018 —
Character and In Memoriam.
Thursday, May 31, 2018
Quaternion Group Models
Tuesday, August 8, 2017
Thursday, June 5, 2014
Twisty Quaternion Symmetry
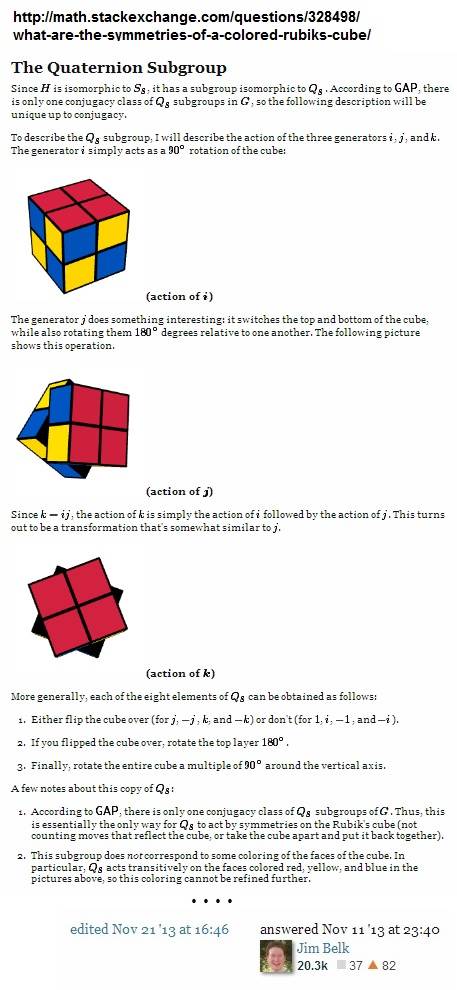
The previous post told how user58512 at math.stackexchange.com
sought in 2013 a geometric representation of Q8 , the quaternion group.
He ended up displaying an illustration that very possibly was drawn,
without any acknowledgement of its source, from my own work.
On the date that user58512 published that illustration, he further
pursued his March 1, 2013, goal of a “twisty” quaternion model.
On March 12, 2013, he suggested that the quaternion group might be
the symmetry group of the following twisty-cube coloring:
Illustration by Jim Belk
Here is part of a reply by Jim Belk from Nov. 11, 2013, elaborating on
that suggestion:
Belk argues that the colored cube is preserved under the group
of actions he describes. It is, however, also preserved under a
larger group. (Consider, say, rotation of the entire cube by 180
degrees about the center of any one of its checkered faces.) The
group Belk describes seems therefore to be a symmetry group,
not the symmetry group, of the colored cube.
I do not know if any combination puzzle has a coloring with
precisely the quaternion group as its symmetry group.
(Updated at 12:15 AM June 6 to point out the larger symmetry group
and delete a comment about an arXiv paper on quaternion group models.)
Saturday, May 31, 2014
Quaternion Group Models:
The ninefold square, the eightfold cube, and monkeys.

For posts on the models above, see quaternion
in this journal. For the monkeys, see
"Nothing Is More Fun than a Hypercube of Monkeys,"
Evelyn Lamb's Scientific American weblog, May 19, 2014:
The Scientific American item is about the preprint
"The Quaternion Group as a Symmetry Group,"
by Vi Hart and Henry Segerman (April 26, 2014):
See also Finite Geometry and Physical Space.
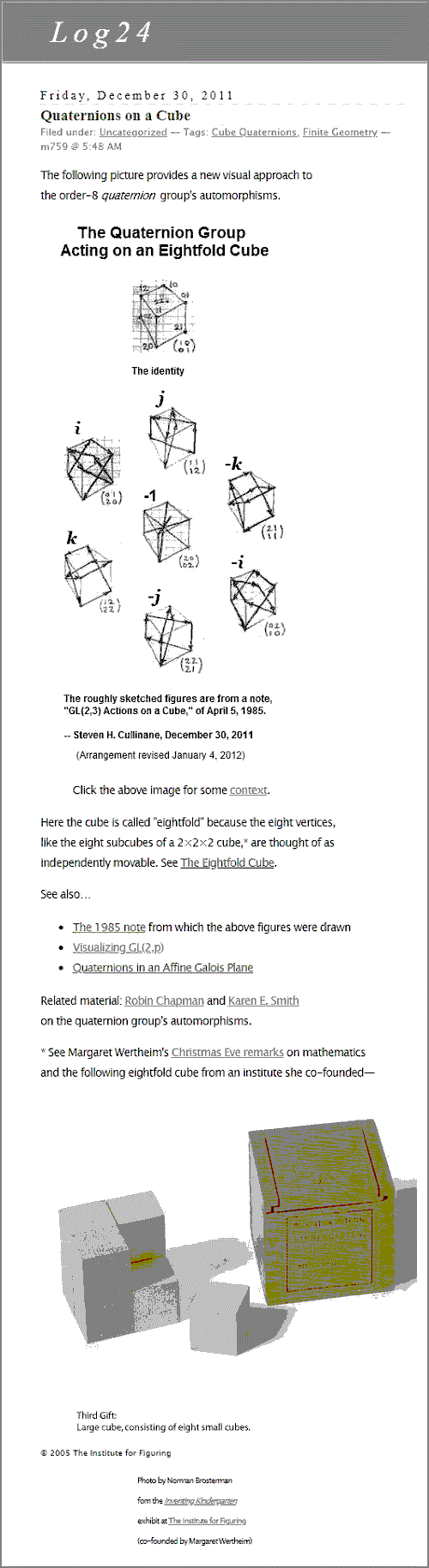
Friday, December 30, 2011
Quaternions on a Cube
The following picture provides a new visual approach to
the order-8 quaternion group's automorphisms.
Click the above image for some context.
Here the cube is called "eightfold" because the eight vertices,
like the eight subcubes of a 2×2×2 cube,* are thought of as
independently movable. See The Eightfold Cube.
See also…
- The 1985 note from which the above figures were drawn
- Visualizing GL(2,p)
- Quaternions in an Affine Galois Plane
Related material: Robin Chapman and Karen E. Smith
on the quaternion group's automorphisms.
* See Margaret Wertheim's Christmas Eve remarks on mathematics
and the following eightfold cube from an institute she co-founded—

© 2005 The Institute for Figuring
Photo by Norman Brosterman
fom the Inventing Kindergarten
exhibit at The Institute for Figuring
(co-founded by Margaret Wertheim)
Saturday, October 16, 2010
Quaternion Day
Brightness at Noon continued
— Sir William Rowan Hamilton, Oct. 16, 1843
See also this journal on 1/09, 2010.
Thursday, February 23, 2023
“Where Whirled and Well”
— Conrad Aiken, Great Circle
And the light shone in darkness and
Against the Word the unstilled world still whirled
About the centre of the silent Word.
— T. S. Eliot, "Ash Wednesday"
About the Centre:
See also Dorm Room.
Friday, December 23, 2022
“Was ist Raum?” — Bauhaus Founder Walter Gropius

"Was ist Raum, wie können wir ihn
erfassen und gestalten?"
The Theory and
Organization of the
Bauhaus (1923)
A relevant illustration:
At math.stackexchange.com on March 1-12, 2013:
“Is there a geometric realization of the Quaternion group?” —
The above illustration, though neatly drawn, appeared under the
cloak of anonymity. No source was given for the illustrated group actions.
Possibly they stem from my Log24 posts or notes such as the Jan. 4, 2012,
note on quaternion actions at finitegeometry.org/sc (hence ultimately
from my note “GL(2,3) actions on a cube” of April 5, 1985).
These references will not appeal to those who enjoy modernism as a religion.
(For such a view, see Rosalind Krauss on grids and another writer's remarks
on the religion's 100th anniversary this year.)
Some related nihilist philosophy from Cormac McCarthy —
"Forms turning in a nameless void."
Wednesday, December 14, 2022
Plan 9 From Moriarty

Some related mathematical windmills —
|
For the eight-limbed star at the top of the quaternion array She drew from her handbag a pale grey gleaming implement that looked by quick turns to me like a knife, a gun, a slim sceptre, and a delicate branding iron—especially when its tip sprouted an eight-limbed star of silver wire. “The test?” I faltered, staring at the thing. “Yes, to determine whether you can live in the fourth dimension or only die in it.” — Fritz Leiber, short story, 1959 |
See as well . . .
Sunday, October 16, 2022
For Broomsday: Turning Eight
Friday, February 12, 2021
Root
A star figure and the Galois quaternion.
The square root of the former is the latter.
(This post was suggested by the definition of
the Dirac operator as a square root.)
Tuesday, July 23, 2019
Saturday, June 8, 2019
Monday, May 27, 2019
The Broom Bridge Eight
"In this way the eight quaternions came into being."
— Legend adapted from Richard Wilhelm.
See as well The Bond with Reality (20th of May, 2019).
Sunday, May 26, 2019
Sunday, January 6, 2019
For Broom Bridge*
GL(2,3) is not unrelated to GL(3,2).
See Quaternion Automorphisms
and Spinning in Infinity.

* See Wikipedia.
Thursday, May 31, 2018
Eightfold Suffering:
A New, Improved Version of Quantum Suffering !

Background for group actions on the eightfold cube —

See also other posts now tagged Quantum Suffering
as well as — related to the image above of the Great Wall —
Hart the Knife*
For Spaceballs fans . . .
A web page from the father of Vi Hart, co-author of the
2014 quaternion-model article cited in the previous post:
* The title was suggested by the following video —
Friday, May 4, 2018
Art & Design

A star figure and the Galois quaternion.
The square root of the former is the latter.
See also a passage quoted here a year ago today
(May the Fourth, "Star Wars Day") —
Saturday, January 20, 2018
The Chaos Symbol of Dan Brown
In the following passage, Dan Brown claims that an eight-ray star
with arrowheads at the rays’ ends is “the mathematical symbol for
entropy.” Brown may have first encountered this symbol at a
questionable “Sacred Science” website. Wikipedia discusses
some even less respectable uses of the symbol.

Related news —
Related symbolism —

A star figure and the Galois quaternion.
The square root of the former is the latter.
Saturday, October 14, 2017
Saturday, September 23, 2017
The Turn of the Year
Wednesday, April 12, 2017
Contracting the Spielraum
The contraction of the title is from group actions on
the ninefold square (with the center subsquare fixed)
to group actions on the eightfold cube.
From a post of June 4, 2014 …
At math.stackexchange.com on March 1-12, 2013:
“Is there a geometric realization of the Quaternion group?” —

The above illustration, though neatly drawn, appeared under the
cloak of anonymity. No source was given for the illustrated group actions.
Possibly they stem from my Log24 posts or notes such as the Jan. 4, 2012,
note on quaternion actions at finitegeometry.org/sc (hence ultimately
from my note “GL(2,3) actions on a cube” of April 5, 1985).
Thursday, February 23, 2017
Too Clever by Half
"What Yokoyama does in Six Four evokes — improbably —
the fastidious ethical parsings of a novel by Henry James,
all qualms and calibrations, and while that might not sound
like a good idea, he makes it work. He writes, fortunately,
in plain, declarative prose (ably translated by Jonathan Lloyd-Davies),
and because Mikami is such an ordinary man the mental gymnastics
he puts himself through are moving and sometimes deeply funny.
A Jamesian police procedural — 'The Wings of the Perp,' maybe?
Not exactly. But this novel is a real, out-of-the-blue original.*
I’ve never read anything like it."
— Terrence Rafferty in the online New York Times
on Tuesday, Feb. 21, 2017
Illustration from this journal on that date —

See also the previous post, "Six Four."
Update of 10 PM ET Feb. 23, 2017 —
A pathetic asterisk for, and by, Rafferty —
* A passage from Rafferty's essay
in The New York Times on July 27, 2003:
"… the message is clear: screenwriters are pathetic.
You can hear, clearly, the voice of Joe Gillis,
describing himself from beyond his watery grave:
'Nobody important, really. Just a movie writer with
a couple of B pictures to his credit. The poor dope.' "
See as well this journal on July 27, 2003.
Tuesday, February 21, 2017
Tetradion …
… Also known as quaternion —

"Diagram of an 8 leaf gathering: Quaternion (8 folio or leaf gathering).
A quaternion is composed of 4 bifolios. Conjugate folios form a bifolio
at either end of a gathering or quire. So in the diagram above folios
1 and 8 which form a bifolio are conjugate folios."
— http://employees.oneonta.edu/farberas/arth/arth214_folder/workshop.htm
The source:
SUNY Oneonta
ARTH 214
History of Northern Renaissance Art
Spring, 2013
Dr. Allen Farber, Associate Professor
Tuesday, February 26: From Workshop to Chamber:
The Paris Book Industry of the Early Fifteenth Century
"Images for class" folder
Synchronology:
An image from Publication, a Log24 post on the above date,
Monday, February 20, 2017
Tetrad
Wiktionary —
|
Russian noun . . .
тетра́дь • (tetrádʹ) f inan
Etymology
|
See also Tetrad in this journal.
Friday, August 26, 2016
Structure a Set, Set a Structure

A star figure and the Galois quaternion.
The square root of the former is the latter.
See also a search in this journal for "Set a Structure."
Tuesday, June 28, 2016
Schicksalstag
Thursday, December 17, 2015
Hint of Reality
From an article* in Proceedings of Bridges 2014 —
|
As artists, we are particularly interested in the symmetries of real world physical objects. Three natural questions arise: 1. Which groups can be represented as the group of symmetries of some real-world physical object? 2. Which groups have actually been represented as the group of symmetries of some real-world physical object? 3. Are there any glaring gaps – small, beautiful groups that should have a physical representation in a symmetric object but up until now have not? |
The article was cited by Evelyn Lamb in her Scientific American
weblog on May 19, 2014.
The above three questions from the article are relevant to a more
recent (Oct. 24, 2015) remark by Lamb:
"… finite projective planes [in particular, the 7-point Fano plane,
about which Lamb is writing] seem like a triumph of purely
axiomatic thinking over any hint of reality…."
For related hints of reality, see Eightfold Cube in this journal.
* "The Quaternion Group as a Symmetry Group," by Vi Hart and Henry Segerman
Thursday, August 13, 2015
Anniversary
Today is reportedly the anniversary of the death,
in Paris in 1822, of Jean Robert Argand.
Some related material …
"Wessel's fame as a mathematician rests solely
on this paper, which was published in 1799,
giving for the first time a geometrical interpretation
of complex numbers. Today we call this geometric
interpretation the Argand diagram but Wessel's
work came first. It was rediscovered by Argand
in 1806 and again by Gauss in 1831. ….
Of course it is not unreasonable to call the
geometrical interpretation of complex numbers
the Argand diagram since it was Argand's work
which was influential. It was so named before
the world of mathematics learnt of Wessel's prior
publication. In fact Wessel's paper was not
noticed by the mathematical community until 1895…."
See also Tilting at Whirligigs (Log24 on March 8, 2008)
and The Galois Quaternion.
Wednesday, June 24, 2015
Logos
The Santa Fe Institute logo, together with the previous post,
suggests a review of Whirligig and Quaternion for Goldstein.
Tuesday, October 21, 2014
Eerie Twist
(Continued from Nov. 15, 2011)
Ben Bradlee, legendary Washington Post editor, dies at 93
See also a post of Jan. 20, 2011, and an earlier post on Twelfth Night, 2010.

A star figure and the Galois quaternion.
The square root of the former is the latter.
Thursday, October 16, 2014
Seeking Kleos
Rebecca Newberger Goldstein, quoted in a webpage dated
October 7, 2014 (presumably according to Australian time):
"For the Athenians, kleos mattered more than anything,
according to Goldstein.
'Kleos is fame: it’s the deed that brings fame, it’s the poem
that sings your triumphs, it’s having your life replicated in
other minds, acquiring a kind of moreness, a kind of
secular immortality.' "
Related material:
A check of Goldstein's definition…

… and an image for Broomsday:

From Argument for the Existence of Rebecca (Feb. 6, 2010)
Broom Bridge Day
Wikipedia on Broom (or Broome, or Brougham) Bridge,
where on 16 October 1843 Hamilton discovered quaternions:
"The 16 October is sometimes referred to as
Broomsday (in reference to Broome Bridge)
and as a nod to the literary commemorations
on 16 June (Bloomsday in honour of James Joyce)."
See also, in this journal, The Craft.
Monday, October 13, 2014
Raiders of the Lost Theorem
(Continued from Nov. 16, 2013.)
The 48 actions of GL(2,3) on a 3×3 array include the 8-element
quaternion group as a subgroup. This was illustrated in a Log24 post,
Hamilton’s Whirligig, of Jan. 5, 2006, and in a webpage whose
earliest version in the Internet Archive is from June 14, 2006.
One of these quaternion actions is pictured, without any reference
to quaternions, in a 2013 book by a Netherlands author whose
background in pure mathematics is apparently minimal:

In context (click to enlarge):
Update of later the same day —
Lee Sallows, Sept. 2011 foreword to Geometric Magic Squares —
“I first hit on the idea of a geometric magic square* in October 2001,**
and I sensed at once that I had penetrated some previously hidden portal
and was now standing on the threshold of a great adventure. It was going
to be like exploring Aladdin’s Cave. That there were treasures in the cave,
I was convinced, but how they were to be found was far from clear. The
concept of a geometric magic square is so simple that a child will grasp it
in a single glance. Ask a mathematician to create an actual specimen and
you may have a long wait before getting a response; such are the formidable
difficulties confronting the would-be constructor.”
* Defined by Sallows later in the book:
“Geometric or, less formally, geomagic is the term I use for
a magic square in which higher dimensional geometrical shapes
(or tiles or pieces ) may appear in the cells instead of numbers.”
** See some geometric matrices by Cullinane in a March 2001 webpage.
Earlier actual specimens — see Diamond Theory excerpts published in
February 1977 and a brief description of the original 1976 monograph:
“51 pp. on the symmetries & algebra of
matrices with geometric-figure entries.”
— Steven H. Cullinane, 1977 ad in
Notices of the American Mathematical Society
The recreational topic of “magic” squares is of little relevance
to my own interests— group actions on such matrices and the
matrices’ role as models of finite geometries.
Wednesday, June 4, 2014
Monkey Business
The title refers to a Scientific American weblog item
discussed here on May 31, 2014:
Some closely related material appeared here on
Dec. 30, 2011:
A version of the above quaternion actions appeared
at math.stackexchange.com on March 12, 2013:
"Is there a geometric realization of Quaternion group?" —

The above illustration, though neatly drawn, appeared under the
cloak of anonymity. No source was given for the illustrated group actions.
Possibly they stem from my Log24 posts or notes such as the Jan. 4, 2012,
note on quaternion actions at finitegeometry.org/sc (hence ultimately
from my note "GL(2,3) actions on a cube" of April 5, 1985).
Thursday, February 6, 2014
The Representation of Minus One
For the late mathematics educator Zoltan Dienes.
“There comes a time when the learner has identified
the abstract content of a number of different games
and is practically crying out for some sort of picture
by means of which to represent that which has been
gleaned as the common core of the various activities.”
— Article by “Melanie” at Zoltan Dienes’s website
Dienes reportedly died at 97 on Jan. 11, 2014.
From this journal on that date —

A star figure and the Galois quaternion.
The square root of the former is the latter.
Update of 5:01 PM ET Feb. 6, 2014 —
An illustration by Dienes related to the diamond theorem —
See also the above 15 images in …
… and versions of the 4×4 coordinatization in The 4×4 Relativity Problem
(Jan. 17, 2014).
Wednesday, January 15, 2014
Entertainment Theory
From "Entertainment," a 1981 story by M. A. Foster—
|
"For some time, Cormen had enjoyed a peculiar suspicion, which he had learned from his wanderings around the city, and cultivated with a little notebook, in which he had made a detailed series of notes and jottings, as well as crude, but effective, charts and maps of certain districts. 'Cormen's Problem,' as it was known, was familiar to the members of the circle in which he moved; in fact, if he had not been so effective with his productions and so engaging in his personality, they might have considered him a bore. It seemed, so the suspicion went, that the city was slowly shrinking, as evidenced by abandoned districts along the city edges. Beyond the empty houses were ruins, and beyond that, traces of foundations and street lines. Moreover, it had recently dawned on him that there were no roads out of the city, although there were no restraints. One hardly noticed this—it was the norm. But like many an easy assumption, once broken it became increasingly obvious. Cormen's acquaintances were tolerant of his aberration, but generally unsympathetic. What he needed was proof, something he could demonstrate in black and white—and color if required. But the city was reluctant, so it appeared, to give up its realities so easily. The Master Entertainment Center, MEC, would not answer direct queries about this, even though it would obediently show him presentations, pictorial or symbolic as he required, of the areas in question. But it was tiresome detail work, in which he had to proceed completely on his own." |
Lily Collins in City of Bones (2013)—
American Folk Art (see August 23, 2011) —

See as well Ballet Blanc .
Tuesday, January 14, 2014
Release Date
The premiere of the Lily Collins film Abduction
(see previous post) was reportedly in Sydney, Australia,
on August 23, 2011.
From that date in this journal—

For the eight-limbed star at the top of the quaternion array above, She drew from her handbag a pale grey gleaming implement that looked by quick turns to me like a knife, a gun, a slim sceptre, and a delicate branding iron—especially when its tip sprouted an eight-limbed star of silver wire. “The test?” I faltered, staring at the thing. “Yes, to determine whether you can live in the fourth dimension or only die in it.” — Fritz Leiber, short story, 1959 |
Related material from Wikipedia, suggested by the reference quoted
in this morning's post to "a four-dimensionalist (perdurantist) ontology"—
"… perdurantism also applies if one believes there are temporal
but non-spatial abstract entities (like immaterial souls…)."
Saturday, January 11, 2014
Star Wars (continued)

A star figure and the Galois quaternion.
The square root of the former is the latter.
"… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte."
Monday, January 6, 2014
Triumph of the Will
"… the human will cannot be simultaneously
triumphant and imaginary."
— Ross Douthat, Defender of the Faith,
in this afternoon's New York Times at 3:25* PM ET
Some— even some Catholics— might say the will
cannot be triumphant unless imaginary.
Related material: The Galois Quaternion: A Story.
See also C. S. Lewis on enchantment.
* Cf., in this journal, the most recent 3/25 ,
and a bareword —
Click image for some context.
Thursday, October 3, 2013
Loosey in the Sky
"Righty tighty, lefty loosey." — Folk saying
See also a figure from this journal
on Lee Marvin's birthday in 2011 —
The square root of the former is the latter.
Tuesday, May 14, 2013
Snakes on a Plane

Detail from the video in the previous post:

For other permutations of points in the
order-3 affine plane—
See Quaternions in an Affine Galois Plane
and Group Actions, 1984-2009.
See, too, the Mathematics and Narrative post
from April 28, 2013, and last night's
For Indiana Spielberg.
Sunday, February 10, 2013
The Cleaning
"In 2005 Arthur Jaffe succeeded Sir Michael Atiyah as
Chair of the Board of the Dublin Institute for Advanced Study,
School of Theoretical Physics."
Related material:
Biddies in this journal and…
Detail:

An early version of quaternions.
Friday, December 28, 2012
Cube Koan
|
From Don DeLillo's novel Point Omega — I knew what he was, or what he was supposed to be, a defense intellectual, without the usual credentials, and when I used the term it made him tense his jaw with a proud longing for the early weeks and months, before he began to understand that he was occupying an empty seat. "There were times when no map existed to match the reality we were trying to create." "What reality?" "This is something we do with every eyeblink. Human perception is a saga of created reality. But we were devising entities beyond the agreed-upon limits of recognition or interpretation. Lying is necessary. The state has to lie. There is no lie in war or in preparation for war that can't be defended. We went beyond this. We tried to create new realities overnight, careful sets of words that resemble advertising slogans in memorability and repeatability. These were words that would yield pictures eventually and then become three-dimensional. The reality stands, it walks, it squats. Except when it doesn't." He didn't smoke but his voice had a sandlike texture, maybe just raspy with age, sometimes slipping inward, becoming nearly inaudible. We sat for some time. He was slouched in the middle of the sofa, looking off toward some point in a high corner of the room. He had scotch and water in a coffee mug secured to his midsection. Finally he said, "Haiku." I nodded thoughtfully, idiotically, a slow series of gestures meant to indicate that I understood completely. "Haiku means nothing beyond what it is. A pond in summer, a leaf in the wind. It's human consciousness located in nature. It's the answer to everything in a set number of lines, a prescribed syllable count. I wanted a haiku war," he said. "I wanted a war in three lines. This was not a matter of force levels or logistics. What I wanted was a set of ideas linked to transient things. This is the soul of haiku. Bare everything to plain sight. See what's there. Things in war are transient. See what's there and then be prepared to watch it disappear." |
What's there—

This view of a die's faces 3, 6, and 5, in counter-
clockwise order (see previous post) suggests a way
of labeling the eight corners of a die (or cube):
123, 135, 142, 154, 246, 263, 365, 456.
Here opposite faces of the die sum to 7, and the
three faces meeting at each corner are listed
in counter-clockwise order. (This corresponds
to a labeling of one of MacMahon's* 30 colored cubes.)
A similar vertex-labeling may be used in describing
the automorphisms of the order-8 quaternion group.
For a more literary approach to quaternions, see
Pynchon's novel Against the Day .
* From Peter J. Cameron's weblog:
"The big name associated with this is Major MacMahon,
an associate of Hardy, Littlewood and Ramanujan,
of whom Robert Kanigel said,
His expertise lay in combinatorics, a sort of
glorified dice-throwing, and in it he had made
contributions original enough to be named
a Fellow of the Royal Society.
Glorified dice-throwing, indeed…"
Wednesday, November 14, 2012
Group Actions
The December 2012 Notices of the American
Mathematical Society has an ad on page 1564
(in a review of two books on vulgarized mathematics)
for three workshops next year on “Low-dimensional
Topology, Geometry, and Dynamics”—

(Only the top part of the ad is shown; for further details
see an ICERM page.)
(ICERM stands for Institute for Computational
and Experimental Research in Mathematics.)
The ICERM logo displays seven subcubes of
a 2x2x2 eight-cube array with one cube missing—

The logo, apparently a stylized image of the architecture
of the Providence building housing ICERM, is not unlike
a picture of Froebel’s Third Gift—

© 2005 The Institute for Figuring
Photo by Norman Brosterman from the Inventing Kindergarten
exhibit at The Institute for Figuring (co-founded by Margaret Wertheim)
The eighth cube, missing in the ICERM logo and detached in the
Froebel Cubes photo, may be regarded as representing the origin
(0,0,0) in a coordinatized version of the 2x2x2 array—
in other words the cube invariant under linear , as opposed to
more general affine , permutations of the cubes in the array.
These cubes are not without relevance to the workshops’ topics—
low-dimensional exotic geometric structures, group theory, and dynamics.
See The Eightfold Cube, A Simple Reflection Group of Order 168, and
The Quaternion Group Acting on an Eightfold Cube.
Those who insist on vulgarizing their mathematics may regard linear
and affine group actions on the eight cubes as the dance of
Snow White (representing (0,0,0)) and the Seven Dwarfs—
 .
.
Sunday, June 17, 2012
Congruent Group Actions
A Google search today yielded no results
for the phrase "congruent group actions."
Places where this phrase might prove useful include—
- Actions of the quaternion group in finite geometry
- Affine group actions in finite geometry
- The "symmetric generation" technique of R. T. Curtis
Saturday, June 16, 2012
Chiral Problem
In memory of William S. Knowles, chiral chemist, who died last Wednesday (June 13, 2012)—
Detail from the Harvard Divinity School 1910 bookplate in yesterday morning's post—
"ANDOVER–HARVARD THEOLOGICAL LIBRARY"
Detail from Knowles's obituary in this morning's New York Times—
William Standish Knowles was born in Taunton, Mass., on June 1, 1917. He graduated a year early from the Berkshire School, a boarding school in western Massachusetts, and was admitted to Harvard. But after being strongly advised that he was not socially mature enough for college, he did a second senior year of high school at another boarding school, Phillips Academy in Andover, N.H.
Dr. Knowles graduated from Harvard with a bachelor’s degree in chemistry in 1939….
"This is the relativity problem: to fix objectively a class of equivalent coordinatizations and to ascertain the group of transformations S mediating between them."
— Hermann Weyl, The Classical Groups, Princeton University Press, 1946, p. 16
From Pilate Goes to Kindergarten—

The six congruent quaternion actions illustrated above are based on the following coordinatization of the eightfold cube—

Problem: Is there a different coordinatization
that yields greater symmetry in the pictures of
quaternion group actions?
A paper written in a somewhat similar spirit—
"Chiral Tetrahedrons as Unitary Quaternions"—
ABSTRACT: Chiral tetrahedral molecules can be dealt [with] under the standard of quaternionic algebra. Specifically, non-commutativity of quaternions is a feature directly related to the chirality of molecules….
Saturday, May 19, 2012
G8
"The group of 8" is a phrase from politics, not mathematics.
Of the five groups of order 8 (see today's noon post),
the one pictured* in the center, Z2 × Z2 × Z2 , is of particular
interest. See The Eightfold Cube. For a connection of this
group of 8 to the last of the five pictured at noon, the
quaternion group, see Finite Geometry and Physical Space.
* The picture is of the group's cycle graph.
Monday, May 7, 2012
More on Triality
John Baez wrote in 1996 ("Week 91") that
"I've never quite seen anyone come right out
and admit that triality arises from the
permutations of the unit vectors i, j, and k
in 3d Euclidean space."
Baez seems to come close to doing this with a
somewhat different i , j , and k — Hurwitz
quaternions— in his 2005 book review
quoted here yesterday.
See also the Log24 post of Jan. 4 on quaternions,
and the following figures. The actions on cubes
in the lower figure may be viewed as illustrating
(rather indirectly) the relationship of the quaternion
group's 24 automorphisms to the 24 rotational
symmetries of the cube.

Sunday, May 6, 2012
Triality continued
This post continues the April 9 post
commemorating Élie Cartan's birthday.
That post mentioned triality .
Here is John Baez reviewing
On Quaternions and Octonions:
Their Geometry, Arithmetic, and Symmetry
by John H. Conway and Derek A. Smith
(A.K. Peters, Ltd., 2003)—

"In this context, triality manifests itself
as the symmetry that cyclically permutes
the Hurwitz integers i , j , and k ."
Related material— Quaternion Acts in this journal
as well as Finite Geometry and Physical Space.
Wednesday, April 18, 2012
Adam in Eden
…. and John Golding, an authority on Cubism who "courted abstraction"—
"Adam in Eden was the father of Descartes." — Wallace Stevens
Fictional symbologist Robert Langdon and a cube—
From a Log24 post, "Eightfold Cube Revisited,"
on the date of Golding's death—

A related quotation—
"… quaternions provide a useful paradigm
for studying the phenomenon of 'triality.'"
— David A. Richter's webpage Zometool Triality
See also quaternions in another Log24 post
from the date of Golding's death— Easter Act.
Monday, April 9, 2012
Easter Act
"And when he had apprehended him,
he put him in prison, and delivered him
to four quaternions of soldiers to keep him;
intending after Easter to bring him forth to the people."
Monday, February 6, 2012
Savage Logic
|
Saturday, October 16, 2010 m759 @ 12:00 PM Brightness at Noon continued – Sir William Rowan Hamilton, See also this journal on 1/09, 2010. |
This post was suggested by the date
of a user comment in Wikipedia.
Sunday, January 22, 2012
Souvenir*
From life's box of chocolates…
Happy birthday to Piper Laurie.
* Those who prefer their
souvenirs without sentiment
may consult the quaternions.
Wednesday, January 4, 2012
Revision
I revised the cubes image and added a new link to
an explanatory image in posts of Dec. 30 and Jan. 3
(and at finitegeometry.org). (The cubes now have
quaternion "i , j , k " labels and the cubes now
labeled "k " and "-k " were switched.)
Tuesday, January 3, 2012
Theorum
In memory of artist Ronald Searle—
Searle reportedly died at 91 on December 30th.
From Log24 on that date—
Click the above image for some context.
Update of 9:29 PM EST Jan. 3, 2012—
|
Theorum
Theorum (rhymes with decorum, apparently) is a neologism proposed by Richard Dawkins in The Greatest Show on Earth to distinguish the scientific meaning of theory from the colloquial meaning. In most of the opening introduction to the show, he substitutes "theorum" for "theory" when referring to the major scientific theories such as evolution. Problems with "theory" Dawkins notes two general meanings for theory; the scientific one and the general sense that means a wild conjecture made up by someone as an explanation. The point of Dawkins inventing a new word is to get around the fact that the lay audience may not thoroughly understand what scientists mean when they say "theory of evolution". As many people see the phrase "I have a theory" as practically synonymous with "I have a wild guess I pulled out of my backside", there is often confusion about how thoroughly understood certain scientific ideas are. Hence the well known creationist argument that evolution is "just a theory" – and the often cited response of "but gravity is also just a theory". To convey the special sense of thoroughness implied by the word theory in science, Dawkins borrowed the mathematical word "theorem". This is used to describe a well understood mathematical concept, for instance Pythagoras' Theorem regarding right angled triangles. However, Dawkins also wanted to avoid the absolute meaning of proof associated with that word, as used and understood by mathematicians. So he came up with something that looks like a spelling error. This would remove any person's emotional attachment or preconceptions of what the word "theory" means if it cropped up in the text of The Greatest Show on Earth , and so people would (in "theory ") have no other choice but to associate it with only the definition Dawkins gives. This phrase has completely failed to catch on, that is, if Dawkins intended it to catch on rather than just be a device for use in The Greatest Show on Earth . When googled, Google will automatically correct the spelling to theorem instead, depriving this very page its rightful spot at the top of the results.
|
Some backgound— In this journal, "Diamond Theory of Truth."
Friday, October 7, 2011
Enigma Variations
For Yom Kippur

4. Image— Argument for the Existence of Rebecca
Some literary and cinematic background—

"Are you the butterfly… ?"
Friday, September 2, 2011
Rigged?
Sarah Tomlin in a Nature article on the July 12-15 2005 Mykonos meeting on Mathematics and Narrative—
"Today, Mazur says he has woken up to the power of narrative, and in Mykonos gave an example of a 20-year unsolved puzzle in number theory which he described as
Michel Chaouli in "How Interactive Can Fiction Be?" (Critical Inquiry 31, Spring 2005), pages 613-614—
"…a simple thought experiment….*
… If the cliffhanger is done well, it will not simply introduce a wholly unprepared turn into the narrative (a random death, a new character, an entirely unanticipated obstacle) but rather tighten the configuration of known elements to such a degree that the next step appears both inevitable and impossible. We feel the pressure rising to a breaking point, but we simply cannot foresee where the complex narrative structure will give way. This interplay of necessity and contingency produces our anxious— and highly pleasurable— speculation about the future path of the story. But if we could determine that path even slightly, we would narrow the range of possible outcomes and thus the uncertainty in the play of necessity and contingency. The world of the fiction would feel, not open, but rigged."
* The idea of the thought experiment emerged in a conversation with Barry Mazur.
Barry Mazur in the preface to his 2003 book Imagining Numbers—
"But the telltale adjective real suggests two things: that these numbers are somehow real to us and that, in contrast, there are unreal numbers in the offing. These are the imaginary numbers .
The imaginary numbers are well named, for there is some imaginative work to do to make them as much a part of us as the real numbers we use all the time to measure for bookshelves.
This book began as a letter to my friend Michel Chaouli. The two of us had been musing about whether or not one could 'feel' the workings of the imagination in its various labors. Michel had also mentioned that he wanted to 'imagine imaginary numbers.' That very (rainy) evening, I tried to work up an explanation of the idea of these numbers, still in the mood of our conversation."
See also The Galois Quaternion and 2/19.

New York Lottery last evening
Tuesday, August 23, 2011
Four Winds

Related remarks —
- Sunday's Log24 post Cuatro Vientos on Jack Kerouac
- The phrase dies natalis in Log24
-
Another weblog in 2004 on the dies natalis of Jack Kerouac.
That weblog gives the dies as Oct. 20, but other sources
say Kerouac died at 5:15 AM on Oct. 21. - A Log24 post on Oct. 20 last year related to the Oct. 21 date.
For the eight-limbed star at the top of the quaternion array above,
see "Damnation Morning" in this journal—
She drew from her handbag a pale grey gleaming implement that looked by quick turns to me like a knife, a gun, a slim sceptre, and a delicate branding iron—especially when its tip sprouted an eight-limbed star of silver wire. “The test?” I faltered, staring at the thing. “Yes, to determine whether you can live in the fourth dimension or only die in it.” — Fritz Leiber, short story, 1959
See also Feb. 19, 2011.
Sunday, June 26, 2011
Gloomy Sunday
This evening's NY Lottery numbers were 531 and 8372.
Hermeneutics—
From a Google search for "531 Log24"—
| Log24 on Sunday, Sept. 21, 2008
531 , Revelation without belief 116. Evening (Belief), Belief without… The date, 5/24, of the entries linked to in Thursday's noon Log24 entry… Pynchon on Quaternions – Log24 8. on Page 531 : "… to imps of ingenious discomfort. "Is this a stag affair, or are there likely to be one or two lady Quaternion- ists?…" |
The "531" linked to in the Sept. 21, 2008, post above is a mini-drama ending at midnight on 5/31, 2008— the conclusion of Mental Health Month.
And the above 4-digit evening number suggests a search for births on 8/3/72 that yields—
Erika Marozsán, Hungarian actress, 38.
Marozsán starred in "Gloomy Sunday—A Song of Love and Death" (A German/Hungarian film from 1999).
Wikipedia informs us that this "is inaccurately claimed to be the world's longest running film."
Whether Marozsán is a Quaternionist, I do not know.
For love, death, and quaternions, see the post Metaphor from Feb. 22 linked to in this afternoon's Sunday Dinner.
Sunday, May 1, 2011
Delos
The late translator Helen Lane in Translation Review , Vol. 5, 1980—
"Among the awards, I submit, should be one for the entire oeuvre of a lifetime "senior" translator— and one for the best first translation…. Similar organization, cooperation, and fund-finding for a first-rate replacement for the sorely missed Delos ."
This leads to one of the founders of Delos , the late Donald Carne-Ross, who died on January 9, 2010.
For one meditation on the date January 9, see Bridal Birthday (last Thursday).
Another meditation, from the date of Carne-Ross's death—
Saturday, January 9, 2010
Positional Meaning"The positional meaning of a symbol derives from its relationship to other symbols in a totality, a Gestalt, whose elements acquire their significance from the system as a whole." – Victor Turner, The Forest of Symbols , Ithaca, NY, Cornell University Press, 1967, p. 51, quoted by Beth Barrie in "Victor Turner." To everything, turn, turn, turn … |
See also Delos in this journal.
Friday, March 18, 2011
Defining Configurations*
The On-Line Encyclopedia of Integer Sequences has an article titled "Number of combinatorial configurations of type (n_3)," by N.J.A. Sloane and D. Glynn.
From that article:
- DEFINITION: A combinatorial configuration of type (n_3) consists of an (abstract) set of n points together with a set of n triples of points, called lines, such that each point belongs to 3 lines and each line contains 3 points.
- EXAMPLE: The unique (8_3) configuration consists of the triples 125, 148, 167, 236, 278, 347, 358, 456.
The following corrects the word "unique" in the example.

* This post corrects an earlier post, also numbered 14660 and dated 7 PM March 18, 2011, that was in error.
The correction was made at about 11:50 AM on March 20, 2011.
_____________________________________________________________
Update of March 21
The problem here is of course with the definition. Sloane and Glynn failed to include in their definition a condition that is common in other definitions of configurations, even abstract or purely "combinatorial" configurations. See, for instance, Configurations of Points and Lines , by Branko Grunbaum (American Mathematical Society, 2009), p. 17—
In the most general sense we shall consider combinatorial (or abstract) configurations; we shall use the term set-configurations as well. In this setting "points" are interpreted as any symbols (usually letters or integers), and "lines" are families of such symbols; "incidence" means that a "point" is an element of a "line". It follows that combinatorial configurations are special kinds of general incidence structures. Occasionally, in order to simplify and clarify the language, for "points" we shall use the term marks, and for "lines" we shall use blocks. The main property of geometric configurations that is preserved in the generalization to set-configurations (and that characterizes such configurations) is that two marks are incident with at most one block, and two blocks with at most one mark.
Whether or not omitting this "at most one" condition from the definition is aesthetically the best choice, it dramatically changes the number of configurations in the resulting theory, as the above (8_3) examples show.
Update of March 22 (itself updated on March 25)
For further background on configurations, see Dolgachev—

Note that the two examples Dolgachev mentions here, with 16 points and 9 points, are not unrelated to the geometry of 4×4 and 3×3 square arrays. For the Kummer and related 16-point configurations, see section 10.3, "The Three Biplanes of Order 4," in Burkard Polster's A Geometrical Picture Book (Springer, 1998). See also the 4×4 array described by Gordon Royle in an undated web page and in 1980 by Assmus and Sardi. For the Hesse configuration, see (for instance) the passage from Coxeter quoted in Quaternions in an Affine Galois Plane.
Update of March 27
See the above link to the (16,6) 4×4 array and the (16,6) exercises using this array in R.D. Carmichael's classic Introduction to the Theory of Groups of Finite Order (1937), pp. 42-43. For a connection of this sort of 4×4 geometry to the geometry of the diamond theorem, read "The 2-subsets of a 6-set are the points of a PG(3,2)" (a note from 1986) in light of R.W.H.T. Hudson's 1905 classic Kummer's Quartic Surface , pages 8-9, 16-17, 44-45, 76-77, 78-79, and 80.
Saturday, February 19, 2011
Brightness at Noon (continued)
From Epiphany Revisited —

A star figure and the Galois quaternion.
The square root of the former is the latter.
… Todo lo sé por el lucero puro
que brilla en la diadema de la Muerte.
Thursday, January 6, 2011
Epiphany Riddle
"Spaces and geometries, those which we perceive,
which we can’t perceive, or which only some of us perceive,
are a recurring theme in Against the Day ."
"大哉大哉 宇宙之谜
美哉美哉 真理之源"
"Great indeed is the riddle of the universe.
Beautiful indeed is the source of truth."
— Shing-Tung Yau, Chairman,
Department of Mathematics, Harvard University
"Always keep a diamond in your mind."
— King Solomon at the Paradiso
Image from stoneship.org
Wednesday, November 10, 2010
Scavenger Hunt
A description in Pynchon's Against the Day of William Rowan Hamilton's October 16th, 1843, discovery of quaterions—
"The moment, of course, is timeless. No beginning, no end, no duration, the light in eternal descent, not the result of conscious thought but fallen onto Hamilton, if not from some Divine source then at least when the watchdogs of Victorian pessimism were sleeping too soundly to sense, much less frighten off, the watchful scavengers of Epiphany."
New York Lottery yesterday, on Hermann Weyl's birthday— Midday 106, Evening 865.
Here 106 suggests 1/06, the date of Epiphany, and 865 turns out to be the title number of Weyl's Symmetry at Princeton University Press—
http://press.princeton.edu/titles/865.html.
Symmetry and quaternions are, of course, closely related.
Friday, November 5, 2010
Seize the Day*
From last night's post—
"… right now…. winning the day…."
— President Obama on the 16th of October (between 5:19 and 5:37 PM EDT)
This journal on the 16th of October—
Saturday, October 16, 2010Quaternion DayBrightness at Noon continued – Sir William Rowan Hamilton, Oct. 16, 1843 See also this journal on 1/09, 2010. |
Related religious material from Thomas Pynchon—

* Material related to this post's title, "Seize the Day"—
Indirectly related — an ad for the new film Black Swan
accompanying a Halloween story in yesterday's online New York Times —
More directly related —
Black Swan Theory at Wikipedia —
Monday, October 18, 2010
For St. Luke’s Day —
The Turning
"To everything, turn, turn, turn…
… there is a season, turn, turn, turn…"
For less turning and more seasons, see a search in this journal for
fullness + multitude + "cold mountain."
Tuesday, October 12, 2010
King Solomon’s Mind
"Always keep a diamond in your mind."
— Tom Waits/Kathleen Brennan song performed by Solomon Burke at the Paradiso in Amsterdam
|
"The text is a two-way mirror — The French Mathematician |
 |
Saturday, August 7, 2010
The Matrix Reloaded
For aficionados of mathematics and narrative —
Illustration from
"The Galois Quaternion— A Story"
This resembles an attempt by Coxeter in 1950 to represent
a Galois geometry in the Euclidean plane—
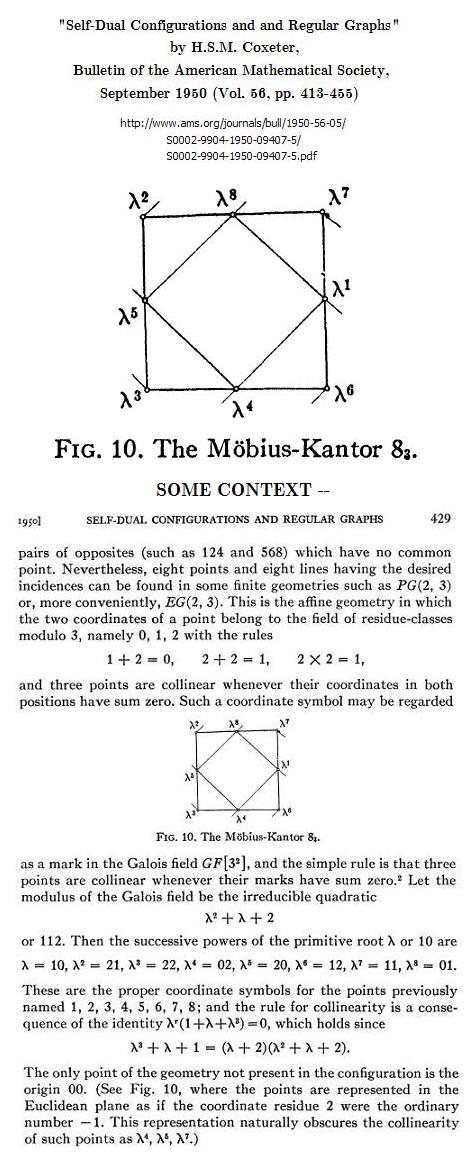
The quaternion illustration above shows a more natural way to picture this geometry—
not with dots representing points in the Euclidean plane, but rather with unit squares
representing points in a finite Galois affine plane. The use of unit squares to
represent points in Galois space allows, in at least some cases, the actions
of finite groups to be represented more naturally than in Euclidean space.
See Galois Geometry, Geometry Simplified, and
Finite Geometry of the Square and Cube.
Saturday, March 13, 2010
Space Cowboy
From yesterday's Seattle Times—
According to police, employees of a Second Avenue mission said the suspect, clad in black and covered in duct tape, had come into the mission "and threatened to blow the place up." He then told staffers "that he was a vampire and wanted to eat people."
The man… also called himself "a space cowboy"….
This suggests two film titles…

and Apollo's 13—
The 13 symmetry axes of the (Euclidean) cube–
exactly one axis for each pair of opposite
subcubes in the (Galois) 3×3×3 cube–
Saturday, February 13, 2010
Entertainment continued
“Logic is all about the entertaining of possibilities.”
– Colin McGinn, Mindsight: Image, Dream, Meaning,
Harvard University Press, 2004
Geometry of Language,
continued from St. George's Day, 2009—

Related material:
Prima Materia,
The Galois Quaternion,
and The Wake of Imagination.
See also the following from a physicist
(not of the most orthodox sort, but his remarks
here on Heisenberg seem quite respectable)–
Saturday, February 6, 2010
Conceptual Art, continued–
Argument for the Existence of Rebecca
Adapted from YouTube's "Mathematics and Religion," starring Rebecca Newberger Goldstein, author of the recent novel 36 Arguments for the Existence of God—

The added Quaternion picture is from
Groundhog Day, 2009.
Wednesday, February 3, 2010
Attitude Adjustment
"A generation lost in space"
— American Pie
Click image for details.
See also the concepts of inner-direction
and other-direction in The Lonely Crowd
by David Riesman et al. Riesman was,
according to Harvard Square Library,
a contract termination lawyer for
Sperry Gyroscope before turning
to sociology.
EXERCISE — Discuss inner- and
other-direction in education and
in journalism, using the material
in Monday's entry on the
New York Times dunce cap —

— contrasted with the webpage
excerpted below —
Monday, February 1, 2010
For St. Bridget’s Day
"But wait, there's more!"
– Stanley Fish, NY Times Jan. 28
From the editors at The New York Times who, left to their own devices, would produce yet another generation of leftist morons who don't know the difference between education and entertainment–
A new Times column starts today–

The quality of the column's logo speaks for itself. It pictures a cone with dashed lines indicating height and base radius, but unlabeled except for a large italic x to the right of the cone. This enigmatic variable may indicate the cone's height or slant height– or, possibly, its surface area or volume.
Instead of the column's opening load of crap about numbers and Sesame Street, a discussion of its logo might be helpful.
The cone plays a major role in the historical development of mathematics.
Some background from an online edition of Euclid—
"Euclid proved in proposition XII.10 that the cone with the same base and height as a cylinder was one third of the cylinder, but he could not find the ratio of a sphere to the circumscribed cylinder. In the century after Euclid, Archimedes solved this problem as well as the much more difficult problem of the surface area of a sphere."
For Archimedes and the surface area of a sphere, see (for instance) a discussion by Kevin Brown. For more material on Archimedes, see "Archimedes: Volume of a Sphere," by Doug Faires (2001)– Archimedes' heuristic argument from mechanics that involves the volume of a cone– and Archimedes' more rigorous approach in The Works of Archimedes, edited by T. L. Heath (1897).
The work of Euclid and Archimedes on volumes was, of course, long before the discovery of calculus. For a helpful discussion of cone volumes involving high-school-level calculus, see, for instance, the following–

The Times editors apparently feel that
few of their readers are capable of
such high-school-level sophistication.
For some other geometric illustrations
perhaps more appealing than the Times's

dunce cap, see the symbol of
today's saint– a Bridget Cross—
and a web page on
visualized quaternions.
Saturday, January 9, 2010
Positional Meaning
"The positional meaning of a symbol derives from its relationship to other symbols in a totality, a Gestalt, whose elements acquire their significance from the system as a whole."
— Victor Turner, The Forest of Symbols, Ithaca, NY, Cornell University Press, 1967, p. 51, quoted by Beth Barrie in "Victor Turner."
To everything, turn, turn, turn…
— Peter Seeger
Wednesday, January 6, 2010
Brightness at Noon, continued
The Galois Quaternion
|
I had foreseen it all in precise detail. i = an imaginary being Here, on this complex space, |
Related material:
The Galois Quaternion
Click for context.
(See also Nativity and the end
of this morning's post.)
Epiphany Revisited
|
January 06, 2007 Picture of Nothing
“Varnedoe’s lectures were ultimately about faith, about his faith in the power of abstraction, and abstraction as a kind of anti-religious faith in itself….” Related material: The more industrious scholars will derive considerable pleasure from describing how the art-history professors and journalists of the period 1945-75, along with so many students, intellectuals, and art tourists of every sort, actually struggled to see the paintings directly, in the old pre-World War II way, like Plato’s cave dwellers watching the shadows, without knowing what had projected them, which was the Word.” — Tom Wolfe, The Painted Word “Concept (scholastics’ verbum mentis)– theological analogy of Son’s procession as Verbum Patris, 111-12″ — Index to Joyce and Aquinas, by William T. Noon, S.J., Yale University Press 1957, second printing 1963, page 162
“So did God cause the big bang? Overcome by metaphysical lassitude, I finally reach over to my bookshelf for The Devil’s Bible. Turning to Genesis I read: ‘In the beginning there was nothing. And God said, ‘Let there be light!’ And there was still nothing, but now you could see it.'”
— Jim Holt, Big-Bang Theology, from Slate‘s “High Concept” department  “Bang.” “…Mondrian and Malevich are not discussing canvas or pigment or graphite or any other form of matter. They are talking about Being or Mind or Spirit. From their point of view, the grid is a staircase to the Universal….” For properties of the “nothing” represented by the 3×3 grid, see The Field of Reason. For religious material related to the above and to Epiphany, a holy day observed by some, see Plato, Pegasus, and the Evening Star and Shining Forth.
|
Some Context:
See also Nativity.
Tuesday, October 13, 2009
Tuesday October 13, 2009
This morning’s New York Times reports the deaths of Nuremberg interrogator Richard W. Sonnenfeldt and of avant-garde novelist and Beckett scholar Raymond Federman.
Symbols from this journal on the dates of their deaths:

For connotations of the symbol appropriate to the name Sonnenfeldt, see the link to A Sunrise for Sunrise in the entry of Saturday, Oct. 10.

— James Joyce, Finnegans Wake
Friday, October 9, 2009
Friday October 9, 2009
"…strict grids of nine pictures
establish an egalitarian
framework…."
Some are more
egalitarian
than others.
Thursday, September 3, 2009
Thursday September 3, 2009
“Music and mathematics are among the pre-eminent wonders of the race. Levi-Strauss sees in the invention of melody ‘a key to the supreme mystery’ of man– a clue, could we but follow it, to the singular structure and genius of the species. The power of mathematics to devise actions for reasons as subtle, witty, manifold as any offered by sensory experience and to move forward in an endless unfolding of self-creating life is one of the strange, deep marks man leaves on the world. Chess, on the other hand, is a game in which thirty-two bits of ivory, horn, wood, metal, or (in stalags) sawdust stuck together with shoe polish, are pushed around on sixty-four alternately coloured squares. To the addict, such a description is blasphemy. The origins of chess are shrouded in mists of controversy, but unquestionably this very ancient, trivial pastime has seemed to many exceptionally intelligent human beings of many races and centuries to constitute a reality, a focus for the emotions, as substantial as, often more substantial than, reality itself. Cards can come to mean the same absolute. But their magnetism is impure. A mania for whist or poker hooks into the obvious, universal magic of money. The financial element in chess, where it exists at all, has always been small or accidental.
To a true chess player, the pushing about of thirty-two counters on 8×8 squares is an end in itself, a whole world next to which that of a mere biological or political or social life seems messy, stale, and contingent. Even the patzer, the wretched amateur who charges out with his knight pawn when the opponent’s bishop decamps to R4, feels this daemonic spell. There are siren moments when quite normal creatures otherwise engaged, men such as Lenin and myself, feel like giving up everything– marriage, mortgages, careers, the Russian Revolution– in order to spend their days and nights moving little carved objects up and down a quadrate board. At the sight of a set, even the tawdriest of plastic pocket sets, one’s fingers arch and a coldness as in a light sleep steals over one’s spine. Not for gain, not for knowledge or reknown, but in some autistic enchantment, pure as one of Bach’s inverted canons or Euler’s formula for polyhedra.”
— George Steiner in “A Death of Kings,” The New Yorker, issue dated September 7, 1968, page 133
“Examples are the stained-glass windows of knowledge.” —Nabokov

Click above images for some context.
Log24 entries of May 30, 2006, as well as “For John Cramer’s daughter Kathryn”– August 27, 2009— and related material at Wikipedia (where Kathryn is known as “Pleasantville”).
Wednesday, April 8, 2009
Wednesday April 8, 2009
Is God
“For every kind of vampire,
there is a kind of cross.”
— Thomas Pynchon in
Gravity’s Rainbow
“Since 1963, when Pynchon’s first novel, V., came out, the writer– widely considered America’s most important novelist since World War II– has become an almost mythical figure,
— Nancy Jo Sales in the November 11, 1996, issue of New York Magazine
(Click on images for their
source in past entries.)
In a Nutshell:
“Plato’s Ghost evokes Yeats’s lament that any claim to worldly perfection inevitably is proven wrong by the philosopher’s ghost….”
— Princeton University Press on Plato’s Ghost: The Modernist Transformation of Mathematics (by Jeremy Gray, September 2008)
|
Monday, February 2, 2009
Monday February 2, 2009
Against the Day
is a novel by Thomas Pynchon
published on Nov. 21, 2006, in
hardcover, and in paperback on
Oct. 30, 2007 (Devil's Night).
Perhaps the day the title
refers to is one of the above
dates… or perhaps it is–

|
The Candlebrow Conference in Pynchon's Against the Day: The conferees had gathered here from all around the world…. Their spirits all one way or another invested in, invested by, the siegecraft of Time and its mysteries. "Fact is, our system of so-called linear time is based on a circular or, if you like, periodic phenomenon– the earth's own spin. Everything spins, up to and including, probably, the whole universe. So we can look to the prairie, the darkening sky, the birthing of a funnel-cloud to see in its vortex the fundamental structure of everything–" "Um, Professor–"…. … Those in attendance, some at quite high speed, had begun to disperse, the briefest of glances at the sky sufficing to explain why. As if the professor had lectured it into being, there now swung from the swollen and light-pulsing clouds to the west a classic prairie "twister"…. … In the storm cellar, over semiliquid coffee and farmhouse crullers left from the last twister, they got back to the topic of periodic functions…. "Eternal Return, just to begin with. If we may construct such functions in the abstract, then so must it be possible to construct more secular, more physical expressions." "Build a time machine." "Not the way I would have put it, but if you like, fine." Vectorists and Quaternionists in attendance reminded everybody of the function they had recently worked up…. "We thus enter the whirlwind. It becomes the very essence of a refashioned life, providing the axes to which everything will be referred. Time no long 'passes,' with a linear velocity, but 'returns,' with an angular one…. We are returned to ourselves eternally, or, if you like, timelessly." "Born again!" exclaimed a Christer in the gathering, as if suddenly enlightened. Above, the devastation had begun. |
Sunday, February 1, 2009
Sunday February 1, 2009
"For every kind of vampire,
there is a kind of cross."
— Gravity's Rainbow
Sunday, March 9, 2008
Saturday, March 8, 2008
Saturday March 8, 2008
Whirligigs

An answer:
“The whirligig of time”
— Shakespeare, Twelfth Night
and
Hamilton’s Whirligigs
Related material:
Rotation in the complex plane.
The plane was discovered
in the late 1700’s by Wessel:
|
Caspar Wessel
by J.J. O’Connor “Wessel’s paper [in Danish] was not noticed by the mathematical community until 1895… A French translation… was published in 1897 but an English translation of this most remarkable work was not published until 1999 (exactly 200 years after it was first published)…. We have called Wessel’s work remarkable, and indeed although the credit has gone to Argand, many historians of mathematics feel that Wessel’s contribution was [1]:-
In the [1] article the approaches by Argand and Wessel are compared and contrasted. Of course Wessel was a surveyor and his paper was motivated by his surveying and cartography work:-
However more is claimed for Wessel’s single mathematical paper than the first geometric interpretation of complex numbers. In [3] Crowe credits Wessel with being the first person to add vectors. Again this shows the depth of Wessel’s thinking but again, as the paper was unnoticed it had no influence on mathematical development despite appearing in the Memoirs of the Royal Danish Academy which by any standard was a major source of publications…. 1. … Biography in Dictionary of Scientific Biography (New York 1970-1990). 3. M.J. Crowe, A History of Vector Analysis (Notre Dame, 1967).” |
Thursday, May 31, 2007
Thursday May 31, 2007
Blitz by anonymous
New Delhi user
From Wikipedia on 31 May, 2007:
Shown below is a list of 25 alterations to Wikipedia math articles made today by user 122.163.102.246.
All of the alterations involve removal of links placed by user Cullinane (myself).
The 122.163… IP address is from an internet service provider in New Delhi, India.
The New Delhi anonymous user was apparently inspired by an earlier blitz by Wikipedia administrator Charles Matthews. (See User talk: Cullinane.)
Related material:
Ashay Dharwadker and Usenet Postings
and Talk: Four color theorem/Archive 2.
See also some recent comments from 122.163…
at Talk: Four color theorem.
May 31, 2007, alterations by
user 122.163.102.246:
- 17:17 Orthogonality (rm spam)
- 17:16 Symmetry group (rm spam)
- 17:14 Boolean algebra (rm spam)
- 17:12 Permutation (rm spam)
- 17:10 Boolean logic (rm spam)
- 17:08 Gestalt psychology (rm spam)
- 17:05 Tesseract (rm spam)
- 17:02 Square (geometry) (rm spam)
- 17:00 Fano plane (rm spam)
- 16:55 Binary Golay code (rm spam)
- 16:53 Finite group (rm spam)
- 16:52 Quaternion group (rm spam)
- 16:50 Logical connective (rm spam)
- 16:48 Mathieu group (rm spam)
- 16:45 Tutte–Coxeter graph (rm spam)
- 16:42 Steiner system (rm spam)
- 16:40 Kaleidoscope (rm spam)
- 16:38 Efforts to Create A Glass Bead Game (rm spam)
- 16:36 Block design (rm spam)
- 16:35 Walsh function (rm spam)
- 16:24 Latin square (rm spam)
- 16:21 Finite geometry (rm spam)
- 16:17 PSL(2,7) (rm spam)
- 16:14 Translation plane (rm spam)
- 16:13 Block design test (rm spam)
The deletions should please Charles Matthews and fans of Ashay Dharwadker’s work as a four-color theorem enthusiast and as editor of the Open Directory sections on combinatorics and on graph theory.
There seems little point in protesting the deletions while Wikipedia still allows any anonymous user to change their articles.
— Cullinane 23:28, 31 May 2007 (UTC)
Thursday, January 26, 2006
Thursday January 26, 2006
On Beauty
“Something beautiful fills the mind yet invites the search for something beyond itself, something larger or something of the same scale with which it needs to be brought into relation. Beauty, according to its critics, causes us to gape and suspend all thought. This complaint is manifestly true: Odysseus does stand marveling before the palm; Odysseus is similarly incapacitated in front of Nausicaa; and Odysseus will soon, in Book 7, stand ‘gazing,’ in much the same way, at the season-immune orchards of King Alcinous, the pears, apples, and figs that bud on one branch while ripening on another, so that never during the cycling year do they cease to be in flower and in fruit. But simultaneously what is beautiful prompts the mind to move chronologically back in the search for precedents and parallels, to move forward into new acts of creation, to move conceptually over, to bring things into relation, and does all this with a kind of urgency as though one’s life depended on it.”

The above symbol of Apollo suggests, in accordance with Scarry’s remarks, larger structures. Two obvious structures are the affine 4-space over GF(3), with 81 points, and the affine plane over GF(32), also with 81 points. Less obvious are some related projective structures. Joseph Malkevitch has discussed the standard method of constructing GF(32) and the affine plane over that field, with 81 points, then constructing the related Desarguesian projective plane of order 9, with

Miniquaternion geometry: An introduction to the study of projective planes, by T. G. Room and P. B. Kirkpatrick. Cambridge Tracts in Mathematics and Mathematical Physics, No. 60. Cambridge University Press, London, 1971. viii+176 pp.
For “miniquaternions” of a different sort, see my entry on Visible Mathematics for Hamilton’s birthday last year:

Thursday, January 5, 2006
Thursday January 5, 2006

For details, see Visualizing GL(2,p).
Saturday, November 12, 2005
Saturday November 12, 2005

Representation
of a quaternion
Related material:
“Oh, I wasn’t about to hole up
in a monastery. I still wanted–
What did I want?
I wanted a Roc’s egg….”
— Robert A. Heinlein
Glory Road
(Log24, St. Peter’s Day, 2004)
Thursday, August 25, 2005
Thursday August 25, 2005
Train of Thought
Part I: The 24-Cell
From S. H. Cullinane,
Visualizing GL(2,p),
March 26, 1985–
| From John Baez, “This Week’s Finds in Mathematical Physics (Week 198),” September 6, 2003: Noam Elkies writes to John Baez:
The enrapturing discoveries of our field systematically conceal, like footprints erased in the sand, the analogical train of thought that is the authentic life of mathematics – Gian-Carlo Rota |
Like footprints erased in the sand….
“Hello! Kinch here. Put me on to Edenville. Aleph, alpha: nought, nought, one.”
“A very short space of time through very short times of space….
Am I walking into eternity along Sandymount strand?”
— James Joyce, Ulysses, Proteus chapter
A very short space of time through very short times of space….
“It is demonstrated that space-time should possess a discrete structure on Planck scales.”
— Peter Szekeres, abstract of Discrete Space-Time
“A theory…. predicts that space and time are indeed made of discrete pieces.”
— Lee Smolin in Atoms of Space and Time (pdf), Scientific American, Jan. 2004
“… a fundamental discreteness of spacetime seems to be a prediction of the theory….”
— Thomas Thiemann, abstract of Introduction to Modern Canonical Quantum General Relativity
“Theories of discrete space-time structure are being studied from a variety of perspectives.”
— Quantum Gravity and the Foundations of Quantum Mechanics at Imperial College, London
The above speculations by physicists
are offered as curiosities.
I have no idea whether
any of them are correct.
Related material:
Stephen Wolfram offers a brief
History of Discrete Space.
For a discussion of space as discrete
by a non-physicist, see John Bigelow‘s
Space and Timaeus.
in a Discrete Space
physics, there are of course many
purely mathematical discrete spaces.
See Visible Mathematics, continued
(Aug. 4, 2005):

Saturday, August 6, 2005
Saturday August 6, 2005
the seventh anniversary
of his death:
A Miniature
Rosetta Stone

John Baez discussed (Sept. 6, 2003) the analogies of Weil, and he himself furnished another such Rosetta stone on a much smaller scale:
“… a 24-element group called the ‘binary tetrahedral group,’ a 24-element group called ‘SL(2,Z/3),’ and the vertices of a regular polytope in 4 dimensions called the ’24-cell.’ The most important fact is that these are all the same thing!”
For further details, see Wikipedia on the 24-cell, on special linear groups, and on Hurwitz quaternions,
The group SL(2,Z/3), also known as “SL(2,3),” is of course derived from the general linear group GL(2,3). For the relationship of this group to the quaternions, see the Log24 entry for August 4 (the birthdate of the discoverer of quaternions, Sir William Rowan Hamilton).
The 3×3 square shown above may, as my August 4 entry indicates, be used to picture the quaternions and, more generally, the 48-element group GL(2,3). It may therefore be regarded as the structure underlying the miniature Rosetta stone described by Baez.
“The typical example of a finite group is GL(n,q), the general linear group of n dimensions over the field with q elements. The student who is introduced to the subject with other examples is being completely misled.”
— J. L. Alperin, book review,
Bulletin (New Series) of the American
Mathematical Society 10 (1984), 121
Thursday, August 4, 2005
Thursday August 4, 2005
Today's mathematical birthdays:
Saunders Mac Lane, John Venn,
and Sir William Rowan Hamilton.
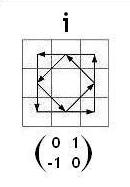
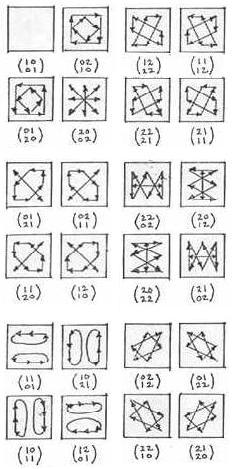
It is well known that the quaternion group is a subgroup of GL(2,3), the general linear group on the 2-space over GF(3), the 3-element Galois field.
The figures below illustrate this fact.

Related material: Visualizing GL(2,p)
— J. L. Alperin, book review,
Bulletin (New Series) of the American
Mathematical Society 10 (1984), 121